A Spectral/hp-Based Stabilized Solver with Emphasis on the Euler Equations
Abstract
:1. Introduction
2. Governing Equations
3. Spectral/ Approximation
4. Finite Element Formulation
4.1. Stabilized Finite Element
4.2. High-Order Stabilization Parameter
4.3. Temporal Evolution
5. Numerical Examples
5.1. Kovasznay Flow
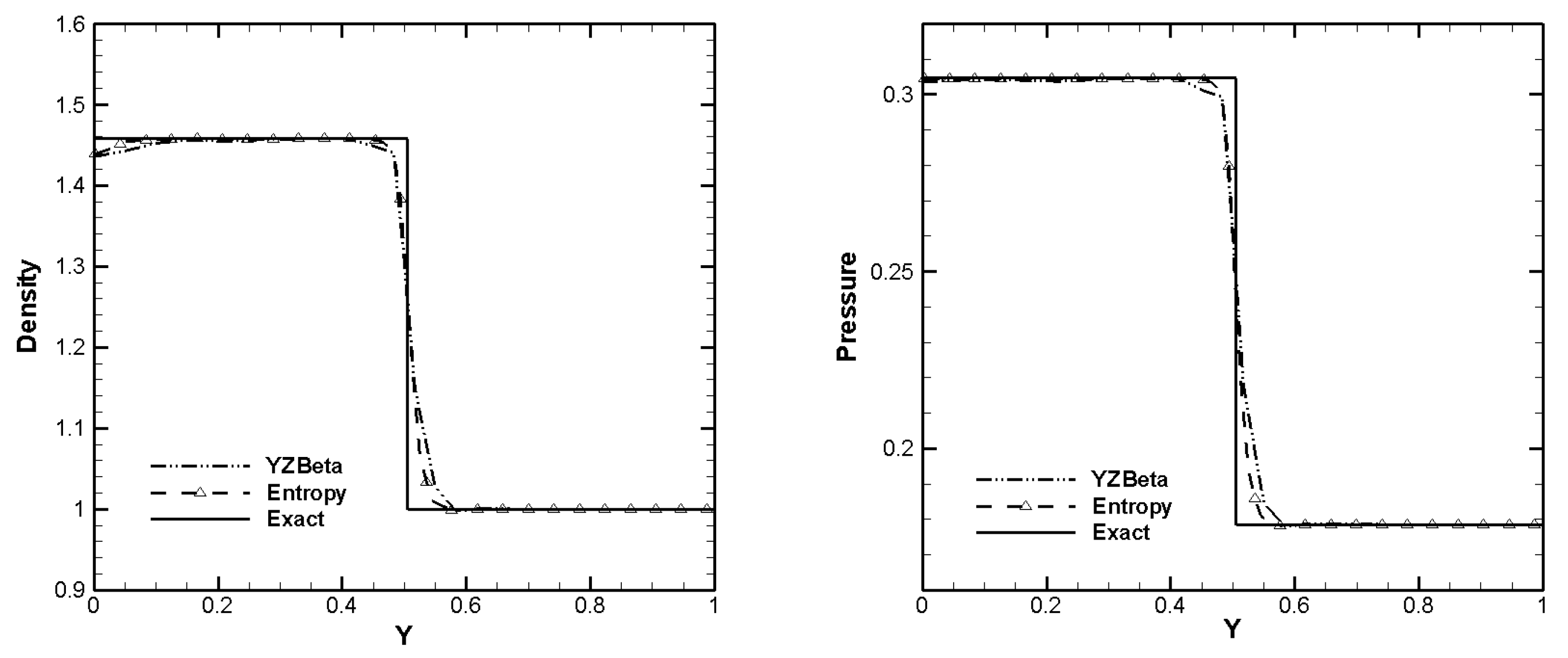
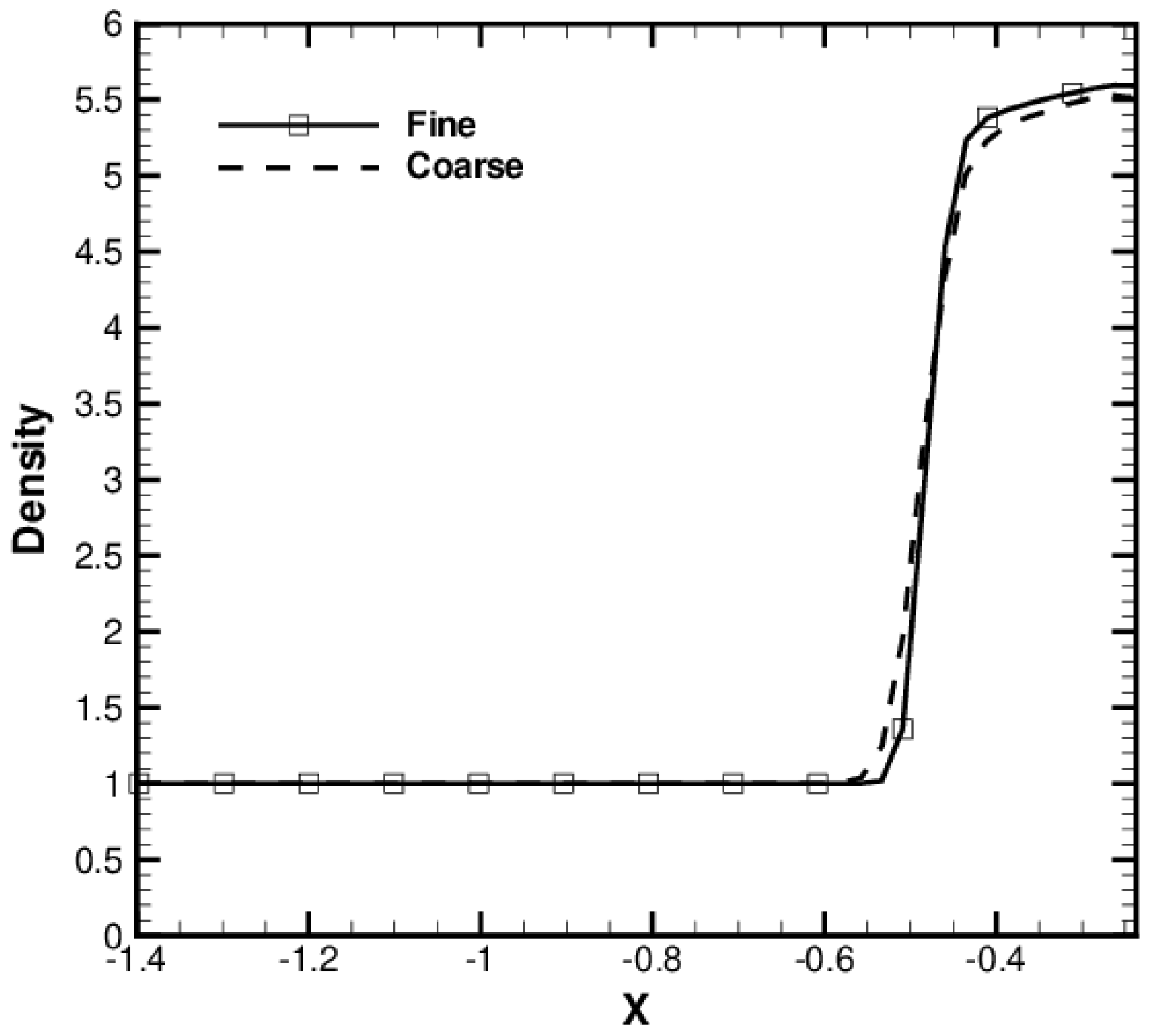
5.2. Sod’s Shock Problem
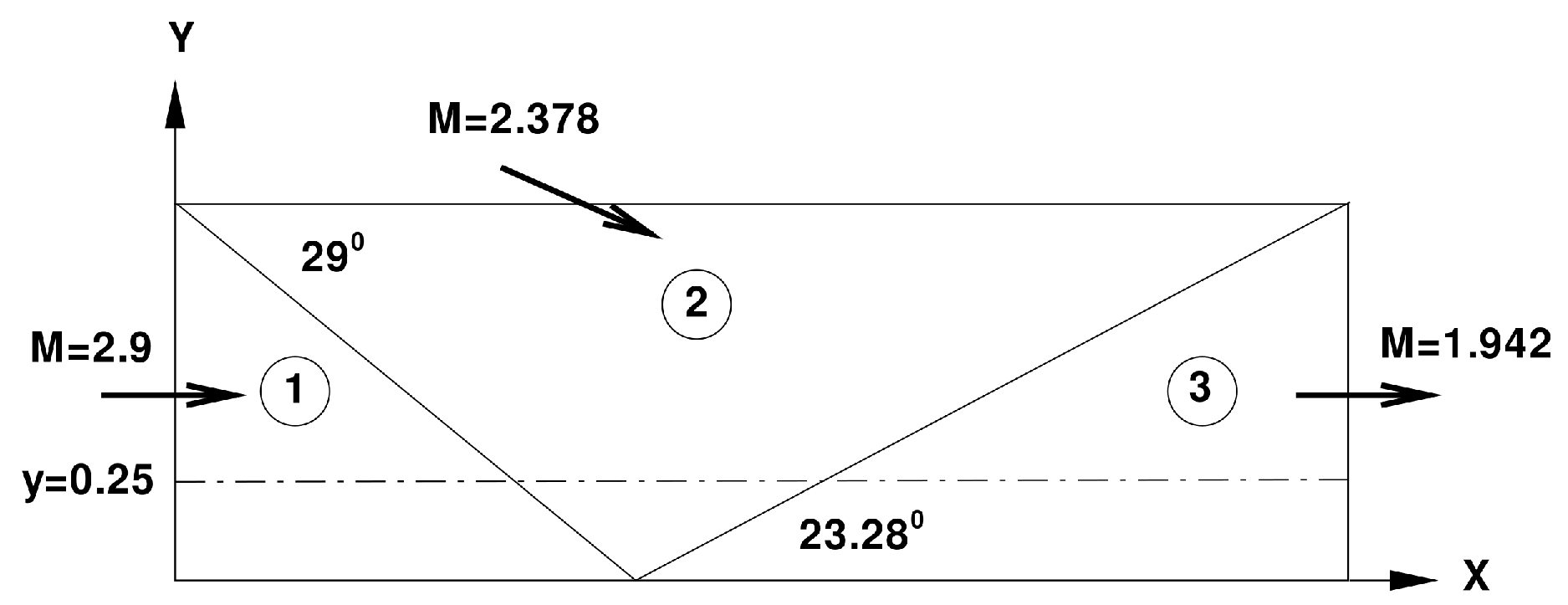
5.3. Oblique Shock
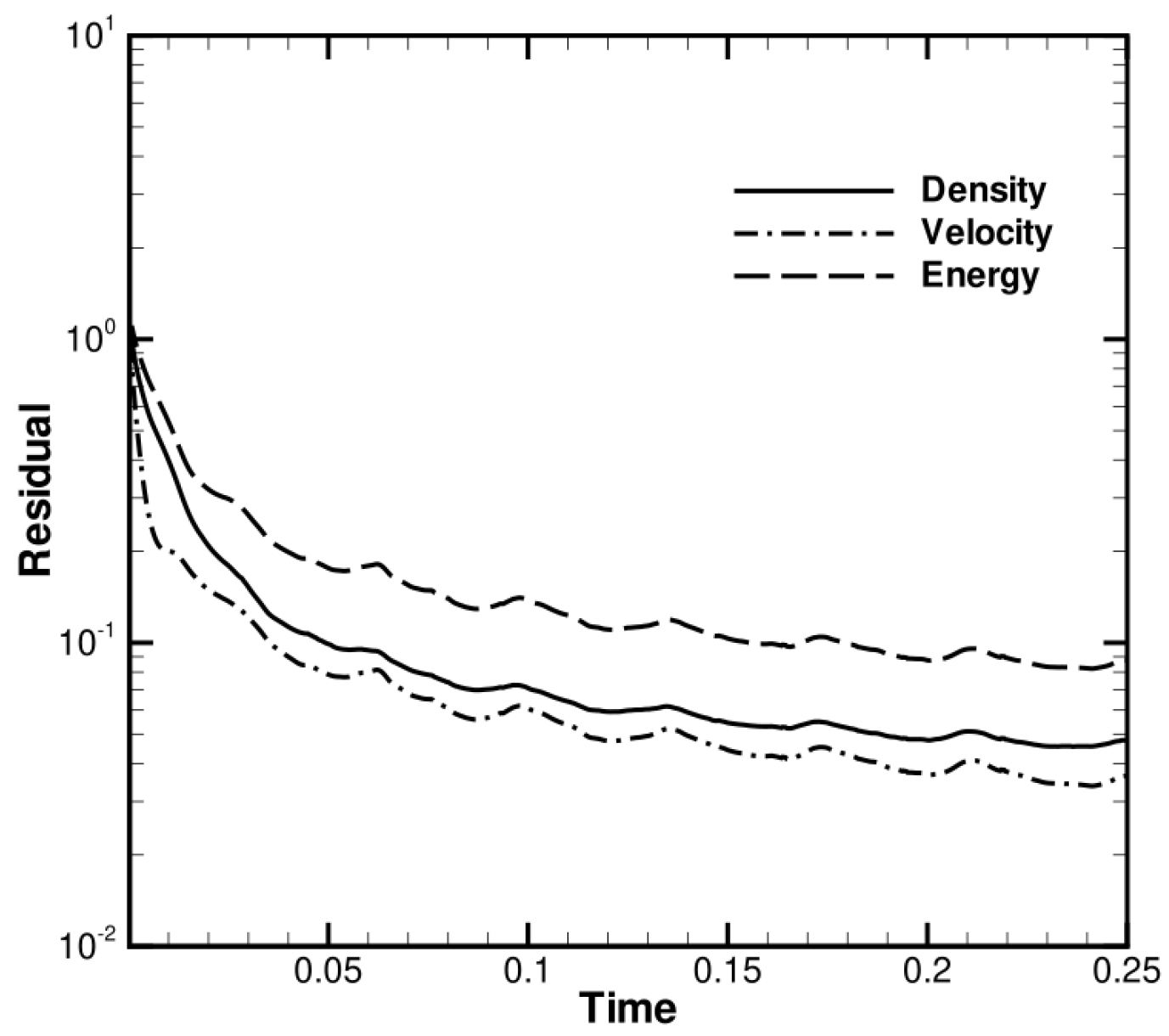
5.4. Reflected Shock Problem
5.5. 2D Explosion Problem
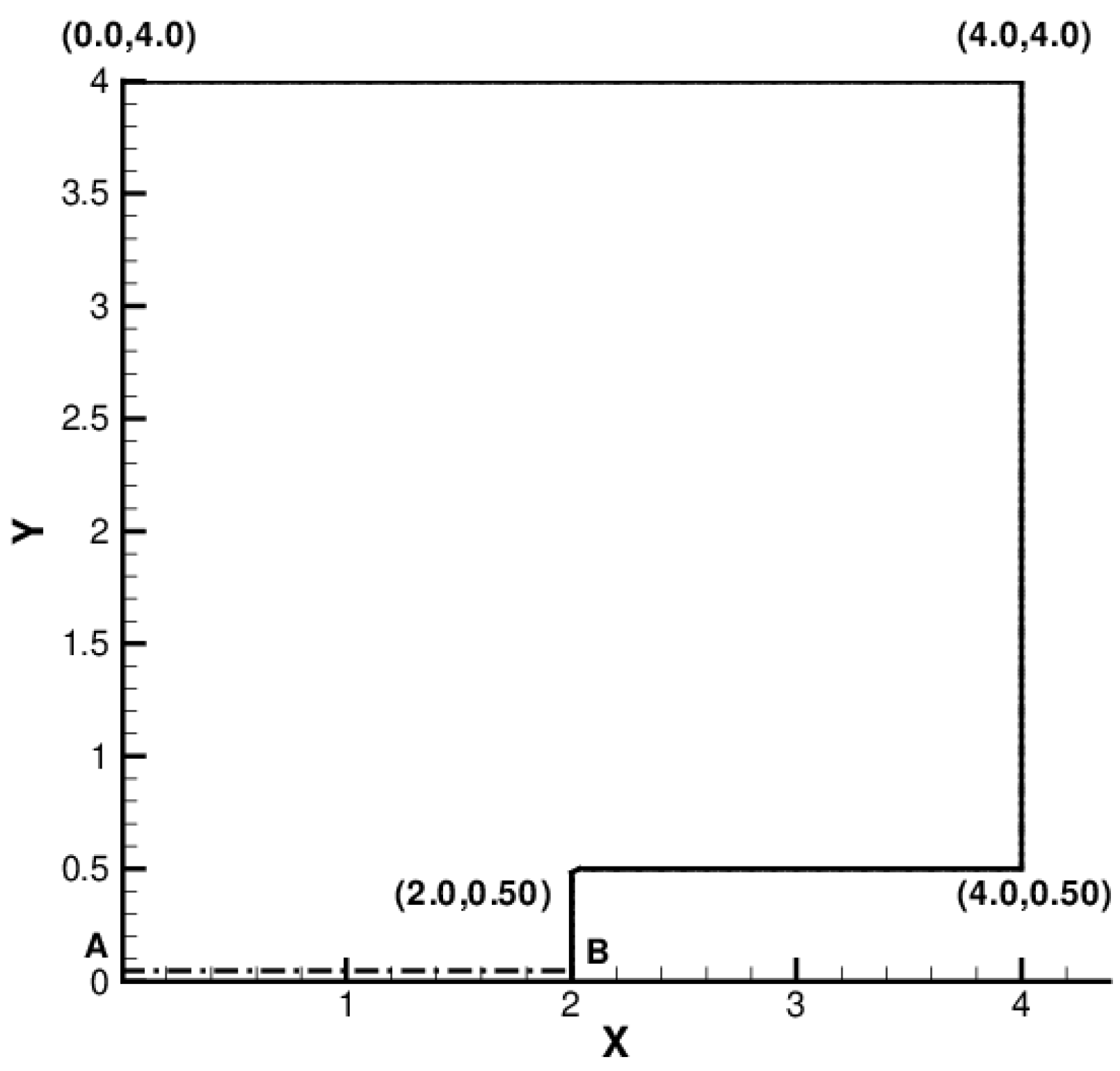
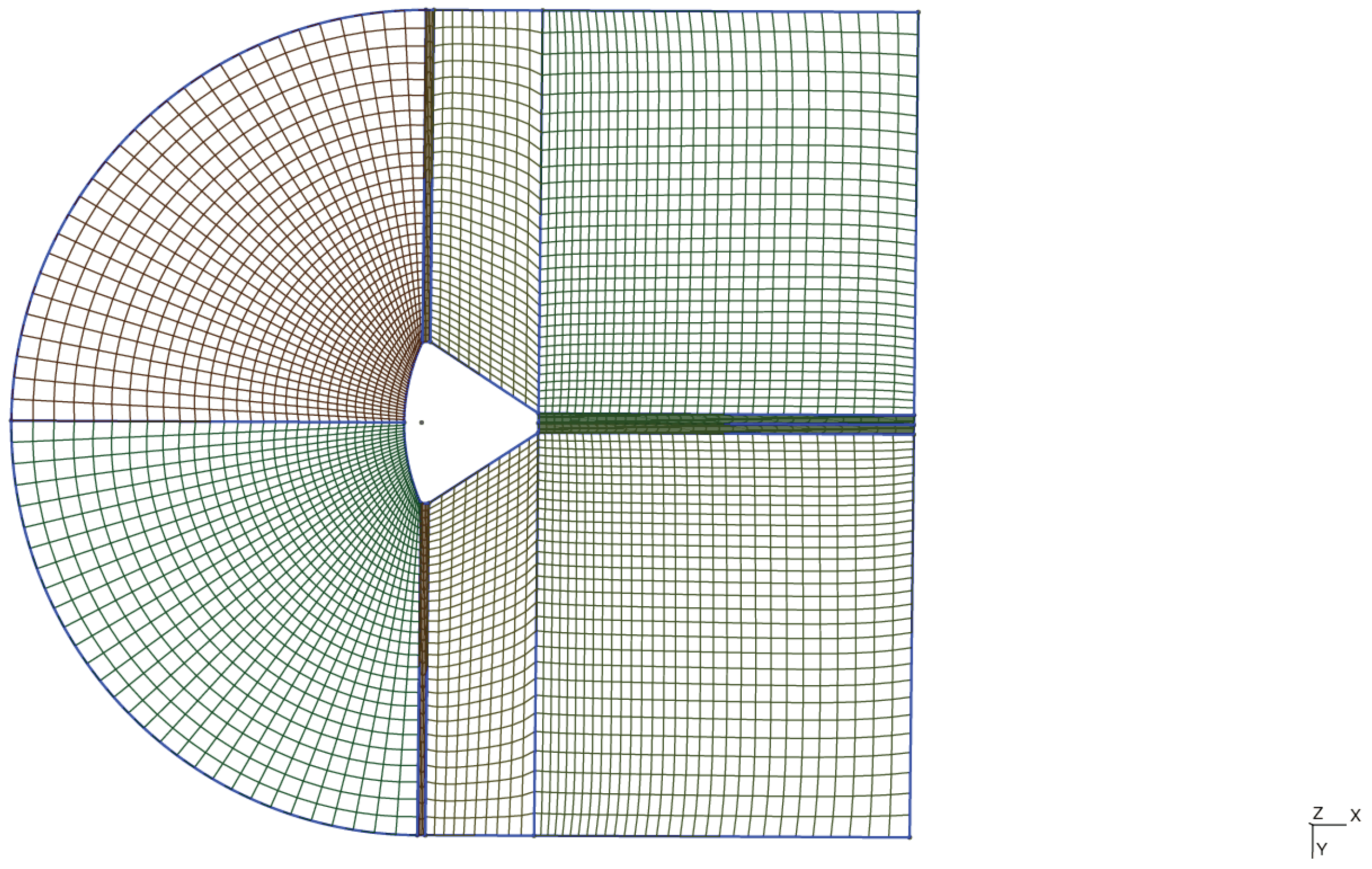
5.6. 2D Inviscid Flow Past Step Problem
5.6.1. Spectral/ Framework
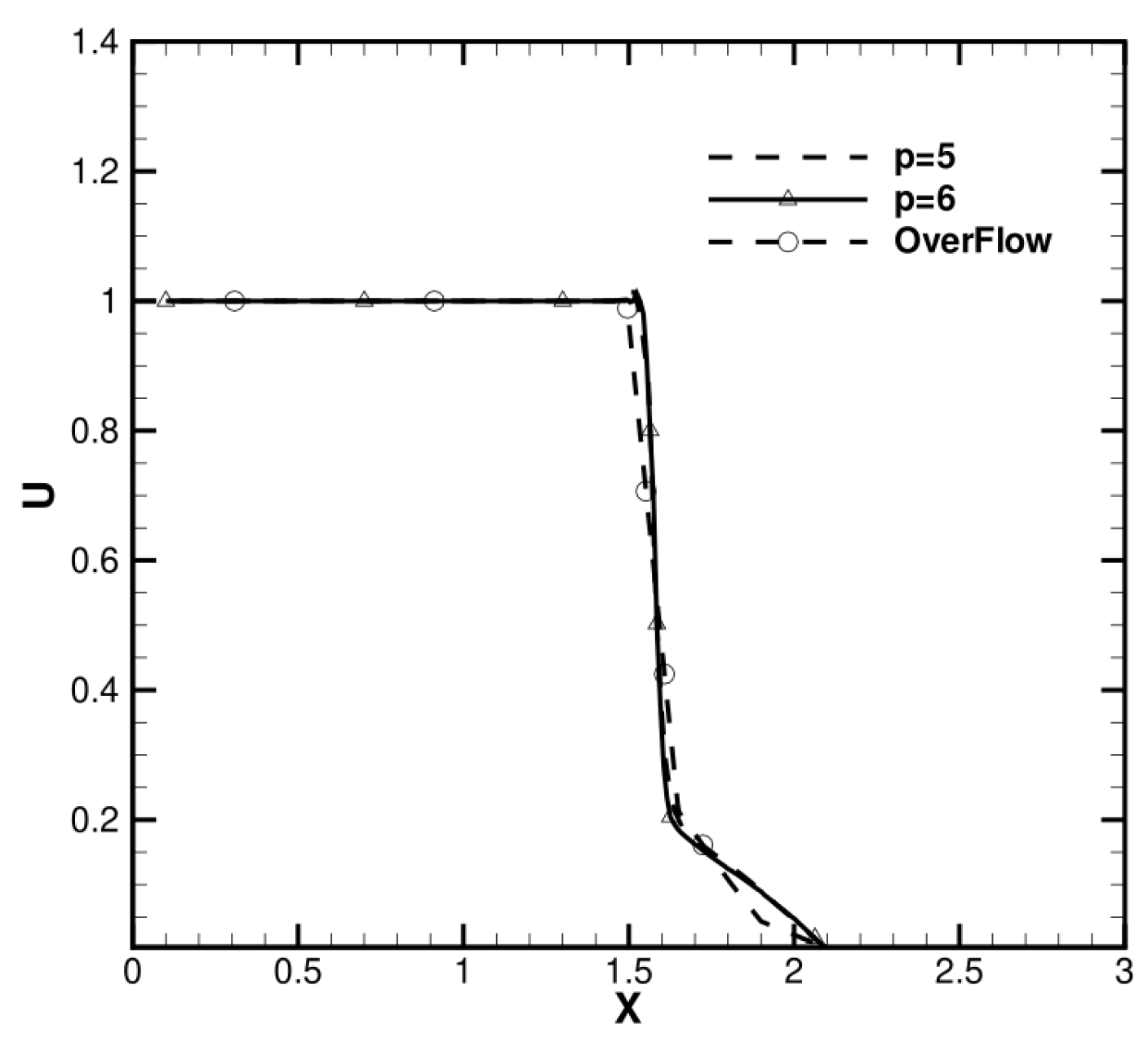
5.6.2. OverFlow Problem
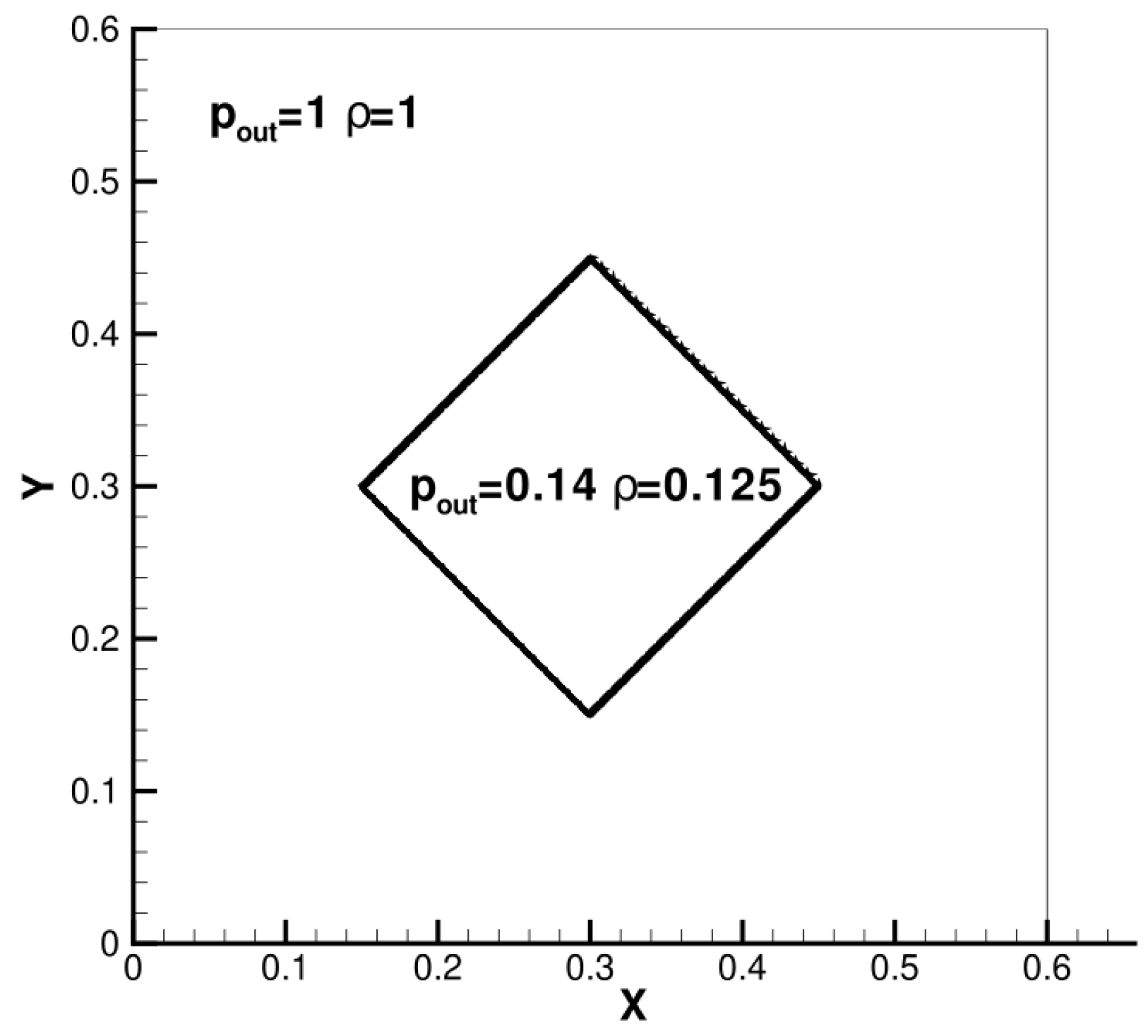
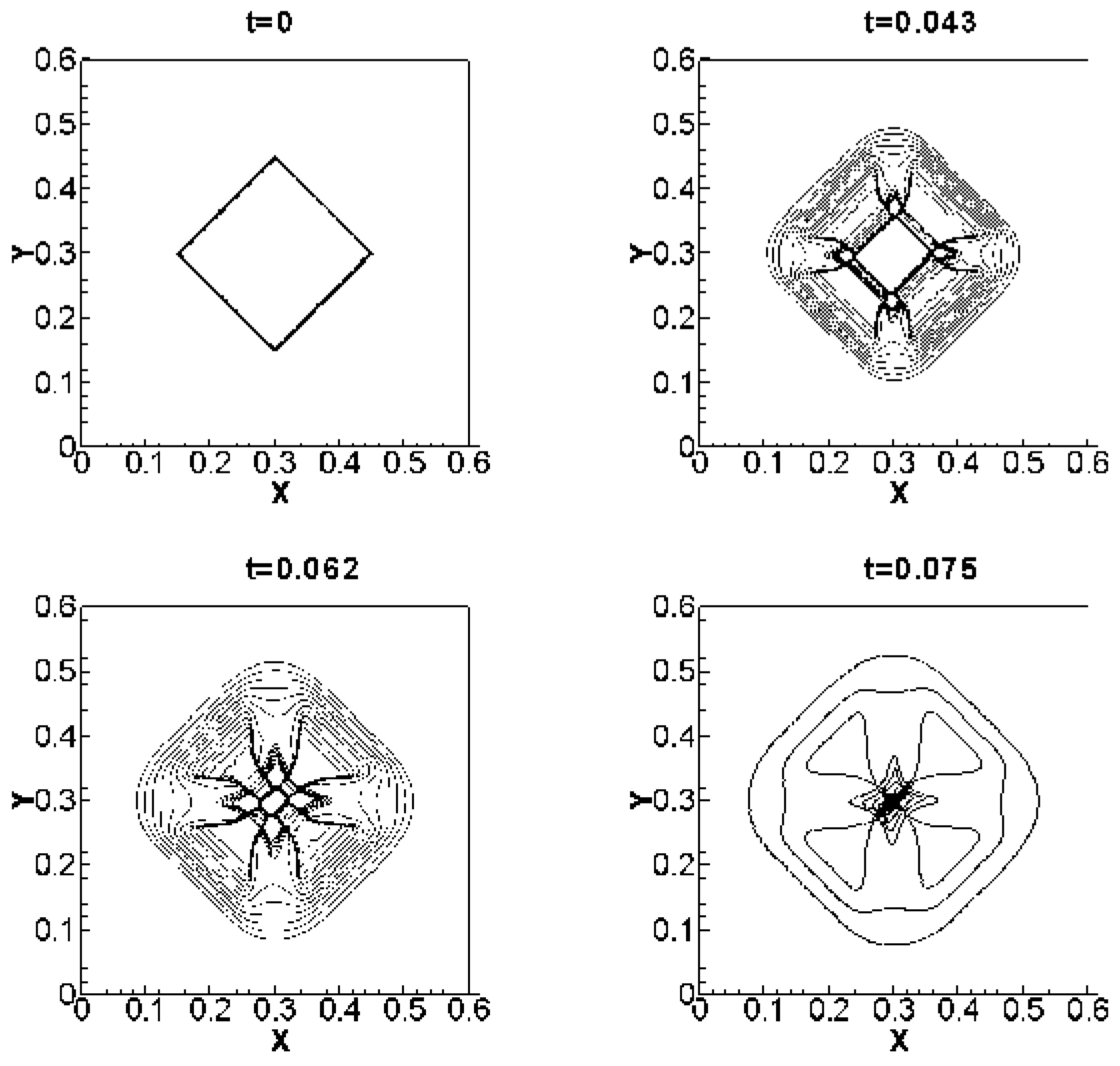
5.7. Implosion Problem
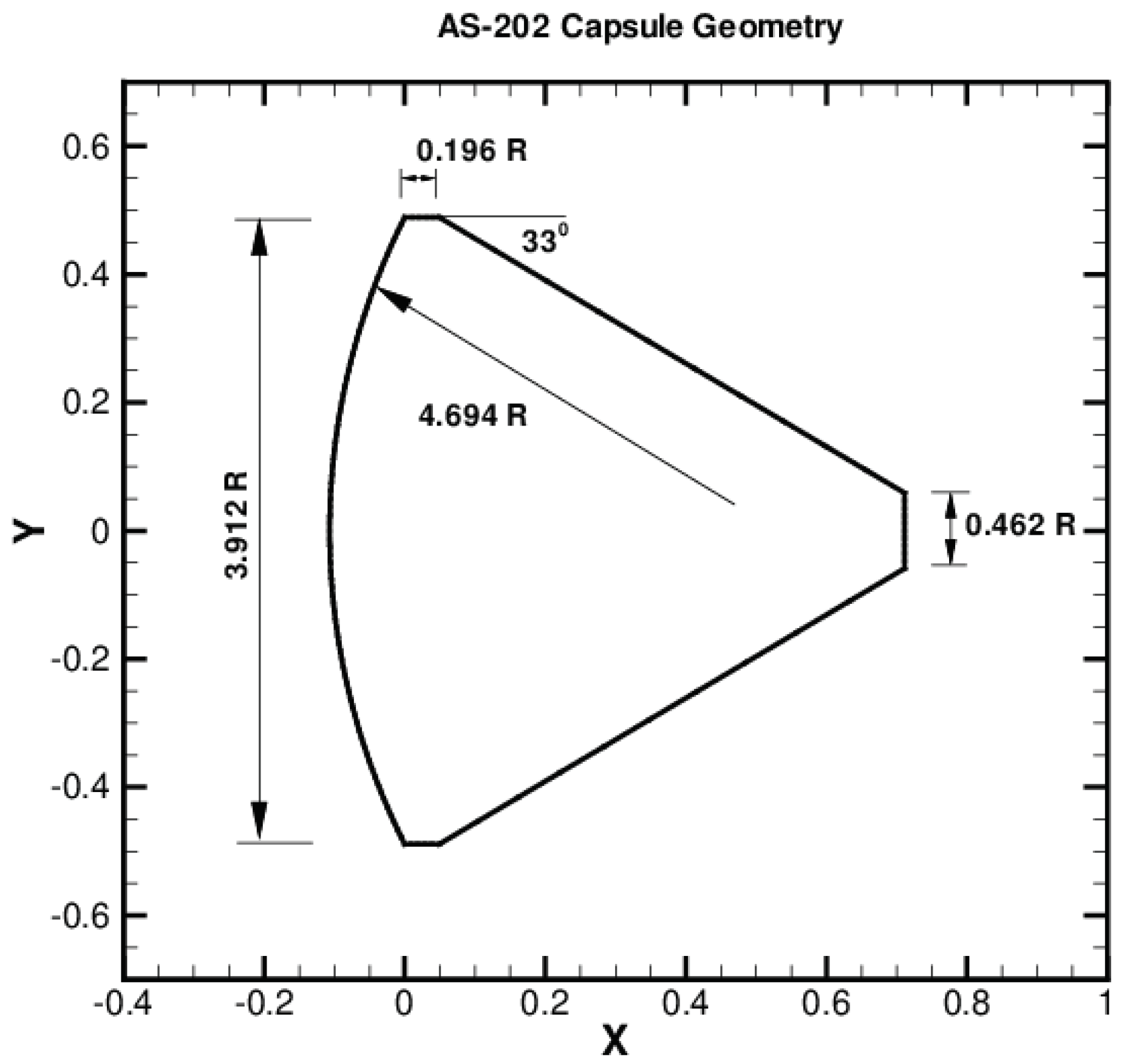
5.8. AS-202 Capsule
6. Conclusions
7. Dedication
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hughes, T.J.; Tezduyar, T.E. Development of Time-Accurate Finite Element Techniques for First Order Hyperbolic Systems with Emphasis on the Compressible Euler Equations; NASA: Washington, DC, USA, 1982. [Google Scholar]
- Hughes, T.J.; Tezduyar, T.E. Finite element methods for first-order hyperbolic systems with particular emphasis on the compressible Euler equations. Comput. Methods Appl. Mech. Eng. 1984, 45, 217–284. [Google Scholar] [CrossRef]
- Tezduyar, T.; Hughes, T. Finite element formulations for convection dominated flows with particular emphasis on the compressible Euler equations. In Proceedings of the 21st Aerospace Sciences Meeting, Reno, NV, USA, 10–13 January 1983; p. 125. [Google Scholar]
- Prabhu, D.K.; Tannehill, J.C. Numerical solution of space shuttle orbiter flowfield including real-gas effects. J. Spacecr. Rocket. 1986, 23, 264–272. [Google Scholar] [CrossRef]
- Ranjan, R.; Vedula, P.; Vogiatzis, K.; Josyula, E. Numerical Simulation of Hypersonic Turbulent Flows using High Order Methods. In Proceedings of the 2018 AIAA Aerospace Sciences Meeting, Kissimmee, FL, USA, 8–12 January 2018; p. 0980. [Google Scholar]
- Ranjan, R.; Chronopoulos, A.T.; Feng, Y. Computational algorithms for solving spectral/hp stabilized incompressible flow problems. J. Math. Res. 2016, 8, 21. [Google Scholar] [CrossRef]
- Ranjan, R.; Feng, Y.; Chronopolous, A.T. Augmented stabilized and galerkin least squares formulations. J. Math. Res. 2016, 8, 1. [Google Scholar] [CrossRef]
- Ranjan, R.; Feng, Y.; Chronopolous, A.T. Stabilized and Galerkin Least Squares Formulations; Technical Report; Department of Computer Science, University of Texas at San Antonio: San Antonio, TX, USA, 2016; Volume 1, pp. 1–42. [Google Scholar]
- Hughes, T.J.; Scovazzi, G.; Tezduyar, T.E. Stabilized methods for compressible flows. J. Sci. Comput. 2010, 43, 343–368. [Google Scholar] [CrossRef]
- Nichols, R.H.; Buning, P. Users manual for Overflow 2.2. Overflow 2019, v. 2.2n. [Google Scholar]
- Wissink, A.M.; Sitaraman, J.; Jayaraman, B.; Roget, B.; Lakshminarayan, V.K.; Potsdam, M.A.; Jain, R.; Bauer, A.; Strawn, R. Recent advancements in the Helios rotorcraft simulation code. In Proceedings of the 54th AIAA Aerospace Sciences Meeting, San Diego, CA, USA, 4–8 January 2016; p. 0563. [Google Scholar]
- Allu, S.; Surana, K.S.; Romkes, A.; Reddy, J.N. Numerical Solutions of BVPs in 2-D Viscous Compressible Flows Using hpk Framework. Int. J. Comput. Methods Eng. Sci. Mech. 2009, 10, 158–171. [Google Scholar] [CrossRef]
- Surana, K.; Ahmadi, A.; Reddy, J. k-version of Finite Element Method for Non-Linear Differential Operators in BVP. Int. J. Comput. Eng. Sci. 2004, 5, 133–207. [Google Scholar]
- Ibrahim, M.; Liu, F.; Liu, S. Concentration of mass in the pressureless limit of Euler equations for power law. Nonlinear Anal. Real World Appl. 2019, 47, 224–235. [Google Scholar] [CrossRef]
- Ibrahim, M.; Din, A.; Yusuf, A.; Lv, Y.P.; Jahanshahi, H.; Aly, A.A. Euler equations for isentropic gas dynamics with general pressure law. Adv. Contin. Discret. Models 2022, 2022, 10. [Google Scholar] [CrossRef]
- Huang, M.; Wang, Y.; Shao, Z. Piston problem for the isentropic Euler equations for a modified Chaplygin gas. Phys. Fluids 2023, 35, 016119. [Google Scholar] [CrossRef]
- Oden, J.T.; Demkowicz, L. hp adaptive finite element methods in computational fluid dynamics. Comput. Methods Appl. Mech. Eng. 1991, 89, 11–40. [Google Scholar] [CrossRef]
- Löhner, R.; Morgan, K.; Zienkiewicz, O. An adaptive finite element procedure for compressible high speed flows. Comput. Methods Appl. Mech. Eng. 1985, 51, 441–465. [Google Scholar] [CrossRef]
- Gottlieb, D.; Gottlieb, S. Spectral methods for compressible reactive flows. Comptes Rendus Méc. 2005, 333, 3–16. [Google Scholar] [CrossRef]
- Cantwell, C.D.; Moxey, D.; Comerford, A.; Bolis, A.; Rocco, G.; Mengaldo, G.; De Grazia, D.; Yakovlev, S.; Lombard, J.E.; Ekelschot, D.; et al. Nektar++: An open-source spectral/hp element framework. Comput. Phys. Commun. 2015, 192, 205–219. [Google Scholar] [CrossRef]
- Karamanos, G.; Karniadakis, G.E. A spectral vanishing viscosity method for large-eddy simulations. J. Comput. Phys. 2000, 163, 22–50. [Google Scholar] [CrossRef]
- Cottrell, J.A.; Hughes, T.J.; Bazilevs, Y. Isogeometric Analysis: Toward Integration of CAD and FEA; John Wiley & Sons: Chichester, UK, 2009. [Google Scholar]
- Jaeschke, A.M. Isogeometric Analysis for Compressible Flows with Application in Turbomachinery. Ph.D. Dissertation, TU Delft, Delft, The Netherlands, 2015; pp. 1–107. [Google Scholar]
- Ranjan, R.; Catabriga, L.; Feng, Y. High-Order Spectral/hp-Based Solver for Compressible Navier–Stokes Equations. AIAA J. 2022, 60, 2972–2986. [Google Scholar] [CrossRef]
- Ferziger, J.H.; Peric, M. Computational Methods for Fluid Dynamics; Springer Science & Business Media: Cham, Switzerland, 2012. [Google Scholar]
- Tannehill, J.C.; Anderson, D.A.; Pletcher, R.H. Computational fluid dynamics and heat transfer. In Series in Computational and Physical Processes in Mechanics and Thermal Sciences; CRC Press: Boca Raton, FL, USA, 2016. [Google Scholar]
- Versteeg, H.K.; Malalasekera, W. An Introduction to Computational Fluid Dynamics the Finite Volume Method; Prentice Hall: Maldon, UK, 1995. [Google Scholar]
- Roe, P.L. Characteristic-Based Schemes for the Euler Equations. Annu. Rev. Fluid Mech. 1986, 18, 337–365. [Google Scholar] [CrossRef]
- Agarwal, R. Computational fluid dynamics of whole-body aircraft. Annu. Rev. Fluid Mech. 1999, 31, 125–169. [Google Scholar] [CrossRef]
- Gisler, G.R. Tsunami Simulations. Annu. Rev. Fluid Mech. 2008, 40, 71–90. [Google Scholar] [CrossRef]
- Pirozzoli, S. Numerical Methods for High-Speed Flows. Annu. Rev. Fluid Mech. 2011, 43, 163–194. [Google Scholar] [CrossRef]
- Hirsch, C. Numerical computation of internal and external flows. In Computational Methods for Inviscid and Viscous Flows; Vrije Universiteit Brussel: Brussels, Belgium, 1990; Volume 2. [Google Scholar]
- Karniadakis, G.E.; Sherwin, S. Spectral/hp Element Methods for CFD; Oxford University Press: New York, NY, USA, 1999. [Google Scholar]
- Marsden, A.L. Optimization in Cardiovascular Modeling. Annu. Rev. Fluid Mech. 2014, 46, 519–546. [Google Scholar] [CrossRef]
- Mavriplis, D.J. Unstructured grid techniques. Annu. Rev. Fluid Mech. 1997, 29, 473–514. [Google Scholar] [CrossRef]
- Kirk, B.S.; Carey, G.F. Development and validation of a SUPG finite element scheme for the compressible Navier–Stokes equations using a modified inviscid flux discretization. Int. J. Numer. Methods Fluids 2008, 57, 265–293. [Google Scholar] [CrossRef]
- Catabriga, L.; Coutinho, A.L. Implicit SUPG solution of Euler equations using edge-based data structures. Comput. Methods Appl. Mech. Eng. 2002, 191, 3477–3490. [Google Scholar] [CrossRef]
- Catabriga, L.; Coutinho, A.; Tezduyar, T.E. Finite element SUPG parameters computed from local dof-matrices for compressible flows. In Proceedings of the 24th Iberian Latin-American Congress on Computational Methods in Engineering, Ouro Preto, Brazil, 29–31 October 2003. [Google Scholar]
- Catabriga, L.; Coutinho, A.L.; Tezduyar, T.E. Compressible flow SUPG stabilization parameters computed from degree-of-freedom submatrices. Comput. Mech. 2006, 38, 334–343. [Google Scholar] [CrossRef]
- Hughes, T.J.; Mallet, M. A new finite element formulation for computational fluid dynamics: III. The generalized streamline operator for multidimensional advective-diffusive systems. Comput. Methods Appl. Mech. Eng. 1986, 58, 305–328. [Google Scholar] [CrossRef]
- Tezduyar, T.E.; Senga, M. SUPG finite element computation of inviscid supersonic flows with YZβ shock-capturing. Comput. Fluids 2007, 36, 147–159. [Google Scholar] [CrossRef]
- Jiang, B.n. The Least-Squares Finite Element Method: Theory and Applications in Computational Fluid Dynamics and Electromagnetics; Springer Science: New York, NY, USA, 2013. [Google Scholar]
- Shakib, F.; Hughes, T.J.; Johan, Z. A new finite element formulation for computational fluid dynamics: X. The compressible Euler and Navier–Stokes equations. Comput. Methods Appl. Mech. Eng. 1991, 89, 141–219. [Google Scholar] [CrossRef]
- Bova, S.; Bond, R.; Kirk, B. Stabilized finite element scheme for high speed flows with chemical non-equilibrium. In Proceedings of the 48th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition, Orlando, FL, USA, 4–7 January 2010; p. 1560. [Google Scholar]
- Kirk, B.S. Adaptive Finite Element Simulation of Flow and Transport Applications on Parallel Computers. Ph.D. Thesis, The University of Texas at Austin, Austin, TX, USA, 2007. [Google Scholar]
- Kirk, B.; Bova, S.; Bond, R. A Streamline-Upwind Petrov-Galerkin Finite Element Scheme for Non-Ionized Hypersonic Flows in Thermochemical Nonequilibrium. In Proceedings of the 49th AIAA Aerospace Sciences Meeting including the New Horizons Forum and Aerospace Exposition, Orlando, FL, USA, 4–7 January 2011; p. 134. [Google Scholar]
- Aliabadi, S.K. Parallel Finite Element Computations in Aerospace Applications. Ph.D. Thesis, University of Minnesota, Minneapolis, MN, USA, 1994. [Google Scholar]
- Aliabadi, S.K.; Tezduyar, T.E. Parallel fluid dynamics computations in aerospace applications. Int. J. Numer. Methods Fluids 1995, 21, 783–805. [Google Scholar] [CrossRef]
- Le Beau, G.J. The Finite Element Computation of Compressible Flows. Ph.D. Thesis, University of Minnesota, Minneapolis, MN, USA, 1990. [Google Scholar]
- Senga, M. Improved SUPG Formulations for Compressible Flows. Ph.D. Thesis, Rice University, Houston, TX, USA, 2006. [Google Scholar]
- Bento, S.S.; Barbosa, P.W.; Santos, I.P.; de Lima, L.M.; Catabriga, L. A Nonlinear Finite Element Formulation Based on Multiscale Approach to Solve Compressible Euler Equations. In Proceedings of the International Conference on Computational Science and Its Applications, Trieste, Italy, 3–6 July 2017; pp. 735–743. [Google Scholar]
- Bento, S.S.; de Lima, L.M.; Sedano, R.Z.; Catabriga, L.; Santos, I.P. A Nonlinear Multiscale Viscosity Method to Solve Compressible Flow Problems. In Proceedings of the 16th International Conference on Computational Science and Its Applications ICCSA 2016, Beijing, China, 4–7 July 2016; Proceedings, Part I. pp. 3–17. [Google Scholar]
- Kovasznay, L. Laminar flow behind a two-dimensional grid. In Mathematical Proceedings of the Cambridge Philosophical Society; Cambridge University Press: Cambridge, UK, 1948; Volume 44, pp. 58–62. [Google Scholar]
- Toro, E.F. Riemann Solvers and Numerical Methods for Fluid Dynamics: A Practical Introduction; Springer Science & Business Media: Heidelberg/Berlin, Germany, 2013. [Google Scholar]
- Nazarov, M. An Adaptive Finite Element Method for the Compressible Euler Equations. Ph.D. Thesis, KTH, Stockholm, Sweden, 2009. [Google Scholar]
- Chhunchha, A.C. Aerodynamic Heating Analysis of Re-Entry Space Capsule Using Computational Fluid Dynamics. Master’s Thesis, California State University, Long Beach, CA, USA, 2018. [Google Scholar]
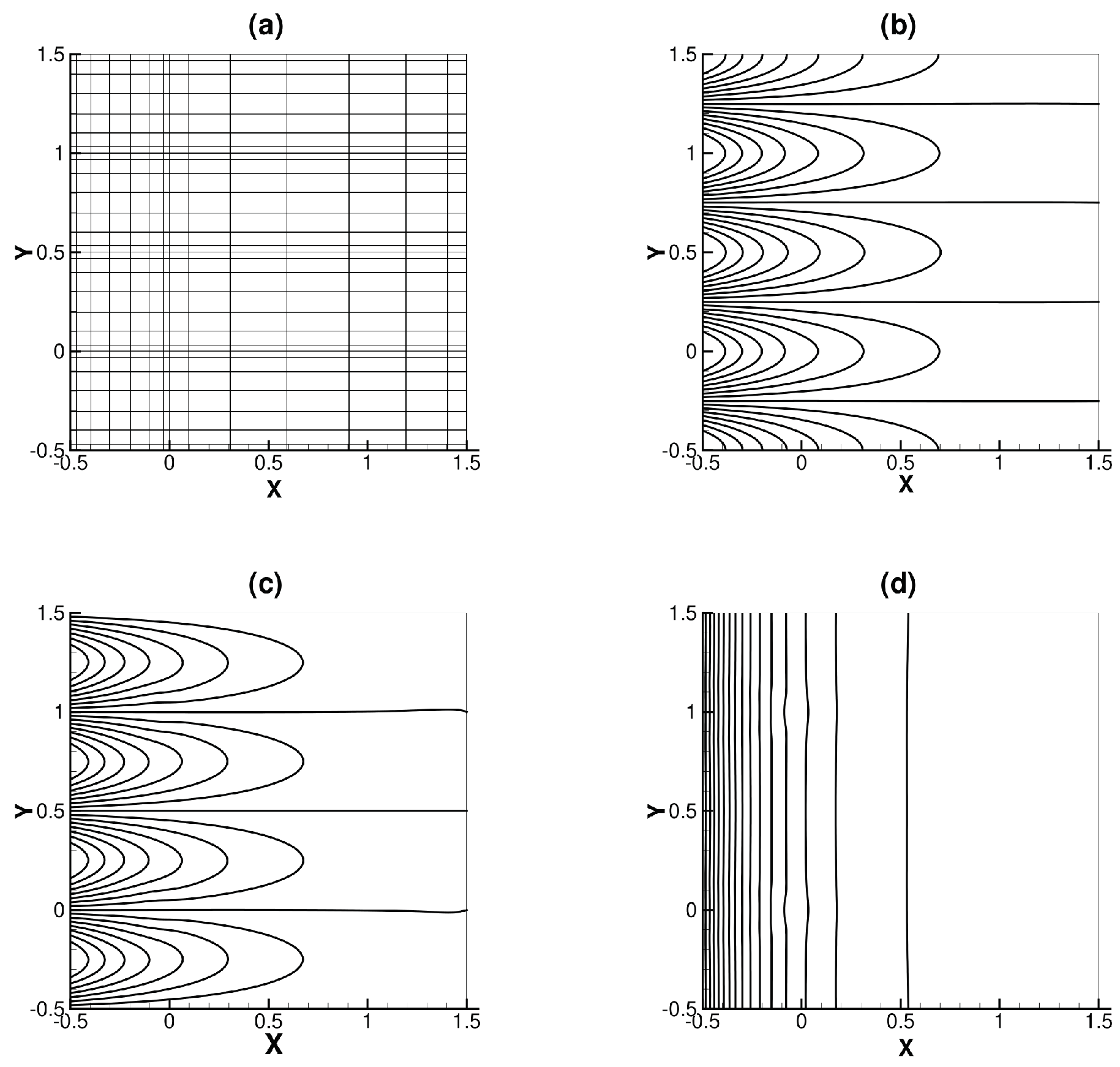
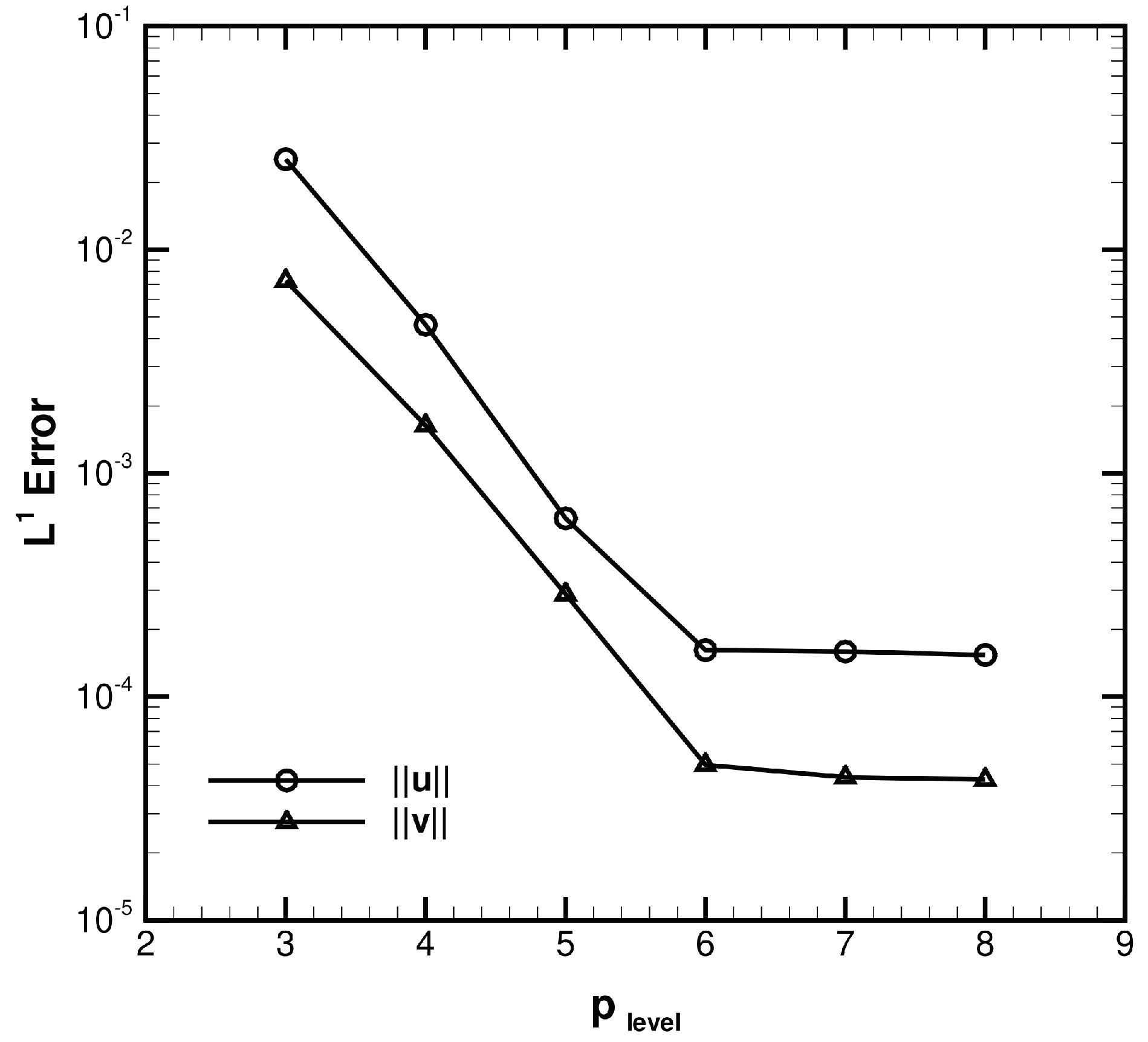
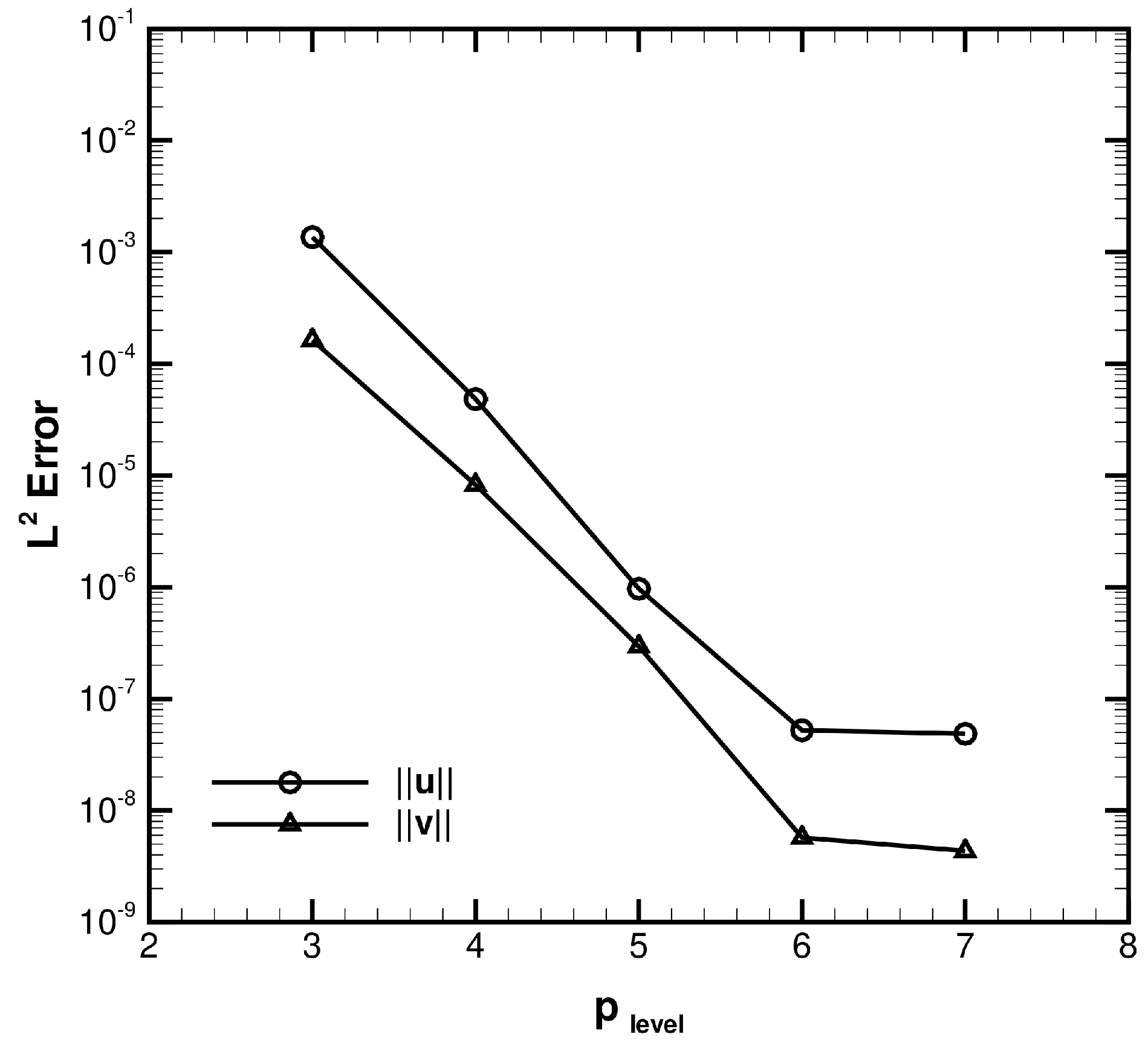
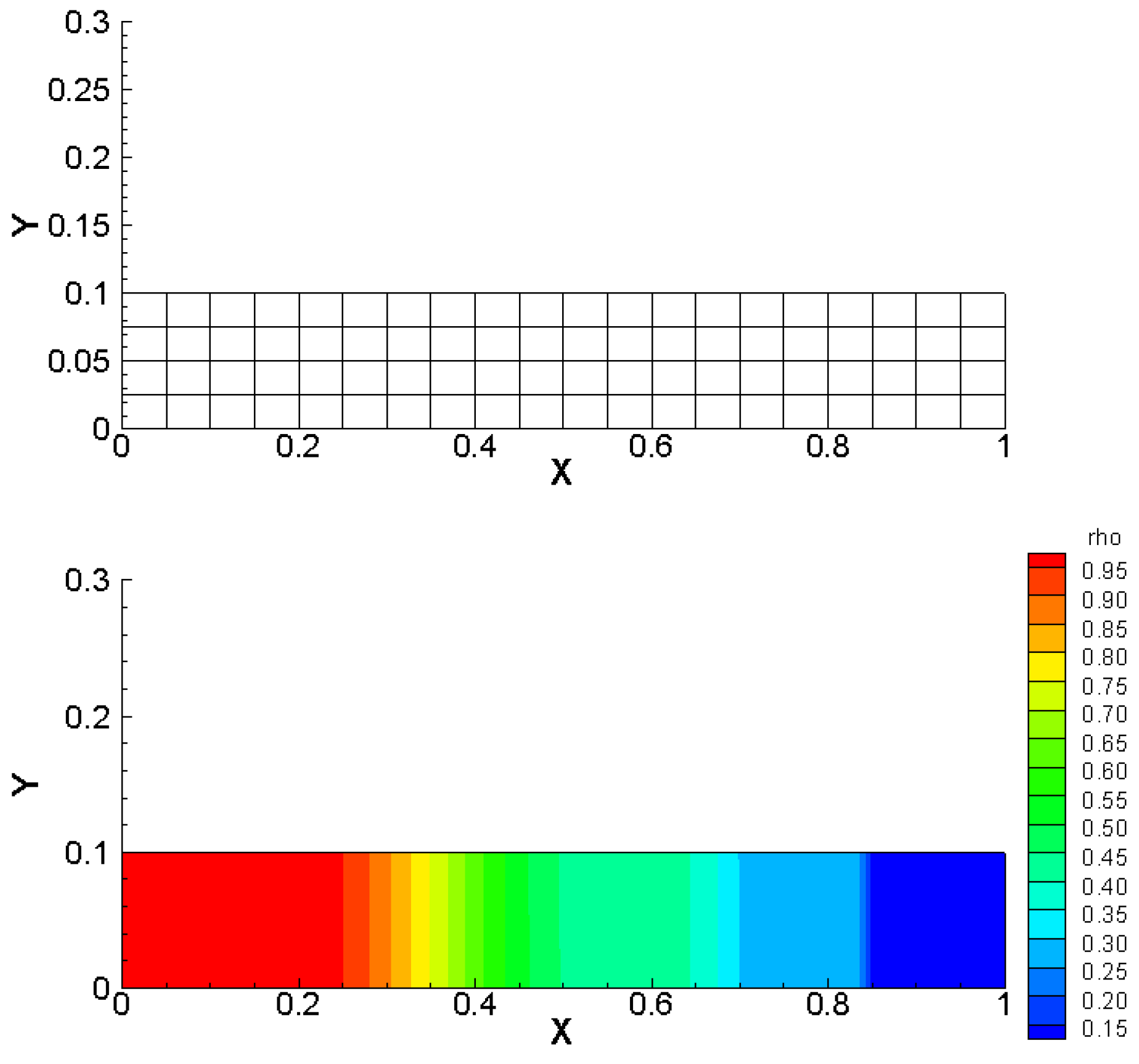












| Time (s) | ||
|---|---|---|
| 3 | 91 | 7 |
| 4 | 153 | 25 |
| 5 | 231 | 86 |
| 6 | 325 | 248 |
| 7 | 435 | 808 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ranjan, R.; Catabriga, L.; Araya, G. A Spectral/hp-Based Stabilized Solver with Emphasis on the Euler Equations. Fluids 2024, 9, 18. https://doi.org/10.3390/fluids9010018
Ranjan R, Catabriga L, Araya G. A Spectral/hp-Based Stabilized Solver with Emphasis on the Euler Equations. Fluids. 2024; 9(1):18. https://doi.org/10.3390/fluids9010018
Chicago/Turabian StyleRanjan, Rakesh, Lucia Catabriga, and Guillermo Araya. 2024. "A Spectral/hp-Based Stabilized Solver with Emphasis on the Euler Equations" Fluids 9, no. 1: 18. https://doi.org/10.3390/fluids9010018
APA StyleRanjan, R., Catabriga, L., & Araya, G. (2024). A Spectral/hp-Based Stabilized Solver with Emphasis on the Euler Equations. Fluids, 9(1), 18. https://doi.org/10.3390/fluids9010018








