Flow and Aeroacoustic Characteristics of Underexpanded Supersonic Jets Exhausting from a Conical Converging Nozzle
Abstract
1. Introduction
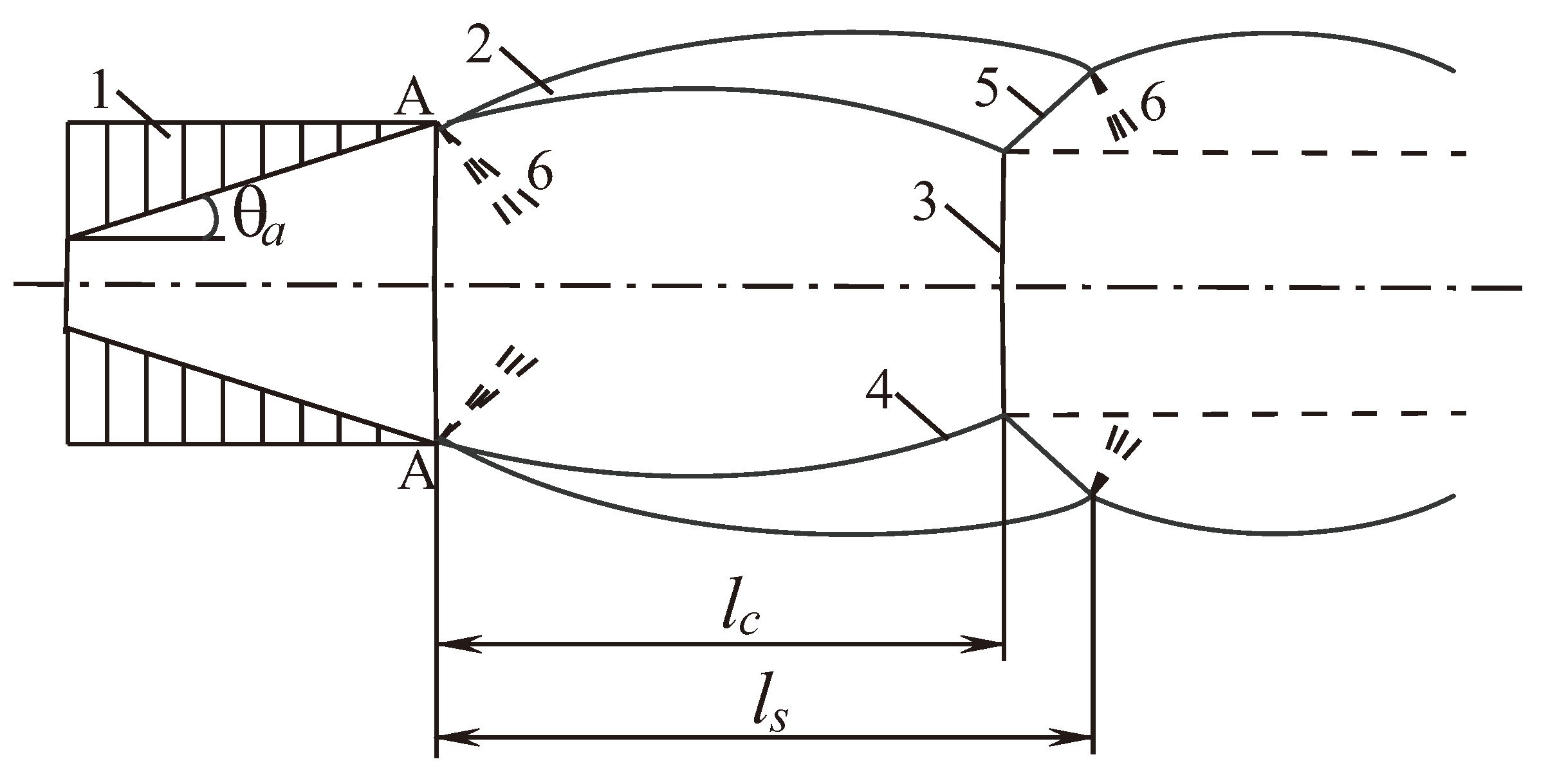
2. Structure of Supersonic Jet
3. Sources of Jet Noise
3.1. Mixing Noise
3.2. Broadband Shock Wave Noise
3.3. Discrete Tone
4. Jet Flow Field
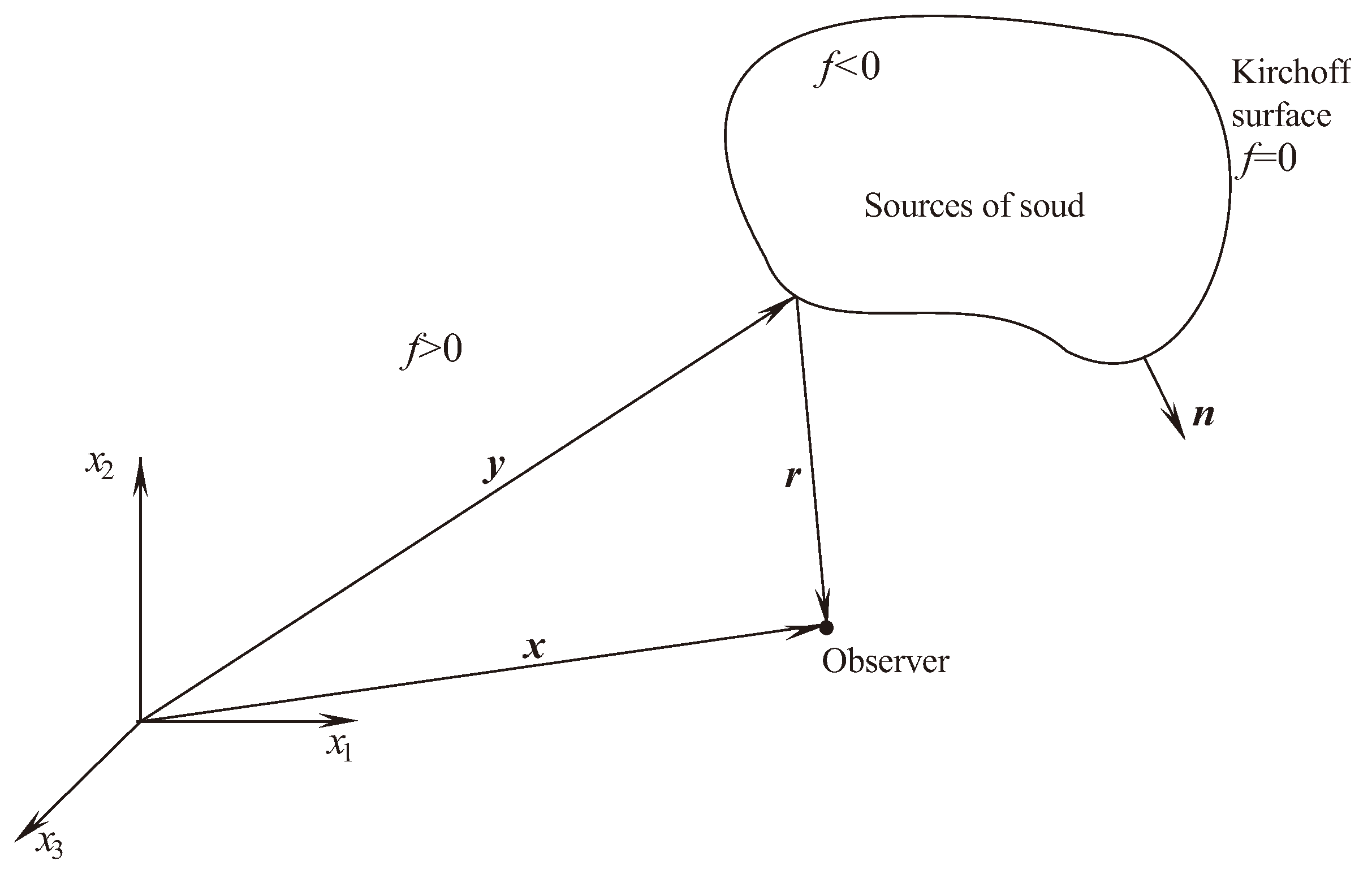
5. Jet Acoustic Field
- —
- Farassat’s formulation I
- —
- Farassat’s formulation II
6. Numerical Method
6.1. CFD Solver
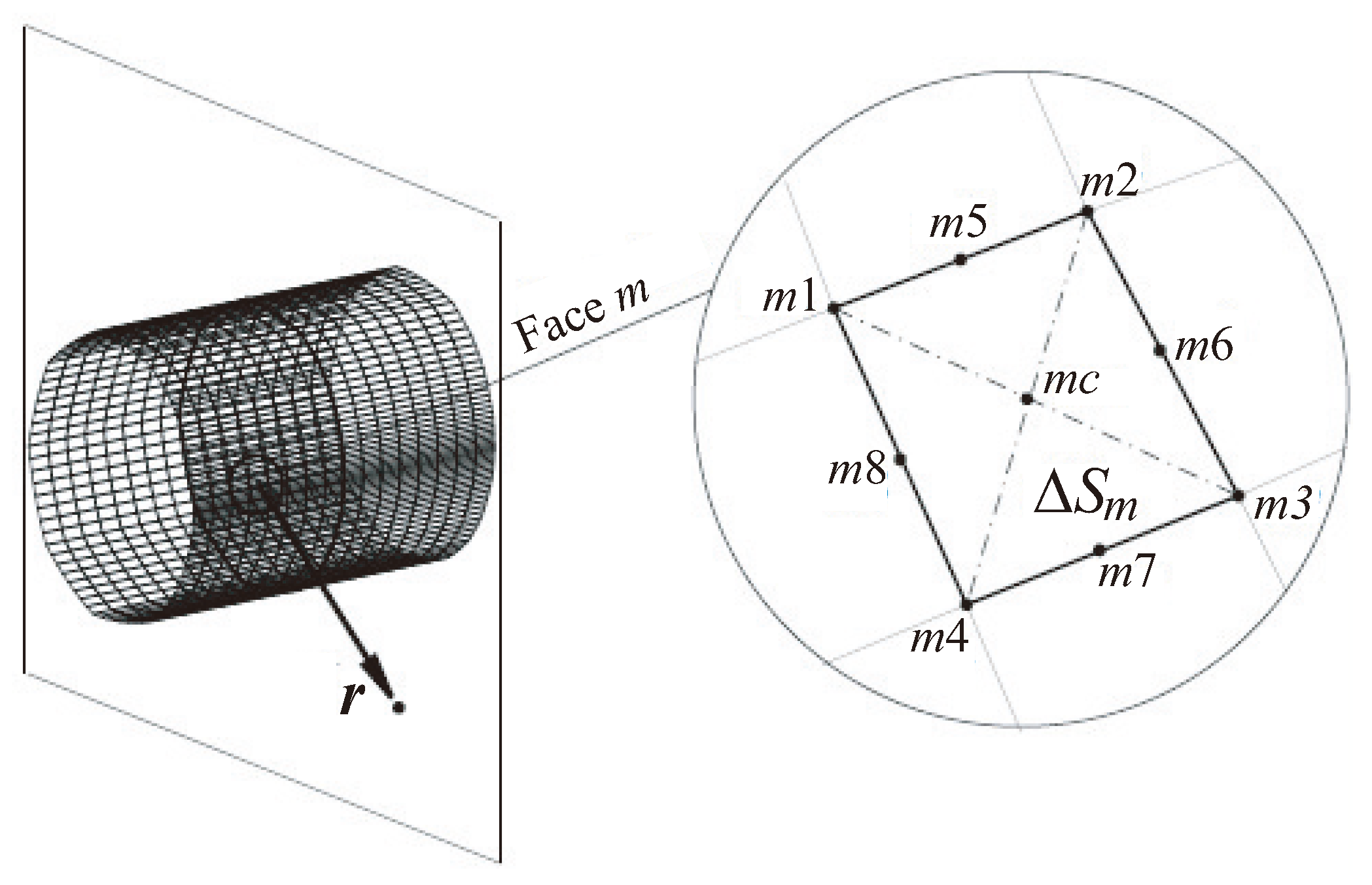
6.2. CAA Solver
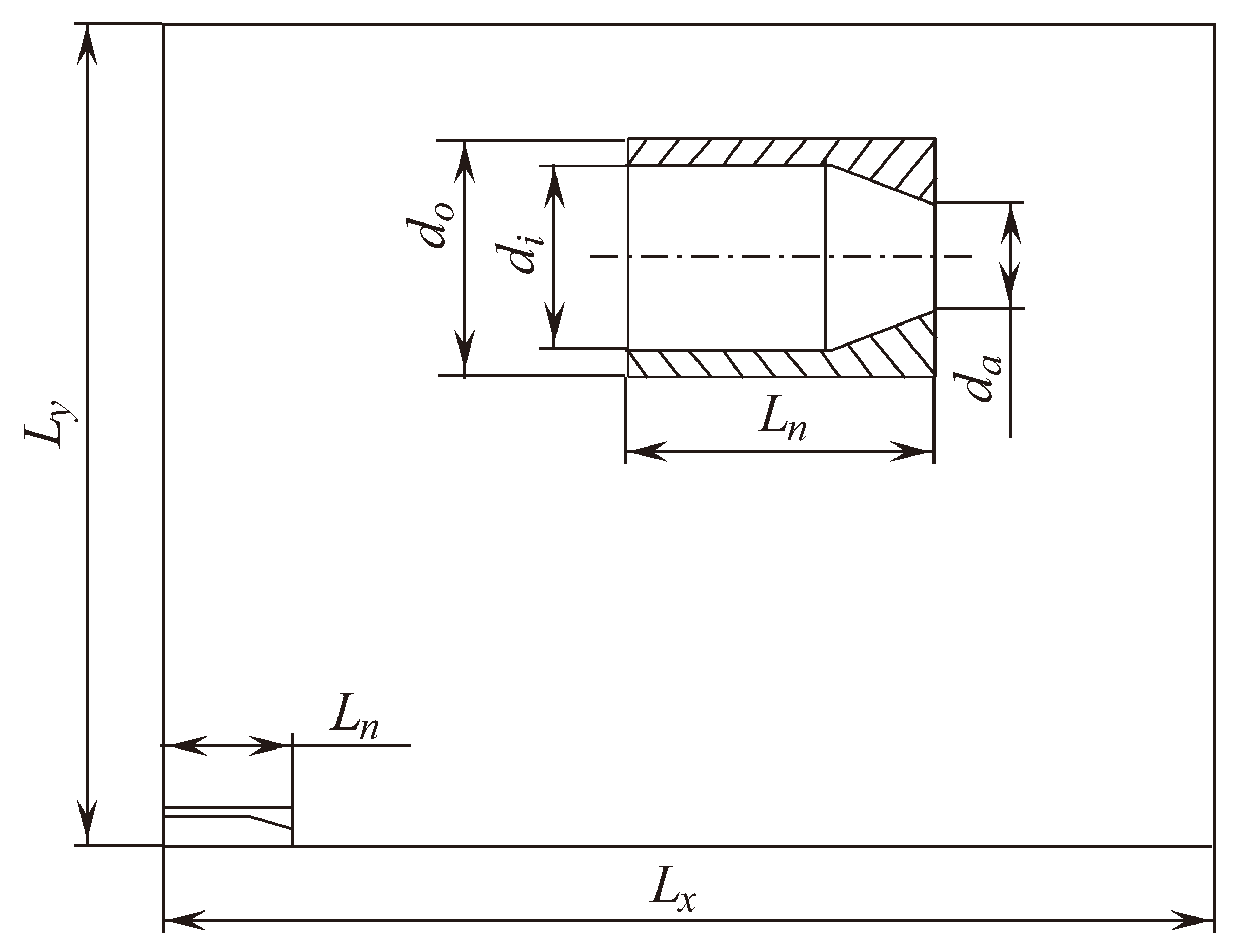
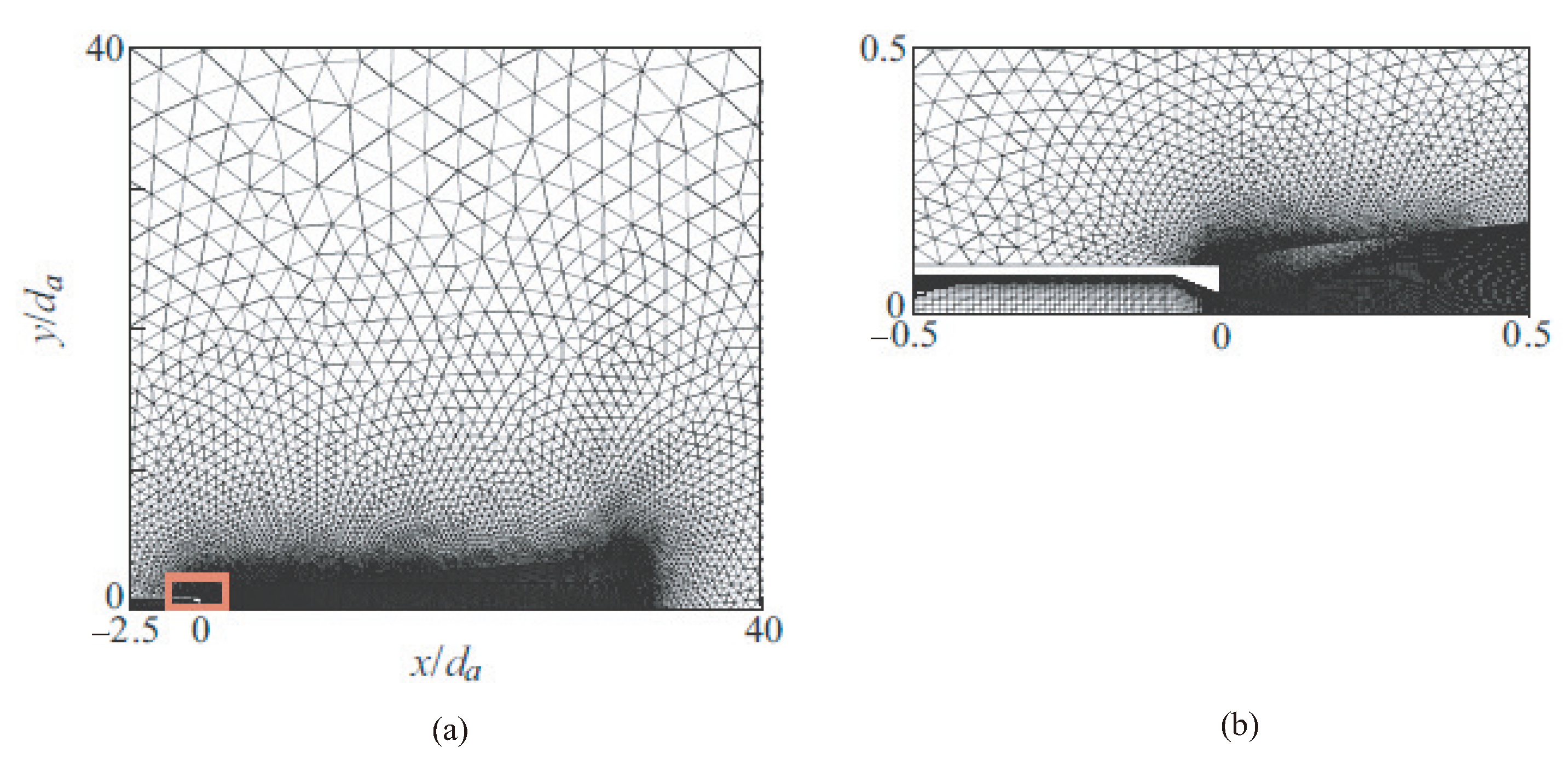
7. Geometry and Grid
8. Results and Discussion
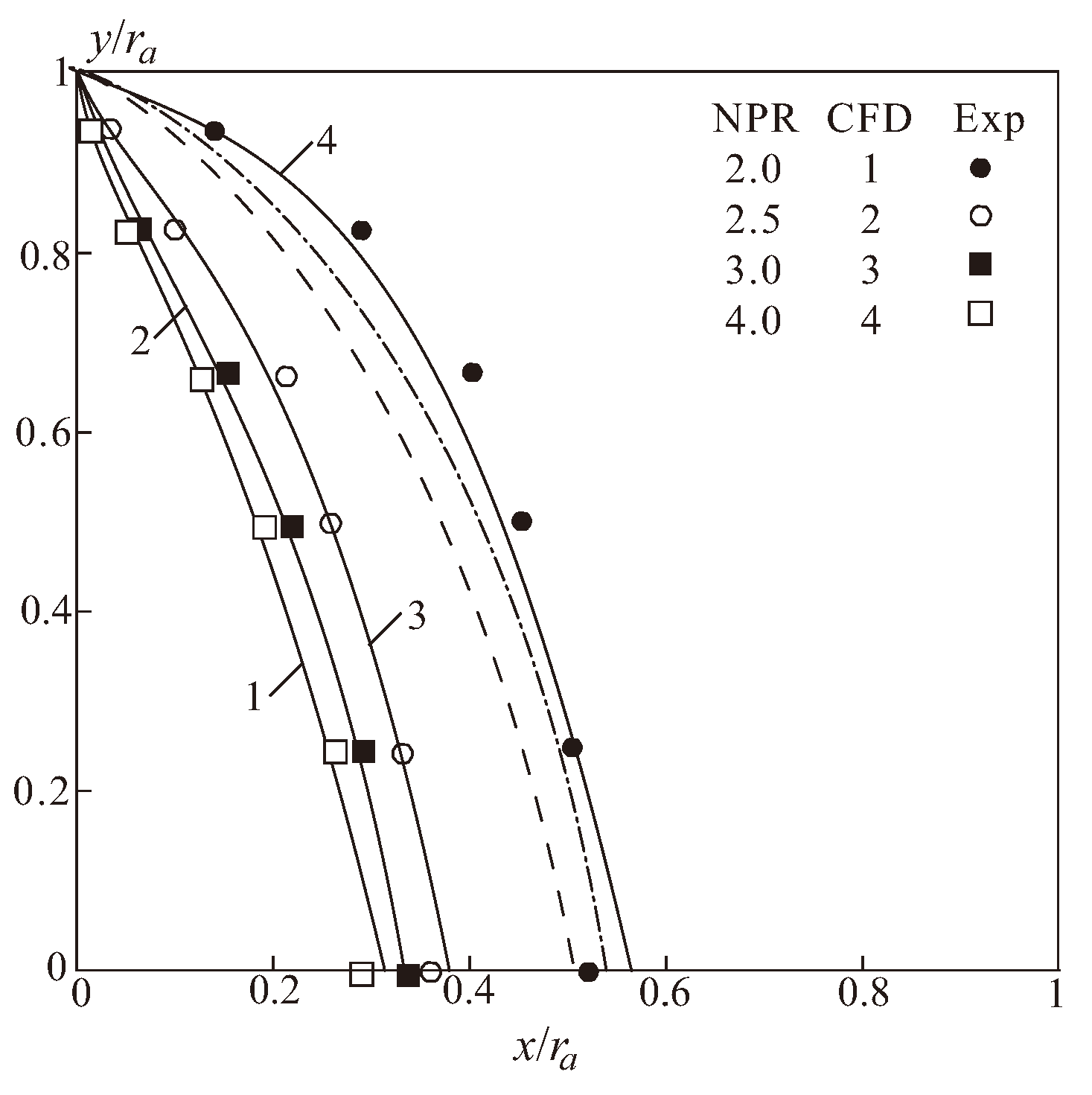
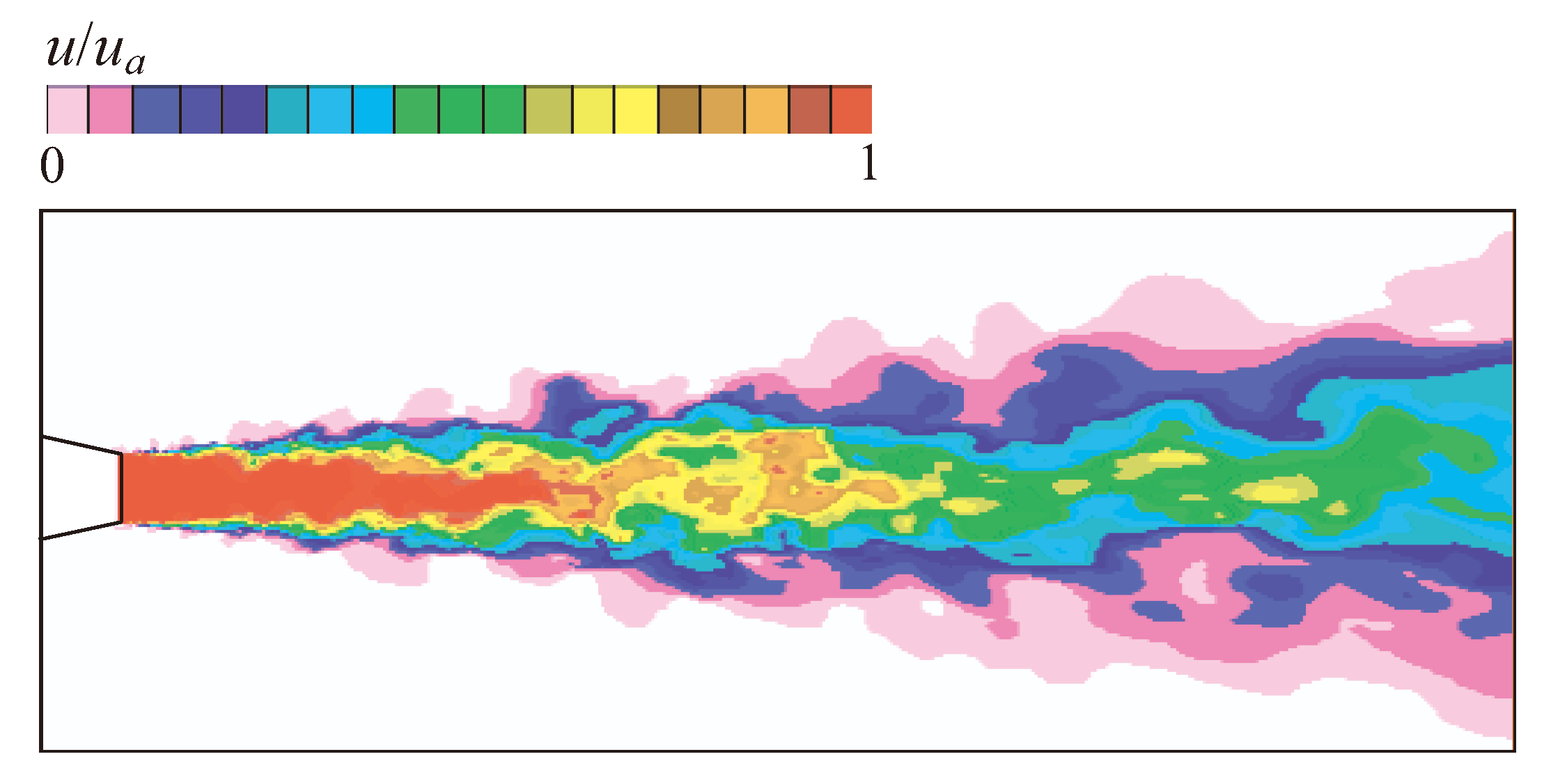
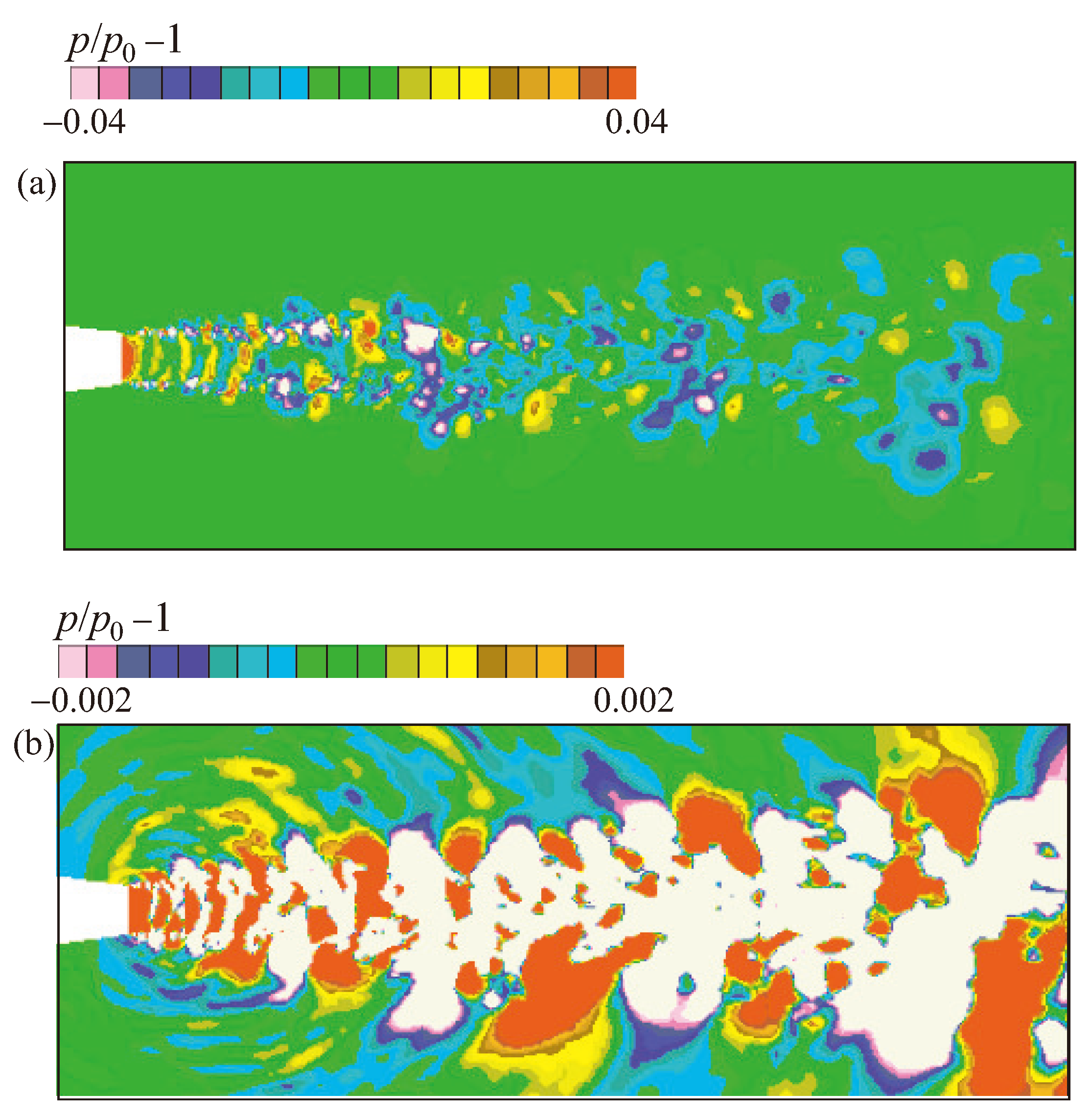
8.1. Jet Structure
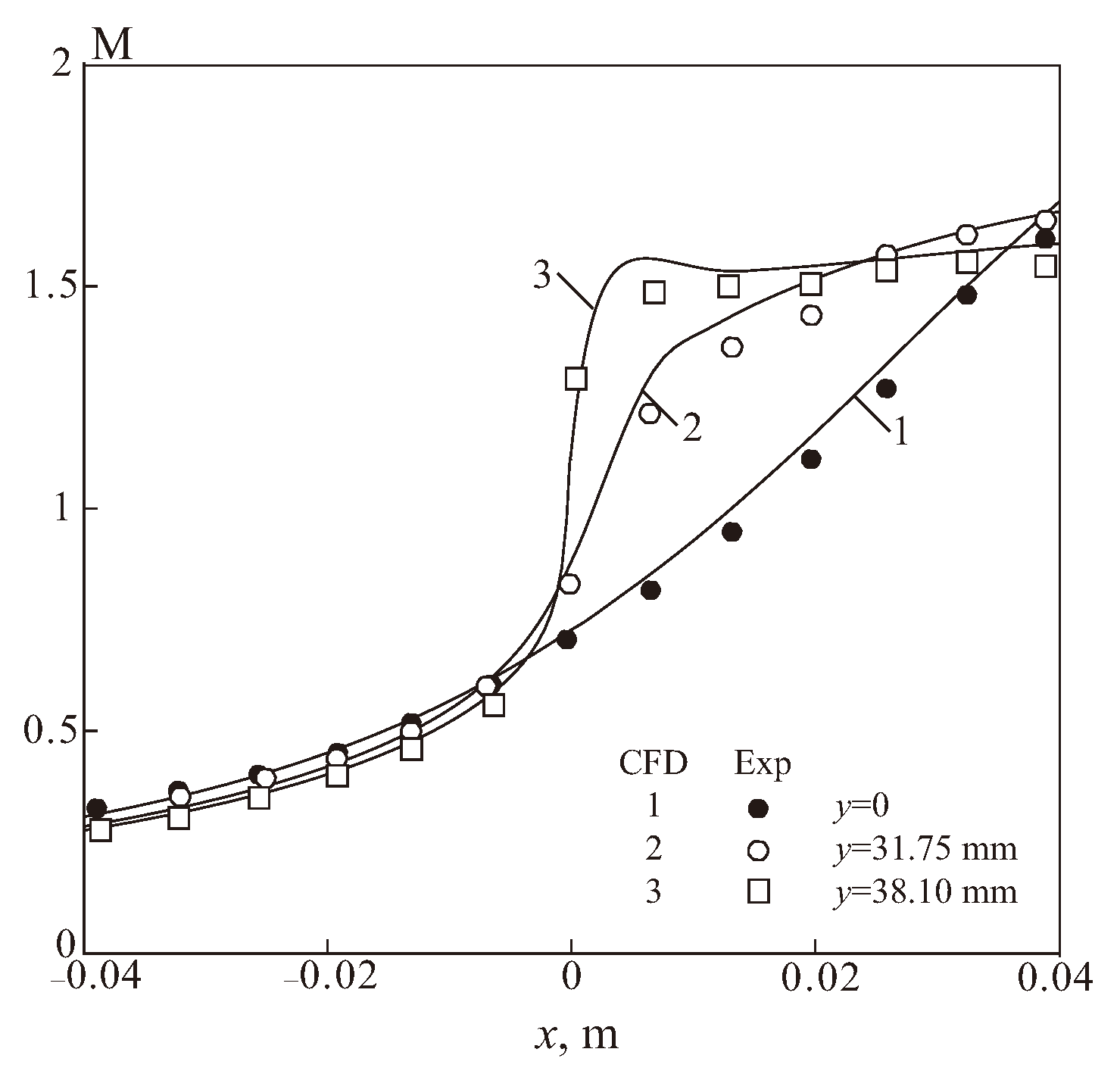
8.2. Local Quantities
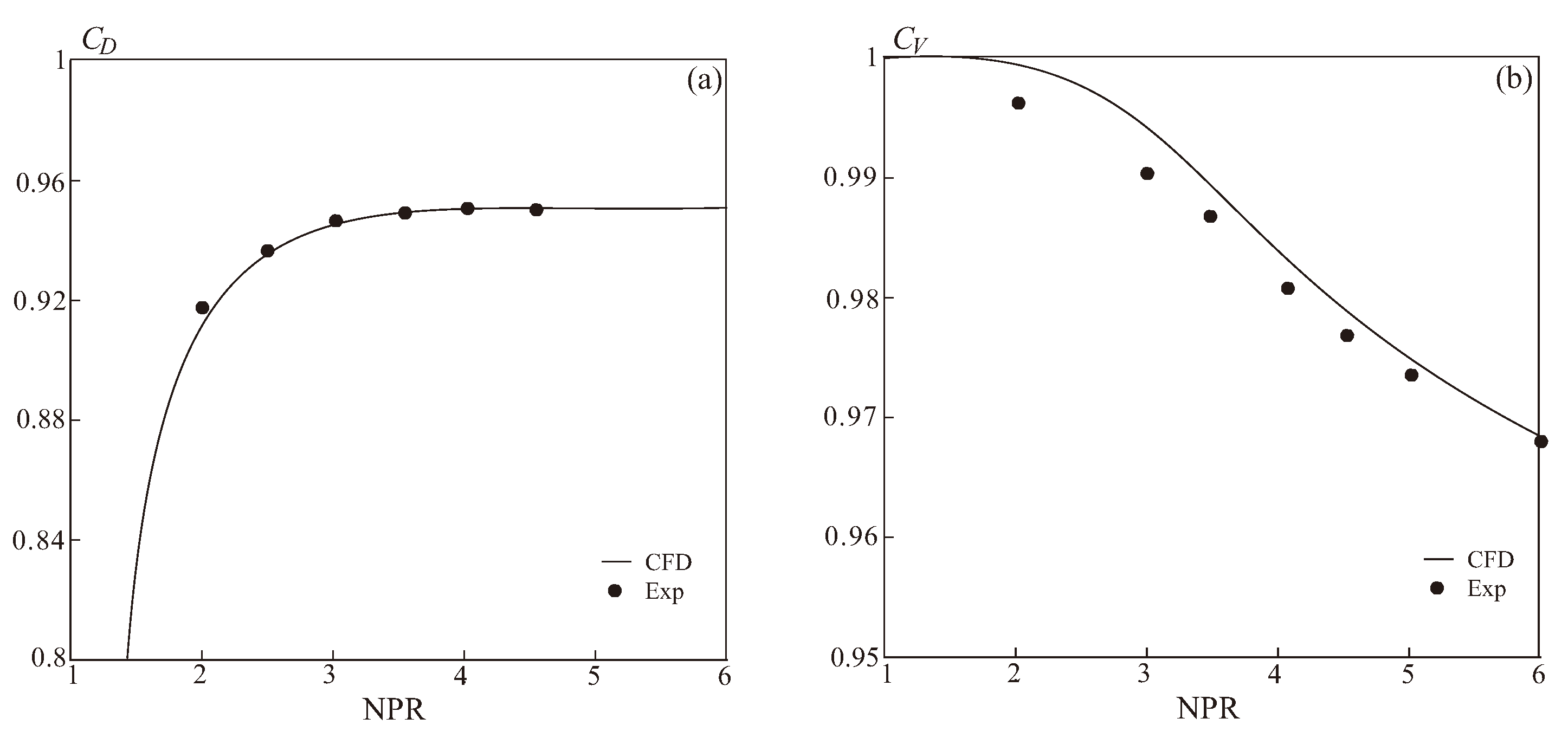
8.3. Discharge and Thrust Coefficients
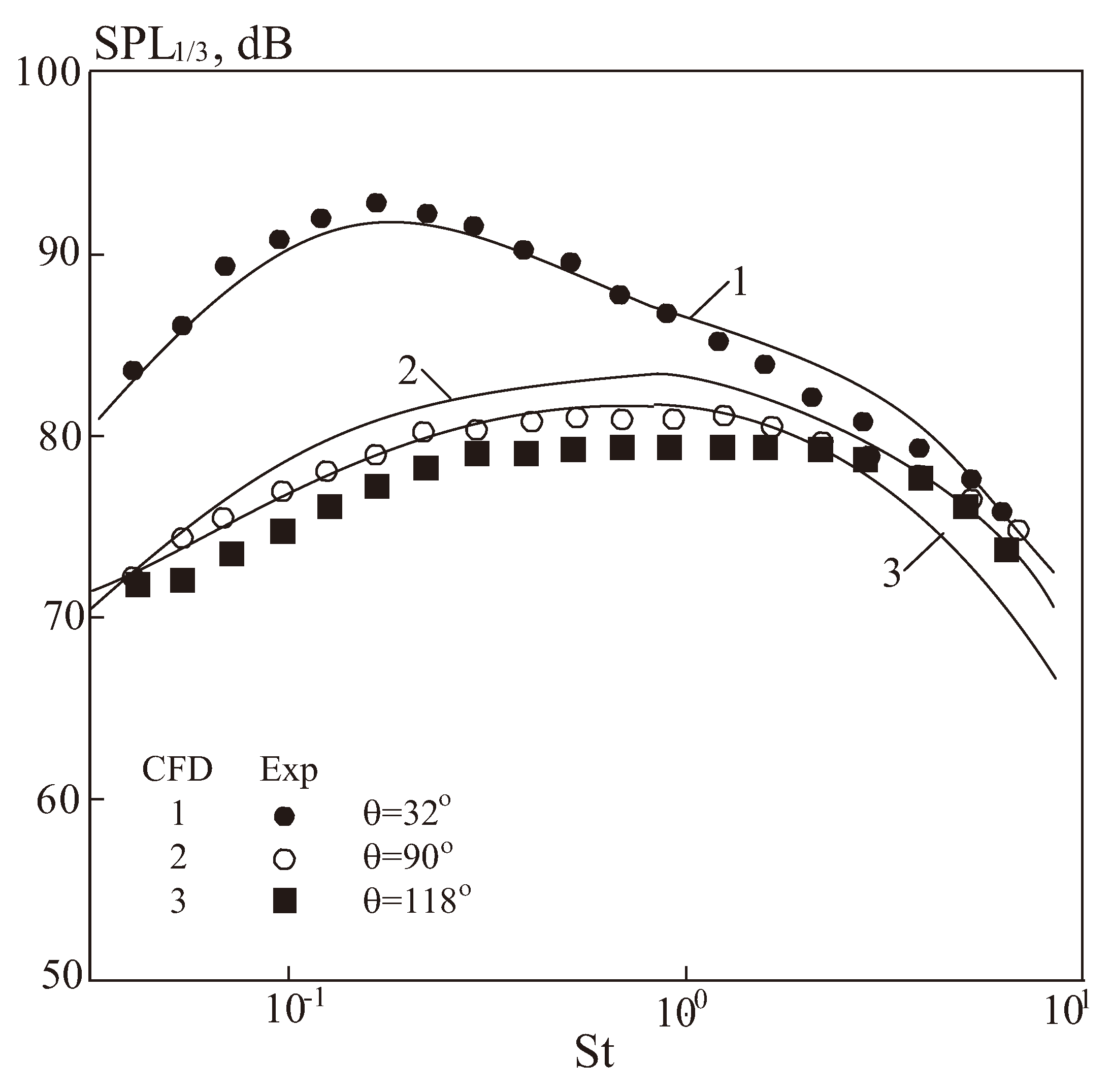
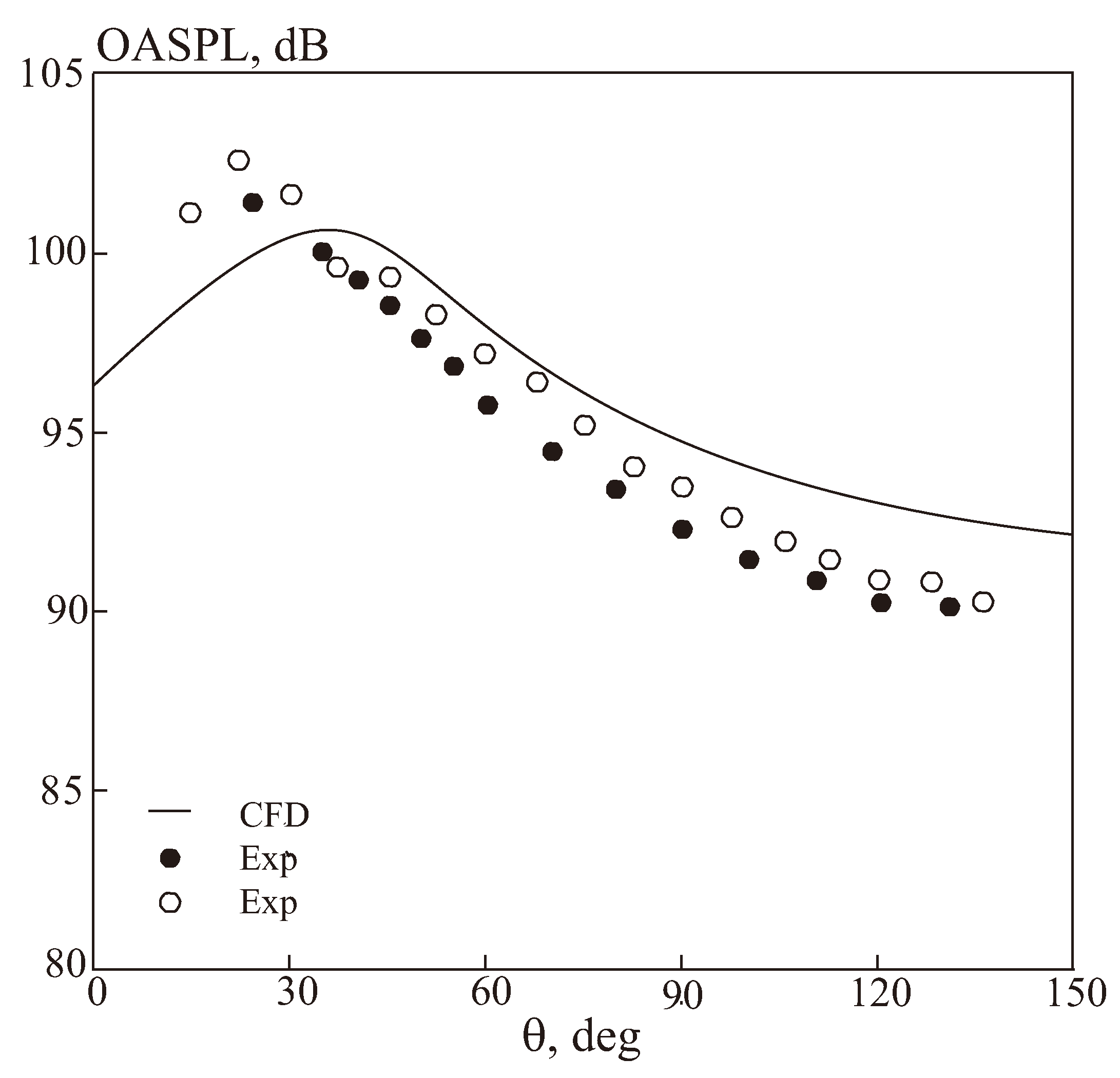
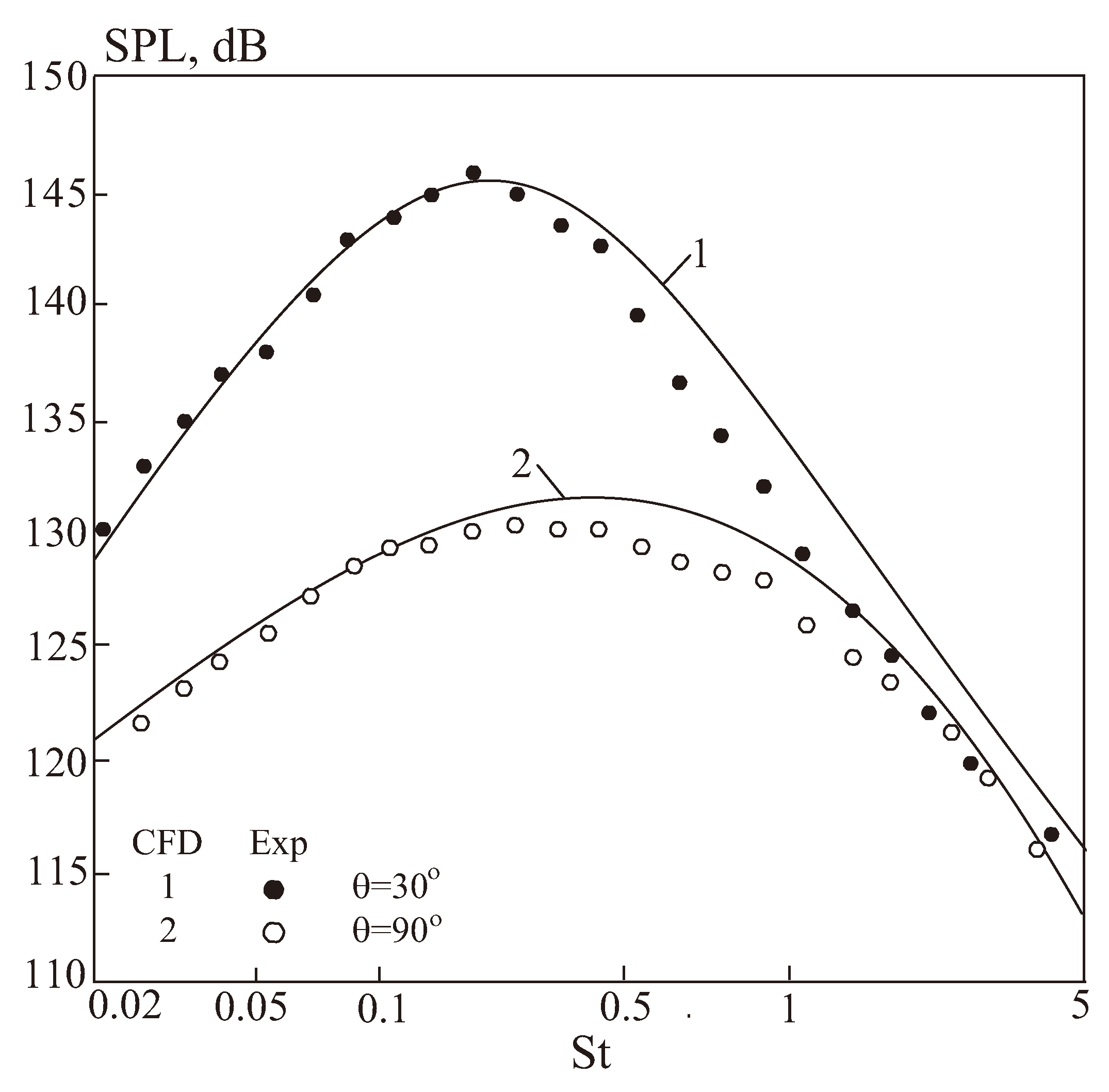
8.4. Noise Generation
9. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Smirnov, N.N. Ensuring safety of space flights. Acta Astronaut. 2017, 135, 1–5. [Google Scholar] [CrossRef]
- Smirnov, N.N. Space flight safety: Past and future. Acta Astronaut. 2022, 194, 353–357. [Google Scholar] [CrossRef]
- Raman, G. Supersonic jet screech: Half-century from Powell to the present. J. Sound Vib. 1999, 225, 543–571. [Google Scholar] [CrossRef]
- Nguyen, D.T.; Maher, B.; Hassan, Y. Effects of nozzle pressure ratio and nozzle-to-plate distance to flowfield characteristics of an under-expanded jet impinging on a flat surface. Aerospace 2019, 6, 4. [Google Scholar] [CrossRef]
- Cui, K.; Liu, H.; Jiang, W.; Yu, D. Thrust noise cause analysis and supression of a cusped field thruster. Acta Astronaut. 2021, 179, 322–329. [Google Scholar] [CrossRef]
- Chiu, H.H.; Sommerfield, M. Theory of combustion noise. Acta Astronaut. 2024, 1, 967–984. [Google Scholar] [CrossRef]
- Chin, C.; Li, M.; Harkin, C.; Rochwerger, T.; Chan, L.; Ooi, A. Investigation of the flow structures in supersonic free and impinging jet flows. J. Fluids Eng. 2013, 135, 031202. [Google Scholar] [CrossRef]
- Zapryagaev, V.; Kiselev, N.; Gubanov, D. Shock-wave structure of supersonic jet flows. Aerospace 2018, 5, 60. [Google Scholar] [CrossRef]
- Boyko, V.M.; Dostovalov, A.V.; Zapryagaev, V.I.; Kavun, I.N.; Kiselev, N.P.; Pivovarov, A.A. Study of the structure of supersonic non-isobaric jets. Sci. Notes TsAGI 2010, 41, 44–57. [Google Scholar]
- Emami, B.; Bussman, M.; Tran, H. Application, realizability and shock unsteadiness to k–ε simulations of under expanded axisymmetric supersonic free jets. J. Fluids Eng. 2010, 132, 041104. [Google Scholar] [CrossRef]
- Nichols, D.S. Accounting for Shocks in the Ke–Kw Turbulence Model. In Proceedings of the 41st AIAA Fluid Dynamics Conference and Exhibit, Honolulu, HI, USA, 27–30 June 2011. [Google Scholar]
- Oh, Y.H.; Bushnell, D.M. Influence of External Disturbances and Compressibility on Free Turbulent Mixing; NASA Report SP-347; NASA: Washington, DC, USA, 1975. [Google Scholar]
- Sarkar, S. Modeling the Pressure-Dilatation Correlation; NASA Report CR-187566; NASA: Washington, DC, USA, 1991. [Google Scholar]
- Thies, A.T.; Tam, C.K.W. Computation of turbulent axisymmetric and nonaxisymmetric jet flows using the k–epsilon model. AIAA J. 1996, 34, 309–316. [Google Scholar] [CrossRef]
- Tam, C.K.W.; Auriault, L. Jet mixing noise from fine-scale turbulence. AIAA J. 1999, 37, 145–153. [Google Scholar] [CrossRef]
- Khavaran, A.; Kenzakowski, D.C.; Mielke Fagan, A.F. Hot jets and sources of jet noise. Int. J. Aeroacoustics 2010, 9, 491–532. [Google Scholar] [CrossRef]
- Alam, M.M.A.; Setoguchi, T.; Matsuo, S.; Kim, H.D. Nozzle geometry variations on the discharge coefficient. Propulsionand Power Res. 2016, 5, 22–33. [Google Scholar] [CrossRef][Green Version]
- Kim, J.-H.; Kearney-Fischer, M.; Samimy, M.; Gogineni, S. Far-field noise control in supersonic jets from conical and contoured nozzles. J. Eng. Gas Turbines Power 2011, 133, 081201. [Google Scholar] [CrossRef]
- Emelyanov, V.N.; Tsvetkov, A.I.; Volkov, K.N. Mechanisms of generation and sources of noise in supersonic jets. J. Akustika 2019, 32, 144–150. [Google Scholar] [CrossRef]
- Ignatius, J.K.; Sankaran, S.; Kumar, A.R.; Satyanarayana, T.N.V.; Chakravarthy, S.R. Suppression of jet noise by staged water injection during launch vehicle lift-off. Int. J. Aeroacoustics 2008, 7, 223–241. [Google Scholar] [CrossRef]
- Sankaran, S.; Ignatius, J.K.; Ramkumar, R.; Satyanarayana, T.N.V.; Chakravarthy, S.R.; Panchapakesan, N.R. Suppression of high mach number rocket jet noise by water injection. J. Spacecr. Rocket 2009, 46, 1089–1099. [Google Scholar] [CrossRef]
- Zoppellari, E.; Juve, D. Reduction of Hot Jet Noise by Water Injection; AIAA Paper 98-2204; AIAA: Reston, VA, USA, 1998. [Google Scholar]
- Kandula, M. Effective Jet Properties for the Estimation of Turbulent Mixing Noise Reduction by Water Injection; AIAA Paper 2007-3645; AIAA: Reston, VA, USA, 2007. [Google Scholar]
- Tam, C. Supersonic jet noise. Annu. Rev. Fluid Mech. 1995, 27, 17–43. [Google Scholar] [CrossRef]
- Ffowcs-Williams, J.E. The noise from turbulence convected at high speed. Philos. Trans. R. Lond. 1963, A255, 469–503. [Google Scholar]
- Phillips, O.M. On the generation of sound by supersonic turbulent shear layers. J. Fluid Mech. 1960, 9, 1–28. [Google Scholar] [CrossRef]
- Tam, C.K.W.; Chen, P.; Seiner, J.M. Relationship between the instability waves and noise of high-speed jets. AIAA J. 1992, 7, 1747–1752. [Google Scholar] [CrossRef]
- Parthasarathy, S.P.; Massier, P.E. Mach wave emission from supersonic jets. AIAA J. 1997, 15, 1462–1468. [Google Scholar] [CrossRef]
- Harper-Bourne, M.; Fisher, M. The noise from shock waves in supersonic jets. AGARD CP 1973, 2, 1–13. [Google Scholar]
- Norum, T.D.; Seiner, J.M. Broadband shock noise from supersonic jets. AIAA J. 1982, 20, 68–73. [Google Scholar] [CrossRef]
- Alkislar, M.B.; Krothapalli, A.M.; Lourenco, L. Structure of a screeching rectangular jet: A stereoscopic particle image velocimetry study. J. Fluid Mech. 2003, 489, 121–154. [Google Scholar] [CrossRef]
- Farr, R.; Chang, C.-L.; Jones, J.H.; Dougherty, N.S. On the Comparison of the Long Penetration Mode (LPM) Supersonic Counterflowing Jet to the Supersonic Screech Jet; AIAA Paper 2015-3126; AIAA: Reston, VA, USA, 2015. [Google Scholar]
- Breitsamter, A.; Schmid, A. Airbrake-induced fin-buffet loads on fighter aircraft. J. Aircr. 2008, 45, 1619–1630. [Google Scholar] [CrossRef]
- Frohlich, J.; von Terzi, D. Hybrid LES/RANS methods for the simulation of turbulent flows. Prog. Aerosp. Sci. 2008, 44, 349–377. [Google Scholar] [CrossRef]
- Farassat, F. Linear acoustic formulae for calculation of rotating blade noise. AIAA J. 1981, 19, 1122–1130. [Google Scholar] [CrossRef]
- Farassat, F. Prediction of advanced propeller noise in the time domain. AIAA J. 1984, 24, 578–584. [Google Scholar] [CrossRef]
- Brentner, K.S.; Farassat, F. An analitical comparision of the acoustics analogy and Kirchhoff formulation for moving surfaces. AIAA J. 1998, 36, 1379–1386. [Google Scholar] [CrossRef]
- Volkov, K.N.; Emelyanov, V.N.; Karpenko, A.G.; Teterina, I.V. Simulating flows of viscous incompressible fluid on graphics processors using the splitting scheme and multigrid method. Comput. Math. Math. Phys. 2019, 59, 136–149. [Google Scholar] [CrossRef]
- Chakravarthy, S.R.; Osher, S. A New Class of High-Accuracy TVD Schemes for Hyperbolic Conservation Laws; AIAA Paper 85-0363; AIAA: Reston, VA, USA, 1985. [Google Scholar]
- Thornock, R.L.; Brown, E.F. An experimental study of compressible flow through convergent-conical nozzles, including a comparison with theoretical results. ASME J. Basic Eng. 1972, 94, 926–932. [Google Scholar] [CrossRef]
- Spotts, N.; Guzik, S.; Gao, X. A CFD Analysis of Compressible Flow through Convergent-Conical Nozzles; AIAA Paper 2013-3734; AIAA: Reston, VA, USA, 2013. [Google Scholar]
- Thornock, R.L.; Sokhey, J.S. Propulsion Aerodynamic Workshop. Comparison of Participant Analyses with Experimental Results for Convergent Conical Nozzle Flowfields and Performance; AIAA Paper 2013-3735; AIAA: Reston, VA, USA, 2013. [Google Scholar]
- Dippold, V.F. Computational Simulations of Convergent Nozzles for the AIAA 1st Propulsion Aerodynamics Workshop; NASA Report TM-2014-218329; NASA: Washington, DC, USA, 2014. [Google Scholar]
- Roache, P.J. Perspective: A method for uniform reporting of grid refinement studies. J. Fluids Eng. 1994, 116, 405–413. [Google Scholar] [CrossRef]
- Viswanathan, K. Aeroacoustics of hot jets. J. Fluid Mech. 2004, 516, 39–82. [Google Scholar] [CrossRef]
- Tanna, H.K. An experimental study of jet noise. Part I. Turbulent mixing noise. J. Sound Vib. 1977, 50, 405–428. [Google Scholar] [CrossRef]
- Bogey, C.; Barre, S.; Fleury, V.; Bailly, C.; Juve, D. Experimental study of the spectral properties of near-field and far-field jet noise. Int. J. Aeroacoustics 2007, 6, 73–92. [Google Scholar] [CrossRef]
- Andre, B.; Castelain, T.; Bailly, C. Broadband shock-associated noise in screeching and non-screeching underexpanded supersonic jets. AIAA J. 2013, 51, 665–673. [Google Scholar] [CrossRef]









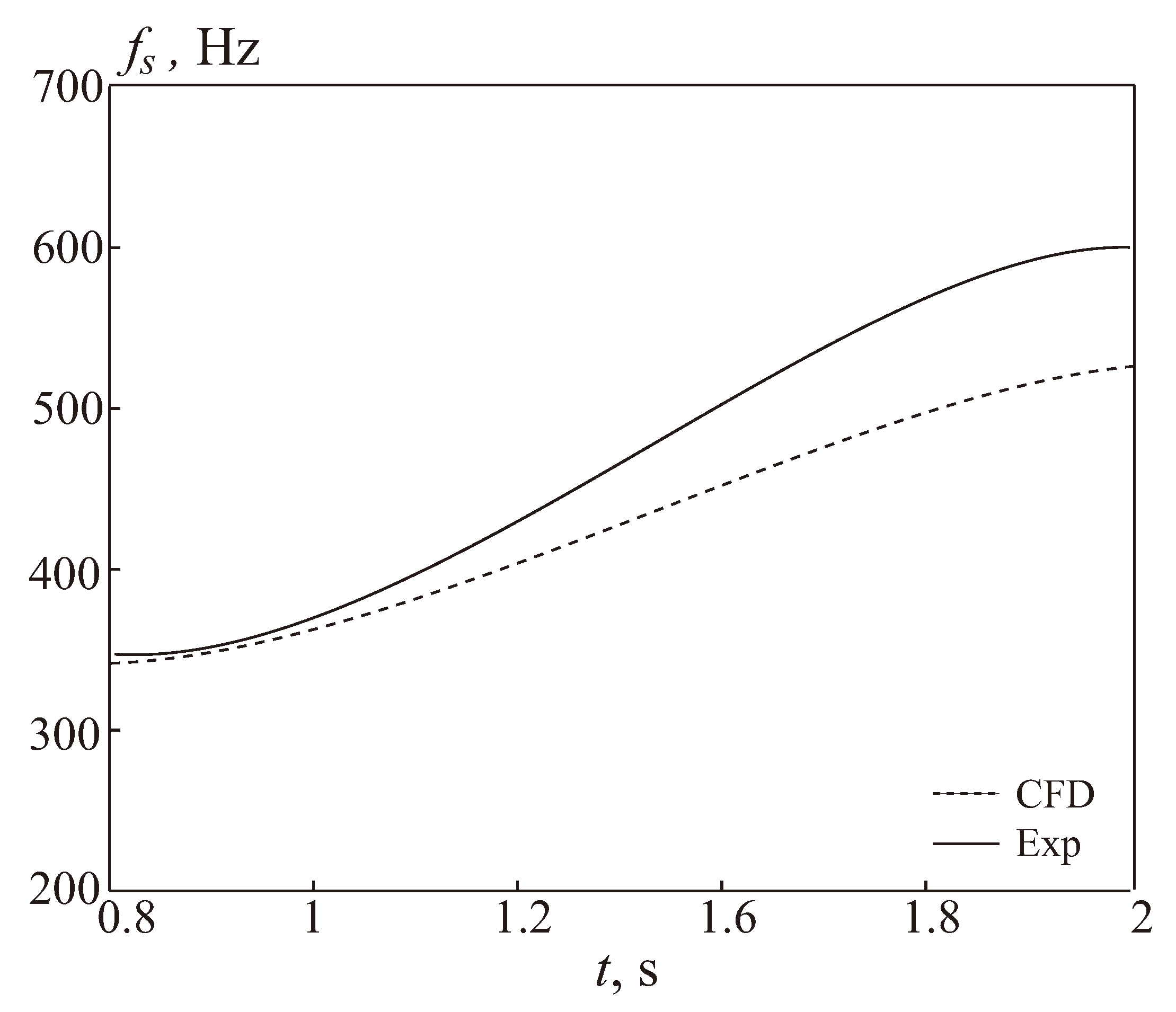
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Volkov, K. Flow and Aeroacoustic Characteristics of Underexpanded Supersonic Jets Exhausting from a Conical Converging Nozzle. Fluids 2024, 9, 148. https://doi.org/10.3390/fluids9070148
Volkov K. Flow and Aeroacoustic Characteristics of Underexpanded Supersonic Jets Exhausting from a Conical Converging Nozzle. Fluids. 2024; 9(7):148. https://doi.org/10.3390/fluids9070148
Chicago/Turabian StyleVolkov, Konstantin. 2024. "Flow and Aeroacoustic Characteristics of Underexpanded Supersonic Jets Exhausting from a Conical Converging Nozzle" Fluids 9, no. 7: 148. https://doi.org/10.3390/fluids9070148
APA StyleVolkov, K. (2024). Flow and Aeroacoustic Characteristics of Underexpanded Supersonic Jets Exhausting from a Conical Converging Nozzle. Fluids, 9(7), 148. https://doi.org/10.3390/fluids9070148





