Modular Multifunctional Composite Structure for CubeSat Applications: Embedded Battery Prototype Thermal Analysis
Abstract
1. Introduction
“The basic design of the satellite is comprised of a cube structure with a stack of circuit boards inside. Each face of the satellite will be covered with solar cells. The center of the satellite will have two rechargeable batteries. The batteries split the functions of the satellite into two parts. One half of the satellite contains the satellite computer, communications electronics, and attitude control system. The other half is available for a payload.”
2. Electrical Model of the Embedded Battery Tile
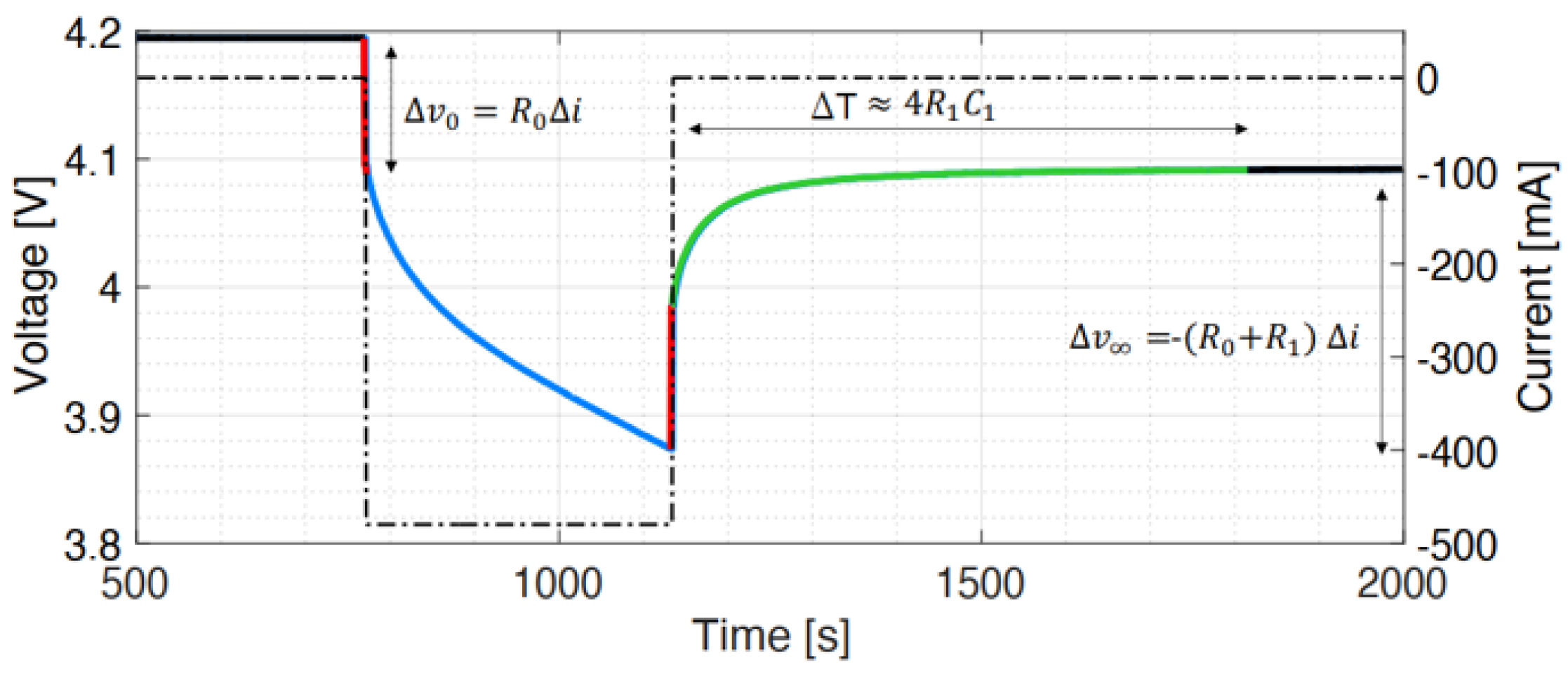
2.1. Randles Circuits
2.2. Hybrid Pulse Power Characterization Tests
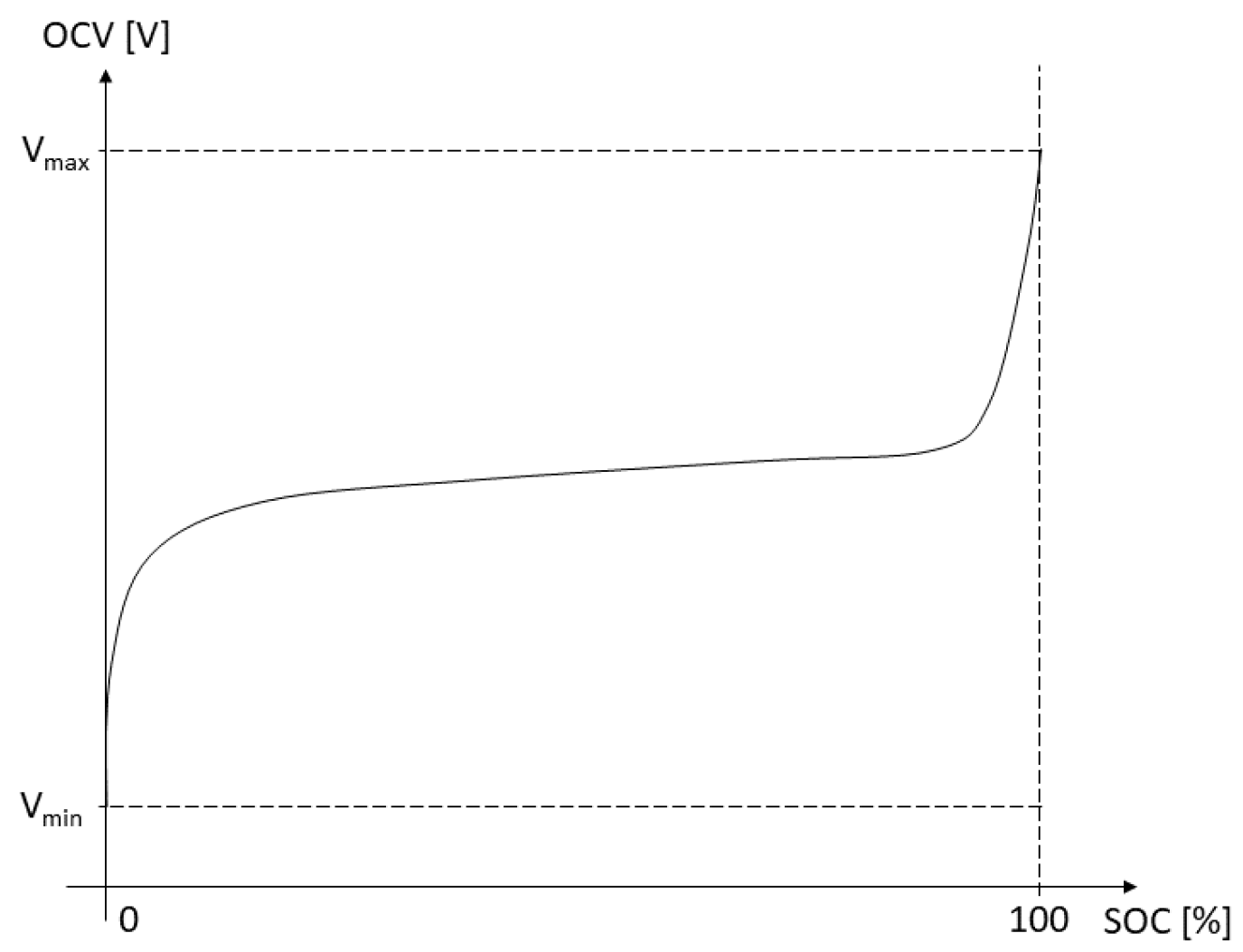
- The procedure starts with a fully charged battery in equilibrium state, i.e., the initial output voltage (Figure 2) is equal to .
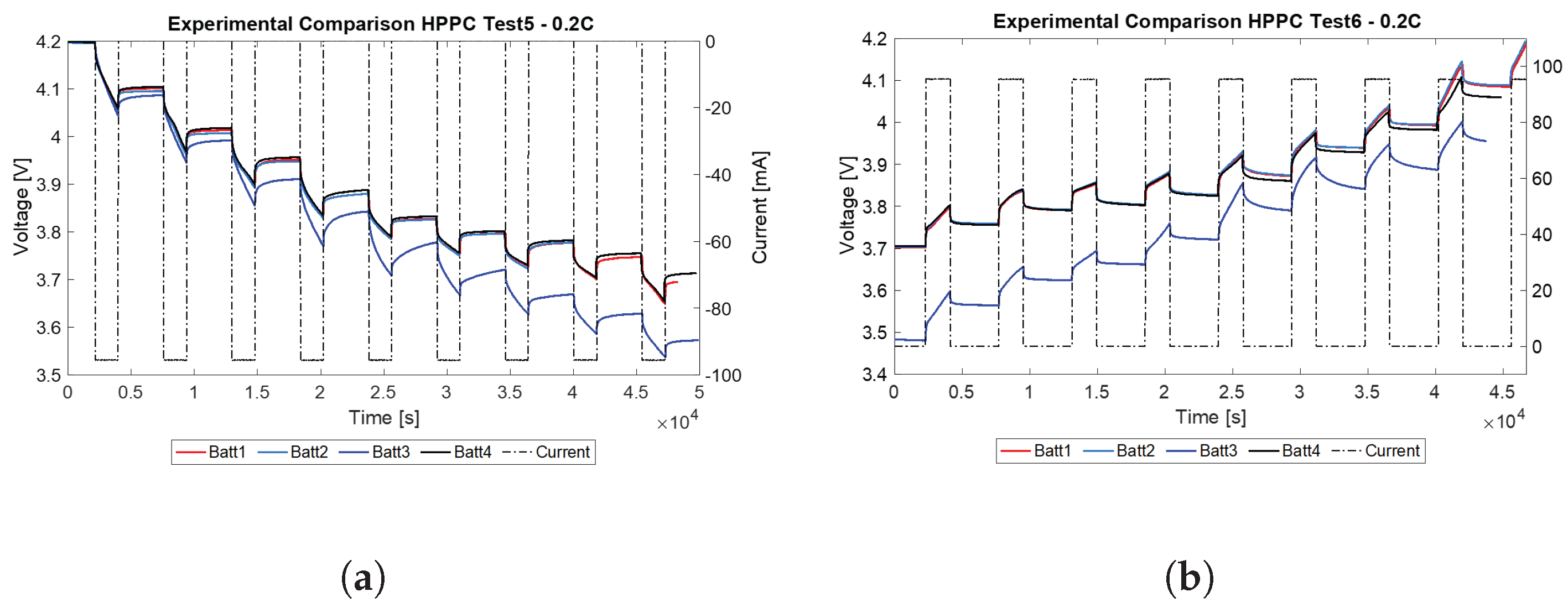
- A preselected value of discharge current (Figure 2) is imposed to the battery terminals, for a preselected period of time. The current time history is represented in Figure 4a with a black dash–point line. The amount of charge removed from the battery can be determined by multiplying the discharge current by its duration; thus, the SOC at the end of the application of the current is known. The output voltages of four batteries are plotted with solid lines in Figure 4a.
- Then, a rest period with zero current is imposed, until the battery reaches again the equilibrium state.
- The process is repeated until the moment the minimum voltage is reached. The test is then interrupted for safety reasons.
- The batteries are initially fully discharged and in equilibrium conditions, i.e., the initial output voltage (Figure 2) is equal to .
- A rest period at zero input current is imposed, until the battery reaches the equilibrium state.
- The process is repeated until the moment the maximum voltage is reached. The test is then interrupted for safety reasons.
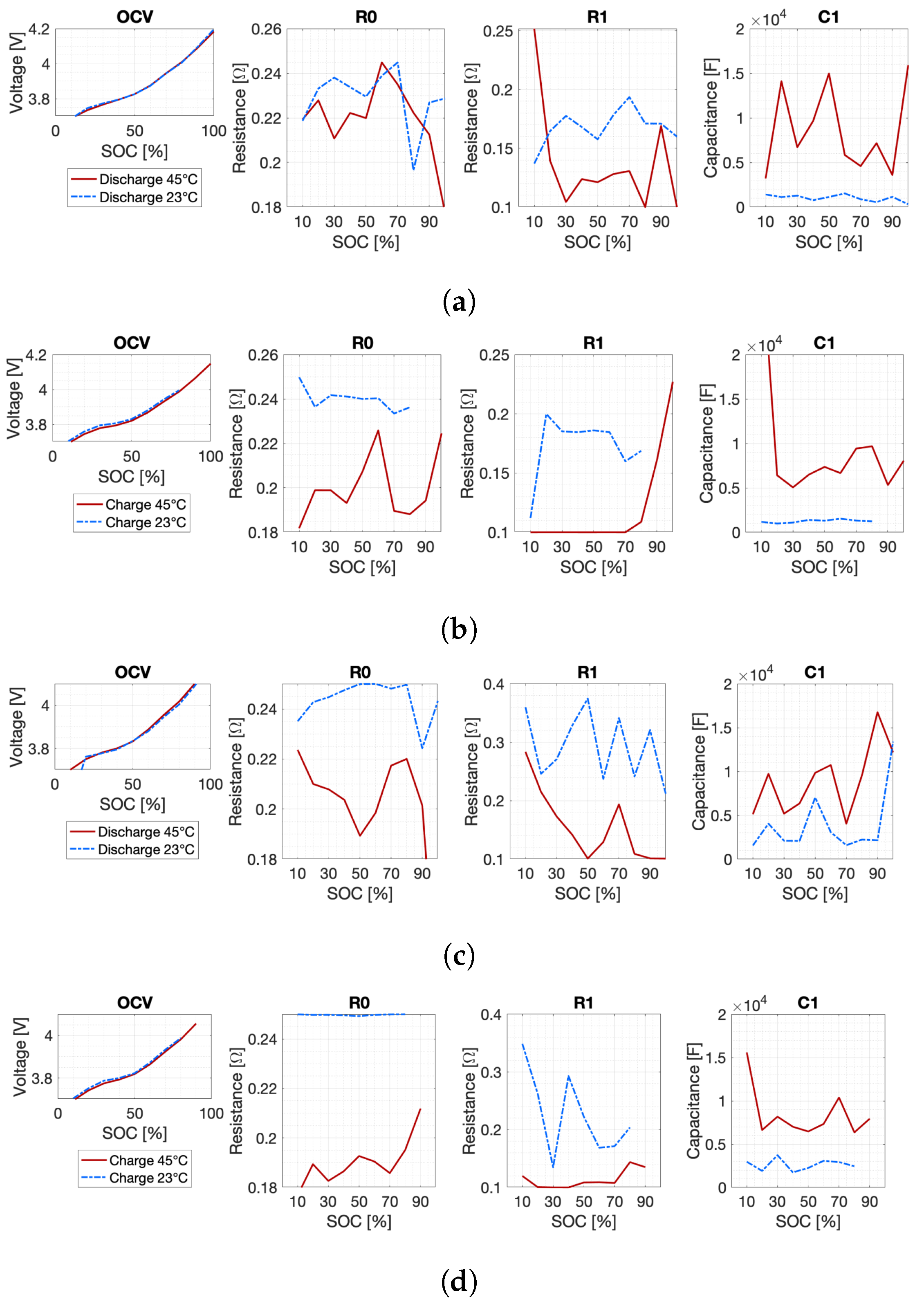
2.3. LiPo Batteries Characterization
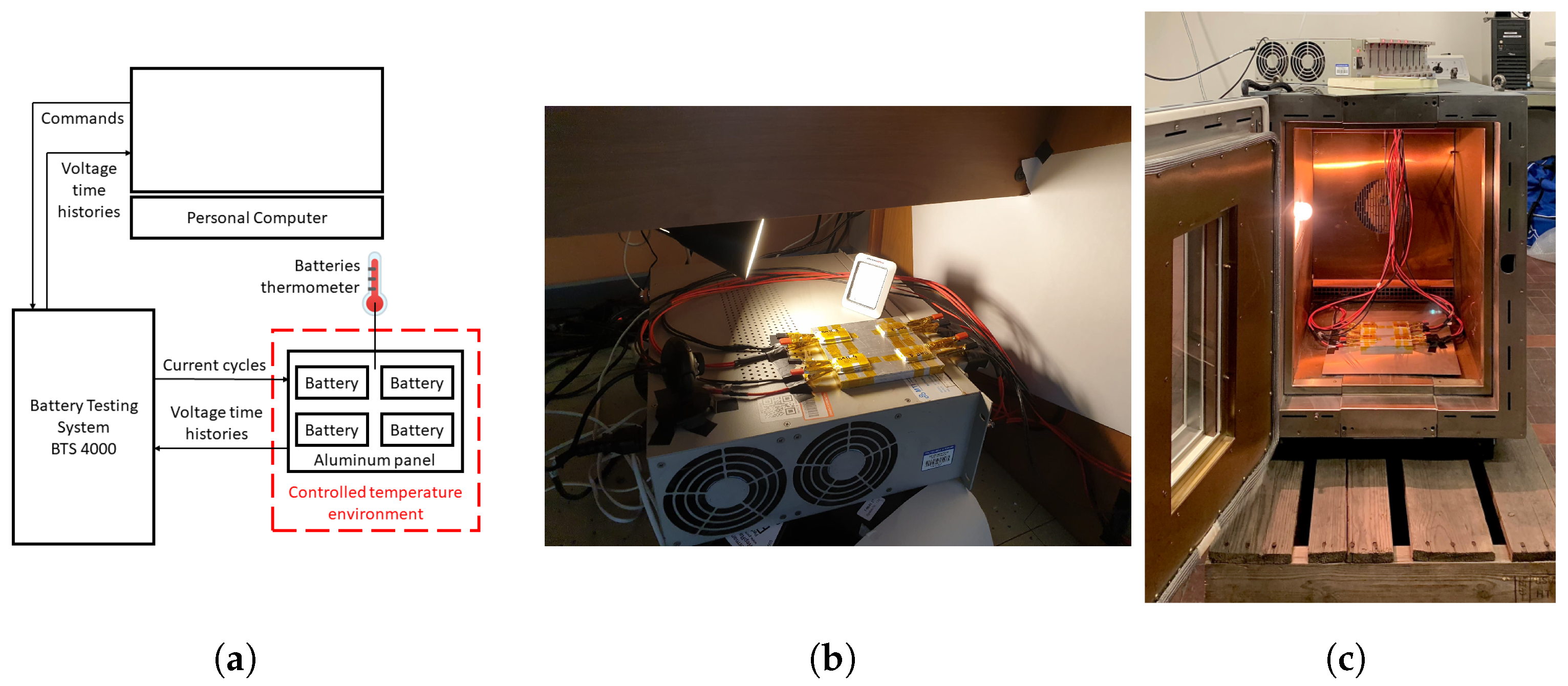
Experimental Setup and HPPC Testing Results
3. Embedded Battery Tile Thermal Analysis
3.1. Orbital Environment
3.2. ARAMIS Thermal Model
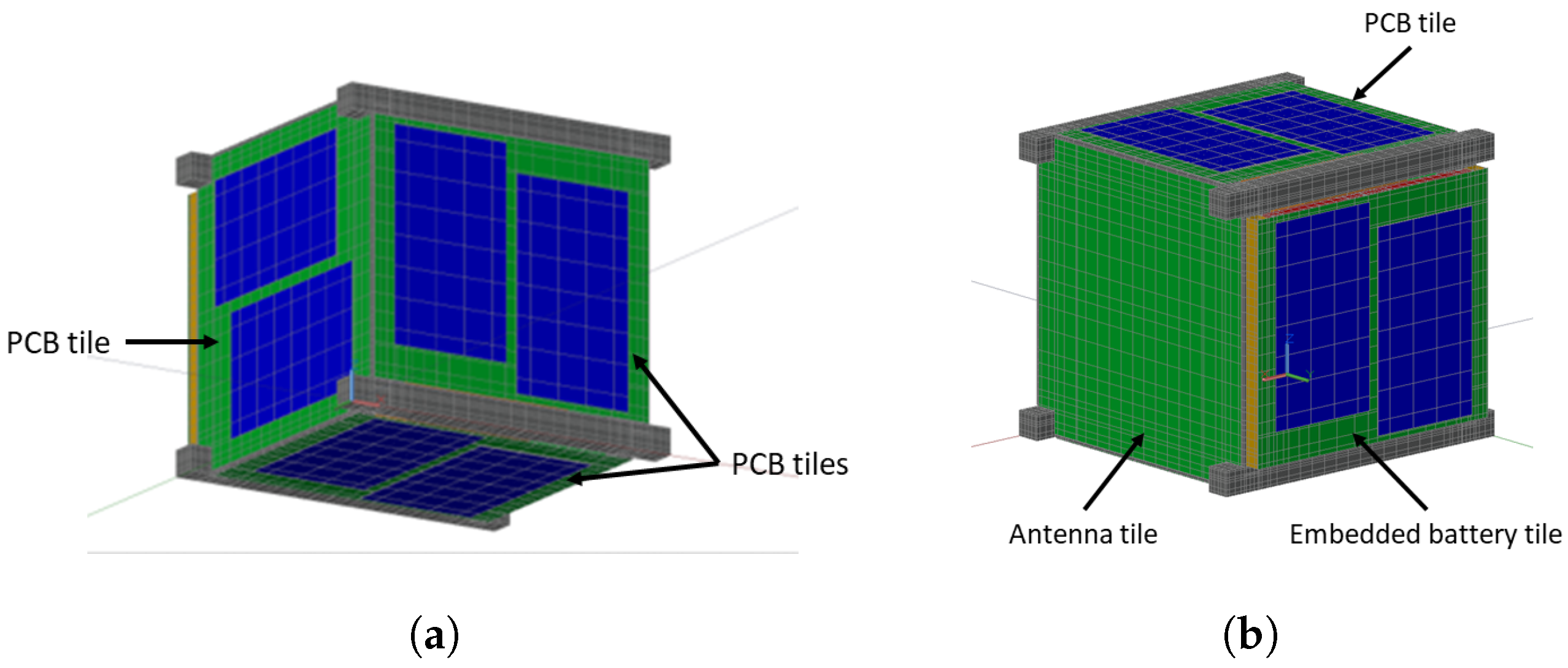
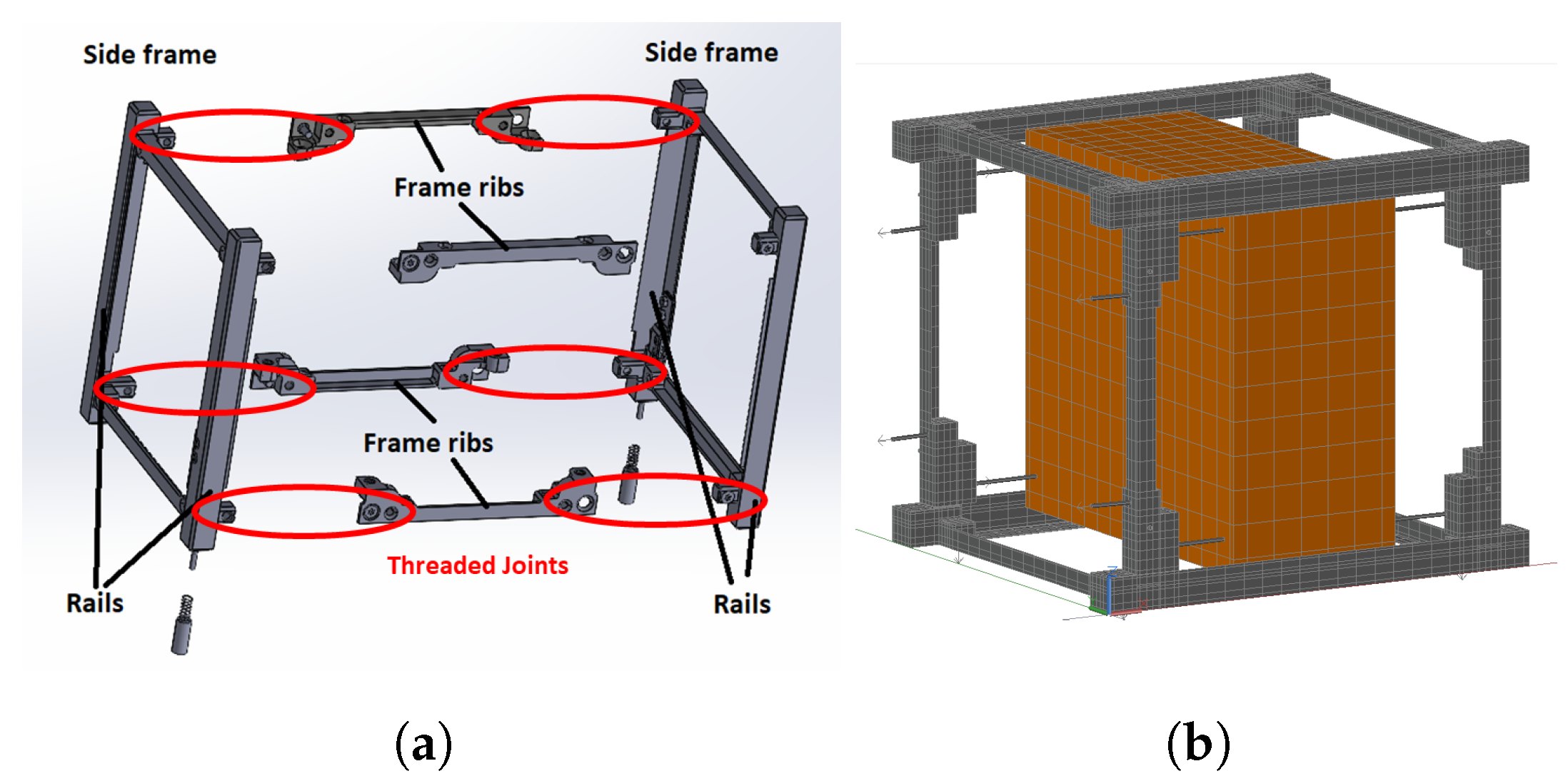
3.2.1. Skeleton
3.2.2. Payload
3.2.3. PCB and Antenna Tiles
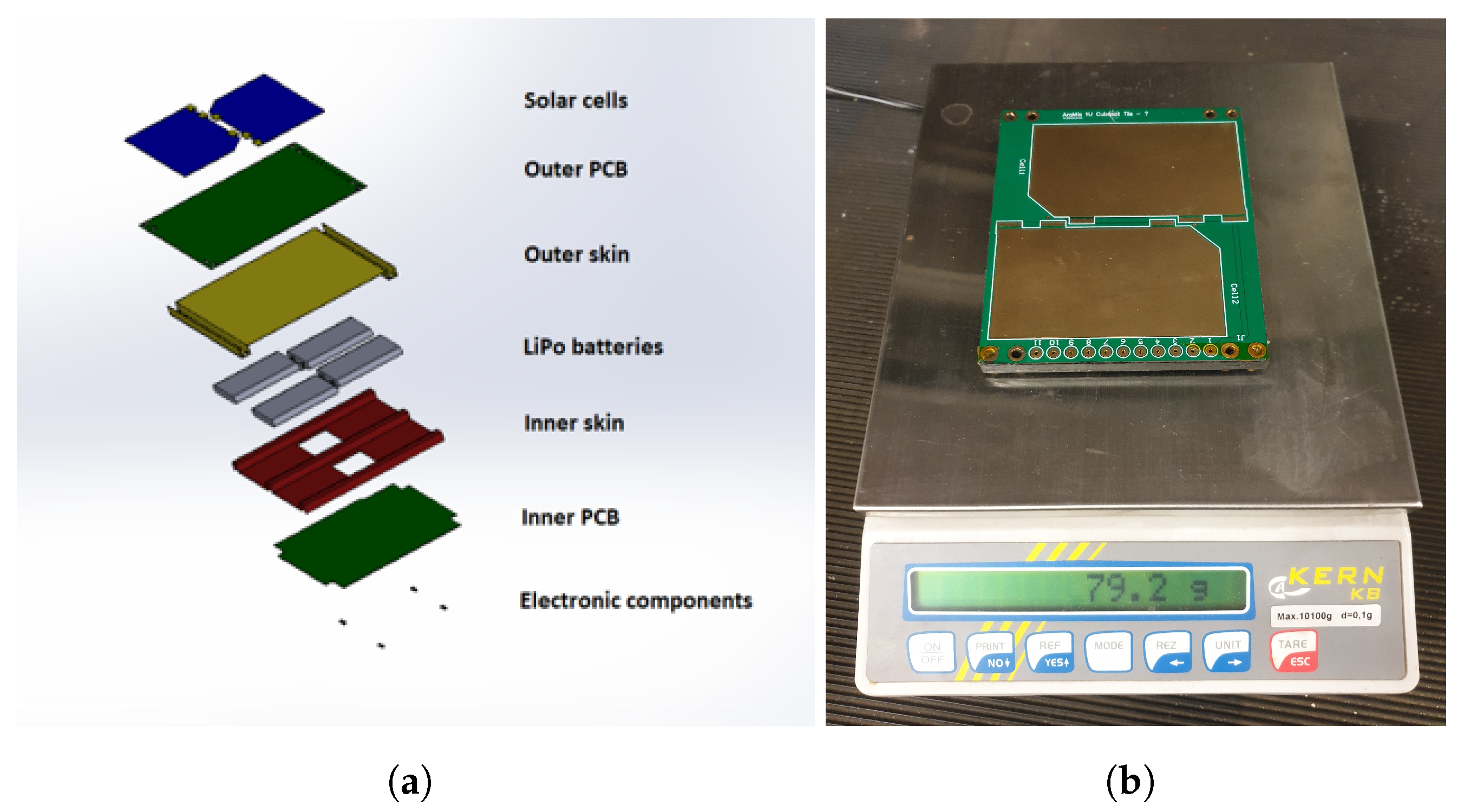
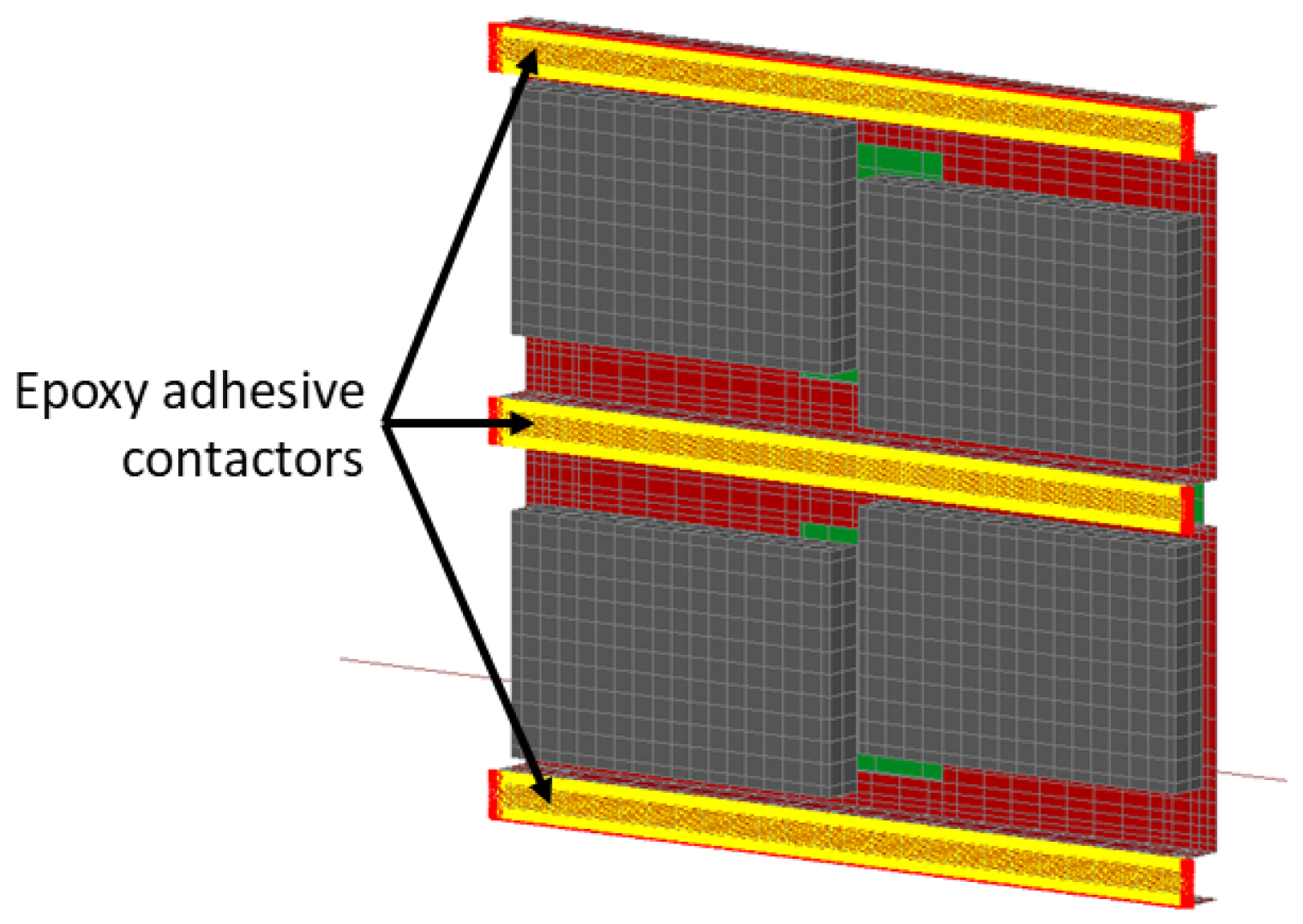
3.2.4. Embedded Battery Tile
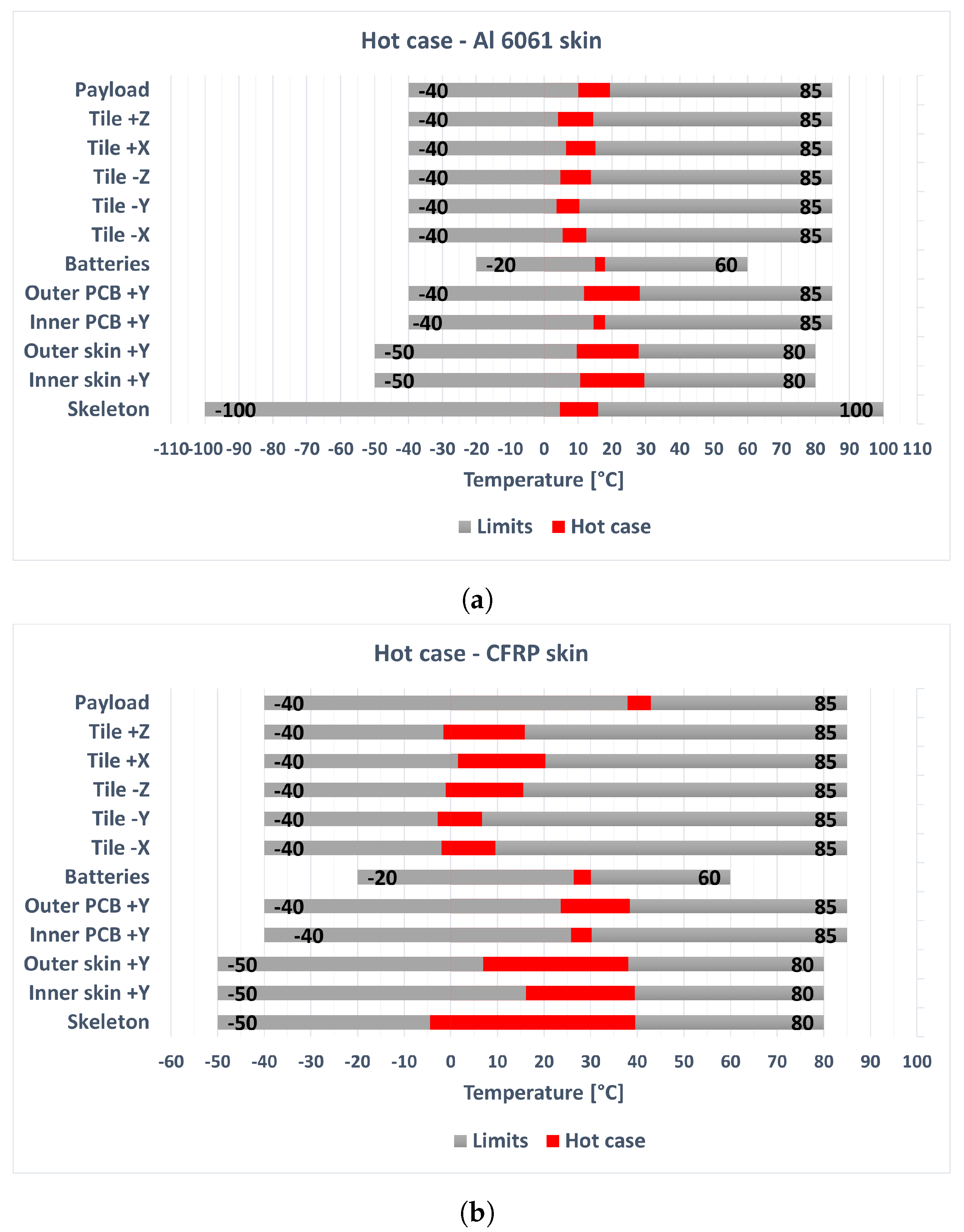
3.3. Analysis and Results
- To acquire a sensitivity about the effect of each parameter on the satellite temperature field (Section 3.3.1);
- To find the combinations of parameters that allow spacecraft components operation within the specified temperature limits (Section 3.3.2).
3.3.1. Parametric Analysis
Orbital Decay
Skeleton
Payload
Embedded Battery Tile Skins
Tiles Top Coating
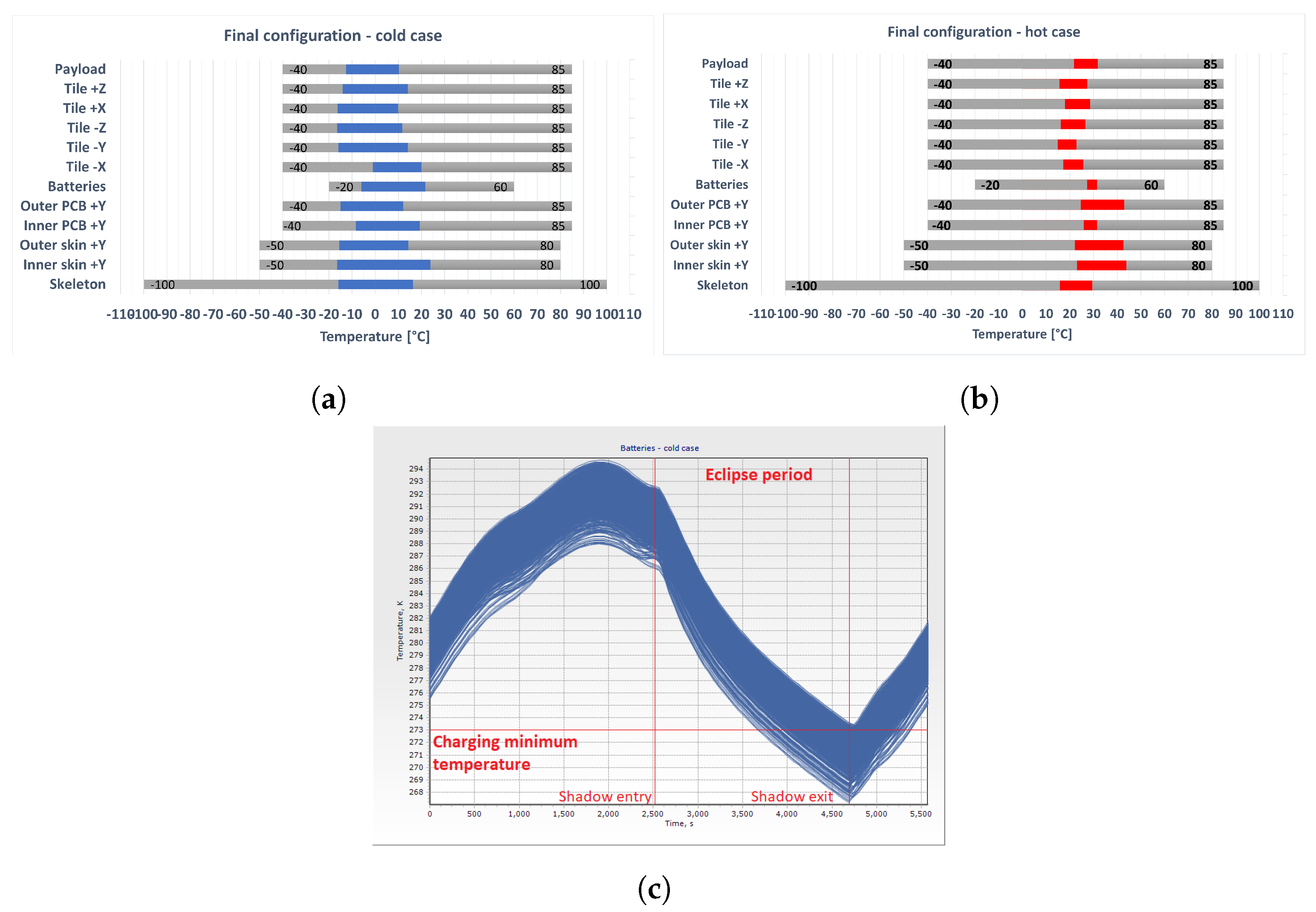
3.3.2. Final Configuration
- Payload: The payload is usually imposed by the mission goals and its thermal properties have an influence on the temperature field which is not negligible but not decisive either (see Section Payload). The thermophysical properties of a 50-50 payload have been chosen, that correspond to a payload made of 50% aluminum 6061 alloy and 50% PCB (Table 5). The thermo-optical properties of the PCB solder mask have been selected (Table 9).
- Skeleton: The aluminum alloy 6061 has been chosen for the skeleton (Table 3), to avoid excessive temperature gradients of the CFRP skeleton (see Section Skeleton).
- CFRP skins for the embedded battery tile: Since the high thermal conductivity CFRP has only local thermal effects and high costs (see Section Embedded Battery Tile Skins), the ordinary CFRP is selected (Table 10).
- Top coat: The tiles’ top coat is of primary importance for the passive thermal control of the whole satellite (see Section Tiles Top Coating). The AZ Technology AMJ-750-LSBU (Table 9) top coat has been chosen to achieve the suitable thermal behavior of the satellite.
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Heidt, H.; Puig-Suari, J.; Moore, A.; Nakasuka, S.; Twiggs, R. CubeSat: A new Generation of Picosatellite for Education and Industry Low-Cost Space Experimentation. In Proceedings of the 14th Annual/USU Conference on Small Satellites, Logan, UT, USA, 21–24 August 2000. [Google Scholar]
- Nanosats Database. Available online: https://www.nanosats.eu/ (accessed on 10 July 2024).
- Areda, E.; Cordova-Alarcon, J.; Masui, H.; Cho, M. Development of Innovative CubeSat Platform for Mass Production. Aerospace 2022, 12, 9087. [Google Scholar] [CrossRef]
- Asp, L.E.; Johansson, M.; Lindbergh, G.; Xu, J.; Zenkert, D. Structural battery composites: A review. Funct. Compos. Struct. 2019, 1, 042001. [Google Scholar] [CrossRef]
- Thomas, J.P.; Qidway, M.A.; Pogue, W.R., III; Rohatgi, A. Multifunctional structure-battery composites for marine systems. J. Compos. Mater. 2013, 47, 5–26. [Google Scholar] [CrossRef]
- Speretta, S.; Reyneri, L.M.; Sansoé, C.; Tranchero, M.; Passerone, C.; Del Corso, D. Modular architecture for satellites. In Proceedings of the 58th International Astronautical Congress, Hyderabad, India, 24–28 September 2007. [Google Scholar]
- Adam, T.J.; Liao, G.; Petersen, J.; Geier, S.; Finke, B.; Wierach, P.; Kwade, A.; Wiedemann, M. Multifunctional composites for future energy storage in aerospace structures. Energies 2018, 11, 335. [Google Scholar] [CrossRef]
- Capovilla, G.; Cestino, E.; Reyneri, L. Modular Multifunctional Composite Structure for CubeSat Applications: Embedded Battery Prototype Modal Analysis. Aerospace 2023, 10, 1009. [Google Scholar] [CrossRef]
- Arianespace. Vega User’s Manual, Issue 4 Revision 0. 2014. Available online: https://newspaceeconomy.ca/wp-content/uploads/2022/06/vega-users-manual_issue-04_april-2014.pdf (accessed on 2 February 2025).
- Arianespace. Ariane 6 User’s Manual, Issue 2 Revision 0. 2021. Available online: https://www.arianespace.com/wp-content/uploads/2021/03/Mua-6_Issue-2_Revision-0_March-2021.pdf (accessed on 5 November 2023).
- SpaceX. Falcon User’s Guide. 2021. Available online: https://www.spacex.com/media/falcon-users-guide-2021-09.pdf (accessed on 5 November 2023).
- Plett, G.L. Battery Modeling, 1st ed.; Volume 1: Battery Management Systems; Artech House: Boston, MA, USA, 2015. [Google Scholar]
- ECE4710/5710: Modeling, Simulation, and Identification of Battery Dynamics Course Notes by G. L. Plett. Available online: http://mocha-java.uccs.edu/ECE5710/index.html (accessed on 9 August 2024).
- Rahmoun, A.; Biechl, H. Modeling of Li-ion batteries using equivalent circuit diagrams. Prz. Elektrotech. 2012, 88, 152–156. [Google Scholar]
- LiPol. LP452540 Battery Datasheet. June 2019. Available online: https://www.lipolbattery.it/ (accessed on 2 February 2025).
- Neware Technology Limited. Battery Testing System User Manual, Version 1.0. 2013. Available online: https://newarebattery.com/wp-content/uploads/2019/05/BTS4000-User-Manual.pdf (accessed on 21 November 2024).
- Bateau-Meyer, S.; L’Eplattenier, P.; Deng, J.; Zhu, M.; Bae, C.; Miller, T. Randles Circuit Parameters Set Up for Battery Simulations in LS-DYNA®. In Proceedings of the 15th International LS-DYNA® Users Conference, Detroit, MI, USA, 10–12 June 2018. [Google Scholar]
- Ortiz Longo, C.R.; Rickman, S.L. Method for the Calculation of Spacecraft Umbra and Penumbra Shadow Terminator Points. NASA Technical Paper 3547, April 1995. Available online: https://ntrs.nasa.gov/citations/19950023025 (accessed on 3 December 2024).
- Capovilla, G. Development of Next Generation Multifunctional Composite Structures for CubeSats, Pico- and Nanosatellites. Ph.D. Thesis, Politecnico di Torino, Torino, Italy, September 2022. [Google Scholar]
- Kearney, M.-A. A Thermal Simulation Tool for CubeSats for Dynamic in-Orbit Scenarios, Verified with Flight Data from the nSight-1 Mission. Master Thesis, Stellenbosch University, Stellenbosch, South Africa, March 2020. Available online: https://scholar.sun.ac.za/bitstream/handle/10019.1/108309/kearney_thermal_2020.pdf?sequence=2&isAllowed=y (accessed on 25 December 2024).
- The CubeSat Program. CubeSat Design Specification Rev. 13. California Polytechnic State University, February 2014, San Luis Obispo. Available online: https://static1.squarespace.com/static/5418c831e4b0fa4ecac1bacd/t/56e9b62337013b6c063a655a/1458157095454/cds_rev13_final2.pdf (accessed on 25 December 2024).
- Çengel, Y.A. Termodinamica e Trasmissione Del Calore, 3rd ed.; McGraw-Hill: Milan, Italy, 2009; ISBN 978-88-38-66514-1. [Google Scholar]
- Joven, R.; Das, R.; Ahmed, A.; Roozbehjavan, P.; Minaie, B. Thermal properties of carbon fiber/epoxy composites with different fabric weaves. In Proceedings of the SAMPE International Symposium Proceedings, Charleston, SC, USA, 22–25 October 2012. [Google Scholar]
- PC/104 Embedded Consortium. PC/104 Specification Version 2.6. Published Online, October 2008. Available online: https://pc104.org/wp-content/uploads/2015/02/PC104_Spec_v2_6.pdf (accessed on 25 December 2022).
- Azar, K.; Graebner, J.E. Experimental Determination of Thermal Conductivity of Printed Wiring Boards. In Proceedings of the Twelfth IEEE SEMI-THERM Symposium, Austin, TX, USA, 5–7 March 1996; pp. 169–182. [Google Scholar] [CrossRef]
- Northwest Engineering. FR4 Thermal Properties to Consider During Design. Available online: https://www.nwengineeringllc.com/article/fr4-thermal-properties-to-consider-during-design.php (accessed on 2 February 2025).
- Rogers Corporation. RO3000 Series Circuit Materials. Available online: https://www.rogerscorp.cn/-/media/project/rogerscorp/documents/advanced-electronics-solutions/english/data-sheets/ro3000-laminate-data-sheet-ro3003----ro3006----ro3010----ro3035.pdf (accessed on 2 February 2025).
- Anvari, A.; Farhani, F.; Niaki, K.S. Comparative Study on Space Qualified Paints Used for Thermal Control of a Small Satellite. Iran. J. Chem. Eng. 2009, 6, 50–62. [Google Scholar]
- AZ Technology. Summary of AZ Technology Paints. Available online: https://www.aztechnology.com/products/paints.html (accessed on 2 February 2025).
- Campanudo Carvalhais, D. Thermal Modelling and Experiments for Small Satellites. Master’s Thesis, Universidade de Beira Interior, Covilhã, Portugal, December 2019. [Google Scholar]
- Rodrı’guez Gallegos, C.D.; Alvarez Alvarado, M.S. Analysis of the Stationary and Transient Behavior of a Photovoltaic Solar Array: Modeling and Simulation. Int. J. Comput. Appl. Technol. 2015, 127, 26–33. [Google Scholar]
- Redmond, M.; Mastropietro, A.J. Thermophysical and Optical Properties of Materials Considered for use on the LDSD Test Vehicle. In Proceedings of the 45th International Conference on Environmental Systems ICES-2015-24, Bellevue, WA, USA, 12–16 July 2015. [Google Scholar]
- Maleki, H.; Wang, H.; Porter, W.; Hallmark, J. Li-Ion polymer cells thermal property changes as a function of cycle-life. J. Power Sources 2014, 263, 223–230. [Google Scholar] [CrossRef]
- Mathewson, S. Experimental Measurements of LiFePO4 Battery Thermal Characteristics. Master’s Thesis, University of Waterloo, Waterloo, ON, Canada, 2014. [Google Scholar]
- Janson, S.W.; Welle, R.P.; Rose, T.S.; Rowen, D.W.; Hinkley, D.A.; Hardy, B.S.; La Lumondiere, S.D.; Maul, G.A.; Werner, N.I. The NASA Optical Communication and Sensors Demonstration program: Preflight up-date. In Proceedings of the 29th AIAA Conference on Small Satellites, Logan, UT, USA, 8–13 August 2015. [Google Scholar]
- Ali, A.; Ullah, K.; Ur Rehman, H.; Bari, I.; Reyneri, L.M. Thermal characterisation analysis and modelling techniques for CubeSat-sized spacecrafts. Aeronaut. J. 2017, 121, 1858–1878. [Google Scholar] [CrossRef]
- Correa, G.; Santarelli, M.; Borello, F.; Cestino, E.; Romeo, G. Flight test validation of the dynamic model of a fuel cell system for ultra-light aircraft. Proc. Inst. Mech. Eng. G J. Aerosp. Eng. 2014, 229, 917–932. [Google Scholar] [CrossRef]

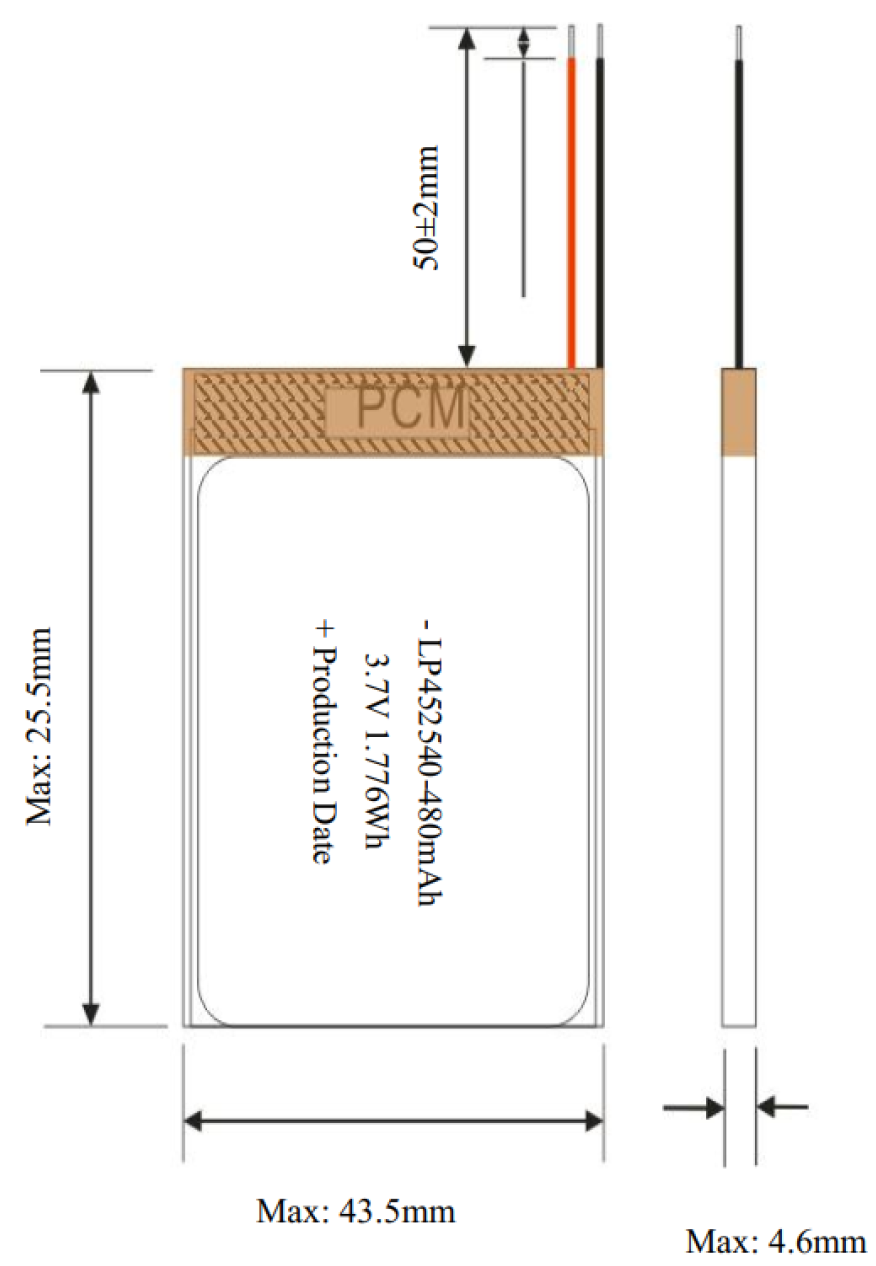






| Typical capacity | 480 | mAh |
| Charging cut-off voltage | 4.2 | V |
| Discharging cut-off voltage | 3.0 | V |
| Standard charging | 96 (0.2C) | mA |
| Max charging | 480 (1.0C) | mA |
| Standard discharging | 96 (0.2C) | mA |
| Max discharging | 480 (1.0C) | mA |
| Charging temperature limits | 0∼45 | °C |
| Discharging temperature limits | −20∼60 | °C |
| Reference temperature interval | 23 ± 2 | °C |
| Step [-] | i [mA] | t [min] | Δz [mAh] |
|---|---|---|---|
| 0.2C discharge | |||
| Constant current discharge | 96 | 30 | 48 |
| Rest | 0 | 60 | 0 |
| 0.2C charge | |||
| Constant current charge | −96 | 30 | −48 |
| Rest | 0 | 60 | 0 |
| 1C discharge | |||
| Constant current discharge | 480 | 6 | 48 |
| Rest | 0 | 60 | 0 |
| 1C charge | |||
| Constant current charge | −480 | 6 | −48 |
| Rest | 0 | 60 | 0 |
| Property | Unit | Value | Reference |
|---|---|---|---|
| Aluminum 6061 thermophysical properties | |||
| Conductivity | W/m/K | 167 | Cullimore and Ring (C&R) |
| Specific heat | J/kg/K | 896 | C&R |
| Density | kg/m3 | 2700 | C&R |
| Bare aluminum thermo-optical properties | |||
| Solar absorptivity | - | 0.09 | [22], pp. A40–A42 |
| IR emissivity | - | 0.05 | [22], pp. A40–A42 |
| Hard-anodized aluminum thermo-optical properties | |||
| Solar absorptivity | - | 0.78 | C&R |
| IR emissivity | - | 0.84 | C&R |
| Combination Number [-] | Aluminum 6061 Component [%] | PCB Component [%] |
|---|---|---|
| 1 | 20 | 80 |
| 2 | 50 | 50 |
| 3 | 80 | 20 |
| Combination Number | Thermal Conductivity, in-Plane | Thermal Conductivity, Through-Plane | Specific Heat | Density |
|---|---|---|---|---|
| [-] | [W/m/K] | [W/m/K] | [J/kg/K] | [kg/m3] |
| 1 | 73.83 | 0.40 | 821.81 | 2696.58 |
| 2 | 108.77 | 0.64 | 849.65 | 2697.86 |
| 3 | 143.71 | 1.60 | 877.47 | 2699.14 |
| PCB Lamination | ||
|---|---|---|
|
Layer Number | Material |
Thickness [μm] |
| 1 | Top coat | 25 |
| 2 | Cu | 35 |
| 3 | FR4 | 230 |
| 4 | Cu | 18 |
| 5 | FR4 | 230 |
| 6 | Cu | 18 |
| 7 | FR4 | 230 |
| 8 | Cu | 35 |
| 9 | Solder mask | 25 |
| Antenna PCB Lamination | ||
|---|---|---|
|
Layer Number | Material |
Thickness [μm] |
| 1 | Top coat | 25 |
| 2 | Cu | 35 |
| 3 | Rogers | 230 |
| 4 | Cu | 18 |
| 5 | Rogers | 230 |
| 6 | Cu | 18 |
| 7 | Rogers | 230 |
| 8 | Cu | 35 |
| 9 | Solder mask | 25 |
| Property | Unit | Value | Reference |
|---|---|---|---|
| FR4 thermophysical properties | |||
| Conductivity, in-plane | W/m/K | 0.81 | [25] |
| Conductivity, through-plane | W/m/K | 0.29 | [25] |
| Specific heat | J/kg/K | 1100 | [26] |
| Density | kg/m3 | 1800 | Own experimental data [8] |
| Rogers thermophysical properties (RO3006) | |||
| Conductivity | W/m/K | 0.79 | [27] |
| Specific heat | J/kg/K | 860 | [27] |
| Density | kg/m3 | 2600 | [27] |
| PCB Thermo-Optical Properties | |||
|---|---|---|---|
| Property | Unit | Value | Reference |
| AZ Technology AZW-LAII (lower solar absorptivity) | |||
| Solar absorptivity | - | 0.10 | ([28], Table 3) |
| IR emissivity | - | 0.91 | ([28], Table 3) |
| Chemglaze A276 (intermediate solar absorptivity) | |||
| Solar absorptivity | - | 0.35 | ([28], Table 1) |
| IR emissivity | - | 0.88 | ([28], Table 1) |
| AZ Technology AMJ-750-LSBU (higher solar absorptivity) | |||
| Solar absorptivity | - | 0.76 | [29] |
| IR emissivity | - | 0.81 | [29] |
| PCB solder mask (inner face) | |||
| Solar absorptivity | - | 0.80 | ([30], p. 53) |
| IR emissivity | - | 0.89 | ([30], p. 53) |
| CFRP Thermophysical Properties | |||
|---|---|---|---|
| Property | Unit | Value | Reference |
| Conductivity, in-plane | W/m/K | 3.3 | ([23], Figure 8) |
| Conductivity, through-plane | W/m/K | 0.6 | ([23], Figure 8) |
| Specific heat | J/kg/K | 900 | ([23], Figure 6) |
| Density | kg/m3 | 1540 | Own experimental data [8] |
| CFRP thermo-optical properties | |||
| Solar absorptivity | - | 0.91 | ([32], Table 1) |
| IR emissivity | - | 0.83 | ([32], Table 1) |
| Battery Properties | |||
|---|---|---|---|
| Property | Unit | Value | Reference |
| Conductivity, in-plane | W/m/K | 32.52 | ([33], p. 225) |
| Conductivity, through-plane | W/m/K | 1.19 | ([33], p. 225) |
| Specific heat | J/kg/K | 986 | ([33], p. 225) |
| Density | kg/m3 | 2500 | Own experimental data [8] |
| Battery thermo-optical properties | |||
| Solar absorptivity | - | 0.86 | ([34], p. 94) |
| IR emissivity | - | 0.86 | ([34], p. 94) |
| Current | Thermal Power | Working Point | |
|---|---|---|---|
| C Rating | [mA] | [mW] | |
| 0.2 | 96.0 | 2.3 | Standard charging and standard discharging |
| 0.5 | 240.0 | 14.4 | - |
| 1.0 | 480.0 | 57.6 | Maximum charging and maximum discharging |
| Component | Temperature Differences (EOL-Nominal) [°C] Cold Case | Temperature Differences (EOL-Nominal) [°C] Hot Case | ||
|---|---|---|---|---|
| Minimum | Maximum | Minimum | Maximum | |
| Skeleton | 3.8 | 4.9 | −12.8 | −3.5 |
| Inner Skin | 4.0 | 5.0 | −18.7 | −2.0 |
| Outer Skin | 4.0 | 5.1 | −17.4 | −1.7 |
| Inner PCB | 3.8 | 4.9 | −17.0 | −2.8 |
| Outer PCB | 4.0 | 4.9 | −19.4 | −1.7 |
| Batteries | 3.8 | 4.9 | −16.5 | −2.8 |
| PCB tile −X | 3.8 | 4.9 | −11.7 | −1.3 |
| PCB tile −Y | 3.9 | 5.0 | −12.3 | −2.3 |
| PCB tile −Z | 4.1 | 5.1 | −13.4 | −3.8 |
| Antenna tile +X | 4.1 | 4.9 | −15.6 | −3.9 |
| PCB tile +Z | 3.9 | 4.8 | −13.0 | −3.2 |
| Payload | 3.8 | 4.7 | −12.1 | −3.5 |
| Component | Temperature Differences (High K − Ordinary K) [°C] Cold Case | Temperature Differences (High K − Ordinary K) [°C] Hot Case | ||
|---|---|---|---|---|
| Minimum | Maximum | Minimum | Maximum | |
| Skeleton | −0.1 | −0.4 | 1.5 | 0.9 |
| Inner Skin | 2.6 | −14.1 | 2.2 | −10.2 |
| Outer Skin | 1.7 | −2.8 | 2.6 | −8.3 |
| Inner PCB | −0.4 | −0.2 | 1.6 | 1.5 |
| Outer PCB | 1.7 | −0.4 | 0.9 | −8.3 |
| Batteries | −0.2 | −0.4 | 1.9 | 1.4 |
| PCB tile −X | −0.1 | −0.4 | 1.7 | 1.9 |
| PCB tile −Y | −0.2 | −0.2 | 1.5 | 1.4 |
| PCB tile −Z | −0.2 | −0.3 | 1.5 | 1.3 |
| Antenna tile +X | −0.3 | −0.3 | 1.4 | 1.2 |
| PCB tile +Z | −0.2 | −0.3 | 1.6 | 1.3 |
| Payload | −0.1 | −0.4 | 1.7 | 1.5 |
| Component | Temperature Differences (Low − High ) [°C] Cold Case | Temperature Differences (Low − High ) [°C] Hot Case | ||
|---|---|---|---|---|
| Minimum | Maximum | Minimum | Maximum | |
| Skeleton | −5.6 | −9.1 | −3.8 | −4.9 |
| Inner Skin | −5.3 | −7.8 | −4.3 | −6.0 |
| Outer Skin | −5.4 | −8.6 | −4.3 | −6.2 |
| Inner PCB | −5.8 | −8.6 | −4.7 | −5.1 |
| Outer PCB | −5.4 | −9.0 | −4.5 | −6.2 |
| Batteries | −5.8 | −8.5 | −4.8 | −5.1 |
| PCB tile −X | −5.6 | −9.4 | −3.9 | −4.6 |
| PCB tile −Y | −5.6 | −8.8 | −3.7 | −4.3 |
| PCB tile −Z | −5.6 | −7.9 | −3.8 | −4.5 |
| Antenna tile +X | −5.6 | −9.2 | −3.8 | −4.8 |
| PCB tile +Z | −5.6 | −9.4 | −3.8 | −4.7 |
| Payload | −6.0 | −8.8 | −4.0 | −4.3 |
| Component | Temperatures [°C] Cold Case | Temperatures [°C] Hot Case | ||
|---|---|---|---|---|
| Minimum | Maximum | Minimum | Maximum | |
| Skeleton | −15.9 | 16.4 | 15.8 | 29.4 |
| Inner Skin | −16.4 | 23.9 | 23.1 | 43.9 |
| Outer Skin | −15.6 | 14.4 | 22.2 | 42.7 |
| Inner PCB | −8.4 | 19.2 | 25.8 | 31.5 |
| Outer PCB | −15.0 | 12.2 | 24.6 | 43.0 |
| Batteries | −6.0 | 21.6 | 27.4 | 31.6 |
| PCB tile −X | −1.1 | 19.9 | 17.2 | 25.7 |
| PCB tile −Y | −16.0 | 14.1 | 14.9 | 22.8 |
| PCB tile −Z | −16.4 | 11.7 | 16.3 | 26.6 |
| Antenna tile +X | −16.3 | 9.9 | 18.0 | 28.5 |
| PCB tile +Z | −14.1 | 14.0 | 15.6 | 27.4 |
| Payload | −12.6 | 10.1 | 21.9 | 31.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Capovilla, G.; Cestino, E.; Reyneri, L.; Valpiani, F. Modular Multifunctional Composite Structure for CubeSat Applications: Embedded Battery Prototype Thermal Analysis. Batteries 2025, 11, 172. https://doi.org/10.3390/batteries11050172
Capovilla G, Cestino E, Reyneri L, Valpiani F. Modular Multifunctional Composite Structure for CubeSat Applications: Embedded Battery Prototype Thermal Analysis. Batteries. 2025; 11(5):172. https://doi.org/10.3390/batteries11050172
Chicago/Turabian StyleCapovilla, Giorgio, Enrico Cestino, Leonardo Reyneri, and Federico Valpiani. 2025. "Modular Multifunctional Composite Structure for CubeSat Applications: Embedded Battery Prototype Thermal Analysis" Batteries 11, no. 5: 172. https://doi.org/10.3390/batteries11050172
APA StyleCapovilla, G., Cestino, E., Reyneri, L., & Valpiani, F. (2025). Modular Multifunctional Composite Structure for CubeSat Applications: Embedded Battery Prototype Thermal Analysis. Batteries, 11(5), 172. https://doi.org/10.3390/batteries11050172








