Fatigue Performance Analysis of an Existing Orthotropic Steel Deck (OSD) Bridge
Abstract
:1. Introduction
2. The Orthotropic Bridge Deck Structural System
2.1. Fatigue Phenomenon in the Orthotropic Plates
- Nucleation: the period of life for the detail from the undamaged state up to the formation of a superficial defect starting from the initial microscopic damage;
- Stable propagation: increase in the size of the damage inside the material in a direction orthogonal to the applied stress field;
- Collapse and final failure: following the propagation of the crack, the resistant section is no longer able to withstand the applied load.
- Defects in the welds;
- Local vibration of the ribs and plates;
- Adoption of construction details that are not very suitable for fatigue resistance;
- Exposure to the corrosive environment;
- Development of surface defects;
- Onset of secondary stresses not foreseen in the project.
2.2. Models for the Fatigue Assessment
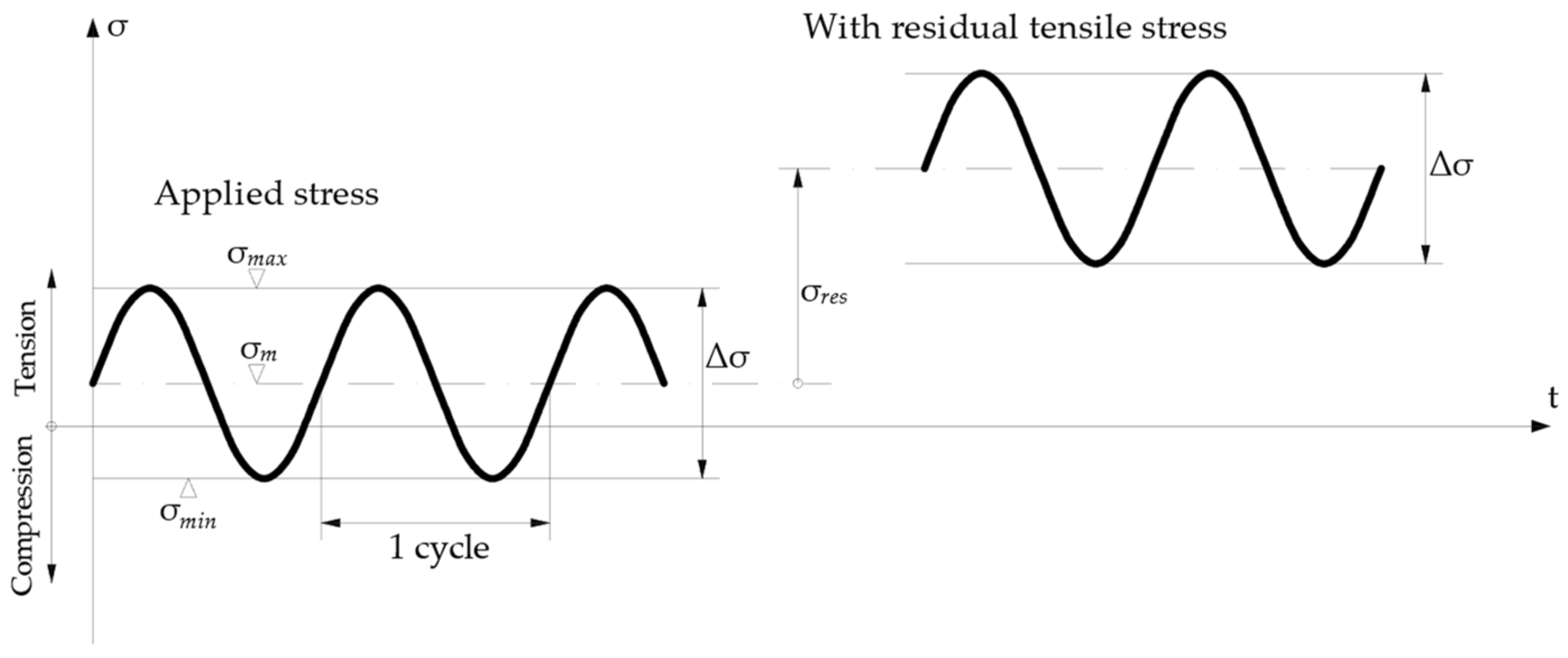
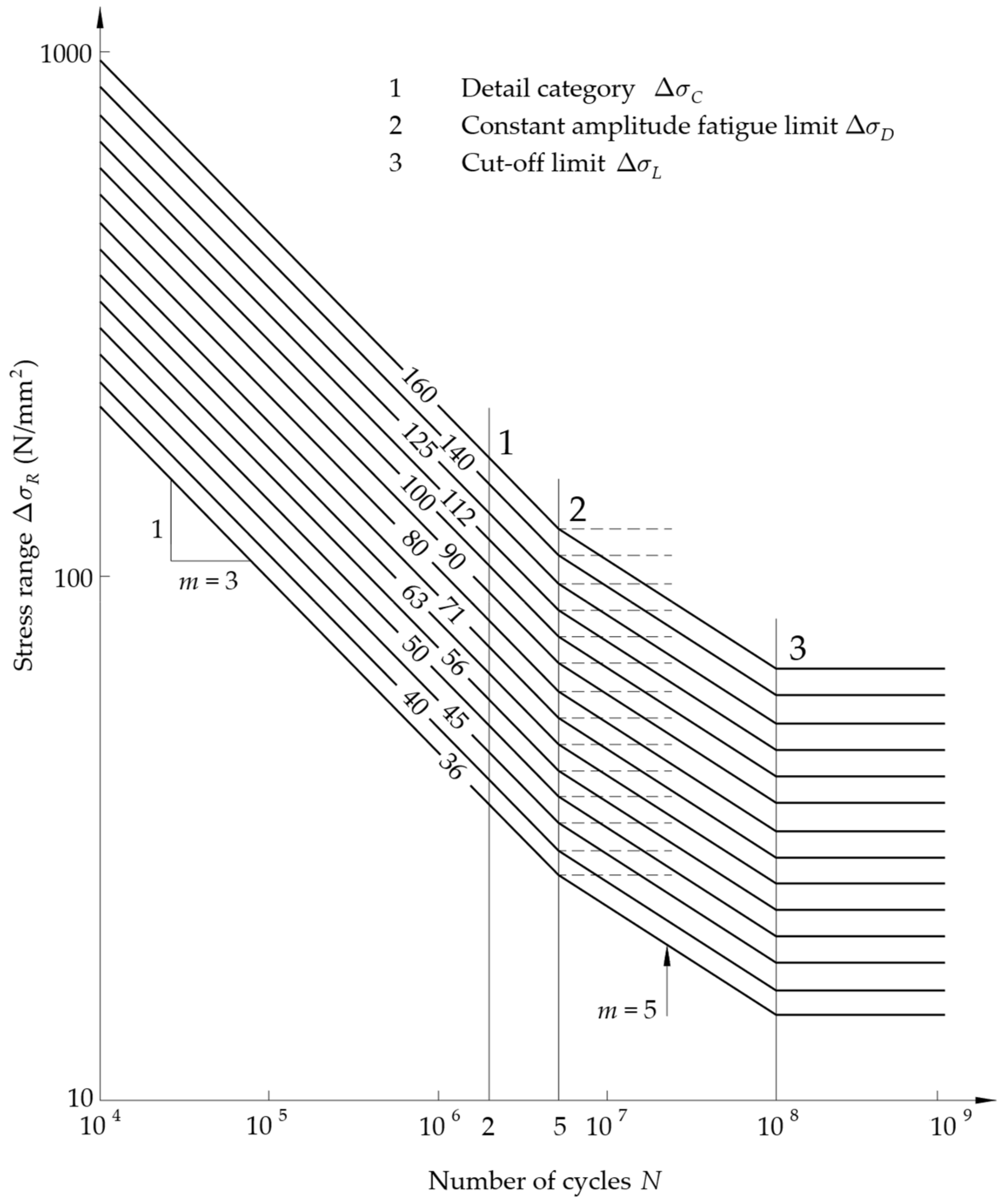
2.2.1. Wöhler’s Curve
2.2.2. Palmgreen–Miner Linear Damage Accumulation Criterion
- : number of cycles with a constant stress range obtained by the load spectrum;
2.3. Normative Approach
- Fatigue load model 1 (FLM1), prescribes infinite fatigue lifetime, stress range is assumed based on static calculation without any calculation of load cycles, very conservative;
- Fatigue load model 2 (FLM2), prescribes infinite fatigue lifetime, stress range is assumed from an ideal frequent configuration without any calculation of load cycles, conservative;
- Fatigue load model 3 (FLM3), prescribes the assessment of damage accumulation with an equivalent stress range and traffic volume;
- Fatigue load model 4 (FLM4), prescribes the assessment of damage accumulation based on a set of heavy lorries with assigned relative traffic frequency;
- Fatigue load model 5 (FLM5), prescribes the assessment of damage accumulation based on the experimental assessment of the fatigue spectrum.
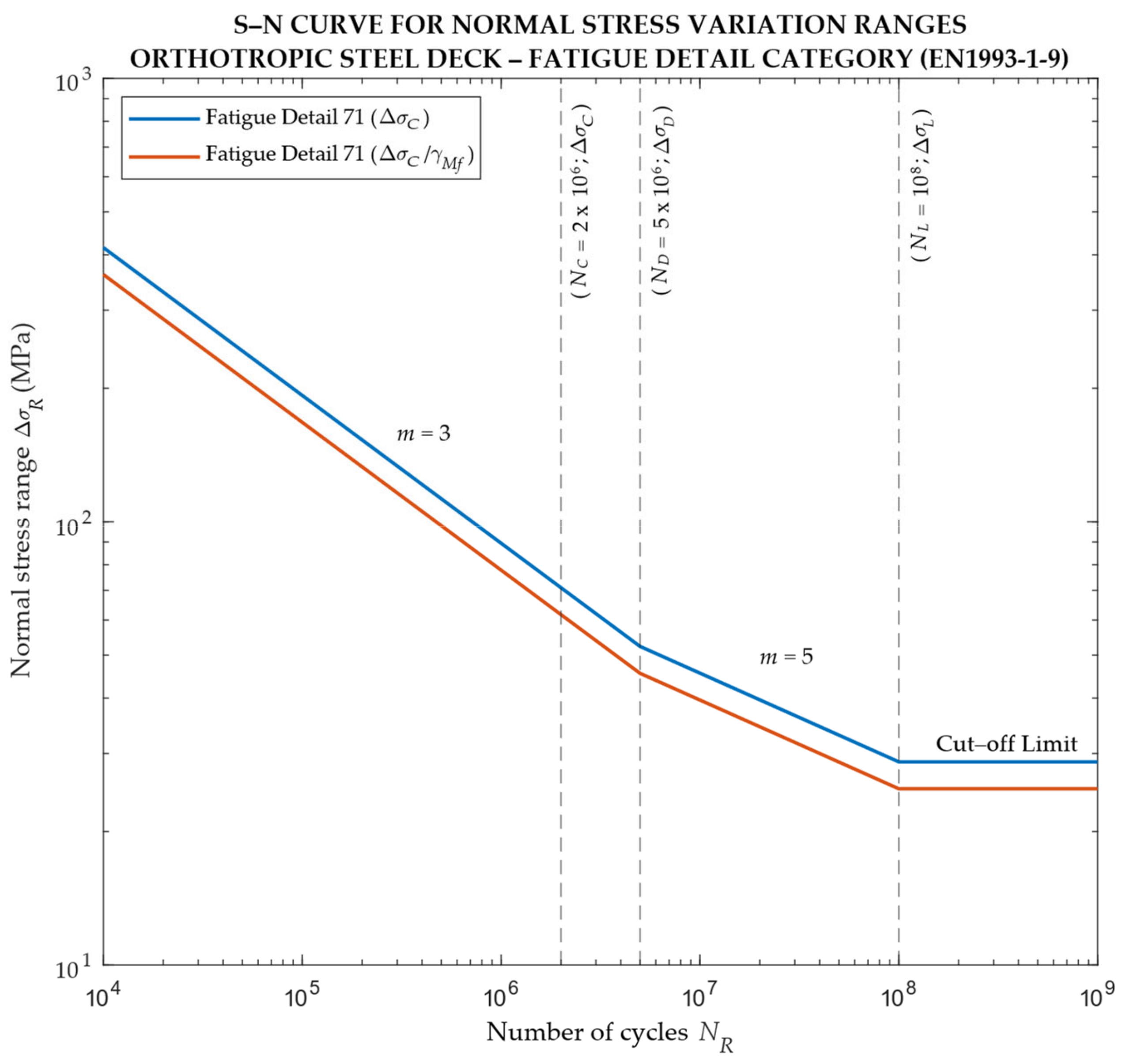
2.3.1. Normative S-N Curve
2.3.2. Unlimited Fatigue Life Approach
- max (): design value of the maximum stress range spectrum and induced in detail from FLM2, with ;
- : fatigue strength taken as the constant amplitude fatigue limit of the considered construction details;
- : partial factor for stress range;
- : partial factor for fatigue strength.
2.3.3. Fatigue Damage Accumulation Approach
- the stress variation intervals and their number of cycles;
- the mean stress, where it is necessary to consider the influence of the mean stress.
- : number of cycles with a constant stress range induced by the load spectrum during the lifetime prescribed for the analysis;
- : resistance (in cycles) obtained for a constant stress range , considering the partial factors, and , derived from the design S-N curve for the considered detail;
- = 1: limit damage value.
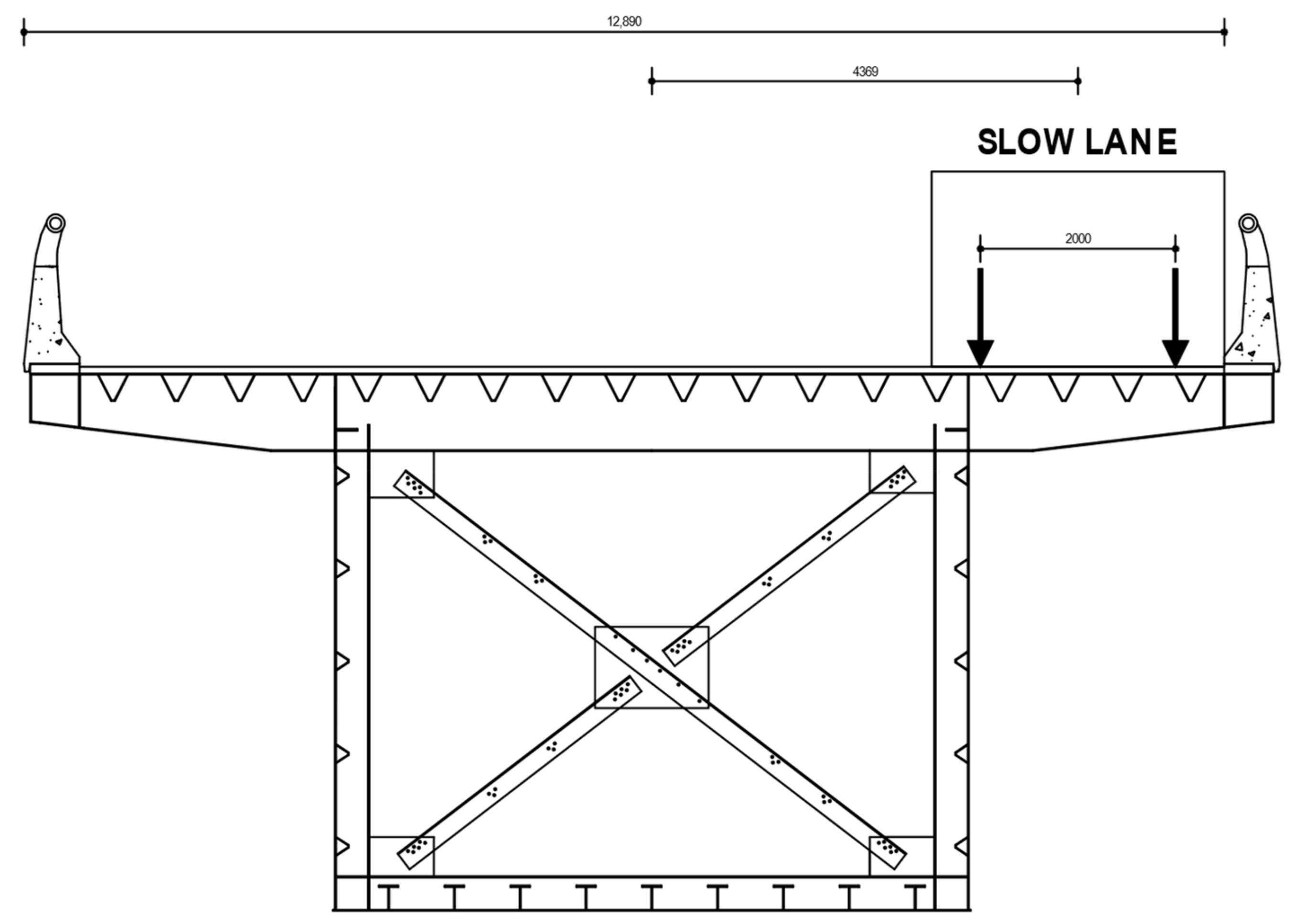
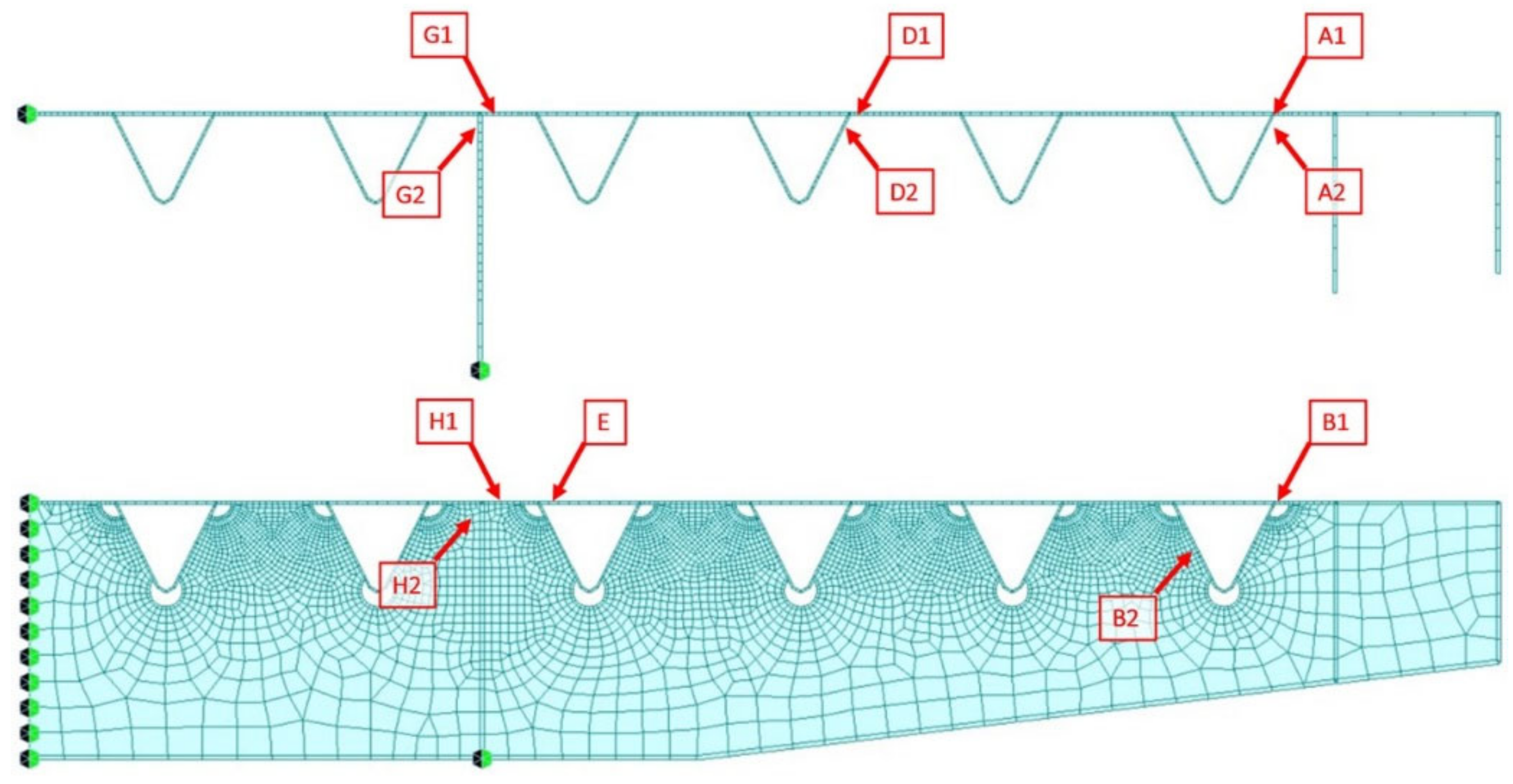
3. Case Study: Fatigue Life Assessment of an Existing OSD Viaduct
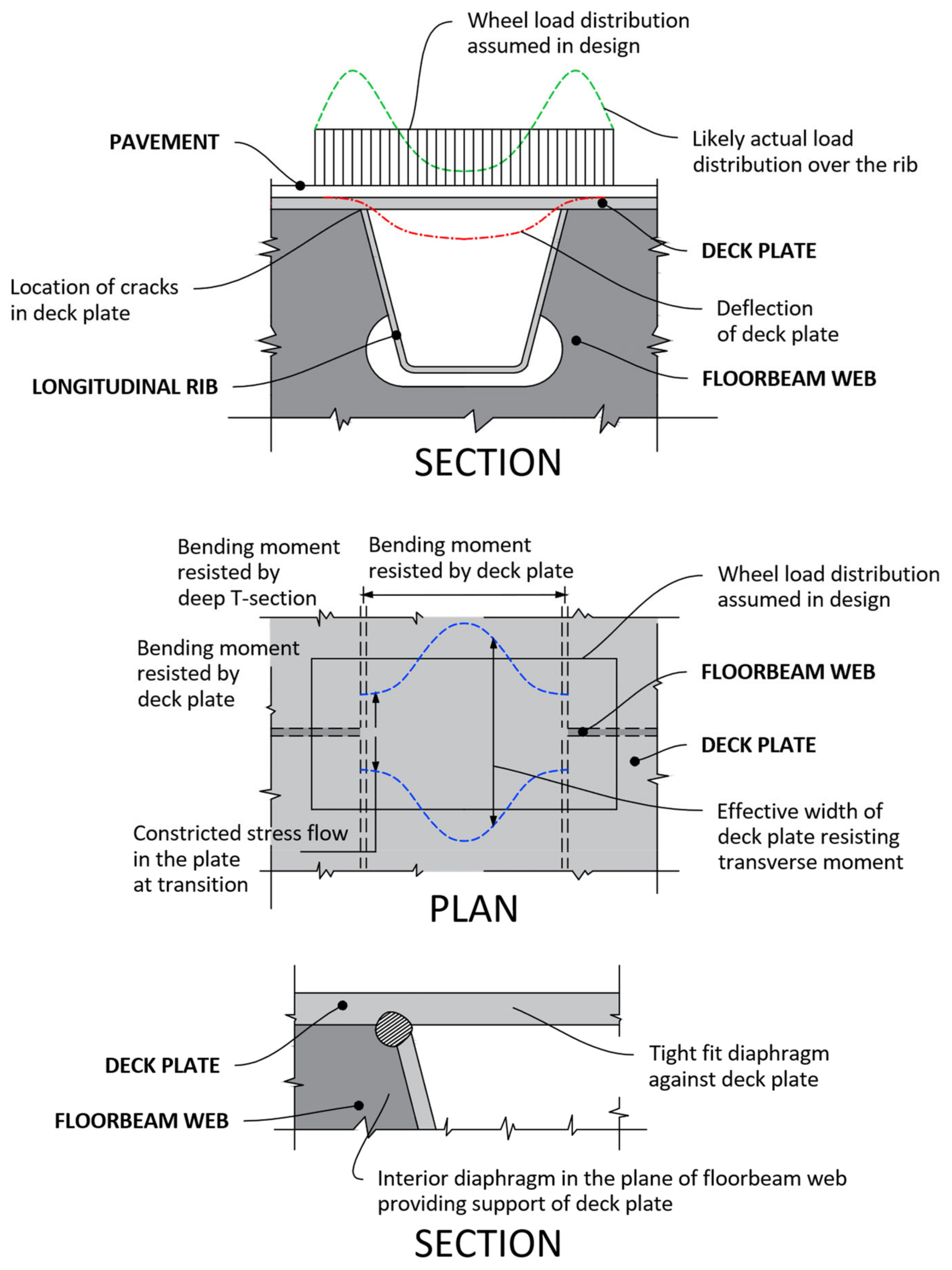
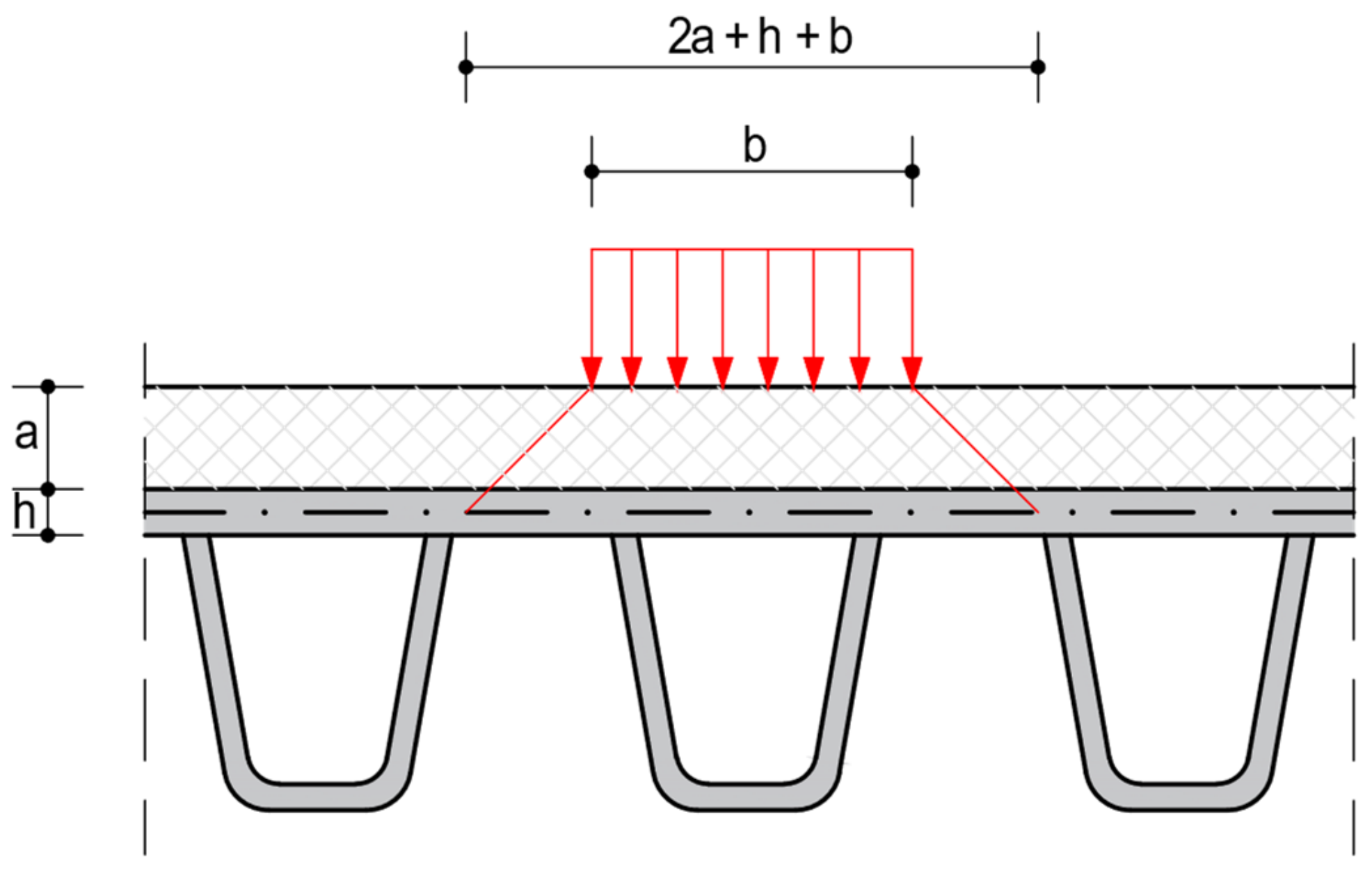
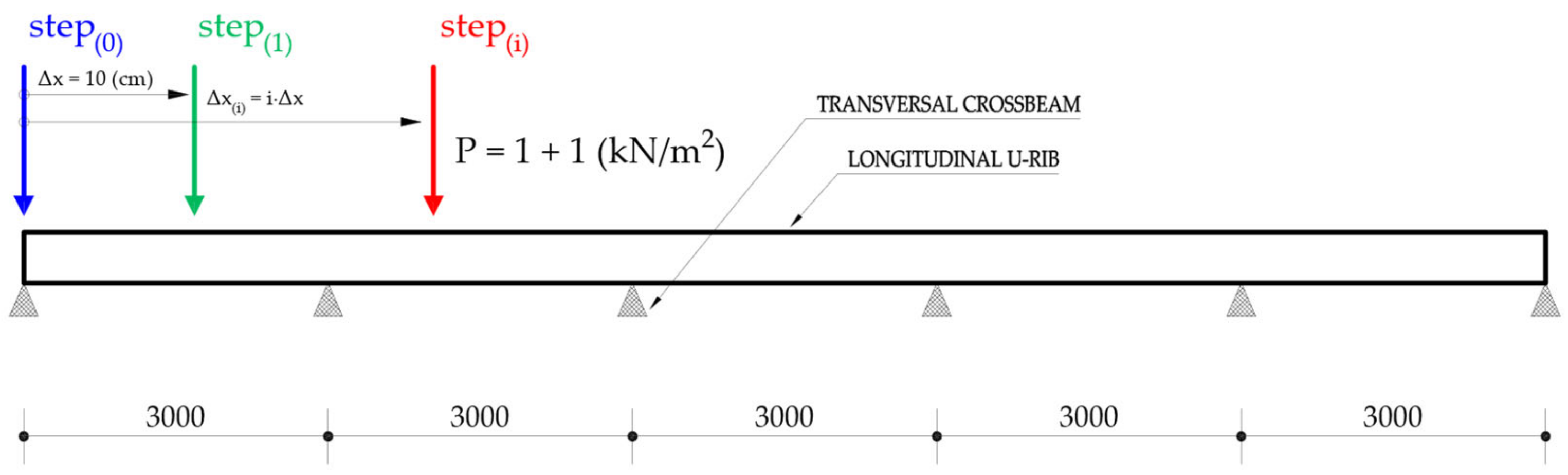
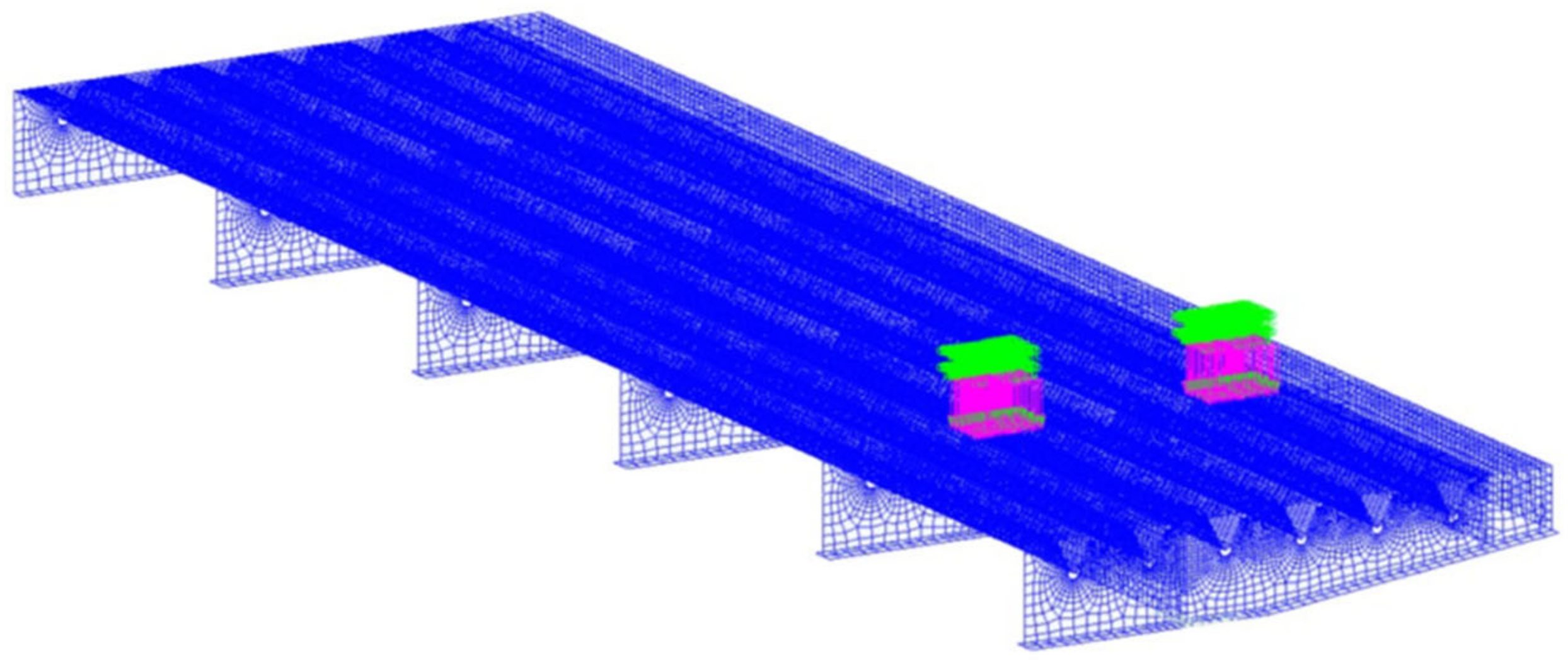
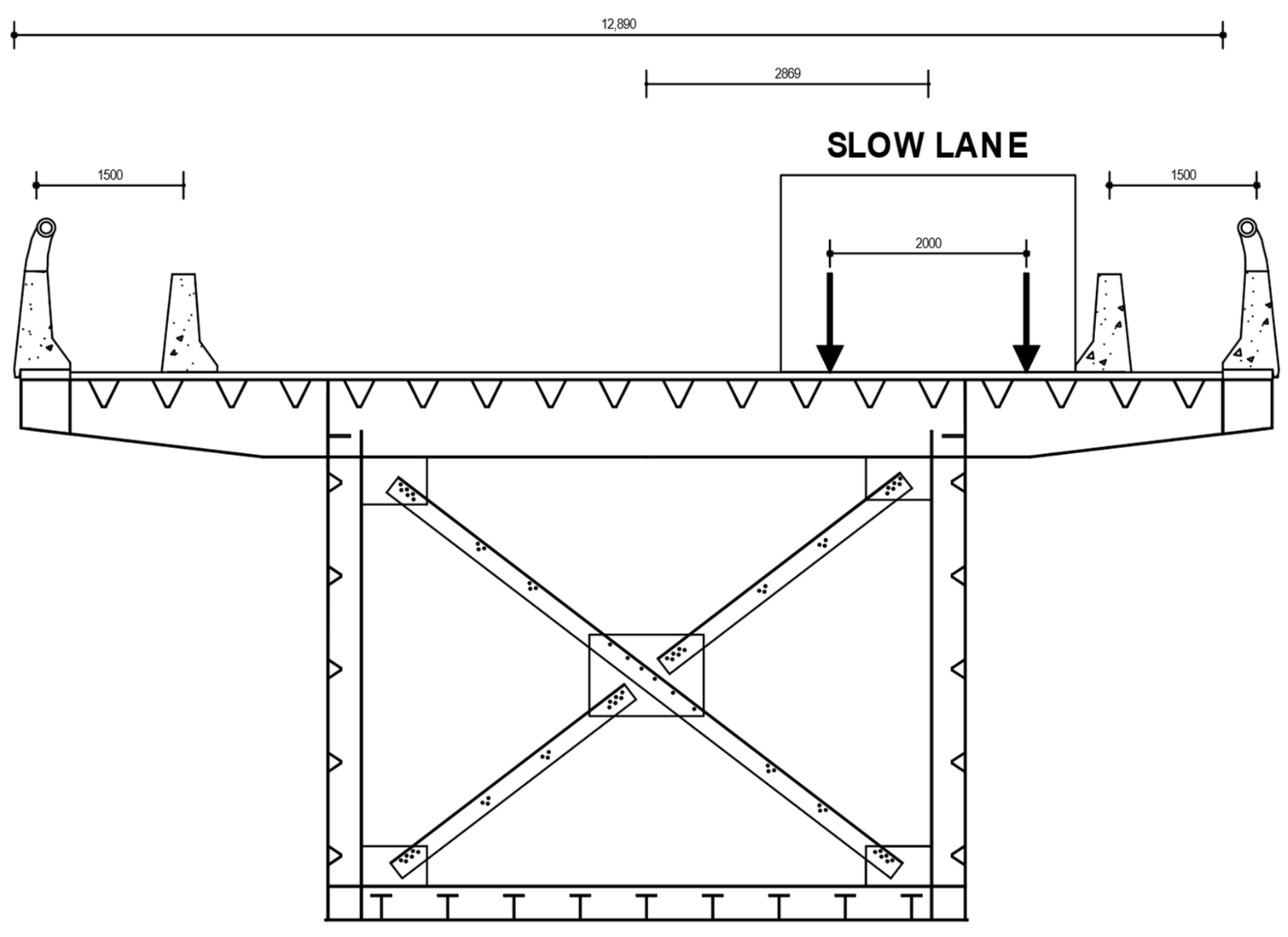
3.1. Numerical Model
- Longitudinal extrusion of the upper plate of the deck with a mesh of 10 cm;
- Simulation of structural continuity through the assignment of “supports”: displacements in the three global directions were constrained in correspondence to the core of the transverse rib, the upper plate, and the core of the body;
- Rib copying with a step of 3 m for five times;
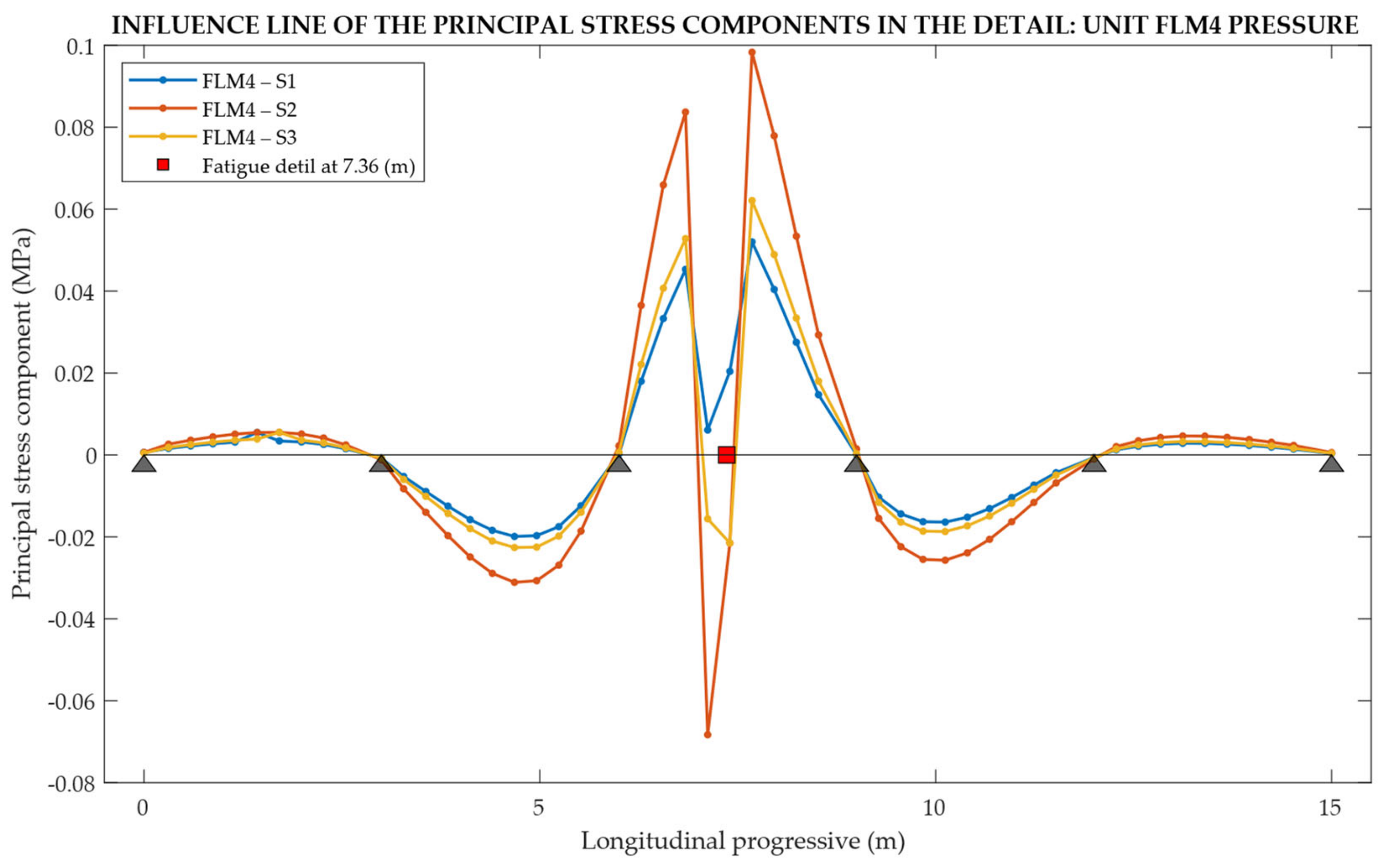
- Assignment of static “pressure moving load loads” (Figure 15).
3.2. Fatigue Analysis
3.2.1. Number of Fatigue Cycles
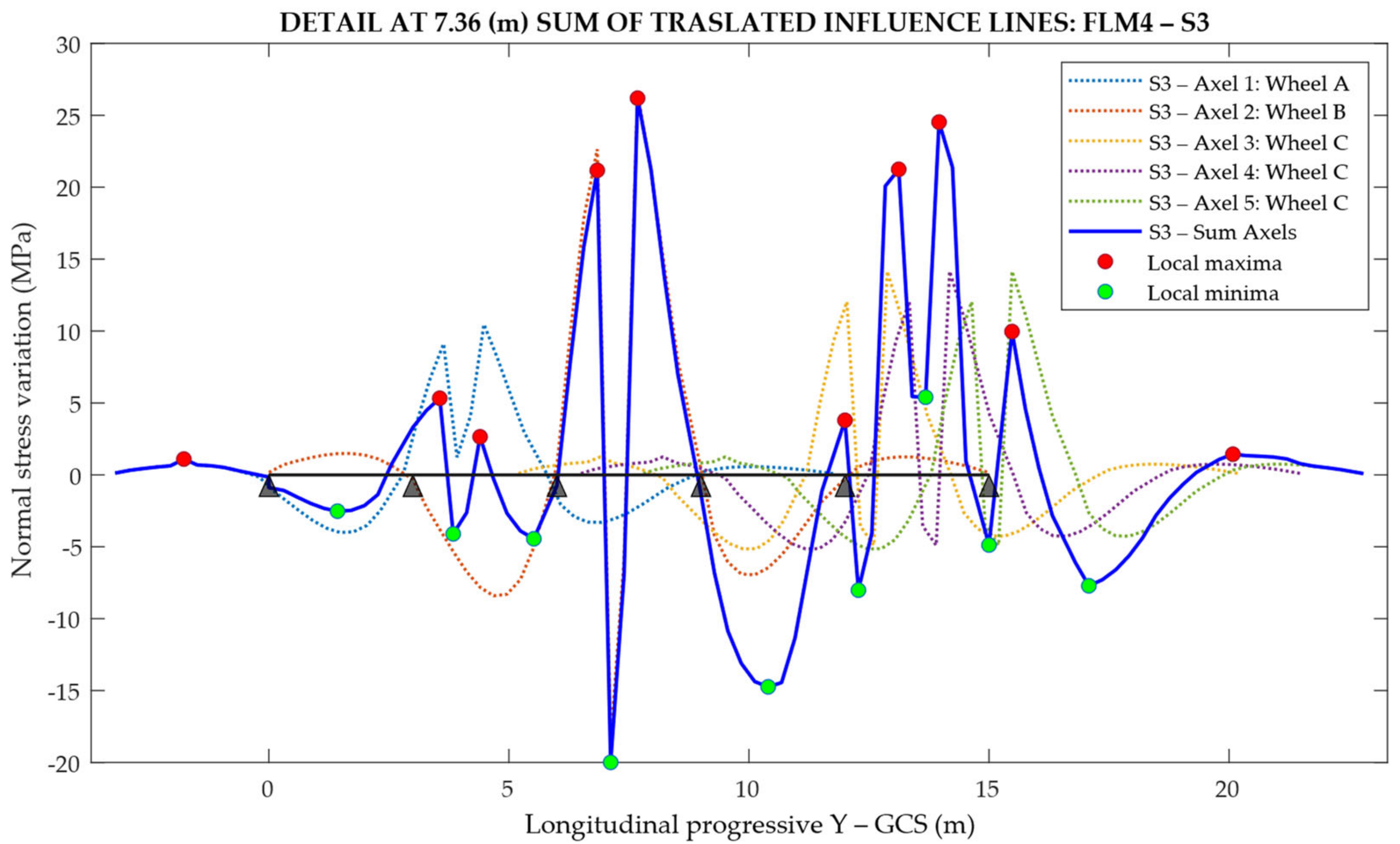
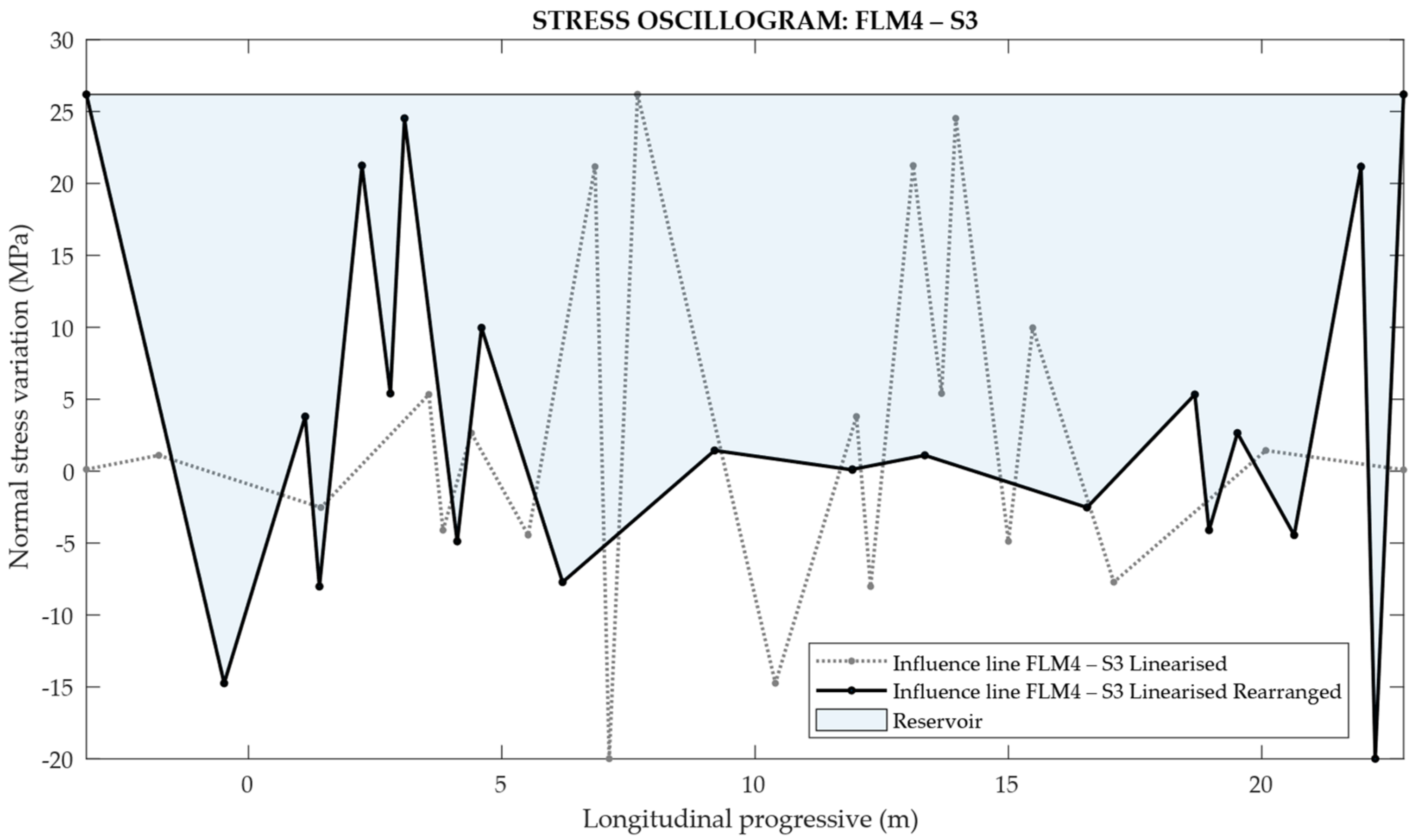
3.2.2. Post-Processing
3.2.3. Fatigue Assessment
3.3. Results and Discussion
3.3.1. Rib-to-Deck (Plate) Detail
3.3.2. Verification Summary
- Group 1 (R): A1, A2, B1, and B2: critical details in the original condition. After the lane number reduction and placement of the additional New Jersey barrier, these details are unloaded;
- Group 2 (L): D1, D2, G1, G2, H1, and H2: those details are less critical in the original condition, though some of them will accumulate damage after the lane reduction due also to the overlapping of the load silhouette (Figure 32).
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- AISC. Design Manual for Orthotropic Steel Plate Deck Bridges; American Institute of Steel Construction: New York, NY, USA, 1963; ISBN 978-1-56424-004-0. [Google Scholar]
- Connor, R.J.; Federal Highway Administration; Fisher, J.; Gatti, W.; Gopalartnam, V.; Kozy, B. Manual for Design, Construction and Maintenance of Orthotropic Steel Deck Bridges; Federal Highway Administration, U.S. Department of Transportation: Washington, DC, USA, 2012. [Google Scholar]
- Troitsky, M.S. Orthotropic Bridges—Theory and Design, 2nd ed.; The National Academies of Sciences, Engineering, and Medicine: Washington, DC, USA, 1987; pp. 57–63. [Google Scholar]
- Russo, F.; Mertz, D.R.; Frank, K.H.; Wilson, K.E. Design and Evaluation of Steel Bridges for Fatigue and Fracture—Reference Manual; National Highway Institute (US): Vienna, VA, USA, 2016; ISBN 979-8611492352. [Google Scholar]
- Tecchio, G.; Lorenzoni, F.; Caldon, M.; Donà, M.; Da Porto, F.; Modena, C. Monitoring of orthotropic steel decks for experimental evaluation of residual fatigue life. J. Civ. Struct. Health Monit. 2017, 3, 517–539. [Google Scholar] [CrossRef]
- Yan, F.; Chen, W.; Lin, Z. Prediction of fatigue life of welded details in cable-stayed orthotropic steel deck bridges. Eng. Struct. 2016, 127, 344–368. [Google Scholar] [CrossRef]
- Zhang, Q.; Liu, Y.; Bao, Y.; Jia, D.; Bu, Y.; Li, Q. Fatigue Performance of Orthotropic Steel-Concrete Composite Deck with Large-Size Longitudinal U-Shaped Ribs. Eng. Struct. 2017, 150, 864–874. [Google Scholar] [CrossRef]
- Zou, S.; Cao, R.; Deng, L.; Wang, W. Effect of stress reversals on fatigue life evaluation of OSD considering the transverse distribution of vehicle loads. Eng. Struct. 2022, 265, 114400. [Google Scholar] [CrossRef]
- Zhang, Q.; Li, J.; Yuan, D.; Bu, Y. Fatigue performance of rib-to-deck joint in orthotropic steel bridge deck with new type of both-side fillet welded joints. In Proceedings of the IABSE Symposium Nantes 2018: Tomorrow’s Megastructures, Nantes, France, 19–21 September 2018; pp. S7-19–S7-26. [Google Scholar]
- Kainuma, S.; Yang, M.; Jeong, Y.S.; Inokuchi, S.; Kawabata, A.; Uchida, D. Experimental investigation for structural parameter effects on fatigue behavior of rib-to-deck welded joints in orthotropic steel decks. Eng. Fail. Anal. 2017, 79, 520–537. [Google Scholar] [CrossRef]
- Ji, B.; Liu, R.; Chen, C.; Maeno, H.; Chen, X. Evaluation on root-deck fatigue of orthotropic steel bridge deck. J. Constr. Steel Res. 2013, 90, 174–183. [Google Scholar] [CrossRef]
- Wang, Q.; Ji, B.; Xia, J.; Fu, Z. Fatigue assessment of rib-deck welds in orthotropic steel decks integrating the effect of load dispersal through asphalt surfacing. Structures 2020, 28, 1701–1712. [Google Scholar] [CrossRef]
- Wang, D.; Xiang, C.; Ma, Y.; Chen, A.; Wang, B. Experimental study on the root-deck fatigue crack on orthotropic steel decks. Mater. Des. 2021, 203, 109601. [Google Scholar] [CrossRef]
- Huang, Y.; Zhang, Q.; Bao, Y.; Bu, Y. Fatigue assessment of longitudinal rib-to-crossbeam welded joints in orthotropic steel bridge decks. J. Constr. Steel Res. 2019, 159, 53–66. [Google Scholar] [CrossRef]
- Cheng, B.; Abdelbaset, H.; Li, H.T.; Tian, L.; Zhao, J. Fatigue behavior of rib-to-floorbeam welded connection in UHPC reinforced OSDs subjected to longitudinal flexural. Eng. Fail. Anal. 2022, 137, 106383. [Google Scholar] [CrossRef]
- Zhu, Z.; Xiang, Z.; Li, J.; Carpinteri, A. Fatigue damage investigation on diaphragm cutout detail on orthotropic bridge deck based on field measurements and FEM. Thin-Walled Struct. 2020, 157, 107106. [Google Scholar] [CrossRef]
- Zhu, Z.; Xiang, Z.; Li, J.; Huang, Y.; Ruan, S. Fatigue behavior of orthotropic bridge decks with two types of cutout geometry based on field monitoring and FEM analysis. Eng. Struct. 2020, 209, 109926. [Google Scholar] [CrossRef]
- Chen, S.; Huang, Y.; Zhou, C.; Gu, P. Experimental and numerical study on fatigue performance of U-rib connections. J. Constr. Steel Res. 2019, 163, 105796. [Google Scholar] [CrossRef]
- Zhu, A.; Li, M.; Zhu, H.; Xiao, H.; Ge, H. Fatigue behaviour of orthotropic steel bridge decks with inner bulkheads. J. Constr. Steel Res. 2018, 146, 63–75. [Google Scholar] [CrossRef]
- Li, J.; Zhu, Z. Effects of full internal bulkheads on fatigue behaviors of orthotropic steel decks. J. Constr. Steel Res. 2022, 196, 107400. [Google Scholar] [CrossRef]
- Dong, P. A structural stress definition and numerical implementation for fatigue analysis of welded joints. Int. J. Fatigue 2001, 23, 865–876. [Google Scholar] [CrossRef]
- Dong, P. A robust structural stress method for fatigue analysis of offshore/marine structures. Trans. ASME 2005, 127, 68–74. [Google Scholar] [CrossRef]
- Dong, P.; Prager, M.; Osage, D. The design master S-N curve in ASME Div 2 rewrite and its validations. Weld. World 2007, 51, 53–63. [Google Scholar] [CrossRef]
- Yang, H.; Wang, P.; Qian, H.; Dong, P. Analysis of fatigue test conditions for reproducing weld toe cracking into U-rib wall in orthotropic bridge decks. Int. J. Fatigue 2022, 162, 106976. [Google Scholar] [CrossRef]
- Yang, H.; Wang, P.; Qian, H.; Niu, S.; Dong, P. An experimental investigation into fatigue behaviors of single- and double-sided U rib welds in orthotropic bridge decks. Int. J. Fatigue 2022, 159, 106827. [Google Scholar] [CrossRef]
- Yang, H.; Wang, P.; Qian, H. Fatigue behaviors of typical of orthotropic steel bridges in multiaxial stress states using traction structural stress. Int. J. Fatigue 2020, 141, 105862. [Google Scholar] [CrossRef]
- Pei, X.; Dong, P.; Xing, S. A structural strain parameter for a unified treatment of fatigue behaviors of welded components. Int. J. Fatigue 2019, 124, 444–460. [Google Scholar] [CrossRef]
- Pei, X.; Dong, P.; Kim, M.H. A simplified structural strain method for low-cycle fatigue evaluation of girth-welded pipe connections. Int. J. Fatigue 2020, 139, 105732. [Google Scholar] [CrossRef]
- Sonsino, C.M. Consideration of allowable equivalent stresses for fatigue design of welded joints according to the notch stress concept with the reference radii rref = 1.00 and 0.05 mm. Weld. World 2009, 53, 64–75. [Google Scholar] [CrossRef]
- Karakas, Ö.; Baumgartner, J.; Susmel, L. On the use of fictitious notch radius equal to 0.3 mm to design against fatigue welded joints made of wrought magnesium alloy AZ31. Int. J. Fatigue 2020, 139, 105747. [Google Scholar] [CrossRef]
- Karakas, Ö.; Zhang, G.; Sonsino, C.M. Critical distance approach for the fatigue strength assessment of magnesium welded joints in contrast to Neuber’s effective stress method. Int. J. Fatigue 2018, 112, 21–35. [Google Scholar] [CrossRef]
- Luo, P.; Zhang, Q.; Bao, Y.; Zhou, A. Fatigue evaluation of rib-to-deck welded joint using averaged strain energy density method. Eng. Struct. 2018, 177, 682–694. [Google Scholar] [CrossRef]
- Wu, W.; Kolstein, H.; Veljkovic, M. Fatigue resistance of rib-to-deck welded joint in OSDs, analysed by fracture mechanics. J. Constr. Steel Res. 2019, 162, 105700. [Google Scholar] [CrossRef]
- Maljaars, J.; Bonet, E.; Pijpers, R.J.M. Fatigue resistance of the deck plate in steel orthotropic deck strcutures. Eng. Fract. Mech. 2018, 201, 214–228. [Google Scholar] [CrossRef]
- Wang, Q.; Ji, B.; Li, C.; Fu, Z. Fatigue evaluation of rib-to-deck welds: Crack-propagation-life predictive model and parametric analysis. J. Constr. Steel Res. 2020, 173, 106248. [Google Scholar] [CrossRef]
- Xiao, Z.G.; Yamada, K.; Inoue, J.; Yamaguchi, K. Fatigue cracks in longitudinal ribs of steel orthotropic deck. Int. J. Fatigue 2006, 28, 409–416. [Google Scholar] [CrossRef]
- Invernizzi, S.; Montagnoli, F.; Carpinteri, A. Fatigue Assessment of the Collapsed XXth Century Cable-Stayed Polcevera Bridge in Genoa. Procedia Struct. Integr. 2019, 18, 237–244. [Google Scholar] [CrossRef]
- Invernizzi, S.; Montagnoli, F.; Carpinteri, A. The collapse of the Morandi’s bridge: Remarks about fatigue and corrosion. In Proceedings of the IABSE Symposium Wroclaw 2020: Synergy of Culture and Civil Engineering—History and Challenges, Wroclaw, Poland, 7–9 October 2020; pp. 1040–1047. [Google Scholar]
- Invernizzi, S.; Montagnoli, F.; Carpinteri, A. Corrosion Fatigue Investigation on the Possible Collapse Reasons of Polcevera Bridge in Genoa. Lect. Notes Mech. Eng. 2020, 151–159. [Google Scholar] [CrossRef]
- Invernizzi, S.; Montagnoli, F.; Carpinteri, A. Very high cycle corrosion fatigue study of the collapsed Polcevera Bridge, Italy. J. Bridge Eng. 2022, 27, 04021102. [Google Scholar] [CrossRef]
- Chen, W.-F.; Duan, L. Bridge Engineering Handbook: Construction and Maintenance: Chapter 14, Orthotropic Deck Bridges; Mangus, A.R., Sun, S., Eds.; CRC Press: Boca Raton, FL, USA, 2014; ISBN 978-1-4398-5233-0. [Google Scholar]
- Masera, D.; Mairone, M.; Asso, R. Il Fenomeno della Fatica nei Ponti in Acciaio a Piastra Ortotropa. Strade Autostrade 2021, 149, 57–63. [Google Scholar]
- Qian, Z.H.; Abruzzese, D. Fatigue failure of welded connections at orthotropic bridges. Frat. E Integrità Strutt. 2009, 3, 105–112. [Google Scholar] [CrossRef] [Green Version]
- ASTM. American National Standard ANSI/ASTM E206-72; ASTM: West Conshohocken, PA, USA, 1979. [Google Scholar]
- Nussbaumer, A.; Borges, L.; Davaine, L. Fatigue Design of Steel and Composite Structures: Eurocode 3: Design of Steel Structures, Part 1–9 Fatigue; Eurocode 4: Design of Composite Steel and Concrete Structures; John Wiley & Sons: Hoboken, NJ, USA, 2012; ISBN 978-3-433-60120-4. [Google Scholar]
- Wolchuk, R. Steel Orthotropic Decks: Developments in the 1990s. Transp. Res. Rec. 1999, 1688, 30–37. [Google Scholar] [CrossRef]
- Carpinteri, A.; Montagnoli, F.; Invernizzi, S. Scaling and fractality in fatigue resistance: Specimen-size effects on Wöhler’s curve and fatigue limit. Fatigue Fract. Eng. Mater. Struct. 2020, 43, 1869–1879. [Google Scholar] [CrossRef]
- Carpinteri, A.; Montagnoli, F. Scaling and fractality in subcritical fatigue crack growth: Crack-size effects on Paris’ law and fatigue threshold. Fatigue Fract. Eng. Mater. Struct. 2020, 43, 788–801. [Google Scholar] [CrossRef]
- Carpinteri, A.; Montagnoli, F. Scaling and fractality in fatigue crack growth: Implications to Paris’ law and Wöhler’s curve. Procedia Struct. Integr. 2019, 14, 957–963. [Google Scholar] [CrossRef]
- Montagnoli, F.; Invernizzi, S.; Carpinteri, A. Fractality and size effect in fatigue damage accumulation: Comparison between Paris and Wöhler’s curve. Lect. Notes Mech. Eng. 2020, 188–196. [Google Scholar] [CrossRef]
- Invernizzi, S.; Montagnoli, F.; Carpinteri, A. Experimental evidence of specimen-size effects on EN-AW6082 aluminum alloy in VHCF regime. Appl. Sci. 2021, 11, 4272. [Google Scholar] [CrossRef]
- Invernizzi, S.; Paolino, D.; Montagnoli, F.; Tridello, A.; Carpinteri, A. Comparison between fractal and statistical approaches to model size effects in VHCF. Metals 2022, 12, 1499. [Google Scholar] [CrossRef]
- European Committee for Standardization. EN 1993-1-9 Eurocode 3: Design of Steel Structures Part 1–9 Fatigue; European Committee for Standardization: Brussels, Belgium, 2005. [Google Scholar]
- European Committee for Standardization. EN 1993-2 Eurocode 3: Design of Steel Structures: Steel Bridges. Brussels, European Committee for Standardization Part 2: Steel Bridges; European Committee for Standardization: Brussels, Belgium, 2007. [Google Scholar]
- European Committee for Standardization. EN 1991-2 Eurocode 1: Actions on Structures Part 2: Traffic Loads on Bridges; European Committee for Standardization: Brussels, Belgium, 2005. [Google Scholar]
- Miner, M.A. Cumulative Damage in Fatigue. J. Appl. Mech. 1945, 12, 149–164. [Google Scholar] [CrossRef]
- Palmgren, A. “Die Lebensdauer von Kugellagern” (The Service Life of Ball Bearings). Z. Des Ver. Dtsch. 1924, 68, 339–341. [Google Scholar]
- Masera, D.; Mairone, M. Modellazione e Analisi di un Viadotto Esistente con Impalcato a Cassone in Acciaio a Piastra Ortotropa. Available online: https://www.harpaceas.it/case-studies/modellazione-e-analisi-di-un-viadotto-esistente-con-impalcato-a-cassone-in-acciaio-a-piastra-ortotropa (accessed on 2 July 2022).
- Asso, R.; Cucuzza, R.; Rosso, M.M.; Masera, D.; Marano, G.C. Bridges Monitoring: An Application of AI with Gaussian Processes. In Proceedings of the 4th International Conference on Evolutionary and Deterministic Methods for Design, Optimization and Control; Institute of Structural Analysis and Antiseismic Research National Technical University of Athens, Athens, Greece, 28–30 June 2021. [Google Scholar]
- Mairone, M. Study of an Existing Viaduct with Box-Girder Orthotropic Steel Deck. Master’s Thesis, Politecnico di Torino, Turin, Italy, 2021. [Google Scholar]
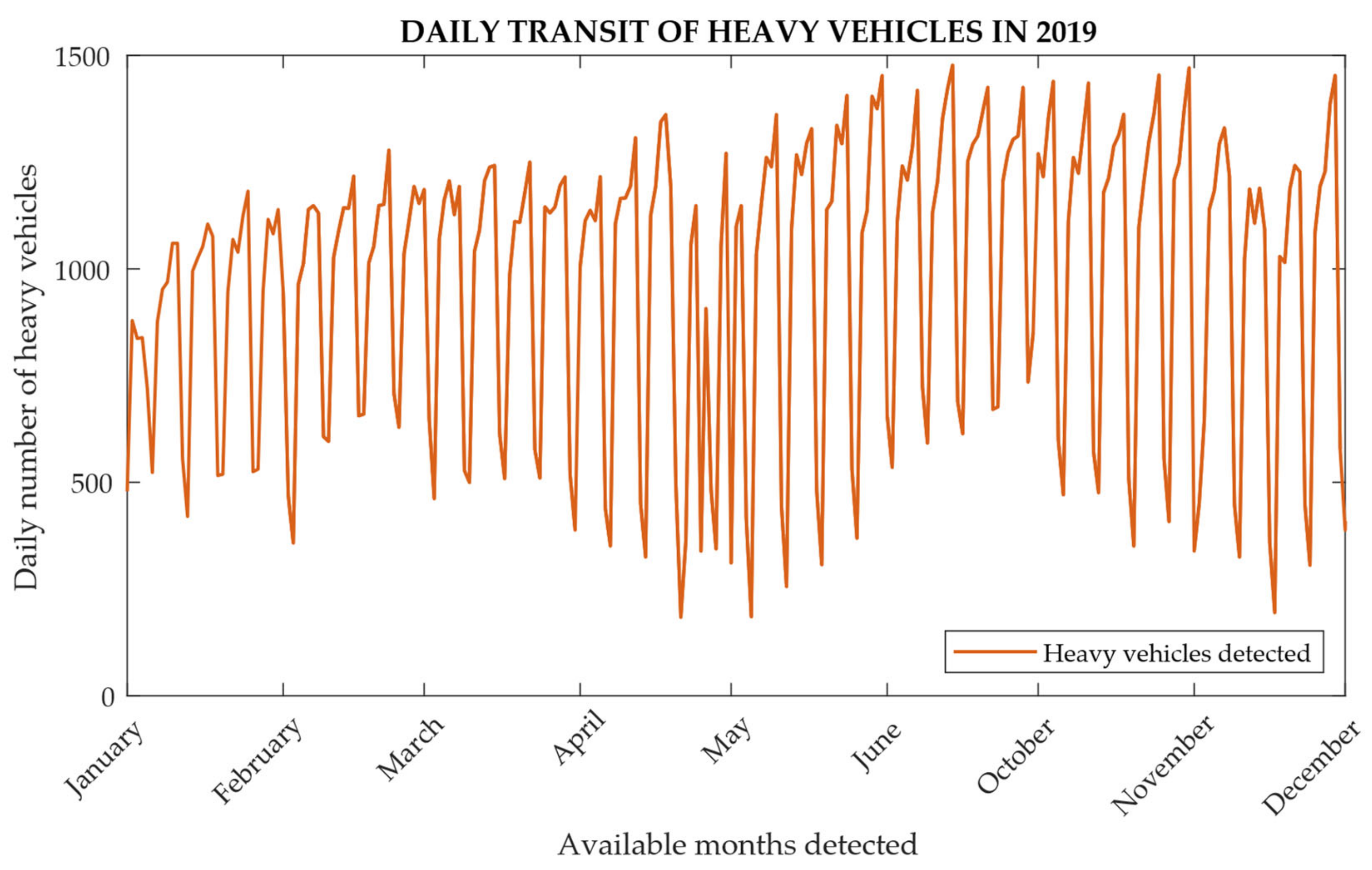
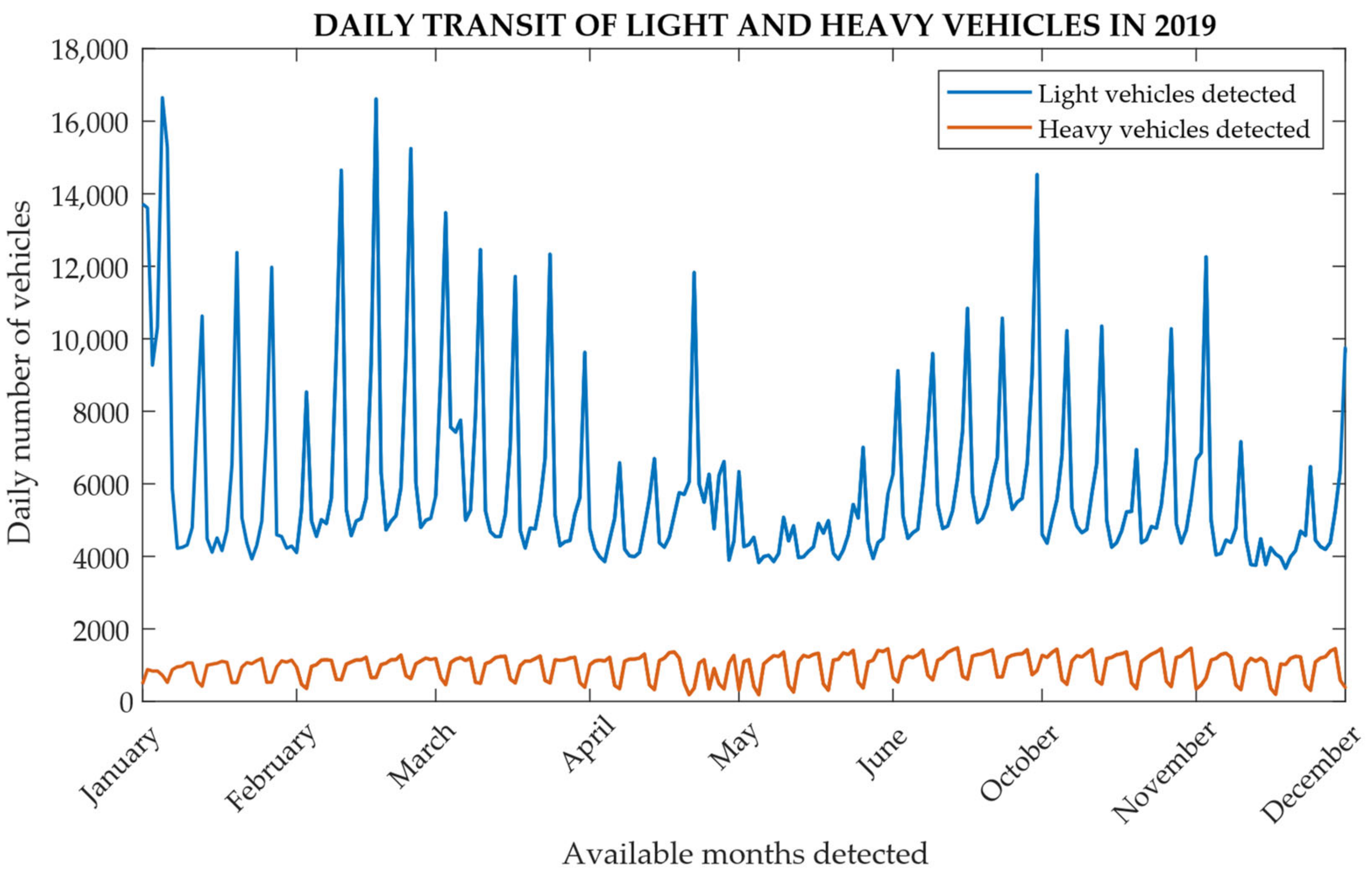
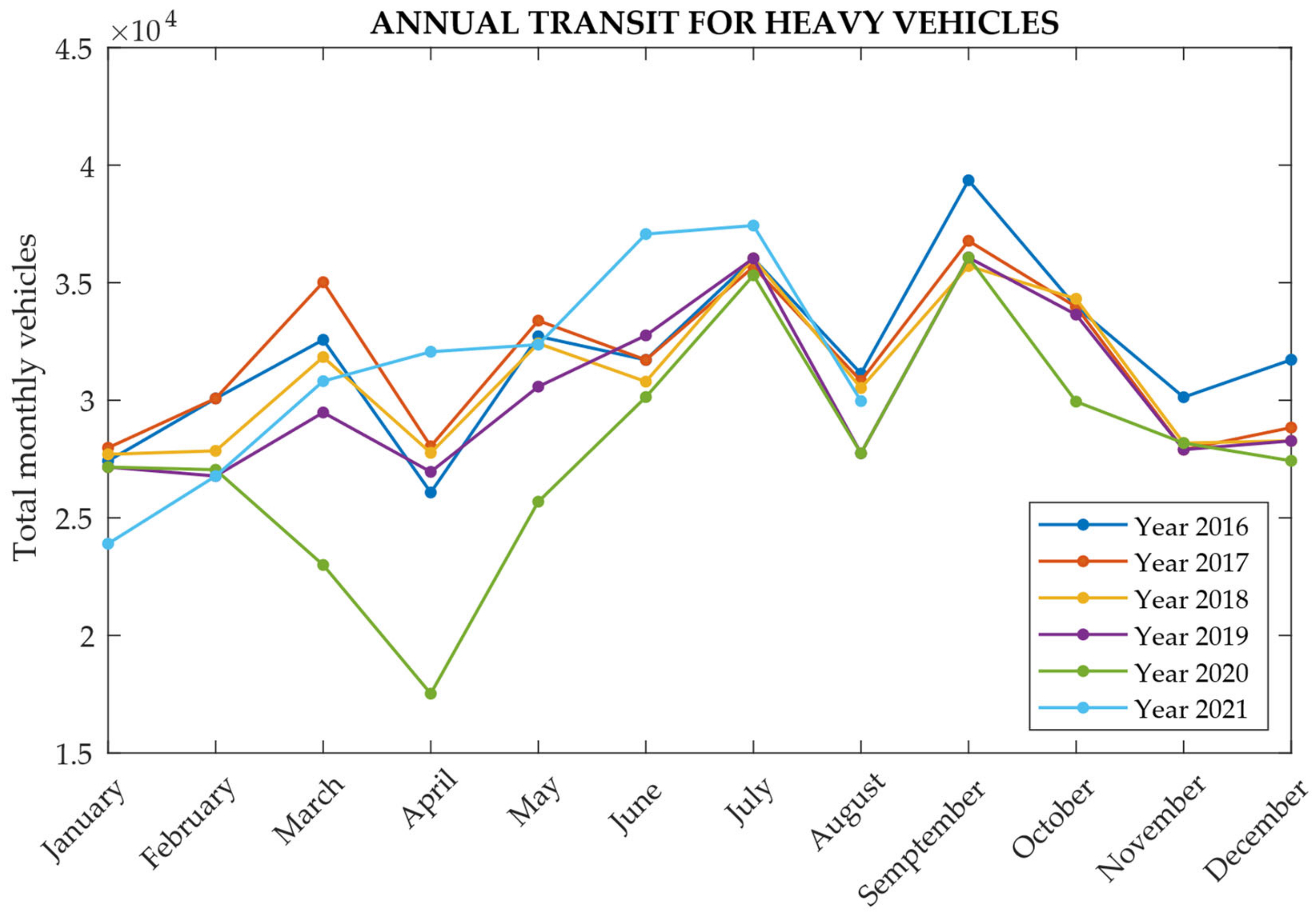
- Traffic Observatory Archive. Available online: https://www.stradeanas.it/it/le-strade/osservatorio-del-traffico/archivio-osservatorio-del-traffico (accessed on 1 September 2021).
- De Jong, F.B.P.; Bijlaard, F.S.K. Renovation Techniques for Fatigue Cracked Orthotropic Steel Bridge Decks; Technische Universiteit Delft: Delft, The Netherlands, 2007; ISBN 978-90-90-21412-2. [Google Scholar]
- AASHTO. LRFD Bridge Design Specifications; American Association of State Highway and Transportation Officials: Washington, DC, USA, 2008. [Google Scholar]
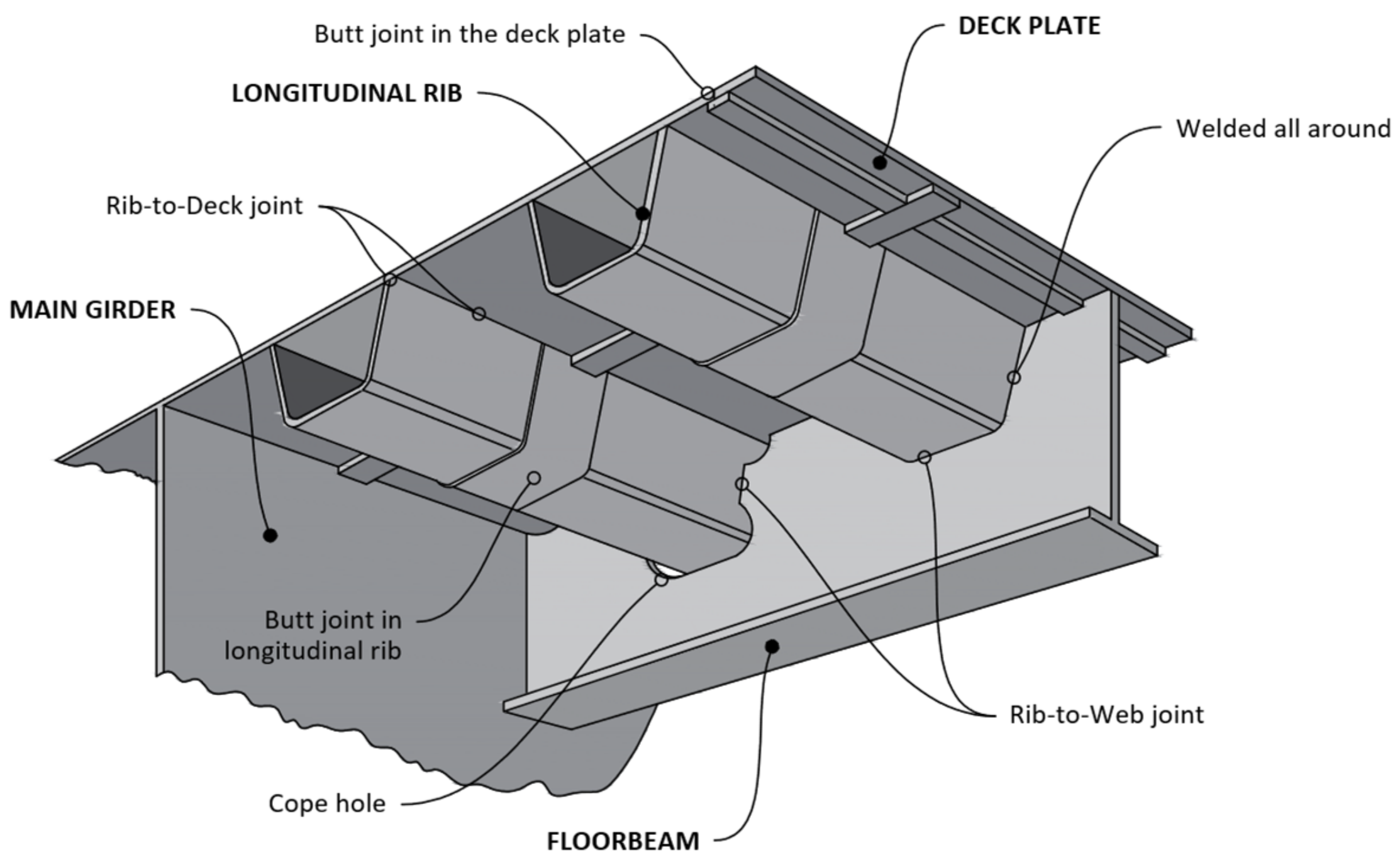
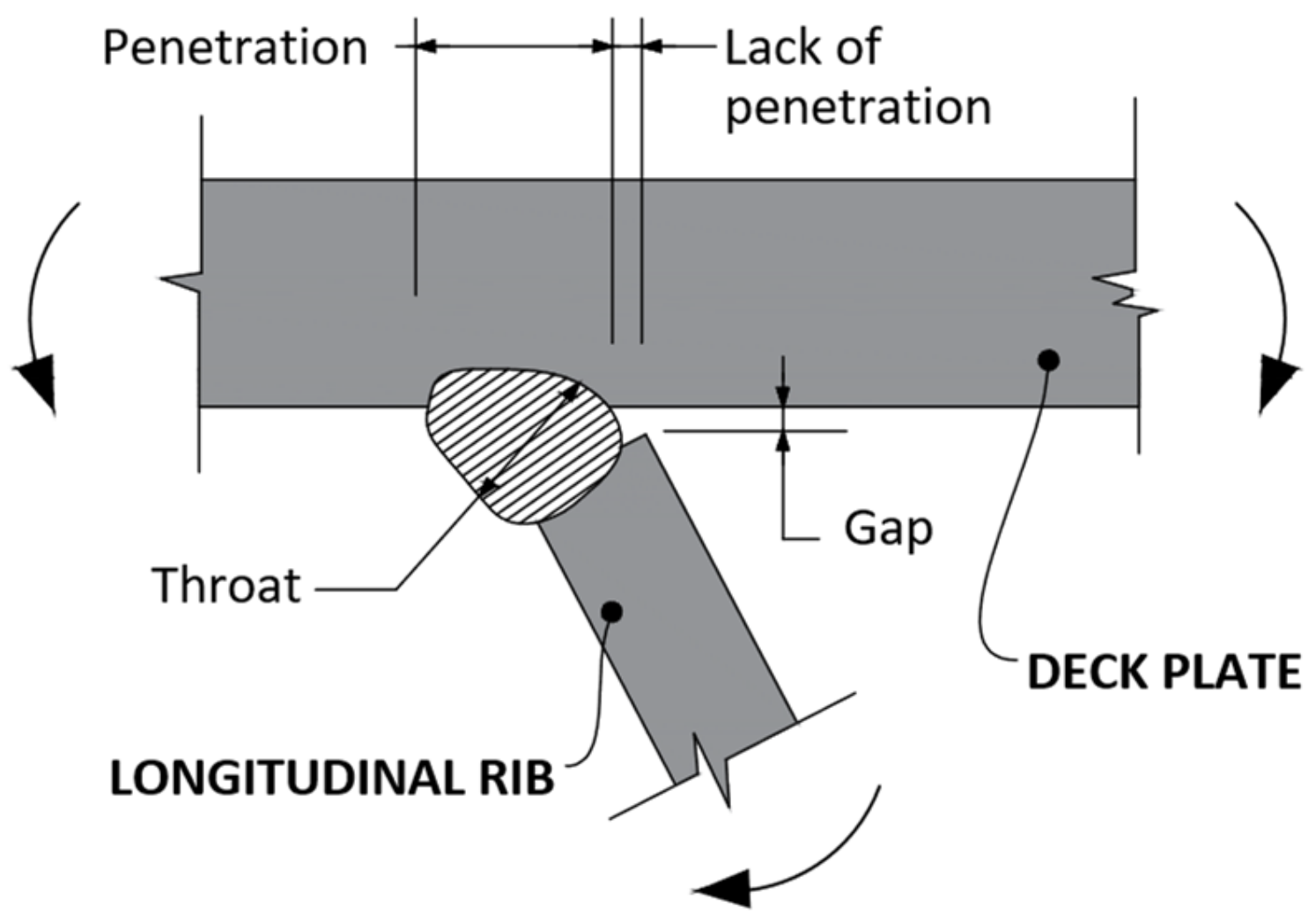
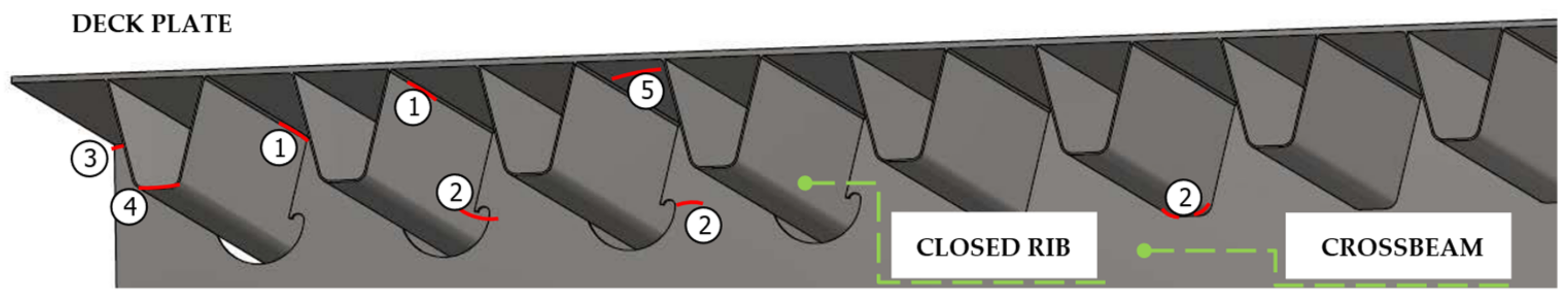

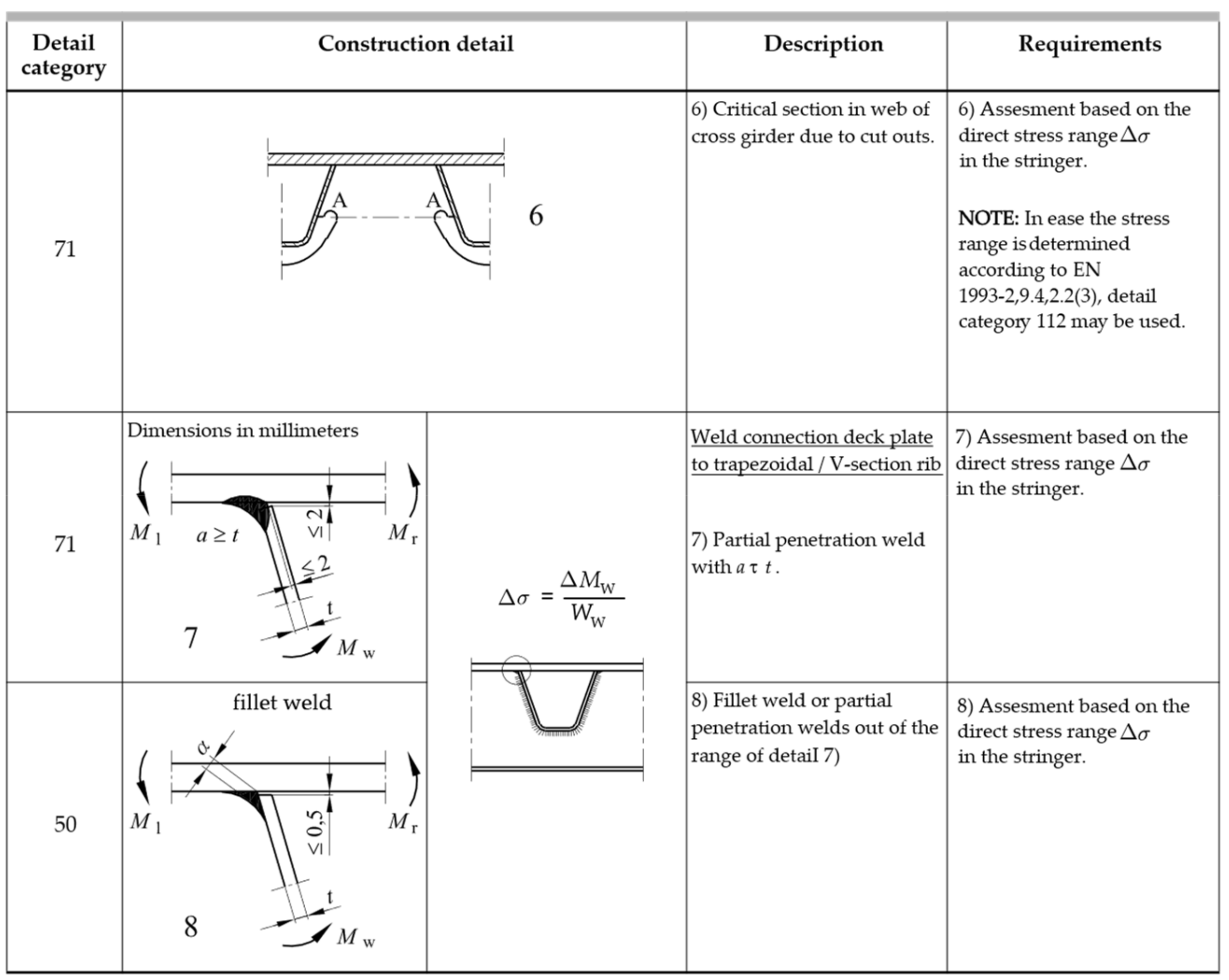






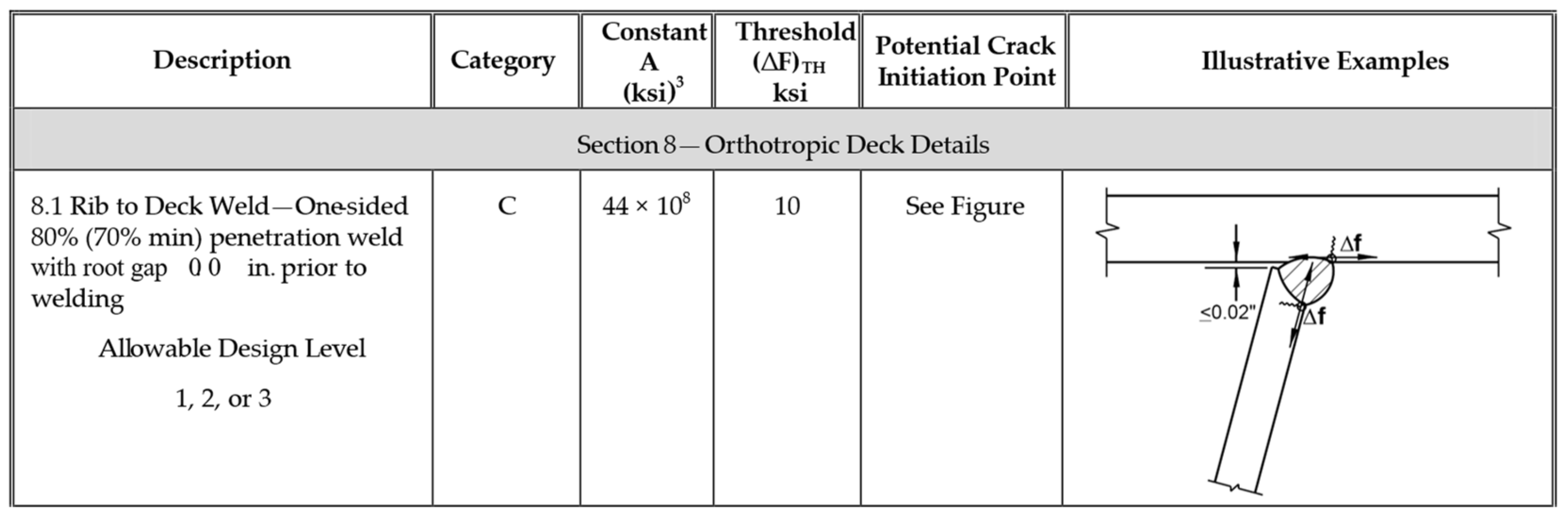
| Type of Intersection Welding | Category | |
|---|---|---|
| 1. | Rib—deck | 50, 71 |
| 2. | Rib—cross-beams | 36, 71, 80 |
| 3. | Rib or web—cross-beams | 50, 71 |
| 4. | Rib—rib | 80, 90, 112 |
| 5. | Deck plate | 80, 90, 112 |
| Assessment | Consequence | |
|---|---|---|
| Low Consequence | High Consequence | |
| Damage tolerant | 1.00 | 1.15 |
| Safe life | 1.15 | 1.35 |
| FATIGUE LOAD MODEL 4—EQUIVALENT VEHICLES | ||
|---|---|---|
| Lorry Silhouette [-] | Medium Distance [%] | Annual Traffic [n° Vehicle] |
 | 40% | 145,356 |
 | 10% | 36,339 |
 | 30% | 109,016 |
 | 15% | 54,508 |
 | 5% | 18,169 |
| TOTAL ANNUAL TRAFFIC: | 100% | 363,388 |
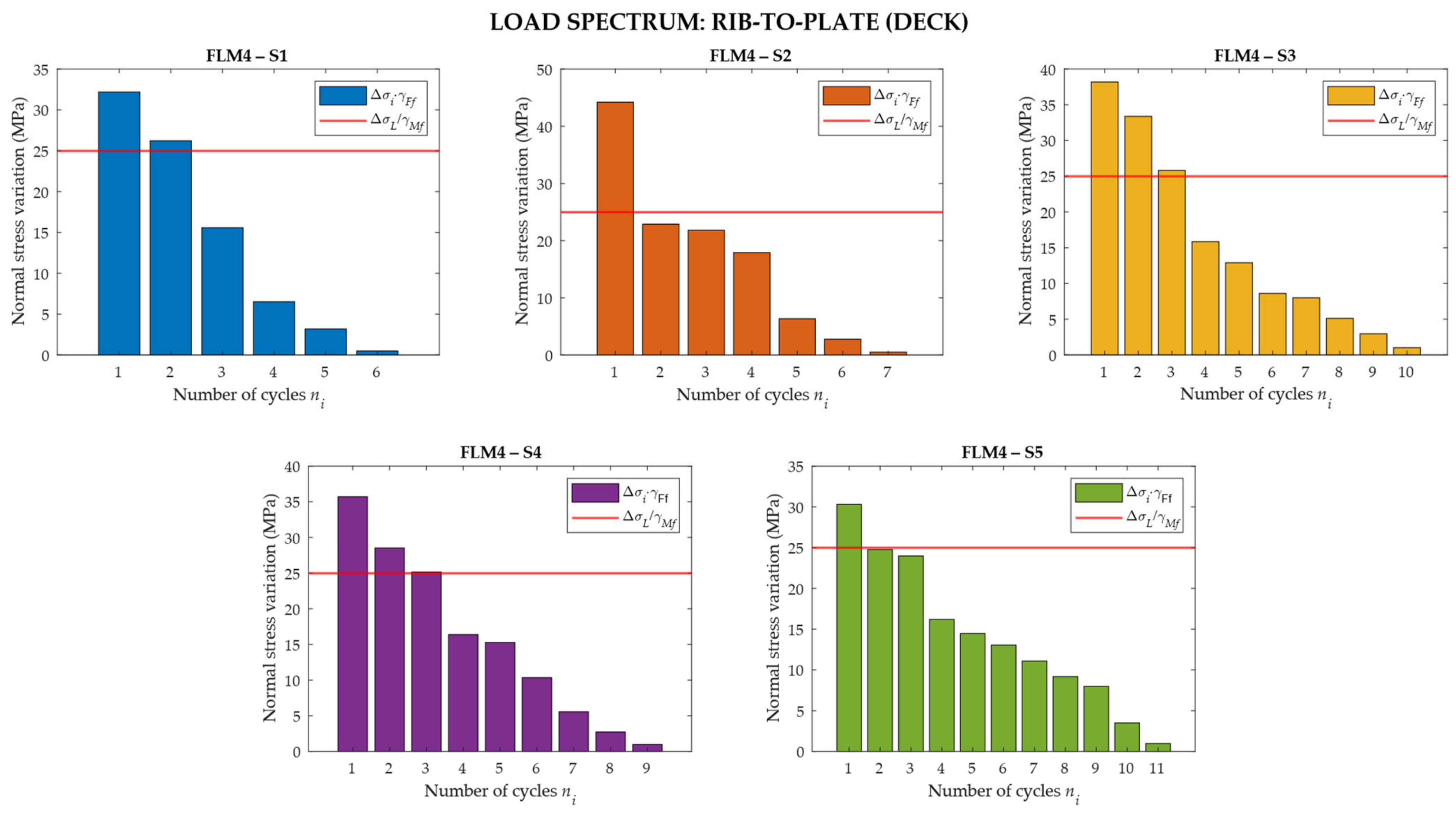
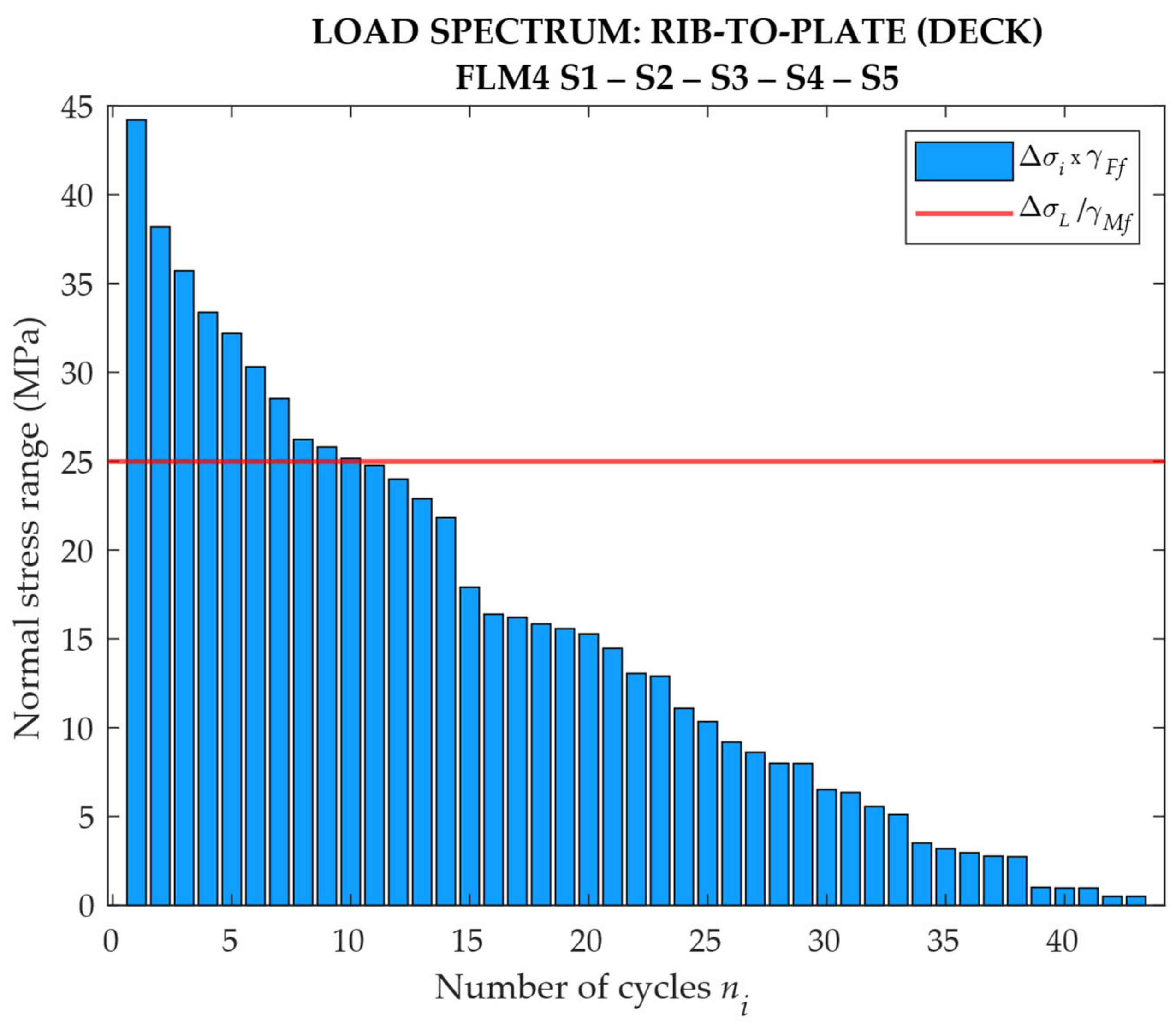
| FATIGUE LOAD MODEL 4—LORRY SILHOUETTE S1 | ||||||
|---|---|---|---|---|---|---|
| Count | Stress Range | |||||
| [-] | (MPa) | (MPa) | (-) | [Cycles] | [Cycles] | [Damage/Year] |
| 1 | 6.52 | 6.52 | - | 0 | ∞ | 0 |
| 1 | 0.50 | 0.50 | - | 0 | ∞ | 0 |
| 1 | 3.19 | 3.19 | - | 0 | ∞ | 0 |
| 1 | 15.57 | 15.57 | - | 0 | ∞ | 0 |
| 1 | 26.22 | 26.22 | 5 | 72,678 | 78,578,566 | 9.25 × 10−4 |
| 1 | 32.19 | 32.19 | 5 | 72,678 | 28,191,034 | 2.58 × 10−3 |
| 145,355 | 3.50 × 10−3 | |||||
| FATIGUE LOAD MODEL 4—LORRY SILHOUETTE S2 | ||||||
|---|---|---|---|---|---|---|
| Count | Stress Range | |||||
| [-] | (MPa) | (MPa) | (-) | [Cycles] | [Cycles] | [Damage/Year] |
| 1 | 21.82 | 21.82 | - | 0 | ∞ | 0 |
| 1 | 6.35 | 6.35 | - | 0 | ∞ | 0 |
| 1 | 0.50 | 0.50 | - | 0 | ∞ | 0 |
| 1 | 2.77 | 2.77 | - | 0 | ∞ | 0 |
| 1 | 17.91 | 17.91 | - | 0 | ∞ | 0 |
| 1 | 22.88 | 22.88 | - | 0 | ∞ | 0 |
| 1 | 44.21 | 44.21 | 5 | 36,339 | 5,769,049 | 6.30 × 10−3 |
| 36,339 | 6.30 × 10−3 | |||||
| FATIGUE LOAD MODEL 4—LORRY SILHOUETTE S3 | ||||||
|---|---|---|---|---|---|---|
| Count | Stress Range | |||||
| [-] | (MPa) | (MPa) | (-) | [Cycles] | [Cycles] | [Damage/Year] |
| 1 | 8.61 | 8.61 | - | 0 | ∞ | 0 |
| 1 | 15.84 | 15.84 | - | 0 | ∞ | 0 |
| 1 | 12.89 | 12.89 | - | 0 | ∞ | 0 |
| 1 | 1.01 | 1.01 | - | 0 | ∞ | 0 |
| 1 | 2.95 | 2.95 | - | 0 | ∞ | 0 |
| 1 | 5.11 | 5.11 | - | 0 | ∞ | 0 |
| 1 | 8.00 | 8.00 | - | 0 | ∞ | 0 |
| 1 | 25.80 | 25.80 | 5 | 36,339 | 85,261,194 | 4.26 × 10−4 |
| 1 | 33.37 | 33.37 | 5 | 36,339 | 23,527,024 | 1.54 × 10−3 |
| 1 | 38.19 | 38.19 | 5 | 36,339 | 11,996,175 | 3.03 × 10−3 |
| 109,016 | 5.00 × 10−3 | |||||
| FATIGUE LOAD MODEL 4—LORRY SILHOUETTE S4 | ||||||
|---|---|---|---|---|---|---|
| Count | Stress Range | |||||
| [-] | (MPa) | (MPa) | (-) | [Cycles] | [Cycles] | [Damage/Year] |
| 1 | 15.27 | 15.27 | - | 0 | ∞ | 0 |
| 1 | 0.98 | 0.98 | - | 0 | ∞ | 0 |
| 1 | 2.73 | 2.73 | - | 0 | ∞ | 0 |
| 1 | 5.57 | 5.57 | - | 0 | ∞ | 0 |
| 1 | 10.35 | 10.35 | - | 0 | ∞ | 0 |
| 1 | 16.38 | 16.38 | - | 0 | ∞ | 0 |
| 1 | 25.16 | 25.16 | 5 | 13,627 | 96,636,761 | 1.41 × 10−4 |
| 1 | 28.52 | 28.52 | 5 | 13,627 | 51,610,205 | 2.64 × 10−4 |
| 1 | 35.72 | 35.72 | 5 | 13,627 | 16,758,125 | 8.13 × 10−4 |
| 1 | 35.72 | 35.72 | 5 | 13,627 | 16,758,125 | 8.13 × 10−4 |
| 54,508 | 2.03 × 10−3 | |||||
| FATIGUE LOAD MODEL 4—LORRY SILHOUETTE S5 | ||||||
|---|---|---|---|---|---|---|
| Count | Stress Range | |||||
| [-] | (MPa) | (MPa) | (-) | [Cycles] | [Cycles] | [Damage/Year] |
| 1 | 11.09 | 11.09 | - | 0 | ∞ | 0 |
| 1 | 16.21 | 16.21 | - | 0 | ∞ | 0 |
| 1 | 9.19 | 9.19 | - | 0 | ∞ | 0 |
| 1 | 13.05 | 13.05 | - | 0 | ∞ | 0 |
| 1 | 0.97 | 0.97 | - | 0 | ∞ | 0 |
| 1 | 3.50 | 3.50 | - | 0 | ∞ | 0 |
| 1 | 7.99 | 7.99 | - | 0 | ∞ | 0 |
| 1 | 14.47 | 14.47 | - | 0 | ∞ | 0 |
| 1 | 23.98 | 23.98 | - | 0 | ∞ | 0 |
| 1 | 24.76 | 24.76 | - | 0 | ∞ | 0 |
| 1 | 30.31 | 30.31 | 5 | 18,169 | 38,096,333 | 4.77 × 10−4 |
| 18,169 | 4.77 × 10−4 | |||||
| EN 1993 Parte1-9—Fatigue Detail 71 | FLM4 | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| Δyears | |||||||||
| (-) | (-) | (MPa) | (MPa) | (MPa) | (MPa) | (MPa) | (MPa) | [Damage/Year] | |
| 1.00 | 1.15 | 71 | 52.31 | 28.73 | 61.74 | 45.49 | 25 | 0.0173 | 57.77 |
| Original Configuration—Group 1 R | FLM4 | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Detail ID | Detail Type | Category | Component | ID Element | Cycles | DTOT | Δ Years | Year DTOT = 1 | ||
| (MPa) | N° Total | N° Relevant | [Damage/Year] | |||||||
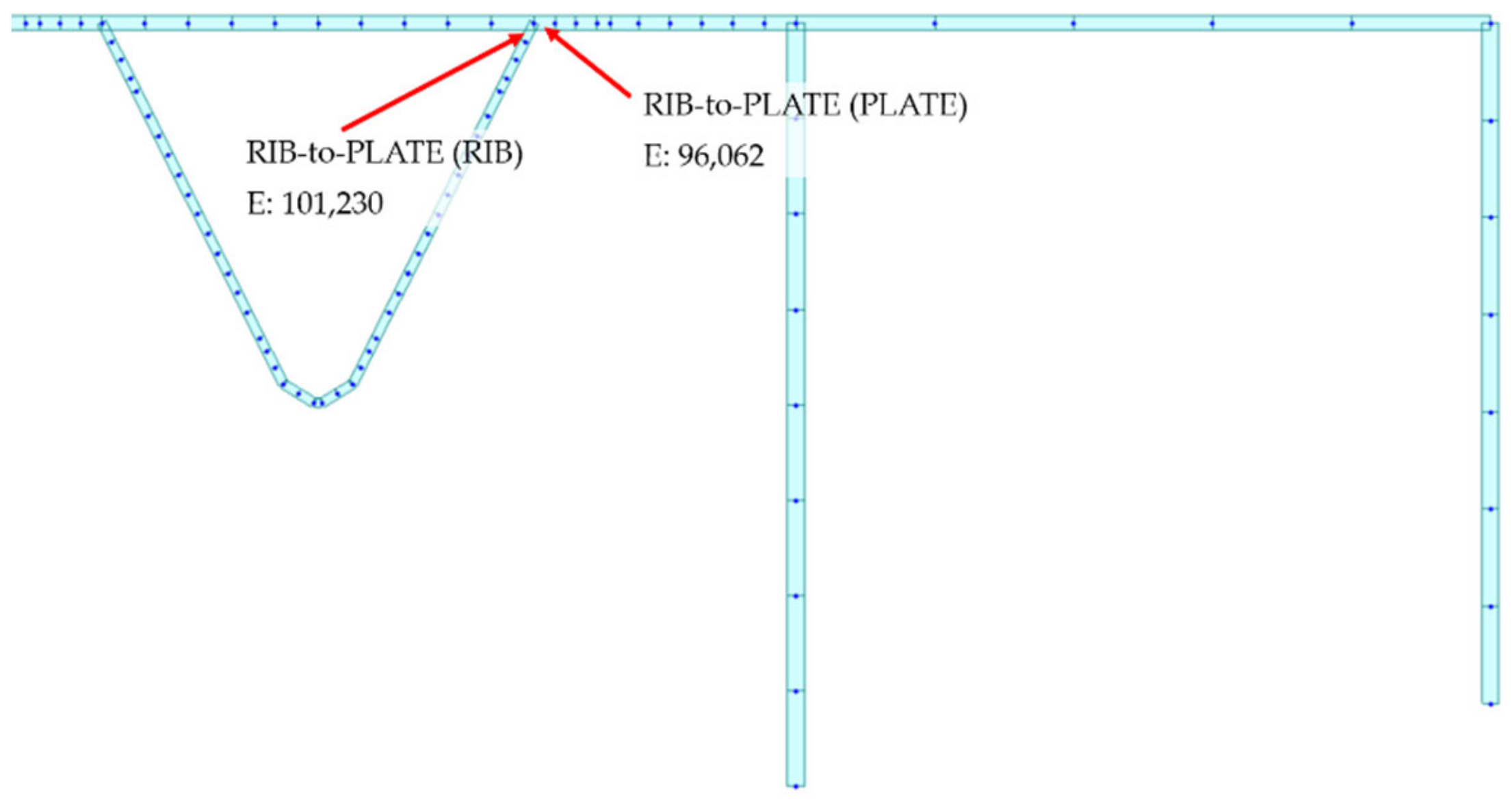
| A1 | Rib-to-Plate | 71 | Deck | Yes | 96,062 | 44 | 11 | 0.017 | 57.8 | 2052 |
| A2 | Rib | Yes | 101,230 | 27 | 9 | 0.047 | 21.1 | 2015 | ||
| B1 | Rib-to-Floor beam | 71 | Deck | Yes | 63,110 | 26 | 7 | 0.012 | 82.5 | 2077 |
| B2 | 80 | Rib | Yes | 63,356 | 36 | 0 | 0 | ∞ | ∞ | |
| Original Configuration_Group 2 (L) | Fatigue Load Model 4 | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| ID Detail | Detail | Category | Part | ||||||
| (MPa) | S1 | S2 | S3 | S4 | S5 | Tot | |||
| D1 | Rib-to-Plate | 71 | Deck | 1.45 × 105 | 3.63 × 104 | 1.09 × 105 | 5.45 × 104 | 1.82 × 104 | 3.63 × 105 |
| D2 | Rib | 0 | 0 | 0 | 0 | 0 | 0 | ||
| E | Rib-to-Floor beam | 71 | Deck | 1.45 × 105 | 3.63 × 104 | 1.09 × 105 | 5.45 × 104 | 1.82 × 104 | 3.63 × 105 |
| B2 | 80 | Rib | 0 | 0 | 1.09 × 105 | 0 | 0 | 0 | |
| G1 | Main-Girder (span) | 71 | Deck | 0 | 0 | 0 | 0 | 0 | 0 |
| G2 | M-G | 0 | 0 | 0 | 0 | 0 | 0 | ||
| H1 | Main-Girder (rib) | 71 | Deck | 0 | 0 | 0 | 0 | 0 | 0 |
| H2 | M-G | 0 | 0 | 0 | 0 | 0 | 0 | ||
| ID Detail | [Cycles] | ||||||||
| S1 | S2 | S3 | S4 | S5 | Tot | ||||
| D1 | 1.94 × 107 | 6.86 × 107 | 8.65 × 106 | 1.47 × 108 | 2.03 × 107 | 2.64 × 108 | |||
| D2 | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ | |||
| E | 2.26 × 107 | 7.36 × 107 | 9.17 × 106 | 2.80 × 107 | 2.21 × 107 | 1.55 × 108 | |||
| B2 | ∞ | ∞ | 5.90 × 107 | ∞ | ∞ | 5.90 × 107 | |||
| G1 | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ | |||
| G2 | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ | |||
| H1 | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ | |||
| H2 | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ | |||
| ID Detail | Damage [Damage/Year] | Δ Years | Year DTOT = 1 | ||||||
| S1 | S2 | S3 | S4 | S5 | Tot | ||||
| D1 | 7.49 × 10−3 | 3.21 × 10−3 | 1.26 × 10−2 | 2.62 × 10−3 | 8.96 × 10−4 | 2.68 × 10−2 | 37 | 2031 | |
| D2 | 0 | 0 | 0 | 0 | 0 | 0 | ∞ | ∞ | |
| E | 6.42 × 10−3 | 4.93 × 10−4 | 1.19 × 10−2 | 1.95 × 10−3 | 8.23 × 10−4 | 2.34 × 10−3 | 42 | 2036 | |
| B2 | 0 | 0 | 1.85 × 10−3 | 0 | 0 | 0 | 541 | 2535 | |
| G1 | 0 | 0 | 0 | 0 | 0 | 0 | ∞ | ∞ | |
| G2 | 0 | 0 | 0 | 0 | 0 | 0 | ∞ | ∞ | |
| H1 | 0 | 0 | 0 | 0 | 0 | 0 | ∞ | ∞ | |
| H2 | 0 | 0 | 0 | 0 | 0 | 0 | ∞ | ∞ | |
| Lane Reduction, Group 2 (L) | Fatigue Load Model 4 | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| ID Detail | Detail | Category | Part | ||||||
| (MPa) | S1 | S2 | S3 | S4 | S5 | Tot | |||
| D1 | Rib-to-Plate | 71 | Deck | 0 | 0 | 0 | 0 | 0 | 0 |
| D2 | Rib | 1.45 × 105 | 3.63 × 104 | 1.09 × 105 | 5.45 × 104 | 1.82 × 104 | 3.63 × 105 | ||
| E | Rib-to-Floor beam | 71 | Deck | 0 | 0 | 0 | 0 | 0 | 0 |
| B2 | 80 | Rib | 0 | 0 | 0 | 1.09 × 105 | 0 | 1.09 × 105 | |
| G1 | Main-Girder (span) | 71 | Deck | 0 | 0 | 0 | 0 | 0 | 0 |
| G2 | M-G | 0 | 0 | 0 | 0 | 0 | 0 | ||
| H1 | Main-Girder (rib) | 71 | Deck | 0 | 0 | 0 | 0 | 0 | 0 |
| H2 | M-G | 0 | 0 | 0 | 0 | 0 | 0 | ||
| ID Detail | [Cycles] | ||||||||
| S1 | S2 | S3 | S4 | S5 | Tot | ||||
| D1 | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ | |||
| D2 | 1.48 × 107 | 1.00 × 107 | 7.85 × 106 | 1.22 × 108 | 1.71 × 107 | 1.71 × 108 | |||
| E | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ | |||
| B2 | ∞ | ∞ | ∞ | 5.90 × 107 | ∞ | 5.90 × 107 | |||
| G1 | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ | |||
| G2 | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ | |||
| H1 | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ | |||
| H2 | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ | |||
| ID Detail | Damage [Damage/Year] | ||||||||
| S1 | S2 | S3 | S4 | S5 | Tot | ||||
| D1 | 0 | 0 | 0 | 0 | 0 | 0 | |||
| D2 | 9.82 × 10−3 | 3.62 × 10−3 | 1.39 × 10−2 | 3.06 × 10−3 | 1.06 × 10−3 | 3.14 × 10−2 | |||
| E | 0 | 0 | 0 | 0 | 0 | 0 | |||
| B2 | 0 | 0 | 0 | 1.85 × 10−3 | 0 | 0 | |||
| G1 | 0 | 0 | 0 | 0 | 0 | 0 | |||
| G2 | 0 | 0 | 0 | 0 | 0 | 0 | |||
| H1 | 0 | 0 | 0 | 0 | 0 | 0 | |||
| H2 | 0 | 0 | 0 | 0 | 0 | 0 | |||
| Detail | Category | Component | Original Configuration | New Barriers and Lane Reduction | Full Load History | ||
|---|---|---|---|---|---|---|---|
| Damage 1994 ÷ 2019 | Yearly Damage since 2019 | Damage since 1994 | |||||
| ID | (MPa) | [Total Damage] | [Damage/Year] | Δ Years | [Final Damage] | Year DTOT = 1 | |
| D1 | 71 | Deck | 6.97 × 10−1 | 0 | ∞ | 6.97 × 10−1 | ∞ |
| D2 | Rib | 0 | 3.14 × 10−2 | 32 | 1.00 | 2051 | |
| E | 71 | Deck | 6.26 × 10−1 | 0 | ∞ | 6.26 × 10−1 | ∞ |
| B2 | 80 | Rib | 4.80 × 10−2 | 1.85 × 10−3 | 541 | 1.00 | 2535 |
| G1 | 71 | Deck | 0 | 0 | ∞ | 0 | ∞ |
| G2 | M-G | 0 | 0 | ∞ | 0 | ∞ | |
| H1 | 71 | Deck | 0 | 0 | ∞ | 0 | ∞ |
| H2 | M-G | 0 | 0 | ∞ | 0 | ∞ | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mairone, M.; Asso, R.; Masera, D.; Invernizzi, S.; Montagnoli, F.; Carpinteri, A. Fatigue Performance Analysis of an Existing Orthotropic Steel Deck (OSD) Bridge. Infrastructures 2022, 7, 135. https://doi.org/10.3390/infrastructures7100135
Mairone M, Asso R, Masera D, Invernizzi S, Montagnoli F, Carpinteri A. Fatigue Performance Analysis of an Existing Orthotropic Steel Deck (OSD) Bridge. Infrastructures. 2022; 7(10):135. https://doi.org/10.3390/infrastructures7100135
Chicago/Turabian StyleMairone, Mattia, Rebecca Asso, Davide Masera, Stefano Invernizzi, Francesco Montagnoli, and Alberto Carpinteri. 2022. "Fatigue Performance Analysis of an Existing Orthotropic Steel Deck (OSD) Bridge" Infrastructures 7, no. 10: 135. https://doi.org/10.3390/infrastructures7100135
APA StyleMairone, M., Asso, R., Masera, D., Invernizzi, S., Montagnoli, F., & Carpinteri, A. (2022). Fatigue Performance Analysis of an Existing Orthotropic Steel Deck (OSD) Bridge. Infrastructures, 7(10), 135. https://doi.org/10.3390/infrastructures7100135







