An Experimental Approach towards Motion Modeling and Control of a Vehicle Transiting a Non-Newtonian Environment
Abstract
:1. Introduction
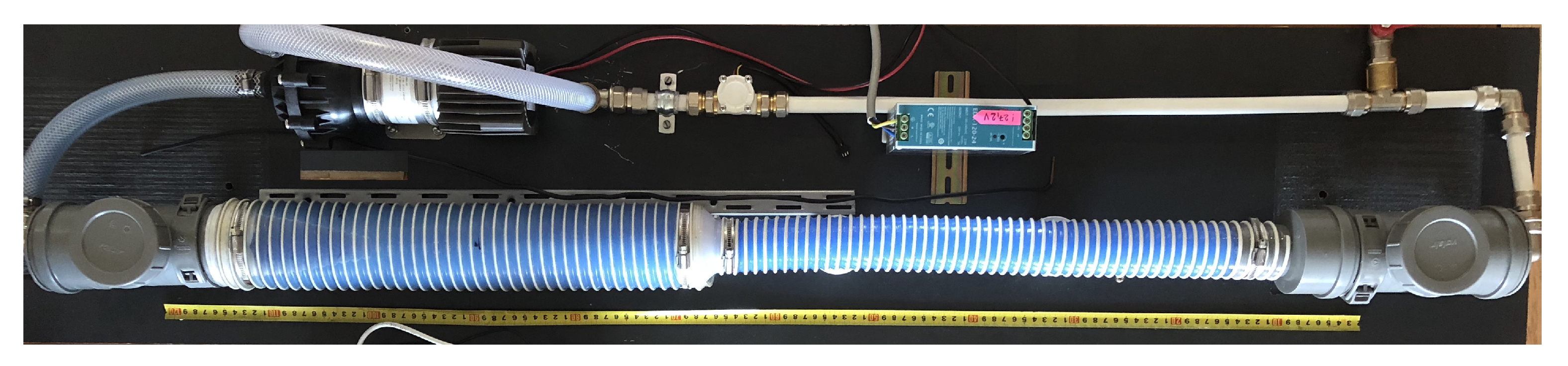
2. Experimental Non-Newtonian Environment Framework
2.1. Non-Newtonian Cardiovascular Homology
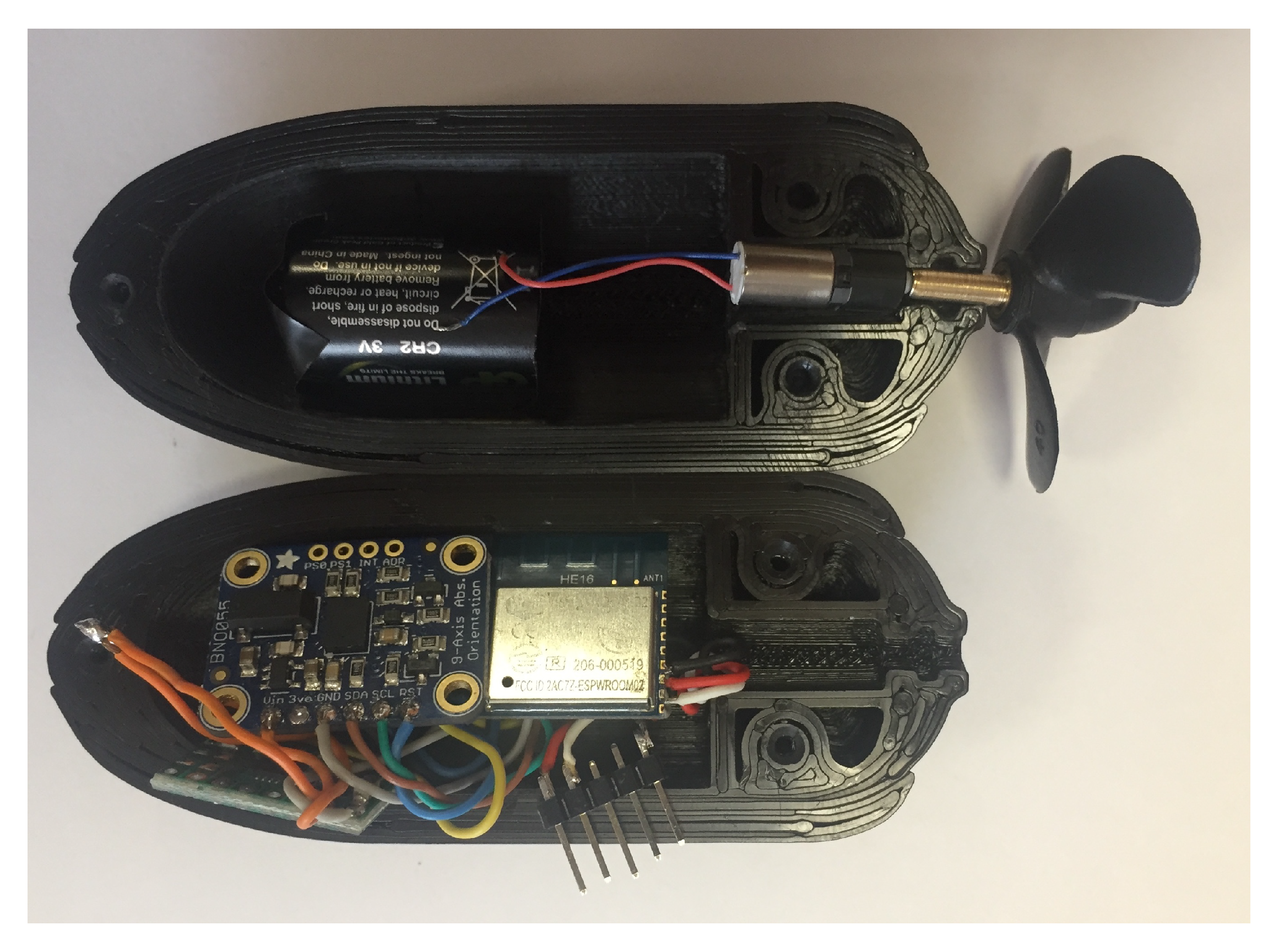
2.2. Autonomous Submerged Vehicle
2.3. Environmental Impedance Variation Detection
2.4. Submersible Miniaturization Possibilities
3. Motion Dynamics Modeling for Submerged Objects in a Non-Newtonian Environment
3.1. Developing a General Navigation Model for Submerged Objects in a Newtonian Setting
3.2. Newtonian Model Calibration towards Non-Newtonian Feature Mitigation
4. Motion Controller Development
5. Experimental Validation of the Proposed Methodology
5.1. Dedicated Model for the Autonomous Submersible’s Motion in the Cardiovascular Replica
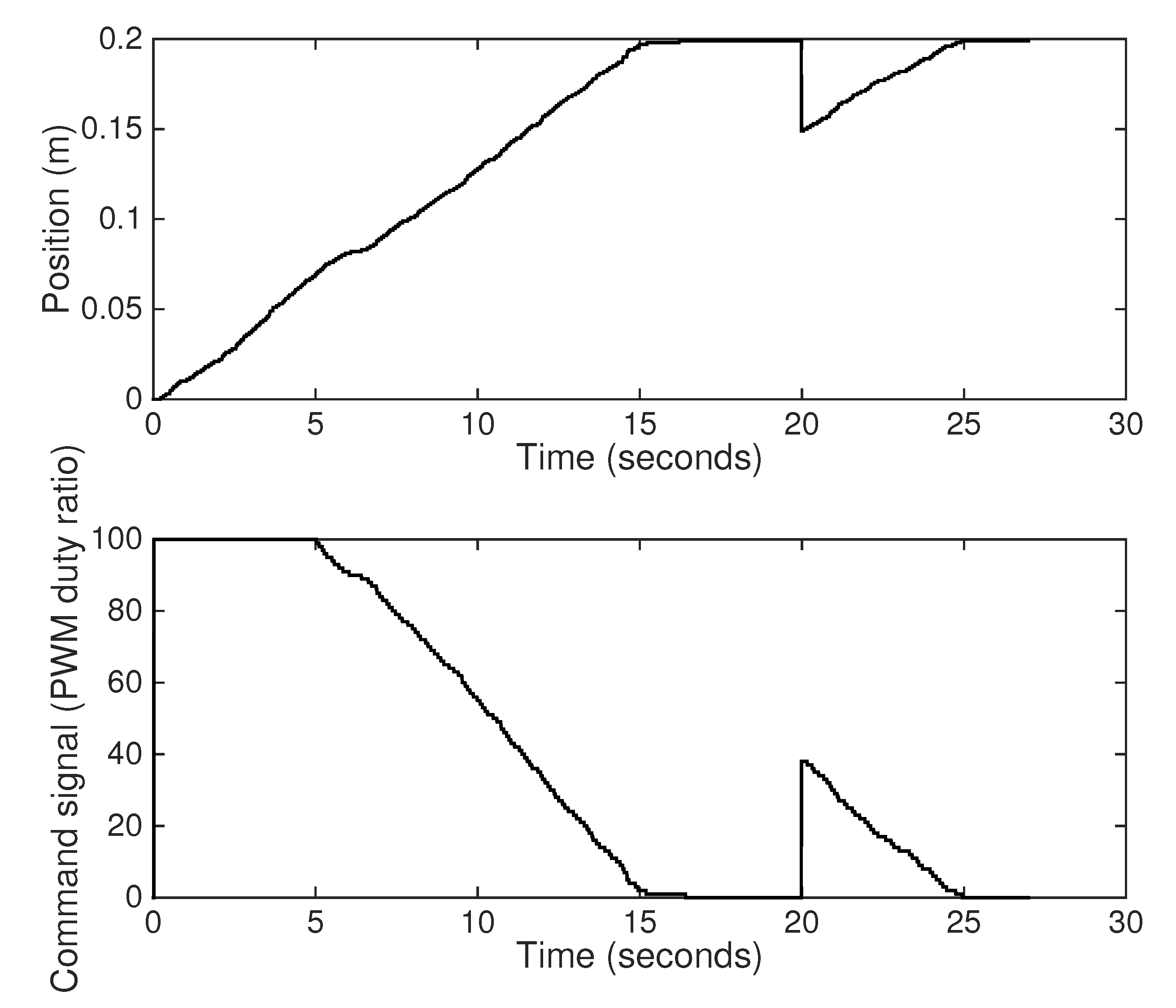
5.2. Controlling the Vehicle’s Position
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
Appendix A. Software Development for Data Processing and Motion Control
| Algorithm 1: An insight into the submersible’s software logic |
 |
Appendix B. Impedance Variation in the Non-Newtonian Fluid


References
- Berglundand, A.; Liddle, J.A. Simultaneous positioning and orientation of a single nano-object by flow control: Theory and simulations. New J. Phys. 2011, 13, 27. [Google Scholar]
- Fahmy, T.M.; Fong, P.M.; Goyal, A.; Saltzman, W.M. Targeted for drug delivery. Mater. Today 2005, 8, 18–26. [Google Scholar] [CrossRef]
- Singh, R.; Lillard, J.W. Nanoparticle-based targeted drug delivery. Exp. Mol. Pathol. 2009, 86, 215–223. [Google Scholar] [CrossRef] [Green Version]
- Freeman, A.; Mayhew, E. Targeted drug delivery. Cancer 1986, 58, 573–583. [Google Scholar] [CrossRef]
- Li, H.; Tan, J.; Zhang, M. Dynamics Modeling and Analysis of a Swimming Microrobot for Controlled Drug Delivery. IEEE Trans. Autom. Sci. Eng. 2009, 6, 220–227. [Google Scholar]
- Ionescu, C.M. A memory-based model for blood viscosity. Commun. Nonlinear Sci. Numer. Simul. 2017, 45, 29–34. [Google Scholar] [CrossRef]
- Ionescu, C.M.; De Keyser, R. Time domain validation of a fractional order model for human respiratory system. In Proceedings of the IEEE Mediterranean Electrotechnical Conference-MELECON, Ajaccio, France, 5–7 May 2008; pp. 89–95. [Google Scholar]
- Ionescu, C.; Lopes, A.; Copot, D.; Machado, J.A.T.; Bates, J.H.T. The role of fractional calculus in modeling biological phenomena: A review. Commun. Nonlinear Sci. Numer. Simul. 2017, 51, 141–159. [Google Scholar] [CrossRef]
- Atanaga, A. Application of Fractional Calculus to Epidemiology. Fractional Dynamics. 2016. Available online: https://www.degruyter.com/document/doi/10.1515/9783110472097-011/html (accessed on 24 August 2021).
- Almeida, R.; Brito da Cruz, A.; Martins, N.; Monteiro, M. An epidemiological MSEIR model described by the Caputo fractional derivative. Int. J. Dyn. Control. 2019, 7, 776–784. [Google Scholar] [CrossRef] [Green Version]
- Al-Sulami, H.; El-Shahed, M.; Nieto, J.J.; Shammakh, W. On Fractional Order Dengue Epidemic Model. Math. Probl. Eng. 2014, 2014, 456537. [Google Scholar] [CrossRef]
- Pinto, C.M.; Carvalho, A.R. Fractional complex-order model for HIV infection with drug resistance during therapy. J. Vib. Control. 2016, 22, 2222–2239. [Google Scholar] [CrossRef]
- Birs, I.; Muresan, C.; Copot, D.; Nascu, I.; Ionescu, C. Identification for control of suspended objects in non-Newtonian fluids. Fract. Calc. Appl. Anal. 2019, 22, 1378–1394. [Google Scholar] [CrossRef]
- Wijngaard, R.R.; Steiner, J.F.; Kraaijenbrink, P.D.A.; Klug, C.; Adhikari, S.; Banerjee, A.; Pellicciotti, F.; van Beek, L.P.H.; Bierkens, M.F.P.; Lutz, A.F.; et al. Modeling the response of the Langtang glacier and the Hintereisferner to a changing climate since the Little Ice Age. Front. Earth Sci. 2019, 7, 143. [Google Scholar] [CrossRef]
- Langevin, C.D. Modeling axisymmetric flow and transport. Ground Water 2008, 46, 579–590. [Google Scholar] [CrossRef]
- Ye, Z.; Zhang, Y.; Wang, Y.; Huang, Z.; Song, B. Chest CT manifestations of new coronavirus disease 2019 (COVID-19): A pictorial review. Eur. Radiol. 2020, 30, 4381–4389. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mason, R.J. Pathogenesis of COVID-19 from a cell biology perspective. Eur. Respir. J. 2020, 55, 2000607. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gomez-Espinosa, A.; Cuan-Urquizo, E.; González-García, J. Autonomous Underwater Vehicles: Localization, Navigation, and Communication for Collaborative Missions. Appl. Sci. 2020, 10, 2020. [Google Scholar]
- Koutsiaris, G. A velocity profile equation for blood flow in small arterioles and venules of small mammals in vivo and an evaluation based on literature data. Clin. Hemorheol. Microcirc. 2019, 43, 321–334. [Google Scholar] [CrossRef]
- Womersley, J.R. An Elastic Tube Theory of Pulse Transmission and Oscillatory Flow in Mammalian Arteries; Tech. Rep. WADC-TR56-614; Wright Air Development Center: Dayton, OH, USA, 1957. [Google Scholar]
- Coffman, J.D.; Lempert, J.A. Venous flow velocity, venous volume and arterial blood flow. Circulation 1975, 52, 141–145. [Google Scholar] [CrossRef] [Green Version]
- Wynn, R.B. Autonomous Underwater Vehicles (AUVs): Their past, present and future contributions to the advancement of marine geoscience. Mar. Geol. 2004, 352, 451–468. [Google Scholar] [CrossRef] [Green Version]
- Birs, I.; Muresan, C.; Folea, S.; Prodan, O. An experimental nanomedical platform for controller validation on targeted drug delivery. In Proceedings of the 2017 Australian and New Zealand Control Conference (ANZCC), Gold Coast, QLD, Australia, 17–20 December 2017; pp. 161–165. [Google Scholar]
- Xiao, C.; Lachance, B.; Sunahara, G.; Luong, J.H.T. Assessment of Cytotoxicity Using Electric Cell-Substrate Impedance Sensing: Concentration and Time Response Function Approach Analytical. Chemistry 2002, 74, 5748–5753. [Google Scholar] [CrossRef]
- Ren, d.; Chui, C.O. Feasibility of Tracking Multiple Single-Cell Properties with Impedance Spectroscopy. ACS Sens. 2018, 3, 1005–1015. [Google Scholar] [CrossRef]
- Sene, A.; Khan, A.A.; Cox, D.; Nakamura, R.E.I.; Santeford, A.; Kim, B.M.; Sidhu, R.; Onken, M.D.; Harbour, J.W.; Hagbi-Levi, S.; et al. Impaired cholesterol efflux in senescent macrophages promotes age-related macular degeneration. Cell Metab. 2013, 17, 549–561. [Google Scholar] [CrossRef] [Green Version]
- Birs, I.R.; Ghita, M.; Ghita, M.; Copot, D.; Muresan, C.I.; Ionescu, C.M. An interdisciplinary, low-cost methodological framework for analysing dynamical material properties for control-related applications. IFAC Pap. 2019, 52, 159–164. [Google Scholar] [CrossRef]
- Siwatch, P.; Sharma, K.; Arora, A.; Tripathi, S.K. Review of supercapacitors: Materials and devices. J. Energy Storage 2019, 21, 801–825. [Google Scholar]
- Sidebottom, M.A.; Pitenis, A.A.; Junk, C.P.; Kasprzak, D.J.; Blackman, G.S.; Burch, H.E.; Harris, K.L.; Sawyer, W.G.; Krick, B.A. Ultralow wear Perfluoroalkoxy (PFA) and alumina composites. Wear 2016, 362, 179–185. [Google Scholar] [CrossRef] [Green Version]
- Tajmar, M.; Kößling, M.; Weikert, M.; Monette, M. The SpaceDrive Project—First Results on EMDrive and Mach-Effect Thrusters. In Proceedings of the Space Propulsion Conference, Seville, Spain, 14–18 May 2018. [Google Scholar]
- Zhao, Y.; Liu, Y.; Wen, G.; Ren, W.; Chen, G. Designing Distributed Specified-Time Consensus Protocols for Linear Multiagent Systems Over Directed Graphs. IEEE Trans. Autom. Control. 2019, 64, 2945–2952. [Google Scholar] [CrossRef]
- Pozo, B.G.; Garate, J.I.; Araujo, J.A.; Ferreiro, S. Energy Harvesting Technologies and Equivalent Electronic Structural Models—Review. Electronics 2018, 8, 486. [Google Scholar] [CrossRef] [Green Version]
- Pandikumar, A.; Rameshkumar, P. Graphene-Based Electrochemical Sensors for Biomolecules; Elsevier: Amsterdam, The Netherlands, 2017. [Google Scholar]
- Li, Y.; Chen, Y.Q.; Podlubny, I. Mittag—Leffler stability of fractional order nonlinear dynamic systems. Automatica 2009, 45, 1965–1969. [Google Scholar] [CrossRef]
- Li, Y.; Chen, Y.Q.; Podlubny, I. Stability of fractional-order nonlinear dynamic systems: Lyapunov direct method and generalized Mittag–-Leffler stability. Comput. Math. Appl. 2010, 59, 1810–1821. [Google Scholar] [CrossRef] [Green Version]
- Ionescu, C.M.; Kelly, J.F. Fractional calculus for respiratory mechanics: Power law impedance, viscoelasticity and tissue heterogeneity. Chaos Solitons Fractals 2017, 102, 433–440. [Google Scholar] [CrossRef]
- Silvatti, A.P.; Cerveri, P.; Telles, T.; Dias, F.A.S.; Baroni, G.; Barros, R.M.O. Quantitative underwater 3D motion analysis using submerged video cameras: Accuracy analysis and trajectory reconstruction. Comput. Methods Biomech. Biomed. Eng. 2002, 16, 1240–1248. [Google Scholar] [CrossRef] [Green Version]
- Dilen, D.R. The motion of floating and submerged objects in the Chattahoochee River, Atlanta, GA. J. Forensic Sci. 1984, 29, 1027–1037. [Google Scholar] [CrossRef]
- Jones, D.A.; Clarke, D.B.; Brayshaw, I.B.; Barillon, J.L.; Anderson, B. The Calculation of Hydrodynamic Coefficients for Underwater Vehicles. 2002. Available online: https://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.589.9638&rep=rep1&type=pdf (accessed on 24 August 2021).
- Bachmeyer, R.; Whitcomb, L.L.; Grosenbaugh, M.A. An accurate four quadrant nonlinear dynamical model for marine thrusters: Theory and experimental validation. IEEE J. Ocean. Eng. 2000, 25, 146–158. [Google Scholar] [CrossRef]
- Le Page, Y.G. Hydrodynamics and Control of an Autonomous Underwater Vehicle Equipped with a Vectored Thruster; Florida Atlantic University: Boca Raton, FL, USA, 2000. [Google Scholar]
- Fossen, T.I. Marine Control Systems Guidance, Navigation, and Control of Ships, Rigs and Underwater Vehicles; Marine Cybernetics: Trondheim, Norway, 2002. [Google Scholar]
- Carlton, J.S. Marine Propellers and Propulsion; Butterworth-Heinemann: Oxford, UK, 1994; pp. 95–115. [Google Scholar]
- Birs, I.; Muresan, C.; Prodan, O.; Folea, S.; Ionescu, C.M. Analytical modeling and preliminary fractional order velocity control of a small scale submersible. In Proceedings of the 2018 SICE International Symposium on Control Systems (SICE ISCS), Tokyo, Japan, 9–11 March 2018; pp. 157–162. [Google Scholar]
- Snyman, J.A. An Introduction to Basic Optimization Theory and Classical and New Gradient-Based Algorithms; Practical Mathematical Optimization; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Pardalos, P.M.; Resende, M.G.C. Handbook of Applied Optimization; Oxford University Press: Oxford, UK, 2002. [Google Scholar]
- Watanabe, T.; Yoshikawa, T. Grasping Optimization Using a Required External Force Set. IEEE Trans. Autom. Sci. Eng. 2007, 4, 52–66. [Google Scholar] [CrossRef]
- Vilanova, R.; Visioli, A. PID Control in the Third Millennium; Springer: London, UK, 2012. [Google Scholar]
- Machado, J.A.T.; Galhao, A.M.S.F.; Trujillo, J.J. On development of fractional calculus during the last fifty years. Scientometrics 2014, 98, 577–582. [Google Scholar] [CrossRef] [Green Version]
- Jifeng, W.; Yuankai, L. Frequency domain analysis and applications for fractional-order control systems. J. Phys. Conf. Ser. 2005, 13, 268. [Google Scholar] [CrossRef]
- Magin, R.; Vinagre, B.; Podlubny, I. Can Cybernetics and Fractional Calculus Be Partners? Searching for New Ways to Solve Complex Problems. IEEE Syst. Man Cybern. Mag. 2018, 4, 23–28. [Google Scholar] [CrossRef]
- Liu, H.; Pan, Y.; Li, S.; Chen, Y. Adaptive Fuzzy Backstepping Control of Fractional-Order Nonlinear Systems. IEEE Trans. Syst. Man Cybern. Syst. 2017, 47, 2209–2217. [Google Scholar] [CrossRef]
- Bugliarello, G.; Sevilla, J. Velocity distribution and other characteristics of steady and pulsatile blood flow in fine glass tubes. Biorheology 1970, 7, 85–107. [Google Scholar] [CrossRef]
- Srivastava, N. Analysis of Flow Characteristics of the Blood Flowing through an Inclined Tapered Porous Artery with Mild Stenosis under the Influence of an Inclined Magnetic Field. J. Biophys. 2014, 2014. [Google Scholar] [CrossRef]
- Beschi, M.; Padula, F.; Visioli, A. The generalised isodamping approach for robust fractional PID controllers design. Int. J. Control. 2015, 90, 1157–1164. [Google Scholar] [CrossRef]
- Muresan, C.; Birs, I.; Ionescu, C.; De Keyser, R. Tuning of fractional order proportional integral/proportional derivative controllers based on existence conditions. Proc. Inst. Mech. Eng. Part I J. Syst. Control. Eng. 2018, 233, 384–391. [Google Scholar] [CrossRef]
- Oustaloup, A.; Levron, F.; Mathieu, B.; Nanot, F.M. Frequency-Band Complex Noninteger Differentiator: Characterization and Synthesis. IEEE Trans. Circuits Syst.-Fundam. Theory Appl. 2020, 2020, 25–39. [Google Scholar] [CrossRef]
- Tricaud, C.; Chen, Y.Q. An approximate method for numerically solving fractional order optimal control problems of general form. Comput. Math. Appl. 2010, 59, 1644–1655. [Google Scholar] [CrossRef] [Green Version]
- De Keyser, R.; Muresan, C.I.; Ionescu, C. An efficient algorithm for low-order direct discrete-time implementation of fractional order transfer functions. ISA Trans. 2018, 74, 229–238. [Google Scholar] [CrossRef]
- Blevins, R.D. Formulas for Natural Frequency and Mode Shape; Kreiger Publishing: Malabar, FL, USA, 1979. [Google Scholar]
- Wu, W.T.; Massoudi, M. Recent Advances in Mechanics of Non-Newtonian Fluids; MDPI: Basel, Switzerland, 2020. [Google Scholar]






| Parameter | Value |
|---|---|
| 0.3 | |
| 0.6 | |
| d | 0.04 m |
| r | 0.02 m |
| A | 0.0013 m |
| 1060 kg/m | |
| 0.031 m | |
| 0.2473 | |
| V | 0.1 m/s |
| m | 0.1 kg |
| 1 m/s |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Birs, I.; Muresan, C.; Prodan, O.; Folea, S.; Ionescu, C. An Experimental Approach towards Motion Modeling and Control of a Vehicle Transiting a Non-Newtonian Environment. Fractal Fract. 2021, 5, 104. https://doi.org/10.3390/fractalfract5030104
Birs I, Muresan C, Prodan O, Folea S, Ionescu C. An Experimental Approach towards Motion Modeling and Control of a Vehicle Transiting a Non-Newtonian Environment. Fractal and Fractional. 2021; 5(3):104. https://doi.org/10.3390/fractalfract5030104
Chicago/Turabian StyleBirs, Isabela, Cristina Muresan, Ovidiu Prodan, Silviu Folea, and Clara Ionescu. 2021. "An Experimental Approach towards Motion Modeling and Control of a Vehicle Transiting a Non-Newtonian Environment" Fractal and Fractional 5, no. 3: 104. https://doi.org/10.3390/fractalfract5030104
APA StyleBirs, I., Muresan, C., Prodan, O., Folea, S., & Ionescu, C. (2021). An Experimental Approach towards Motion Modeling and Control of a Vehicle Transiting a Non-Newtonian Environment. Fractal and Fractional, 5(3), 104. https://doi.org/10.3390/fractalfract5030104










