A Mathematical Study of a Coronavirus Model with the Caputo Fractional-Order Derivative
Abstract
:1. Introduction
2. Material and Methods
3. Results
3.1. Existence and Uniqueness
3.2. Non-Negativity and Boundedness
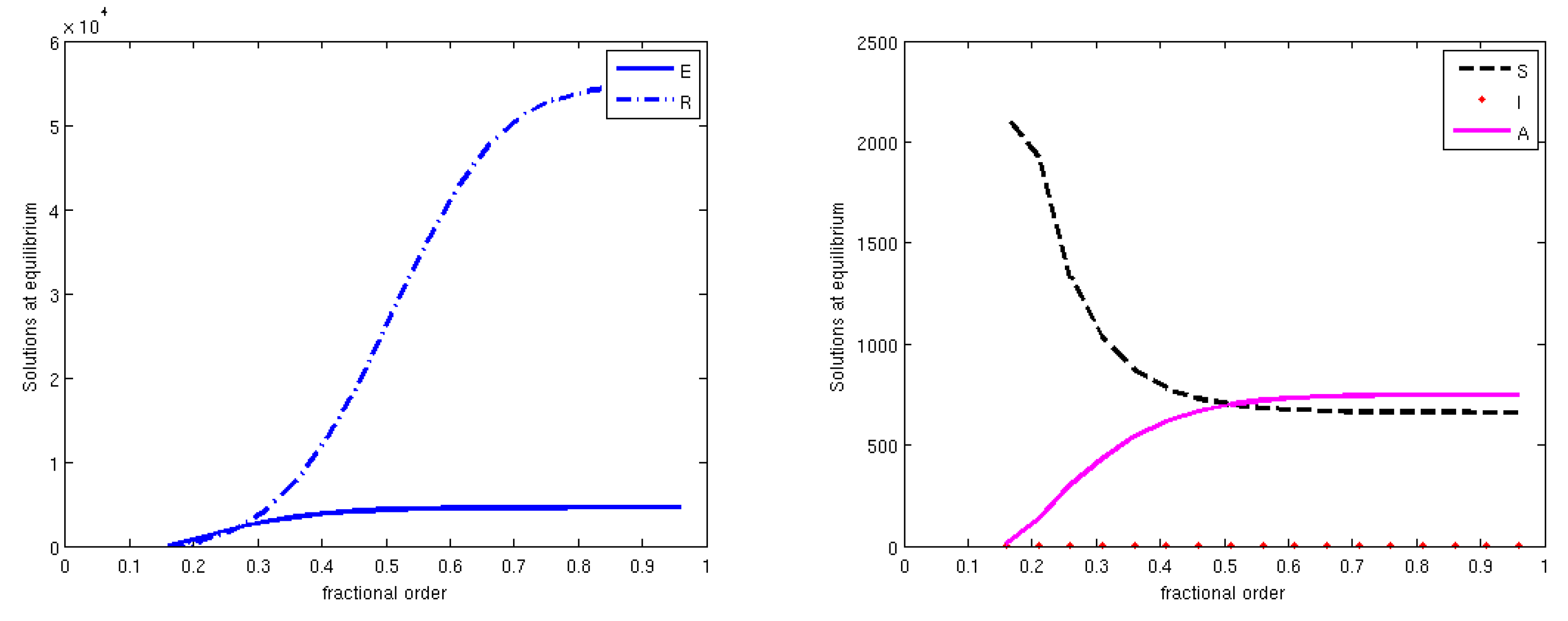
3.3. Equilibrium Points and Local Stability
- The coronavirus-free equilibrium , where . It is always feasible.
- The coronavirus-symptomatic-infected-free equilibrium , which is feasible ifwhereand
- The coronavirus endemic equilibrium , which is feasible ifwhere
3.4. The Basic Reproduction Number
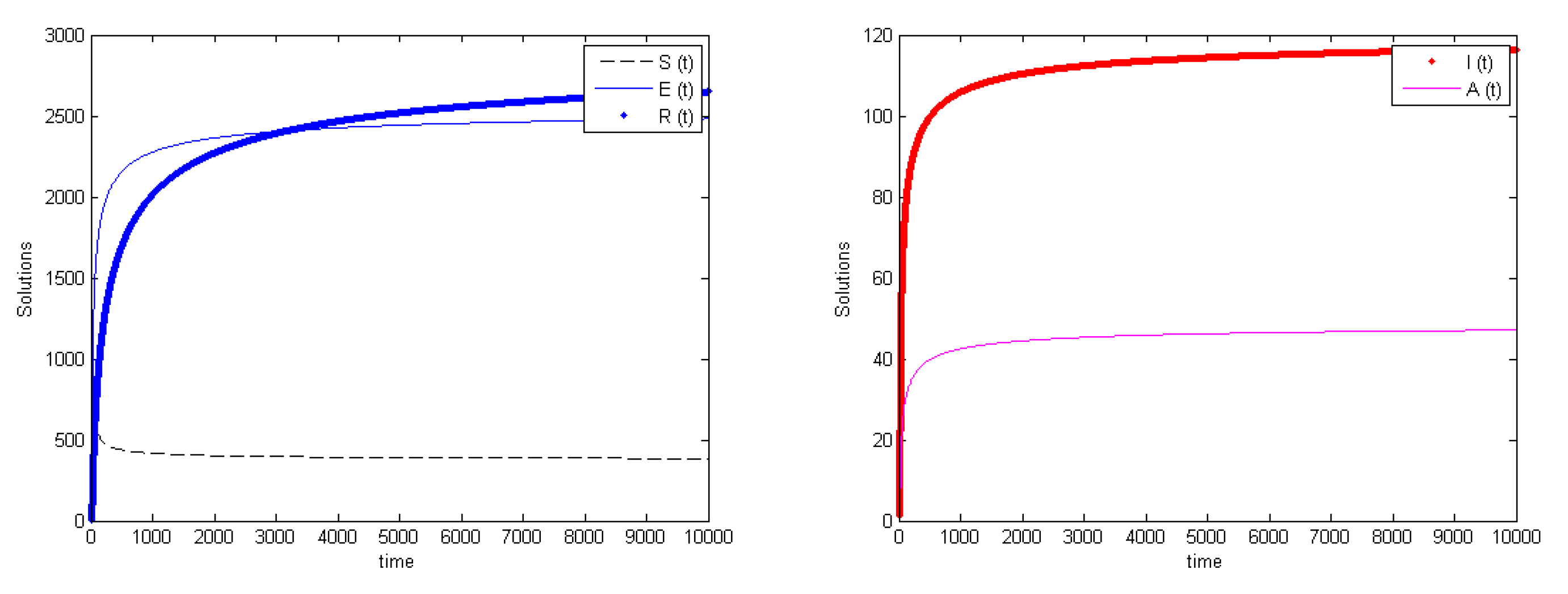
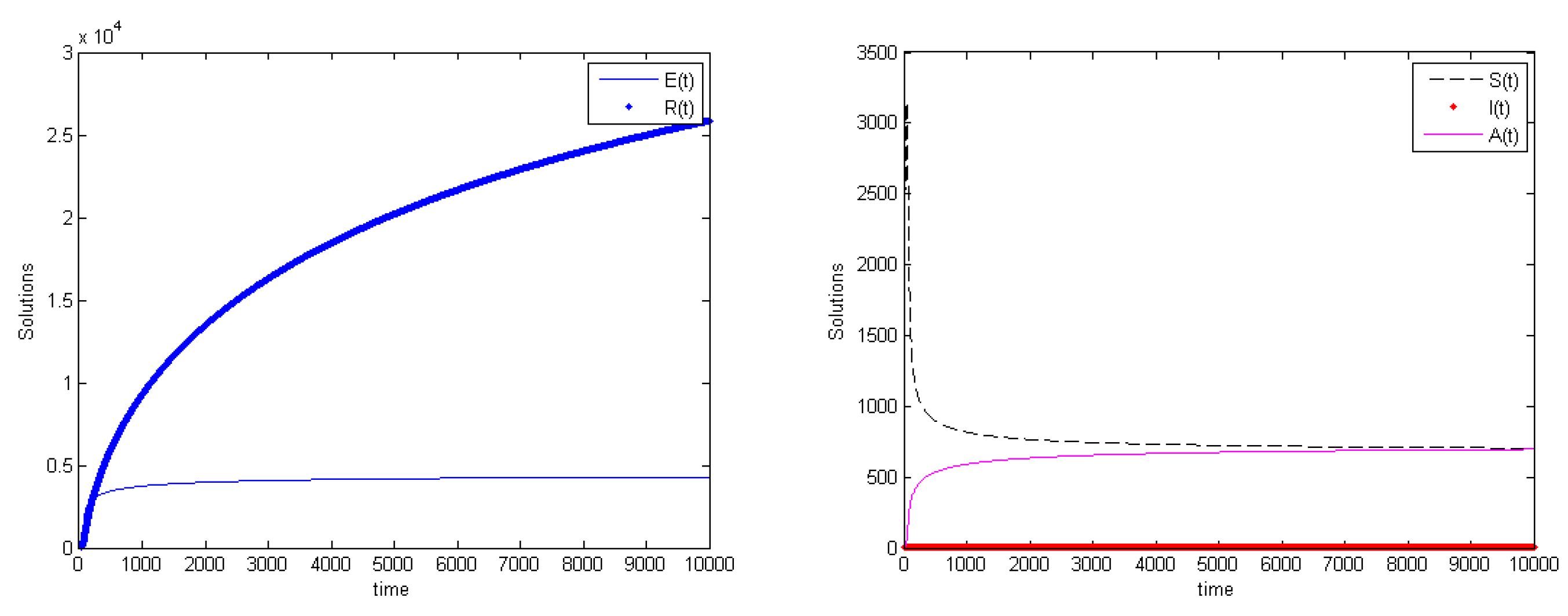
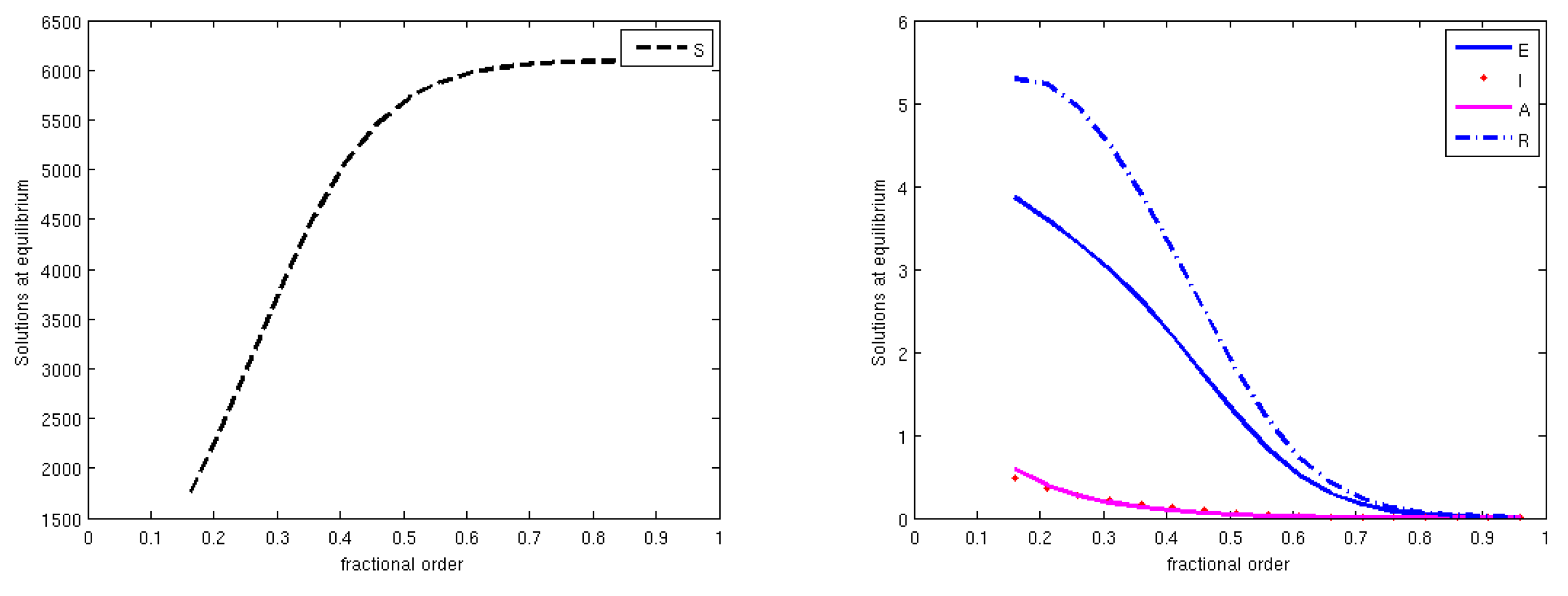
3.5. Global Stability
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Diethelma, K.; Ford, N.J. Analysis of Fractional Differential Equations. J. Math. Anal. Appl. 2002, 265, 229–248. [Google Scholar] [CrossRef] [Green Version]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; Elsevier: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Podlubny, I. Fractional Differential Equations; Academic Press: London, UK, 1999. [Google Scholar]
- Petras, I. Fractional-Order Nonlinear Systems: Modeling, Analysis and Simulation; Springer: Berlin, Germany, 2011. [Google Scholar]
- Caputo, M. Elasticità e dissipazione (Elasticity and Dissipation); Zanichelli: Bologna, Italy, 1969. [Google Scholar]
- Li, H.L.; Zhang, L.; Hu, C.; Jiang, Y.L.; Teng, Z. Dynamical analysis of a fractional-order predator-prey model incorporating a prey refuge. J. Appl. Math. Comput. 2017, 54, 435–449. [Google Scholar] [CrossRef]
- Nugraheni, K.; Trisilowati, T.; Suryanto, A. Dynamics of a fractional order eco-epidemiological model. J. Trop. Life Sci. 2017, 7, 243–250. [Google Scholar] [CrossRef] [Green Version]
- Ahmad, Z.; Arif, M.; Ali, F.; Khan, I.; Nisar, K.S. A report on COVID-19 epidemic in Pakistan using SEIR fractional model. Sci. Rep. 2020, 10, 22268. [Google Scholar] [CrossRef]
- Panwar, V.S.; Uduman, P.S.S.; Gómez-Aguilar, J.F. Mathematical modeling of coronavirus disease COVID-19 dynamics using CF and ABC non-singular fractional derivatives. Chaos Solitons Fractals 2021, 145, 110757. [Google Scholar] [CrossRef]
- Kumar, P.; Erturk, V.S. The analysis of a time delay fractional COVID-19 model via Caputo type fractional derivative. Math. Methods Appl. Sci. 2020, 1–14. [Google Scholar] [CrossRef]
- Ogunrinde, R.B.; Nwajeri, U.K.; Fadugba, S.E.; Ogunrinde, R.R.; Oshinubi, K.I. Dynamic model of COVID-19 and citizens reaction using fractional derivative. Alex. Eng. J. 2021, 60, 2001–2012. [Google Scholar] [CrossRef]
- Bahloul, M.A.; Chahid, A.; Laleg-Kirati, T.-M. Fractional-Order SEIQRDP Model for Simulating the Dynamics of COVID-19 Epidemic. IEEE Open J. Eng. Med. Biol. 2020, 1, 249–256. [Google Scholar] [CrossRef]
- Peter, O.J.; Shaikh, A.S.; Ibrahim, M.O.; Nisar, K.S.; Baleanu, D.; Khan, I.; Abioye, A.I. Analysis and Dynamics of Fractional Order Mathematical Model of COVID-19 in Nigeria Using Atangana-Baleanu Operator. Comput. Mater. Contin. 2021, 145, 1823–1848. [Google Scholar] [CrossRef]
- Atangana, A.; Araz, S.İ. Mathematical model of COVID-19 spread in Turkey and South Africa: Theory, methods and applications. Adv. Differ. Equ. 2020, 2020, 659. [Google Scholar] [CrossRef]
- Atangana, A.; Araz, S.İ. A novel COVID-19 model with fractional differential operators with singular and non-singular kernels: Analysis and numerical scheme based on Newton polynomial. Alex. Eng. J. 2021, 60, 3781–3806. [Google Scholar] [CrossRef]
- Buonomo, B. Effects of information-dependent vaccination behavior on coronavirus outbreak: Insights from a SIRI model. Ric. Mat. 2020, 69, 483–499. [Google Scholar] [CrossRef] [Green Version]
- Kassa, S.M.; Njagarah, J.B.H.; Terefe, Y.A. Analysis of the mitigation strategies for COVID-19: From mathematical modelling perspective. Chaos Solitons Fractals 2020, 138, 109968. [Google Scholar] [CrossRef] [PubMed]
- Shaikh, A.S.; Shaikh, I.N.; Nisar, K.S. A mathematical model of COVID-19 using fractional derivative: Outbreak in India with dynamics of transmission and control. Adv. Differ. Equ. 2020, 373. [Google Scholar] [CrossRef] [PubMed]
- Mohammad, M.; Trounev, A.; Cattani, C. The dynamics of COVID-19 in the UAE based on fractional derivative modeling using Riesz wavelets simulation. Adv. Differ. Equ. 2021, 115. [Google Scholar] [CrossRef]
- Naik, P.A.; Yavuz, M.; Qureshi, S.; Zu, J.; Townley, S. Modeling and analysis of COVID-19 epidemics with treatment in fractional derivatives using real data from Pakistan. Eur. Phys. J. Plus 2020, 135, 795. [Google Scholar] [CrossRef]
- Choi, S.K.; Kang, B.; Koo, N. Stability for Caputo fractional differential systems. Abstr. Appl. Anal. 2014, 2014, 631419. [Google Scholar] [CrossRef]
- Wei, Z.; Li, Q.; Che, J. Initial value problems for fractional differential equations involving Riemann-Liouville sequential fractional derivative. J. Math. Anal. Appl. 2010, 367, 260–272. [Google Scholar] [CrossRef] [Green Version]
- van den Driessche, P.; Watmough, J. A simple SIS epidemic model with a backward bifurcation. J. Math. Biol. 2000, 40, 525–540. [Google Scholar] [CrossRef]
- Huo, J.; Zhao, H.; Zhu, L. The effect of vaccines on backward bifurcation in a fractional order HIV model. Nonlinear Anal. Real World Appl. 2015, 26, 289–305. [Google Scholar] [CrossRef]
- Vargas-De-Leon, C. Volterra-type Lyapunov functions for fractional-order epidemic systems. Commun. Nonlinear Sci. Numer. Simul. 2015, 24, 75–85. [Google Scholar] [CrossRef]
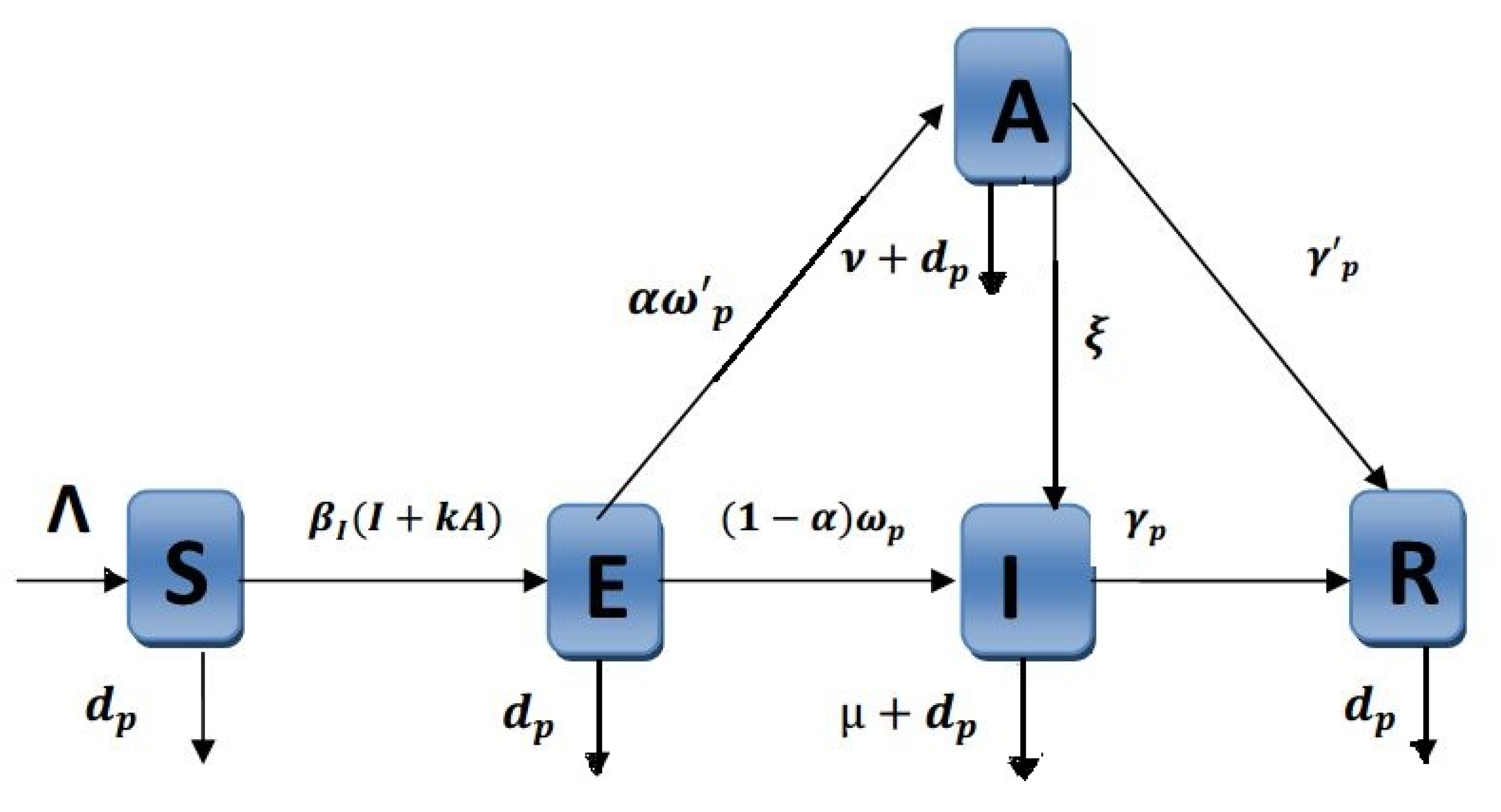


| susceptibles recruitment rate, | |
| natural mortality, | |
| disease transmission rate, | |
| k | transmissibility ratio between asymptomatics and symptomatics, |
| disease-related mortality for asymptomatics, | |
| disease-related mortality for symptomatic individuals, | |
| progression rate from exposed to symptomatic, | |
| progression rate from exposed to asymptomatic, | |
| fraction of exposed that turn asymptomatic, | |
| progression rate from asymptomatic to symptomatic, | |
| recovery rate from symptomatic infection, | |
| recovery rate from asymptomatic infection. |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Belgaid, Y.; Helal, M.; Lakmeche, A.; Venturino, E. A Mathematical Study of a Coronavirus Model with the Caputo Fractional-Order Derivative. Fractal Fract. 2021, 5, 87. https://doi.org/10.3390/fractalfract5030087
Belgaid Y, Helal M, Lakmeche A, Venturino E. A Mathematical Study of a Coronavirus Model with the Caputo Fractional-Order Derivative. Fractal and Fractional. 2021; 5(3):87. https://doi.org/10.3390/fractalfract5030087
Chicago/Turabian StyleBelgaid, Youcef, Mohamed Helal, Abdelkader Lakmeche, and Ezio Venturino. 2021. "A Mathematical Study of a Coronavirus Model with the Caputo Fractional-Order Derivative" Fractal and Fractional 5, no. 3: 87. https://doi.org/10.3390/fractalfract5030087
APA StyleBelgaid, Y., Helal, M., Lakmeche, A., & Venturino, E. (2021). A Mathematical Study of a Coronavirus Model with the Caputo Fractional-Order Derivative. Fractal and Fractional, 5(3), 87. https://doi.org/10.3390/fractalfract5030087






