Lyapunov Direct Method for Nonlinear Hadamard-Type Fractional Order Systems
Abstract
:1. Introduction
2. Preliminaries
3. Hadamard-Type Fractional Inequalities
4. Stability of Hadamard-Type Systems
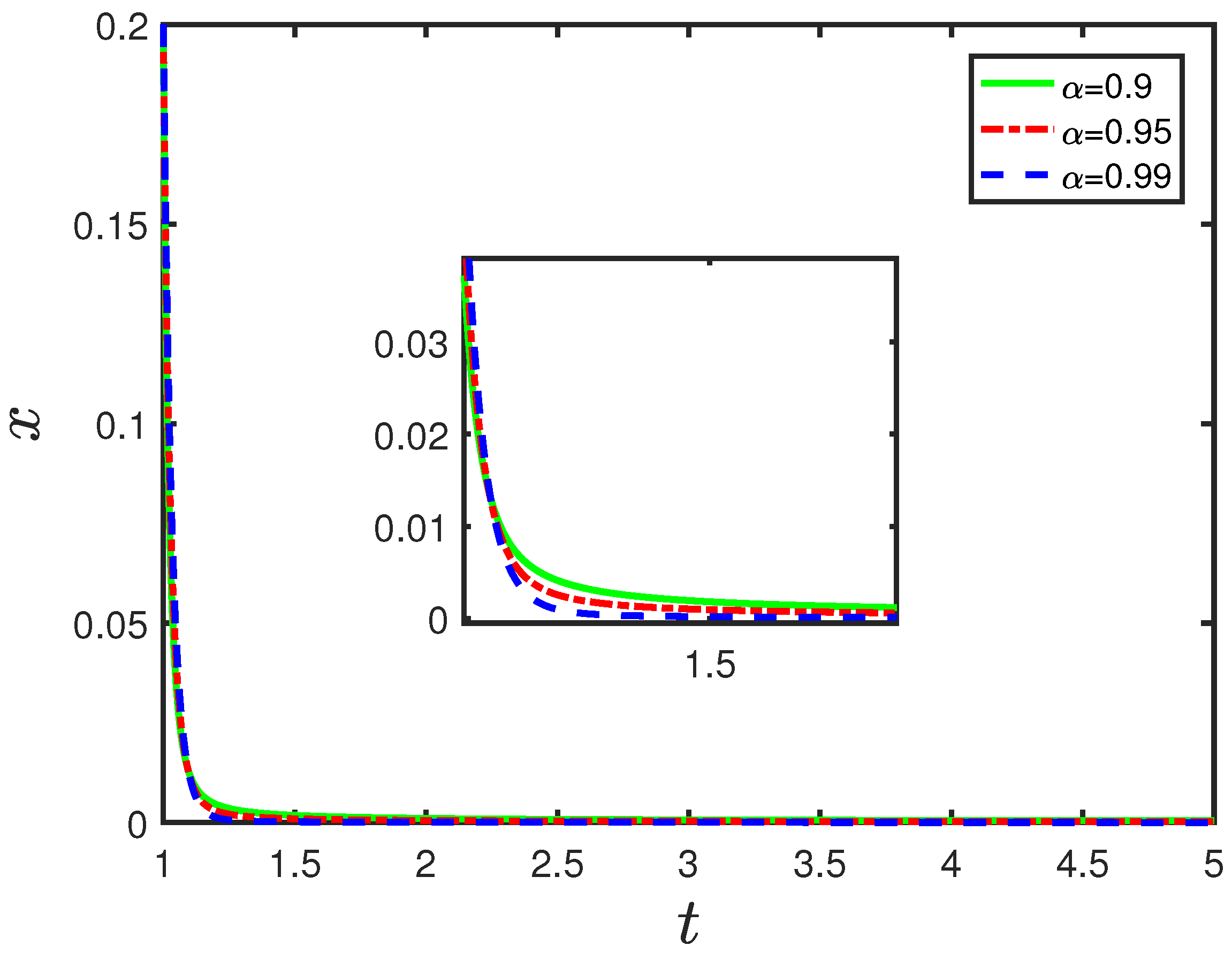
5. Numerical Examples
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and aAplications of Fractional Differential Equations; Elsevier: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Rahimy, M. Applications of fractional differential equation. Appl. Math. Sci. 2010, 4, 2453–2461. [Google Scholar]
- Dai, H.; Chen, W.S. New power law inequalities for fractional derivative and stability analysis of fractional order systems. Nonlinear Dyn. 2017, 87, 1531–1542. [Google Scholar] [CrossRef]
- Sabatier, J.; Agrawal, O.P.; Machado, J.A.T. Advances in Fractional Calculus; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Uchaikin, V.V. Fractional Derivatives for Physicists and Engineers; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Li, Z.M.; Ma, W.; Ma, N.R. Partial topology identification of tempered fractional-order complex networks via synchronization method. Math. Methods Appl. Sci. 2021. [Google Scholar] [CrossRef]
- Hu, T.C.; Li, L.L.; Wu, Y.Q.; Sun, W.G. Consensus dynamics in noisy trees with given parameters. Mod. Phys. Lett. B. 2022, 36, 2150608. [Google Scholar] [CrossRef]
- İlhan, E. Analysis of the spread of Hookworm infection with Caputo-Fabrizio fractional derivative. Turkish J. Sci. 2022, 7, 43–52. [Google Scholar]
- Ma, W.Y.; Li, Z.M.; Ma, N.R. Synchronization of discrete fractional-order complex networks with and without unknown topology. Chaos 2022, 32, 013112. [Google Scholar] [CrossRef] [PubMed]
- Podlubny, I. Fractional Differential Equations; Academic Press: Cambridge, MA, USA, 1999. [Google Scholar]
- Li, C.P.; Deng, W.H. Remarks on fractional derivatives. Appl. Math. Comput. 2007, 187, 777–784. [Google Scholar] [CrossRef]
- Anatoly, A.K. Hadamard-type fractional calculus. J. Korean. Math. Sos. 2001, 38, 1191–1204. [Google Scholar]
- Gong, Q.Z.; Qian, D.L.; Li, C.P.; Guo, P. On the Hadamard Type Fractional Differential System, Fractional Dynamics and Control; Springer: New York, NY, USA, 2012. [Google Scholar]
- Katugampola, U.N. A new approach to generalized fractional derivatives. Bull. Math. Anal. Appl. 2014, 6, 1–15. [Google Scholar]
- İlknur, K.; Akcetin, E.; Yaprakdal, P. Numerical approximation for the spread of SIQR model with Caputo fractional order derivative. Turk. J. Sci. 2020, 5, 124–139. [Google Scholar]
- Akdemir, A.O.; Butt, S.I.; Nadeem, M.; Ragusa, M.A. New general variants of Chebyshev type inequalities via generalized fractional integral operators. Mathematics 2021, 9, 122. [Google Scholar] [CrossRef]
- Ma, N.R.; Ma, W.Y.; Li, Z.M. Multi-Model Selection and Analysis for COVID-19. Fractal Fract. 2021, 6, 120. [Google Scholar] [CrossRef]
- Brandibur, O.; Kaslik, E. Stability Analysis for a Fractional-Order Coupled FitzHugh–Nagumo-Type Neuronal Model. Fractal Fract. 2022, 6, 257. [Google Scholar] [CrossRef]
- Li, C.P.; Li, Z.Q. Stability and logarithmic decay of the solution to Hadamard-type fractional differential equation. J. Nonlinear Sci. 2021, 31, 1–60. [Google Scholar] [CrossRef]
- Ahmad, B.; Alsaedi, A.; Ntouyas, S.K.; Tariboon, J. Hadamard-Type Fractional Differential Equations; Springer: Berlin/Heidelberg, Germany, 2017. [Google Scholar]
- Garra, R.; Mainardi, F.; Spada, G. A generalization of the Lomnitz logarithmic creep law via Hadamard fractional calculus. Chaos Solitons Fractals 2017, 102, 333–338. [Google Scholar] [CrossRef] [Green Version]
- Ma, L. On the kinetics of Hadamard-type fractional defferential systems. Fractals 2020, 23, 553–570. [Google Scholar]
- Brandibur, O.; Kaslik, E. Stability properties of a two-dimensional system involving one Caputo derivative and applications to the investigation of a fractional-order Morris–Lecar neuronal model. Nonlinear Dyn. 2017, 90, 2371–2386. [Google Scholar] [CrossRef] [Green Version]
- Li, Y.; Chen, Y.Q.; Podlubny, I. Stability of fractional-order nonlinear dynamic systems: Lyapunov direct method and generalized Mittag-Leffler stability. Comput. Math. Appl. 2010, 59, 1810–1821. [Google Scholar] [CrossRef] [Green Version]
- Liu, S.; Li, X.Y.; Jiang, W.; Zhou, X.F. Mittag-Leffler stability of nonlinear fractional neutral singular systems. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 3961–3966. [Google Scholar] [CrossRef]
- Duarte-Mermoud, M.A.; Aguila-Camacho, N.; Gallegos, J.A. Using general quadratic Lyapunov functions to prove Lyapunov uniform stability for fractional order systems. Commun. Nonlinear Sci. Numer. Simul. 2015, 22, 650–659. [Google Scholar] [CrossRef]
- Deng, W.H.; Li, C.P.; Lü, J.H. Stability analysis of linear fractional differential system with multiple time delays. Nonlinear Dyn. 2007, 48, 409–416. [Google Scholar] [CrossRef]
- Liu, S.; Wu, X.; Zhou, X.F. Asymptotical stability of Riemann-Liouville fractional nonlinear systems. Nonlinear Dyn. 2016, 86, 65–71. [Google Scholar] [CrossRef]
- Liu, S.; Wu, X.; Zhou, X.F. Asymptotical stability of Riemann-Liouville fractional singular systems with multiple time-varying delays. Appl. Math. Lett. 2017, 65, 32–39. [Google Scholar] [CrossRef]
- Ma, L.; Wu, B. Finite-time stability of Hadamard fractional differential equations in weighted Banach spaces. Nonlinear Dyn. 2022, 107, 3749–3766. [Google Scholar] [CrossRef]
- Hadamard, J. Essai sur l’étude des Fonctions, Données par Leur Développement de Taylor; Gauthier-Villars: France, French, 1892. [Google Scholar]
- Jarad, F.; Baleanu, D.; Abdeljawad, A. Caputo-type modification of the Hadamard fractional derivatives. Adv. Differ. Equ. 2012, 2012, 142. [Google Scholar] [CrossRef] [Green Version]
- Ma, L. Comparison theorems for Caputo-Hadamard fractional differential equations. Fractals 2019, 27, 1950036. [Google Scholar] [CrossRef]
- Li, C.; Li, Z.; Wang, Z. Mathematical analysis and the local discontinuous Galerkin method for Caputo-Hadamard fractional partial differential equation. J. Sci. Comput. 2020, 85, 41. [Google Scholar] [CrossRef]
- Wen, X.J.; Wu, Z.M.; Lu, J.G. Stability analysis of a class of nonlinear fractional-order systems. IEEE Trans. Circuits Syst. II Express Briefs. 2008, 55, 1178–1182. [Google Scholar] [CrossRef]
- Chen, G.S. A generalized Young inequality and some new results on fractal space. Automatica 2012, 1, 56–59. [Google Scholar]
- Aguila-Camacho, N.; Duarte-Mermoud, M.A.; Gallegos, J.A. Lyapunov functions for fractional order systems. Commun. Nonlinear Sci. Numer. Simul. 2014, 19, 2951–2957. [Google Scholar] [CrossRef]
- Ding, D.S.; Qi, D.L.; Wang, Q. Non-linear Mittag–Leffler stabilisation of commensurate fractional-order non-linear systems. IET Control Theory Appl. 2015, 9, 681–690. [Google Scholar] [CrossRef]
- Fernandez-Anaya, G.; Nava-Antonio, G.; Jamous-Galante, J.; Muñoz-Vega, R.; Hernández-Martínez, E. Lyapunov functions for a class of nonlinear systems using Caputo derivative. Commun. Nonlinear Sci. Numer. Simul. 2017, 43, 91–99. [Google Scholar] [CrossRef]
- Wang, G.T.; Pei, K.; Chen, Y.Q. Stability analysis of nonlinear Hadamard fractional differential system. J. Franklin. Inst. 2019, 356, 6538–6546. [Google Scholar] [CrossRef]
- Corduneanu, C. Principles of Differential and Integral Equations; American Mathematical Soc.: Providence, RI, USA, 2008. [Google Scholar]
- Li, Y.; Chen, Y.Q.; Podlubny, I. Mittag-Leffler stability of fractional order nonlinear dynamic systems. Automatica 2009, 45, 1965–1969. [Google Scholar] [CrossRef]
- Khalil, H.K.; Grizzle, J.W. Nonlinear Systems; Prentice Hall: Hoboken, NJ, USA, 2002. [Google Scholar]
- Gohar, M.; Li, C.P.; Yin, C.T. On Caputo-Hadamard fractional differential equations. Int. J. Comput. Math. 2020, 97, 1459–1483. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dai, C.; Ma, W. Lyapunov Direct Method for Nonlinear Hadamard-Type Fractional Order Systems. Fractal Fract. 2022, 6, 405. https://doi.org/10.3390/fractalfract6080405
Dai C, Ma W. Lyapunov Direct Method for Nonlinear Hadamard-Type Fractional Order Systems. Fractal and Fractional. 2022; 6(8):405. https://doi.org/10.3390/fractalfract6080405
Chicago/Turabian StyleDai, Changping, and Weiyuan Ma. 2022. "Lyapunov Direct Method for Nonlinear Hadamard-Type Fractional Order Systems" Fractal and Fractional 6, no. 8: 405. https://doi.org/10.3390/fractalfract6080405
APA StyleDai, C., & Ma, W. (2022). Lyapunov Direct Method for Nonlinear Hadamard-Type Fractional Order Systems. Fractal and Fractional, 6(8), 405. https://doi.org/10.3390/fractalfract6080405





