Novel Precise Solitary Wave Solutions of Two Time Fractional Nonlinear Evolution Models via the MSE Scheme

Abstract

We construct soliton solutions of the complex time fractional Schrodinger model (tFSM), as well as the space–time fractional differential model (stFDM), leading wave spread through electrical transmission lines model (ETLM) in low pass with the help of modified simple equation scheme. The approach provides us with generalized rational exponential function solutions with some free parameters. A few well-known solitary wave resolutions are derived, starting from the generalized rational solutions selecting specific values of the free constants. The precise solutions acquired via the technique signify that the scheme is comparatively easier to execute and attractive in view of the results. No auxiliary equation is needed to solve any nonlinear fractional models by the scheme. Additionally, we observed that the numerical results are very encouraging for researchers conducting further research on stFDMs in mathematics and physics.

1. Introduction

The technique of fractional differentiation is helpful when expressing the recollection and heritable character of materials and process. Modeling of microscopic multifaceted dynamics in the fields of fluid dynamics, diffusive convey in biological modeling, physical science, signal processing, networking systems, system detection, electromagnetic waves, earthquake happenings, astrophysics, and finance is impossible without using a fractional derivative [1,2,3,4,5,6,7,8] instead of classical differential models. The Riemann–Liouville derivative or Grunwald–Letnikov derivative or Caputo derivative are the three ways of defining fractional derivatives [1,2,3,4,5,6]. In recent times, fractional nonlinear progressive models have played a significant rule in the modeling of various microscopic multifaceted dynamics phenomena. These mathematical tools have been highlighted in many research studies due to their numerous forms in diverse applications in the above fields. Due to a few limitations of the derivatives, a comfortable fractional derivative was introduced in the literature [8,9]. For a clear understanding of the physical character of fractional differential models, it is essential to acquire precise or numerical solutions of the fractional nonlinear evolution models. In the last five decades, dynamical researchers have faced many complexities when it comes to inventing the precise solutions of fractional nonlinear evolution models. Recently, some effective new techniques have been proposed and improved the old methods for searching for exact solutions to the fractional differential models with nonlinearity. The proposed techniques in recent literature are first integral [9], (1/G)-expansion method [10], homotopy perturbation transform [11], unified [12], exp-function [13], Ricatti equation [14], modified extended tanh [15], modified simple equation [16],$(G^{\prime }/G)$-expansion [17], Hirota bilinear [18], variable coefficients [19,20] methods, and so on. Among these techniques, the MSE scheme [17] is more effective and concise when it comes to deriving the solitonic nature of both fractional and non-fractional nonlinear differential models. In addition to this, Jumarie [21] proposed a modified Riemann–Liouville fractional derivative to convert the FPDM to an ordinary differential model (ODM). Many dynamical researchers have used the above techniques [8,9,22] as an accurate conversion method from FPDM to ODM, searching for precise solutions to fractional nonlinear models.

The goal of the present research is to derive precise solutions of a complex tFSM [22,23,24] and a stFDM, overriding wave transmission into a low pass ETLM [25] using the MSE method. We will establish more general solutions in-terms of exponential functions involving free constants via direct integrations of the scheme; selecting different conditions on the free constants, various periodic, soliton, and other solitary wave solutions will be derived.

The complex tFSM was proposed by Nick Laskin in 1999, with the form [22,23,24]:

$$\frac{{\partial }^{\gamma }\varphi }{\partial t^{\gamma }}+i\frac{{\partial }^2\varphi }{\partial x^2}+\frac{\partial }{\partial x}({\left|\varphi \right|}^2\varphi )=0,0<\gamma <1$$

(1)

The above fractional model of a nonlinear Schrodinger model (FSM) is the most efficient universal model in quantum mechanics, which describes various physical nonlinear systems. For example, a nonlinear Schrodinger equation is used to illustrate the progression of low motion-changing envelopes of quasi mono-chromatic emissions in a feebly nonlinear dispersion medium. The nonlinear Schrodinger model (NLSM) never describes the time progression of a quantum status. Such a Schrodinger model has found its various applications in complicated wave transmission in inhomogeneous dispersive medium, as follows: dynamics in particle accelerators [26], non-uniform dielectric media, solitary waves in piezoelectric semiconductors, proposing Bose–Einstein condensates in hydrodynamics field theory and plasma wave propagation, nonlinear optical waves, quantum condensates, and heat pulse in solids [27,28,29,30,31].

We also shed light on a FPDM with the property of nonlinearity recitation the wave proliferation in low–pass electrical spread line [25]:

$$\frac{{\partial }^{2\alpha }\varphi }{\partial t^2}-v\frac{{\partial }^{2\alpha }}{\partial t^2}\left({\varphi }^2\right)+B\frac{{\partial }^{2\alpha }}{\partial t^2}\left({\varphi }^3\right)-{\rho }^2\frac{{\partial }^{2\alpha }\varphi }{\partial x^2}-\frac{{\rho }^4}{12}\frac{{\partial }^{4\alpha }\varphi }{\partial x^4}=0;0<\alpha <1,$$

(2)

Solitary signal solutions of electrical transmission lines model are essential for diverse applications of the areas, such as linking wireless transmitters and recipients with their antennas, satellite signals processing, mobile networking systems, computer networking, and superior speed CPU information buses. Furthermore, in an electronic communication system, a transmission line is a dedicated model, whereas previous formation was premeditated to transmit alternately during the progression of radio incidence. NLTLs are also ensuring an efficient path to verify how the excitations perform within the nonlinear media and modeling in the exotic chattels of media schemes.

2. Properties of Conformable Fractional Derivative

Now, we want to go over the conformable fractional derivatives [8,9,31,32,33]: let the function $\phi :[0,\infty )\to \Re$; this derivative of $\phi$ for order $\gamma$ is described by $T_{\gamma }(\phi )(t)=\begin{array}{c}\lim \\ \delta \to 0^{+}\end{array}\frac{\phi (t+\delta t^{1-\gamma })-\phi (t)}{\delta }$,$t>0$ and $0<\gamma \le 1$, where $T_{\gamma }$ is the conformable fractional differential operator.

Some important properties:

(i)

$T_{\gamma }(a\phi +b\varphi )=a{T}_{\gamma }(\phi )+b{T}_{\gamma }(\varphi )$, $\forall a,b\in \Re$

(ii)

$T_{\gamma }(t^{\beta })=\beta t^{\beta -\gamma }$, $\forall \beta \in \Re$

(iii)

$T_{\gamma }(\nu )=0$, $\nu =const.$

(iv)

$T_{\gamma }(\phi \circ \varphi )(t)=t^{1-\gamma }{\phi }^{\prime }(\varphi (t)){\varphi }^{\prime }(t)$

(v)

$T_{\gamma }(\frac{\phi }{\varphi })=\frac{\varphi T_{\gamma }(\phi )-\phi T_{\gamma }(\varphi )}{{\varphi }^2}$

3. The Fractional Complex Transformation

In this portion, we have discussed the fractional transformation for the fractional-order PDE,

$$P(\varphi ,\frac{{\partial }^{\gamma }\varphi }{\partial t^{\gamma }},\frac{\partial \varphi }{\partial x},\frac{\partial \varphi }{\partial y},\frac{{\partial }^{2\gamma }\varphi }{\partial t^{2\gamma }},\frac{{\partial }^2\varphi }{\partial t^2})=0,t\ge 0,0<\gamma \le 1,$$

(3)

where $u=u(x,\hspace{0.17em}t)$ is an unknown function; $P$ is a polynomial function due to a few particular variables, involving higher nonlinearity with utmost-order derivatives of the unknown function. To find the solution of Equation (3), we use the modified simple equation (MSE) method [16]. We have executed some key steps of the MSE scheme. The steps are:

Step 1: Thinking of a transformation of a complex time fractional nonlinear model,

$u(x,t)=u(\zeta )\exp (i\tau )$ with traveling wave variables

$$\hspace{0.17em}\zeta =ik(x-\frac{2g{t}^{\gamma }}{\gamma }) \mathrm{and} \hspace{0.17em}\tau =(gx-\frac{h{t}^{\gamma }}{\gamma }).$$

(4)

Using $u(x,t)=u(\zeta )$ as well as a space–time fractional wave variable,

$$\zeta =\frac{k_1}{\mathsf{\Gamma }(1+\gamma )}{t}^{\gamma }+\frac{k_2}{\mathsf{\Gamma }(1+\gamma )}{x}^{\gamma },$$

(5)

anyone isable to renovate the nonlinear FPDM Equation (3) to a nonlinear ODE:

$${\rm K}(u,u\prime ,u″,\dots )=0$$

(6)

in which the upper dash signifies the derivative of $u$ w.r. to $\zeta$.

Step 2: In this step, we preserve the trial solution to Equation (6) as:

$$u(\zeta )={{\displaystyle \sum _{i=0}^N{a}_i\left[\frac{s^{\prime }(\zeta )}{s(\zeta )}\right]}}^i,$$

(7)

where the constants $a_i(0,1,2,3, \dots , N)$ and the function $s(\zeta )$ are unspecified to be calculated, and in which $a_N\ne 0$, whereas the solutions are predefined or expressed in the form of a few recognized differential models in $(G^{\prime }/G)$-expansion [17], and Ricatti equation schemes [14], but the MSE scheme $s(\zeta )$ is neither pre-identified nor a result of a pre-identified differential model. These are the special characteristics of the MSE technique. Thus, the solution is more useful and realistic according to this method.

Step 3: Integers $N$ of Equation (7) has to be estimated through balancing the uppermost-order derivative as well as the uppermost-order nonlinear terms of $u(\zeta )$ from Equation (7).

Step 4: Inserting Equation (7) together with Equation (5) to evaluate the $s(\zeta )$. After simplification, one can get an expression in $\left(\frac{1}{s(\zeta )}\right)$. Setting all coefficients of the expression in different powers of $s(\zeta )$ to zero, we attain a few constraints for $a_i(0,1,2, \dots , N)$ together with other necessary parameters. Equation (3) can solve this by using these values.

Step 5: Putting values of the obtained constants in the previous step4 and $s(\zeta )$ into Equation (7), we yield the solutions of Equation (3).

4. Solution of Complex Fractional Models via MSE Scheme

This sector will apply the MSE procedure to acquire the innovative precise solution to the complex tFSM and the stFDM governing wave transmission in low pass ETLM.

4.1. The Complex tFSE

Consider the complex tFSM, which is defined in Equation (1). Let us consider the transformation for complex FSM $\varphi (x,t)=s(\zeta )\exp (i\tau )$, and corresponding traveling variables are $\hspace{0.17em}\zeta =ik(x+\frac{2g{t}^{\gamma }}{\gamma })$,$\hspace{0.17em}\tau =(gx+\frac{h{t}^{\gamma }}{\gamma })$ and adapt this complex nonlinear FSM to thenonlinear integer order SM as:

$$\{\begin{array}{l}\frac{{\partial }^{\gamma }\varphi }{\partial t^{\gamma }}=i(gs+2hk{s}^{\prime })\exp (i\tau )\\ \frac{{\partial }^2\varphi }{\partial x^2}=-(h^{2}s+2hk{s}^{\prime }+k^2{s}^{″})\exp (i\tau )\\ \frac{\partial }{\partial x}({\left|\varphi \right|}^2\varphi )=i(h^{3}s+3k^2{s}^{″})\exp (i\tau )\end{array}$$

(8)

We achieve the complex PSE with the help of Equation (10),

$$(g-h^2)s-k^2{s}^{″}+h{s}^3+3k{s}^2{s}^{\prime }=0$$

(9)

balancing $s^{″}$ and $s^2{s}^{″}$ on Equation (9), $\Rightarrow n=\frac{1}{2}$. Thus, pertaining to a recent transformation $s(\zeta )=u^{\frac{1}{2}}(\zeta )$ in Equation (9), we clasp into ODEs

$$4h{u}^3+4(g-h)u^2+6k{u}^2{u}^{\prime }+k^2{u^{\prime }}^2-2k^{2}u{u}^{″}=0$$

(10)

Case 1: Balancing the highest $u{u}^{″}$ with the terms of nonlinearity $u^{2}u$ in Equation (10) present $\Rightarrow n=1$.

As a result, Equation (7) reduces to

$$u(\zeta )={\ell }_0+{\ell }_1\left(\frac{s^{\prime }(\zeta )}{s(\zeta )}\right)$$

(11)

where ${\ell }_0$ and ${\ell }_1\ne 0$.

Differentiating Equation (11) two times and situating Equation (11), as well as derivatives to Equation (10), we have an expression in $s^k,(k=0,1,2,\dots )$. Linking coefficients of the expression of identical power of $s$ to zero, we gain a constraint solving yields with the ethics for ${\ell }_0$ and ${\ell }_1$.

$$-4{\ell }_0^2{h}^2+4h{\ell }_0^3+4{\ell }_0^{2}g=0,$$

(12)

$$\begin{array}{l}-8{\ell }_0{\ell }_1{h}^2{s}^{\prime }(\zeta )+12h{\ell }_0^2{\ell }_1{s}^{\prime }(\zeta )+6k{\ell }_0^2{\ell }_1{s}^{″}(\zeta )-2k^2{\ell }_0{\ell }_1{s}^{‴}(\zeta )\\ +8{\ell }_0{\ell }_{1}g{s}^{\prime }(\zeta )=0\end{array},$$

(13)

$$\begin{array}{l}-4{\ell }_0^2{h}^2{(s^{\prime }(\zeta ))}^2+12h{\ell }_0{\ell }_1^2{(s^{\prime }(\zeta ))}^2-6k{\ell }_0^2{\ell }_1{(s^{\prime }(\zeta ))}^2+6k^2{\ell }_0{\ell }_1{s}^{\prime }(\zeta )s^{″}(\zeta )+\\ 12k{\ell }_1^2{\ell }_0{s}^{\prime }(\zeta )s^{″}(\zeta )-2k^2{\ell }_1^{2}s(\zeta )s^{‴}(\zeta )+k^2{\ell }_1^2{(s^{″}(\zeta ))}^2+4{\ell }_1^{2}g{(s^{\prime }(\zeta ))}^3=0\end{array},$$

(14)

$$\begin{array}{l}4h{\ell }_1^3{(s^{\prime }(\zeta ))}^3-4k^2{\ell }_0{\ell }_1{(s^{\prime }(\zeta ))}^3-12k{\ell }_0{\ell }_1^2{(s^{\prime }(\zeta ))}^3+4k^2{\ell }_1^2{s}^{″}(\zeta ){(s^{\prime }(\zeta ))}^2+\\ 6k{\ell }_1^3{s}^{″}(\zeta ){(s^{\prime }(\zeta ))}^2=0\end{array},$$

(15)

$$-3k^2{\ell }_1^2{(s^{\prime }(\zeta ))}^4-6k{\ell }_1^3{(s^{\prime }(\zeta ))}^4=0.$$

(16)

Equation (12) implies

$${\ell }_0=0,\frac{h^2-g}{h}$$

and from Equation (16), we attain

$${\ell }_1=-\frac{k}{2},\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\because {\ell }_1\ne 0$$

Phase 1: ${\ell }_0=0,{\ell }_1=-\frac{k}{2}$

Using Equation (15) together with Phase1, we obtain

$$s^{\prime }(\zeta )=\frac{k}{2h}{s}^{″}(\zeta ).$$

(17)

Equation (13) is given by using Equation (17); then,

$$\frac{s^{‴}(\zeta )}{s^{″}(\zeta )}=\frac{g}{k}.$$

(18)

Integrating Equation (18) implies

$$s^{″}=c_1\exp (\frac{g}{k}\zeta ),$$

(19)

where $c_1$ is the arbitrary constant.

From Equation (17), by putting the value of $s^{″}$. from Equation (19),

$$s^{\prime }=\frac{k}{2h}{c}_1\exp (\frac{g}{k}\zeta ).$$

(20)

Again, integrating Equation (20),

$$s(\zeta )=c_2+\frac{k^2{c}_1}{2gh}\exp (\frac{g}{k}\zeta ),$$

(21)

where $c_1$ and $c_2$ are arbitrary constants.

Thus, the solution is

$$\varphi (x,t)={\left[-\frac{k}{2}\frac{\frac{k}{2h}{c}_1\exp (igk(x-\frac{k{t}^{\gamma }}{\gamma }))}{c_2+\frac{k^2{c}_1}{2gh}\exp (igk(x-\frac{k{t}^{\gamma }}{\gamma }))}\right]}^{1/2}\exp \left[i(gx+\frac{h{t}^{\alpha }}{\gamma })\right]$$

(22)

For the particular value $c_2=\frac{k^2{c}_1}{2gh}$, the solution of Equation (22) reduces to soliton solution

$$\varphi (x,t)={\left[-\frac{g}{4}(1+\tanh (\frac{igk}{2}(x+\frac{k{t}^{\gamma }}{\gamma })))\right]}^{1/2}\exp \left[i(gx+\frac{h{t}^{\alpha }}{\gamma })\right]$$

(23)

For the particular value $c_2=-\frac{k^2{c}_1}{2gh}$, the solution of Equation (22) reduces to soliton solution

$$\varphi (x,t)={\left[\frac{g}{4}\left\{1+\coth (\frac{igk}{2}(x+\frac{k{t}^{\gamma }}{\gamma }))\right\}\right]}^{1/2}\exp \left[i(gx+\frac{h{t}^{\alpha }}{\gamma })\right]$$

(24)

Phase 2:${\ell }_0=\frac{h^2-g}{h},{\ell }_1=-\frac{1}{2}k,$

Equation (15), together with Phase 2, gives

$$s^{\prime }(\zeta )=\frac{kh}{2(3h^2-2g)}{s}^{″}(\zeta ).$$

(25)

Equation (13) is given by using Equation (25); then,

$$\frac{s^{‴}(\zeta )}{s^{″}(\zeta )}=\frac{10h^4+6g^2-16h^{2}g}{kh(3h^2-2g)}’$$

(26)

Integrating Equation (26) implies

$$s^{″}=c_1\exp \left[(\frac{10h^4+6g^2-16h^{2}g}{kh(3h^2-2g)})\zeta \right],$$

(27)

where $c_1$ is the arbitrary constant.

Equations (25) and (27) imply the following,

$$s^{\prime }(\zeta )=\frac{kh}{2(3h^2-2g)}\left[c_1\exp \left\{(\frac{10h^4+6g^2-16h^{2}g}{kh(3h^2-2g)})\zeta \right\}\right].$$

(28)

Again, integrating Equation (28), then

$$s(\zeta )=c_2+\frac{k^2{h}^2{c}_1}{2(10h^4+6g^2-16h^{2}g)}\exp \left[(\frac{10h^4+6g^2-16h^{2}g}{kh(3h^2-2g)})\zeta \right],$$

(29)

where $c_1$ and $c_2$ are arbitrary constants.

Thus, the solution is

$$\varphi (x,t)={\left[\frac{h^2-g}{h}-\frac{\frac{k^{2}h{c}_1}{4(3h^2-2g)}\exp \left\{R\right\}}{c_2+\frac{k^2{h}^2{c}_1}{2(10h^4+6g^2-16h^{2}g)}\exp \left\{R\right\}}\right]}^{1/2}\exp (i\tau ),$$

(30)

where $\hspace{0.17em}R=\left[\frac{10h^4+6g^2-16h^{2}g}{kh(3h^2-2g)}\right]ik(x+\frac{2g{t}^{\gamma }}{\gamma })$ and $\tau =i(gx+\frac{h{t}^{\alpha }}{\gamma })$.

For the particular value $c_2=\frac{k^2{h}^2{c}_1}{2(10h^4+6g^2-16h^{2}g)}$, the solution of Equation (30) reduces to soliton solution

$$\varphi (x,t)={\left[\frac{h^2-g}{h}-\frac{(10h^4+6g^2-16h^{2}g)}{4(3h^2-2g)}(1+\tanh (\frac{R}{2}))\right]}^{1/2}\exp \left[i(gx+\frac{h{t}^{\alpha }}{\gamma })\right],$$

(31)

For the particular value $c_2=-\frac{k^2{h}^2{c}_1}{2(10h^4+6g^2-16h^{2}g)}$, the solution of Equation (30) reduces to soliton solution

$$\varphi (x,t)={\left[\frac{h^2-g}{h}+\frac{(10h^4+6g^2-16h^{2}g)}{4(3h^2-2g)}\left\{1+\coth (\frac{R}{2})\right\}\right]}^{1/2}\exp \left[i(gx+\frac{h{t}^{\gamma }}{\gamma })\right],$$

(32)

Remark 1.

Alam and Li [24] investigated the tFSM with the help of the Riccati equation as an Auxiliary equation that was not always suitable, but our solutions are derived directly via integration without help of an Auxiliary equation. In addition to this, we obtained more general solutions Equations (22) and (30), including arbitrary constants $c_1,\hspace{0.17em}{c}_2$ For arbitrary choice of the parameters, all similar solutions of [24] are possible, and there are even more other solutions. See Appendix A of Ref. [24].

4.2. The stFDM Governing Wave Propagation in Low-Pass ETLM

Consider the following stFDM governing wave transmission in low pass ETLM, which is given in Equation (2). Here, $v,\hspace{0.17em}\rho ,\hspace{0.17em}B$ are unknown invariables and $\varphi (x,t)$ is the voltage within the TLM. Here, $x$ indicates the proliferation space co-ordinate and $t$ indicates the idle time. Substantial evaluation facts of Equation (2) with Kirchhoff’s rule are specified Ref. [25].

Let us consider $\varphi (x,t)=u(\xi )$,

$$\xi =\frac{k_1}{\mathsf{\Gamma }(1+\alpha )}·t^{\alpha }+\frac{k_2}{\mathsf{\Gamma }(1+\alpha )}·x^{\alpha }.$$

(33)

Convert this nonlinear complex ETL Equation (2) into the ODE via the beyond Equation (33).

Then, ETL Equation (2) is reduced to the following ODE

$$(k_1^2-\rho k_2^2)u-k_1^{2}v{u}^2+B{k}_1^2{u}^3-\frac{{\rho }^4}{12}{k}_2^2{u}^{″}=0$$

(34)

Balancing the term $u^{″}(\xi )$ and $u^3(\xi )$ of Equation (34) provides $\Rightarrow n=1$.

We assumed the auxiliary solution of Equation (34);

$$u(\xi )=a_0+a_1\left(\frac{s^{\prime }(\xi )}{s(\xi )}\right)’$$

(35)

where $a_0$ and $a_1\ne 0$ are constants.

Differentiating Equation (35) two times, and setting Equation (35) as well as derivatives interested in Equation (34), we have an expression of $s^k,(k=0,1,2,\dots )$. Connecting coefficients of the expression of identical supremacy of $s$ to zero, we attain a constraint; solving it yields the values for $a_0$ and $a_1$.

$$B{k}_1^2{a}_0^3-k_1^{2}v{a}_0^2-a_{0}A{k}_2^2+a_0{k}_1^2=0,$$

(36)

$$\begin{array}{l}-a_{1}A{k}_2^2{s}^{\prime }(\xi )+k_1^2{a}_1{s}^{\prime }(\xi )-2v{k}_1^2{a}_0{a}_1{s}^{\prime }(\xi )+3B{k}_1^2{a}_0^2{a}_1{s}^{\prime }(\xi )\\ -\frac{1}{12}{a}_1{A}^4{k}_2^2{s}^{‴}(\xi )=0,\end{array}$$

(37)

$$-v{a}_1{k}_1^2{(s^{\prime }(\xi ))}^2+3B{k}_1^2{a}_0{a}_1^2{(s^{\prime }(\xi ))}^2+\frac{1}{4}{A}^4{k}_2^2{a}_1{s}^{″}(\xi )s^{\prime }(\xi )=0,$$

(38)

$$B{k}_1^2{a}_1^3{(s^{\prime }(\xi ))}^3-\frac{1}{6}{A}^4{k}_2^2{a}_1{(s^{\prime }(\xi ))}^3=0.$$

(39)

Equation (39) implies

$$a_1=0,\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}{a}_1=\pm \frac{A^2{k}_2}{\sqrt{6B}{k}_1}.$$

Again, from Equation (36),

$${\mathrm{a}}_0=0,\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}{a}_0=\frac{v{k}_1\pm \sqrt{L}}{2B{k}_1},$$

where $L=4AB{k}_2^2+v^2{k}_1^2-4B{k}_1^2.$

Phase 1:$a_0=0,a_1=\frac{A^2{k}_2}{\sqrt{6B}{k}_1}$

Equation (38), together with Phase 1, gives

$$s^{\prime }(\xi )=\frac{\sqrt{6B}{A}^2{k}_2}{4v{k}_1}{s}^{″}(\xi ).$$

(40)

From Equation (37) with Phase 1, by using Equation (40),

$$\frac{s^{‴}(\xi )}{s^{″}(\xi )}=\frac{3\sqrt{6B}}{v{A}^2{k}_1{k}_2}(k_1^2-A^2{k}_2)$$

(41)

Integrating Equation (41) implies

$$s^{″}=c_1\exp (\frac{3\sqrt{6B}}{v{A}^2{k}_1{k}_2}(k_1^2-A^2{k}_2).$$

(42)

From Equation (40)

$$s^{\prime }=\frac{\sqrt{6B}{A}^2{k}_2}{4v{k}_1}{c}_1\exp (\frac{3\sqrt{6B}}{v{A}^2{k}_1{k}_2}(k_1^2-A^2{k}_2)).$$

(43)

Again, integrating Equation (43), we reach

$$s(\xi )=c_2+c_1\frac{A^4{k}_2^2}{12(k_1^2-A^2{k}_2)}\exp (\frac{3\sqrt{6B}}{v{A}^2{k}_1{k}_2}(k_1^2-A^2{k}_2)).$$

(44)

Thus, the solution is

$$\varphi (x,t)=\frac{A^4{k}_2^2{c}_1}{4v{k}_1}\left[\frac{\exp (\pm \frac{3\sqrt{6B}}{v{A}^2{k}_1{k}_2}(k_1^2-A^2{k}_2)}{c_2+c_1\frac{A^4{k}_2^2}{12(k_1^2-A^2{k}_2)}\exp (\pm \frac{3\sqrt{6B}}{v{A}^2{k}_1{k}_2}(k_1^2-A^2{k}_2)}\right].$$

(45)

For the particular value $c_2=\frac{c_1{A}^4{k}_2^2}{12(k_1^2-A^2{k}_2)}$, the solution of Equation (45) reduces to soliton solution

$$\phi (x,t)=\left[-\frac{3(k_1^2-A^2{k}_2^2)}{2v{k}_1}\left\{1\pm \tanh (\frac{3\sqrt{6B}}{2v{A}^2{k}_1{k}_2}(k_1^2-A^2{k}_2)\right\}\right].$$

(46)

For the particular value $c_2=-\frac{k^2{c}_1}{2gh}$, the solution of Equation (45) reduces to soliton solution

$$\phi (x,t)=\left[\frac{3(k_1^2-A^2{k}_2^2)}{2v{k}_1}\left\{1\pm \coth (\frac{3\sqrt{6B}}{2v{A}^2{k}_1{k}_2}(k_1^2-A^2{k}_2)\right\}\right].$$

(47)

Phase 2:

$$a_0=\frac{v{k}_1\pm \sqrt{L}}{2B{k}_1},\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}{a}_1=\pm \frac{A^2{k}_2}{\sqrt{6B}{k}_1}$$

Equation (38), together with Phase 2, gives

$$s^{\prime }(\xi )=\frac{\sqrt{6B}{A}^2{k}_2}{2(-v{k}_1\pm 3\sqrt{L})}{s}^{″}(\xi ).$$

(48)

From Equation (38) with Phase 3, then applying Equation (48),

$$\frac{s^{‴}(\xi )}{s^{″}(\xi )}=\frac{3\sqrt{6B}{A}^2{k}_2\left\{4B(A{k}_2^2-k_1^2)+(v{k}_1\pm \sqrt{L})(v{k}_1\mp 3\sqrt{L})\right\}}{2B{A}^4{k}_2^2(v{k}_1\pm 3\sqrt{L})}$$

(49)

Integrating Equation (49) implies

$$s^{″}=c_1\exp (\pm Q\xi ).$$

(50)

where $Q=\frac{3\sqrt{6B}{A}^2{k}_2\left\{4B(A{k}_2^2-k_1^2)+(v{k}_1\pm \sqrt{L})(v{k}_1\mp 3\sqrt{L})\right\}}{2B{A}^4{k}_2^2(v{k}_1\pm 3\sqrt{L})}$.

From Equation (48)

$$s^{\prime }=\frac{-\sqrt{6B}{A}^2{k}_2}{(v{k}_1\pm 3\sqrt{L})}{c}_1\exp (\pm Q\xi ).$$

(51)

Again, integrating Equation (51), then

$$s(\xi )=c_2-c_{1}H\exp (Q\xi ).$$

(52)

where $H=\frac{A^{4}B{k}_2^2}{3\left\{4B(A^2{k}_2-k_1^2)+(v{k}_1+\sqrt{L})(v{k}_1-3\sqrt{L})\right\}}$.

Thus, the solution is

$$\varphi (x,t)=\frac{(v{k}_1\pm \sqrt{L})}{2B{k}_1}-\frac{A^4{k}_2^2{c}_1}{2k_1(v{k}_1\pm 3\sqrt{L})}\left[\frac{\exp (\pm Q\xi )}{c_2-c_{1}H\exp (\pm Q\xi )}\right]$$

(53)

For the particular value $c_2=-c_{1}H$, the solution of Equation (53) reduces to soliton solution

$$\varphi (x,t)=\frac{(v{k}_1\pm \sqrt{L})}{2B{k}_1}+\frac{A^4{k}_2^2}{4k_{1}H(v{k}_1\pm 3\sqrt{L})}\left[1\pm \tanh (\frac{Q\xi }{2})\right].$$

(54)

Additionally, for the particular value $c_2=c_{1}H$, the solution of Equation (53) reduces to soliton solution

$$\varphi (x,t)=\frac{(v{k}_1\pm \sqrt{L})}{2B{k}_1}-\frac{A^4{k}_2^2}{4k_{1}H(v{k}_1\pm 3\sqrt{L})}\left[1\pm \coth (\frac{Q\xi }{2})\right].$$

(55)

where $H=\frac{A^{4}B{k}_2^2}{3\left\{4B(A^2{k}_2-k_1^2)+(v{k}_1+\sqrt{L})(v{k}_1-3\sqrt{L})\right\}}$, $Q=\frac{3\sqrt{6B}{A}^2{k}_2\left\{4B(A{k}_2^2-k_1^2)+(v{k}_1\pm \sqrt{L})(v{k}_1\mp 3\sqrt{L})\right\}}{2B{A}^4{k}_2^2(v{k}_1\pm 3\sqrt{L})}$ and $L=4AB{k}_2^2+v^2{k}_1^2-4B{k}_1^2$.

Remark 2.

Abdoulkary et al. [25] investigated the total differential model that governed wave transmission in low pass ETLM with help of the Auxiliary equation, but we integrated stFDM-governing wave transmission in low pass ETLM directly via integration without the help of any Auxiliary equation. This conformable fractional derivative covers all the phenomena of a total differential model and is able to explain more with fractionality.

5. Graphical Representations

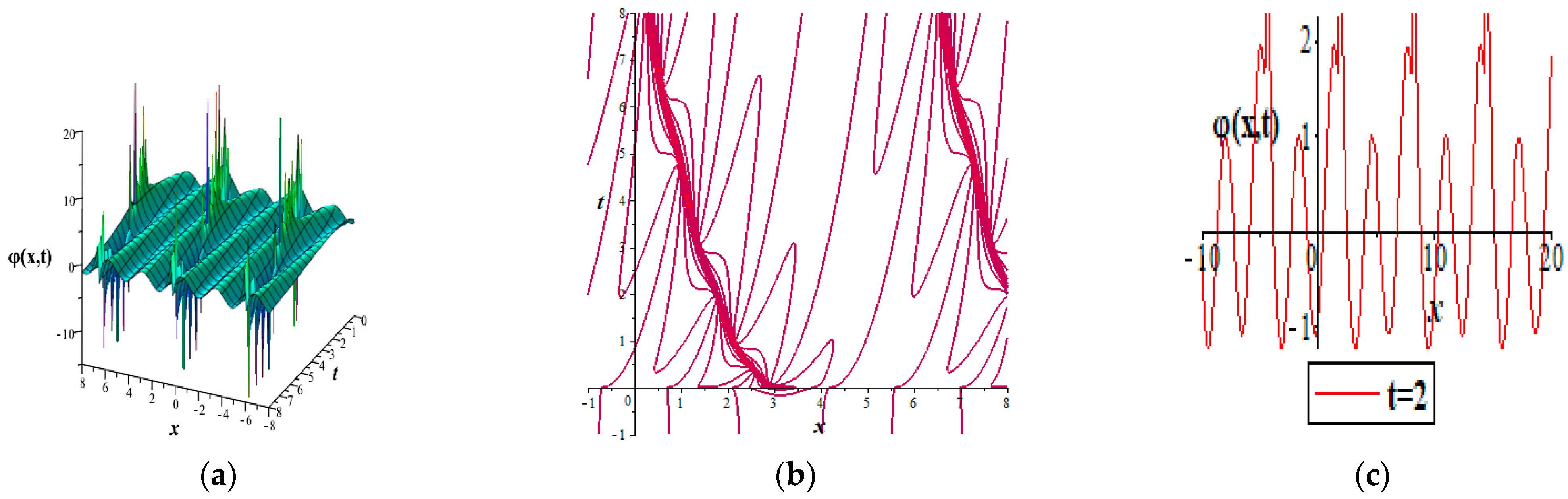
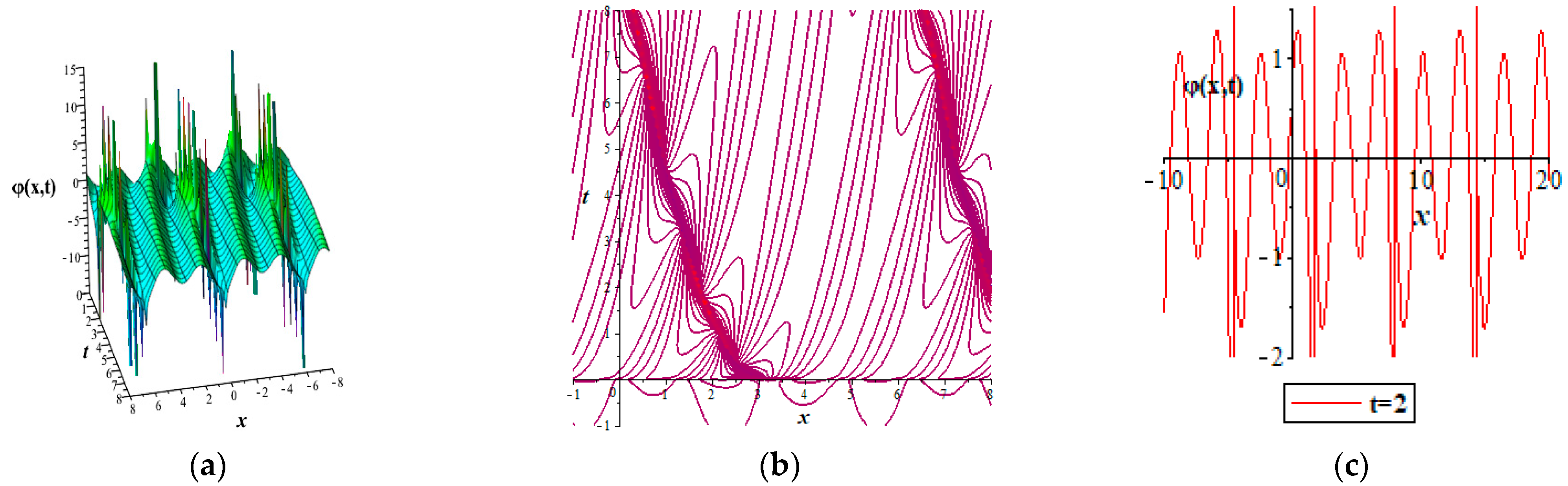
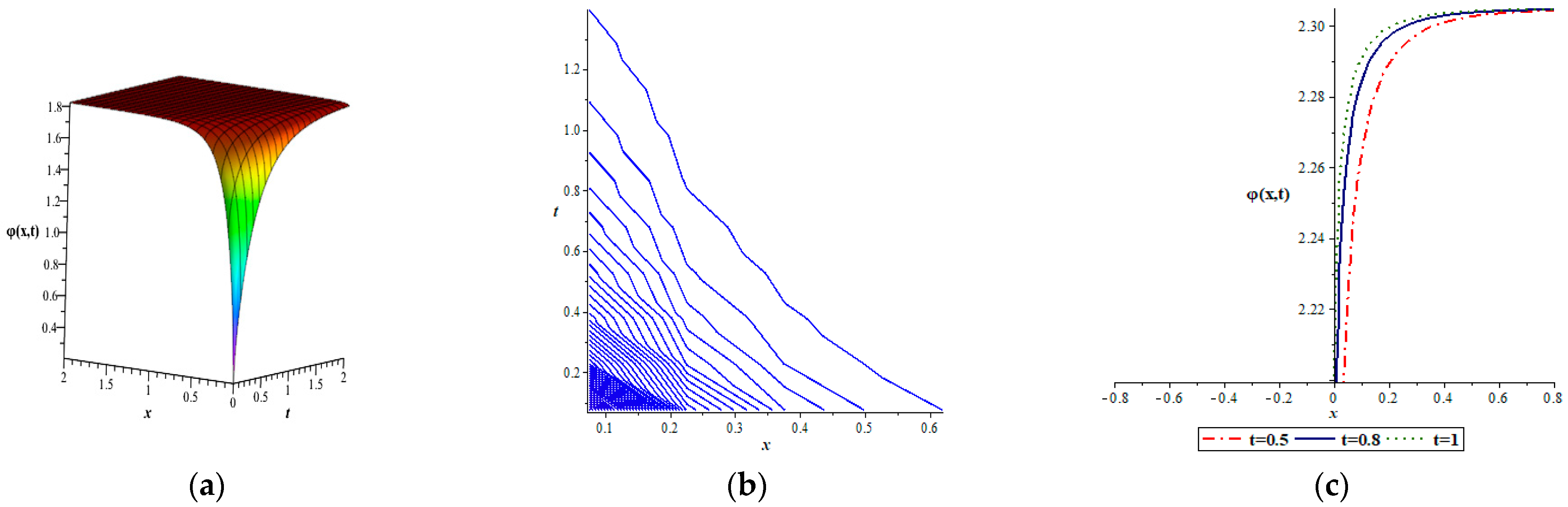
5.1. The Physical Illustration of Solutions to the Complex tFSM

In this subsection, we will illustrate one of the results of the complex tFSM. The achieve solutions are explored, but a few are illustrated as real and complex plots in Figure 1 and Figure 2 for the same unknown parametric values of $k=0.5,\hspace{0.17em}\hspace{0.17em}\gamma =0.5,h=1,g=-2,\hspace{0.17em}t=2$, respectively. The figures indicate fluctuations in amplitude, direction, and wave deformation, as well as differences in the particle-type nature of waves in favor of all gained solutions. The result is $\varphi (x,t)$ to Equation (22) for solution Set 1. For Set 2, we get a similar type of solution which is not illustrated below.

The obtained solutions may illustrate the progression of low motion in changing envelopes of quasi monochromatic emissions in feebly nonlinear dispersion medium, and may be applicable in complicated wave transmission in inhomogeneous dispersive medium as: dynamics in particle accelerators [26], non-uniform dielectric media, solitary waves in piezoelectric semiconductors, proposals of Bose–Einstein condensates in hydrodynamics field theory, quantum condensates, and heat pulse in solids [27,28,29,30,31].

5.2. The Physical Illustration of Solutions to stFDM-Governing Signal Transmission in Low-Pass ETLM

Two set of outcomes are carried out in this study of ETLM. Entire solutions are explored, but a few are illustrated in Figure 3 of Equation (46). The figures indicate the fluctuations in amplitude, direction, wave shape, as well as particle-type nature of waves in favor of every gained signal with space $x$, time $t$. The solitary wave via $\varphi (x,t)$ is part of Equation (46) of solution Set 1. Equation (54) of solution Set 2 is represented in Figure 4.

The obtained solutions may be illustrated solitary signals of an electrical transmission lines model, and will be very essential to its diverse application in different areas, such as linking wireless transmitters and recipients with their antennas, satellite signals processing, mobile networking system, computer networking, and superior speed CPU information buses.

6. Concluding Remarks and Future Tasks

We have applied the modified simple equation scheme to the complex tFSM and the stFDM governing wave proliferation in low pass ETLM due to construction of solitary wave solutions. We have retrieved generalized rational exponential function solutions of the fractional-order models, including some arbitrary parameters via direct integrations of the scheme. Selecting different conditions on the free constants, various periodic, soliton and other solitary wave solutions are derived. The acquired results among liberated constants might be significant to elucidate various worldly phenomena, including special solitonic behaviors. Ultimately, we conclude that this scheme is capable of execution on a range of nonlinear FEMs that arise in mathematics, physics, and engineering branches. In the near future, it is suitable for researcher to modify these models on the generalized fraction derivative [34], as it provides more advance phenomena of a conformable derivative. We shall convey this in applications on various nonlinear complex models in the near future. Moreover, bright bell, dark bell, rogue wave, and interactions solutions [35] will be the next step of interesting researchers on such fractional models.