The Mathematics of ‘Natural Beauty’ in the Architecture of Andrea Palladio and Le Corbusier: An Analysis of Colin Rowe’s Theory of Formal Complexity Using Fractal Dimensions
Abstract
:1. Introduction
1.1. Rowe’s Arguments about Palladio and Le Corbusier
1.2. Scale-Free Self-Similarity and Relationship to the Golden Ratio
2. Research Hypotheses
2.1. Hypothesis 1
2.2. Hypothesis 2
3. Measuring Fractal Dimensions in Architecture
Fractal Dimensions and Applications in Architectural Research
4. Research Methodology
4.1. Stage 1: Image Preparation
4.2. Stage 2: Computational Analysis
4.3. Stage 3: Extracting Derived Measures
4.4. Limitations
5. Results
5.1. Elevations
5.2. Floor Plans
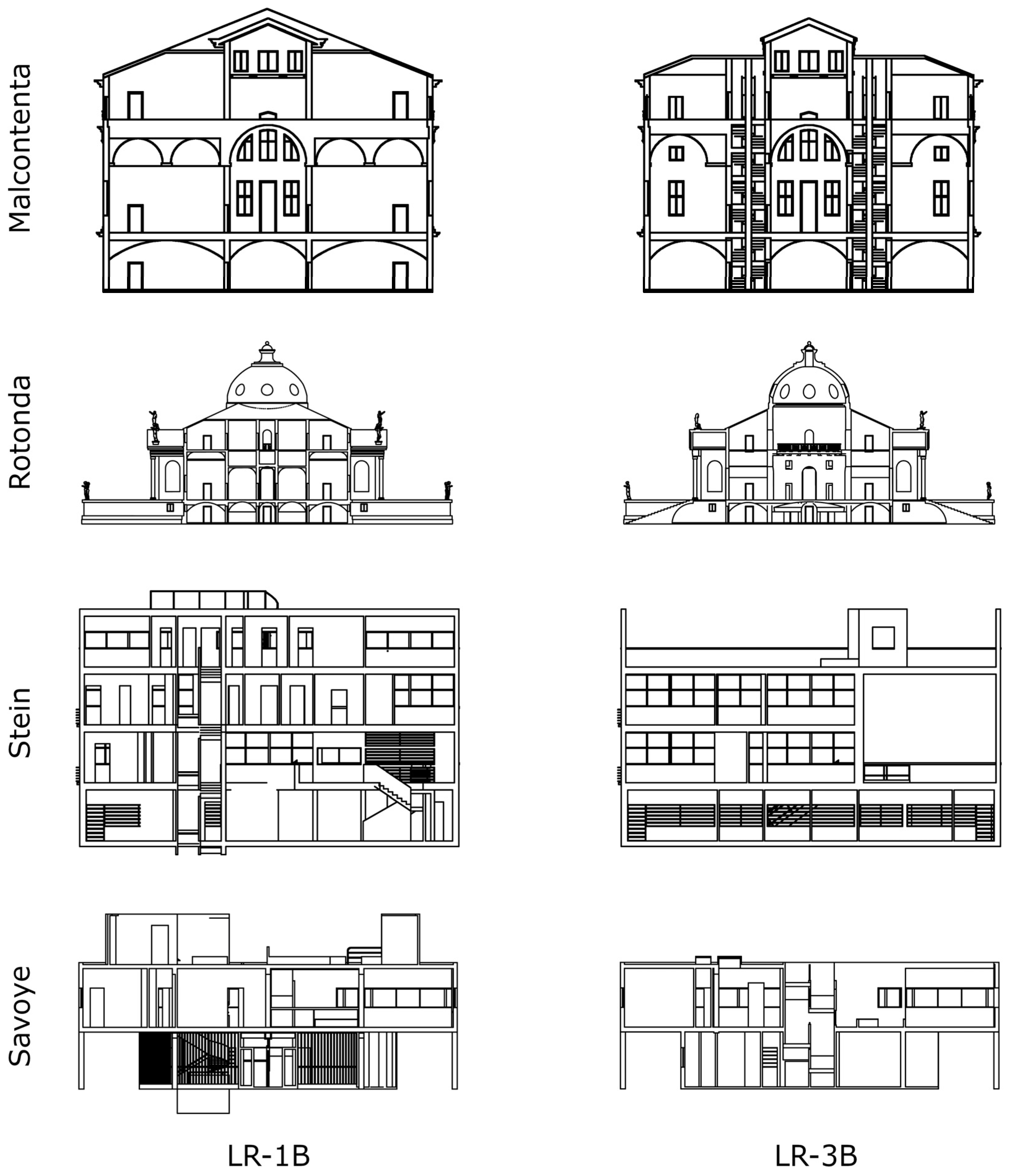
5.3. Sections
6. Discussion
6.1. Hypothesis 1
6.2. Hypothesis 2
6.3. Discussion
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ostwald, M.J.; Vaughan, J. The Fractal Dimension of Architecture; Birkhauser: Basel, Switzerland; Springer: Cham, Switzerland, 2016. [Google Scholar]
- Driscoll, J.C. Fractal Patterns as Fitness Criteria in Genetic Algorithms Applied as a Design Tool in Architecture. Nexus Netwirk J. 2021, 23, 21–37. [Google Scholar] [CrossRef]
- An, W.; Wang, C.; Zhang, H.; Bi, Z. Measuring the Formal Complexity of Architectural Curved Surfaces Based on 3D Box-Counting Dimension. Nexus Netwirk J. 2022, 24, 753–766. [Google Scholar] [CrossRef]
- Vaughan, J.; Ostwald, M.J. Examining the Position of Wright’s Fallingwater in the Context of His Larger Body of Work: An Analysis Using Fractal Dimensions. Fractal Fract. 2022, 6, 187. [Google Scholar] [CrossRef]
- Lee, J.H.; Ostwald, M.J. The ‘visual attractiveness’ of architectural facades: Measuring visual complexity and attractive strength in architecture. Archit. Sci. Rev. 2023, 66, 42–52. [Google Scholar] [CrossRef]
- Ostwald, M.J. The fractal analysis of architecture: Calibrating the box-counting method using scaling coefficient and grid disposition variables. Environ. Plan. B Plan. Des. 2013, 40, 644–663. [Google Scholar] [CrossRef]
- Stamps, A.E., III. Fractals, skylines, nature and beauty. Landsc. Urban Plan. 2002, 60, 163–184. [Google Scholar] [CrossRef]
- Vaughan, J.; Ostwald, M.J. Quantifying the Changing Visual Experience of Architecture: Combining Movement with Visual Complexity. In Across: Architectural Research Throught to Practice: 48th International Conference of the Architectural Science Association (ANZAScA); Madeo, F., Schnabel, M.A., Eds.; The Architectural Science Association; Genoa Universtiy Press: Genoa, Italy, 2014; pp. 557–568. [Google Scholar]
- Lee, J.; Ostwald, M. Fractal Dimension Calculation and Visual Attention Simulation: Assessing the Visual Character of an Architectural Façade. Buildings 2021, 11, 163. [Google Scholar] [CrossRef]
- Ostwald, M.J.; Dawes, M.J. The Mathematics of the Modernist Villa; Williams, K., Ed.; Birkhäuser: Cham, Switzerland, 2018. [Google Scholar]
- Lorenz, W.E. Estimating the Fractal Dimension of Architecture: Using Two Measurement Methods Implemented in AutoCAD by VBA. In Proceedings of the Digital Physicality, eCAADe 2012, Prague, Czech Republic, 12–14 September 2012; pp. 505–513. [Google Scholar]
- Lorenz, W.E. Fractal geometry of architecture: Implementation of the box-counting method in a CAD-software, in Computation: The New Realm of Architectural Design. In Proceedings of the eCAADe 2009, Istanbul, Turkey, 16–19 September 2009; pp. 697–704. [Google Scholar]
- Rowe, C. The Mathematics of the Ideal Villa. Archit. Rev. 1947, 100–104. [Google Scholar]
- Palladio, A. The Architecture of A. Palladio in Four Books; 1715 Translation ed.; Giacamo Lioni: London, UK, 1715. [Google Scholar]
- Rowe, C.; Slutzky, R. The Mathematics of the Ideal Villa and Other Essays; MIT Press: Cambridge, MA, USA, 1976; p. 240. [Google Scholar]
- Mandelbrot, B.B. Les Objets Fractals: Forme, Hasard et Dimension; Flammarion: Paris, France, 1975. [Google Scholar]
- Kruft, H.-W. A History of Architectural Theory: From Vitruvius to the Present; Zwemmer: London, UK; Princeton Architectural Press: New York, NY, USA, 1994. [Google Scholar]
- Wittkower, R. Architectural Principles in the Age of Humanism, 4th ed.; St. Martins’s Press: London, UK; New York, NY, USA, 1988. [Google Scholar]
- Stewart, D.E.; Corbusier, L. Towards a New Architecture; Rodker: London, UK, 1931. [Google Scholar]
- Rowe, C.; Koetter, F. Collage City; MIT Press: Cambridge, MA, USA, 1979. [Google Scholar]
- Rowe, C. As I Was Saying: Recollections and Miscellaneous Essays; MIT Press: Cambridge, MA, USA, 1995. [Google Scholar]
- Rowe, C. The Architecture of Good Intentions: Towards a Possible Retrospect; Academy: London, UK, 1994. [Google Scholar]
- Fletcher, R. Palladio’s Villa Emo: The Golden Proportion Hypothesis Defended. Nexus Netwirk J. 2001, 3, 105–112. [Google Scholar] [CrossRef]
- March, L. Palladio’s Villa Emo: The Golden Proportion Hypothesis Rebutted. Nexus Netwirk J. 2001, 3, 85–104. [Google Scholar] [CrossRef]
- Mitrovic, B.; Djordjević, I. Palladio’s Theory of Proportions and the Second Book of the Quattro Libri dell’Architettura. J. Soc. Archit. Hist. 1990, 49, 279–292. [Google Scholar]
- Tikhonova, O. New Approach to Proportional Analysis of Palladio’s Designs in Book II with Particular Attention to Loggias. Nexus Netw. J. 2019, 21, 359–381. [Google Scholar] [CrossRef]
- Wassel, S.R. The Mathematics of Palladio’s Villas: Workshop ’98. Nexus Netwirk J. 1998, 1, 121–128. [Google Scholar] [CrossRef]
- Howard, D.; Longair, M. Harmonic Proportion and Palladio’s Quattro Libri. J. Soc. Archit. Hist. 1982, 41, 116–143. [Google Scholar] [CrossRef]
- Fischler, R. The early relationship of Le Corbusier to the ‘golden number’. Environ. Plan. B 1979, 6, 95–103. [Google Scholar] [CrossRef]
- Stiny, G.; Gips, J. An evaluation of Palladian plans. Environ. Plan. B Plan. Des. 1978, 5, 199–206. [Google Scholar] [CrossRef]
- Salingaros, N.A. Life and Complexity in Architecture From a Thermodynamic Analogy. Phys. Essays 1997, 10, 165–173. [Google Scholar] [CrossRef]
- Mandelbrot, B.B. The Fractal Geometry of Nature, Updated and Augmented; Freeman: San Francisco, CA, USA, 1982. [Google Scholar]
- Voss, R.F. Characterization and Measurement of Random Fractals. Phys. Scr. 1986, T13, 27–32. [Google Scholar] [CrossRef]
- Avestas, P.; Matsopoulos, G.; Nikita, K. Applications of fractal theory on medical data processing. In Advanced Infrastructures for Future Healthcare; Marsh, A., Grndinetti, L., Kauranne, T., Eds.; IOS Press: Amsterdam, The Netherlands, 2000; pp. 425–441. [Google Scholar]
- Li, J.; Du, Q.; Sun, C. An improved box counting method for image fractal dimension estimation. Pattern Recognit. 2009, 42, 2460–2469. [Google Scholar] [CrossRef]
- Rasband, W. ImageJ 1997–2018; National Institute of Health: Bethesda, MD, USA, 2018. [Google Scholar]
- Karperien, A. FracLac for Image J 1999–2013; National Institute of Health: Bethesda, MD, USA, 2013. [Google Scholar]
- Ostwald, M.J. “Fractal architecture”:Late twentieth century connections between architecture and fractal geometry. Nexus Netwirk J. 2001, 3, 73–84. [Google Scholar] [CrossRef]
- Batty, M. Longley, Fractal Cities: A Geometry of Form and Function; Academic Press: San Deigo, CA, USA, 1994. [Google Scholar]
- Bechhoefer, W.; Appleby, M. Fractals, Music and Vernacular Architecture: An Ex-periment in Contextual Design. Crit. Methodol. Study Tradit. Environ. 1997, 97, 1–33. [Google Scholar]
- Cagdas, G.; Gozubuyuk, G.; Ediz, O. Fractal Based Generative Design for Harmony Between Old and New. In Generative Art 2005, Proceedings of the 8th Generative Art Conference; Soddu, C., Ed.; Domus Argenia: Serramanna, Italy, 2005. [Google Scholar]
- Wen, K.-C.; Kao, Y.-N. An Analytic Study of Architectural Design Style by Frac-tal Dimension Method. In Proceedings of the 22nd International Symposium on Automation and Robotics in Construction, Ferrara, Italy, 11–14 September 2005. [Google Scholar]
- Burkle-Elizondo, G.; Valdez-Cepeda, R.D. Fractal analysis of Mesoamerican pyr-amids. Nonlinear Dyn. Psychol. Life Sci. 2006, 10, 105–122. [Google Scholar]
- Cooper, J.C.; Oskrochi, R. Fractal Analysis of Street Vistas: A Potential Tool for Assessing Levels of Visual Variety in Everyday Street Scenes. Environ. Plan. B Plan. Des. 2008, 35, 349–363. [Google Scholar] [CrossRef]
- Ostwald, M.J.; Vaughan, J. Limits and Errors Optimising Image Pre-Processing Standards for Architectural Fractal Analysis. ArS Archit. Sci. 2013, 7, 1–19. [Google Scholar]
- Bovill, C. Fractal Geometry in Architecture and Design; Design science collection; Birkhäuser: Boston, MA, USA, 1996. [Google Scholar]
- Lorenz, W.E. Fractals and fractal architecture. In Faculty of Architecture and Spatial Planning; Vienna University of Technology: Vienna, Australia, 2003. [Google Scholar]
- Capo, D. The Fractal Nature of the Architectural Orders. Nexus Network J. 2004, 6, 30–40. [Google Scholar] [CrossRef] [Green Version]
- Ostwald, M.J.; Tucker, C. Reconsidering Bovill’s Method for Determining the Fractal Geometry of Architecture. In Proceedings of the 41st Annual Conference of the Architectural Science Association ANZAScA, Melbourne, Australia, 14–16 November 2007; pp. 182–190. [Google Scholar]
- Stamps, A.E. Psychology and the Aesthetics of the Built Environment; Kluwer Academic: Boston, MA, USA, 2000. [Google Scholar]
- Ostwald, M.J.; Vaughan, J.; Chalup, S.K. Data-cluster analysis of correlations be-tween façade complexity and orientation in Modernist architecture. In Proceedings of the 43rd Annual Conference of the Architectural Science Association, ANZAScA2009, Launceston, Australia, 25–27 November 2009; pp. 729–736. [Google Scholar]
- Ando, T. Le Corbusier: Houses; Toto: Kitakyushu, Japan, 2001; p. 416. [Google Scholar]
- Stamps, A.E., III. Architectural detail, Van der Laan septaves and pixel counts. Des. Stud. 1999, 20, 83–97. [Google Scholar] [CrossRef]
- Ching, F. Architecture: Form, Space and Order; John Wiley and Sons: Hoboken, NJ, USA, 2007. [Google Scholar]
- Moore, C.; Allen, G.; Lyndon, D. The Place of Houses; Holt, Rinehart and Watson: New York, NY, USA, 1974. [Google Scholar]
- Dawes, M.J.; Lee, J.H.; Ostwald, M.J. Intelligibility and cellularity in the villas of Palladio and Le Corbusier: Examining Rowe’s observations. Int. J. Archit. Res. 2021. ahead of print. [Google Scholar] [CrossRef]

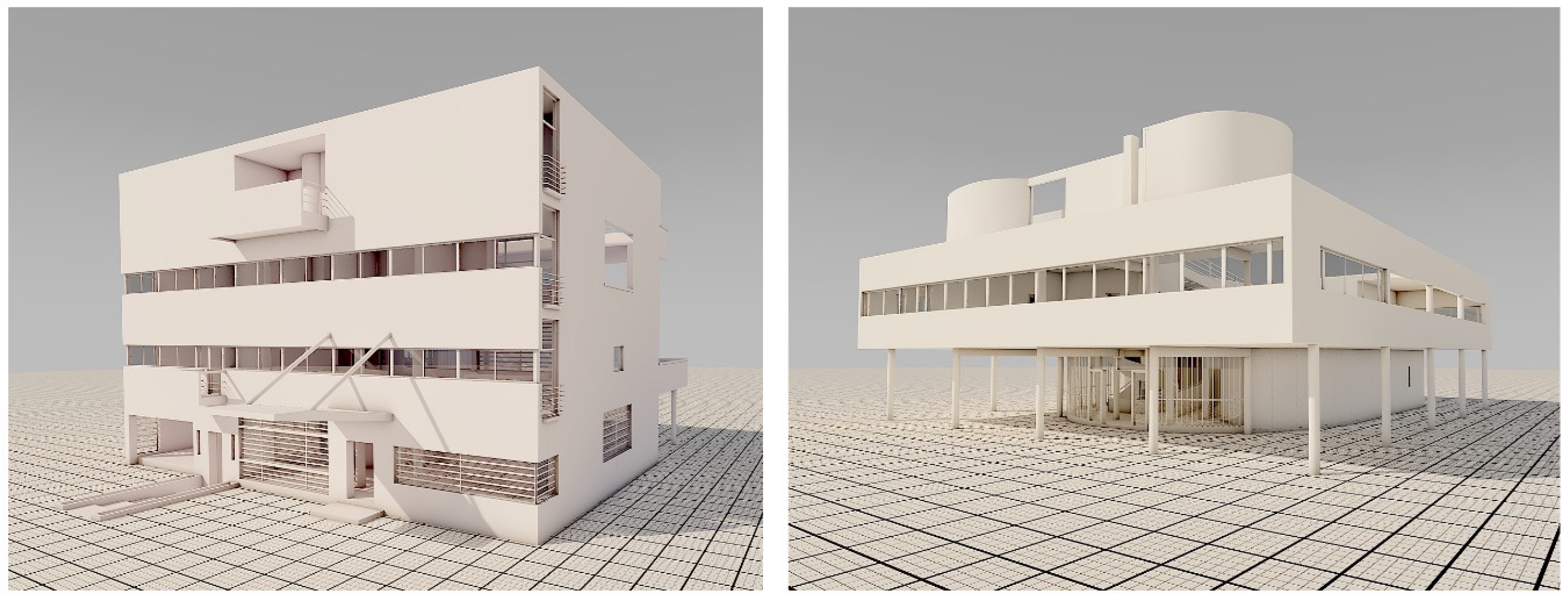
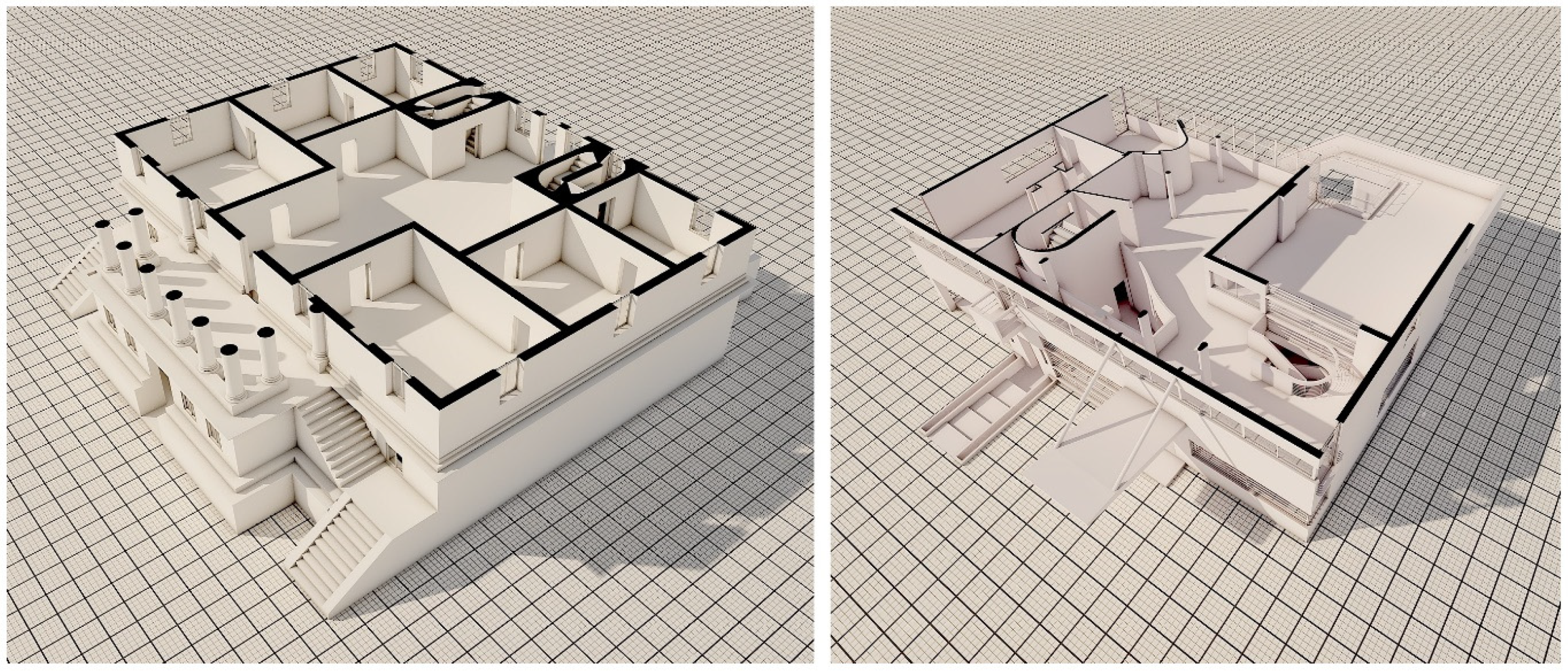

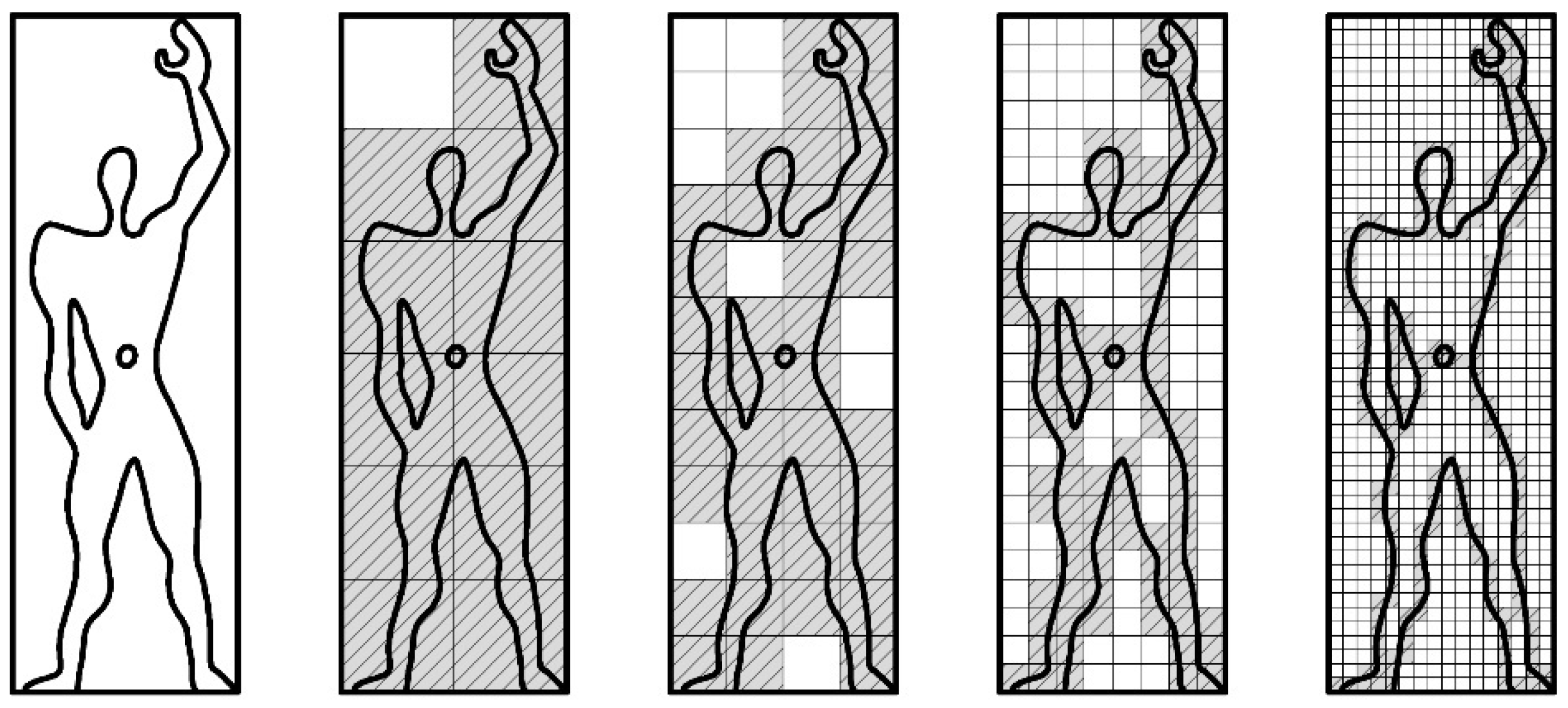
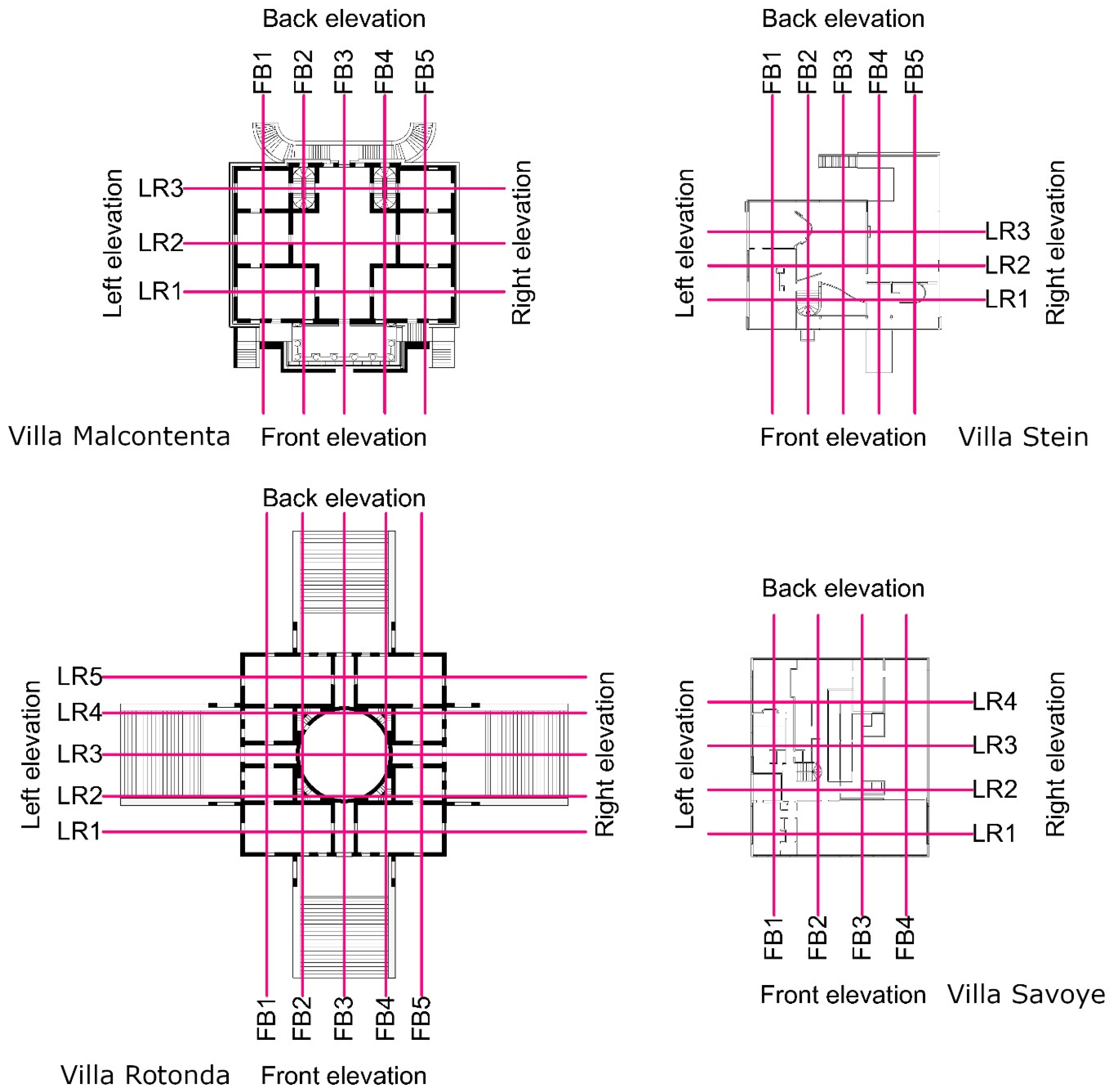


| Elevation | Malcontenta | Rotonda | Stein | Savoye |
|---|---|---|---|---|
| Front | 1.6387 | 1.5855 | 1.4733 | 1.4497 |
| Left | 1.4851 | 1.5927 | 1.4458 | 1.3544 |
| Rear | 1.5881 | 1.5855 | 1.5897 | 1.5114 |
| Right | 1.4843 | 1.5927 | 1.3744 | 1.4731 |
| Mean | 1.5490 | 1.5891 | 1.4708 | 1.4471 |
| SD | 0.0712 | 0.0296 | 0.0896 | 0.0669 |
| Plan | Malcontenta | Rotonda | Stein | Savoye |
|---|---|---|---|---|
| Ground | 1.4502 | 1.5361 | 1.4294 | 1.1879 |
| First | 1.5701 | 1.6352 | 1.3904 | 1.3438 |
| Second | 1.5566 | 1.6560 | 1.4027 | 1.3565 |
| Third | - | - | 1.4278 | - |
| Fourth | - | - | 1.3214 | - |
| Mean | 1.5256 | 1.6091 | 1.3943 | 1.2961 |
| SD | 0.0657 | 0.0564 | 0.0440 | 0.0939 |
| Section | Malcontenta | Rotonda | Stein | Savoye |
|---|---|---|---|---|
| FB-L1 | 1.4188 | 1.5159 | 1.4710 | 1.4163 |
| FB-L2 | 1.5015 | 1.5121 | 1.5247 | 1.5167 |
| FB-L3 | 1.4522 | 1.5280 | 1.5008 | 1.5178 |
| FB-L4 | 1.5036 | 1.5658 | 1.5063 | 1.4853 |
| FB-L5 | 1.5056 | 1.5401 | 1.4628 | - |
| FB-R1 | 1.5056 | 1.5401 | 1.4720 | 1.5366 |
| FB-R2 | 1.5036 | 1.5658 | 1.4537 | 1.5117 |
| FB-R3 | 1.4522 | 1.5280 | 1.4739 | 1.4514 |
| FB-R4 | 1.5015 | 1.5121 | 1.4990 | 1.3626 |
| FB-R5 | 1.4188 | 1.5159 | 1.5215 | - |
| FB Mean | 1.4763 | 1.5324 | 1.4886 | 1.4748 |
| LR-F1 | 1.4905 | 1.4638 | 1.6068 | 1.3944 |
| LR-F2 | 1.4706 | 1.5067 | 1.6147 | 1.5390 |
| LR-F3 | 1.5861 | 1.5345 | 1.5960 | 1.4859 |
| LR-F4 | - | 1.5600 | - | 1.4765 |
| LR-F5 | - | 1.5158 | - | - |
| LR-B1 | 1.5127 | 1.5158 | 1.6401 | 1.5686 |
| LR-B2 | 1.4981 | 1.5600 | 1.5922 | 1.4631 |
| LR-B3 | 1.6357 | 1.5345 | 1.6034 | 1.3884 |
| LR-B4 | - | 1.5067 | - | 1.4026 |
| LR-B5 | - | 1.4638 | - | - |
| LR Mean | 1.5323 | 1.5161 | 1.6089 | 1.4648 |
| Mean | 1.4973 | 1.5243 | 1.5487 | 1.4698 |
| SD | 0.0546 | 0.0283 | 0.0640 | 0.0618 |
| Villa | Sections | Plans | df | t | ||
|---|---|---|---|---|---|---|
| M | SD | M | SD | |||
| Malcontenta | 1.4973 | 0.0546 | 1.5256 | 0.0571 | 26.0000 | −1.3320 |
| Rotonda | 1.5243 | 0.0283 | 1.6091 | 0.0546 | 14.6160 | −4.9890 * |
| Stein | 1.5337 | 0.0640 | 1.3943 | 0.0419 | 24.7720 | 7.5180 * |
| Savoye | 1.4701 | 0.0599 | 1.2961 | 0.0804 | 27.0000 | 6.6910 * |
| Malcontenta | Rotonda | df | t | |||
|---|---|---|---|---|---|---|
| M | SD | M | SD | |||
| Plan | 1.5256 | 0.0571 | 1.6091 | 0.0546 | 22.0000 | −3.6580 * |
| Elevation | 1.5490 | 0.0712 | 1.5891 | 0.0296 | 20.0320 | −2.0770 |
| Stein | Savoye | df | t | |||
| M | SD | M | SD | |||
| Plan | 1.3943 | 0.0419 | 1.2961 | 0.0804 | 14.6590 | 3.9250 * |
| Elevation | 1.4708 | 0.0825 | 1.4471 | 0.0611 | 30.0000 | 0.9210 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dawes, M.J.; Ostwald, M.J.; Lee, J.H. The Mathematics of ‘Natural Beauty’ in the Architecture of Andrea Palladio and Le Corbusier: An Analysis of Colin Rowe’s Theory of Formal Complexity Using Fractal Dimensions. Fractal Fract. 2023, 7, 139. https://doi.org/10.3390/fractalfract7020139
Dawes MJ, Ostwald MJ, Lee JH. The Mathematics of ‘Natural Beauty’ in the Architecture of Andrea Palladio and Le Corbusier: An Analysis of Colin Rowe’s Theory of Formal Complexity Using Fractal Dimensions. Fractal and Fractional. 2023; 7(2):139. https://doi.org/10.3390/fractalfract7020139
Chicago/Turabian StyleDawes, Michael J., Michael J. Ostwald, and Ju Hyun Lee. 2023. "The Mathematics of ‘Natural Beauty’ in the Architecture of Andrea Palladio and Le Corbusier: An Analysis of Colin Rowe’s Theory of Formal Complexity Using Fractal Dimensions" Fractal and Fractional 7, no. 2: 139. https://doi.org/10.3390/fractalfract7020139
APA StyleDawes, M. J., Ostwald, M. J., & Lee, J. H. (2023). The Mathematics of ‘Natural Beauty’ in the Architecture of Andrea Palladio and Le Corbusier: An Analysis of Colin Rowe’s Theory of Formal Complexity Using Fractal Dimensions. Fractal and Fractional, 7(2), 139. https://doi.org/10.3390/fractalfract7020139







