Dynamic Analysis and Suppression Strategy Research on a Novel Fractional-Order Ferroresonance System

Abstract

Ferroresonance is characterized by overvoltage and irregular operation in power systems, which can greatly endanger system equipment. Mechanism analysis of the ferroresonance phenomenon depends mainly on model accuracy. Due to the fractional-order characteristics of capacitance and inductance, fractional-order models are more universal and accurate than integer-order models. A typical 110 kV ferroresonance model is first established. The influence of the excitation amplitude on the dynamic behavior is analyzed. The fractional-order ferroresonance model is then introduced, and the effects of the fractional order and flux-chain order on the system’s motion state are studied via bifurcation diagrams and phase portraits. In order to suppress the nonlinear dynamic behavior of fractional-order ferroresonance systems, a novel fractional-order fast terminal sliding mode control method based on finite-time theory and the frequency distributed model is proposed. A new fractional-order sliding mode surface and control law using a saturation function are developed. A robust fractional-order sliding mode controller could achieve finite-time stabilization and tracking despite model uncertainties and external disturbances. Compared with conventional sliding mode methods, the simulation results highlight the effectiveness and superiority. The research provides a theoretical basis for ferroresonant analysis and suppression in large-scale interconnected power grids.

1. Introduction

Ferroresonance is a complex nonlinear oscillation phenomenon in power systems [1,2] that is usually caused by the specific parameter values of nonlinear magnetic induction components, such as electromagnetic coupling power transformers, core magnetizing inductances, and stray or coupling capacitances [3,4]. The rapid changes in core flux for nonlinear magnetizing inductance may cause overvoltage or overcurrent with extremely abnormal waveforms, which may give rise to irreversible damage to the power equipment and endanger the security of power networks [5]. Unlike resonance, ferroresonant oscillations are related not only to the system frequency, but also to other parameters like the magnetic flux of the transformer, damping resistors, and reactors; the system voltage magnitude; and the switching transient and steady state. Several studies with respect to the ferroresonant oscillation phenomenon, which is caused by nonlinear characteristics of the circuit in power transformers, have been widely reported in the literature [6,7,8]. Djebli et al. [9] established an accurate dynamic model of a transformer considering the dependence of frequency and peak induction, and the impact of switching time and the eddy current in the core lamination on ferroresonant oscillations was studied. Heidary et al. [10] applied a new smart solid-state ferroresonance limiter (SSFL) to stabilize the chaotic oscillations of the voltage transformer (VT), and the influences of the proposed SSFL on the ferroresonance overvoltages were investigated. Morteza et al. [11] constructed a precise model of a single-phase transformer during ferroresonance considering a hysteresis model of the core, and the simulation results of fundamental and sub-harmonic ferroresonance modes were compared with the experimental results. Zou et al. [12] further established an accurate ferroresonance simulation model of a three-phase low-frequency transformer circuit and used the ferroresonance experiment to prove the precise prediction of the proposed model.

It can be found from the above literature that research on the ferromagnetic resonance phenomenon in power systems mainly focuses on resonant system parameters and the influence of external excitation on its dynamic behavior. The previous research results did not fully consider the influence of hysteresis. The results cannot reflect the chaotic characteristics of overvoltage effectively. Meanwhile, all of these studies indicate that the model accuracy and fidelity are very crucial to analyze ferroresonance behavior and suppression schemes in power transformers. Therefore, it is very essential to establish a more accurate model to describe the dynamic characteristics of ferroresonance systems and to reveal the mechanism and the influencing factors on internal parameter changes.

Fractional calculus, in various fields of science and engineering [13,14,15], plays a very important role in describing the long-range memory properties of energy storage elements like inductors, capacitors and dampers [16]. At present, fractional-order models and dynamic analysis of the capacitors and inductors have been widely studied in various circuit systems [17,18]. This is indicated by the fact that, due to nonlinear characteristics of circuit elements, the fractional-order and nonlinear nature of the ferroresonance should be taken into full consideration. Furthermore, Yang et al. [19] established a fractional-order ferroresonance model to study the influence of the order and different parameters on the dynamic behavior of the system, and chaotic synchronization based on a fractional-order adaptive sliding mode control method was investigated. However, this study presented ferroresonant circuits of a single-phase transformer, which is not suitable for power transformers with the addition of a winding in the secondary side, and without proposing a proper and effective suppression strategy to weaken the ferroresonance phenomenon. There are few studies on fractional-order ferroresonance. Thus, fractional-order ferroresonance oscillation models based on chaos theory will be employed in this paper to analyze the precise nonlinear behavior of power transformers.

In addition, to enhance the stability of the ferroresonance system, there are two main types of control methods for the suppression of the ferroresonance phenomenon. The first way is that directly changing the system parameters forces the system not to satisfy the ferroresonant conditions, and then the purpose of ferroresonance mitigation is achieved. The second way is to add a combination of damping resistors and reactors in potential transformers (PTs) and power transformers, which consume the energy generated during the ferroresonance process, and then the overvoltage is suppressed. However, ferroresonance may occur in power systems including those with modes of different frequencies, such as fundamental wave resonance, quasi-periodic resonance and chaotic resonance. Moreover, it is affected by many factors, such as the magnetic saturation level, circuit parameters and residual flux, especially for chaotic ferroresonance overvoltage, which shows that the transient voltage is irregular and difficult to predict, and the traditional suppression measures are difficult to implement.

Against this background, several methods to overcome the limitations of ferroresonance suppression associated with chaos control theory have been developed for power transformers [20,21]. To date, several methods and measures have emerged in ferroresonance elimination applications, such as the nonlinear feedback control method [22], the proportional integral (PI) control method [23], and the current-limiting method based on fault current limiter (FCL) [24]. Several scholars have generally been concerned about the hot issue of ferroresonance overvoltage mitigation in power systems. Sabiha et al. [25] proposed a mitigation method for ferroresonance overvoltage using the static compensator (STATCOM) in grid-connected wind energy conversion systems (WECSs). Mosaad et al. [26] provided a new ferroresonance mitigation technique for application of superconductors to improve the high-voltage ride-through capability in DFIG-WECS. Rezaei [27] designed an adaptive algorithm with a bidirectional protective relay to predict ferroresonant states in renewable energy networks. Rojas et al. [28] proposed a solution to the observed ferroresonance phenomenon, and further investigated the ferroresonance mitigation method, consists of the insertion of a shunt resistor in an unconventional rural electrification system.

From the above studies, it can be observed that the nature of ferroresonance is caused by complex nonlinear factors, and the prediction or measurement of ferroresonance mainly depends on the model accuracy, which makes the research content continue to develop in depth and breadth. Moreover, fractional calculus control theory also has obvious advantages in various engineering fields. In particular, finite-time stability combined with fractional calculus control theory [29], which is more advisable for achieve good dynamic performance, including better disturbance rejection property and stronger robustness against uncertainties. On this basis, fractional-order finite-time control has attracted more and more attention. Then, all kinds of fractional-order control methods have been investigated [30,31], such as fractional-order sliding mode control [32], fractional-order PID control [33], fractional-order finite-time control combined with the frequency distributed model [34], and fractional-order adaptive fuzzy control [35]. Although the above literature present the fractional-order control method to be applied to other chaotic system rather than chaotic ferroresonance circuits, the research ideas can be used for reference. Therefore, the ferroresonance problem of power systems still needs more in-depth research and discussion.

Inspired by the aforementioned work, the overall motivation of this paper is to reveal mechanism of ferroresonance phenomenon and explore suppression method of ferroresonance for power systems. The key innovation of this article is that the fractional-order model of the ferroresonance system is established, and a novel fractional-order fast terminal sliding mode control method is proposed. The frequency distributed model and the finite-time control method have also been applied in fractional-order ferroresonance systems. The proposed control method not only possesses the advantages of achieving stabilization and tracking with good robustness, but can also be realized within a finite time, providing a theoretical basis for dynamic parameter design and control. Therefore, it is significant to study the dynamic analysis and suppression strategy for the fractional-order ferroresonance system. The main contributions of this work are summarized as follows:

(1)

A typical 110 kV ferroresonance model is firstly established. The chaotic mechanism of the ferroresonance system and the influence of the excitation amplitude are analyzed comprehensively.

(2)

Based on the fractional calculus theory, a novel fractional-order ferroresonance model is established. The effects of the fractional order and the order of flux chain on the motion state of the system are studied through bifurcation diagrams and phase portraits.

(3)

By means of finite-time theory and the frequency distributed model, a novel fractional-order fast terminal sliding mode control method is proposed, which will solve the stabilization and tracking issue for the fractional-order ferroresonant system with uncertainties and disturbances.

The remaining part of this paper is organized as follows. In Section 2, the dynamic model of ferroresonance system is established, and the mechanism of the ferroresonance phenomenon and influence of the excitation amplitude are analyzed. In Section 3, dynamic analysis of the fractional-order ferroresonance system is investigated. Then, fractional-order finite-time sliding mode controller design is detailed in Section 4, including the frequency distributed model, finite-time controller design, stability analysis and the application and comparison of the proposed control scheme. Finally, some useful conclusions of this paper are provided in Section 5.

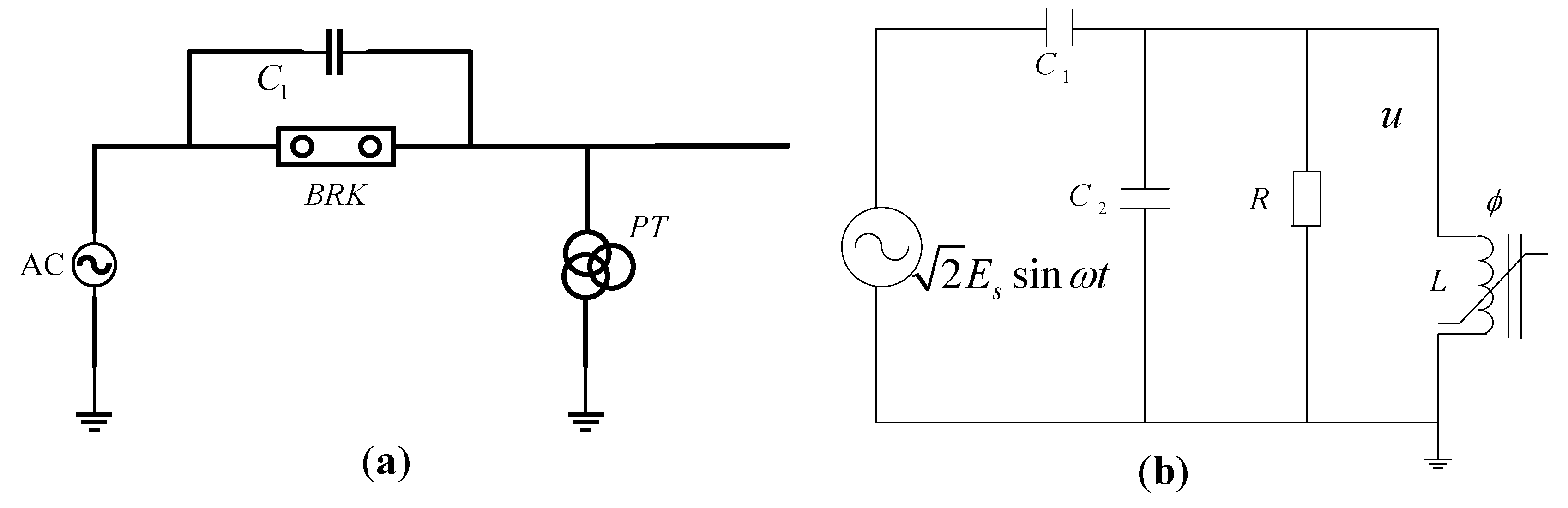
2. Modeling and Analysis of the Ferroresonance System

There are many core inductors and capacitors in the wind farm power system, such as power transformers, electromagnetic voltage transformers, reactors, ground and phase-to-phase capacitors and voltage equalization capacitors, which can be further composed to form a complex oscillation circuit. If the capacitance and inductance parameters reach a certain matching value, the ferromagnetic resonance may be excited, which will cause harm to the whole power grid system. Therefore, an accurate model is established to explore the cause and mechanism of ferromagnetic resonance overvoltage, and corresponding suppression measures are formulated. A typical 110 kV ferroresonant circuit is shown in Figure 1a [36], which consists of the equalizing capacitor C1 of the BRK (breaker) and the core inductor L of the electromagnetic potential transformer (PT). When the breaker is turned off, the interphase capacitance is ignored to simplify the resonant equivalent circuit as shown in Figure 1b.

In Figure 1b, $\sqrt{2}{E}_s\sin \left(\omega t\right)$ is the sinusoidal AC voltage source excitation, $C_1$ is the circuit breaker voltage equalizing capacitance, $C_2$ is the total stray capacitance of the equivalent system, $R$ is a transformer iron loss, $u$ is the coil terminal voltage, and $L$ is the core excitation inductor. The iron core inductor $L$ is regarded as a nonlinear inductor, the expression of its current can be obtained:

$$i_L=a{\varphi }^n+b\varphi ,n=3,5,7,9,11$$

(1)

where $i_L$ is the magnetizing current of the coil, $\varphi$ is a coil flux chain, and $n$ is the power of the flux chain. In fact, $b\varphi$ is a linear property of the model, $a{\varphi }^n$ is a nonlinear state variable considering magnetic saturation. Generally, this is:

$$\left\{\begin{array}{l}n=3\to a=1,b=1\\ n=7\to a=0.41,b=3.42\\ n=11\to a=0.14,b=3.42\end{array}\right.$$

(2)

According to these coefficients of Equation (2), the nonlinear magnetization curve of the inductor is shown in Figure 2. As the power $n$ increases, the magnetizing current $i_L$ also increases, and the curve $\varphi -i$ tends to flatten. The rate of the core saturation curve increases accordingly, and the inflection point can be reached when the current amplitude is lower.

Using Kirchhoff’s law of voltage and current, from Figure 1b, the mathematical model of the equivalent circuit can be described as:

$$\left\{\begin{array}{l}\frac{d\varphi }{dt}=u\\ \frac{du}{dt}=\frac{C_1}{C_1+C_2}\sqrt{2}{E}_s\omega \cos \left(\omega t\right)-\frac{a{\varphi }^n+b\varphi }{C_1+C_2}-\frac{u}{R\left(C_1+C_2\right)}\end{array}\right.$$

(3)

where $u$ is the coil terminal voltage, $\frac{C_1}{C_1+C_2}\sqrt{2}{E}_s\omega$ is the motivating factor, and $\frac{1}{R\left(C_1+C_2\right)}$ is a damping factor. In fact, when the excitation factor value is larger, the resonance is easier to excite. Moreover, the larger the damping factor value, the more the resonance can be suppressed.

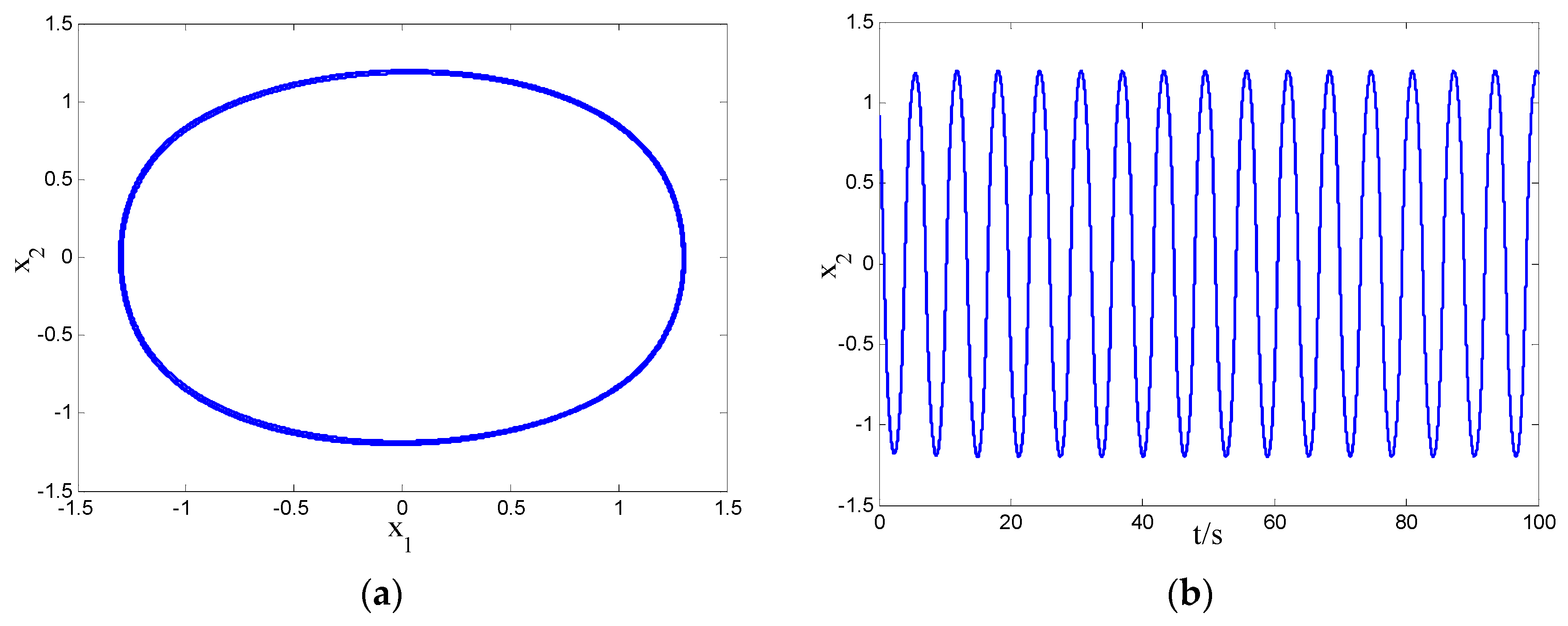
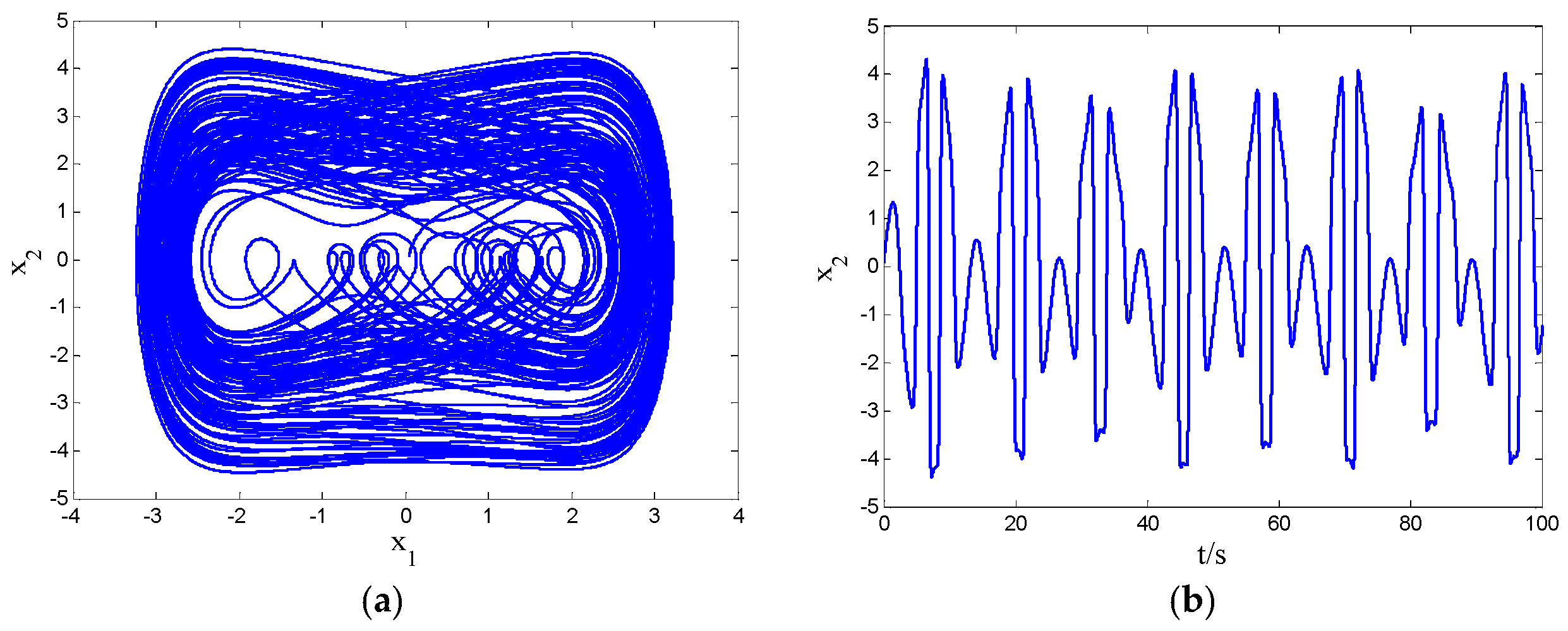
2.1. The Chaotic Mechanism

For the sake of analysis, let $\tau =\omega t$, $dt=\frac{1}{\omega }d\tau$; after normalization of Equation (3), the state equation can be obtained:

$$\left\{\begin{array}{l}\frac{d\varphi }{d\tau }=\frac{u}{\omega }\\ \frac{du}{d\tau }=\frac{C_1}{C_1+C_2}\sqrt{2}{E}_s\cos \left(\tau \right)-\frac{a{\varphi }^n+b\varphi }{\left(C_1+C_2\right)\omega }-\frac{u}{R\omega \left(C_1+C_2\right)}\end{array}\right.$$

(4)

Then, according to Equation (4), $x_1=\varphi$, $x_2=\frac{u}{\omega }$, we have:

$$\left\{\begin{array}{l}\frac{d{x}_1}{d\tau }=x_2\\ \frac{d{x}_2}{d\tau }=\frac{C_1}{\left(C_1+C_2\right)\omega }\sqrt{2}{E}_s\cos \left(\tau \right)-\frac{a{x}_1^n+b{x}_1}{\left(C_1+C_2\right){\omega }^2}-\frac{x_2}{R\omega \left(C_1+C_2\right)}\end{array}\right.$$

(5)

The typical parameters for a 110 kV substation model are as follows: voltage reference $U_b=63.51\mathrm{kV}$, $E_s=63.51\mathrm{kV}$, $\omega =2\pi \times 50\mathrm{rad/s}$, $C_1=2500\mathrm{pF}$, $C_2=100\mathrm{pF}$, $R=1000\mathrm{M}\Omega$, and the corresponding per unit value of these are: $\omega =1\mathrm{pu}$, $C=2200\mathrm{pF}\to 0.022\mathrm{pu}$, $R=1000\mathrm{M}\Omega \to 1\mathrm{pu}$. When the external incentive is zero, i.e., $\frac{C_1}{\left(C_1+C_2\right)\omega }\sqrt{2}E\cos \left(\tau \right)=0$, the system becomes autonomous (excluding time $t$ ). Then, the origin $O\left(0,0\right)$ is the unique equilibrium point, and its Jacobi matrix can be given by:

$$J=\left[\begin{array}{cc}0& 1\\ -0.0186\left(n{x}_1^{n-1}+1\right)& -0.0186\end{array}\right]$$

(6)

At the equilibrium point $O\left(0,0\right)$, the corresponding characteristic roots of the system are ${\lambda }_{1,2}=-0.0093\pm 0.136i$. According to Melnikov’s theory, the autonomous system is stable at the equilibrium point, if the system enters into the chaotic state, and the external excitation must not be zero.

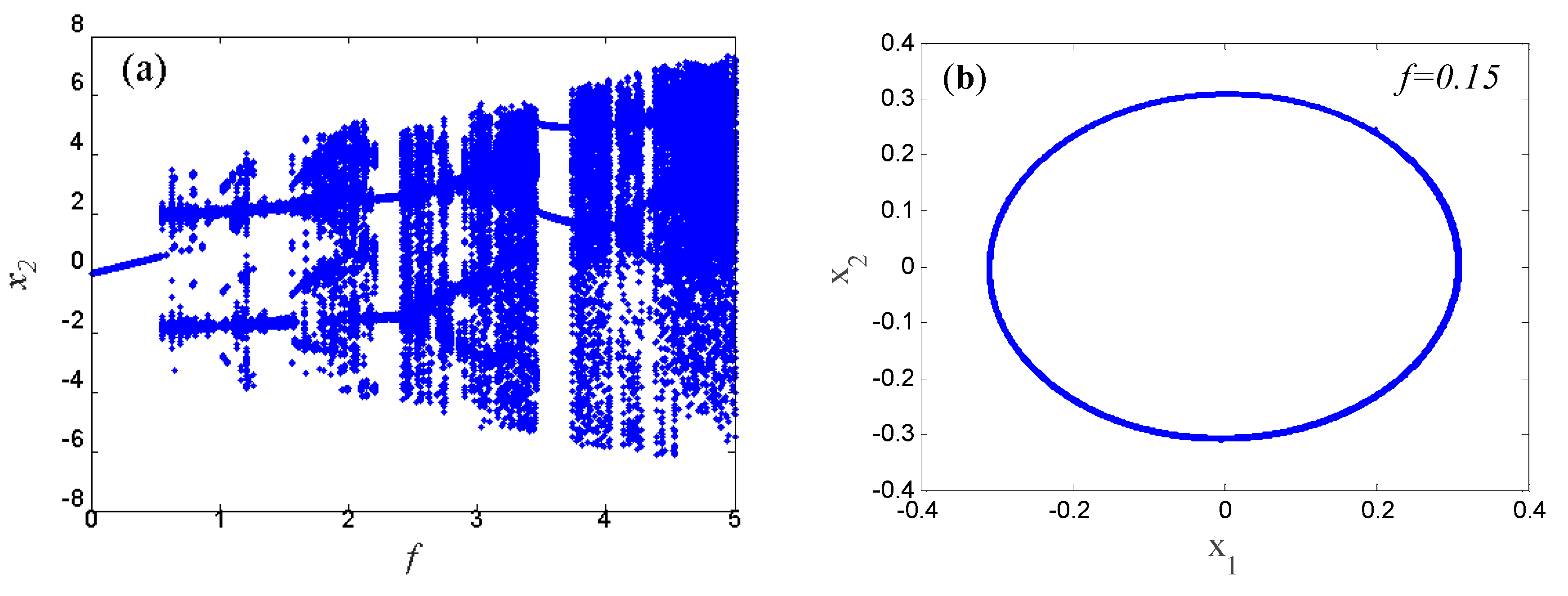
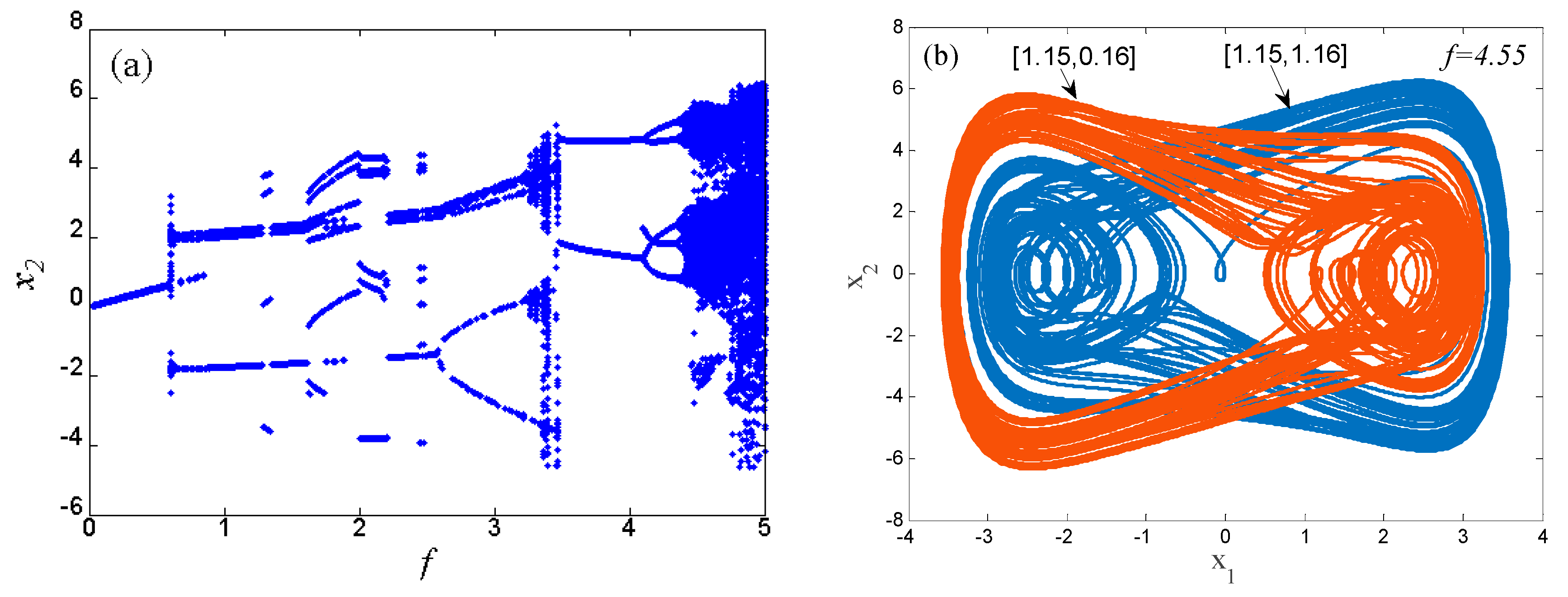
2.2. Excitation Amplitude Effects

To gain further insight into the dynamic characteristics of the ferroresonance in the power system (5) subjected to external excitation, the amplitude of external excitation is selected as the bifurcation parameter. The initial conditions are set as $\left[x_1,x_2\right]=\left[0.05,0.06\right]$. The remaining system parameters are held constant. As clearly illustrated in Figure 3, the nonlinear dynamic behavior is highly complex. Specifically, it can be observed that as the excitation amplitude increases, the system undergoes a period-doubling bifurcation phenomenon, and the oscillation response process transitions from period 1→ period doubling → chaotic motion.

When the excitation amplitude is taken as $f=0.45$, the phase diagram and time history diagram of the oscillation response are shown in Figure 4. The system maintains a single-period motion state—that is to say, it is in a low-frequency periodicity oscillation state.

With the increase in the excitation amplitude, as depicted in Figure 5, when $f=0.75$, the system enters a periodic motion state, showcasing a four-phase cycle in both the phase diagram and time history diagram. In this state, the system experiences multi-period low-frequency oscillation. When $f=1.3598$, from Figure 6, the system’s phase diagram and time history reveal a transition to a chaotic state, marked by a chaotic singular attractor in the phase space. This indicates high sensitivity to both the excitation amplitude and initial values. In addition, the value of Feigenbaum can be obtained as 4.669201669 through theoretical analysis and calculation. This also means that the voltage or current of the system should be subject to significant fluctuations. These fluctuations can lead to system malfunction, posing a threat to the power system’s safety.

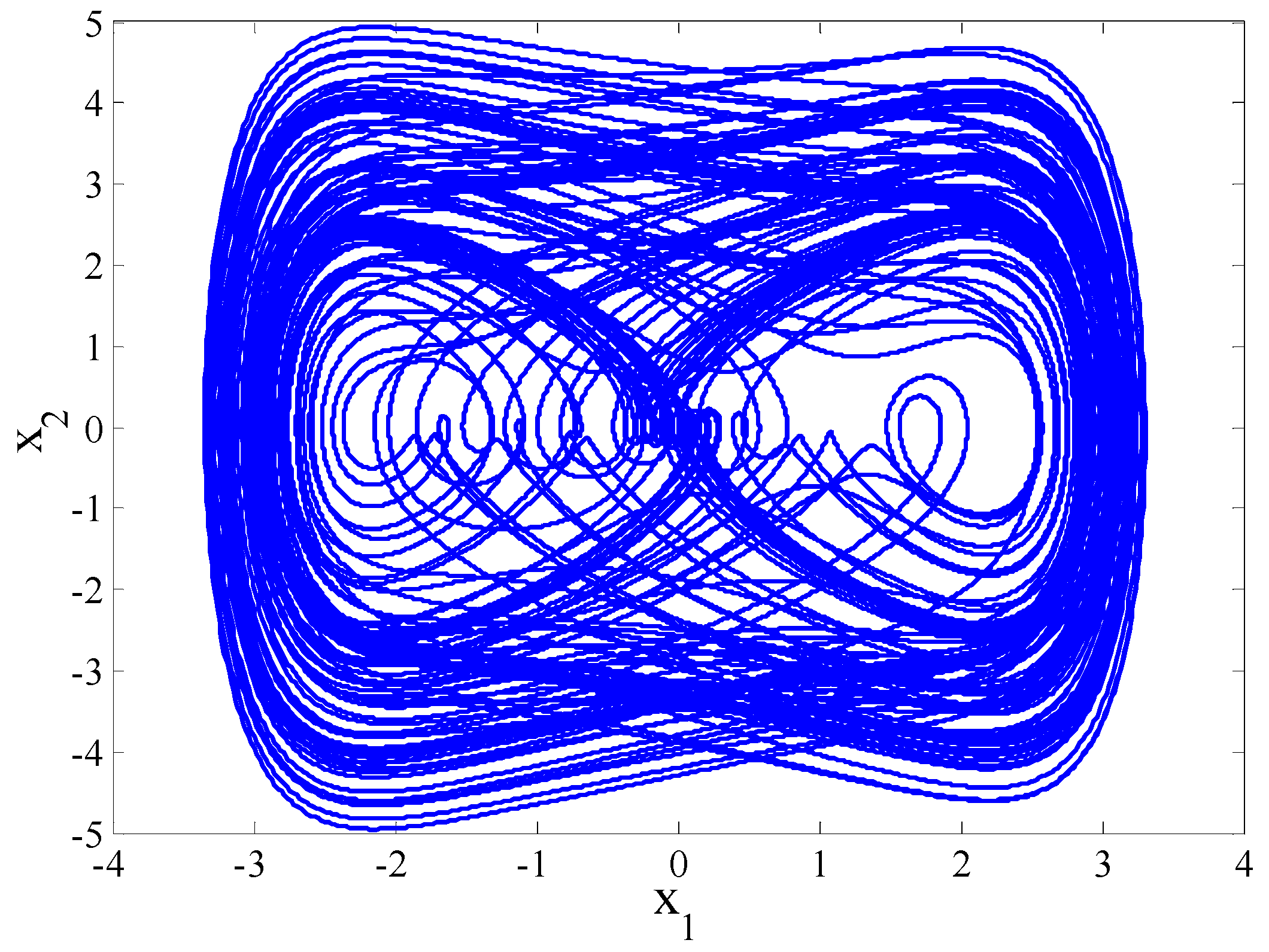
3. Dynamic Analysis of the Fractional-Order Ferroresonance System

In this subsection, the definitions and some useful lemmas of fractional-order derivatives are recalled. Then, following the integer-order model in Equation (5), the fractional-order ferroresonance model can be established based on fractional-order calculus theory.

Definition 1.

[37] The αth-order Caputo fractional integral of a function  $f\left(t\right)$  is described by:

$${}_{t_0}{}^{C}I_t^{\alpha }f\left(t\right)=\frac{1}{\Gamma \left(\alpha \right)}{\displaystyle \underset{t_0}{\overset{t}{\int }}{\left(t-\tau \right)}^{\alpha -1}f\left(\tau \right)d\tau }$$

(7)

If  $f\left(t\right)=t^{\beta }$  with  $\beta >-1$ , then  ${}_{t_0}{}^{C}I_t^{\alpha }f\left(t\right)=\frac{\Gamma \left(\beta +1\right)}{\Gamma \left(\alpha +\beta +1\right)}{t}^{\alpha +\beta },t>0$.

Definition 2.

[37] The αth-order Caputo fractional derivative of a function  $f\left(t\right)$  is defined as:

$${}_{t_0}{}^{C}D_t^{\alpha }f\left(t\right)=\left\{\begin{array}{l}\frac{1}{\Gamma \left(m-\alpha \right)}{\displaystyle \underset{t_0}{\overset{t}{\int }}\frac{f^{\left(m\right)}\left(\tau \right)}{{\left(t-\tau \right)}^{\alpha -m+1}}d\tau ,m-1<\alpha <m}\\ \frac{d^{m}f\left(t\right)}{d{t}^m},\alpha =m\end{array}\right.$$

(8)

 where  $m$  is the smallest integer number.

Definition 3.

[37] The αth-order Riemann–Liouville (R-L) fractional derivative of a function  $f\left(t\right)$  is defined as:

$${}_{t_0}{}^{RL}D_t^{\alpha }f\left(t\right)=\frac{1}{\Gamma \left(m-\alpha \right)}\frac{d^m}{d{t}^m}{\displaystyle \underset{t_0}{\overset{t}{\int }}\frac{f\left(\tau \right)}{{\left(t-\tau \right)}^{\alpha -m+1}}d\tau ,m-1<\alpha <m}$$

(9)

Lemma 1.

[38] If  $0<\alpha <1$ ,  $f\left(t\right)\in C$ , R-L and Caputo fractional derivatives can be associated with each other as follows:

$${}_{t_0}{}^{C}D_t^{\alpha }f\left(t\right)={}_{t_0}{}^{RL}D_t^{\alpha }f\left(t\right)-\frac{f\left(t_0\right)}{\Gamma \left(1-\alpha \right){\left(t-t_0\right)}^{\alpha }}$$

(10)

Particularly, when  $t_0=0$ , then  ${}_{t_0}{}^{RL}D_t^{\alpha }f\left(t\right)={}_{t_0}{}^{C}D_t^{\alpha }f\left(t\right)+\frac{f\left(0\right)}{\Gamma \left(1-\alpha \right)}{t}^{-\alpha }$.

Lemma 2.

[39] Consider the differential equation system  $\dot{x}=f(x)$ , if there is a function  $V(x)$  that is continuous and positive-definite, and we have:

$$\dot{V}\left(x\right)\le -a{V}^{1-\frac{1}{2\rho }}\left(x\right)-b{V}^{1+\frac{1}{2\rho }}\left(x\right)$$

where  $a>0$ ,  $b>0$  and  $\rho >0$  are three constants. Then, the system can realize fixed-time stability. When  $x=0\iff V\left(x\right)=0$ , the fixed time  $T$  is estimated as  $T=\frac{\pi \rho }{\sqrt{ab}}$.

In the previous section, the chaotic dynamic characteristics of the integer-order ferromagnetic resonance model under external excitation are introduced in detail. However, the nonlinear elements such as capacitors and inductors may not be completely integer-order state, and the introduction of fractional-order parameters can more accurately describe their essential characteristics. In view of this, based on the fractional-order calculus theory, the integer-order ferromagnetic resonance model (5) can be extended to fractional-order form. In order to study the typical nonlinear dynamic behaviors of the fractional-order ferroresonance system, the mathematical expression can be written as follows:

$$\left\{\begin{array}{l}\frac{d^{\alpha }{x}_1}{d{\tau }^{\alpha }}=x_2\\ \frac{d^{\alpha }{x}_2}{d{\tau }^{\alpha }}=\frac{C_1}{\left(C_1+C_2\right)\omega }\sqrt{2}{E}_s\cos \left(\tau \right)-\frac{a{x}_1^n+b{x}_1}{\left(C_1+C_2\right){\omega }^2}-\frac{x_2}{R\omega \left(C_1+C_2\right)}\end{array}\right.$$

(11)

where $\alpha$ represents fractional order, by applying the generalized Adams–Bashforth–Moulton method [40], the fractional differential system’s dynamic response can be computed by solving Equation (11) in MATLAB. On this basis, the chaotic or bifurcation diagram in regard to the fractional order $\alpha$ and other parameters can be obtained.

As shown in Figure 7, set the order to $\alpha =0.99$, the flux linkage index to $n=3$, and the excitation amplitude to $f\in \left[0,5\right]$, with the other parameters as above. It can be shown in Figure 7a that the ferromagnetic resonance system exhibits multiple periodic windows as the external excitation amplitude $f$ increases. Within the simulation region, it is chaotic both before and after the periodic states, indicating an alternating pattern of periodic and chaotic states. This observation supports the notion that chaotic motion evolves from periodic states.

As shown in Figure 7b, when $f=0.15$, the oscillatory response of the system is in a periodic 1 motion state, and the corresponding bifurcation diagram reveals a shrinking and overlapping polyline. When $f=0.75$, the system’s oscillation response shifts to a period-doubling motion state, as depicted in Figure 7c. Further, when $f=1.3598$, the system enters a chaotic oscillation state. As can be shown in Figure 7d, the state space trajectory of the system exhibits a distinctive double scroll shape. This means that the system is in an overvoltage state.

In order to demonstrate the influence of fractional-order variation on the dynamic behavior of the ferromagnetic resonance system, as shown in Figure 8a, when the power of the fractional order is set as $\alpha =0.97$, and the flux chain $n=3$, all other parameters remain unchanged, we explore the bifurcation parameter in the range $0\le f\le 5$. Notably, the chaotic region is becoming smaller, and the system predominantly exhibits periodic motion within most parameter ranges. This emphasizes the close relationship between the system’s bifurcation and chaotic behavior and the fractional order.

When $f=4.55$, the system enters a chaotic oscillation state, as illustrated in Figure 8b. Because of the sensitivity of chaotic systems to initial values, we select the initial conditions of $\left[1.15,1.16\right]$ and $\left[1.15,0.16\right]$, the resulting phase space trajectory displays an approximate symmetric structure with various forms of attractors. This observation underscores that the resonant state of the system is influenced not only by the magnitude of the external excitation amplitude but also by other factors.

We delve deeper into the influence of order variation in the inductor flux chain on the dynamic behavior of the ferromagnetic resonance system, as shown in Figure 9a,b. The fractional order is set to $\alpha =0.99$, and parameters such as the order of flux chain $n=7$, the excitation amplitude $f=4$, the initial value $\left[1.15,1.16\right]$, and other parameters are the same as above. It can be shown in Figure 9 that similar to Figure 7, the ferroresonance system also exhibits multiple periodic windows with the increase of the external excitation amplitude $f$. The key distinction lies in the bifurcation parameters corresponding to different chaotic states, as illustrated in Figure 9b. The chaotic attractor in the system phase space consistently maintains a ring shape, and there is not a divergence trend. Compared with Figure 7d, the chaotic motion is more intense, signifying that the increase in the external excitation amplitude and the order of flux chain would cause the system to enter the ferroresonant chaotic state.

When considering the order of flux chain $n=11$, the order of the fractional order $\alpha =0.99$, and the initial value $\left[0.15,0.16\right]$, as depicted in Figure 10a, the bifurcation diagram of the ferromagnetic resonance system is displayed. With the increase in the excitation amplitude $f$, the motion of the system goes through the process of bifurcation, period doubling and chaotic changes. And when the excitation amplitude reaches a specific range, the system would be in a ferromagnetic resonance chaotic state. For instance, taking the excitation amplitude as $f=4$, the chaotic attractor is vividly depicted in Figure 10b. The ring shape and changing trend of the chaotic attractor in the phase space are similar to Figure 9b, displaying typical chaotic motion characteristics.

As can be shown in Figure 7, Figure 9 and Figure 10, it becomes evident that with the increase in the order of flux chain, the scatter region would gradually transition to the chaotic region in the bifurcation diagram. The system, upon entering the chaotic state, becomes extremely complex. Simulation results highlight that extending the ferromagnetic resonance system to fractional calculus introduces significant changes in the state variables. Consequently, the system is more prone to falling into periodic oscillation or even a chaotic state, indicating a heightened susceptibility to ferromagnetic resonance faults.

4. Design of a Fractional-Order Finite-Time Sliding Mode Controller

At present, ferroresonance faults in power systems are mostly analyzed and studied under the condition of integer order. In the previous section, the chaotic dynamic behavior of the fractional-order model is analyzed in detail. This indicates that it is essential for the stability of the system, which is badly harmful to the power system. Therefore, it is very significant to study the chaotic oscillation control of the fractional-order ferroresonant system.

4.1. The Frequency Distributed Model

In this paper, in order to suppress the chaotic oscillation of the fractional-order ferroresonant system, a novel fractional-order fast terminal sliding mode control strategy based on finite-time theory and the frequency distributed model is proposed. Meanwhile, to further illustrate the proposed control method, a frequency distributed model is introduced firstly. Then, based on finite-time stability theory, an appropriate sliding mode controller is proposed including construction of the sliding surface and design of a robust control law. Finally, the simulation comparison is applied to demonstrate the validity and superiority of the proposed method.

To obtain the main results of this paper, the nonlinear fractional-order system is described as follows:

$$D^{\alpha }x(t)=F(x,t),\alpha \in \left(0,1\right)$$

(12)

Then, a time–frequency domain auxiliary function is defined as:

$$\varphi \left(\omega ,t\right)={\displaystyle \underset{0}{\overset{t}{\int }}{e}^{-{\omega }^2(t-\tau )}}F(x,t)d\left(\tau \right)$$

(13)

Lemma 3.

[41] The nonlinear fractional-order system (12) can be reformulated into the following frequency distributed model form:

$$\left\{\begin{array}{l}\frac{\partial \varphi \left(\omega ,t\right)}{\partial t}=-{\omega }^2\varphi \left(\omega ,t\right)+F\left(x,t\right)\\ x(t)={\displaystyle {\int }_0^{\infty }\mu (\omega )\varphi \left(\omega ,t\right)d\omega }\\ \mu \left(\omega \right)=\frac{2\sin \left(\alpha \pi \right)}{\pi }{\omega }^{1-2\alpha }\end{array}\right.$$

(14)

4.2. Finite-Time Control

For the fractional-order ferromagnetic resonance model, the dynamic characteristics of the system are usually affected by model uncertainties and external disturbances, that is, the system (11) can be expressed as the following controlled form.

$$\left\{\begin{array}{l}{D}^{\alpha }{x}_1=x_2\\ D^{\alpha }{x}_2=f\left(x,t\right)+\Delta f\left(x,t\right)+d\left(t\right)+u\left(t\right)\end{array}\right.$$

(15)

where $\alpha \in \left(0,1\right)$ is the fractional order, $f\left(x,t\right)$ is the original term of the system, $\Delta f\left(x,t\right)$ is the uncertainty term of the model, $d\left(t\right)$ is the external disturbance term, and $u\left(t\right)$ is the control input.

Assumption 1.

The state of the fractional-order ferroresonance model is globally bounded, and the upper bound of the model uncertainty can be estimated. That is, there exist two constants  ${\theta }_1>0$  and  ${\theta }_2>0$ , which satisfy the uncertainty term  $\Delta f\left(x,t\right)$  and the external disturbance term  $d\left(t\right)$ :

$$\left|\Delta f\left(x,t\right)\right|\le {\theta }_1,\left|d\left(t\right)\right|\le {\theta }_2$$

(16)

Definition 4.

Choose  $x_d$  as a reference signal, the tracking error is defined as  $e_1=x_1-x_d$ , if there is an arithmetic number  $T=T\left(e_1(0)\right)$ , and the solution of tracking error satisfies:

$$\underset{t\to T}{\lim }‖e_1\left(t\right)‖=0$$

(17)

That is, when  $t\ge T$ ,  $‖e_1\left(t\right)‖\equiv 0$ . So the system will be stable in a finite time  $T$.

Based on Definition 4, we have $D^{\alpha }{e}_1=D^{\alpha }{x}_1-D^{\alpha }{x}_d$. Therefore, by means of Equation (15), the error equation can be expressed as:

$$\left\{\begin{array}{l}{D}^{\alpha }{e}_1=e_2\\ D^{\alpha }{e}_2=f\left(x,t\right)+\Delta f\left(x,t\right)+d\left(t\right)+u\left(t\right)-D^{\alpha }{x}_d\end{array}\right.$$

(18)

According to the design steps of a sliding mode controller, an appropriate sliding surface is constructed firstly, and a novel fractional-order fast terminal sliding mode surface is designed:

$$s(t)=D^{\alpha -1}{e}_2+{\displaystyle {\int }_0^t\left(r_1{e}_1+r_2{\left|e_1\right|}^{c_1}sat\left(e_1\right)\right)dt}$$

(19)

where $s(t)={\left[s_1,s_2\right]}^T$ is the sliding mode surface, $r_1$, $r_2$, $c_1$ is the given sliding mode surface parameter, and meets $r_1>0$, $r_2>0$, $0<c_1<1$, in order to mitigate the chattering phenomenon of the system, the saturation function is usually used to replace the symbolic function, then the saturation function $sat\left(·\right)$ can be expressed as follows:

$$sat(\varphi )=\left\{\begin{array}{l}\raisebox{1ex}{$\varphi $}\!\left/ \!\raisebox{-1ex}{$k$}\right.,\left|\frac{\varphi }{k}\right|\le 1,k>0\\ sign(\raisebox{1ex}{$\varphi $}\!\left/ \!\raisebox{-1ex}{$k$}\right.),\left|\frac{\varphi }{k}\right|>1,k>0\end{array}\right.$$

(20)

When the state trajectory of the system reaches the sliding mode surface (19), one that satisfies $s\left(t\right)=0$, the following equation can be derived:

$$D^{\alpha -1}{e}_2=-{\displaystyle {\int }_0^t\left(r_1{e}_1+r_2{\left|e_1\right|}^{c_1}sat\left(e_1\right)\right)dt}\to D^{\alpha }{e}_2=-r_1{e}_1-r_2{\left|e_1\right|}^{c_1}sat\left(e_1\right)$$

(21)

This means that, if and only if the Equation (21) is stable, the fractional-order sliding surface (19) will converge to zero. In order to prove its stability, a corresponding fractional-order controller is designed, and then the Lyapunov stability theorem is given below.

Theorem 1.

If the sliding mode surface is chosen as the form of Equation (19), then the error dynamic system (21) is stable, and the error state trajectories will converge to zero.

Proof. 

According to the frequency distribution model in the above content, the error dynamic system (21) can be expressed as:

$$\left\{\begin{array}{l}\frac{\partial \varphi \left(\omega ,t\right)}{\partial t}=-{\omega }^2\varphi \left(\omega ,t\right)-\left(r_1{e}_1+r_2{\left|e_1\right|}^{c_1}sat\left(e_1\right)\right)\\ e_1(t)={\displaystyle {\int }_0^{\infty }\mu (\omega )\varphi \left(\omega ,t\right)d\omega }\end{array}\right.$$

(22)

Suppose that there are two continuous Lyapunov functions, and they satisfy:

(1)

$v\left(\omega ,t\right)$ represents the monotonic Lyapunov function about fundamental frequency $\omega$;

(2)

$V_1(t)$ represents the Lyapunov function, which is the sum of the products of all weighting functions $\mu (\omega )$ and monotonic function $\mu (\omega )$;

Then, this specific expression can be obtained:

$$v\left(\omega ,t\right)=\frac{{\varphi }^2\left(\omega ,t\right)}{2},V_1(t)={\displaystyle {\int }_0^{\infty }\mu (\omega )v\left(\omega ,t\right)d\omega }$$

(23)

Based on Equation (23), taking its time derivative, we have:

$$\frac{d{V}_1}{dt}={\displaystyle {\int }_0^{\infty }\mu (\omega )\frac{\partial v\left(\omega ,t\right)}{\partial t}d\omega }={\displaystyle {\int }_0^{\infty }\mu (\omega )\varphi \left(\omega ,t\right)\frac{\partial \varphi \left(\omega ,t\right)}{\partial t}d\omega }$$

(24)

Substituting (22) into (24), we have:

$$\begin{array}{ll}\hfill \frac{d{V}_1}{dt}& ={\displaystyle {\int }_0^{\infty }\mu (\omega )\varphi \left(\omega ,t\right)\left(-{\omega }^2\varphi \left(\omega ,t\right)-\left(r_1{e}_1+r_2{\left|e_1\right|}^{c_1}sat\left(e_1\right)\right)\right)d\omega }\hfill \\ & =-{\displaystyle {\int }_0^{\infty }\mu (\omega ){\omega }^2{\varphi }^2\left(\omega ,t\right)d\omega }-\left(r_1{e}_1+r_2{\left|e_1\right|}^{c_1}sat\left(e_1\right)\right){\displaystyle {\int }_0^{\infty }\mu (\omega )\varphi \left(\omega ,t\right)d\omega }\\ & =-{\displaystyle {\int }_0^{\infty }\mu (\omega ){\omega }^2{\varphi }^2\left(\omega ,t\right)d\omega }-\left(r_1{e}_1+r_2{\left|e_1\right|}^{c_1}sat\left(e_1\right)\right)e_1\\ & =-{\displaystyle {\int }_0^{\infty }\mu (\omega ){\omega }^2{\varphi }^2\left(\omega ,t\right)d\omega }-r_1{e}_1^2-r_2{\left|e_1\right|}^{c_1}{e}_{1}sat\left(e_1\right)\end{array}$$

(25)

According to the definition of the saturation function $sat\left(·\right)$ in Equation (20), it can be obtained:

$$e_1\times sat\left(e_1\right)=\left\{\begin{array}{ll}{e}_1\times sign\left(\raisebox{1ex}{$e_1$}\!\left/ \!\raisebox{-1ex}{$k$}\right.\right)=k\frac{e_1}{k}sign\left(\raisebox{1ex}{$e_1$}\!\left/ \!\raisebox{-1ex}{$k$}\right.\right)=\left|e_1\right|& \left|\frac{e_1}{k}\right|>1\hfill \\ \frac{e_1^2}{k}& \left|\frac{e_1}{k}\right|\le 1\hfill \end{array}\right.,k>0$$

(26)

Equation (26) is substituted into Equation (25), and we conclude that $\frac{d{V}_1}{dt}<0$. Based on Lyapunov stability theory, the error dynamic system (21) is stable, and its state trajectories will converge to zero.□

In order to make the fractional terminal sliding mode surface (19) converge to zero in finite time, the sliding mode reaching law $\dot{s}\left(t\right)=\left(-r_3{\left|s\right|}^{c_2}-r_4{\left|s\right|}^{c_3}\right)sign\left(s\right)$ is designed in advance. According to Assumption 1, to ensure that the state trajectory reaches the preset sliding surface and maintains it, the sliding mode control law is constructed as follows:

$$\begin{array}{ll}\hfill u\left(t\right)=& -\frac{e_2\left(0\right)}{\Gamma \left(1-\alpha \right)}{t}^{-\alpha }-f\left(x,t\right)-r_1{e}_1-r_2{\left|e_1\right|}^{c_1}sat\left(e_1\right)+D^{\alpha }{x}_d\hfill \\ & -\left(r_3{\left|s\right|}^{c_2}+r_4{\left|s\right|}^{c_3}+{\theta }_1+{\theta }_2\right)sign\left(s\right)\end{array}$$

(27)

where $r_3$, $r_4$, $c_2$, $c_3$, and $\epsilon$ are the given control parameters and meet $r_3>0$, $r_4>0$, $c_2=1-\frac{1}{2\epsilon }$, $c_3=1+\frac{1}{2\epsilon }$, and $\epsilon \ge \frac{1}{2}$.

Theorem 2.

Under Assumption 1, consider that the system (15) satisfies the sliding mode surface (19), and when the action of the control law (27) is taken, the state trajectories of the system will converge to the sliding mode surface  $s\left(t\right)=0$  in a finite time.

Proof. 

The positive definite Lyapunov function is selected as $V_2\left(t\right)=\left|s\right|$, taking its time derivative, we obtain:

$${\dot{V}}_2\left(t\right)=sign\left(s\right)\dot{s}$$

(28)

Meanwhile, taking the time derivative of the sliding surface (19), there is:

$$\dot{s}={}_{t_0}{}^{RL}D_t^{\alpha }{e}_2+r_1{e}_1+r_2{\left|e_1\right|}^{c_1}sat\left(e_1\right),{}_{t_0}{}^{RL}D_t^{\alpha }{e}_2={}_{t_0}{}^{C}D_t^{\alpha }{e}_2+\frac{e_2\left(0\right)}{\Gamma \left(1-\alpha \right)}{t}^{-\alpha }$$

(29)

Substituting Equation (29) into Equation (28), we have:

$${\dot{V}}_2\left(t\right)=sign\left(s\right)\left({}_{t_0}{}^{C}D_t^{\alpha }{e}_2+\frac{e_2\left(0\right)}{\Gamma \left(1-\alpha \right)}{t}^{-\alpha }+r_1{e}_1+r_2{\left|e_1\right|}^{c_1}sat\left(e_1\right)\right)$$

(30)

Consider $D^{\alpha }{e}_2=f\left(x,t\right)+\Delta f\left(x,t\right)+d\left(t\right)+u\left(t\right)-D^{\alpha }{x}_d$, Equation (30) can be equivalent to:

$${\dot{V}}_2\left(t\right)=sign\left(s\right)\left(\begin{array}{l}f\left(x,t\right)+u\left(t\right)-D^{\alpha }{x}_d+\frac{e_2\left(0\right)}{\Gamma \left(1-\alpha \right)}{t}^{-\alpha }\\ +r_1{e}_1+r_2{\left|e_1\right|}^{c_1}sat\left(e_1\right)\end{array}\right)+\left(\begin{array}{l}\Delta f\left(x,t\right)\\ +d\left(t\right)\end{array}\right)sign\left(s\right)$$

(31)

According to Assumption 1, it can be deduced that:

$$\left(\Delta f\left(x,t\right)+d\left(t\right)\right)sign\left(s\right)\le \left(\left|\Delta f\left(x,t\right)\right|+\left|d\left(t\right)\right|\right)\left|sign\left(s\right)\right|\le {\theta }_1+{\theta }_2$$

(32)

Following Equation (32), Equation (27) is substituted into Equation (31), and it can easily be concluded that:

$${\dot{V}}_2\left(t\right)\le -sign\left(s\right)\left(\begin{array}{l}{r}_3{\left|s\right|}^{c_2}+r_4{\left|s\right|}^{c_3}\\ +{\theta }_1+{\theta }_2\end{array}\right)sign\left(s\right)+{\theta }_1+{\theta }_2\le -r_3{\left|s\right|}^{c_2}-r_4{\left|s\right|}^{c_3}<0$$

(33)

According to Lyapunov stability theory, the fractional-order system (15) is asymptotically stable. To prove that the system converges stably in a finite time, the inequality (33) can be simplified as:

$$\frac{d{V}_2}{dt}=\frac{d\left|s\right|}{dt}\le -\left(r_3{\left|s\right|}^{c_2}+r_4{\left|s\right|}^{c_3}\right)$$

(34)

Obviously, we yield,

$$dt\le -\frac{d\left|s\right|}{\left(r_3{\left|s\right|}^{c_2}+r_4{\left|s\right|}^{c_3}\right)}$$

(35)

Using the containment relationships among $c_2$ and $c_3$ in Equation (24), considering the first-order integration on both sides of the Equation (35) from $0$ to $t_r$, it is easy to derive that

$${\displaystyle {\int }_0^{t_r}dt}\le -{\displaystyle {\int }_{s\left(0\right)}^{s\left(t_r\right)}\frac{d\left|s\right|}{\left(r_3{\left|s\right|}^{c_2}+r_4{\left|s\right|}^{c_3}\right)}}={\displaystyle {\int }_{s\left(t_r\right)}^{s\left(0\right)}\frac{1}{\left(r_3{\left|s\right|}^{1-\frac{1}{2\epsilon }}+r_4{\left|s\right|}^{1+\frac{1}{2\epsilon }}\right)}}d\left|s\right|$$

(36)

Let $z={\left|s\right|}^{\frac{1}{2\epsilon }}$, $s\left(t_r\right)=0$, Equation (36) can be rewritten as:

$$\begin{array}{c}{\left.t\right|}_0^{t_r}=t_r\le {\displaystyle {\int }_0^{s^{\frac{1}{2\epsilon }}\left(0\right)}\frac{2\epsilon z^{2\epsilon -1}}{\left(r_3{z}^{2\epsilon -1}+r_4{z}^{2\epsilon +1}\right)}}dz={\displaystyle {\int }_0^{s^{\frac{1}{2\epsilon }}\left(0\right)}\frac{2\epsilon }{\left(r_3+r_4{z}^2\right)}}dz\\ =\frac{2\epsilon }{\sqrt{r_3{r}_4}}\arctan \left(\sqrt{\frac{r_4}{r_3}}{s}^{\frac{1}{2\epsilon }}\left(0\right)\right)\le \frac{2\epsilon }{\sqrt{r_3{r}_4}}\cdot \frac{\pi }{2}=\frac{\pi \epsilon }{\sqrt{r_3{r}_4}}\end{array}$$

(37)

Based on Definition 4, the state trajectories of the system (6)–(24) will converge to the sliding mode surface $s\left(t\right)=0$ within a finite time $t_r\le \frac{\pi \epsilon }{\sqrt{r_3{r}_4}}$.□

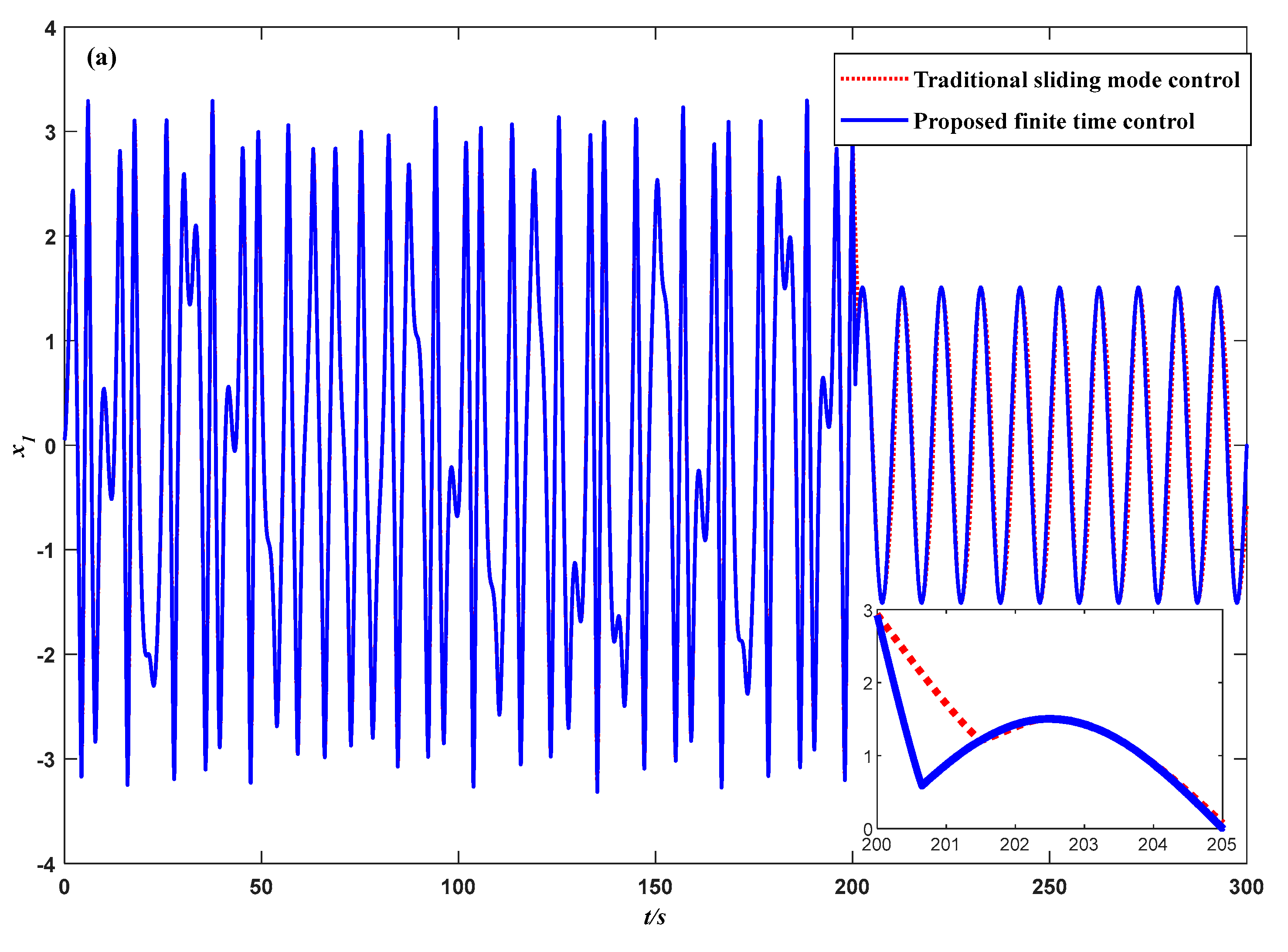
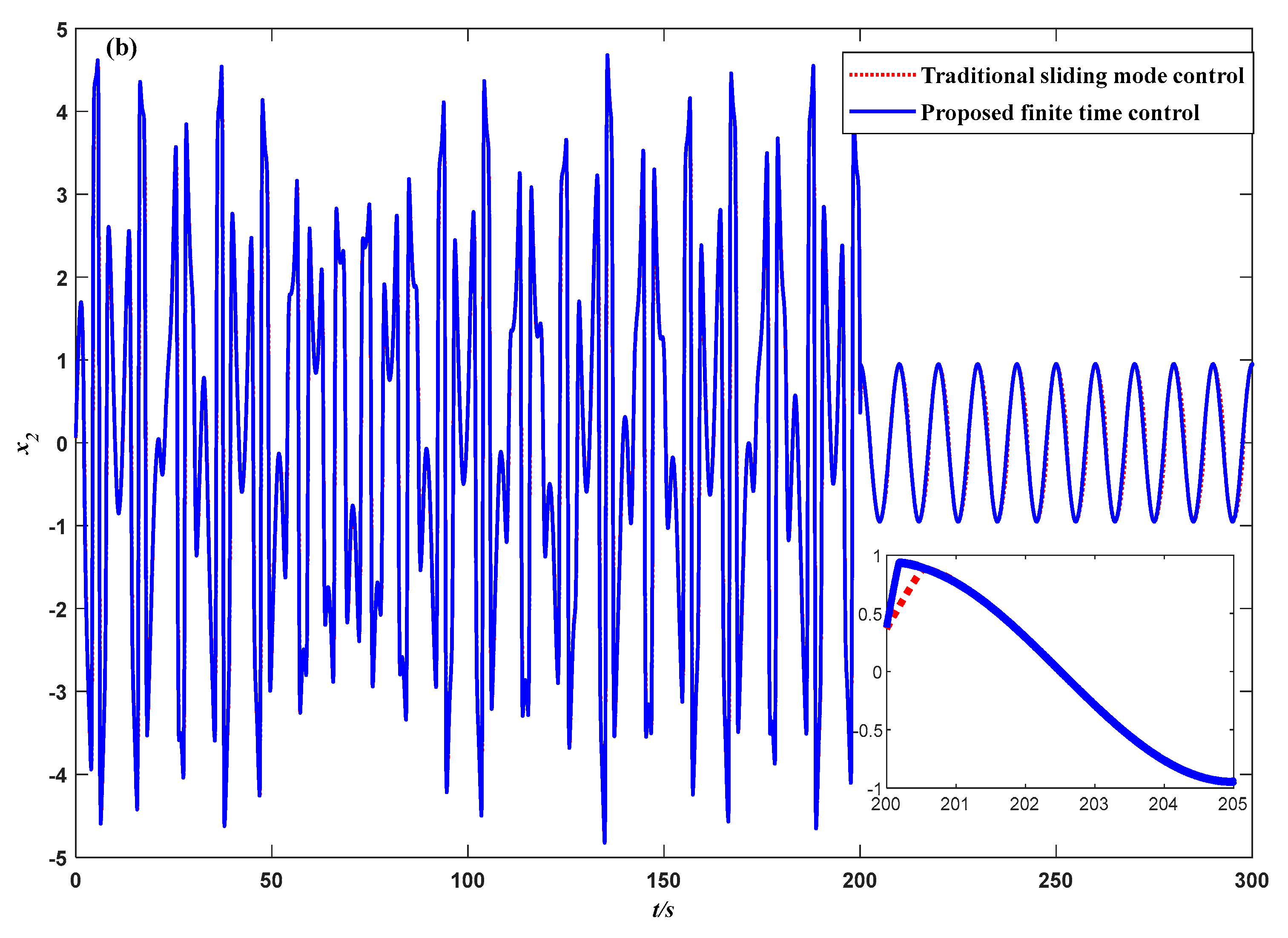
4.3. Simulation and Comparison

In order to validate the correctness of the theoretical results and assess the effectiveness, robustness, and feasibility of the proposed fractional-order control strategy, two illustrative examples are applied to showcase the superiority of the proposed control scheme by comparing with the traditional sliding mode control method. The control objectives are chosen as $x_d=0$, $x_d=0.25+0.2\cos \left(\pi t\right)$, respectively. The detailed control parameter values of the two control methods are provided in

Table 1. The control parameters of system simulation.

Nomenclature	Symbol	Value	Symbol	Value
Sliding mode surface parameters	$r_1$, $r_2$	5, 5	$c_1$	0.5
Tuning parameter	$r_3$, $r_4$	4, 4	$c_2$, $c_3$, $\epsilon$	1.5, 0.6
Upper boundary	${\theta }_1$, ${\theta }_2$	0.1, 0.1	$\alpha$	0.99
Traditional sliding mode method	$r_1$, $r_3$	5, 5	$\left[x_1(0),x_2(0)\right]$	$\left[0.15,0.16\right]$

, while the system parameter values remain consistent with those outlined above. A detailed analysis is given as below.

To make a fair comparison, the uncertainties and external disturbances of the system are chosen as follows:

$$\Delta f\left(x,t\right)+d\left(t\right)=0.1\cos \left(3t\right)x_2-0.1\sin \left(5t\right)$$

Referring to the initial conditions of fractional-order ferroresonance system listed in Table 1, with all other parameters held constant, when the controller is not added, the phase diagram of the system is shown in Figure 11. Evidently, the system is undergoing chaotic oscillation in this state. Therefore, it becomes imperative to implement the proposed control method to effectively suppress the chaotic oscillation.

Case 1:

Stabilize to the equilibrium point $x_d=0$.

This case is employed to verify the superiority of the proposed control method by comparing it with the conventional sliding mode control method. The controller parameters are shown in Table 1. Combined with the sliding mode surface (19) and control law (27), when the control input is applied at $t=200\mathrm{s}$, the control effects of each system state variable are shown in Figure 12a,b. It is clear that the two methods simulate the motion of each system state by using different colors and line styles, respectively. The red dashed line represents the traditional sliding mode control method, while the blue solid line represents the proposed fractional-order finite-time control method.

As shown in Figure 12, before the controller was engaged, each system state variable operated on its own chaotic orbit. When the controller executed at 200 s, the two comparison methods could ensure that the state trajectory would converge to the equilibrium point, which implies that the fractional-order ferroresonance system’s chaotic oscillation behavior could be efficiently suppressed. However, it is clear that the proposed control scheme can stabilize to the equilibrium point in a finite time, the stabilized time is within 1 s, According to (37), the convergence time can be calculated as $t=0.35\mathrm{s}$. And the convergence time of traditional sliding mode control is within 2 s, which demonstrate the effectiveness of the designed controller. By comparing the proposed scheme with the existing method, it can be found that the proposed method not only has the shorter convergence time and the lower overshoot, but also exhibits almost no chattering phenomenon and has effectively overcome the singularity issue, which implies the superiority and effectiveness the proposed fractional-order control method.

Case 2:

Stabilization to periodic orbit $x_d=1.5\sin \left(\pi t/5\right)$.

In order to further validate the robustness and feasibility of the proposed controller, all the control parameters are also presented in Table 1. The uncertain terms and external disturbances are also adopted from above. The control goal is set as tracking a periodic orbit in fractional-order ferroresonance chaotic system. Similarly, the control input is activated at $t=200\mathrm{s}$, in Figure 13a,b, the motion curves of each state variable are simulated by the two control methods. It is clear that both comparison methods can guarantee that the state trajectories converge immediately to periodic orbit. Nonetheless, the convergence time of the proposed control method is significantly shorter. And the stabilized time is within 1 s, which implies that the desired trajectory of the fractional-order ferroresonance system is efficiently tracked in a finite time, and verifies that the superiority of the proposed control scheme. Meanwhile, under system uncertain terms and external disturbances, with the introduction of the proposed robust control scheme, the high amplitude chaotic overvoltage of the system is regulated to the low amplitude harmonic voltage, which greatly reduces harm to the power system and realizes rapid entry of the ferromagnetic resonance system’s chaotic state into a stable condition.

In addition, from the comparison in Figure 13, it becomes evident that the control effectiveness of the proposed finite-time method is basically the same as above $x_d=0$. it is worth noting that the convergence time of the designed controller remains within 0.5 s. In contrast, the control effects of the traditional sliding mode method is obviously worse. For example, the stabilized time of the controller is within 2 s, and the motion curve after adding the controller is not smooth. The main reason for this is the chattering phenomenon caused by the deviation between the actual trajectory and the desired trajectory, which implies the superior performance of the finite-time control method.

From the above analysis, it can be seen that the fractional-order controller based on finite-time theory and the frequency distributed model can effectively stabilize the chaotic behavior, weaken the chattering phenomenon and improve the convergence speed. No matter what state the fractional-order ferroresonance system is in, the proposed controller can make the system quickly reach a steady state. This also implies that the control scheme has good robustness to the different system states.

5. Conclusions and Discussion

This paper mainly studies the dynamic analysis and suppression strategy of the ferroresonant system in detail. The integer-order ferroresonant model is established by considering the effect of external excitation. The mechanisms of ferromagnetic resonance are further revealed. The basic condition for the model to enter the chaotic state is obtained. The influence of the excitation amplitude on the dynamic behavior of the ferroresonance system is analyzed. Fractional calculus is introduced into the modeling of the ferroresonance system, and the effects of the fractional order and the order of flux chain on the motion state of the system are studied through bifurcation diagrams and phase portraits. Compared with the integer-order ferroresonance system, the dynamic behavior is basically similar but the chaotic regions are different. Therefore, the amplitude of external excitation is not the only factor that affects the resonance state.

In order to suppress the chaotic oscillation of the fractional-order ferroresonant system, a novel fractional-order fast terminal sliding mode control based on finite-time theory and the frequency distributed model is proposed, and the simulation comparison is applied to demonstrate the superiority and effectiveness of the proposed method. In summary, the findings of this research work mainly includes the following:

(1)

The increase in the external excitation amplitude will expand the resonance region and unstable region of the ferromagnetic resonance system, and it is easy to enter the chaotic state and destroy the system structure caused by safety accidents.

(2)

Fractional calculus is introduced into the modeling and analysis of the system, which has higher degrees of freedom and more accurate physical characteristics. With the increase in the order of the flux chain, more complex nonlinear dynamic behaviors are obtained, and the factors affecting the ferromagnetic resonance should be more precise.

(3)

As for the suppression strategy of chaotic oscillation, by using the frequency distribution model, the fractional-order model is transformed into an integer-order model. Based on the finite-time method and Lyapunov stability theory, an innovative fractional-order sliding mode controller is designed to realize the stability of the system in finite time, considering the uncertainties of the model and the external disturbances. Compared with the traditional sliding mode method, the effectiveness and superiority of the designed controller are confirmed by the simulation results.

It is worth noting that the proposed control scheme is simple and easy to achieve, and could be implemented to other fractional-order dynamical systems such as power systems, mechanical systems, and neural networks. Future work will consider the effects of system parameter variation, state constraints and control input saturation on system characteristics. Meanwhile, the actual ferromagnetic resonant circuit should be further analyzed, and a controller will be designed for suppression of the ferroresonance overvoltage.