Degradation Characteristics of Coal Samples Under the Dry–Wet Cycle Action of Acidic, High-Salinity Solutions: Experimental Study and Fractal Analysis
Abstract
1. Introduction
2. Methods
2.1. Sample Preparation
2.2. Experimental Method and Procedure
2.3. Equipment
3. Results
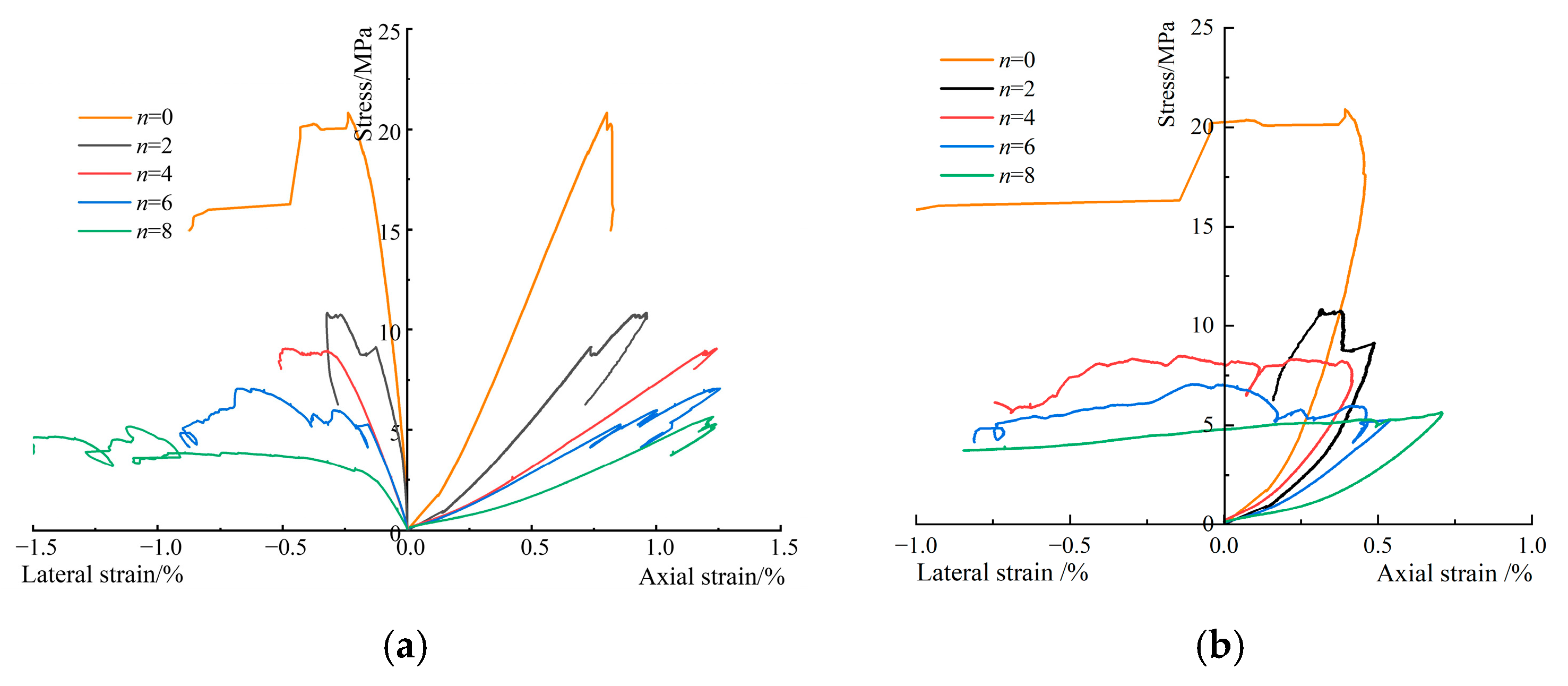
3.1. Stress–Strain Characteristics
- (1)
- Pore Compaction Stage: In the initial loading phase, microcracks and pores within the coal sample are compressed and closed, causing the stiffness to gradually increase. The axial stress–strain curve exhibits an upward curvature with an increasing slope (dσ/dε > 0, d2σ/d2ε > 0). Deformation during this stage is irreversible. The initial damage varies with the number of cycles; with increasing cycle numbers, the damage becomes more severe, and internal initial pores increase, resulting in an extension of the compaction stage.
- (2)
- Linear Elastic Deformation Stage: After most of the initial microcracks and pores are compacted, axial pressure continues to increase, and the specimen enters the linear elastic stage. In this stage, the stress–strain relationship is linear (dσ/dε = 0, d2σ/d2ε = 0). The length and closure degree of internal cracks differ for samples subjected to varying numbers of cycles, leading to variations in the duration of the linear elastic stage.
- (3)
- Stable Crack Propagation Stage: After the linear elastic deformation stage ends, with continued axial pressure increase, localized stress concentration occurs at the tips of internal microcracks. When the intensity of this stress concentration exceeds the cracking strength, the microcracks begin to develop and propagate stably.
- (4)
- Unstable Crack Propagation Stage: As the microcracks propagate and coalesce, plastic deformation increases. The specimen’s load-bearing capacity gradually approaches its limit, and the stress–strain curve begins to curve downward, with a decreasing slope (dσ/dε > 0, d2σ/d2ε < 0). During this stage, the stress–strain curve exhibits a stress drop due to crack propagation. As the specimen nears its peak stress, internal cracks develop rapidly, leading to eventual failure.
- (5)
- Post-Failure Stage: After the peak stress, the specimen retains a certain residual strength, which is provided by the friction between the irregularly shaped fracture surfaces. Since this uniaxial compression test adopts a circumferential displacement-controlled loading method, when the specimen fractures, it undergoes volume expansion, causing a sudden increase in circumferential displacement that exceeds the preset loading rate. To maintain the loading rate, the testing machine releases pressure, resulting in a rebound of axial strain. After stabilization, continued loading leads to a gradual reduction in the specimen’s load-bearing strength due to the formation of internal fracture surfaces.
3.2. Strength and Deformation Parameters
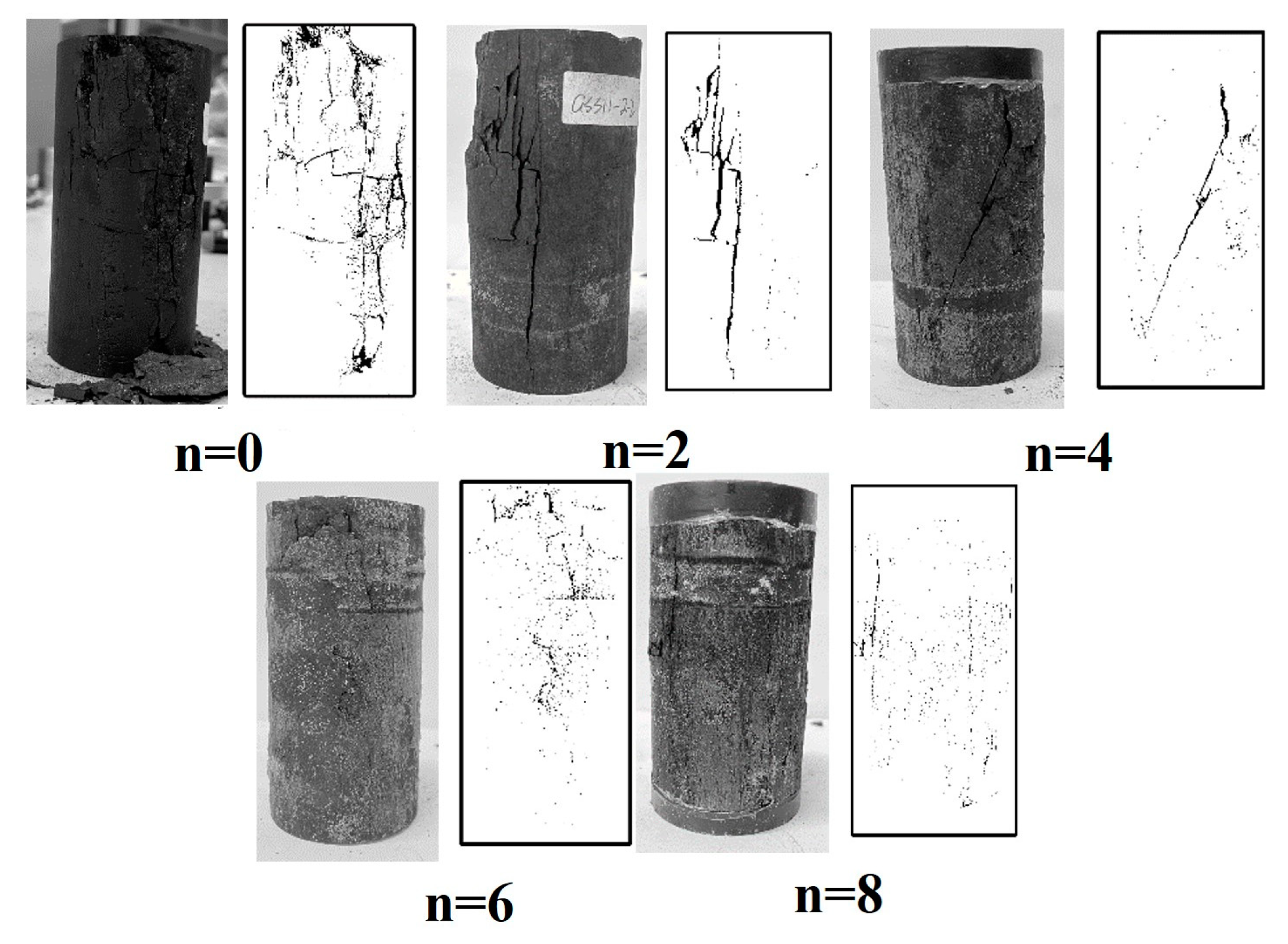
3.3. Destruction Mode
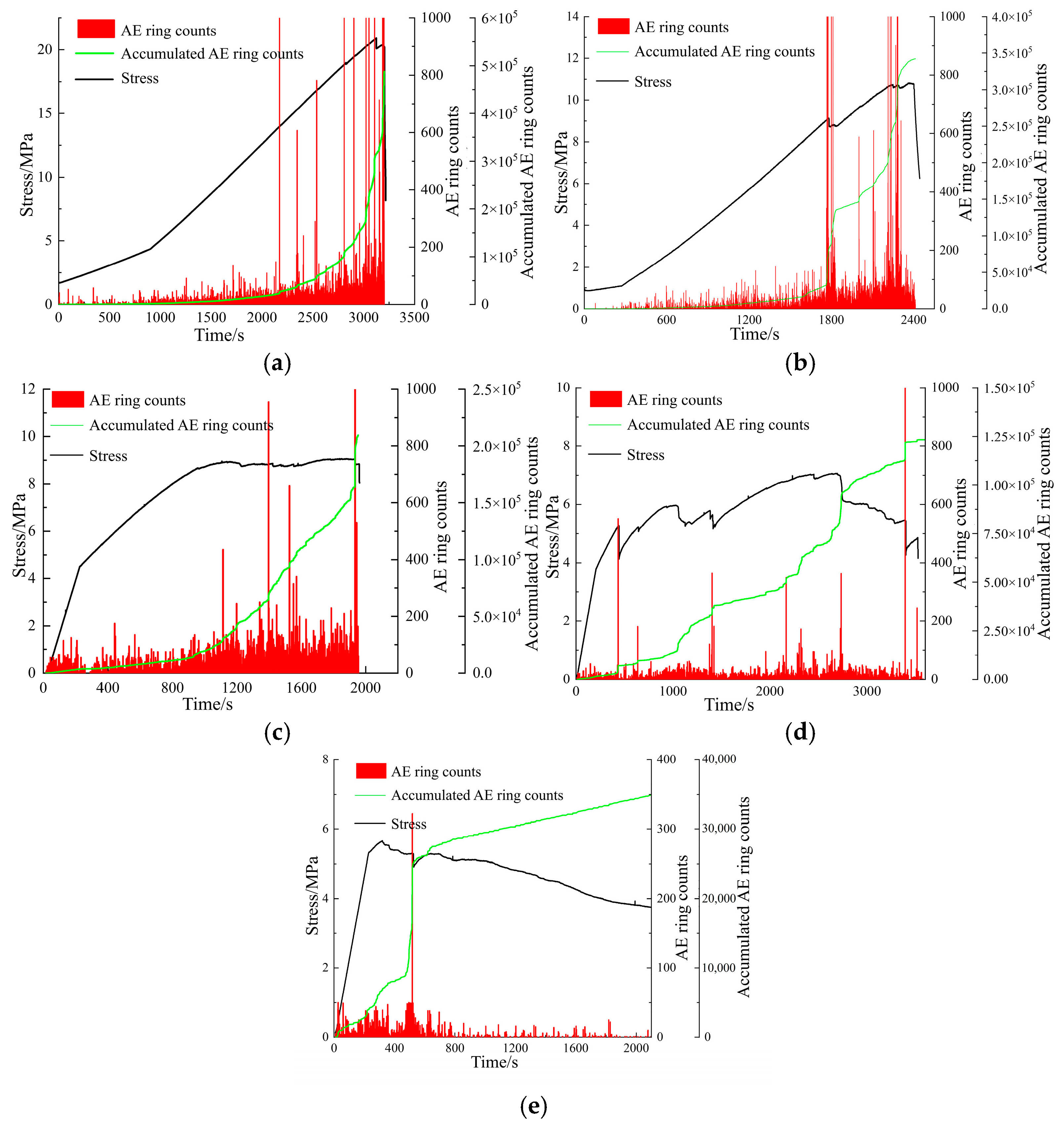
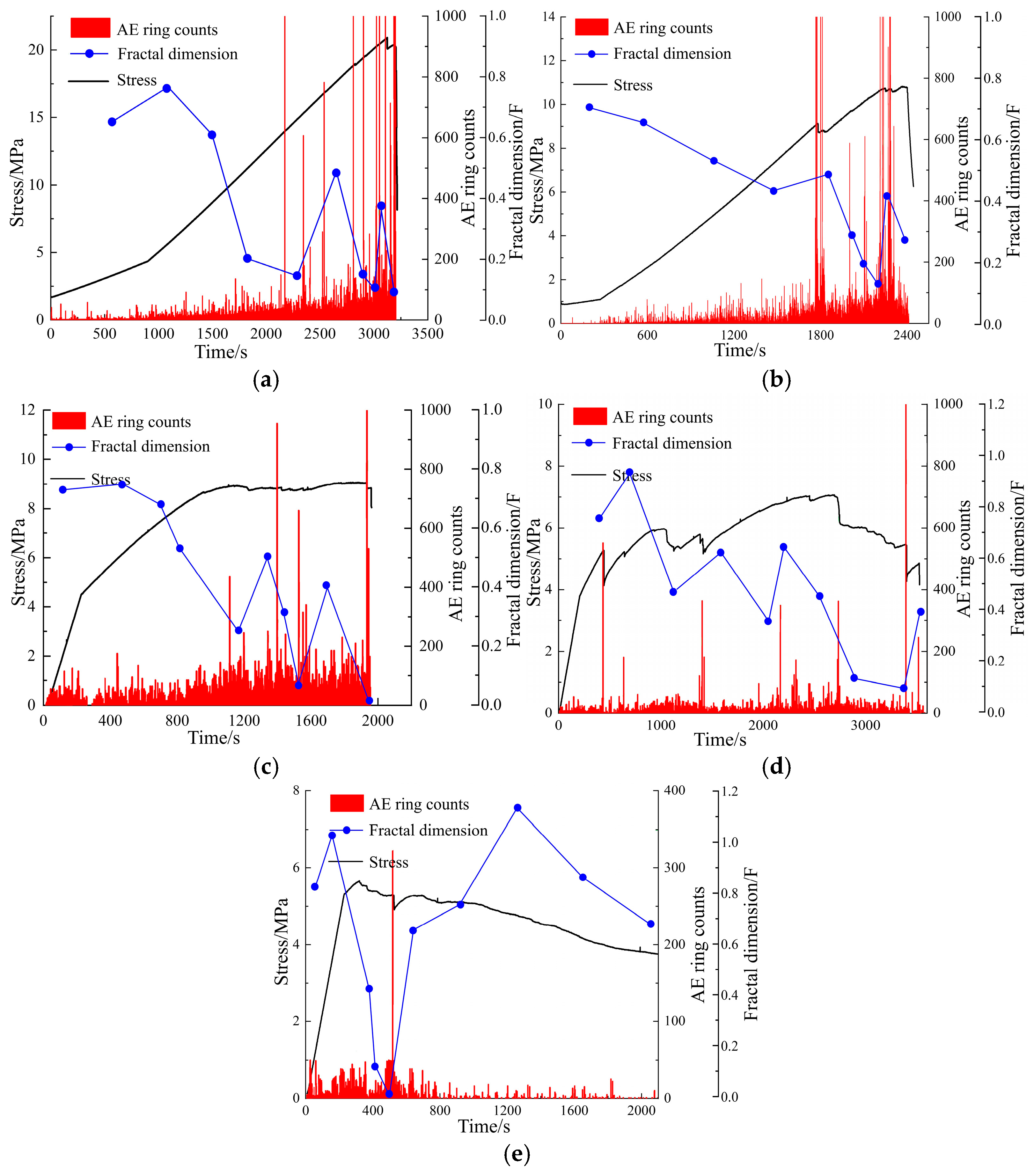
3.4. AE Results
4. Discussion
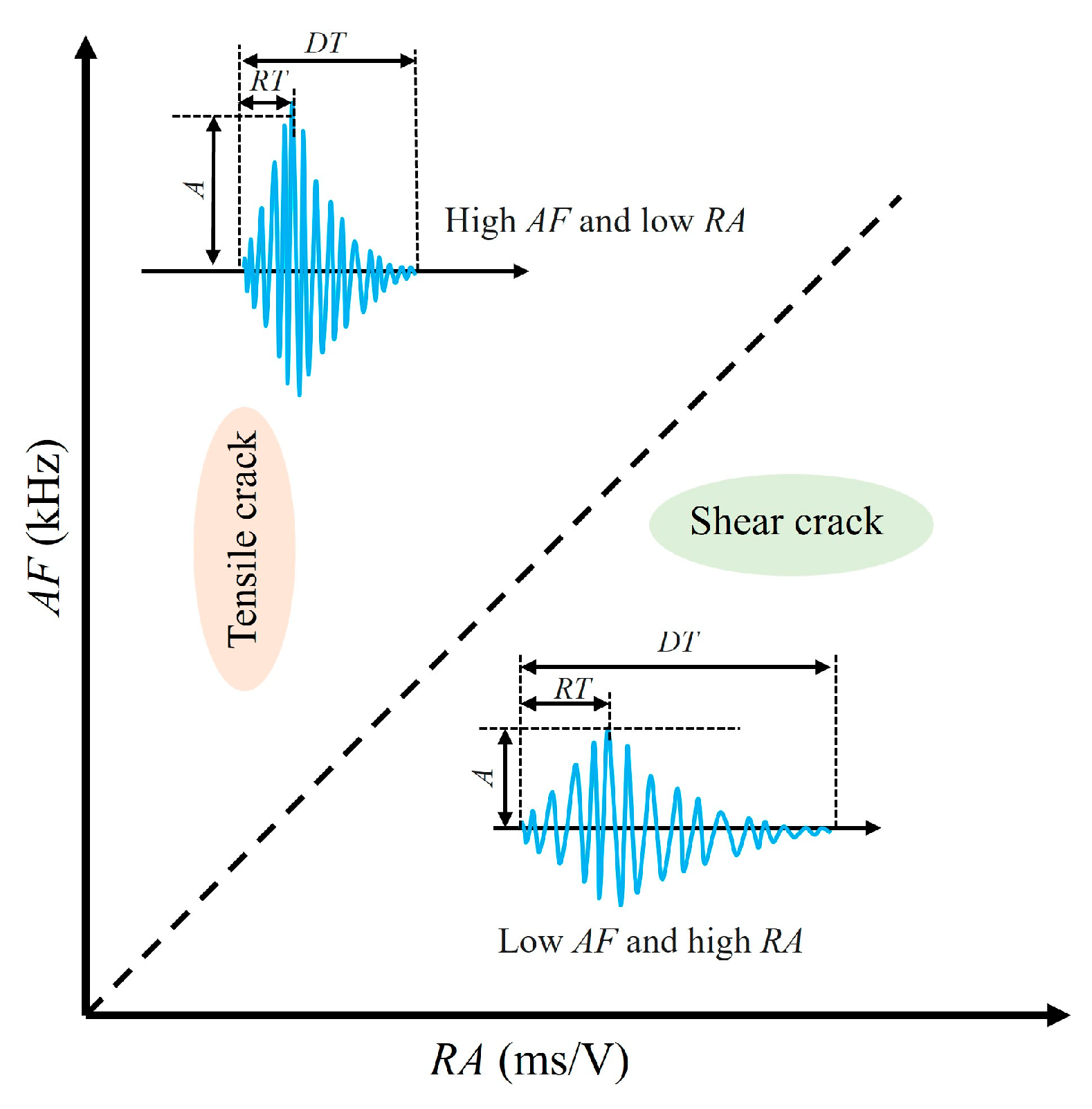
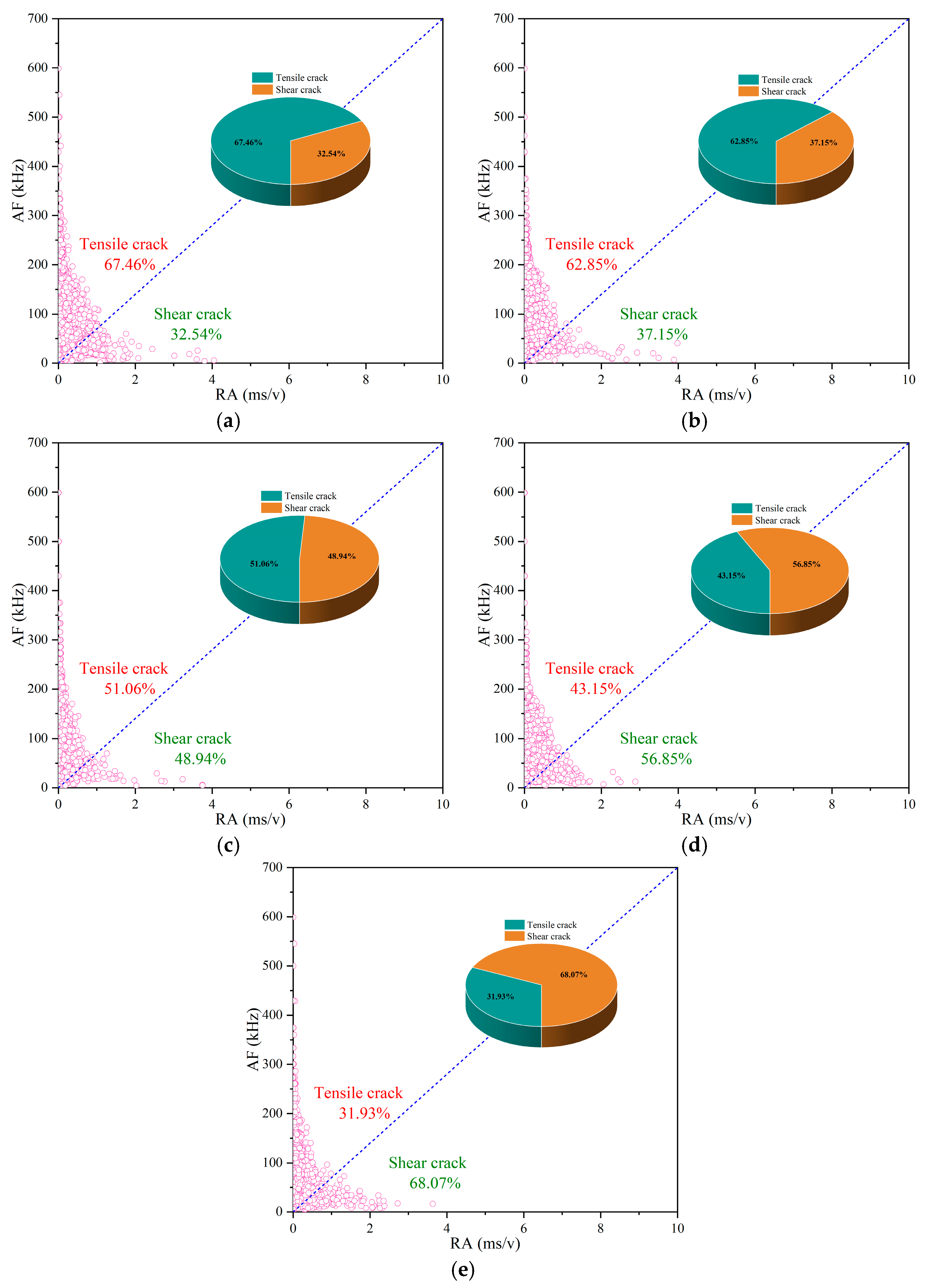
4.1. The RA-AF Distribution Based on AE
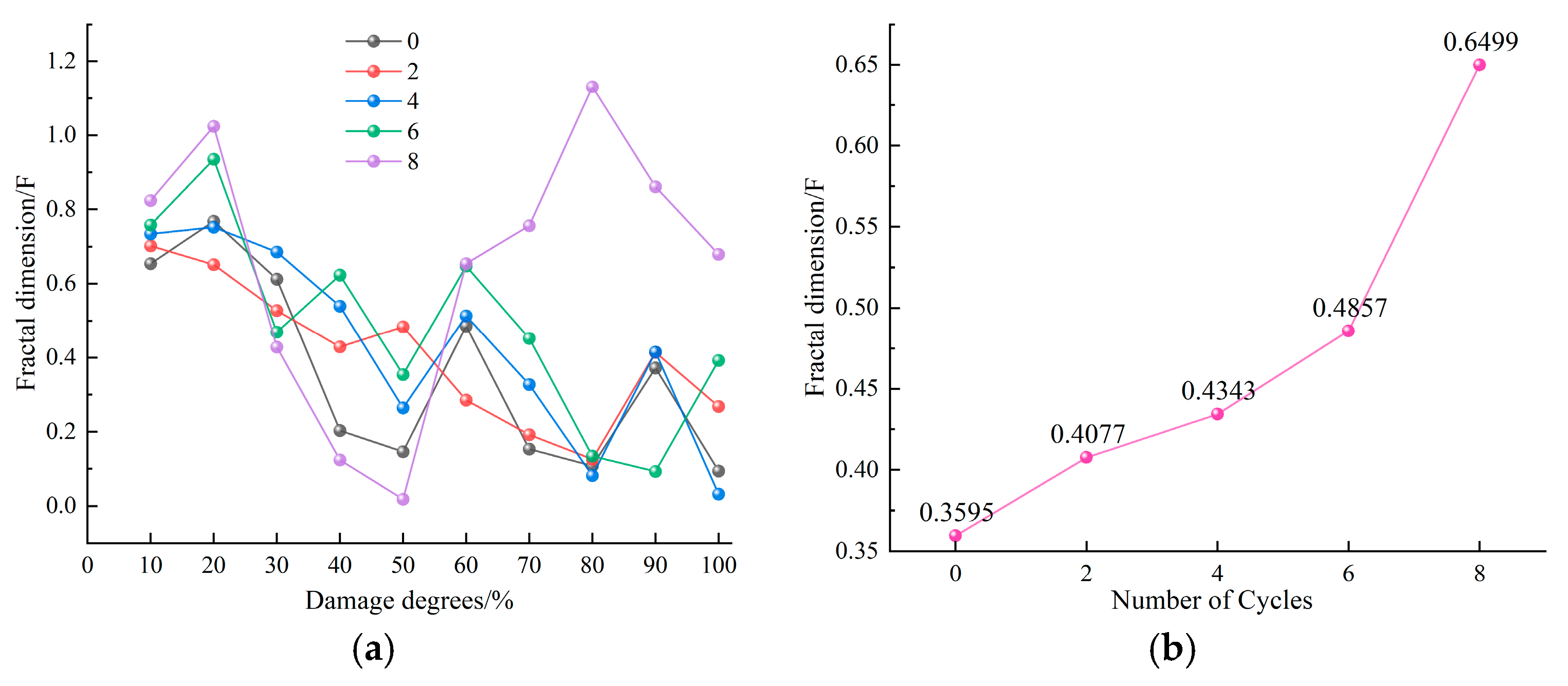
4.2. Fractal Characteristics of AE
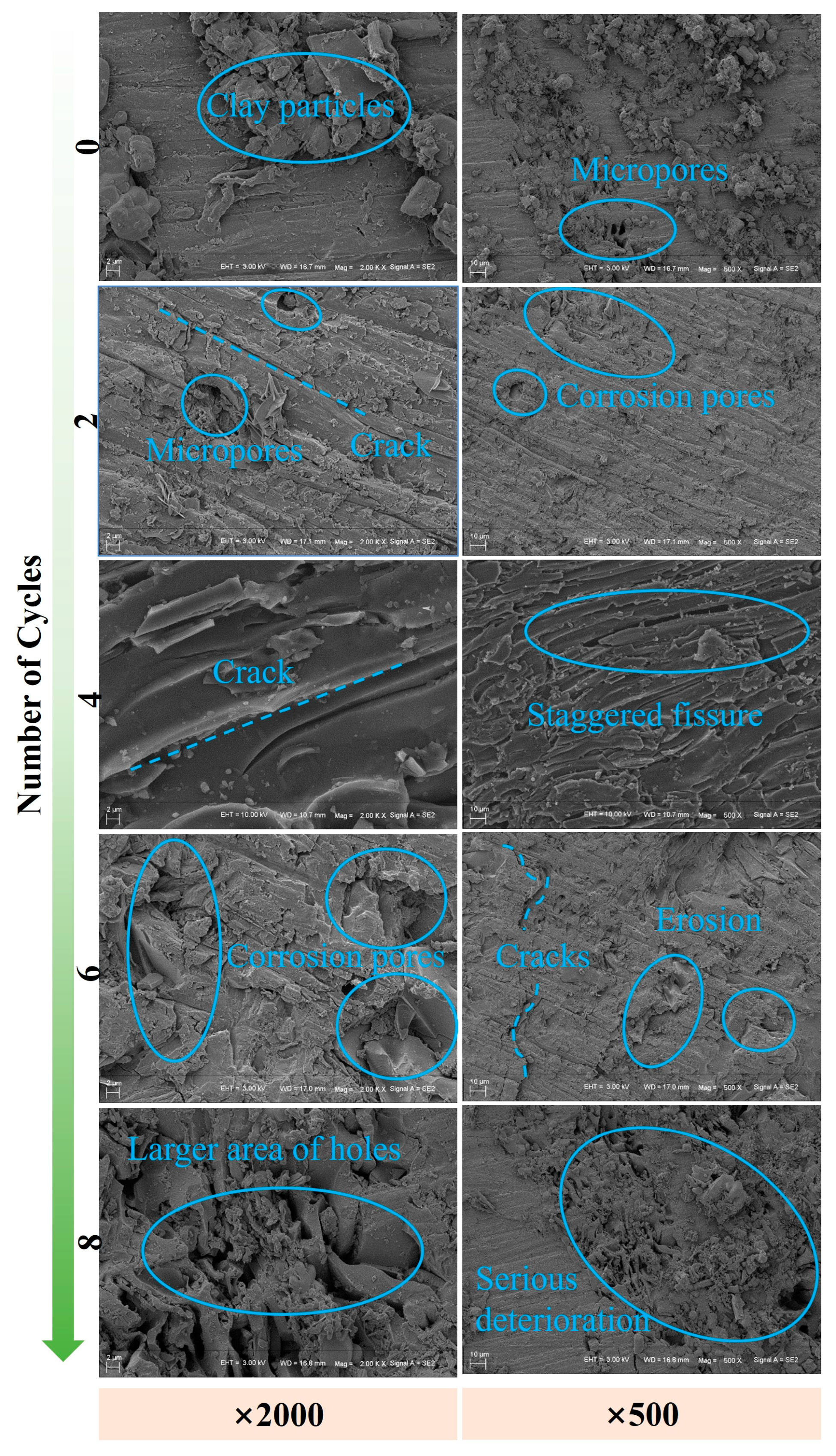
4.3. The Degradation Mechanism Based on the Microscopic Scale
5. Conclusions
- (1)
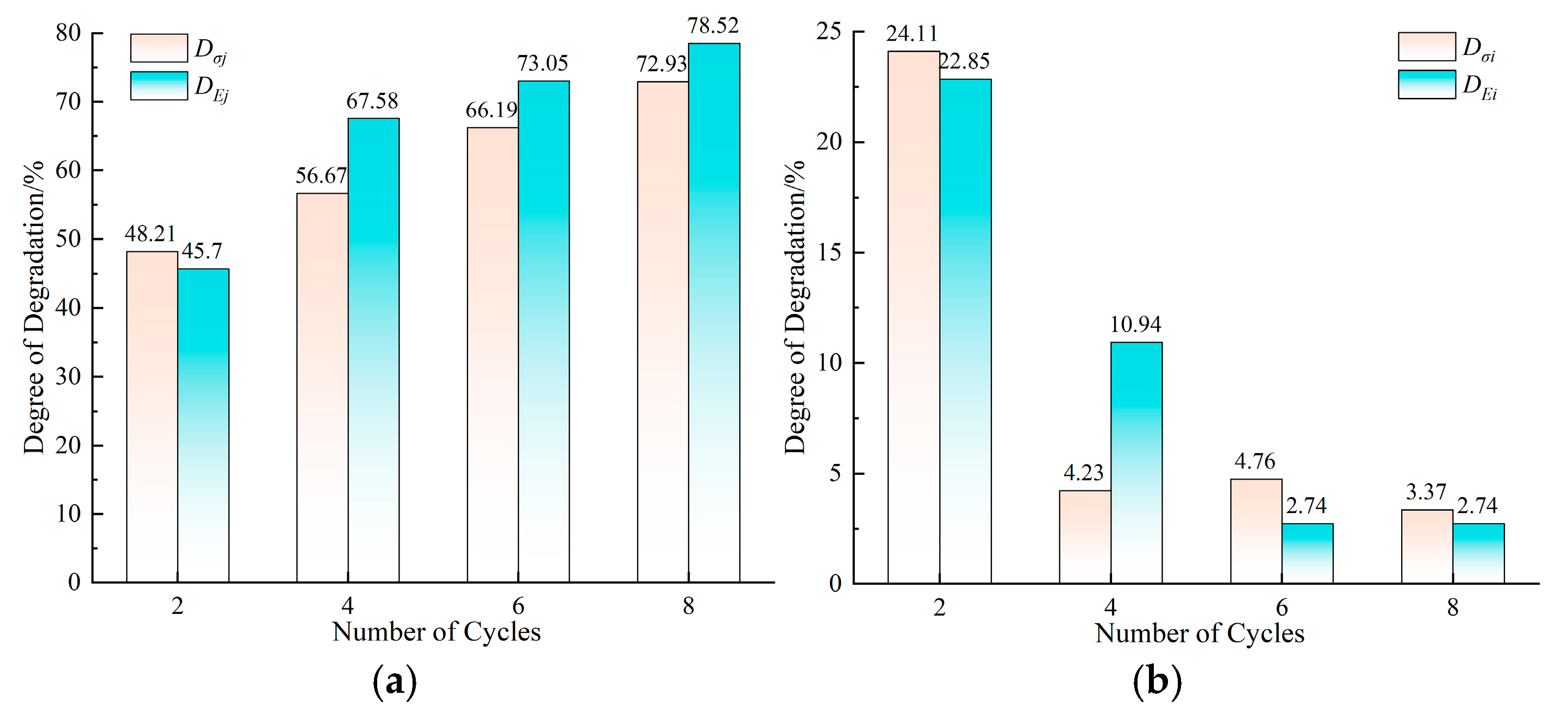
- The effect of dry–wet cycles on the mechanical properties and failure modes of coal samples in acidic, high-salinity solutions is significant. As the number of dry–wet cycles increases, the peak strength and elastic modulus of the coal samples degrade to varying extents, with the degradation of the elastic modulus being more pronounced.
- (2)
- In uniaxial compression tests, coal samples in their natural state exhibit significant brittle failure characteristics. However, as the number of dry–wet cycles increases, the failure mode of the coal samples gradually transitions from tensile failure to shear failure, with cracks extending increasingly in the directions deviating from the maximum principal stress.
- (3)
- The number of dry–wet cycles has a significant effect on the AE signals at different stages of the coal samples. As the number of cycles increases, the initial degree of damage within the coal sample gradually intensifies. The AE ringing count decreases progressively, and the cumulative ringing count also decreases correspondingly. Moreover, with the intensification of solution erosion, the initial internal defects increase, and AE signals become more concentrated in the early loading stages.
- (4)
- With the increase in dry–wet cycles, the coal samples undergo erosion and degradation by the acidic, high-salinity solution, with crack propagation transitioning predominantly to shear failure, characterized by sliding action. Based on the RA-AF classification of acoustic emissions, the proportion of shear cracks increases, and the coal samples shift from tensile failure to shear failure, which is consistent with the macro-failure mode of the coal samples.
- (5)
- During the incremental dry–wet cycles, the mean fractal dimension of the coal samples increases accordingly. The fractal dimension decreases with the increase in AE ringing counts. The continuous reduction in fractal dimension can be regarded as the precursor to the failure process of the coal samples, while the sharp decline in fractal dimension serves as a warning signal for the major failure of the coal samples.
- (6)
- The microscopic structure of coal samples in acidic, high-salinity solutions undergoes a complex damage process under different dry–wet cycles. With an increase in the number of cycles, the microscopic structure evolves progressively, with the development of defects such as pores and cracks becoming more pronounced. The deterioration effect intensifies, and the continuous accumulation of microscopic degradation ultimately leads to macro-scale failure in the coal samples.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wang, S.; Sun, Q.; Geng, J.; Yuan, S.; Gu, C.; Yang, D.; Niu, C.; Lu, T.; Guo, C.; Zhang, H.; et al. Geological Assurance Technology Framework for Damage and Damage Reduction Mining in Western Mining Areas. Coal Geol. Explor. 2024, 52, 1–13. [Google Scholar]
- Huang, B.; Xie, P.; Wu, Y.; Lin, W.; Luo, S.; Wang, S.; Wen, Z.; Chen, J. The Effect of Overlying Rock Fracture and Stress Path Evolution in Steeply Dipping and Large Mining Height Stope. Géoméch. Geophys. Geo-Energy Geo-Resour. 2024, 10, 95. [Google Scholar] [CrossRef]
- Yao, Q.; Yu, L.; Chen, S.; Li, Y.; Li, X. Mining-affected Water Resources and Ecological Effects in Ecologically Fragile Mining Areas of Western. China J. China Coal Soc. 2025, 1–22. [Google Scholar] [CrossRef]
- Shan, P.; Meng, Z.; Xu, H.; Li, C.; Zhang, L.; Xi, B. Research on Accurate Recognition and Refuse Rate Calculation of Coal and Gangue Based on Thermal Imaging of Transporting Situation. Measurement 2024, 244, 116574. [Google Scholar] [CrossRef]
- Gu, D.; Cao, Z.; Li, J.; Wu, B.; Zhang, Y.; Jiang, B.; Guo, Q.; Wang, H.; Wu, Y.; Shi, X.; et al. Development and Application of the Original Test Platform System for Coal Mine Underground Water Reservoir Technology. J. China Coal Soc. 2024, 49, 100–113. [Google Scholar]
- Gu, D.; Li, J.; Cao, Z.; Wu, B.; Jiang, B.; Yang, Y.; Yang, J.; Chen, Y. Development Strategy and Engineering Technology for Protection and Utilization of Mine Water in China. Coal Sci. Technol. 2021, 46, 3079–3089. [Google Scholar]
- Wang, M.; Xi, X.; Guo, Q.F.; Pan, J.L.; Cai, M.F.; Yang, S.T. Sulfate Diffusion in Coal Pillar: Experimental Data and Prediction Model. Int. J. Coal Sci. Technol. 2023, 10, 17. [Google Scholar] [CrossRef]
- Li, N.; Zhang, X.; Wang, D.; Zhao, X. Experimental Study on the Changes of Longitudinal Wave Velocity and Waveform Char-acteristics during the Water Absorption Process of Coal Samples. Rock Mech. Eng. 2017, 36, 1921–1929. [Google Scholar]
- Perera, M.S.A.; Ranjith, P.G.; Peter, M. Effects of Saturation Medium and Pressure on Strength Parameters of Valley Brown Coal: Carbon Dioxide, Water, and Nitrogen Saturations. Energy 2011, 36, 6941–6947. [Google Scholar]
- Poulsen, B.A.; Shen, B.; Williams, D.J.; Huddlestone-Holmes, C.; Erarslan, N.; Qin, J. Strength Reduction on Saturation of Coal and Coal Measures Rocks with Implications for Coal Pillar Strength. Int. J. Rock Mech. Min. Sci. Géoméch. Abstr. 2014, 71, 41–52. [Google Scholar] [CrossRef]
- Vishal, V.; Ranjith, P.; Singh, T. An Experimental Investigation on the Behaviour of Coal Under Fluid Saturation, Using Acoustic Emission. J. Nat. Gas. Sci. Eng. 2015, 22, 428–436. [Google Scholar] [CrossRef]
- Chen, T.; Yao, Q.-L.; Wei, F.; Chong, Z.-H.; Zhou, J.; Wang, C.-B.; Li, J. Effects of Water Intrusion and Loading Rate on Mechanical Properties of and Crack Propagation in Coal–Rock Combinations. J. Cent. South. Univ. 2017, 24, 423–431. [Google Scholar] [CrossRef]
- Yang, K.; Wei, Z.; Dou, L.T.; Chi, X.L.; Liu, W.J.; Zhang, J.Q. Experimental Study on Dynamic Tensile Energy Evolution and Failure Characteristics of Water-bearing Coal Samples. Coal Sci. Technol. 2021, 46, 398–411. [Google Scholar]
- Liu, S.; Yang, G.S.; Dong, X.H. Experimental Study on the Effect of Dry-Wet Cycles on the Mechanical Properties and Damage of Red Sandstone. Coal Sci. Technol. 2019, 47, 101–106. [Google Scholar]
- Abdilor, Y.; Khanlari, M. Influence of Wet-Dry, Freeze-Thaw, and Heat-Cool Cycles on the Physical and Mechanical Properties of Upper Red Sandstones in Central Iran. Bull. Eng. Geol. Environ. 2015, 74, 1287–1300. [Google Scholar]
- Özbek, A. Investigation of the Effects of Wetting–Drying and Freezing–Thawing Cycles on Some Physical and Mechanical Properties of Selected Ignimbrites. Bull. Eng. Geol. Environ. 2013, 73, 595–609. [Google Scholar] [CrossRef]
- Huang, S.; Wang, J.; Qiu, Z.; Kang, K. Effects of Cyclic Wetting-Drying Conditions on Elastic Modulus and Compressive Strength of Sandstone and Mudstone. Processes 2018, 6, 234. [Google Scholar] [CrossRef]
- Ma, D.H.; Yao, H.Y.; Cui, Q.; Cheng, S.L. Analysis of the Effect of Water Chemical Solution on the Mechanical Properties of Limestone. Chin. Sci. Pap. 2019, 14, 846–851. [Google Scholar]
- Wang, Y.J.; Rong, H.R.; Wang, D.L.; Guo, Q.; Mu, B.L.; Zhao, Y.; Zhan, L. Experimental Study on the Mechanical Properties of Yellow Sandstone under Al-kaline Solution Corrosion. J. Hebei Univ. Eng. 2017, 35, 15–20. [Google Scholar]
- Han, T.L.; Shi, J.P.; Chen, Y.S. Study on the Correlation of Fracture Toughness and Strength Parameters of Sandstone under Chemical Corrosion and Dry-Wet Cycles. J. Hydraul. Eng. 2018, 49, 1265–1275. [Google Scholar]
- Chen, W.L.; Xie, J.; Sun, Y. Effect of Acid Rain Corrosion on Uniaxial Compressive Properties of Marble. J. Cent. South Univ. Sci. Technol. 2013, 44, 2897–2902. [Google Scholar]
- Miao, S.J.; Cai, M.F.; Ji, D. Effects and Mechanisms of Granite Damage and Aging under Acidic Chemical Solution. Coal Sci. Technol. 2016, 41, 1137–1144. [Google Scholar]
- Miao, S.J.; Cai, M.F.; Ji, D. Damage Effects of Acidic Chemical Solution on the Mechanical Properties and Parameters of Granite. Coal Sci. Technol. 2016, 41, 829–835. [Google Scholar]
- Huo, R.K.; Wang, L.F.; Li, S.G.; Ding, F.; Wang, G.J.; Qiu, T. Damage Characteristics and Analysis Model of Sandstone under Acid Corrosion. J. Civ. Civil. Environ. Eng. 2022, 44, 1–9. [Google Scholar]
- Gong, C.-G.; Wang, W.; Shao, J.-F.; Wang, R.-B.; Feng, X.-W. Effect of Water Chemical Corrosion on Mechanical Properties and Failure Modes of Pre-Fissured Sandstone Under Uniaxial Compression. Acta Geotech. 2020, 16, 1083–1099. [Google Scholar] [CrossRef]
- Dai, J.; Liu, J.; Liu, C.; Yang, J.; Xue, F.; Tang, Y.; Liu, J.; Liu, D.; Dai, S. Regional Characteristics of Seismicity Associated with Hydraulic Fracturing in the Southern Sichuan Basin of China. Rock. Mech. Rock. Eng. 2024, 57, 6971–6996. [Google Scholar] [CrossRef]
- Dai, J.; Liu, J.; Yang, J.; Xue, F.; Wang, L.; Shi, X.; Dai, S.; Hu, J.; Liu, C. Seismicity Associated with Hydraulic Fracturing in Changning Shale Gas Field, China: Constraints from Source Mechanisms, Stress Field and Fluid Overpressure Thresholds. J. Rock. Mech. Geotech. Eng. 2024, in press. [Google Scholar] [CrossRef]
- Zhang, L.M.; Lai, X.P.; Pan, J.L.; Shan, P.F.; Zhang, Y.; Zhang, Y.; Xu, H.C.; Cai, M.F.; Xi, X. Experimental Investigation on the Mixture Optimization and Failure Mechanism of Cemented Backfill with Coal Gangue and Fly Ash. Powder Technol. 2024, 440, 119751. [Google Scholar] [CrossRef]
- Wen, S.Y.; Han, L.J.; Zong, Y.J.; Meng, Q.B.; Zhang, J. Study on Acoustic Emission Characteristics of Red Sandstone under Uniaxial Compression with Different Water Contents. Coal Sci. Technol. 2013, 41, 46–48. [Google Scholar]
- Ran, Q.C.; Liang, Y.P.; Yang, Z.L.; Zou, Q.L.; Ye, C.F.; Tian, C.L.; Wu, Z.P.; Zhang, B.C.; Wang, W.Z. Deterioration Mechanisms of Coal Mechanical Properties Under Uniaxial Multi-Level Cyclic Loading Considering Initial Damage Effects. Int. J. Rock Mech. Min. Sci. Géoméch. Abstr. 2024, 186, 106006. [Google Scholar] [CrossRef]
- Gao, B.; Li, H.; Li, L.; Su, C. Acoustic emission and fractal characteristics of saturated coal samples in the failure process. J. Min. Saf. Eng. 2015, 32, 665–676. [Google Scholar]
- Tang, L.; Tu, S.; Tu, H.; Miao, K.; Li, W.; Zhao, H.; Ma, J.; Zhang, L. Compressive Failure Characteristics of a Coal–Rock Combination at Different Angles: Experimental Study and Fractal Analysis. Fractal Fract. 2024, 8, 240. [Google Scholar] [CrossRef]
- Guo, Z.Q. High Mineralization Mine Water Treatment Technology and Application. Min. Saf. Environ. 2012, 39, 72–74. [Google Scholar]
- Han, T.; Chen, Y.; Shi, J. Experimental Study of Mechanical Characteristics of Sandstone Subjected to Hydrochemical Erosion. Chin. J. Rock Mech. Eng. 2013, 32 (Suppl. 2), 3064–3072. [Google Scholar]
- Li, G.; Wei, L.; Su, H.; Jing, H.; Zhang, T. Experimental Study on the SHPB Impact Dynamic Properties of Limestone Subjected to Chemical Erosion. J. Rock Mech. Eng. 2018, 37, 2075–2083. [Google Scholar]
- Han, T.; Shi, J.; Chen, Y.; Cao, X. Mechanism damage to mode-i fractured sandstone from chemical solutions and its correlation with strength characteristics. Pure Appl. Geophys. 2019, 176, 5027–5049. [Google Scholar] [CrossRef]
- Bieniawski, Z.T. Mechanism of Brittle Fracture of Rock: Parts I, II, and III. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1967, 4, 395–406. [Google Scholar]
- Zhang, Y.; He, K.; Yang, J.; Pan, J.; Xi, X.; Feng, X.; Zhang, L. Mechanical, Seepage, and Energy Evolution Properties of Multi-Shaped Fractured Sandstone Under Hydro-Mechanical Coupling: An Experimental Study. Minerals 2025, 15, 215. [Google Scholar] [CrossRef]
- Wen, Z.; Du, Z.; Li, S. Research on the Time-dependent Stability of Filling Paste Under the Action of Different Concentrations of Chloride. Salts Coal Sci. Technol. 2025, 1–13. Available online: http://kns.cnki.net/kcms/detail/11.2402.TD.20240509.1104.001.html (accessed on 25 March 2025).
- Pan, J.; Zhang, Y.; Li, P.; Wu, X.; Xi, X. Mechanical Properties and Thermo-Chemical Damage Constitutive Model of Granite Subjected to Thermal and Chemical Treatments Under Uniaxial Compression. Constr. Build. Mater. 2023, 390, 131755. [Google Scholar] [CrossRef]
- Liu, X.; Liu, Z.; Li, X.; Gong, F.; Du, K. Experimental Study on the Effect of Strain Rate on Rock Acoustic Emission Character-istics. Int. J. Rock Mech. Min. Sci. 2020, 133, 104420. [Google Scholar]
- Ohno, K.; Ohtsu, M. Crack Classification in Concrete Based on Acoustic Emission. Constr. Build. Mater. 2010, 24, 2339–2346. [Google Scholar] [CrossRef]
- Li, Y.X.; Xie, H.P.; Liu, J.F. Study on the Fractal Dimension Calculation Method of RGB Images. Rock Mech. Eng. 2008, S1, 2779–2784. [Google Scholar]
- Wu, X.; Liu, X.; Liang, Z.; You, X.; Yu, M. Experimental Study of Fractal Dimension of AE Series of Different Rocks under Uniaxial Compression. Rock Soil. Mech. 2012, 33, 3561–3569. [Google Scholar]
- Liang, Z.Y.; Gao, F.; Lin, J.T.; Feng, S.Z.; Zheng, Z.G. Fractal Characteristics of Acoustic Emission Parameters of Rock under Uniaxial Compres-sion. Mech. Pract. 2009, 32, 43–46. [Google Scholar]
- Wang, G.F. Study on the Kaiser Point Signal Characteristics of Rock Acoustic Emission. Master’s Thesis, Jiangxi University of Science and Technology, Ganzhou, China, 2007. [Google Scholar]
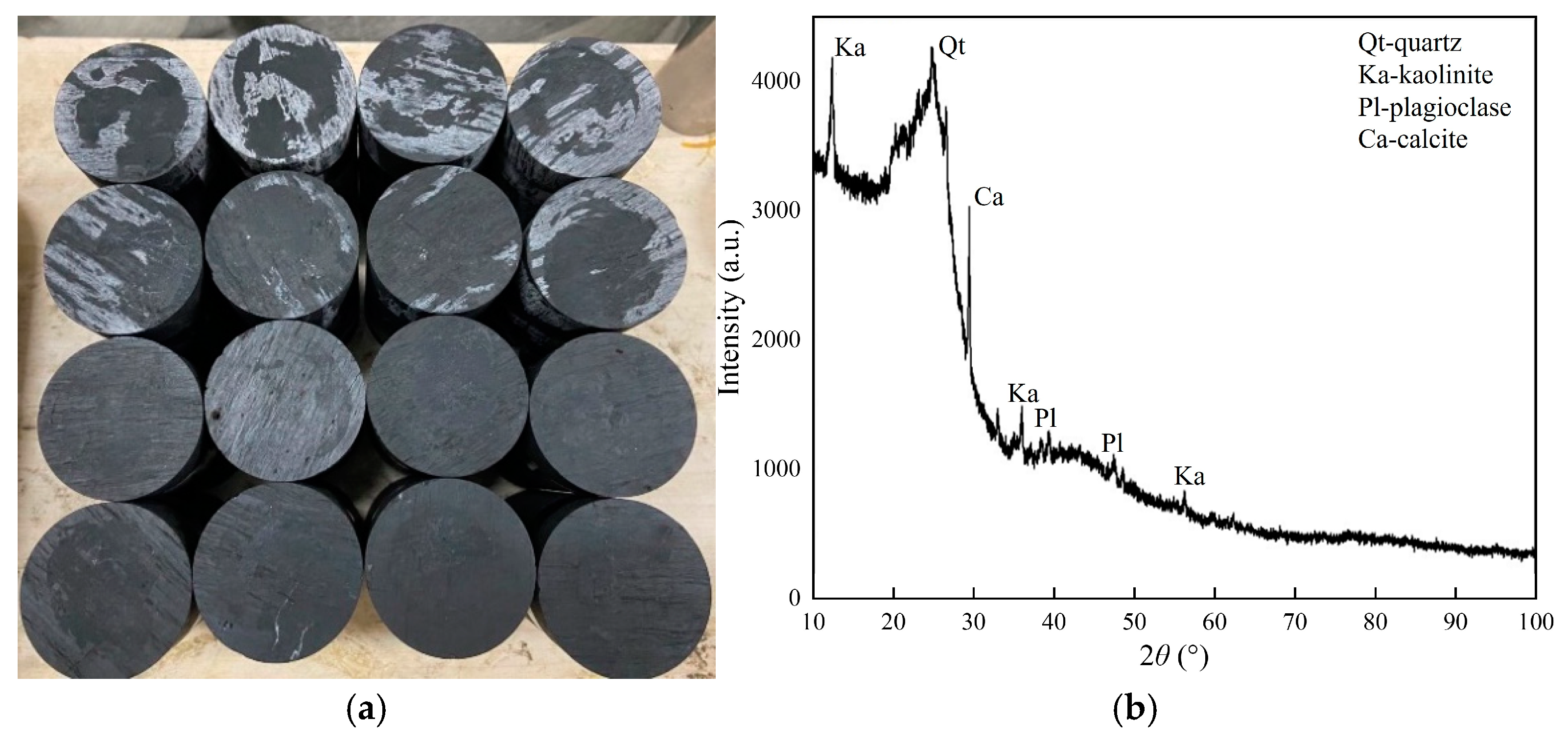



| TDS | Ca2+ | Na+ | K+ | SO42− | Cl− |
|---|---|---|---|---|---|
| /mg·L−1 | /mg·L−1 | /mg·L−1 | /mg·L−1 | /mg·L−1 | /mg·L−1 |
| 4720 | 86 | 1350 | 10.7 | 1145 | 1230 |
| Number of Cycles | Peak Strength/MPa | Elastic Modulus/GPa | Dσj /% | Dσi /% | DEj /% | DEi /% |
|---|---|---|---|---|---|---|
| 0 | 20.91 | 2.56 | - | - | - | - |
| 2 | 10.83 | 1.39 | 48.21 | 24.11 | 45.70 | 22.85 |
| 4 | 9.06 | 0.83 | 56.67 | 4.23 | 67.58 | 10.94 |
| 6 | 7.07 | 0.69 | 66.19 | 4.76 | 73.05 | 2.74 |
| 8 | 5.66 | 0.55 | 72.93 | 3.37 | 78.52 | 2.74 |
| Number of Cycles | Fractal Dimension of Different Damage Degrees | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 10% | 20% | 30% | 40% | 50% | 60% | 70% | 80% | 90% | 100% | |
| 0 | 0.654 | 0.768 | 0.612 | 0.203 | 0.146 | 0.485 | 0.153 | 0.068 | 0.372 | 0.297 |
| 2 | 0.702 | 0.686 | 0.593 | 0.482 | 0.514 | 0.327 | 0.238 | 0.126 | 0.424 | 0.486 |
| 4 | 0.734 | 0.752 | 0.685 | 0.539 | 0.264 | 0.513 | 0.327 | 0.082 | 0.415 | 0.305 |
| 6 | 0.758 | 0.935 | 0.469 | 0.623 | 0.354 | 0.647 | 0.452 | 0.134 | 0.093 | 0.392 |
| 8 | 0.824 | 1.024 | 0.428 | 0.124 | 0.018 | 0.654 | 0.756 | 1.131 | 0.861 | 0.679 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, L.; Wang, M.; Zhang, B.; Xi, X.; Zhang, Y.; Pan, J. Degradation Characteristics of Coal Samples Under the Dry–Wet Cycle Action of Acidic, High-Salinity Solutions: Experimental Study and Fractal Analysis. Fractal Fract. 2025, 9, 221. https://doi.org/10.3390/fractalfract9040221
Zhang L, Wang M, Zhang B, Xi X, Zhang Y, Pan J. Degradation Characteristics of Coal Samples Under the Dry–Wet Cycle Action of Acidic, High-Salinity Solutions: Experimental Study and Fractal Analysis. Fractal and Fractional. 2025; 9(4):221. https://doi.org/10.3390/fractalfract9040221
Chicago/Turabian StyleZhang, Leiming, Min Wang, Bin Zhang, Xun Xi, Ying Zhang, and Jiliang Pan. 2025. "Degradation Characteristics of Coal Samples Under the Dry–Wet Cycle Action of Acidic, High-Salinity Solutions: Experimental Study and Fractal Analysis" Fractal and Fractional 9, no. 4: 221. https://doi.org/10.3390/fractalfract9040221
APA StyleZhang, L., Wang, M., Zhang, B., Xi, X., Zhang, Y., & Pan, J. (2025). Degradation Characteristics of Coal Samples Under the Dry–Wet Cycle Action of Acidic, High-Salinity Solutions: Experimental Study and Fractal Analysis. Fractal and Fractional, 9(4), 221. https://doi.org/10.3390/fractalfract9040221








