A Systematic Survey of FDM Process Parameter Optimization and Their Influence on Part Characteristics
Abstract
:1. Introduction
2. Fused Deposition Modeling
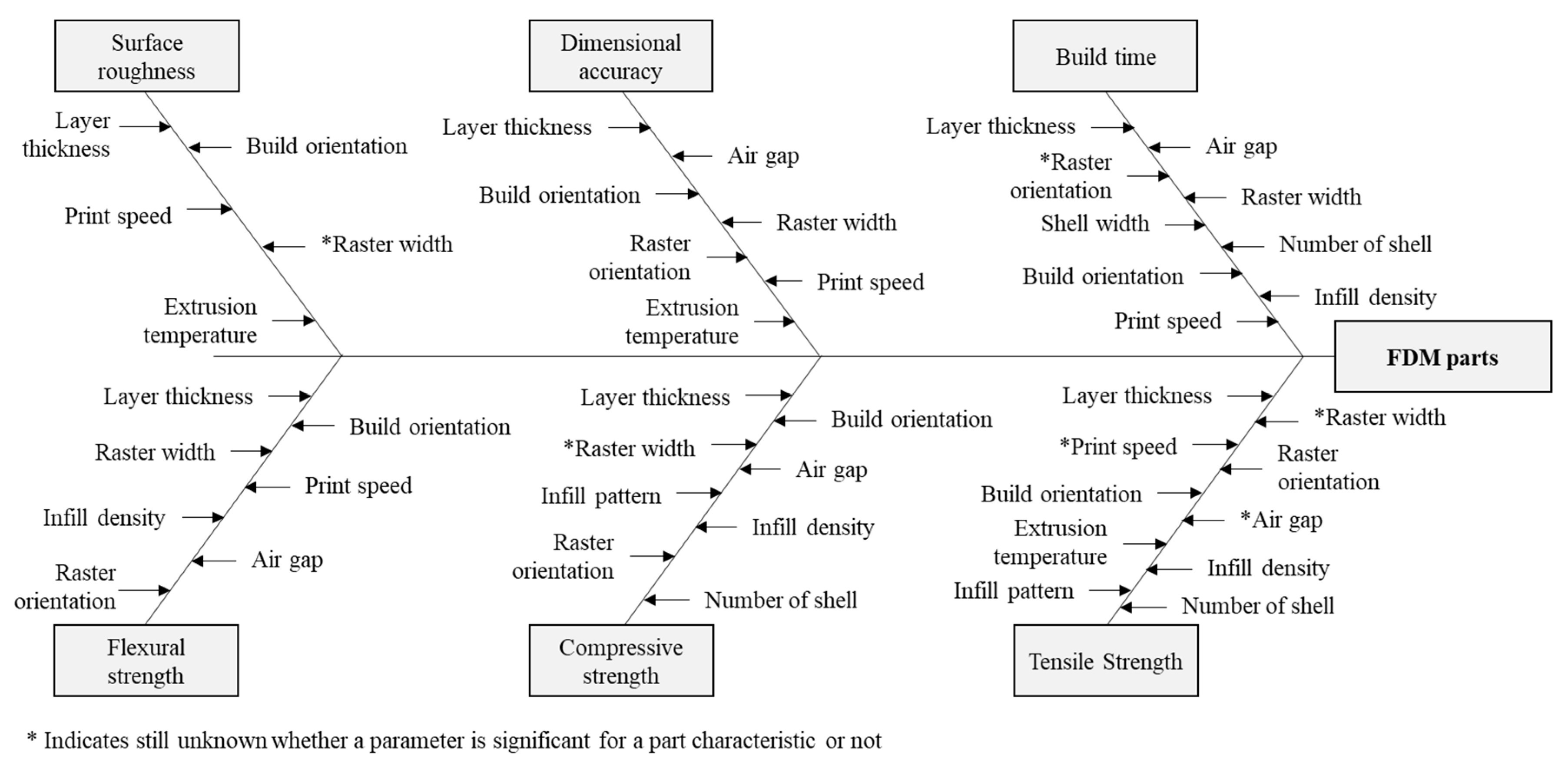
2.1. Process Parameters
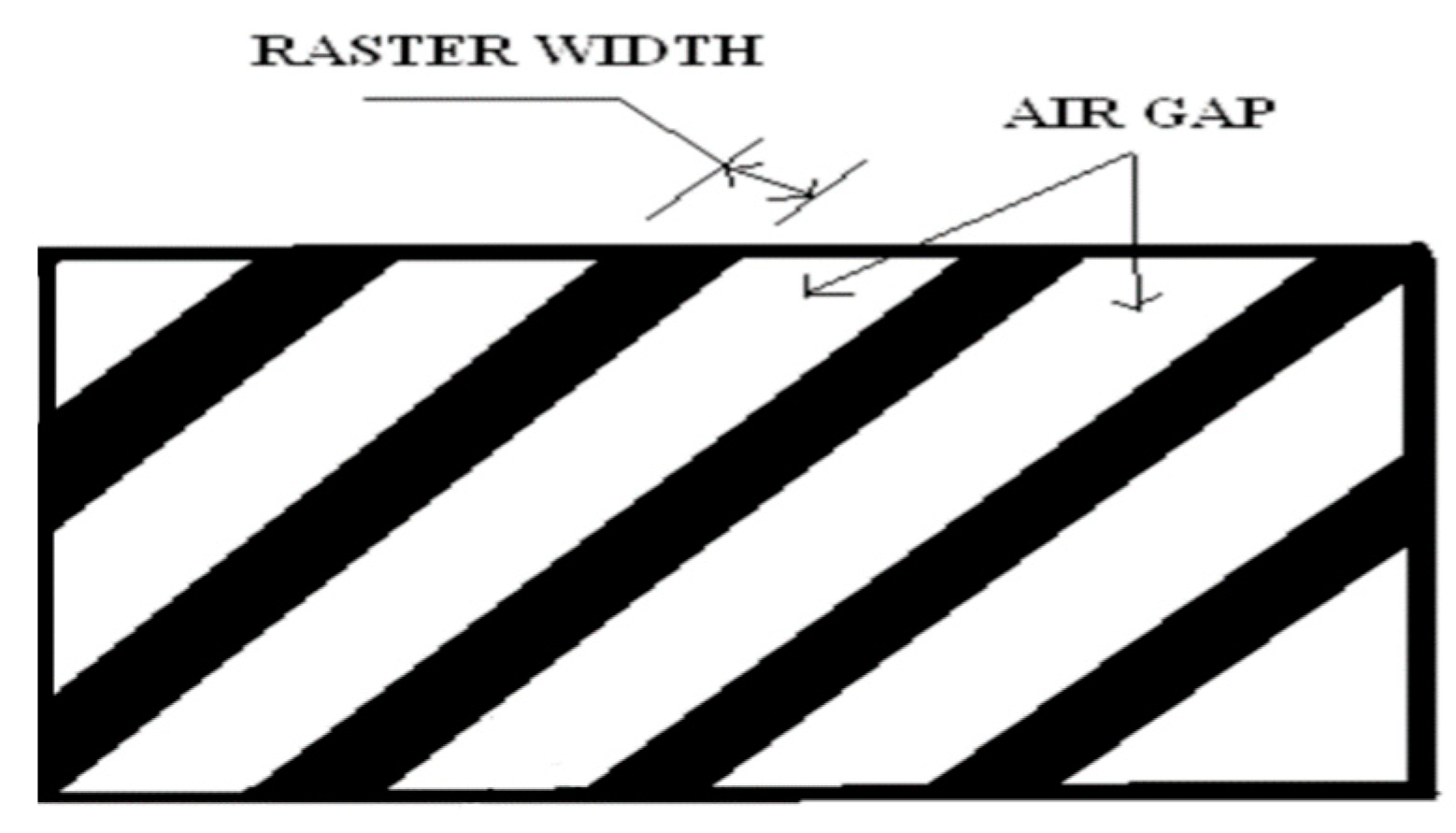
- Air gap: The gap between two adjacent rasters on a deposited layer. The air gap is called negative when two adjacent layers are overlapped.
- Build orientation: Build orientation is defined as the way to orient the part in a build platform with respect to X-, Y-, and Z-axes. In some papers, build orientation represented a quantitative parameter [13,19], but in others, it was considered a categorical parameter [11,20]. Different build orientations are given in Figure 1.
- Extrusion temperature: The temperature at which the filament of a material is heated during the FDM process. Extrusion temperature depends on various aspects, for example, the type of material or print speed.
- Infill density: The outer layers of a three-dimensional (3D) printer object are solid. However, the internal structure, commonly known as the infill, is an invisible inner part covered by the outer layer(s), and it has different shapes, sizes, and patterns. Infill density is the percentage of infill volume with filament material. The strength and mass of FDM build parts depend on the infill density.
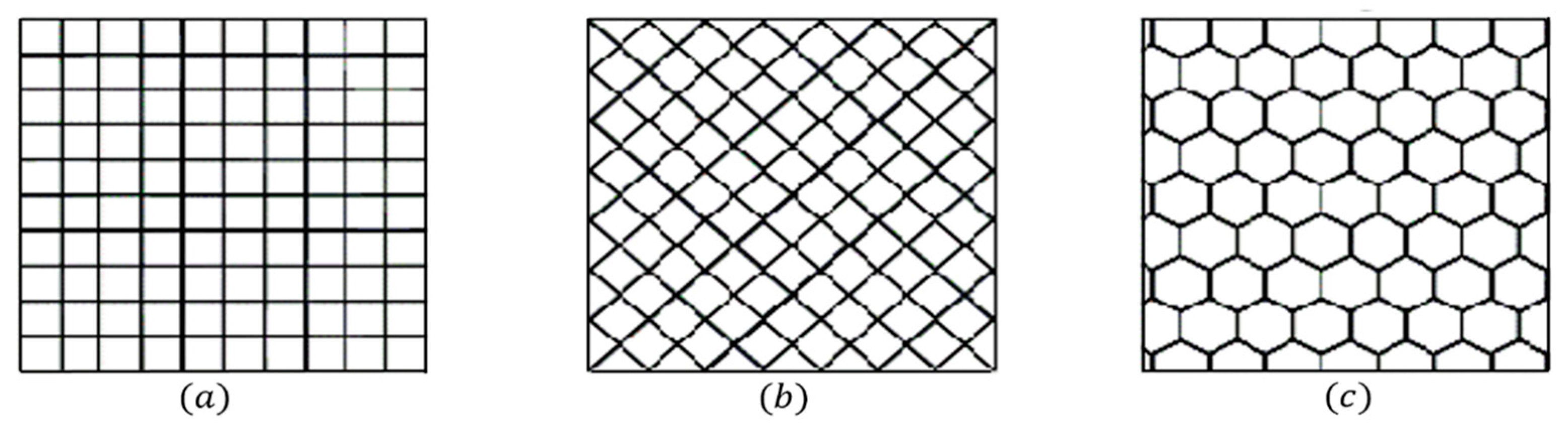
- Infill pattern: Different infill patterns are used in parts to produce a strong and durable internal structure. Hexagonal, diamond, and linear are commonly used infill patterns (Figure 2).
- Layer thickness: This is the height of the deposited layers along the Z-axis, which is generally the vertical axis of an FDM machine. Generally, it is less than the diameter of the extruder nozzle and depends on the diameter of the nozzle.
- Print speed: This is the distance traveled by the extruder along the XY plane per unit time while extruding. Printing time depends on print speed, and the print speed is measured in mm/s.
- Raster width: Raster width is defined as the width of the deposition beads (Figure 3). It depends on the extrusion nozzle diameter.
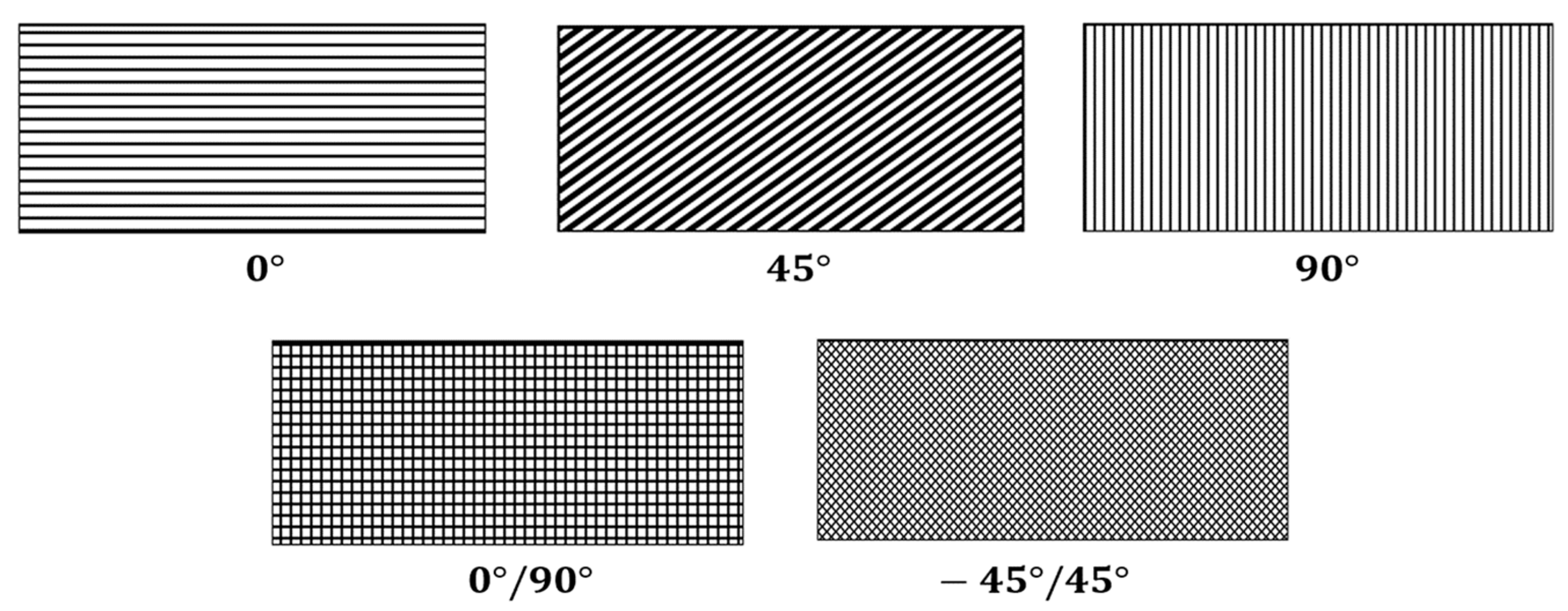
- Raster orientation: This is the direction of the deposition bead with respect to the X-axis of the build platform of the FDM machine (Figure 4).
2.2. FDM Equipment
2.3. Filament Materials
- Acrylonitrile butadiene styrene (ABS): ABS, a thermoplastic and amorphous polymer, is one of the commonly used materials to make 3D printed parts via the FDM process. ABS is a copolymer made of acrylonitrile, butadiene, and styrene; impact resistance and toughness are two important mechanical properties of ABS. ABS has a melting point of 230° (standard for printing, although amorphous), which is higher than polylactic acid (PLA)’s melting point [45]. While PLA is biodegradable, ABS is not, but it offers a lower risk of jamming a nozzle.
- Polylactic acid (PLA): PLA is one of the widely used thermoplastics in FDM. The use of PLA is increasing as it is a biodegradable thermoplastic [46]. Also, it needs less energy and temperature to process prototypes and functional parts with good quality. Now, many desktop 3D printers use PLA as a filament as it does not require a heated bed, although it is prone to jamming a printer nozzle during printing. PLA has higher tensile strength, low warp, and low ductility when compared to ABS. For post-processing, PLA built parts required extra care compared to ABS. In Table 1, some important properties of PLA and ABS are summarized. The presented properties will help choose the right filament for the part to be printed.
- Polycarbonates (PCs): PCs are a group of thermoplastics known for their good strength, durability, and toughness, and some are transparent. They are high-temperature thermoplastics with good heat resistance, good layer for layer bonding, and they provide a good-quality surface.
- Polyether ether ketone (PEEK): PEEK is a thermoplastic with excellent heat resistance, mechanical properties, and chemical stability. It has higher mechanical properties when compared to PLA and ABS. PEEK, a biomaterial, is considered as a promising bone repair material to make prostheses for the human body.
- Polyetherimide (PEI): PEI is widely used in the transportation industry for its high strength-to-weight ratio with low smoke evolution and low smoke toxicity. It requires a high extrusion temperature and bed temperature during printing. Its trade name is ULTEM™ 9085. Due to its low density and toxicity properties, it can be used for aircraft cabins.
- Nylon: Nylon can be chosen as the filament if the requirement is to print more flexible and more durable parts. It has high toughness and impact resistance, but it is highly sensitive to moisture. Nylon can warp about as much as ABS. Like many other FDM filaments, nylon absorbs moisture from the air as it is hygroscopic. Moisture absorption deteriorates filament properties and results in part characteristic degradation.
- Other materials: In addition to the commonly used materials discussed above, there are some other materials that are not commonly used or analyzed as filament materials, for instance, high-impact polystyrene (HIPS), polyphenylsulfone (PPSF), polyethylene terephthalate glycol modified (PETG), thermoplastic polyurethane (TPU), bio-composite filaments, ceramic filaments, and other composite material filaments. Most of these materials are either still in the development process or are not easily obtained on the market.
3. Research on Process Parameter Analysis
3.1. Dimensional Accuracy
3.2. Surface Roughness
3.3. Mechanical Properties
3.3.1. Tensile Strength
3.3.2. Compressive Strength
3.3.3. Flexural Strength
3.4. Build Time
3.5. Part Geometry
3.6. Other Part Characteristics
4. Process Parameter Optimization
5. Discussion and Potential Research Areas
6. Conclusion
- PLA and ABS are the two most widely used materials. Along with PLA and ABS, other materials such as nylon, PETG, and composite materials can be used as filament materials for research purposes, as well for producing functional parts, to get a wider range of material selections and printed part characteristics.
- Some process parameters such as infill pattern, print speed, shell width, or extrusion temperature are less analyzed compared to layer thickness, build orientation, raster width, or raster orientation. The least known process parameters may be considered as variables for future research directions.
- There is limited research that optimized multiple parts’ characteristics simultaneously. Further research on multi-objective process parameter optimizations can be another direction for future research.
- The FDM process is complex. It consists of several steps, and each step has different levels of uncertainty. Consistency of printed FDM parts can be improved by considering uncertainties during design and manufacturing. Additionally, it is also essential to incorporate the uncertainty of mathematical models and algorithms during analysis.
- Toward a multi-disciplinary research direction, various machine learning algorithms and image processing may be applied for predicting part characteristics in the FDM process.
Author Contributions
Funding
Conflicts of Interest
References
- uz Zaman, U.K.; Boesch, E.; Siadat, A.; Rivette, M.; Baqai, A.A. Impact of fused deposition modeling (FDM) process parameters on strength of built parts using Taguchi’s design of experiments. Int. J. Adv. Manuf. Technol. 2019, 101, 1215–1226. [Google Scholar] [CrossRef]
- Chen, H.; Yang, X.; Chen, L.; Wang, Y.; Sun, Y. Application of FDM three-dimensional printing technology in the digital manufacture of custom edentulous mandible trays. Sci. Rep. 2016, 6, 19207. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Letcher, T.; Rankouhi, B.; Javadpour, S. Experimental study of mechanical properties of additively manufactured ABS plastic as a function of layer parameters. In Proceedings of the ASME 2015 International Mechanical Engineering Congress and Exposition (IMECE), Houston, TX, USA, 13–19 November 2015. [Google Scholar]
- Durgun, I.; Ertan, R. Experimental investigation of FDM process for improvement of mechanical properties and production cost. Rapid Prototyp. J. 2014, 20, 228–235. [Google Scholar] [CrossRef]
- Raney, K.; Lani, E.; Kalla, D.K. Experimental characterization of the tensile strength of ABS parts manufactured by fused deposition modeling process. Mater. Today Proc. 2017, 4, 7956–7961. [Google Scholar] [CrossRef]
- Nancharaiah, T.; Raju, D.R.; Raju, V.R. An experimental investigation on surface quality and dimensional accuracy of FDM components. Int. J. Emerg. Technol. 2010, 1, 106–111. [Google Scholar]
- Nidagundi, V.B.; Keshavamurthy, R.; Prakash, C. Studies on parametric optimization for fused deposition modelling process. Mater. Today Proc. 2015, 2, 1691–1699. [Google Scholar] [CrossRef]
- Sood, A.K.; Ohdar, R.K.; Mahapatra, S.S. Experimental investigation and empirical modelling of FDM process for compressive strength improvement. J. Adv. Res. 2012, 3, 81–90. [Google Scholar] [CrossRef] [Green Version]
- Nancharaiah, T. Optimization of process parameters in FDM process using design of experiments. Int. J. Emerg. Technol. 2011, 2, 100–102. [Google Scholar]
- Rayegani, F.; Onwubolu, G.C. Fused deposition modelling (FDM) process parameter prediction and optimization using group method for data handling (GMDH) and differential evolution (DE). Int. J. Adv. Manuf. Technol. 2014, 73, 509–519. [Google Scholar] [CrossRef]
- Chacón, J.; Caminero, M.; García-Plaza, E.; Núñez, P. Additive manufacturing of PLA structures using fused deposition modelling: Effect of process parameters on mechanical properties and their optimal selection. Mater. Des. 2017, 124, 143–157. [Google Scholar] [CrossRef]
- Sood, A.K.; Ohdar, R.K.; Mahapatra, S.S. Parametric appraisal of mechanical property of fused deposition modelling processed parts. Mater. Des. 2010, 31, 287–295. [Google Scholar] [CrossRef]
- Panda, S.K.; Padhee, S.; Anoop Kumar, S.; Mahapatra, S.S. Optimization of fused deposition modelling (FDM) process parameters using bacterial foraging technique. Intell. Inf. Manag. 2009, 1, 89–97. [Google Scholar] [CrossRef]
- Akande, S.O. Dimensional accuracy and surface finish optimization of fused deposition modelling parts using desirability function analysis. Int. J. Eng. Res. Technol 2015, 4, 196–202. [Google Scholar]
- Gurrala, P.K.; Regalla, S.P. Multi-objective optimisation of strength and volumetric shrinkage of FDM parts: a multi-objective optimization scheme is used to optimize the strength and volumetric shrinkage of FDM parts considering different process parameters. Virtual Phys. Prototyp. 2014, 9, 127–138. [Google Scholar] [CrossRef]
- Rao, R.V.; Rai, D.P. Optimization of fused deposition modeling process using teaching-learning-based optimization algorithm. Eng. Sci. Technol. Int. J. 2016, 19, 587–603. [Google Scholar] [CrossRef] [Green Version]
- Mohamed, O.A.; Masood, S.H.; Bhowmik, J.L. Optimization of fused deposition modeling process parameters: A review of current research and future prospects. Adv. Manuf. 2015, 3, 42–53. [Google Scholar] [CrossRef]
- Popescu, D.; Zapciu, A.; Amza, C.; Baciu, F.; Marinescu, R. FDM process parameters influence over the mechanical properties of polymer specimens: A review. Polym. Test. 2018, 69, 157–166. [Google Scholar] [CrossRef]
- Raju, M.; Gupta, M.K.; Bhanot, N.; Sharma, V.S. A hybrid PSO–BFO evolutionary algorithm for optimization of fused deposition modelling process parameters. J. Intell. Manuf. 2018, 1–16. [Google Scholar] [CrossRef]
- Qattawi, A.; Alrawi, B.; Guzman, A. Experimental optimization of fused deposition modelling processing parameters: a design-for-manufacturing approach. Procedia Manuf. 2017, 10, 791–803. [Google Scholar]
- Zaldivar, R.; Witkin, D.; McLouth, T.; Patel, D.; Schmitt, K.; Nokes, J. Influence of processing and orientation print effects on the mechanical and thermal behavior of 3D-Printed ULTEM® 9085 Material. Addit. Manuf. 2017, 13, 71–80. [Google Scholar] [CrossRef]
- Mohamed, O.A.; Masood, S.H.; Bhowmik, J.L.; Nikzad, M.; Azadmanjiri, J. Effect of process parameters on dynamic mechanical performance of fdm PC/ABS printed parts through design of experiment. J. Mater. Eng. Perform. 2016, 25, 2922–2935. [Google Scholar] [CrossRef]
- Cantrell, J.T.; Rohde, S.; Damiani, D.; Gurnani, R.; DiSandro, L.; Anton, J.; Young, A.; Jerez, A.; Steinbach, D.; Kroese, C. Experimental characterization of the mechanical properties of 3D-printed ABS and polycarbonate parts. Rapid Prototyp. J. 2017, 23, 811–824. [Google Scholar] [CrossRef]
- Kumar, G.P.; Regalla, S.P. Optimization of support material and build time in fused deposition modeling (FDM). Appl. Mech. Mater. 2012, 110–116, 2245–2251. [Google Scholar] [CrossRef]
- Montero, M.; Roundy, S.; Odell, D.; Ahn, S.-H.; Wright, P.K. Material characterization of fused deposition modeling (FDM) ABS by designed experiments. Soc. Manuf. Eng. 2001, 10, 1–21. [Google Scholar]
- Es-Said, O.; Foyos, J.; Noorani, R.; Mendelson, M.; Marloth, R.; Pregger, B. Effect of layer orientation on mechanical properties of rapid prototyped samples. Mater. Manuf. Process. 2000, 15, 107–122. [Google Scholar] [CrossRef]
- Chin Ang, K.; Fai Leong, K.; Kai Chua, C.; Chandrasekaran, M. Investigation of the mechanical properties and porosity relationships in fused deposition modelling-fabricated porous structures. Rapid Prototyp. J. 2006, 12, 100–105. [Google Scholar] [CrossRef]
- Vasudevarao, B.; Natarajan, D.P.; Henderson, M.; Razdan, A. Sensitivity of RP surface finish to process parameter variation. In Proceedings of the Solid Freeform Fabrication Proceedings, Austin, TX, USA, 7–9 August 2000; pp. 251–258. [Google Scholar]
- Arivazhagan, A.; Masood, S. Dynamic mechanical properties of ABS material processed by fused deposition modelling. Int. J. Eng. Res. Appl 2012, 2, 2009–2014. [Google Scholar]
- Jami, H.; Masood, S.H.; Song, W. Dynamic response of FDM made ABS parts in different part orientations. Adv. Mater. Res. 2013, 748, 291–294. [Google Scholar] [CrossRef]
- Fernandes, J.; Deus, A.M.; Reis, L.; Vaz, M.F.; Leite, M. Study of the influence of 3D printing parameters on the mechanical properties of PLA. In Proceedings of the 3rd International Conference on Progress in Additive Manufacturing (Pro-AM 2018), Singapore, 14–17 May 2018. [Google Scholar]
- Elkholy, A.; Kempers, R. Investigation into the Influence of Fused Deposition Modeling (FDM) Process Parameters on the Thermal Properties of 3D-Printed Parts. In Proceedings of the 2018 Canadian Society for Mechanical Engineering (CSME) International Congress, Toronto, ON, Canada, 27–30 May 2018. [Google Scholar]
- Liu, X.; Zhang, M.; Li, S.; Si, L.; Peng, J.; Hu, Y. Mechanical property parametric appraisal of fused deposition modeling parts based on the gray Taguchi method. Int. J. Adv. Manuf. Technol. 2017, 89, 2387–2397. [Google Scholar] [CrossRef]
- Torres, J.; Cotelo, J.; Karl, J.; Gordon, A.P. Mechanical property optimization of FDM PLA in shear with multiple objectives. JOM 2015, 67, 1183–1193. [Google Scholar] [CrossRef]
- Peng, A.; Xiao, X.; Yue, R. Process parameter optimization for fused deposition modeling using response surface methodology combined with fuzzy inference system. Int. J. Adv. Manuf. Technol. 2014, 73, 87–100. [Google Scholar] [CrossRef]
- Lee, B.H.; Abdullah, J.; Khan, Z.A. Optimization of rapid prototyping parameters for production of flexible ABS object. J. Mater. Process. Technol. 2005, 169, 54–61. [Google Scholar] [CrossRef]
- Laeng, J.; Khan, Z.A.; Khu, S. Optimizing flexible behaviour of bow prototype using Taguchi approach. J. Appl. Sci. 2006, 6, 622–630. [Google Scholar]
- Wu, J. Study on optimization of 3D printing parameters. IOP Conf. Ser. Mater. Sci. Eng. 2018, 392, 062050. [Google Scholar] [CrossRef]
- Ahn, D.; Kweon, J.-H.; Kwon, S.; Song, J.; Lee, S. Representation of surface roughness in fused deposition modeling. J. Mater. Process. Technol. 2009, 209, 5593–5600. [Google Scholar] [CrossRef]
- Bakar, N.S.A.; Alkahari, M.R.; Boejang, H. Analysis on fused deposition modelling performance. J. Zhejiang Univ.-Sci. A 2010, 11, 972–977. [Google Scholar] [CrossRef]
- Noriega, A.; Blanco, D.; Alvarez, B.; Garcia, A. Dimensional accuracy improvement of FDM square cross-section parts using artificial neural networks and an optimization algorithm. Int. J. Adv. Manuf. Technol. 2013, 69, 2301–2313. [Google Scholar] [CrossRef]
- 3D Printer Filament Comparison Guide. Available online: https://www.matterhackers.com/3d-printer-filament-compare (accessed on 17 July 2019).
- 3D Printer Filament Guide—All You Need to Know in 2019. Available online: https://all3dp.com/1/3d-printer-filament-types-3d-printing-3d-filament/ (accessed on 17 July 2019).
- FDM 3D Printing Materials Compared. Available online: https://www.3dhubs.com/knowledge-base/fdm-3d-printing-materials-compared (accessed on 17 July 2019).
- ABS. Available online: https://reprap.org/wiki/ABS (accessed on 13 July 2019).
- Sin, L.T. Polylactic Acid: PLA Biopolymer Technology and Applications; William Andrew: Boston, MA, USA, 2012. [Google Scholar]
- PLA vs ABS Filament: Plastic Strength, Flexibility Compared! Which Is Better For 3D Printing? Available online: https://www.allthat3d.com/pla-vs-abs/ (accessed on 21 June 2019).
- Messimer, S.L.; Patterson, A.E.; Muna, N.; Deshpande, A.P.; Rocha Pereira, T. Characterization and processing behavior of heated aluminum-polycarbonate composite build plates for the FDM additive manufacturing process. J. Manuf. Mater. Process. 2018, 2, 12. [Google Scholar] [CrossRef]
- Messimer, S.L.; Pereira, T.R.; Patterson, A.E.; Lubna, M.; Drozda, F.O. Full-Density Fused Deposition Modeling Dimensional Error as a Function of Raster Angle and Build Orientation: Large Dataset for Eleven Materials. J. Manuf. Mater. Process. 2019, 3, 6. [Google Scholar] [CrossRef]
- Zhang, Y.; Chou, K. A parametric study of part distortions in fused deposition modelling using three-dimensional finite element analysis. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2008, 222, 959–968. [Google Scholar] [CrossRef]
- Mohamed, O.A.; Masood, S.H.; Bhowmik, J.L. Mathematical modeling and FDM process parameters optimization using response surface methodology based on Q-optimal design. Appl. Math. Model. 2016, 40, 10052–10073. [Google Scholar] [CrossRef]
- Byun, H.-S.; Lee, K.H. Determination of the optimal part orientation in layered manufacturing using a genetic algorithm. Int. J. Prod. Res. 2005, 43, 2709–2724. [Google Scholar] [CrossRef]
- Wang, C.C.; Lin, T.-W.; Hu, S.-S. Optimizing the rapid prototyping process by integrating the Taguchi method with the Gray relational analysis. Rapid Prototyp. J. 2007, 13, 304–315. [Google Scholar] [CrossRef]
- Sood, A.K.; Ohdar, R.; Mahapatra, S.S. Improving dimensional accuracy of fused deposition modelling processed part using grey Taguchi method. Mater. Des. 2009, 30, 4243–4252. [Google Scholar] [CrossRef]
- Mohamed, O.A.; Masood, S.H.; Bhowmik, J.L. Optimization of fused deposition modeling process parameters for dimensional accuracy using I-optimality criterion. Measurement 2016, 81, 174–196. [Google Scholar] [CrossRef]
- Tontowi, A.; Ramdani, L.; Erdizon, R.; Baroroh, D. Optimization of 3D-printer process parameters for improving quality of polylactic acid printed part. Int. J. Eng. Technol. 2017, 9, 589–600. [Google Scholar]
- Beniak, J.; Križan, P.; Šooš, Ľ.; Matúš, M. Research on Shape and Dimensional Accuracy of FDM Produced Parts. IOP Conf. Ser. Mater. Sci. Eng. 2019, 501, 012030. [Google Scholar] [CrossRef]
- Anitha, R.; Arunachalam, S.; Radhakrishnan, P. Critical parameters influencing the quality of prototypes in fused deposition modelling. J. Mater. Process. Technol. 2001, 118, 385–388. [Google Scholar] [CrossRef]
- Thrimurthulu, K.; Pandey, P.M.; Reddy, N.V. Optimum part deposition orientation in fused deposition modeling. Int. J. Mach. Tools Manuf. 2004, 44, 585–594. [Google Scholar] [CrossRef]
- Horvath, D.; Noorani, R.; Mendelson, M. Improvement of surface roughness on ABS 400 polymer using design of experiments (DOE). Mater. Sci. Forum 2007, 561–565, 2389–2392. [Google Scholar] [CrossRef]
- Galantucci, L.M.; Lavecchia, F.; Percoco, G. Experimental study aiming to enhance the surface finish of fused deposition modeled parts. CIRP Ann. 2009, 58, 189–192. [Google Scholar] [CrossRef]
- Valerga, A.; Batista, M.; Salguero, J.; Girot, F. Influence of PLA Filament Conditions on Characteristics of FDM Parts. Materials 2018, 11, 1322. [Google Scholar] [CrossRef]
- Pérez, M.; Medina-Sánchez, G.; García-Collado, A.; Gupta, M.; Carou, D. Surface quality enhancement of fused deposition modeling (FDM) printed samples based on the selection of critical printing parameters. Materials 2018, 11, 1382. [Google Scholar] [CrossRef]
- Bagsik, A.; Schöppner, V.; Klemp, E. FDM part quality manufactured with Ultem* 9085. In Proceedings of the 14th International Scientific Conference on Polymeric Materials, Halle, Germany, 15–17 September 2010; pp. 307–315. [Google Scholar]
- Domingo-Espin, M.; Puigoriol-Forcada, J.M.; Garcia-Granada, A.-A.; Llumà, J.; Borros, S.; Reyes, G. Mechanical property characterization and simulation of fused deposition modeling Polycarbonate parts. Mater. Des. 2015, 83, 670–677. [Google Scholar] [CrossRef]
- Hernandez, R.; Slaughter, D.; Whaley, D.; Tate, J.; Asiabanpour, B. Analyzing the tensile, compressive, and flexural properties of 3D printed ABS P430 plastic based on printing orientation using fused deposition modeling. In Proceedings of the 27th Annual International Solid Freeform Fabrication Symposium, Austin, TX, USA, 12–14 August 2019; pp. 939–950. [Google Scholar]
- Fatimatuzahraa, A.; Farahaina, B.; Yusoff, W. The effect of employing different raster orientations on the mechanical properties and microstructure of Fused Deposition Modeling parts. In Proceedings of the 2011 IEEE Symposium on Business, Engineering and Industrial Applications (ISBEIA), Langkawi, Malaysia, 25–28 September 2011; pp. 22–27. [Google Scholar]
- Croccolo, D.; De Agostinis, M.; Olmi, G. Experimental characterization and analytical modelling of the mechanical behaviour of fused deposition processed parts made of ABS-M30. Comput. Mater. Sci. 2013, 79, 506–518. [Google Scholar] [CrossRef]
- Panda, B.N.; Bahubalendruni, M.R.; Biswal, B.B. Comparative evaluation of optimization algorithms at training of genetic programming for tensile strength prediction of FDM processed part. Procedia Mater. Sci. 2014, 5, 2250–2257. [Google Scholar] [CrossRef]
- Górski, F.; Wichniarek, R.; Kuczko, W.; Andrzejewski, J. Experimental determination of critical orientation of ABS parts manufactured using fused deposition modelling technology. J. Mach. Eng. 2015, 15, 121–132. [Google Scholar]
- Wu, W.; Geng, P.; Li, G.; Zhao, D.; Zhang, H.; Zhao, J. Influence of layer thickness and raster angle on the mechanical properties of 3D-printed PEEK and a comparative mechanical study between PEEK and ABS. Materials 2015, 8, 5834–5846. [Google Scholar] [CrossRef]
- Ziemian, S.; Okwara, M.; Ziemian, C.W. Tensile and fatigue behavior of layered acrylonitrile butadiene styrene. Rapid Prototyp. J. 2015, 21, 270–278. [Google Scholar] [CrossRef]
- Wittbrodt, B.; Pearce, J.M. The effects of PLA color on material properties of 3-D printed components. Addit. Manuf. 2015, 8, 110–116. [Google Scholar] [CrossRef] [Green Version]
- Torres, J.; Cole, M.; Owji, A.; DeMastry, Z.; Gordon, A.P. An approach for mechanical property optimization of fused deposition modeling with polylactic acid via design of experiments. Rapid Prototyp. J. 2016, 22, 387–404. [Google Scholar] [CrossRef]
- Torrado, A.R.; Roberson, D.A. Failure analysis and anisotropy evaluation of 3D-printed tensile test specimens of different geometries and print raster patterns. J. Fail. Anal. Prev. 2016, 16, 154–164. [Google Scholar] [CrossRef]
- Rankouhi, B.; Javadpour, S.; Delfanian, F.; Letcher, T. Failure analysis and mechanical characterization of 3D printed ABS with respect to layer thickness and orientation. J. Fail. Anal. Prev. 2016, 16, 467–481. [Google Scholar] [CrossRef]
- Uddin, M.; Sidek, M.; Faizal, M.; Ghomashchi, R.; Pramanik, A. Evaluating mechanical properties and failure mechanisms of fused deposition modeling acrylonitrile butadiene styrene parts. J. Manuf. Sci. Eng. 2017, 139, 081018. [Google Scholar] [CrossRef]
- Mahmood, S.; Qureshi, A.; Goh, K.L.; Talamona, D. Tensile strength of partially filled FFF printed parts: meta modelling. Rapid Prototyp. J. 2017, 23, 524–533. [Google Scholar] [CrossRef]
- Aw, Y.; Yeoh, C.; Idris, M.; Teh, P.; Hamzah, K.; Sazali, S. Effect of printing parameters on tensile, dynamic mechanical, and thermoelectric properties of FDM 3D printed CABS/ZnO composites. Materials 2018, 11, 466. [Google Scholar] [CrossRef]
- Kung, C.; Kuan, H.-C.; Kuan, C.-F. Evaluation of tensile strength of 3D printed objects with FDM process on RepRap platform. In Proceedings of the 2018 1st IEEE International Conference on Knowledge Innovation and Invention (ICKII), Jeju, Korea, 23–27 July 2018; pp. 369–372. [Google Scholar]
- Vosynek, P.; Navrat, T.; Krejbychova, A.; Palousek, D. Influence of Process Parameters of Printing on Mechanical Properties of Plastic Parts Produced by FDM 3D Printing Technology. MATEC Web Conf. 2018, 237, 02014. [Google Scholar] [CrossRef]
- Deng, X.; Zeng, Z.; Peng, B.; Yan, S.; Ke, W. Mechanical properties optimization of poly-ether-ether-ketone via fused deposition modeling. Materials 2018, 11, 216. [Google Scholar] [CrossRef]
- Rinanto, A.; Nugroho, A.; Prasetyo, H.; Pujiyanto, E. Simultaneous Optimization of TensileStrength, Energy Consumption and Processing Time on FDM Process Using Taguchi and PCR-TOPSIS. In Proceedings of the 2018 4th International Conference on Science and Technology (ICST), Yogyakarta, Indonesia, 7–8 August 2018; pp. 1–5. [Google Scholar]
- Li, H.; Wang, T.; Sun, J.; Yu, Z. The effect of process parameters in fused deposition modelling on bonding degree and mechanical properties. Rapid Prototyp. J. 2018, 24, 80–92. [Google Scholar] [CrossRef]
- Rodríguez-Panes, A.; Claver, J.; Camacho, A. The influence of manufacturing parameters on the mechanical behaviour of pla and abs pieces manufactured by fdm: a comparative analysis. Materials 2018, 11, 1333. [Google Scholar] [CrossRef]
- Ahn, S.-H.; Montero, M.; Odell, D.; Roundy, S.; Wright, P.K. Anisotropic material properties of fused deposition modeling ABS. Rapid Prototyp. J. 2002, 8, 248–257. [Google Scholar] [CrossRef] [Green Version]
- Baich, L.; Manogharan, G.; Marie, H. Study of infill print design on production cost-time of 3D printed ABS parts. Int. J. Rapid Manuf. 2015, 5, 308–319. [Google Scholar] [CrossRef]
- Lužanin, O.; Movrin, D.; Plančak, M. Effect of layer thickness, deposition angle, and infill on maximum flexural force in FDM-built specimens. J. Technol. Plast. 2014, 39, 49–58. [Google Scholar]
- Raut, S.; Jatti, V.S.; Khedkar, N.K.; Singh, T. Investigation of the effect of built orientation on mechanical properties and total cost of FDM parts. Procedia Mater. Sci. 2014, 6, 1625–1630. [Google Scholar] [CrossRef]
- Christiyan, K.J.; Chandrasekhar, U.; Venkateswarlu, K. A study on the influence of process parameters on the Mechanical Properties of 3D printed ABS composite. IOP Conf. Ser. Mater. Sci. Eng. 2016, 114, 012109. [Google Scholar] [CrossRef]
- Ali, F.; Chowdary, B.V.; Maharaj, J. Influence of some process parameters on build time, material consumption, and surface roughness of FDM processed parts: Inferences based on the Taguchi design of experiments. In Proceedings of the 2014 IACJ/ISAM Joint International Conference, Orlando, FL, USA, 25–27 September 2014. [Google Scholar]
- Rathee, S.; Srivastava, M.; Maheshwari, S.; Siddiquee, A.N. Effect of varying spatial orientations on build time requirements for FDM process: A case study. Def. Technol. 2017, 13, 92–100. [Google Scholar] [CrossRef]
- Srivastava, M.; Rathee, S.; Maheshwari, S.; Kundra, T. Multi-objective optimisation of fused deposition modelling process parameters using RSM and fuzzy logic for build time and support material. Int. J. Rapid Manuf. 2018, 7, 25–42. [Google Scholar] [CrossRef]
- Shahrain, M.; Didier, T.; Lim, G.K.; Qureshi, A. Fast deviation simulation for ‘fused deposition modeling’process. Procedia CIRP 2016, 43, 327–332. [Google Scholar] [CrossRef]
- Sun, Q.; Rizvi, G.; Bellehumeur, C.; Gu, P. Effect of processing conditions on the bonding quality of FDM polymer filaments. Rapid Prototyp. J. 2008, 14, 72–80. [Google Scholar] [CrossRef]
- Gajdoš, I.; Slota, J. Influence of printing conditions on structure in FDM prototypes. Teh. Vjesn. 2013, 20, 231–236. [Google Scholar]
- Masood, S.; Rattanawong, W.; Iovenitti, P. Part build orientations based on volumetric error in fused deposition modelling. Int. J. Adv. Manuf. Technol. 2000, 16, 162–168. [Google Scholar] [CrossRef]
- Kuznetsov, V.E.; Tavitov, A.G.; Urzhumtsev, O.D.; Mikhalin, M.V.; Solonin, A.N. Design and Fabrication of Strong Parts from Poly (Lactic Acid) with a Desktop 3D Printer: A Case with Interrupted Shell. Polymers 2019, 11, 760. [Google Scholar] [CrossRef]
- Carneiro, P.M.C.; Gamboa, P. Structural analysis of wing ribs obtained by additive manufacturing. Rapid Prototyp. J. 2019. [Google Scholar] [CrossRef]
- Mercado-Colmenero, J.M.; Rubio-Paramio, M.A.; la Rubia-Garcia, M.D.; Lozano-Arjona, D.; Martin-Doñate, C. A numerical and experimental study of the compression uniaxial properties of PLA manufactured with FDM technology based on product specifications. Int. J. Adv. Manuf. Technol. 2019, 103, 1893–1909. [Google Scholar] [CrossRef]
- Rodríguez, J.F.; Thomas, J.P.; Renaud, J.E. Design of fused-deposition ABS components for stiffness and strength. J. Mech. Des. 2003, 125, 545–551. [Google Scholar] [CrossRef]
- Tymrak, B.; Kreiger, M.; Pearce, J.M. Mechanical properties of components fabricated with open-source 3-D printers under realistic environmental conditions. Mater. Des. 2014, 58, 242–246. [Google Scholar] [CrossRef]
- Górski, F.; Kuczko, W.; Wichniarek, R. Impact strength of ABS parts manufactured using Fused Deposition Modeling technology. Arch. Mech. Technol. Autom. 2014, 31, 3–12. [Google Scholar]
- Srivastava, M.; Rathee, S. Optimisation of FDM process parameters by Taguchi method for imparting customised properties to components. Virtual Phys. Prototyp. 2018, 13, 203–210. [Google Scholar] [CrossRef]
- Dong, G.; Wijaya, G.; Tang, Y.; Zhao, Y.F. Optimizing process parameters of fused deposition modeling by Taguchi method for the fabrication of lattice structures. Addit. Manuf. 2018, 19, 62–72. [Google Scholar] [CrossRef] [Green Version]
- Pandey, P.M.; Thrimurthulu, K.; Reddy, N.V. Optimal part deposition orientation in FDM by using a multicriteria genetic algorithm. Int. J. Prod. Res. 2004, 42, 4069–4089. [Google Scholar] [CrossRef]
- Hu, Z.; Mahadevan, S. Uncertainty quantification in prediction of material properties during additive manufacturing. Scr. Mater. 2017, 135, 135–140. [Google Scholar] [CrossRef]

| Property | PLA | ABS |
|---|---|---|
| Printing temperature (○C) | 180–230 | 210–250 |
| Build platform temperature (○C) | 20–60 | 80–110 |
| Raft | Optional | Mandatory |
| Strength | High | Medium |
| Flexibility | Brittle | Moderately flexible |
| Heat resistance | Low | Moderate |
| Biodegradability | Yes | No |
| Moisture absorption | Yes | Yes |
| Reference | Machine/Equipment | Material | Methods/Tools | Process Parameters | Part Characteristics |
|---|---|---|---|---|---|
| Rodriguez et al. [101] | - | ABS | Fused deposition design optimization tool (FDMOT) | Build orientation and raster orientation | Strength |
| Lee et al. [36] | FDM3000 machine | ABS | Taguchi method (L9), S/N ratio, ANOVA | Layer thickness, raster orientation, raster width, air gap | Elastic performance |
| Laeng et al. [37] | FDM3000 machine | ABS | Taguchi method (L9), S/N ratio, ANOVA | Layer thickness, raster orientation, raster width, air gap | Throwing distance of a bow |
| Zhang et al. [50] | - | ABS | CCD, finite element analysis (FEA), ANOVA, regression | Layer thickness, print speed, raster width, | Residual stress and part distortion |
| Es-Said et al. [26] | FDM 1650 machine | ABS | - | Raster orientation | Ultimate tensile strength, yield strength, flexural strength, and impact strength |
| Panda et al. [13] | FDM Vantage SE machine | ABS | CCD, ANOVA, bacterial foraging optimization | Layer thickness, build orientation, raster orientation, raster width, air gap | Tensile strength, flexural strength, and impact strength |
| Sood et al. [12] | FDM Vantage SE machine | ABS | CCD, ANOVA, response surface plot | Layer thickness, build orientation, raster orientation, raster width, air gap | Tensile strength, flexural strength, and impact strength |
| Fatimatuzahraa et al. [67] | Dimension SST 768 machine | ABS | - | Raster orientation | Tensile strength, flexural strength, impact strength, and deflection test |
| Arivazhagan et al. [29] | FDM Vantage machine | ABS | - | Build style, raster orientation, raster width | Viscosity and modulus |
| Jami et al. [30] | FDM Vantage machine | ABS | Build orientation | Dynamic stress–strain response | |
| Tymark et al. [102] | Open-source 3D printers | ABS and PLA | - | Raster orientation and layer thickness | Tensile strength and elastic modulus |
| Peng et al. [35] | MEM-300 machine | ABS | RSM, Fuzzy inference system (FIS), ANN, GA | Width compensation, layer thickness, extrusion velocity and filling velocity | Build time, dimensional accuracy, warp deformation. |
| Letcher et al. [3] | MakerBot Replicator 2x | ABS | - | Raster orientation and layer thickness | Ultimate tensile strength, modulus of elasticity, elongation |
| Torres et al. [34] | MakerBot Replicator 2 | PLA | Taguchi method, regression, ANOVA | Layer thickness, infill density and postprocessing heat-treatment time | Ultimate shear strength, 0.2% yield strength, proportional limit, shear modulus, and fracture strain |
| Baich et al. [87] | Stratasys Fortus 250mc | ABS | - | Infill pattern | Part cost, tensile, compressive and flexural properties |
| Ziemian et al. [72] | Stratasys Vantage-i machine | ABS | ANOVA | Raster orientation | Tensile strength and fatigue performance |
| Cantrell et al. [23] | Fortus 360mc machine and Ultimaker 2 | ABS and PC | digital image correlation | Raster orientation and build orientation | Tensile and shear properties |
| Qattawi et al. [20] | MakerBot Replicator 2x | PLA | FEA | Build orientation, infill density, print speed, layer thickness, infill pattern, extrusion temperature | Young’s modulus, yield strength, tensile strength, dimensional accuracy |
| Zaldivar et al. [21] | Stratasys Fortus 400 mc | Ultem 9085 | Digital image correlation | Build orientation | Tensile strength, failure strain, modulus Poisson’s ratio, thermal expansion |
| Liu et al. [33] | MakerBot Replicator2 | PLA | Taguchi method, S/N ratio, ANOVA, gray relational analysis | Build orientation, layer thickness, raster orientation, raster width, air gap | Tensile, flexural and impact strength |
| Raju et al. [19] | - | ABS | Taguchi method, S/N ratio, regression, hybrid particle swarm and bacterial foraging optimization (PSO–BFO) | Layer thickness, build orientation, support material, model interior | Hardness, flexural modulus, tensile strength, and surface roughness |
| Aw et al. [79] | RepRap Mendelmax 1.5 | ABS/ZnO and CABS/Zno | - | Infill pattern and infill density | Tensile, dynamic and thermoelectric properties, |
| Deng et al. [82] | PEEK | Taguchi method | Print speed, layer thickness, extrusion temperature, infill density | Tensile strength, elongation, flexural strength, impact strength | |
| Fernandes et al. [31] | Ultimaker 2 | PLA | ANOVA | Infill density, extrusion temperature, raster orientation, and layer thickness | Ultimate tensile strength, yield strength, modulus of elasticity and elongation |
| Ang et al. [27] | FDM 1650 machine | ABS | Fractional DoE | Air gap, raster width, build orientation, build layer and build profile | Compressive properties, porosity |
| Kumar et al. [24] | FDM 200mc | ABS | Full factorial design, ANOVA | Layer thickness, raster orientation, raster width, build orientation, shell width | Build time, support material volume |
| Górski et al. [103] | Dimension BST 1200 machine | ABS | - | Build orientation | Impact strength |
| Mohamed et al. [22] | Stratasys FDM Fortus 400 mc | PC–ABS blend | Fraction factorial design, ANOVA, regression | Layer thickness, air gap, raster orientation, build orientation, road width, and number of shells | Storage modulus, loss modulus, mechanical dumping |
| Elkholy et al. [32] | Ultimaker 2 | PLA | Energy equation | Layer height, raster width | Thermal conductivity |
| Es-Said et al. [26] | FDM 1650 machine | ABS | - | Raster orientation | Tensile strength, modulus of rupture, impact resistance |
| Srivastava et al. [93] | Fortus 250mc | ABS | CCD, fuzzy logic | Layer thickness, air gap, raster orientation, build orientation, road width, and shell width | Build time and support volume |
| Srivastava et al. [104] | Fortus 250mc | ABS | ANOVA, S/N ratio | Layer thickness, air gap, road width, and shell width | Material volume |
| Dong et al. [105] | Ultimaker 2 Extended+ | ABS | Taguchi design, S/N ratio, ANOVA | - | Lattice structure |
| Rinanto et al. [83] | - | PLA | Taguchi method, S/N ratio Process Capability Ratio-Technique for Order Performance by Similarity to Ideal Solution (PCR-TOPSIS) | Extrusion temperature, raster orientation, infill density | Tensile strength, energy consumption, and build time |
| Reference | Optimization Method | Process Parameters | Part Characteristics |
|---|---|---|---|
| Sood et al. [8] | QPSO | Layer thickness, build orientation, raster orientation, raster width, and air gap | Compressive strength |
| Rayegani et al. [10] | DE | Build orientation, raster orientation, raster width, and air gap | Tensile strength |
| Panda et al. [69] | PSO | Layer thickness, raster orientation, raster width, and air gap | Tensile strength |
| Panda et al. [13] | Bacteria foraging optimization (BFO) | Layer thickness, build orientation, raster orientation, raster width, and air gap | Tensile, flexural, and impact strength |
| Sood et al. [54] | Gray Taguchi method | Layer thickness, build orientation, raster orientation, raster width, and air gap | Dimensional accuracy (length, width, and thickness) |
| Liu et al. [33] | Gray Taguchi method | Layer thickness, build orientation, raster orientation, raster width, and air gap | Tensile, flexural, and impact strength |
| Sood et al. [12] | Desirability function | Layer thickness, build orientation, raster orientation, raster width, and air gap | Tensile, flexural, and impact strength |
| Akande [14] | Desirability function | Layer thickness, print speed, and infill density | Dimensional accuracy and surface roughness |
| Peng et al. [35] | Fuzzy logic and GA | Line width compensation, extrusion velocity, filling velocity, and layer thickness | Dimensional accuracy, warp deformation, and build time |
| Srivastava et al. [93] | Fuzzy logic | Layer thickness, build orientation, shell width, raster orientation, raster width, and air gap | Build time and support material volume |
| Rinanto et al. [83] | PCR-TOPSIS | Extrusion temperature, infill density, and raster orientation | Tensile strength, energy consumption, and build time |
| Raju et al. [19] | PSO–BFO | Layer thickness, build orientation, support material, and model interior | Surface roughness, hardness, tensile strength, and flexural modulus |
| Pandey et al. [106] | NSGA-II | Build orientation | Surface roughness and build time |
| Gurrala et al. [15] | NSGA-II | Model interior, horizontal direction, and vertical direction | Tensile strength and volumetric shrinkage |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dey, A.; Yodo, N. A Systematic Survey of FDM Process Parameter Optimization and Their Influence on Part Characteristics. J. Manuf. Mater. Process. 2019, 3, 64. https://doi.org/10.3390/jmmp3030064
Dey A, Yodo N. A Systematic Survey of FDM Process Parameter Optimization and Their Influence on Part Characteristics. Journal of Manufacturing and Materials Processing. 2019; 3(3):64. https://doi.org/10.3390/jmmp3030064
Chicago/Turabian StyleDey, Arup, and Nita Yodo. 2019. "A Systematic Survey of FDM Process Parameter Optimization and Their Influence on Part Characteristics" Journal of Manufacturing and Materials Processing 3, no. 3: 64. https://doi.org/10.3390/jmmp3030064
APA StyleDey, A., & Yodo, N. (2019). A Systematic Survey of FDM Process Parameter Optimization and Their Influence on Part Characteristics. Journal of Manufacturing and Materials Processing, 3(3), 64. https://doi.org/10.3390/jmmp3030064





