Modelling of Powder Removal for Additive Manufacture Postprocessing
Abstract
1. Introduction
1.1. Granular Flow
- Quasi-static or granular solid or elastic-quasi-static. For a densely packed bed of particles sheared at a low rate, the stress is independent of the shear rate.
- Dense or granular liquid or elastic-inertial. Multiple and enduring contacts dominate, resulting in the stress being proportional to the shear rate.
1.2. Computational Modelling of Granular Flow
- Microscopic models and particle-based simulations,
- Statistical mechanics and kinetic theories, and
- Continuum and phenomenological models.
1.2.1. Particle-Based Modelling
1.2.2. Continuum Modelling
1.3. Powder Flow Modelling for AM
1.4. Overview and Structure of This Paper
2. System of Equations
2.1. Solver Development
- Drag coefficient,
- Granular viscosity (collisional, kinetic and frictional terms),
- Granular pressure (collisional and kinetic terms),
- Granular conductivity (collisional and kinetic terms),
- Radial distribution function,
- Particle bulk density,
- Production of granular energy by particle-particle collision, and
- Production and dissipation of granular energy by gas-particle slip.
2.2. Governing Equations
2.3. Sub Models
3. Sub-Models: Development and Validation
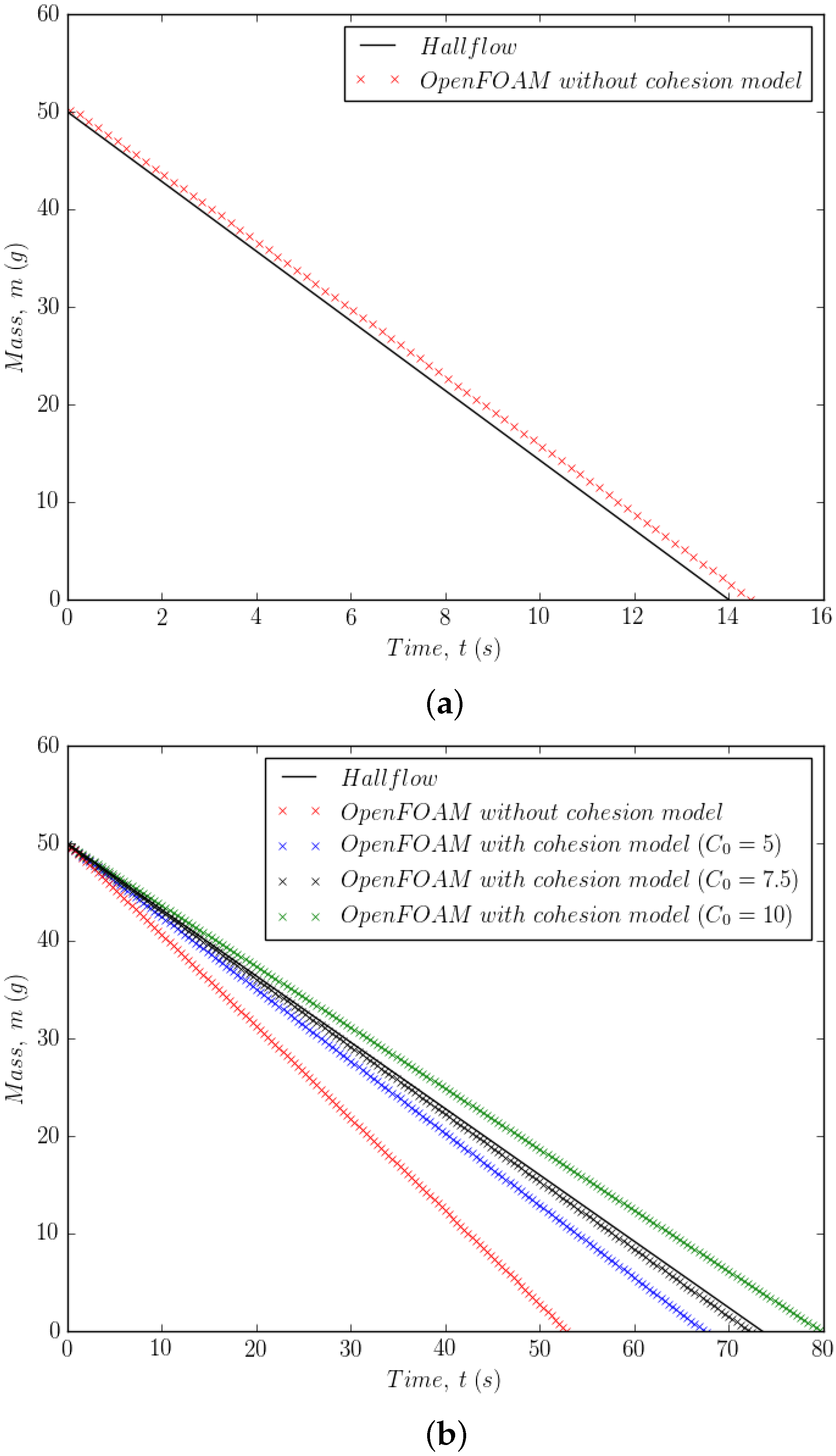
3.1. Cohesive Forces
- Inconel 625—, → Geldart Type B powder (cohesive force weaker than gravity);
- AlSi10Mg—, → Geldart Type A powder (cohesive force as important as gravity).
3.1.1. Modelling Cohesive Forces
3.1.2. Results—The Hallflow Test
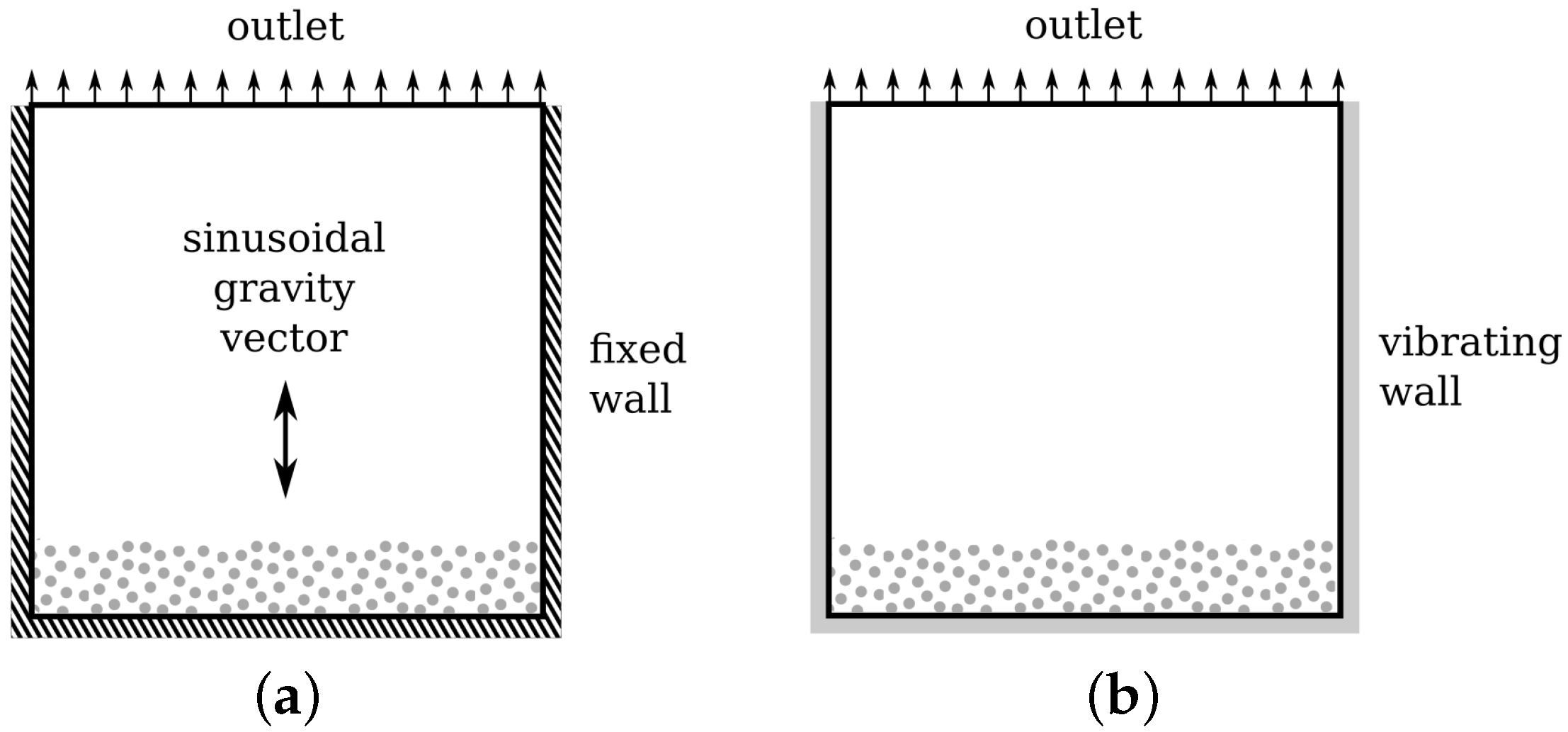
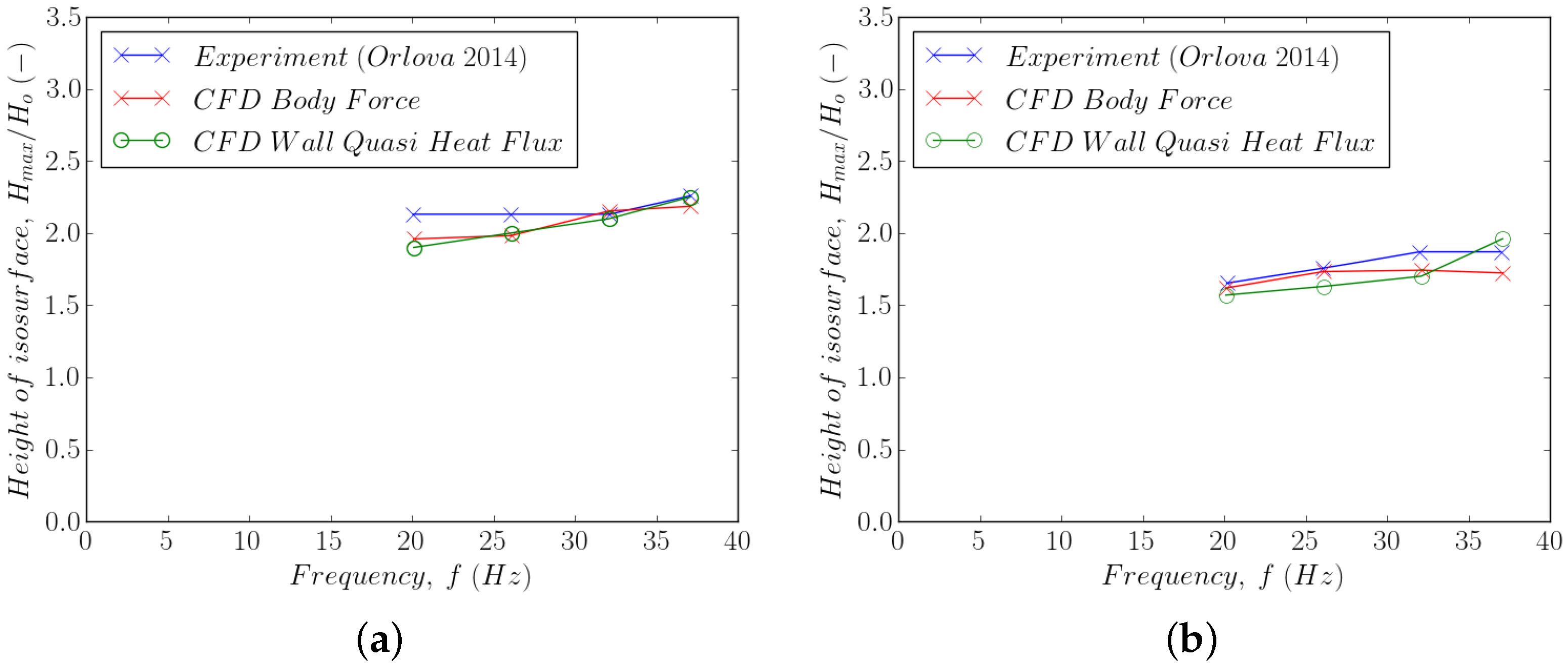
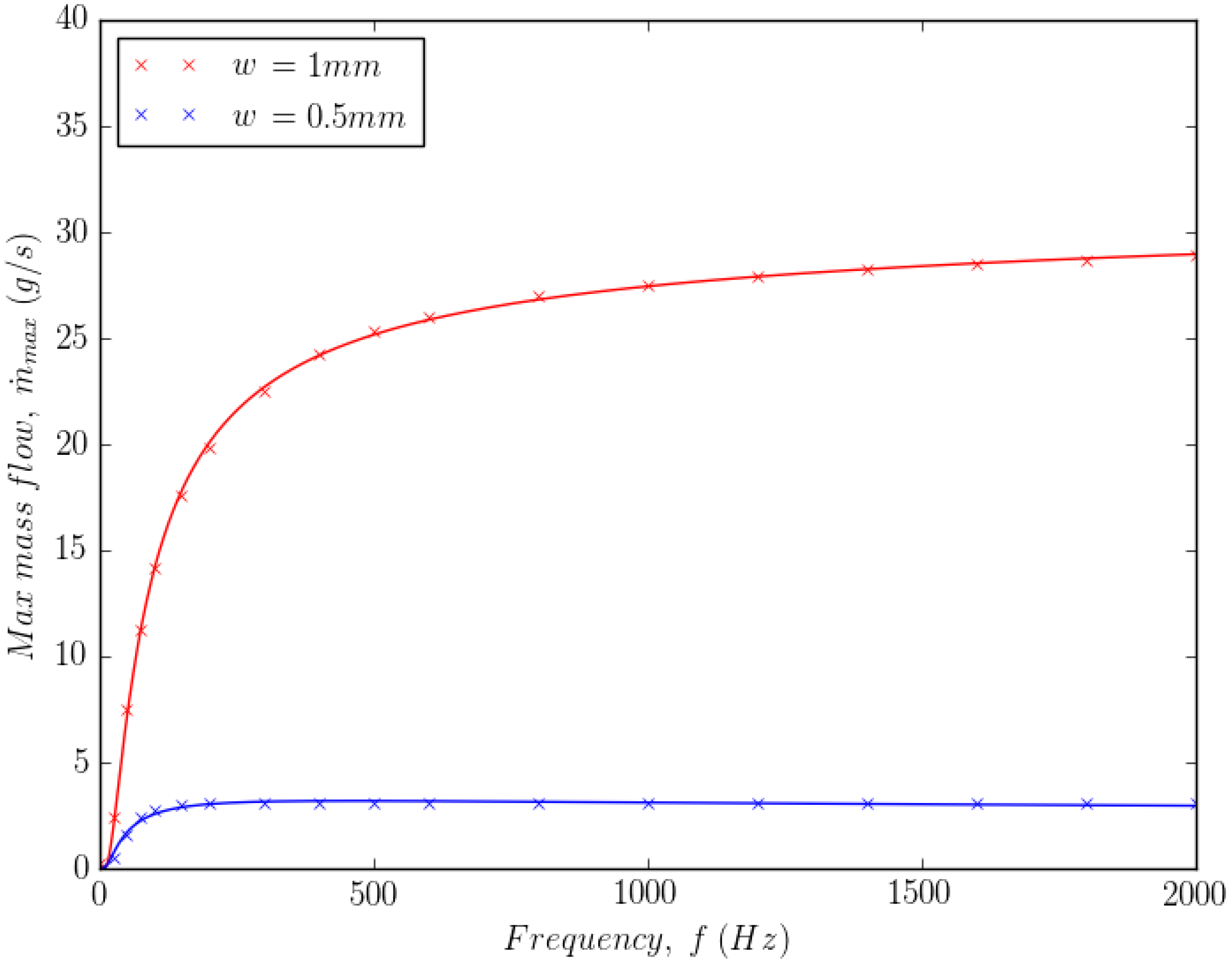
3.2. Wall Vibration
3.2.1. Wall-Normal Vibration Test Case
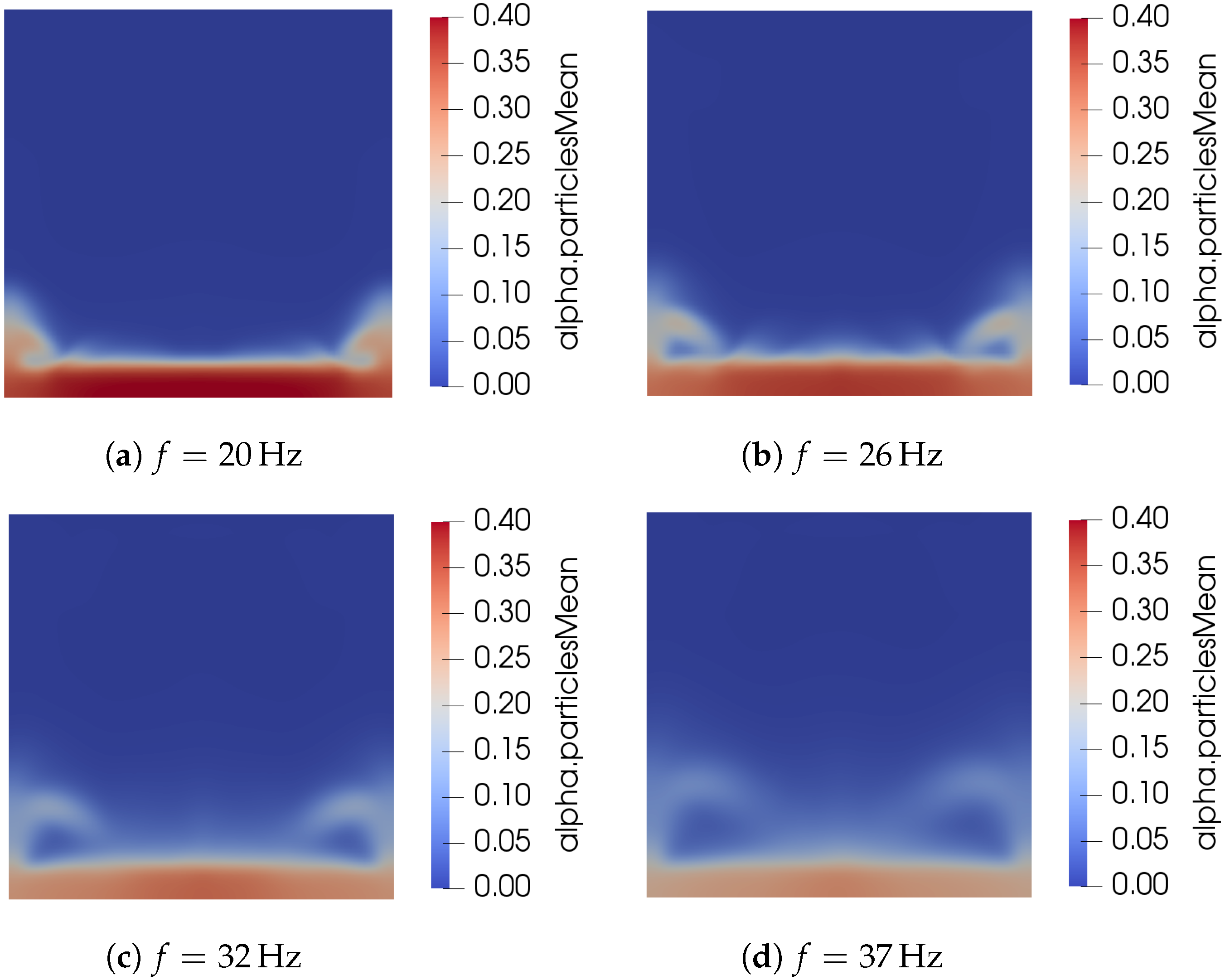
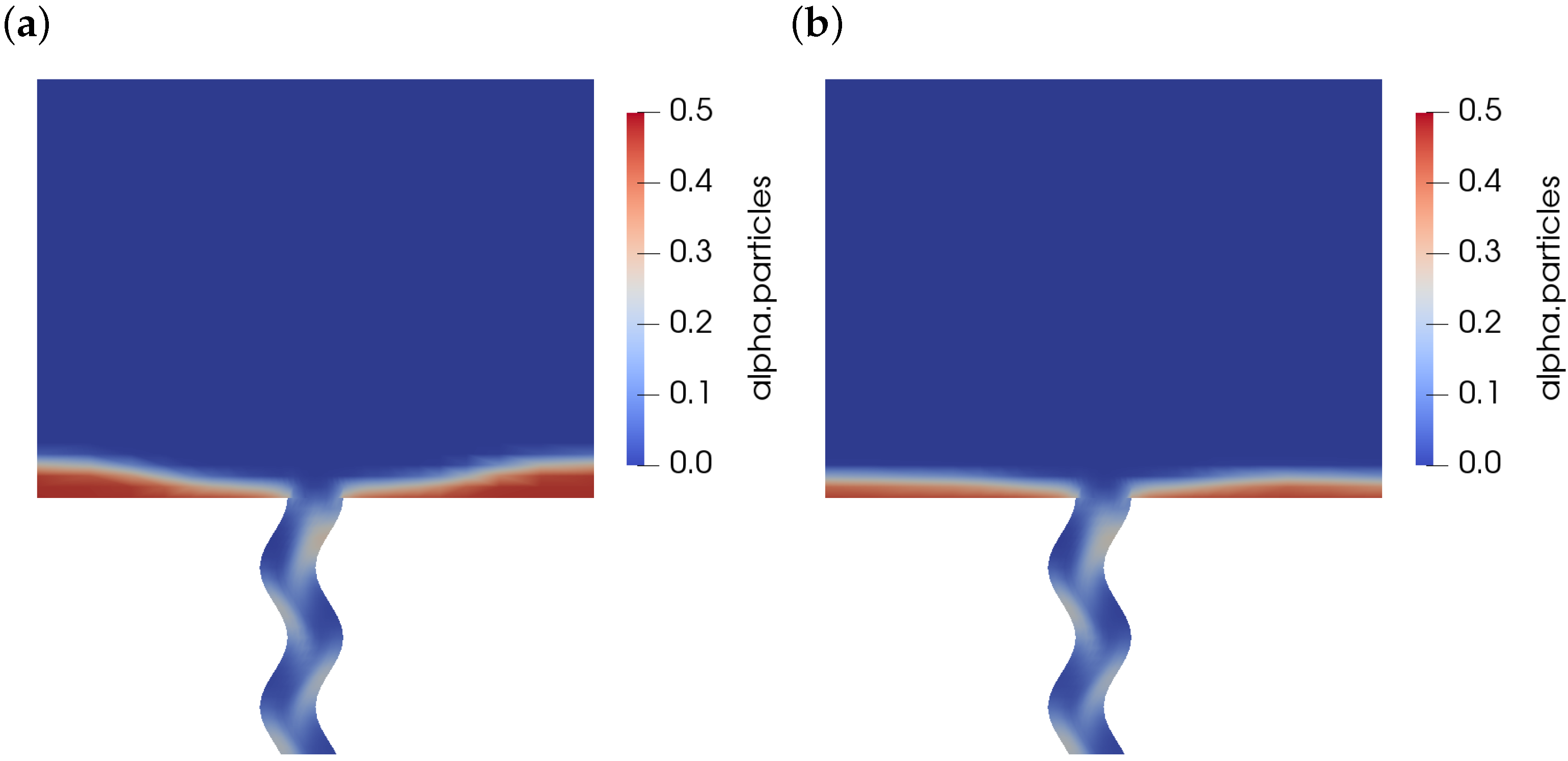
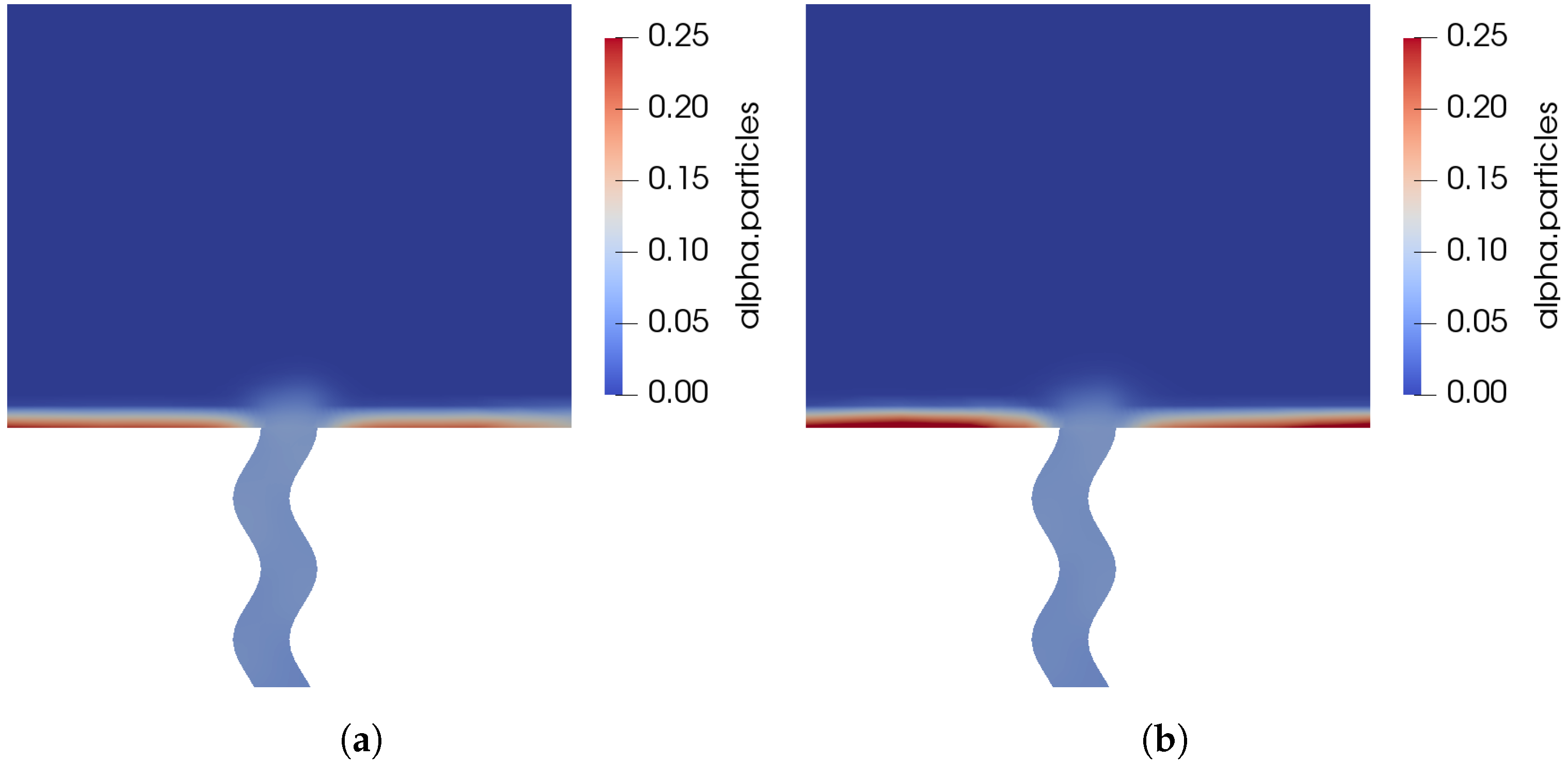
3.2.2. Effect of Vibration on Solid Volume Fraction Field
3.2.3. Effect of Vibration on Surface Height
3.2.4. Effect on Granular Temperature
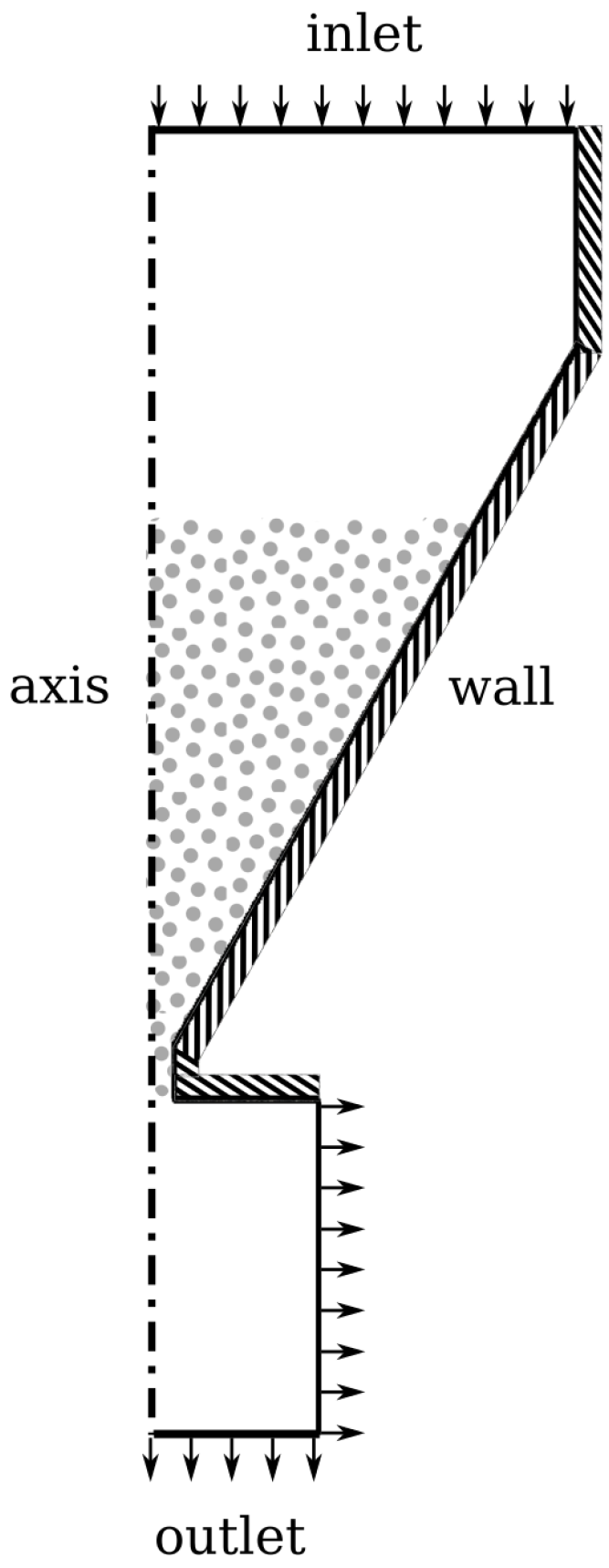
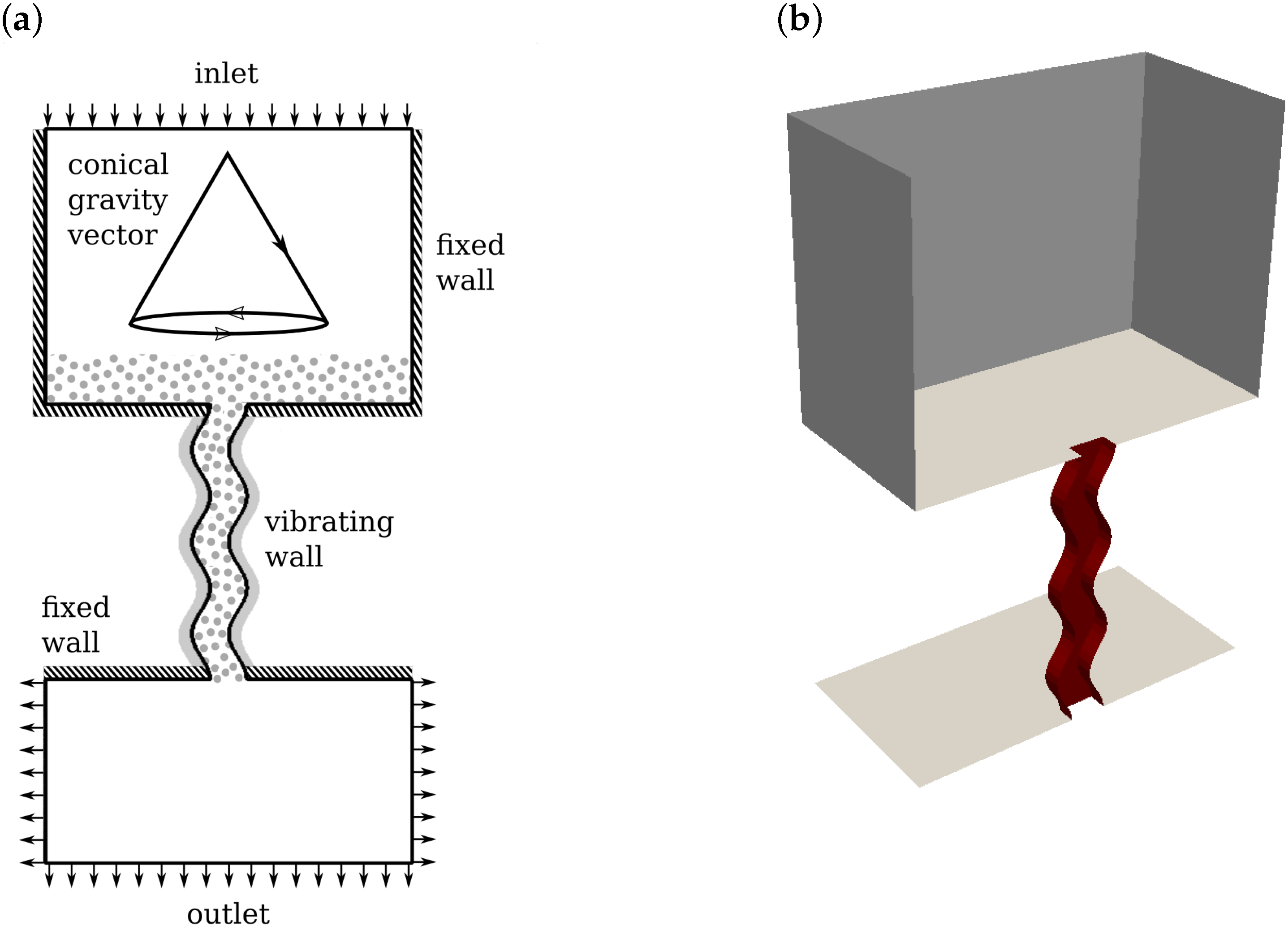
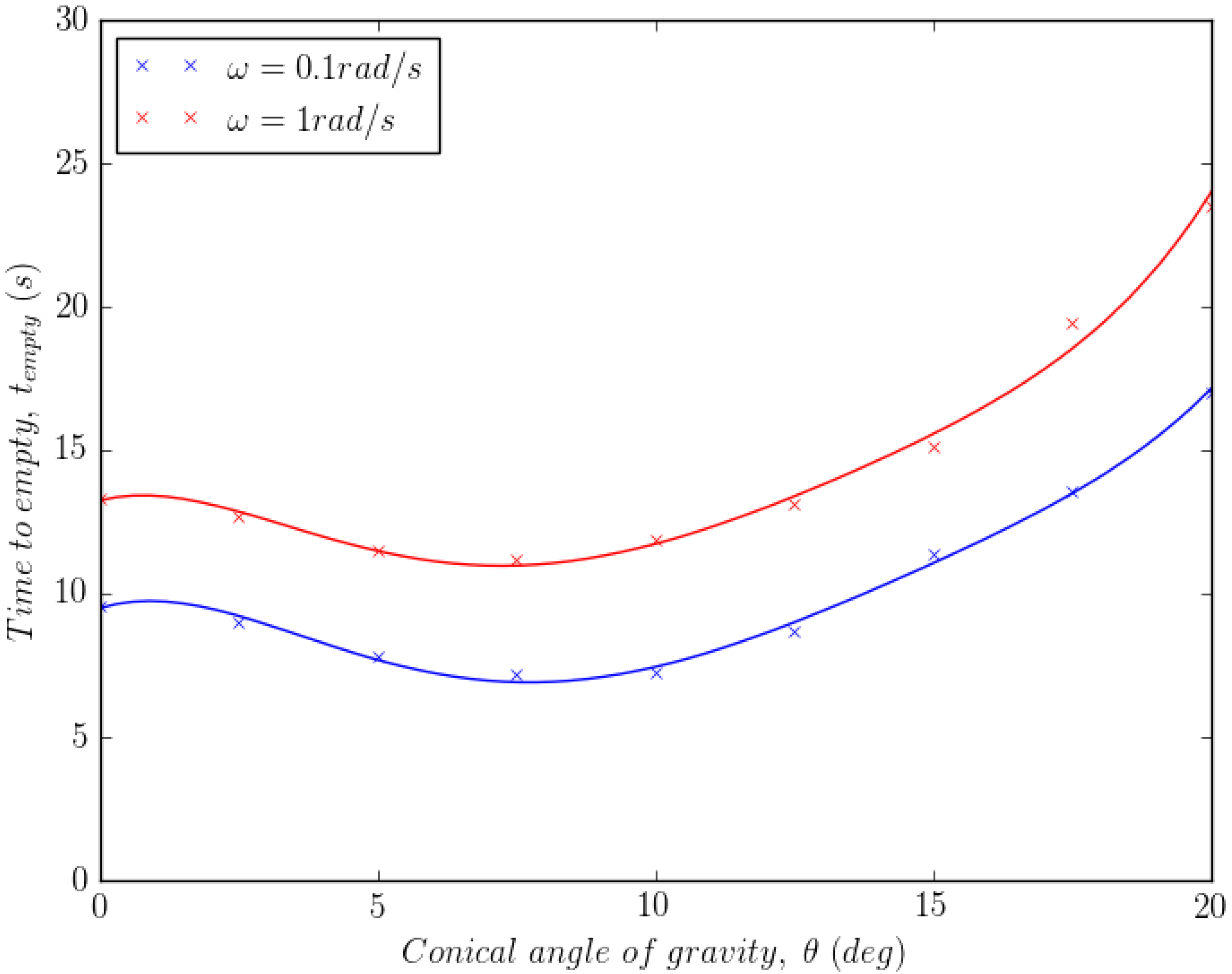
4. Modelling of a Complex Duct
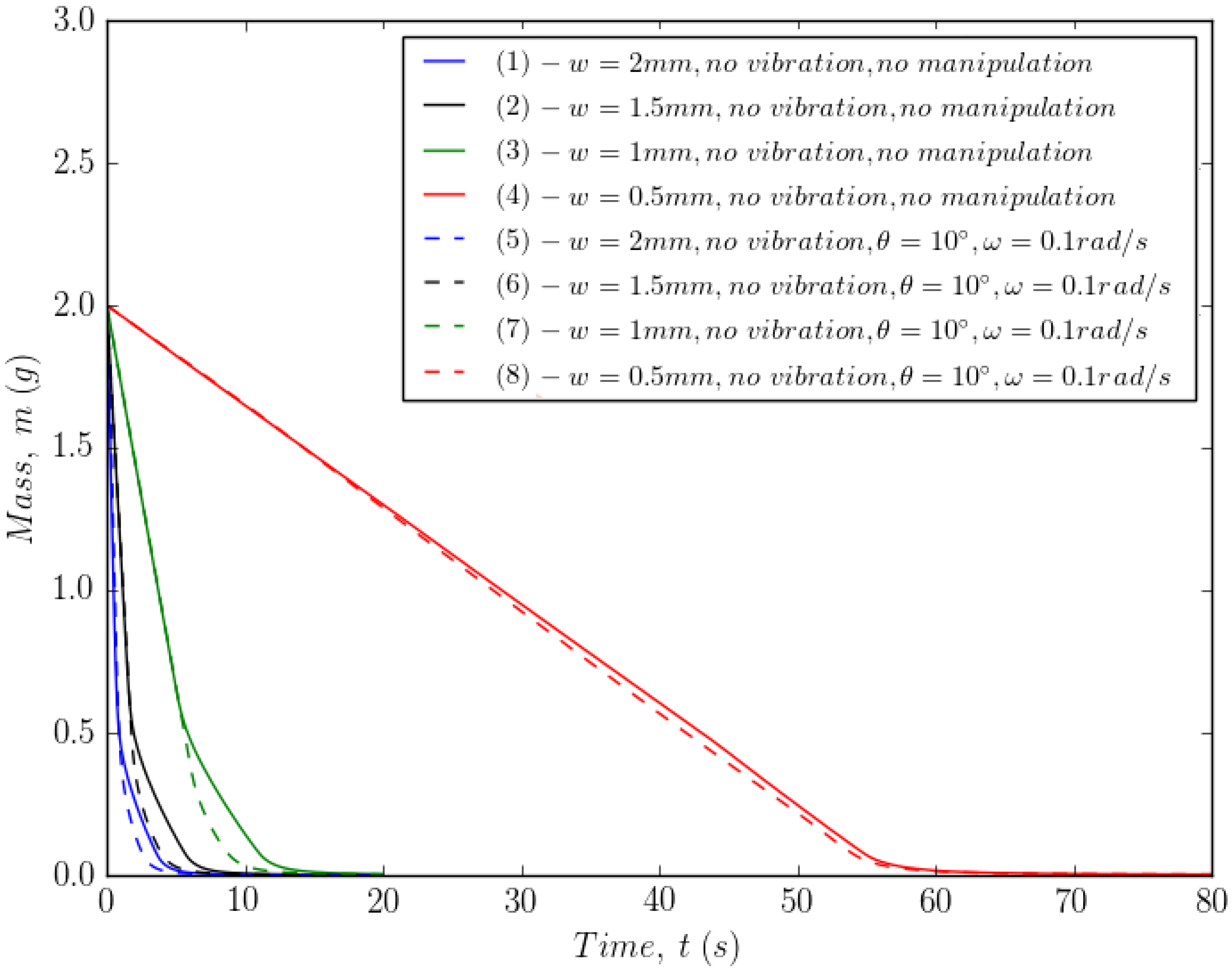
4.1. Test Case Description
4.2. Effect of Manipulation/Vibration
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| AM | Additive Manufacture |
| CCM | Computational Continuum Mechanics |
| CFD | Computational Fluid Dynamics |
| DEM | Discrete Element Model |
| EBM | Electron Beam Melting |
| FE | Finite Element |
| FEA | Finite Element Analysis |
| FV | Finite Volume |
| MDM | Molecular Dynamics Model |
| PEEK | Polyether ether ketone |
| PEK | Polyetherketone |
| PBF | Powder Bed Fusion |
| SLM | Selective Laser Melting |
References
- ISO. ISO/ASTM52921-13: Standard Terminology for Additive Manufacturing—Coordinate Systems and Test Methodologies; ISO Subcommittee F42.01; ASTM International: West Conshohocken, PA, USA, 2019. [Google Scholar]
- Brighenti, R.; Cosma, M.P.; Marsavina, L.; Spagnoli, A.; Terzano, M. Laser-based additively manufactured polymers: A review on processes and mechanical models. J. Mater. Sci. 2021, 56, 961–998. [Google Scholar] [CrossRef]
- Grossin, D.; Montón, A.; Navarrete-Segado, P.; Özmen, E.; Urruth, G.; Maury, F.; Maury, D.; Frances, C.; Tourbin, M.; Lenormand, P.; et al. A review of additive manufacturing of ceramics by powder bed selective laser processing (sintering/melting): Calcium phosphate, silicon carbide, zirconia, alumina, and their composites. Open Ceram. 2021, 5, 100073. [Google Scholar] [CrossRef]
- Frazier, W.E. Metal additive manufacturing: A review. J. Mater. Eng. Perform. 2014, 23, 1917–1928. [Google Scholar] [CrossRef]
- Renishaw Plc. Investigating the Effects of Multiple Re-Use of Ti6Al4V Powder in Additive Manufacturing (AM). 2016. Available online: https://manufacturing.report/whitepapers/investigating-the-effects-of-multiple-re-use-of-ti6al4v-powder-in-additive-manufacturing (accessed on 5 August 2021).
- Vayre, B.; Vignat, F.; Villeneuve, F. Identification on some design key parameters for additive manufacturing: Application on Electron Beam Melting. Procedia CIRP 2013, 7, 264–269. [Google Scholar] [CrossRef]
- Dalquist, S.; Gutowski, T. Life Cycle Analysis of Conventional Manufacturing Techniques: Sand Casting. Manuf. Eng. Mater. Handl. Eng. 2004, 2004, 631–641. [Google Scholar] [CrossRef]
- MB Associates. Best Practice Guide for Foundry Sector of India; Bureau of Energy Efficiency (BEE) and Institute for Industrial Productivity (IIP): Kendal, UK, 2012. Available online: http://www.iipinetwork.org/resources/best-practice-guide-for-indias-foundry-sector (accessed on 5 August 2021).
- Duru, P.; Nicolas, M.; Hinch, J.; Guazzelli, E. Constitutive laws in liquid-fluidized beds. J. Fluid Mech 2002, 452, 371–404. [Google Scholar] [CrossRef]
- Guo, Y.; Curtis, J.S. Discrete Element Method Simulations for Complex Granular Flows. Annu. Rev. Fluid Mech. 2015, 47, 21–46. [Google Scholar] [CrossRef]
- Goldhirsch, I. Rapid granular flows. Ann. Rev. Fluid Mech 2003, 35, 267–293. [Google Scholar] [CrossRef]
- Bagnold, R.A. Experiments on a Gravity-Free Dispersion of Large Solid Spheres in a Newtonian Fluid under Shear. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1954, 225, 49–63. [Google Scholar] [CrossRef]
- Campbell, C.S. Granular material flows—An overview. Powder Technol. 2006, 162, 208–229. [Google Scholar] [CrossRef]
- Popov, V.V., Jr.; Katz-Demyanetz, A.; Garkun, A.; Bamberger, M. The effect of powder recycling on the mechanical properties and microstructure of electron beam melted Ti-6Al-4V specimens. Addit. Manuf. 2018, 22, 834–843. [Google Scholar]
- Aranson, I.S.; Tsimring, L.S. Patterns and collective behavior in granular media: Theoretical concepts. Rev. Mod. Phys. 2006, 78, 641–692. [Google Scholar] [CrossRef]
- Michopoulos, J.G.; Iliopoulos, A.P.; Steuben, J.C.; Birnbaum, A.J.; Lambrakos, S.G. On the multiphysics modeling challenges for metal additive manufacturing processes. Addit. Manuf. 2018, 22, 784–799. [Google Scholar] [CrossRef]
- Drikakis, D.; Frank, M.; Tabor, G. Multiscale Computational Fluid Dynamics. Energies 2019, 12, 3272. [Google Scholar] [CrossRef]
- Radjai, F.; Roux, J.N.; Daouadji, A. Modeling Granular Materials: Century-Long Research across Scales. J. Eng. Mech. 2017, 143, 04017002. [Google Scholar] [CrossRef]
- Aranson, I. Granular Patterns; Oxford University Press: Oxford, UK, 2009. [Google Scholar]
- Durst, F.; Milojevic, F.; Schönung, B. Eulerian and lagrangian predicitons of particulate two-phase flows: A numerical study. Appl. Math. Model. 1984, 8, 101–115. [Google Scholar] [CrossRef]
- Da Cruz, F.; Emam, S.; Prochnow, M.; Roux, J.N.; Chevoir, F. Rheophysics of dense granular materials: Discrete simulation of plane shear flows. Phys. Rev. E Stat. Nonlinear Soft Matter Phys. 2005, 72, 1–17. [Google Scholar] [CrossRef]
- Rognon, P.G.; Roux, J.N.; Naaïm, M.; Chevoir, F. Dense flows of cohesive granular materials. J. Fluid Mech. 2008, 596, 21–47. [Google Scholar] [CrossRef]
- Jop, P.; Forterre, Y.; Pouliquen, O. A constitutive law for dense granular flows. Nature 2006, 441, 727–730. [Google Scholar] [CrossRef]
- Passalacqua, A.; Fox, R.O. Implementation of an iterative solution procedure for multi-fluid gas-particle flow models on unstructured grids. Powder Technol. 2011, 213, 174–187. [Google Scholar] [CrossRef]
- Srivastava, A.; Sundaresan, S. Analysis of a frictional-kinetic model for gas-particle flow. Powder Technol. 2003, 129, 72–85. [Google Scholar] [CrossRef]
- Zheng, Q.; Yu, A. Finite element investigation of the flow and stress patterns in conical hopper during discharge. Chem. Eng. Sci. 2015, 129, 49–57. [Google Scholar] [CrossRef]
- Liu, S.; Zhou, Z.; Zou, R.; Pinson, D.; Yu, A. Flow characteristics and discharge rate of ellipsoidal particles in a flat bottom hopper. Powder Technol. 2014, 253, 70–79. [Google Scholar] [CrossRef]
- Bharadwaj, R.; Khambekar, J.; Orlando, A.; Gao, Z.; Shen, H.; Helenbrook, B.; Royal, T.A.; Weitzman, P. A Comparison of Discrete Element Modeling, Finite Element Analysis, and Physical Experiment of Granular Material Systems in a Direct Shear Cell. AIP Conf. Proc. 2008, 969, 221–228. [Google Scholar] [CrossRef]
- Liu, Y.; Gonzalez, M.; Wassgren, C. Modeling granular material blending in a rotating drum using a finite element method and advection-diffusion equation multiscale model. AIChE J. 2018, 64, 3277–3292. [Google Scholar] [CrossRef]
- Chen, H.; Wei, Q.; Wen, S.; Li, Z.; Shi, Y. Flow behavior of powder particles in layering process of selective laser melting: Numerical modeling and experimental verification based on discrete element method. Int. J. Mach. Tools Manuf. 2017, 123, 146–159. [Google Scholar] [CrossRef]
- Fouda, Y.M.; Bayly, A.E. A DEM study of powder spreading in additive layer manufacturing. Granul. Matter 2020, 22, 10. [Google Scholar] [CrossRef]
- Haeri, S.; Wang, Y.; Ghita, O.; Sun, J. Discrete element simulation and experimental study of powder spreading process in additive manufacturing. Powder Technol. 2016, 306, 45–54. [Google Scholar] [CrossRef]
- Plimpton, S. Fast parallel algorithms for short-range molecular dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Johanson, J.R. A Rolling Theory for Granular Solids. J. Appl. Mech. 1965, 32, 842–848. [Google Scholar] [CrossRef]
- Dec, R.T.; Zavaliangos, A.; Cunningham, J.C. Comparison of various modeling methods for analysis of powder compaction in roller press. Powder Technol. 2003, 130, 265–271. [Google Scholar] [CrossRef]
- Shanjani, Y.; Toyserkani, E. Material spreading and compaction in powder-based solid freeform fabrication methods: Mathematical modeling. In Proceedings of the 19th Annual International Solid Freeform Fabrication Symposium, Austin, TX, USA, 4–8 August 2008; pp. 399–410. [Google Scholar]
- Desai, P.S.; Higgs, C.F. Spreading Process Maps for Powder-Bed Additive Manufacturing Derived from Physics Model-Based Machine Learning. Metals 2019, 9, 1176. [Google Scholar] [CrossRef]
- Martin, S.; Guessasma, M.; Léchelle, J.; Fortin, J.; Saleh, K.; Adenot, F. Simulation of sintering using a Non Smooth Discrete Element Method. Application to the study of rearrangement. Comput. Mater. Sci. 2014, 84, 31–39. [Google Scholar] [CrossRef]
- Martin, S.; Parekh, R.; Guessasma, M.; Léchelle, J.; Fortin, J.; Saleh, K. Study of the sintering kinetics of bimodal powders. A parametric DEM study. Powder Technol. 2015, 270, 637–645. [Google Scholar] [CrossRef]
- Xin, H.; Sun, W.; Fish, J. Discrete element simulations of powder-bed sintering-based additive manufacturing. Int. J. Mech. Sci. 2018, 149, 373–392. [Google Scholar] [CrossRef]
- Wei, H.; Mukherjee, T.; Zhang, W.; Zuback, J.; Knapp, G.; De, A.; DebRoy, T. Mechanistic models for additive manufacturing of metallic components. Prog. Mater. Sci. 2021, 116, 100703. [Google Scholar] [CrossRef]
- Steuben, J.C.; Iliopoulos, A.P.; Michopoulos, J.G. On Multiphysics Discrete Element Modeling of Powder-Based Additive Manufacturing Processes. In Proceedings of the ASME 2016 International Design Engineering Technical Conferences & Computers and Information in Engineering Conference, IDETC/CIE, Charlotte, NC, USA, 21–24 August 2016. [Google Scholar]
- Kloss, C.; Goniva, C.; Hager, A.; Amberger, S.; Pirker, S. Models, algorithms and validation for opensource DEM and CFD-DEM. Prog. Comput. Fluid Dyn. 2012, 12, 140–152. [Google Scholar] [CrossRef]
- Lee, W.H.; Zhang, Y.; Zhang, J. Discrete element modeling of powder flow and laser heating in direct metal laser sintering process. Powder Technol. 2017, 315, 300–308. [Google Scholar] [CrossRef]
- Ibarra-Medina, J.; Pinkerton, A. A numerical investigation of powder heating in coaxial laser metal deposition. In Proceedings of the 36th International MATADOR Conference; Springer: London, UK, 2010. [Google Scholar] [CrossRef]
- Pan, H.; Sparks, T.; Thakar, Y.D.; Liou, F. The Investigation of Gravity-Driven Metal Powder Flow in Coaxial Nozzle for Laser-Aided Direct Metal Deposition Process. J. Manuf. Sci. Eng. 2005, 128, 541–553. [Google Scholar] [CrossRef]
- Zeng, Q.; Tian, Y.; Xu, Z.; Qin, Y. Numerical modelling of the gas-powder flow during the laser metal deposition for additive manufacturing. In Proceedings of the 15 International Conference on Manufacturing Research, London, UK, 5–7 September 2017. [Google Scholar]
- Morimoto, K.; Suzuki, Y.; Kasagi, N. Mechanism of Heat Transfer Enhancement in Recuperators with Oblique Wavy Walls. Therm. Sci. Eng. 2004, 12, 99–100. [Google Scholar]
- Riella, M.; Kahraman, R.; Tabor, G. Reynolds-Averaged Two-Fluid Model prediction of moderately dilute fluid-particle flow over a backward-facing step. Int. J. Multiph. Flow 2018, 106, 95–108. [Google Scholar] [CrossRef]
- Riella, M.; Kahraman, R.; Tabor, G. Inhomogeneity and anisotropy in Eulerian–Eulerian near-wall modelling. Int. J. Multiph. Flow 2019, 114, 9–18. [Google Scholar] [CrossRef]
- Van Wachem, B.G.M. Derivation, Implementation, and Validation of Computer Simulation Models for Gas-Solid Fluidized Beds. Ph.D. Thesis, Delft University of Technology (TU Delft), Delft, The Netherlands, 2000. [Google Scholar]
- Geldart, D. Types of gas fluidization. Powder Technol. 1973, 7, 285–292. [Google Scholar] [CrossRef]
- ASTM B213-20. Standard Test Methods for Flow Rate of Metal Powders Using the Hall Flowmeter; ASTM International: West Conshohocken, PA, USA, 2020. [Google Scholar]
- Gidaspow, D.; Huilin, L. Equation of State and Radial Distribution Functions of FCC Particles in a CFB. AIChE J. 1998, 44, 279–293. [Google Scholar] [CrossRef]
- Van Wachem, B.; Sasic, S. Derivation, simulation and validation of a cohesive particle flow CFD model. AIChE J. 2008, 54, 9–19. [Google Scholar] [CrossRef]
- Ocone, R.; Goodwin, J.; Delebarre, A. Flow structures of Geldart A solids in circulating fluidized beds. Chem. Eng. Res. Des. 2000, 6, 860–865. [Google Scholar] [CrossRef]
- Makkawi, Y.T.; Wright, P.C.; Ocone, R. The effect of friction and inter particle cohesive forces on the hydrodynamics of gas solid flow. A comparative analysis of theoretical predictions and experiments. Powder Technol. 2006, 163, 69–79. [Google Scholar] [CrossRef]
- Vlachos, N.; Chang, I.T.H. Investigation of flow properties of metal powders from narrow particle size distribution to polydisperse mixtures through an improved Hall flowmeter. Powder Technol. 2011, 205, 71–80. [Google Scholar] [CrossRef]
- Kamenetskii, E.S.; Orlova, N.S.; Tagirov, A.M.; Volik, M.V. Three-Dimensional Simulation of a Vibrofluidized Bed with the Use of a Two-Fluid Model of Granular Gas. J. Eng. Phys. Thermophys. 2016, 89, 1459–1465. [Google Scholar] [CrossRef]
- Richman, M.W. Boundary conditions for granular flows at randomly fluctuating bumpy boundaries. Mech. Mater. 1993, 16, 211–218. [Google Scholar] [CrossRef]
- Viswanathan, H.; Sheikh, N.A.; Wildman, R.D.; Huntley, J.M. Convection in three-dimensional vibrofluidized granular beds. J. Fluid Mech. 2011, 682, 185–212. [Google Scholar] [CrossRef][Green Version]
- Orlova, N.S. Comparison of the results of experimental investigations of a vibrofluidized bed with caluclations by a granular gas hydrodynamic model. J. Eng. Phys. Thermophys. 2014, 87, 429–435. [Google Scholar] [CrossRef]
- Morimoto, K.; Suzuki, Y.; Kasagi, N. Optimal Shape Design of Compact Heat Exchangers based on Adjoint Analysis of Momentum and Heat Transfer. J. Therm. Sci. Technol. 2010, 5, 24–35. [Google Scholar] [CrossRef]
- Kahraman, R.; Bacheva, D.; Schmieder, A.; Tabor, G.R. Coupling of Volume of Fluid and Level Set Methods in Condensing Heat Transfer Simulations. Int. J. Comput. Fluid Dyn. 2019, 34, 25–38. [Google Scholar] [CrossRef]
- Gledhill, I.M.A.; Roohani, H.; Forsberg, K.; Eliasson, P.; Skews, B.W.; Nordström, J. Theoretical treatment of fluid flow for accelerating bodies. Theor. Comput. Fluid Dyn. 2016, 30, 449–467. [Google Scholar] [CrossRef]
- Matsusaka, S.; Yamamoto, K.; Masuda, H. Micro-feeding of a fine powder using a vibrating capillary tube. Adv. Powder Technol. 1996, 7, 141–151. [Google Scholar] [CrossRef]
- Kroll, V.W. Uber das Verhalien von Schüttgut in lotrecht schwingenden Gefessen. Forschung auf dem Gebiete des Ingenieurwesens 1954, 20, 2–15. [Google Scholar]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Roberts, A.; Kahraman, R.; Bacheva, D.; Tabor, G. Modelling of Powder Removal for Additive Manufacture Postprocessing. J. Manuf. Mater. Process. 2021, 5, 86. https://doi.org/10.3390/jmmp5030086
Roberts A, Kahraman R, Bacheva D, Tabor G. Modelling of Powder Removal for Additive Manufacture Postprocessing. Journal of Manufacturing and Materials Processing. 2021; 5(3):86. https://doi.org/10.3390/jmmp5030086
Chicago/Turabian StyleRoberts, Andrew, Recep Kahraman, Desi Bacheva, and Gavin Tabor. 2021. "Modelling of Powder Removal for Additive Manufacture Postprocessing" Journal of Manufacturing and Materials Processing 5, no. 3: 86. https://doi.org/10.3390/jmmp5030086
APA StyleRoberts, A., Kahraman, R., Bacheva, D., & Tabor, G. (2021). Modelling of Powder Removal for Additive Manufacture Postprocessing. Journal of Manufacturing and Materials Processing, 5(3), 86. https://doi.org/10.3390/jmmp5030086





