Influence of Alkali Metal Ions on the Structural and Spectroscopic Properties of Sm3+-Doped Silicate Glasses
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussions
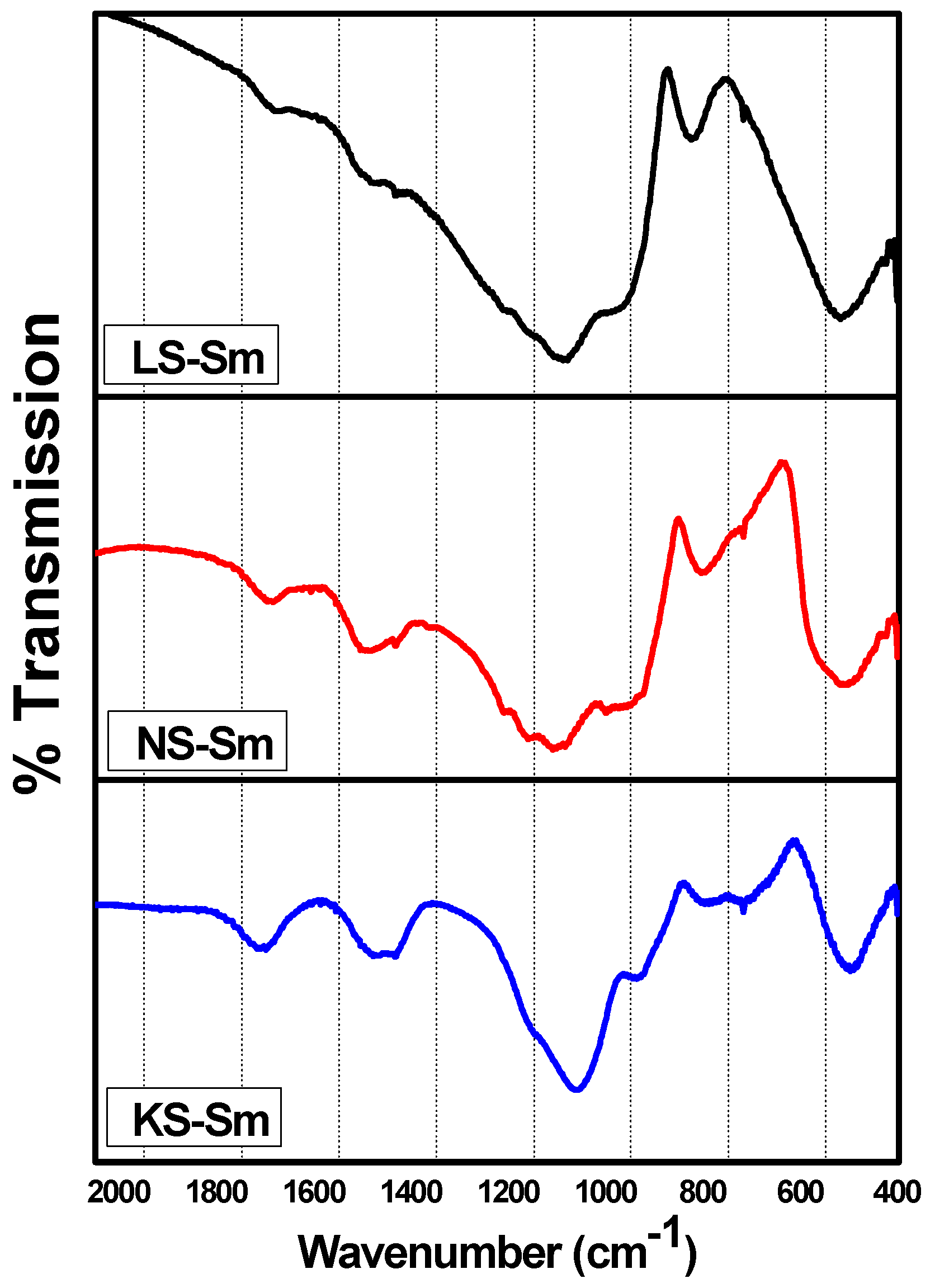
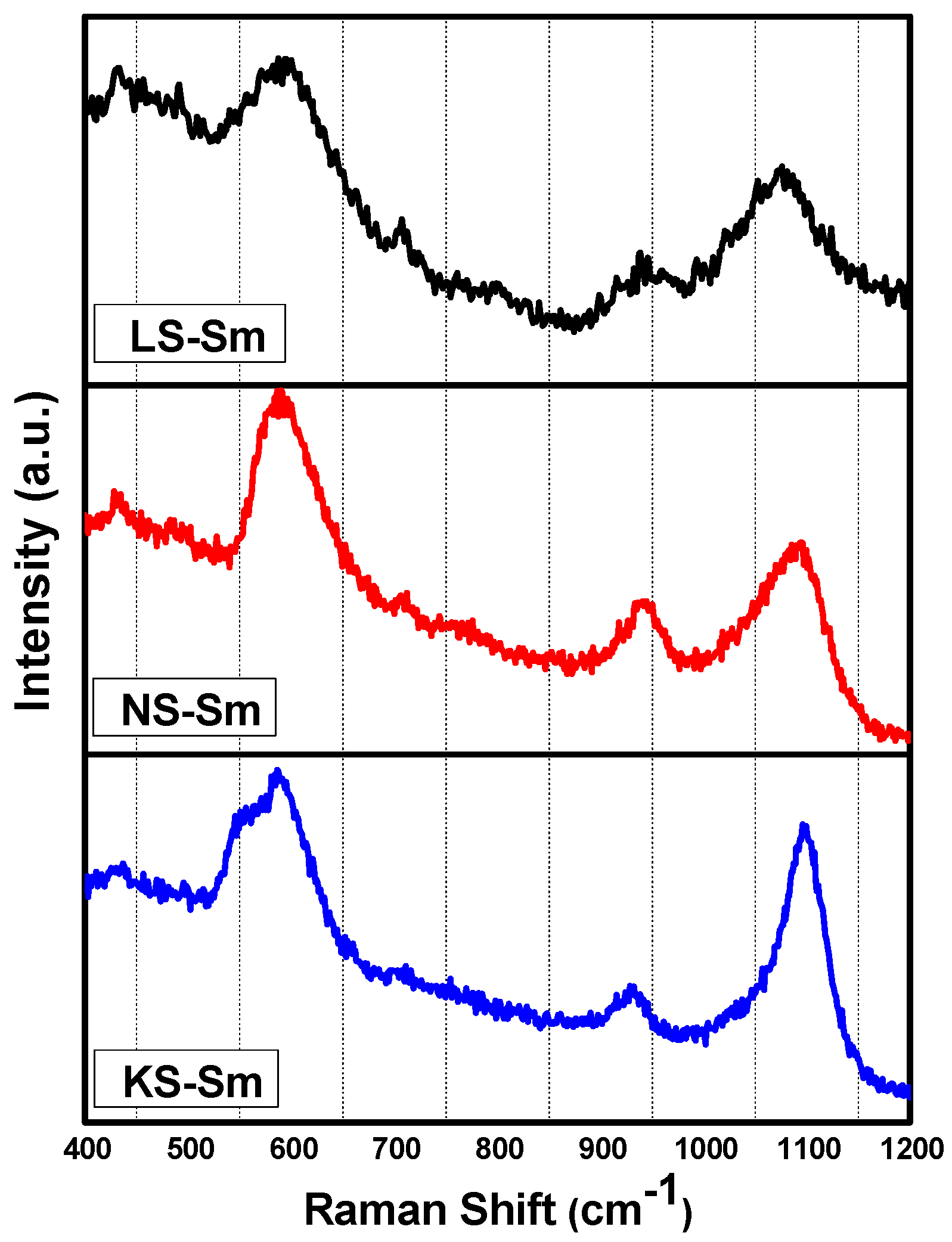
3.1. Structural Analysis
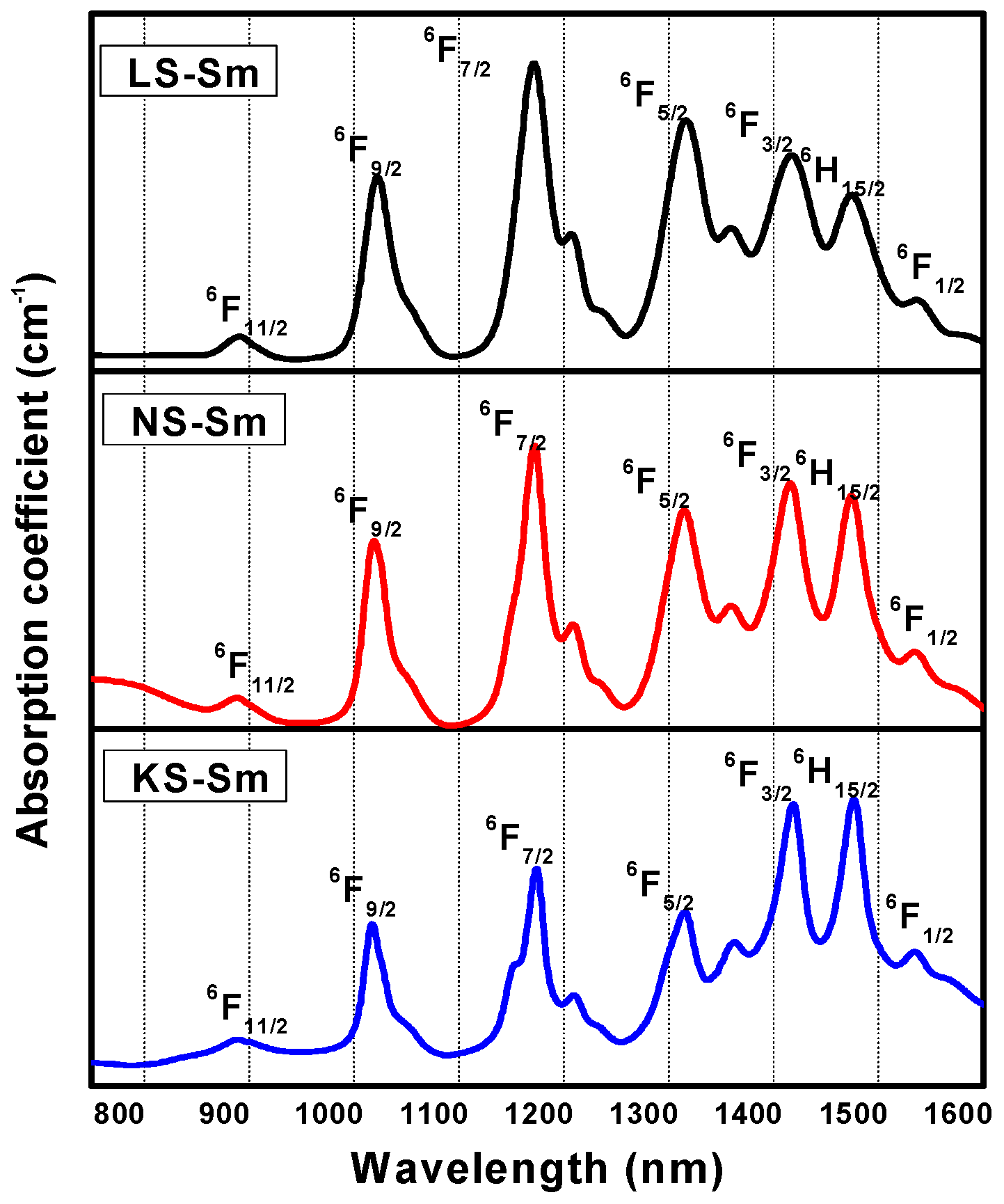
3.2. Optical Properties
| Host Matrix | Order | ||||
|---|---|---|---|---|---|
| LS-Sm3+ (present work) | 1.28 | 3.14 | 4.53 | 0.69 | |
| LCN borate [34] | 0.84 | 4.00 | 5.02 | 0.79 | |
| 30Li2O:70B2O3-Pr3+ [35] | 0.10 | 4.71 | 5.28 | 0.89 | |
| NS-Sm3+ (present work) | 1.27 | 2.69 | 3.15 | 0.85 | |
| NaZnBS-Sm3+ [27] | 0.55 | 9.68 | 9.77 | 0.99 | |
| 30Na2O:70B2O3-Pr3+ [35] | 0.98 | 4.76 | 4.86 | 0.97 | |
| KS-Sm3+ (present work) | 1.04 | 1.12 | 1.61 | 0.69 | |
| KZnBS-Sm3+ [27] | 0.18 | 11.37 | 11.45 | 0.99 | |
| LKG [11] | 0.63 | 4.05 | 4.69 | 0.86 |
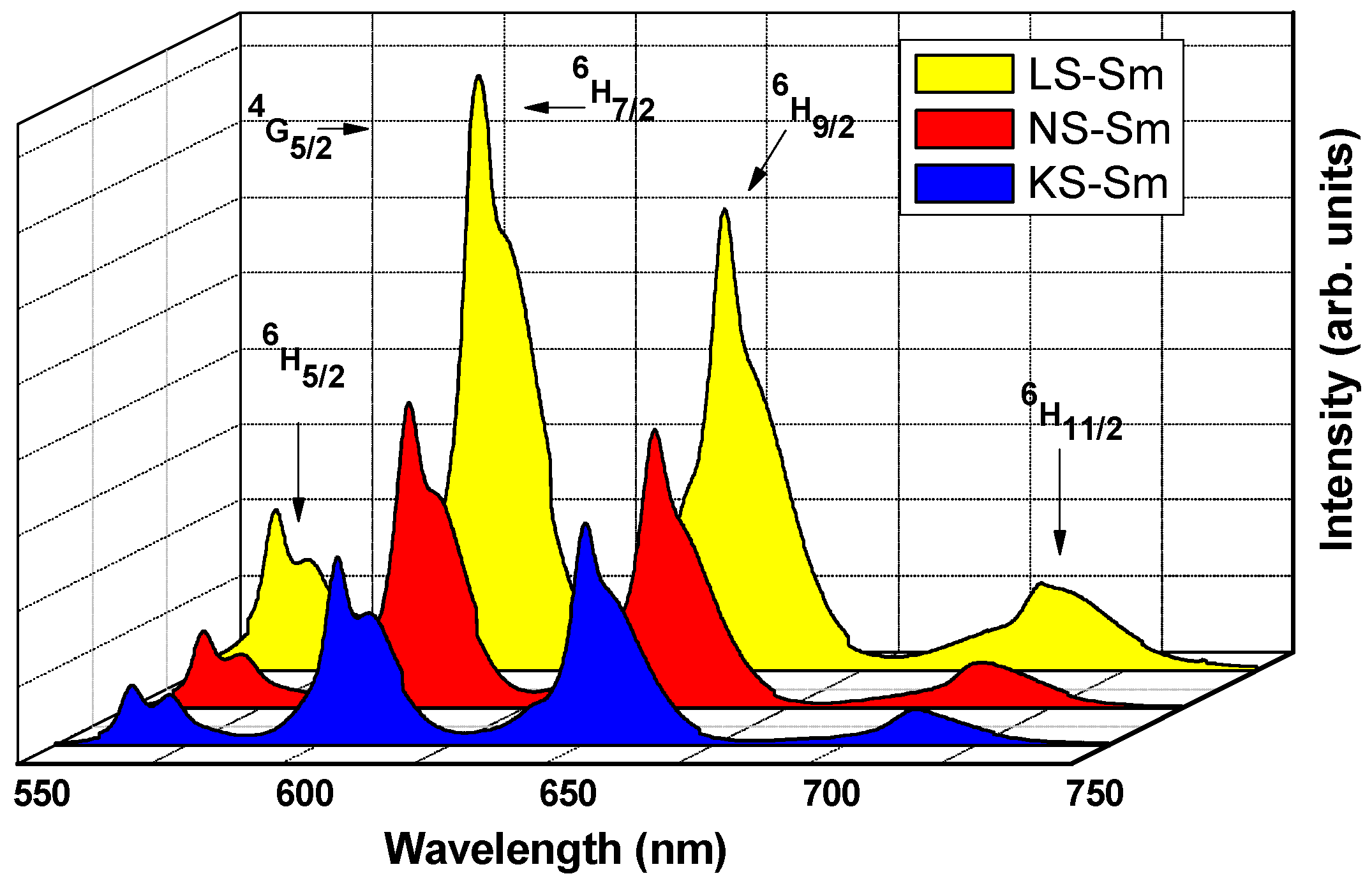
3.3. Emission Spectra and Radiative Properties
4. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Goodwin, D.W. Spectra and Energy Levels of Rare Earth Ions in Crystals. Phys. Bull. 1969, 20, 525. [Google Scholar] [CrossRef]
- Gatterer, K.; Pucker, G.; Jantscher, W.; Fritzer, H.P.; Arafa, A. Suitability of Nd (III) absorption spectroscopic to probe the structure of glasses from the ternary system Na2O-B2O3-SiO2. J. Non-Cryst. Solids 1998, 231, 189–199. [Google Scholar] [CrossRef]
- Sailaja, B.; Joyce Stella, R.; Thitumala Rao, G.; Jaya Raja, B.; Pushpa Manjari, V.; Ravikumar, R.V.S.S.N. Physical, structural and spectroscopic investigations of Sm3+ doped ZnO mixed alkali borate glass. J. Mol. Struct. 2015, 1096, 129–235. [Google Scholar] [CrossRef]
- Cases, R.; Chamarro, M.A. Judd-Ofelt analysis and multiphonon relaxations of rare earth ions in fluorohafnate glasses. J. Solid State Chem. 1991, 90, 313. [Google Scholar] [CrossRef]
- Basiev, T.; Dergachev, A.Y.; Orlavskii, Y.V.; Prohkorov, A.M. Multiphonon nonradiative relaxation from high-lying levels of Nd3+ ions in flouride and oxide laser materials. J. Lumin. 1992, 53, 19. [Google Scholar] [CrossRef]
- Broer, M.M.; Bruce, A.J.; Grodkiewicz, W.H. Resonantly induced refractive index changes in Eu3+-and Er3-doped silicate and phosphate glasses. J. Lumin. 1992, 53, 15. [Google Scholar] [CrossRef]
- Reisfeld, R.; Bornstein, A.; Boehm, L. Optical characteristics and intensity parameters of Sm3+ in GeO2, ternary germanate, and borate glasses. J. Solid State Chem. 1975, 14, 14. [Google Scholar] [CrossRef]
- Rodriguez, V.D.; Martin, I.R.; Alcala, R.; Cases, R. Optical properties and cross relaxation among Sm3+ ions in fluorzincate glasses. J. Lumin. 1992, 54, 231. [Google Scholar] [CrossRef]
- Ratnakaram, Y.C.; Thirupathi Naidu, D.; Vijaya Kumar, A.; Gopal, N.O. Influence of mixed alkalies on absorption and emission properties of Sm3+ ions in borate glasses. Phys. B 2005, 358, 296–307. [Google Scholar] [CrossRef]
- Annapurna, K.; Dwivedi, R.N.; Kumar, A.; Chaudhuri, A.K.; Buddhudu, S. Temperature dependent luminescence characteristic of Sm3+-doped silicate glass. Spectrochim. Acta A Mol. Biomol. Spectrosc. 2000, 56, 103–109. [Google Scholar] [CrossRef]
- Jayasimhadri, M.; Chon, E.-J.; Jang, K.-W.; Lee, H.S.; Kim, S. Spectroscopic properties and Judd-Ofelt analysis of Sm3+ doped lead-germanate-tellurite glasses. J. Phys. D Appl. Phys. 2008, 41, 175101. [Google Scholar] [CrossRef]
- Sharma, Y.K.; Surana, S.S.L.; Singh, R.K. Spectroscopic investigation and luminescence spectra of soda lime silicate glasses. J. Rare Earths 2009, 27, 773. [Google Scholar] [CrossRef]
- Basavapoornima, C.; Jayasankar, C.K. Spectroscopic and photoluminescence properties of Sm3+ ions in Pb-K-Al-Na phosphate glasses for efficient visible lasers. J. Lumin. 2014, 153, 233–241. [Google Scholar] [CrossRef]
- Isard, J.O. The mixed alkali effect in glass. J. Non-Cryst. Solids 1969, 1, 235–261. [Google Scholar] [CrossRef]
- Day, D.E. Mixed alkali glasses—Their properties and uses. J Non-Cryst. Solids 1976, 21, 343–372. [Google Scholar] [CrossRef]
- Kracek, F.C. The ternary system K2SiO3-Na2SiO3-SiO2. J. Phys. Chem. 1932, 36, 2529–2542. [Google Scholar] [CrossRef]
- Selvi, S.; Marimuthu, K.; Muralidharan, G. Structural and luminescence behavior of Sm3+ ions doped lead boro-telluro-phosphate glasses. J. Lumin. 2015, 159, 207–218. [Google Scholar] [CrossRef]
- Muralidharan, M.N.; Rasmitha, C.A.; Rateesh, R. Photoluminescence and FTIR studies of pure and rare earth doped silica xerogels and aerogels. J. Porous Mater. 2009, 16, 635–640. [Google Scholar] [CrossRef]
- Sanders, D.M.; Person, W.B.; Hench, L.L. Quantitative-analysis of glass structure with the use of infrared reflection spectra. Appl. Spectrosc. 1974, 28, 247–255. [Google Scholar] [CrossRef]
- Matson, D.W.; Sharma, S.K.; Philpotts, J.A. The structure of high-silica alkali-silicate glasses—A Raman spectroscopy investigation. J. Non-Crystal. Solids 1983, 58, 323–352. [Google Scholar] [CrossRef]
- El-Okr, M.; Ibrahem, M.; Farouk, M. Structure and properties of rare-earth-doped glassy systems. J. Non-Cryst. Solids 2008, 69, 2564–2567. [Google Scholar] [CrossRef]
- Srinvasa Rao, C.; Srikumar, T.; Rao, M.C. Physical and optical absorption studies on LIF/NaF/KF- P2O5-B2O3 glasses doped with Sm2O3. IJCRGG 2014, 7, 420–425. [Google Scholar]
- Bhatia, B.; Meena, S.L.; Parihar, V.; Poonia, M. Optical basicity and polarizability of Nd3+-doped bismuth borate glasses. New J. Glass Ceram. 2015, 5, 44–52. [Google Scholar] [CrossRef]
- Judd, B.R. Optical absorption intensities of rare earth ions. Phys. Rev. 1962, 127, 750–761. [Google Scholar] [CrossRef]
- Ofelt, G.S. Intensities of crystal spectra of rare earth ions. J. Chem. Phys. 1962, 37, 511–520. [Google Scholar] [CrossRef]
- Jayasimhadri, M.; Moorthy, L.R.; Saleem, S.A.; Ravikumar, R.V.S.S. Spectroscopic characteristics of Sm3+-doped alkali fluorophosphate glasses. Spectrochim. Acta Part A 2006, 64, 939–944. [Google Scholar] [CrossRef]
- Jayasankar, C.K.; Rukmini, E. Optical properties of Sm3+ ions in zinc and alkali zinc borosulphate glasses. Opt. Mater. 1997, 8, 193–205. [Google Scholar] [CrossRef]
- Chen, B.J.; Shen, L.F.; Pun, E.Y.B.; Lin, H. Sm3+-doped germanate glass channel waveguide as light source for minimally invasive photodynamic therapy surgery. Opt. Express 2012, 20, 879–889. [Google Scholar] [CrossRef]
- Jorgenson, C.K. Orbitals Atoms and Molecules; Academic Press: London, UK, 1962. [Google Scholar]
- Sinha, S.P. Complexes of the Rare Earth; Pergamon Press: Oxford, UK, 1966. [Google Scholar]
- Carnall, W.T.; Crosswhite, H.; Crosswhite, H.M. Energy Level Structure and Transition Probabilities of the Trivalent Lanthanides in LaF3; Report ANL–78–XX–95; Argonne National Laboratory: Lemont, IL, USA, 1978. [Google Scholar]
- Sudhakar, K.S.V.; Srinivasa Reddy, M.; Srinivasa Rao, L.; Veeraiah, N. Influence of modifier oxide on spectroscopic and thermoluminescence characteristics of Sm3+ion in antimony borate glass system. J. Lumin. 2008, 128, 1791–1798. [Google Scholar] [CrossRef]
- Rajesh, D.; Balakrishna, A.; Ratnakaram, Y.C. Luminescence, structural and dielectric properties of Sm3+ impurities in strontium lithium bismuth borate glasses. Opt. Mater. 2012, 35, 108–116. [Google Scholar] [CrossRef]
- Ratnakaram, Y.C.; Thirupathi Naidu, D.; Chakradhar, R.P.S. Spectral studies of Sm3+ and Dy3+ doped lithium cesium mixed alkali borate glasses. J. Non-Cryst. Solids 2006, 353, 3914–3922. [Google Scholar] [CrossRef]
- Takebe, H.; Nageno, Y.; Morinaga, K. Compositional dependence of Judd-Ofelt parameters in silicate, borate, and phosphate glasses. J. Am. Ceram. Sot. 1995, 78, 1161–1168. [Google Scholar] [CrossRef]
- Shanmuga Sundari, S.; Marimuthu, K.; Sivraman, M.; Surendra Babu, S. Composition dependent structural and optical properties of Sm3+-doped sodium borate and sodium fluoroborate glasses. J. Lumin. 2010, 130, 1313–1319. [Google Scholar] [CrossRef]
- Arul Rayappan, I.; Selvaraju, K.; Marimuthu, K. Structural and luminescence investigations on Sm3+ doped sodium fluoroborate glasses containing alkali/alkaline earth metal oxides. Phys. B 2011, 406, 548–555. [Google Scholar] [CrossRef]
- Herrera, A.; Fernandes, R.G.; de Camargo, A.S.S.; Hernandes, A.C.; Buchner, S.; Jacinto, C.; Balzaretti, N.M. Visible–NIR emission and structural properties of Sm3+ doped heavy-metal oxide glass with composition B2O3–PbO–Bi2O3–GeO2. J. Lumin. 2016, 171, 106–111. [Google Scholar] [CrossRef]
- Montoya, I.M.; Balzaretti, N.M. High pressure effect on structural and spectroscopic properties of Sm3+ doped alkali silicate glasses. High-Press. Res. 2017, 37, 296–311. [Google Scholar] [CrossRef]

| Sample Notation | Glass Compositions (mol%) | Glass Appearances | Melting Temperatures (°C) |
|---|---|---|---|
| LS-Sm | 33.0 Li2O + 66.0 SiO2 + 1.0 Sm2O3 | Transparent glass | 1550 |
| NS-Sm | 33.0 Na2O + 66.0 SiO2 + 1.0 Sm2O3 | Transparent glass | 1550 |
| KS-Sm | 33.0 K2O + 66.0 SiO2 + 1.0 Sm2O3 | Transparent glass | 1550 |
| Vibration Mode | LS-Sm Wavenumber (cm−1) | NS-Sm Wavenumber (cm−1) | KS-Sm Wavenumber (cm−1) |
|---|---|---|---|
| Si–O–Si bending vibration | 472 | 468 | 447 |
| O–Si–O bending vibration | 774 | 752 | 745 |
| Si–O–Si symmetric stretching | 916 | 891 | 884 |
| Si–O–Si asymmetric stretching | 1037 | 1060 | 1013 |
| Vibration mode | LS-Sm Wavenumber (cm−1) | NS-Sm Wavenumber (cm−1) | KS-Sm Wavenumber (cm−1) |
|---|---|---|---|
| Si–O | 591 | 589 | 587 |
| Si–O–Si symmetric stretching | 939 | 942 | 930 |
| Si–O–Si antisymmetric stretching | 1073 | 1094 | 1097 |
| Physical Properties | LS-Sm | NS-Sm | KS-Sm |
|---|---|---|---|
| Density, ρ (gm/cm3) (± 0.0005) | 2.4552 | 2.5396 | 2.5598 |
| Refractive index (n) (585 nm) | 1.4930 | 1.4490 | 1.4310 |
| Concentration of Sm3+, N (×1020 ions/cm3) | 2.1896 | 1.8854 | 1.7370 |
| Molar volume (Vm) (cm3/mol) | 27.5024 | 31.9404 | 34.6697 |
| Polaron radius, rp (Å) | 6.6846 | 7.0264 | 7.2211 |
| Interionic distance, ri (nm) | 1.6591 | 1.7439 | 1.7923 |
| Field strength, F (×1016 cm−2) | 6.7139 | 6.0766 | 5.7533 |
| Reflection losses (RL) (×10−2) | 3.9107 | 3.3614 | 3.1433 |
| Molar refraction (Rm) (cm3/mol) | 7.9928 | 8.5671 | 8.9742 |
| Optical dielectric constant (P) | 1.2290 | 1.0996 | 1.0478 |
| Dielectric constant (ε) | 2.2290 | 2.0996 | 2.0478 |
| Molar polarizability (αm) × 10−22 cm3 | 3.1687 | 3.3964 | 3.5578 |
| Transitions 6H5/2 | LS-Sm | NS-Sm | KS-Sm |
|---|---|---|---|
| 6F11/2 | 10,626.99 | 10,655.73 | 10,672.36 |
| 6F9/2 | 9325.81 | 9353.91 | 9369.21 |
| 6F7/2 | 8185.95 | 8183.33 | 8169.19 |
| 6F5/2 | 7316.94 | 7325.95 | 7322.03 |
| 6F3/2 | 6815.18 | 6821.16 | 6806.60 |
| 6H15/2 | 6558.67 | 6560.53 | 6549.88 |
| 6F1/2 | 6288.49 | 6306.04 | 6309.73 |
| 1.01436 | 1.01569 | 1.01573 | |
| −0.01416 | −0.01544 | −0.01548 |
| Transitions 6H5/2 | LS-Sm | NS-Sm | KS-Sm | |||
|---|---|---|---|---|---|---|
| 6F11/2 | 0.602 | 0.568 | 0.363 | 0.407 | 0.309 | 0.205 |
| 6F9/2 | 4.720 | 3.440 | 3.390 | 2.470 | 1.640 | 1.230 |
| 6F7/2 | 3.490 | 4.660 | 2.490 | 3.33 | 1.220 | 1.610 |
| 6F5/2 | 2.360 | 1.890 | 1.630 | 1.370 | 0.648 | 0.595 |
| 6F3/2 | 1.210 | 1.060 | 1.170 | 0.874 | 0.760 | 0.475 |
| 6H15/2 + 6F1/2 | 0.897 | 0.326 | 1.091 | 0.383 | 0.806 | 0.307 |
| 0.9856 | 0.8310 | 0.5036 | ||||
| Transitions from 4G5/2 to | LS-Sm | NS-Sm | KS-Sm | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 6H5/2 | 17,740 | 45.40 | 0.1812 | 3.99 | 17,731 | 36.28 | 0.1951 | 5.37 | 17,728 | 17.19 | 0.1906 | 11.08 |
| 6H7/2 | 16,608 | 110.618 | 0.4415 | 16,584 | 76.318 | 0.4104 | 16,568 | 34.372 | 0.3808 | |||
| 6H9/2 | 15,407 | 58.314 | 0.2327 | 15,408 | 46.000 | 0.2473 | 15,369 | 25.510 | 0.2827 | |||
| 6H11/2 | 14,101 | 26.256 | 0.1048 | 14,065 | 18.945 | 0.1019 | 14,025 | 8.074 | 0.0895 | |||
| 6F3/2 | 10,890 | 0.800 | 0.0032 | 10,905 | 0.705 | 0.0038 | 10,834 | 0.502 | 0.0056 | |||
| 6F5/2 | 10,424 | 5.925 | 0.0236 | 10,395 | 5.257 | 0.0283 | 10,373 | 3.405 | 0.0377 | |||
| 6F7/2 | 9578 | 1.965 | 0.0078 | 9606 | 1.505 | 0.0081 | 9533 | 0.606 | 0.0067 | |||
| 6F9/2 | 8324 | 0.860 | 0.0034 | 8326 | 0.701 | 0.0038 | 8321 | 0.458 | 0.0051 | |||
| 6F11/2 | 7686 | 0.419 | 0.0017 | 7669 | 0.271 | 0.0015 | 7645 | 0.129 | 0.0014 | |||
| Transitions from 4G5/2 to | LS-Sm | NS-Sm | KS-Sm | ||||||
|---|---|---|---|---|---|---|---|---|---|
| 6H5/2 | 564 | 10.14 | 2.69 | 564 | 11.01 | 2.11 | 565 | 12.28 | 0.92 |
| 6H7/2 | 602 | 14.16 | 6.14 | 603 | 12.82 | 4.97 | 604 | 12.68 | 2.31 |
| 6H9/2 | 649 | 14.76 | 4.17 | 650 | 14.83 | 3.49 | 651 | 13.73 | 2.16 |
| 6H11/2 | 710 | 22.91 | 1.73 | 711 | 21.63 | 1.42 | 713 | 18.75 | 0.72 |
| 6F3/2 | 919 | 19.03 | 0.18 | 919 | 17.74 | 0.18 | 921 | 19.60 | 0.12 |
| 6F5/2 | 959 | 27.37 | 1.09 | 962 | 28.36 | 1.01 | 964 | 22.92 | 0.83 |
| 6F7/2 | 1044 | 27.25 | 0.51 | 1043 | 32.19 | 0.35 | 1052 | 24.68 | 0.19 |
| 6F9/2 | 1199 | 70.61 | 0.15 | 1201 | 60.99 | 0.15 | 1204 | 61.82 | 0.10 |
| 6F11/2 | 1301 | 39.59 | 0.18 | 1309 | 33.35 | 0.15 | 1307 | 41.09 | 0.06 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Matos, I.R.M. Influence of Alkali Metal Ions on the Structural and Spectroscopic Properties of Sm3+-Doped Silicate Glasses. Ceramics 2023, 6, 1788-1798. https://doi.org/10.3390/ceramics6030109
Matos IRM. Influence of Alkali Metal Ions on the Structural and Spectroscopic Properties of Sm3+-Doped Silicate Glasses. Ceramics. 2023; 6(3):1788-1798. https://doi.org/10.3390/ceramics6030109
Chicago/Turabian StyleMatos, Israel R. Montoya. 2023. "Influence of Alkali Metal Ions on the Structural and Spectroscopic Properties of Sm3+-Doped Silicate Glasses" Ceramics 6, no. 3: 1788-1798. https://doi.org/10.3390/ceramics6030109
APA StyleMatos, I. R. M. (2023). Influence of Alkali Metal Ions on the Structural and Spectroscopic Properties of Sm3+-Doped Silicate Glasses. Ceramics, 6(3), 1788-1798. https://doi.org/10.3390/ceramics6030109









