Numerical Study on the Effect of Tunnel Slope on Smoke Exhaust Performance in Metro Tunnels

Abstract

Utilizing the intermediate air shaft for smoke exhaust is one of the crucial emergency ventilation methods in metro tunnel fires. To study the impact of metro tunnel slope on smoke exhaust performance of intermediate air shaft, this paper employs numerical simulation to conduct research from the following aspects: the longitudinal distribution of ceiling smoke temperature, visibility distribution, smoke layer height, and the smoke exhaust efficiency of intermediate air shaft. The results demonstrate that as the tunnel slope increases, the maximum ceiling temperature decreases, and the visibility at dangerous height increases. The smoke layer height on the downhill side of a sloped tunnel is higher than that of a horizontal tunnel, while the smoke layer height on the uphill side is lower. Under single-side smoke exhaust mode, the smoke exhaust efficiency of the 2# intermediate air shaft rises as the tunnel slope increases. However, under air supply plus smoke exhaust mode, the smoke exhaust efficiency of the 2# intermediate air shaft decreases with the growing tunnel slope.

1. Introduction

At present, metro systems have emerged as essential lifeline projects in modern cities [1,2]. Owing to the impact of topographic and geological conditions, certain metro lines exhibit a specific slope in their design [3,4]. In the event of sloped tunnel fires, the stack effect causes fire smoke to tend to spread towards uphill side [5]. Consequently, the smoke spread characteristics in sloped tunnels fires are notably different from those in horizontal tunnels.

Previous scholars have conducted a series of experimental and numerical simulation studies on sloped tunnel fires. For instance, Yan et al. [6] carried out six full-scale experiments on sloped tunnel fires and found that due to the influence of stack effect, the smoke spreading distance on uphill side is significantly larger than that on downhill side. Li et al. [7] investigated the influence of tunnel slope on smoke spreading characteristics through small-scale model experiments and numerical simulations. Hu et al. [8] studied the maximum ceiling temperature and longitudinal distribution of smoke temperature under different tunnel slopes based on small-scale model experiments and established a prediction model for the distribution characteristics of fire smoke temperature with considering the influence of tunnel slope. Wan et al. [9] explored the relationship between smoke back-layering length and tunnel slope and found that the smoke back-layering length is independent of heat release rate and fire source location. Moreover, the smoke back-layering length decreases as the tunnel slope increases. Thus, a prediction model of smoke back-layering length considering the influence of tunnel slope has been established. Yang et al. [10,11] investigated the fire smoke spreading characteristics of sloped tunnels when vehicles are blocked through full-scale experiments and small-scale model experiments. The temperature distribution characteristics and smoke stratification characteristics were analyzed. Zhong et al. [12] employed small-scale model experiments and numerical simulation methods. Considering the influence of train fire location, fan operation mode, and tunnel section dimension, they studied the smoke control effect of the ventilation and smoke extraction linkage mode under different fire scenarios in sloped tunnels. Yang et al. [13] investigated the influence of stack effect on smoke spreading behavior by conducting saltwater experiments and discussed the specific relationship between tunnel slope and critical control conditions of smoke back-layering length. Wang et al. [14] and Jiang et al. [15] utilized numerical simulation to study the smoke spreading characteristics of tunnels with complex slope structures, such as V-shaped tunnels and herringbone tunnels.

It can be found that the research on sloped tunnel fires mainly concentrates on the characteristics of smoke spreading and temperature distribution. However, there is a dearth of research on the influence of tunnel slope on the smoke exhaust performance of intermediate air shaft, especially in the research on fire area risk parameters, such as visibility and smoke layer height. These parameters are crucial for the evacuation of people in tunnel fire scenarios. As an important means of emergency ventilation in metro tunnel fires, the smoke control performance of intermediate air shaft directly impacts the evacuation and rescue environment of tunnel fires. Therefore, this paper systematically investigates the smoke exhaust performance of intermediate air shaft in sloped tunnels. The conclusions can provide theoretical and technical support for the emergency disposal of sloped metro tunnel fires.

2. Numerical Simulation

2.1. Introduction of Software

Due to the enormous cost of manpower and material resources required for fire experiments and the certain degree of danger associated with fire experiments, while numerical simulation is a more flexible and operable approach with the characteristics of environmental safety and low cost, it is also easy to simulate various fire scenarios. Therefore, this paper employs numerical simulation for research. The simulation software utilized is Fire Dynamics Simulator (version 6.7.1). The accuracy of this software in building fire simulation has been verified in the research of Guo et al. [16] and Weng et al. [17]. The main control equations followed in the calculation and solution are as follows:

Mass conservation equation:

$${\displaystyle \frac{\partial \rho }{\partial t}}+\nabla \cdot \rho u=0.$$

(1)

Momentum conservation equation:

$$\rho ({\displaystyle \frac{\partial u}{\partial t}}+(\mathit{u}\cdot \nabla )\mathit{u})+\nabla p=\rho g+f+\nabla \cdot \tau .$$

(2)

Energy conservation equation:

$${\displaystyle \frac{\partial }{\partial \mathrm{t}}}(\rho h)+\nabla \cdot \rho \mathit{hu}={\displaystyle \frac{Dp}{Dt}} - \nabla \cdot q_r+\nabla \cdot k\nabla T+\sum _l\nabla \cdot h_l\rho K_l\nabla Y_l.$$

(3)

Component conservation equation:

$${\displaystyle \frac{\partial }{\partial t}}(\rho Y_l)+\nabla \cdot Y_{l}u=\nabla \cdot \rho K_l\nabla Y_l+{\dot{m}\prime \prime \prime }_l.$$

(4)

State equation:

$$p_0=\rho \mathit{TR}{\sum }_i({\displaystyle \frac{Y_i}{M_i}})=\rho \mathrm{TR}/M,$$

(5)

where ρ is density; t is time; u is velocity vector; g is gravitational acceleration; f is vector of external applied force; τ is viscous force tensor; p is pressure; h is enthalpy; q_r is heat radiation flux; T is temperature; subscript l represents the component l; Y_l is concentration of the component l. ${\dot{m}\prime \prime \prime }_l$ is mass generation rate of the component l in unit space; K_l is the diffusion coefficient of component l; R is gas constant; M is molecular mass of the mixed gas; and subscript i represents the component i.

The turbulence model adopts Smagorinsky large eddy model LES, and the turbulence viscosity coefficient can be expressed as follows:

$${\mu }_{\mathrm{LES}}=\rho {(C_s\Delta )}^2{[2(\mathit{defu})\cdot (\mathit{defu}) - {\displaystyle \frac{2}{3}}{(\nabla \cdot u)}^2]}^{{\displaystyle \frac{1}{2}}},$$

(6)

where C_s is the empirical coefficient and Δ is the mesh characteristic scale.

The relationship among thermal diffusion, material diffusion and turbulent viscosity coefficient is as follows:

$$k_{\mathrm{LES}}={\displaystyle \frac{{\mu }_{\mathrm{LES}}{C}_{\mathrm{p}}}{{\mathrm{P}}_{\mathrm{r}}}},$$

(7)

$${(\rho K)}_{l, \mathrm{LES}}={\displaystyle \frac{{\mu }_{\mathrm{LES}}}{{\mathrm{S}}_{\mathrm{c}}}},$$

(8)

where P_r is the Prandtl number and S_c is the Schmidt number.

The combustion model adopts the mixed fraction model, which is defined as follows:

$$Z={\displaystyle \frac{s{Y}_{\mathrm{F}} - (Y_{\mathrm{O}}- Y_{\mathrm{O}}^{\infty })}{s{Y}_{\mathrm{F}}^{\mathrm{I}}+Y_{\mathrm{O}}^{\infty }}},$$

(9)

$$s={\displaystyle \frac{v_{\mathrm{O}}{M}_{\mathrm{O}}}{v_{\mathrm{F}}{M}_{\mathrm{F}}}},$$

(10)

where Y_F is the mass fraction of the fuel; $Y_{\mathrm{F}}^{\mathrm{I}}$ is the mass fraction at the fuel source; Y_O is the mass fraction of oxygen; $Y_{\mathrm{O}}^{\infty }$ is the mass fraction of oxygen in the initial environment; M_O and M_F are the relative molecular weights of oxygen and fuel; v_O and v_F are the calculated coefficients of oxygen and fuel in combustion chemical reaction; and s is the coefficient introduced.

Radiative heat transfer is solved by finite volume method, and the radiative transfer equation as follows:

$$\mathit{s}\cdot \nabla I_{\lambda }(x, \mathit{s})= -[\kappa (x, \lambda )+{\sigma }_{\mathit{s}}(x, \lambda )]I(x, \mathit{s})+B(x, \lambda )+{\displaystyle \frac{{\sigma }_s(x, \lambda )}{4p}}{\int }_{4p}\Phi (\mathit{s}, \mathit{s}\prime ){\mathrm{I}}_{\lambda }({\sigma }_s(x, \lambda ))\mathrm{d}\Omega \prime ,$$

(11)

where I_λ(x, s) is radiation intensity with wavelength λ; κ(x, λ) and σ(x, λ) are local absorption and scattering coefficients; B(x, λ) is radiation source term; Φ(s, $\mathit{s}\mathit{\prime }$) is dissipation coefficient; and s is intensity direction vector.

2.2. Physical Model

The numerical model was constructed using FDS. Based on extensive investigations of metro engineering data, related standards, and design specifications [18,19,20], this study carried out on-site investigations on metro tunnel engineering in many cities across China. Combining actual metro tunnel sizes and considering the universality of research conclusions for modeling design. The scheme of model tunnel is shown in Figure 1. The total length of the model tunnel is set at 2000.0 m. The tunnel height is 5.0 m. The tunnel wall material is concrete, and the thermal conductivity is 1.8 W/(m·K). The left side of tunnel is downhill side, and the right side is uphill side. The fire source is located at the longitudinal center of the tunnel. The location of the fire source is specified as the zero point of coordinate. Two intermediate air shafts are set at x = −500 m and x = 500 m, respectively, and the air shafts are perpendicular to the horizontal plane, as shown in Figure 1. The sizes of the smoke exhaust vents of intermediate air shafts are both set as 4.0 m × 4.0 m. The airflow volumes of tunnel fans inside the intermediate air shafts are both 60 m³/s. The tunnel fans can rotate forward or reverse to realize the functions of air supply or smoke exhaust. The two ends of the model tunnel are set as open boundaries. The ambient temperature is set as 20.0 °C, and the ambient pressure is set as 101.0 kPa.

2.3. Fire Scenario

In this study, a total of 20 experimental conditions were designed, as presented in

Table 1. Experimental conditions.

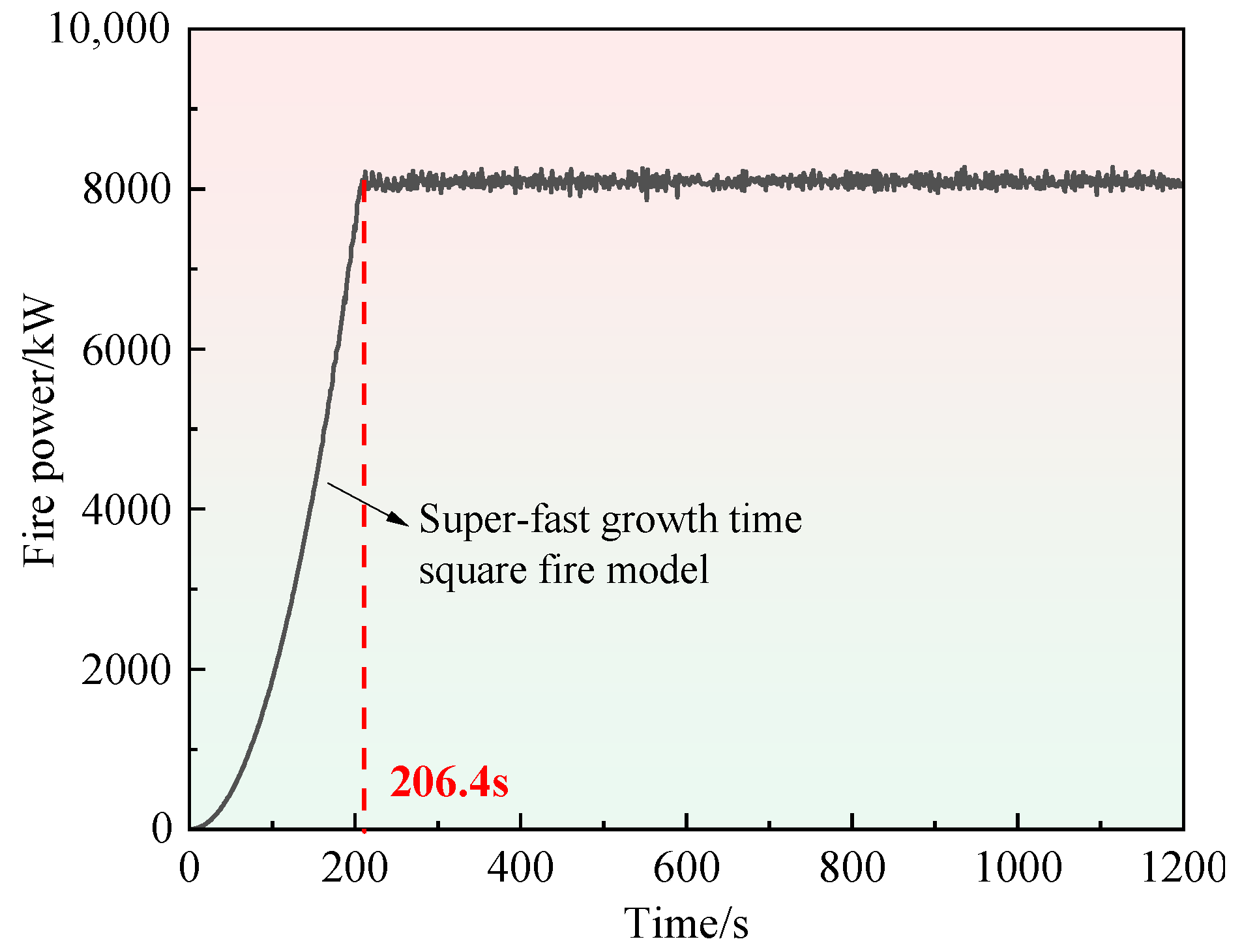
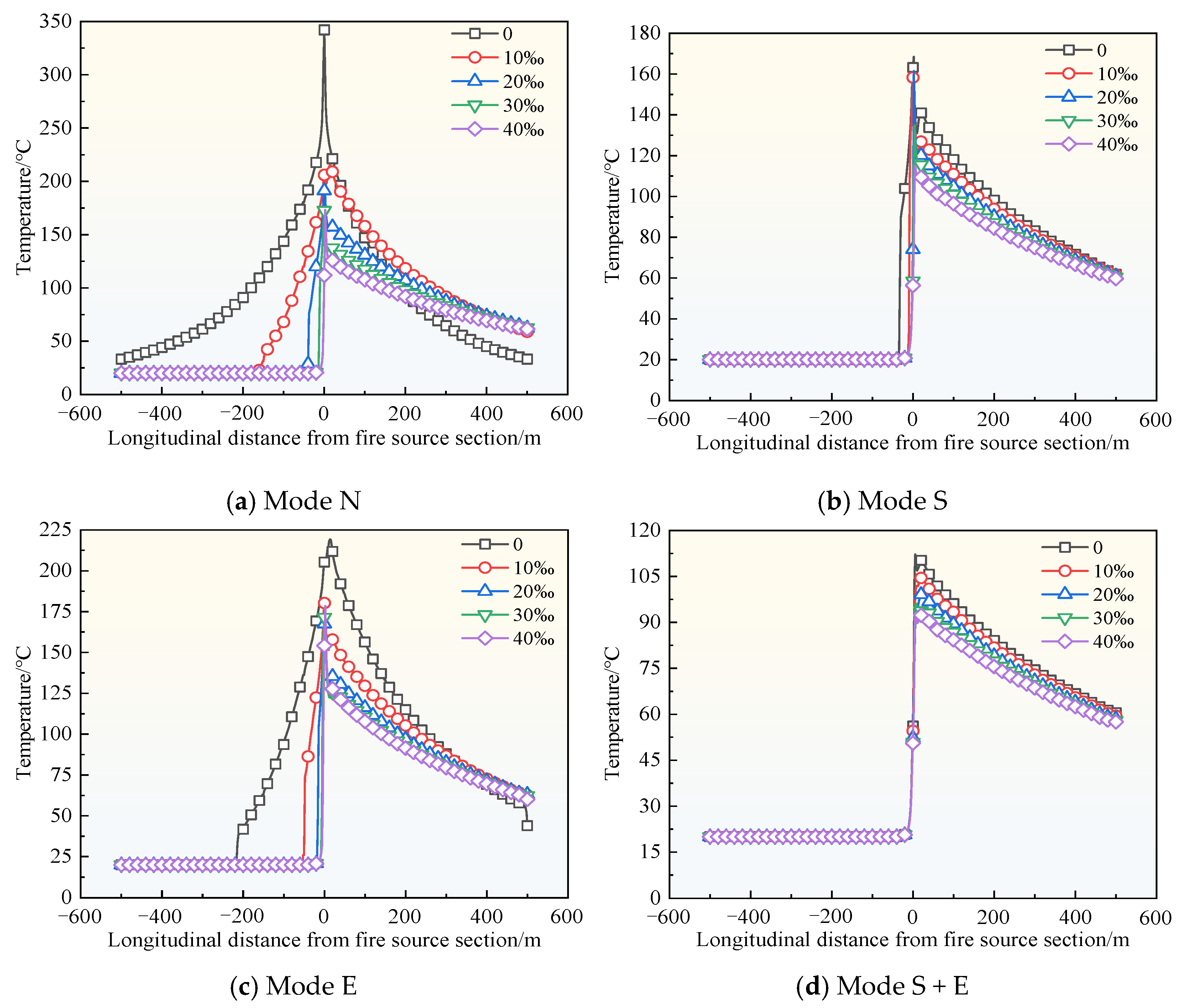
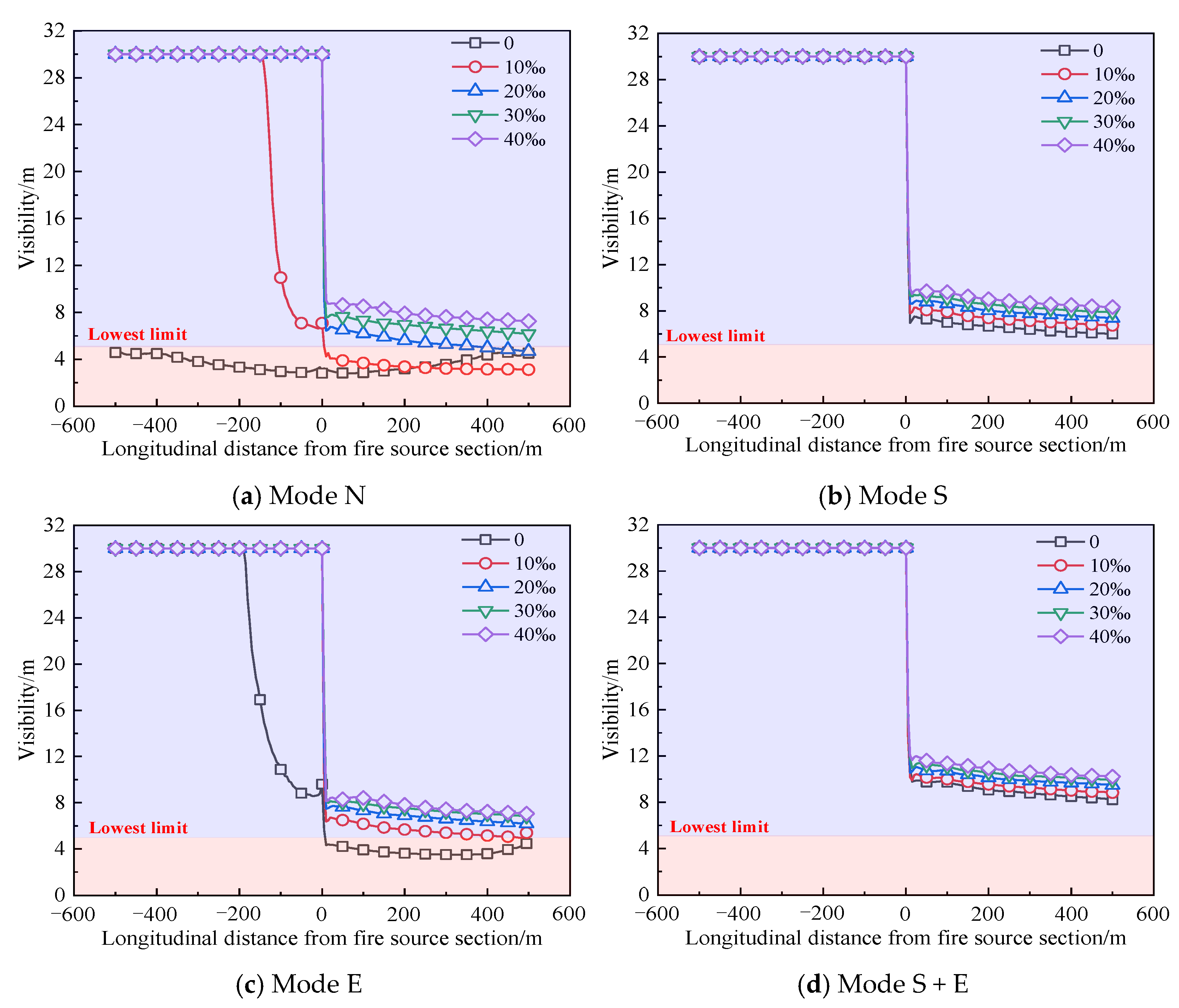
Experimental Condition	Tunnel Slope	Ventilation Mode
1–5	0, 10‰, 20‰, 30‰, 40‰	Natural ventilation (N)
6–10		Single-side air supply (S)
11–15		Single-side smoke exhaust (E)
16–20		Air supply + smoke exhaust (S + E)

. Standard for Fire Protection Design of Metro (GB 51298-2018) [20] indicates that in the fire protection design in China, the heat release rate of a metro train carriage can be adopted as 7.5 MW. Therefore, in this study, the heat release rate was set at 8.0 MW to simulate a train carriage fire. The super-fast growth time square fire model was employed, and the heat release rate growth coefficient is 0.1878 kW/s² [21]. That is, the maximum heat release rate can be reached in 206.4 s after a train carriage catches fire, as illustrated in Figure 2. The Code for Design of Metro (GB 50157-2013) [22] stipulates that the maximum slope of the metro tunnel main line cannot exceed 30‰, the maximum slope of a difficult section cannot exceed 35‰, and the maximum slope of a connecting line cannot exceed 40‰. Thus, in this study, the model tunnel slopes were set as 0, 10‰, 20‰, 30‰, and 40‰ to explore the influence of tunnel slopes. In the setting of ventilation and smoke exhaust mode, four ventilation modes were considered: natural ventilation, single-side air supply (only the 1# intermediate air shaft starts air supply mode), single-side smoke exhaust (only the 2# intermediate air shaft starts smoke exhaust mode), and air supply plus smoke exhaust (the 1# intermediate air shaft starts air supply mode plus the 2# intermediate air shaft starts smoke exhaust mode). At this time, the airflow organization direction of mechanical ventilation is from the downhill side to the uphill side of the tunnel, which is consistent with the direction of airflow velocity induced by the stack effect. Thus, the stack effect can enhance the airflow organization of mechanical ventilation.

Sensors and measuring points are arranged at key positions. Among them, 501 thermocouples are longitudinally arranged on the model tunnel ceiling to measure the temperature. The distance between adjacent thermocouples is uniformly set to 2.0 m. In total, 201 visibility measuring points are longitudinally arranged along the tunnel at a height of 2.1 m from the ground. The interval between adjacent measuring points is 5.0 m. Moreover, 201 smoke layer height measuring points are longitudinally arranged along the tunnel. The interval between adjacent measuring points is also set at 5.0 m. The arrangement area for all the above measuring points is within the range of x = −500~500 m.

2.4. Mesh Refinement and Boundary Conditions

The mesh division of the computational domain has a significant impact on the accuracy of numerical simulation. The mesh size is a key parameter in numerical simulation. Generally, a smaller mesh can yield more accurate calculation results, but it also imposes higher requirements on hardware configuration and computational time. Thus, the mesh size should neither be too large nor too small. Therefore, before conducting formal fire simulation research, it is necessary to verify and analyze the mesh independence of the numerical model. Comprehensively considering the accuracy and efficiency of the numerical calculation results, an appropriate mesh size should be selected. In fire simulation studies, it is generally believed that numerical simulation results are better when the value of the ratio D^*/δx is 4 to 16. The definition of the characteristic fire source diameter is shown in Equation (12) [23].

$$D^{*}={({\displaystyle \frac{Q}{{\rho }_{\mathrm{a}}{C}_{\mathrm{p}}{T}_{\mathrm{a}}\sqrt{\mathrm{g}}}})}^{2/5},$$

(12)

where D^* is the characteristic diameter of the fire source; Q is the heat release rate, kW; ρ_a is ambient density, kg/m³; C_p is specific heat capacity, kJ/kg·K; T_a is ambient temperature, K; and g is gravity acceleration, m/s².

For a heat release rate of 8.0 MW, the recommended value range of the mesh size δx is 0.138 m to 0.551 m. Therefore, in this study, four mesh sizes of 0.5 m × 0.5 m × 0.5 m, 0.4 m × 0.4 m × 0.4 m, 0.25 m × 0.25 m × 0.25 m, and 0.125 m × 0.125 m × 0.125 m are selected to test the mesh independence of FDS model. The longitudinal distribution of ceiling temperature in a horizontal tunnel under natural ventilation for the four mesh sizes is shown in Figure 3.

As can be seen in Figure 3, the longitudinal distribution of ceiling temperature for the mesh sizes of 0.5 m × 0.5 m × 0.5 m and 0.4 m × 0.4 m × 0.4 m is quite different from that of 0.25 m × 0.25 m × 0.25 m and 0.125 m × 0.125 m × 0.125 m. However, the results of 0.25 m × 0.25 m × 0.25 m and 0.125 m × 0.125 m × 0.125 m are not significantly different. Therefore, in order to ensure the accuracy of numerical simulation, the mesh size is selected as 0.25 m × 0.25 m × 0.25 m.

3. Analysis and Discussion

3.1. Longitudinal Distribution of Tunnel Ceiling Temperature

The longitudinal distribution of tunnel ceiling temperature holds great significance for the safety assessment of tunnel structure and the prediction of fire risks [24]. Figure 4 presents the longitudinal distribution of ceiling temperature under different ventilation modes. It can be observed that under mode N, the longitudinal ceiling temperature of the horizontal tunnel is symmetrically distributed. The maximum ceiling temperature is located directly above the fire source, which is 342.08 °C. However, when the tunnel has a slope, due to stack effect [5], the longitudinal ceiling temperature is asymmetrically distributed. The greater the tunnel slope, the lower the maximum ceiling temperature and the shorter the smoke back-layering length. The phenomenon that the fire smoke spreads upstream is called the smoke back-layering phenomenon, and the longitudinal distance between the smoke front and the fire source is called the smoke back-layering length. When the tunnel slopes are 10‰, 20‰, 30‰, and 40‰, the maximum tunnel ceiling temperatures are 215.19 °C, 191.32 °C, 173.32 °C, and 172.64 °C, respectively. The smoke back-layering lengths are 160 m, 36 m, 18 m, and 8 m, respectively. This phenomenon is attributed to the elevation difference between the two ends of a sloped tunnel. When a fire occurs, the stack effect will generate an induced airflow directed from downhill to uphill, which enhances the convective heat transfer between the hot smoke and the cool airflow. The greater the tunnel slope, the more significant the stack effect.

When the ventilation mode is mode S or mode E, compared with mode N, the maximum ceiling temperature and smoke back-layering length are significantly decreased. Moreover, the cooling effect of mode S is better than that of mode E. When the tunnel slopes are 0, 10‰, 20‰, 30‰, and 40‰, the maximum tunnel ceiling temperatures under mode S are 168.50 °C, 161.45 °C, 159.29 °C, 134.83 °C, and 115.03 °C, respectively. The maximum tunnel ceiling temperatures under mode E are 219.25 °C, 180.25 °C, 178.53 °C, 174.55 °C, and 170.85 °C, respectively. In addition, when the ventilation mode is mode S + E, the cooling effect is at its best. The maximum tunnel ceiling temperatures are 112.21 °C, 104.56 °C, 99.07 °C, 96.20 °C, and 92.97 °C, respectively. At this time, there is no smoke back-layering phenomenon in each slope scene.

3.2. Visibility Distribution

The extinction of fire smoke will diminish the visibility of the surrounding environment of the fire area. Higher visibility can offer a better environment for personnel evacuation and emergency rescue in tunnel fires. Zhu et al. [25] indicated that the lowest limit of visibility for safe evacuation in metro tunnels is 5.0 m. Figure 5 shows the longitudinal distribution of visibility near the fire location in the stage of full fire development. It can be observed that as the tunnel slope increases, the visibility on the downhill side exhibits an increasing trend. After mode S + E is adopted, the visibility on the downhill side in each slope scenario does not decrease, and the visibility on uphill side is also better than that of other ventilation modes. This is because the larger the tunnel slope, the greater the induced airflow velocity, and the fan linkage mode on both sides of the fire source is better than the single-side operation mode.

Dangerous height is the minimum requirement for safety evacuation and emergency rescue in building fire, and it is also the minimum requirement that must be achieved in the design of smoke exhaust system. The Technical Standard for Smoke Management Systems in Buildings (GB 51251-2017) [26] stipulates that the dangerous height in smoke control and exhaust design should be calculated according to Equation (13).

$$H_{\mathrm{q}}=1.6+0.1H$$

(13)

where H_q is the dangerous height, m, and H is the net height of smoke exhaust building, m.

Figure 6 presents the longitudinal distribution of visibility at a dangerous height under different experimental conditions. It can be found that under mode N, the visibilities in the horizontal tunnel are all lower than 5.0 m, making the evacuation and rescue environment unfavorable. When the tunnel slope is 10‰, there is a sudden change in visibility on the downhill side at x = −145 m, which indicates that this position is the front of the smoke back-layering at a dangerous height. In the range of x = −145~0 m, the visibility rapidly drops from 30.0 m to 6.67 m, and the average visibility on the uphill side is 3.42 m. When the tunnel slope exceeds 20‰, the visibilities of the downhill side do not decrease significantly, and the environment is more favorable for evacuation and rescue at this time. With the increase in tunnel slope, the visibility of uphill side increases, and when the tunnel slopes are 20‰, 30‰ and 40‰, the average visibilities of uphill side are 5.57 m, 6.89 m, and 7.98 m, respectively. When mechanical ventilation was employed to assist smoke extraction, the visibility on the downhill side, except for the area of x = −190~0 m in the horizontal tunnel under mode E, did not decrease significantly in other scenes or areas. For the uphill side, the average visibilities of 0, 10‰, 20‰, 30‰, and 40‰ tunnel slopes under mode S are 6.64 m, 7.40 m, 8.08 m, 8.57 m, and 9.02 m, respectively. The average visibilities under mode E are 3.80 m, 5.67 m, 6.87 m, 7.52 m, and 7.78 m, respectively. The average visibilities under mode S + E are 9.07 m, 9.50 m, 10.14 m, 10.55 m, and 10.89 m, respectively. Therefore, the visibility increases with the increase in tunnel slope, and the visibilities of mode S + E are higher than those of mode S and mode E.

3.3. Smoke Layer Height

The smoke layer height is an important parameter for characterizing the downward settlement of smoke and its associated risks. Maintaining the smoke stratification structure and keeping the toxic and harmful smoke in the upper area of the tunnel is crucial for emergency rescue and personnel evacuation in fire scenarios [27]. Figure 7 shows the distribution of smoke layer heights under different ventilation modes. It can be observed that under mode N, the smoke layer height at the fire source section of the horizontal tunnel is approximately 2.69 m. With the increase in longitudinal distance from the fire source section, the smoke layer height shows a downward trend. This is because the smoke temperature at the fire source section is higher, and the smoke in the lower region can be affected by thermal radiation of fire source. As a result, the thermal buoyancy of smoke is obvious, and the hot smoke is difficult to settle down. While during the process of smoke longitudinal spreading, the smoke will entrain cold air continuously. As a result, the temperature decreases gradually, the buoyancy effect is weakened, and the smoke settlement effect is strengthened. For the downhill side, when the tunnel slopes are 10‰, 20‰, and 30‰, the smoke layer heights change rapidly at x = −160 m, x = −40 m and x = −20 m, respectively, which is consistent with the position of smoke back-layering front. For the uphill side, when the tunnel has a slope, the smoke layer height is significantly lower than that of horizontal tunnel. This is because the stack effect can promote the spreading of fire smoke to the uphill side. The amount of smoke on the uphill side in a sloped tunnel is significantly greater than that in a horizontal tunnel, thus lowering the smoke layer height. When mechanical ventilation is employed to assist smoke extraction, the difference in smoke layer heights under different ventilation modes is small on the uphill side. The average smoke layer height is approximately 1.2 m. However, the effect of smoke settlement on the downhill side under mode E is more obvious than under mode S and mode S + E.

3.4. Smoke Exhaust Efficiency

Smoke exhaust efficiency is defined as the percentage of smoke discharged from the smoke exhaust vent during the fire smoke generation within a unit time. This is an important parameter for evaluating the smoke exhaust effect of ventilation modes [28]. In the study of fire smoke control, the amount of smoke generated is not easy to calculate. Therefore, in this study, the smoke extraction efficiency is characterized by the ratio of the oxycarbide discharge rate from the smoke exhaust vent to the oxycarbide generation rate in tunnel fires. In the numerical simulation, the oxycarbide discharge rate from the smoke exhaust vent can be calculated by measuring the gas exhaust volume and oxycarbide concentration per unit time at each smoke exhaust vent. The generation rate of oxycarbide in the fire can be solved by the combustion reaction equation [29,30].

Under mode N and mode S, the smoke exhaust efficiencies of the 2# intermediate air shaft are all less than 0.1%, indicating that if the smoke exhaust mode was not activated in the 2# intermediate air shaft tunnel fan, the smoke exhaust effect can be ignored. Figure 8 shows the smoke exhaust efficiency of the 2# intermediate air shaft under mode E and mode S + E. It can be found that when mode E was adopted, with the increase in tunnel slope, the smoke exhaust efficiencies of the 2# intermediate air shaft tend to increase, and the influence of tunnel slope on this effect is more obvious. When mode S + E was adopted, the smoke exhaust efficiencies of the 2# intermediate air shaft are all above 80%, indicating that the generated smoke can be mainly discharged from the 2# intermediate air shaft. In addition, with the increase in tunnel slope, the smoke exhaust efficiencies of the 2# intermediate air shaft show a decreasing trend. This is because, under the superposition of air supply of the 1# intermediate air shaft and the stack effect, the inertia force of fire smoke spreading uphill is larger. The larger the tunnel slope, the more significant the stack effect, causing part of fire smoke to spread over the 2# intermediate air shaft and continue uphill in the tunnel.

4. Conclusions

In this paper, numerical simulation has been employed to study the smoke exhaust performance of intermediate air shafts in sloped metro tunnels from four aspects: the longitudinal distribution of ceiling temperature, visibility distribution, smoke layer height, and the smoke exhaust efficiency of the intermediate air shaft. The main conclusions are as follows:

(1) The larger the tunnel slope, the lower the maximum tunnel ceiling temperature, and the shorter the smoke back-layering length. The adoption of mechanical ventilation to assist smoke extraction can significantly reduce the tunnel ceiling temperature, and the cooling effect of mode S + E is the most effective.

(2) As the tunnel slope increases, the visibility at the dangerous height position shows an increasing trend. When the ventilation mode is mode S or mode S + E, the visibility of the downhill side does not decrease significantly. The visibility on the uphill side under mode S + E is higher than that under mode S and mode E.

(3) The smoke layer height on the downhill side of a sloped tunnel is higher than that of a horizontal tunnel, but the smoke layer height on the uphill side is lower than that of a horizontal tunnel. When mechanical ventilation is employed to assist smoke exhaust, the difference in smoke layer height on the uphill side is small under different ventilation modes, and the average smoke layer height is about 1.2 m. The effect of smoke settlement on the downhill side under mode E is more obvious than under mode S and mode S + E.

(4) When mode E is adopted, with the increase in tunnel slope, the smoke exhaust efficiencies of the 2# intermediate air shaft show an increasing trend. When mode S + E is adopted, the smoke exhaust efficiencies of 2# intermediate air shaft are all above 80%, and the smoke exhaust efficiencies decrease with the increase in tunnel slopes.