Rectified Lorentz Force from Thermal Current Fluctuations
Abstract
1. Introduction
2. Model
3. Discussion
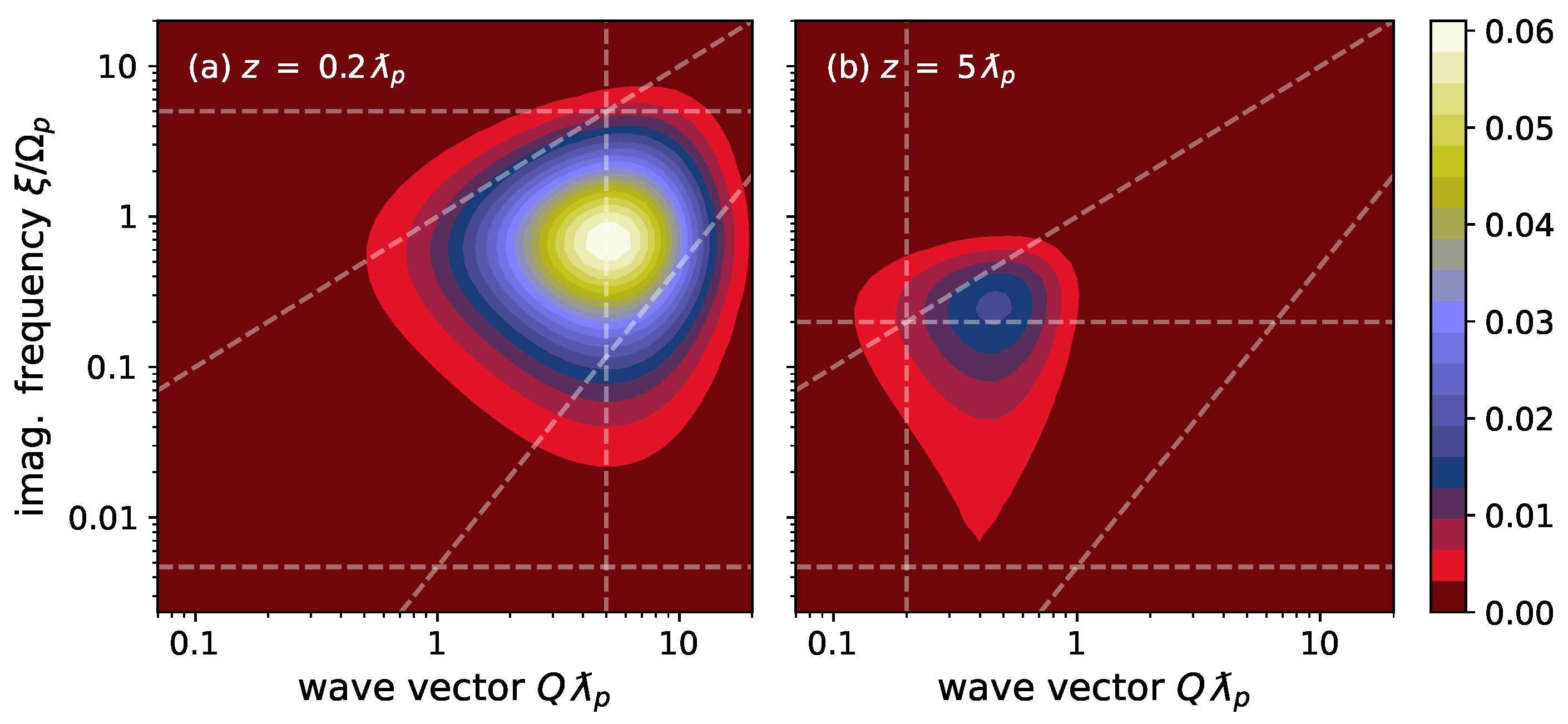
3.1. General Features
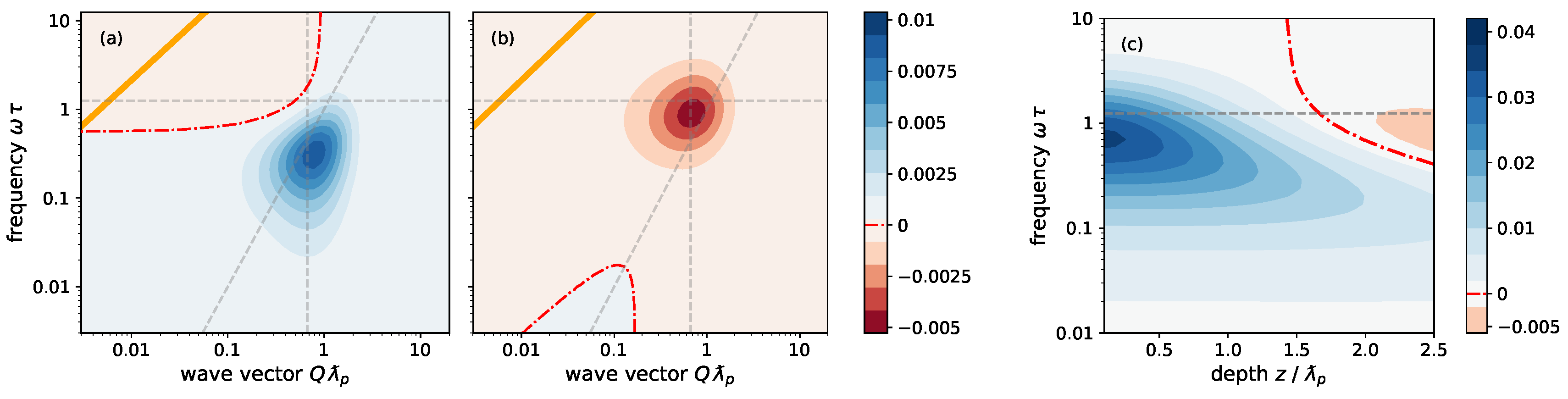
3.2. Thermal Hall Force
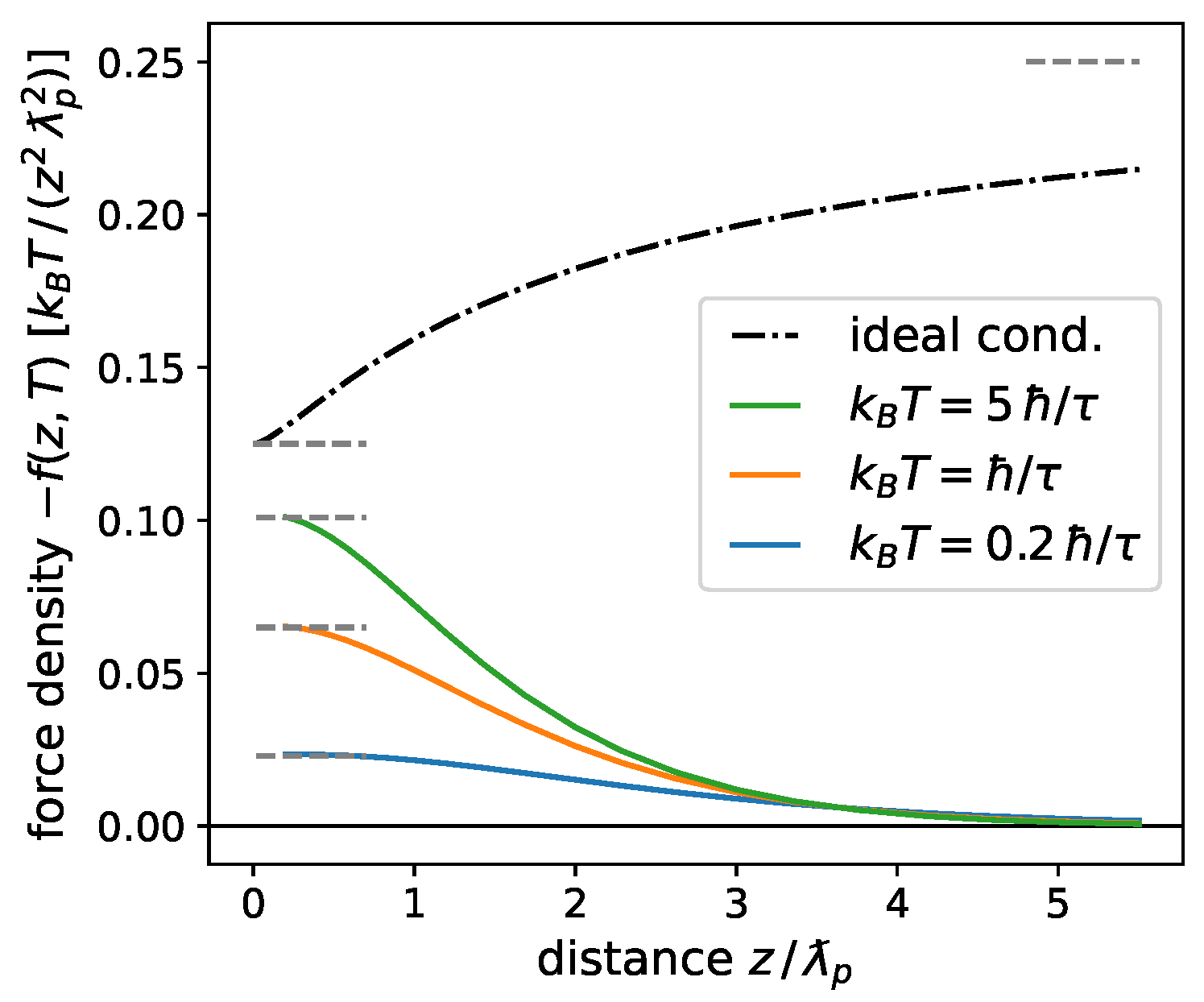
3.3. Physical Consequences
4. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Details of the Calculation
Appendix A.1. Polarisation Vectors
Appendix A.2. Average Poynting Vector
References
- Uhlig, R.P.; Zec, M.; Brauer, H.; Thess, A. Lorentz force eddy current testing: A prototype model. J. Nondestruct. Eval. 2012, 31, 357–372. [Google Scholar] [CrossRef]
- Li, C.; Liu, R.; Dai, S.; Zhang, N.; Wang, X. Vector-based eddy-current testing method. Appl. Sci. 2018, 8, 2289. [Google Scholar] [CrossRef]
- Alkhalil, S.; Kolesnikov, Y.; Thess, A. Lorentz force sigmometry: A novel technique for measuring the electrical conductivity of solid and liquid metals. Meas. Sci. Technol. 2015, 26, 115605. [Google Scholar] [CrossRef]
- Bloembergen, N.; Chang, R.K.; Jha, S.S.; Lee, C.H. Optical second-harmonic generation in reflection from media with inversion symmetry. Phys. Rev. 1969, 178, 1528. [Google Scholar] [CrossRef]
- Sipe, J.E.; So, V.C.Y.; Fukui, M.; Stegeman, G.I. Analysis of second-harmonic generation at metal surfaces. Phys. Rev. B 1980, 21, 4389–4402. [Google Scholar] [CrossRef]
- Renger, J.; Quidant, R.; van Hulst, N.; Palomba, S.; Novotny, L. Free-space excitation of propagating surface plasmon polaritons by nonlinear four-wave mixing. Phys. Rev. Lett. 2009, 103, 266802. [Google Scholar] [CrossRef] [PubMed]
- Renger, J.; Quidant, R.; van Hulst, N.; Novotny, L. Surface-enhanced nonlinear four-wave mixing. Phys. Rev. Lett. 2010, 104, 046803. [Google Scholar] [CrossRef]
- Hille, A.; Moeferdt, M.; Wolff, C.; Matyssek, C.; Rodríguez-Oliveros, R.; Prohm, C.; Niegemann, J.; Grafström, S.; Eng, L.M.; Busch, K. Second harmonic generation from metal nano-particle resonators: Numerical analysis on the basis of the hydrodynamic Drude model. J. Phys. Chem. C 2016, 120, 1163–1169. [Google Scholar] [CrossRef]
- Kadlec, F.; Kužel, P.; Coutaz, J.-L. Optical rectification at metal surfaces. Opt. Lett. 2004, 29, 2674–2676. [Google Scholar] [CrossRef]
- Hoffmann, M.C.; Fülöp, J.A. Intense ultrashort terahertz pulses: Generation and applications. J. Phys. D Appl. Phys. 2011, 44, 083001. [Google Scholar] [CrossRef]
- Trinh, M.T.; Smail, G.; Makhal, K.; Yang, D.S.; Kim, J.; Rand, S.C. Observation of magneto-electric rectification at non-relativistic intensities. Nat. Commun. 2020, 11, 5296. [Google Scholar] [CrossRef] [PubMed]
- Craig, R.A. Dynamic contributions to the surface energy of simple metals. Phys. Rev. B 1972, 6, 1134–1142. [Google Scholar] [CrossRef]
- Morgenstern Horing, N.J.; Kamen, E.; Gumbs, G. Surface correlation energy and the hydrodynamic model of dynamic, nonlocal bounded plasma response. Phys. Rev. B 1985, 31, 8269–8272. [Google Scholar] [CrossRef] [PubMed]
- Barton, G. On the surface energy of metals according to the hydrodynamic model with spatial dispersion. J. Phys. C Sol. State Phys. 1986, 19, 975–980. [Google Scholar] [CrossRef]
- Prange, R.E.; Kadanoff, L.P. Transport theory for electron–phonon interactions in metals. Phys. Rev. 1964, 134, A566–A580. [Google Scholar] [CrossRef]
- Pudell, J.; Maznev, A.; Herzog, M.; Kronseder, M.; Back, C.; Malinowski, G.; von Reppert, A.; Bargheer, M. Layer specific observation of slow thermal equilibration in ultrathin metallic nanostructures by femtosecond X-ray diffraction. Nat. Commun. 2018, 9, 3335. [Google Scholar] [CrossRef]
- Bresson, P.; Bryche, J.F.; Besbes, M.; Moreau, J.; Karsenti, P.L.; Charette, P.G.; Morris, D.; Canva, M. Improved two-temperature modeling of ultrafast thermal and optical phenomena in continuous and nanostructured metal films. Phys. Rev. B 2020, 102, 155127. [Google Scholar] [CrossRef]
- Rytov, S.M.; Kravtsov, Y.A.; Tatarskii, V.I. Principles of Statistical Radiophysics. Volume 3: Elements of Random Fields; Springer: Berlin/Heidelberg, Germany, 1989. [Google Scholar]
- Griniasty, I.; Leonhardt, U. Casimir stress inside planar materials. Phys. Rev. A 2017, 96, 032123. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mostepanenko, V.M.; Svetovoy, V.B. Experimentum crucis for electromagnetic response of metals to evanescent waves and the Casimir puzzle. Universe 2022, 8, 574. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mostepanenko, V.M.; Svetovoy, V.B. Probing the response of metals to low-frequency s-polarized evanescent fields. Europhys. Lett. 2022, 139, 66001. [Google Scholar] [CrossRef]
- Henkel, C. Nano-scale thermal transfer—An invitation to fluctuation electrodynamics. Z. Naturforsch. A 2017, 72, 99–108. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mostepanenko, V.M. Recent measurements of the Casimir force: Comparison between experiment and theory. Mod. Phys. Lett. A 2020, 35, 2040007. [Google Scholar] [CrossRef]
- Conti, S.; Vignale, G. Elasticity of an electron liquid. Phys. Rev. B 1999, 60, 7966–7980. [Google Scholar] [CrossRef]
- Hannemann, M.; Wegner, G.; Henkel, C. No-slip boundary conditions for electron hydrodynamics and the thermal Casimir pressure. Universe 2021, 7, 108. [Google Scholar] [CrossRef]
- Dressel, M.; Grüner, G. Electrodynamics of Solids–Optical Properties of Electrons in Matter; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar] [CrossRef]
- Berlinsky, A.J.; Kallin, C.; Rose, G.; Shi, A.C. Two-fluid interpretation of the conductivity of clean BCS superconductors. Phys. Rev. B 1993, 48, 4074–4079. [Google Scholar] [CrossRef] [PubMed]
- Klimchitskaya, G.L.; Mohideen, U.; Mostepanenko, V.M. The Casimir force between real materials: Experiment and theory. Rev. Mod. Phys. 2009, 81, 1827–1885. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mohideen, U.; Mostepanenko, V.M. Kramers-Kronig relations for plasma-like permittivities and the Casimir force. J. Phys. A 2007, 40, F339–F346. [Google Scholar] [CrossRef]
- Levine, Z.H.; Cockayne, E. The pole term in linear response theory: An example from the transverse response of the electron gas. J. Res. Natl. Inst. Stand. Technol. 2008, 113, 299–304. [Google Scholar] [CrossRef] [PubMed]
- Henkel, C.; Intravaia, F. On the Casimir entropy between ‘perfect crystals’. Int. J. Mod. Phys. A 2010, 25, 2328–2336. [Google Scholar] [CrossRef]
- Sernelius, B.E. Effects of spatial dispersion on electromagnetic surface modes and on modes associated with a gap between two half spaces. Phys. Rev. B 2005, 71, 235114. [Google Scholar] [CrossRef]
- Svetovoy, V.B.; Esquivel, R. The Casimir free energy in high- and low-temperature limits. J. Phys. A 2006, 39, 6777–6784. [Google Scholar] [CrossRef]
- Brownnutt, M.; Kumph, M.; Rabl, P.; Blatt, R. Ion-trap measurements of electric-field noise near surfaces. Rev. Mod. Phys. 2015, 87, 1419–1482. [Google Scholar] [CrossRef]
- Sipe, J.E. New Green-function formalism for surface optics. J. Opt. Soc. Am. B 1987, 4, 481–489. [Google Scholar] [CrossRef]
- Agarwal, G.S. Quantum electrodynamics in the presence of dielectrics and conductors. I. Electromagnetic-field response functions and black-body fluctuations in finite geometries. Phys. Rev. A 1975, 11, 230–242. [Google Scholar] [CrossRef]
- Lifshitz, E.M.; Pitaevskii, L.P. Statistical Physics. Part 2: Theory of the Condensed State; Butterworth–Heinemann Ltd./Elsevier Ltd.: Oxford, UK, 1980. [Google Scholar] [CrossRef]
- Buhmann, S.Y. Dispersion Forces II. Many-Body Effects, Excited Atoms, Finite Temperature and Quantum Friction; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Henkel, C. Rectified Lorentz Force from Thermal Current Fluctuations. Physics 2024, 6, 568-578. https://doi.org/10.3390/physics6020037
Henkel C. Rectified Lorentz Force from Thermal Current Fluctuations. Physics. 2024; 6(2):568-578. https://doi.org/10.3390/physics6020037
Chicago/Turabian StyleHenkel, Carsten. 2024. "Rectified Lorentz Force from Thermal Current Fluctuations" Physics 6, no. 2: 568-578. https://doi.org/10.3390/physics6020037
APA StyleHenkel, C. (2024). Rectified Lorentz Force from Thermal Current Fluctuations. Physics, 6(2), 568-578. https://doi.org/10.3390/physics6020037




