Suspended-Load Backpacks to Reduce the Cost of Carrying Loads with Energy Scavenging Potential—Part 1: Pre-Compression Design
Abstract
:1. Introduction
1.1. Background
1.2. Formulation of the Problem of Interest for This Investigation
1.3. Literature Survey
1.4. Scope and Contribution of This Study
1.5. Organization of the Paper
2. Methods
3. Results and Discussion
4. Concluding Remarks
- i.
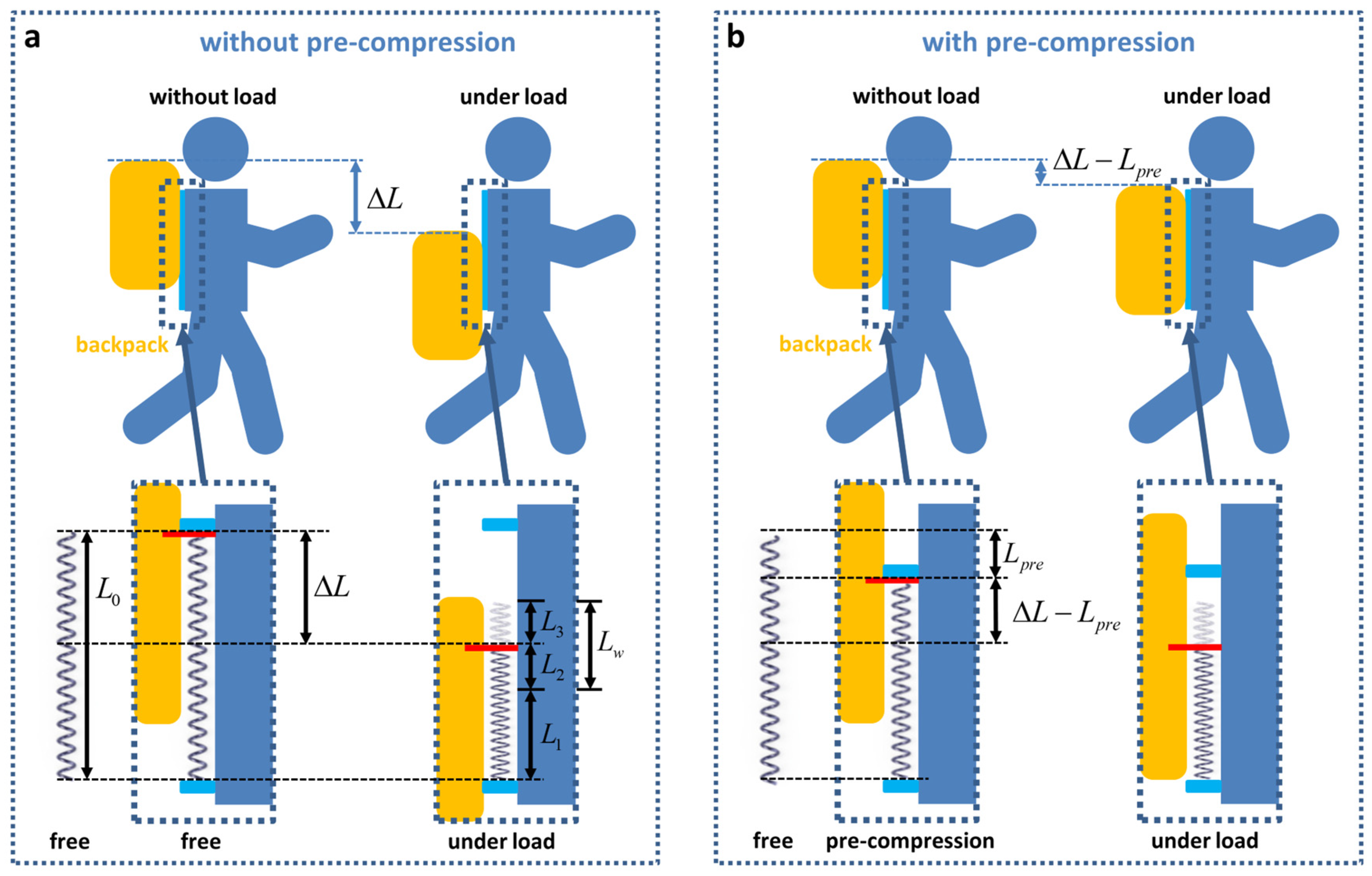
- We propose a new design strategy of pre-compression, using pre-compression springs as the elastic components of the SUSB. Previous researchers used pulleys and rubber ropes as the elastic components. Compared with previous studies, the use of pre-compression springs as elastic elements improves the reliability of the SUSB structure, avoids the inconvenience of nonlinearity and material aging, and adds the ability to flexibly adjust the sliding distance of the backpack.
- ii.
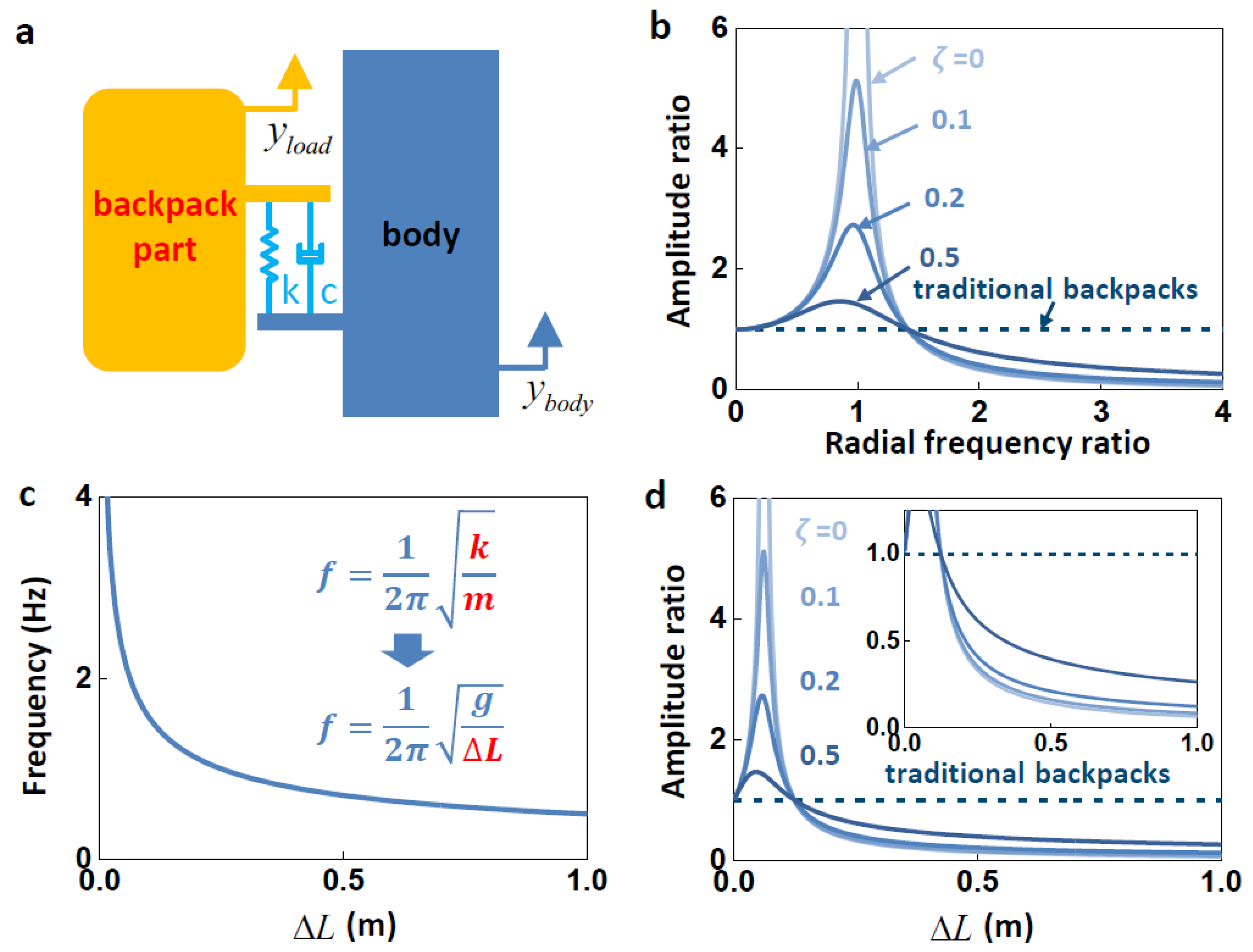
- A theoretical model is developed for the pre-compression SUSB. The natural frequency of the pre-compression SUSB is related to only one parameter, the compression amount ΔL. This simplifies the process of evaluating the performance of the pre-compression SUSB.
- iii.
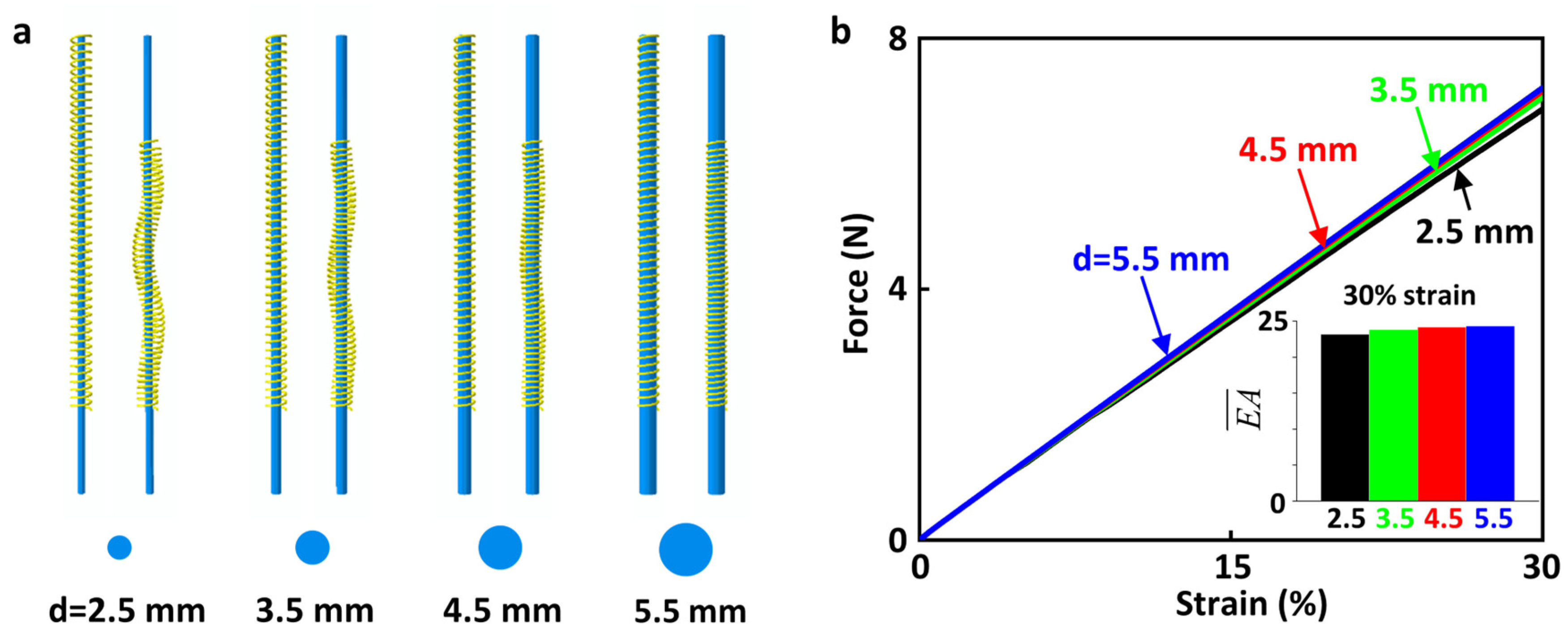
- We manufacture the pre-compression SUSB. The suspension performance of the pre-compression SUSB is verified with experiments. At a radial frequency ratio of 2.5, the amplitude of the backpack part is only 30.6% of that of the carrying part. The effect of the buckling behavior of the compression spring on the suspension effect is investigated by FEA. The diameter of the support pole has little effect on the compression stiffness of the spring.
- iv.
- Previous work added a sliding TENG to the rubber-rope-based SUSB. Because this TENG scavenges energy via the relative movement between the carrying part and the backpack part, the TENG is universal to all SUSBs. In addition to the universal TENG, our SUSB can be equipped with a TENG in the elastic component, which scavenges energy via the relative movement between the elastic components. This work provides a new design idea for the SUSB.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ceylan, E.; Dede, S.; Deger, Y.; Yoruk, I. Investigation of the Effects of Carrying Heavy Load on Prooxidation/Antioxidant Status and Vitamin D3 in Healthy Horses. Asian J. Anim. Vet. Adv. 2008, 4, 41–46. [Google Scholar]
- Forkenbrock, D.J. Comparison of external costs of rail and truck freight transportation. Transp. Res. Part A Policy Pract. 2001, 35, 321–337. [Google Scholar]
- Forkenbrock, D.J. External costs of intercity truck freight transportation. Transp. Res. Part A-Policy Pract. 1999, 33, 505–526. [Google Scholar]
- Woolley-Meza, O.; Thiemann, C.; Grady, D.; Lee, J.J.; Seebens, H.; Blasius, B.; Brockmann, D. Complexity in human transportation networks: A comparative analysis of worldwide air transportation and global cargo-ship movements. Eur. Phys. J. B 2011, 84, 589–600. [Google Scholar]
- Kanafani, A. Aircraft technology and network structure in short-haul air transportation. Transp. Res. Part A Gen. 1981, 15, 305–331. [Google Scholar]
- Knapik, J.J.; Reynolds, K.L.; Harman, E. Soldier load carriage: Historical, physiological, biomechanical, and medical aspects. Mil. Med. 2004, 169, 45–56. [Google Scholar]
- Ren, L.; Jones, R.K.; Howard, D. Dynamic analysis of load carriage biomechanics during level walking. J. Biomech. 2005, 38, 853–863. [Google Scholar]
- Balogun, J.A. Ergonomic Comparison of 3 Modes of Load Carriage. Int. Arch. Occup. Environ. Health 1986, 58, 35–46. [Google Scholar]
- Ramanathan, N.L.; Datta, S.R.; Gupta, M.N. Biomechanics of various modes of load transport on level ground. Indian J. Med. Res. 1972, 60, 1702–1710. [Google Scholar]
- Kim, J.; Lee, G.; Heimgartner, R.; Revi, D.A.; Karavas, N.; Nathanson, D.; Galiana, I.; Eckert-Erdheim, A.; Murphy, P.; Perry, D.; et al. Reducing the metabolic rate of walking and running with a versatile, portable exosuit. Science 2019, 365, 668–672. [Google Scholar]
- Zoss, A.; Kazerooni, H. Design of an electrically actuated lower extremity exoskeleton. Adv. Robot. 2006, 20, 967–988. [Google Scholar]
- Guizzo, E.; Goldstein, H. The rise of the body bots. IEEE Spectr. 2005, 42, 50–56. [Google Scholar] [CrossRef]
- Fontana, M.; Vertechy, R.; Marcheschi, S.; Salsedo, F.; Bergamasco, M. The Body Extender: A Full-Body Exoskeleton for the Transport and Handling of Heavy Loads. IEEE Robot. Autom. Mag. 2014, 21, 34–44. [Google Scholar]
- Collins, S.H.; Wiggin, M.B.; Sawicki, G.S. Reducing the energy cost of human walking using an unpowered exoskeleton. Nature 2015, 522, 212–215. [Google Scholar] [PubMed]
- Zhang, J.; Fiers, P.; Witte, K.A.; Jackson, R.W.; Poggensee, K.L.; Atkeson, C.G.; Collins, S.H. Human-in-the-loop optimization of exoskeleton assistance during walking. Science 2017, 356, 1280–1284. [Google Scholar] [PubMed]
- Nasiri, R.; Ahmadi, A.; Ahmadabadi, M.N. Reducing the Energy Cost of Human Running Using an Unpowered Exoskeleton. IEEE Trans. Neural Syst. Rehabil. Eng. 2018, 26, 2026–2032. [Google Scholar]
- Abohamer, M.K.; Awrejcewicz, J.; Amer, T.S. Modeling and analysis of a piezoelectric transducer embedded in a nonlinear damped dynamical system. Nonlinear Dyn. 2023, 111, 8217–8234. [Google Scholar]
- Wang, X.; Liu, X.; Zhang, J.; Liang, L.; Li, M.; Yao, H.; Hou, T.; Wu, Y.; Zi, Y.; Zheng, H. A high-applicability, high-durability wearable hybrid nanogenerator with magnetic suspension structure toward health monitoring applications. Nano Energy 2022, 103, 107774. [Google Scholar]
- Wang, K.; Wu, C.; Zhang, H.; Li, J.; Li, J. Cylindrical bearing inspired oil enhanced rolling friction based nanogenerator. Nano Energy 2022, 99, 107372. [Google Scholar]
- Abohamer, M.K.; Awrejcewicz, J.; Starosta, R.; Amer, T.S.; Bek, M.A. Influence of the Motion of a Spring Pendulum on Energy-Harvesting Devices. Appl. Sci. 2021, 11, 8658. [Google Scholar]
- Abohamer, M.; Awrejcewicz, J.; Amer, T. Modeling of the vibration and stability of a dynamical system coupled with an energy harvesting device. Alex. Eng. J. 2023, 63, 377–397. [Google Scholar] [CrossRef]
- Xiao, X.; Nashalian, A.; Libanori, A.; Fang, Y.; Li, X.; Chen, J. Triboelectric Nanogenerators for Self-Powered Wound Healing. Adv. Health Mater. 2021, 10, 2100975. [Google Scholar] [CrossRef] [PubMed]
- Choi, D.; Lee, Y.; Lin, Z.-H.; Cho, S.; Kim, M.; Ao, C.K.; Soh, S.; Sohn, C.; Jeong, C.K.; Lee, J.; et al. Recent Advances in Triboelectric Nanogenerators: From Technological Progress to Commercial Applications. ACS Nano 2023, 17, 11087–11219. [Google Scholar] [CrossRef] [PubMed]
- Barman, S.R.; Chan, S.-W.; Kao, F.-C.; Ho, H.-Y.; Khan, I.; Pal, A.; Huang, C.-C.; Lin, Z.-H. A self-powered multifunctional dressing for active infection prevention and accelerated wound healing. Sci. Adv. 2023, 9, eadc8758. [Google Scholar] [CrossRef] [PubMed]
- Zhao, H.; Xu, M.; Shu, M.; An, J.; Ding, W.; Liu, X.; Wang, S.; Zhao, C.; Yu, H.; Wang, H.; et al. Underwater wireless communication via TENG-generated Maxwell’s displacement current. Nat. Commun. 2022, 13, 3325. [Google Scholar] [CrossRef] [PubMed]
- Lu, Y.; Jiang, L.L.; Yu, Y.; Wang, D.H.; Sun, W.T.; Liu, Y.; Yu, J.; Zhang, J.; Wang, K.; Hu, H.; et al. Liquid-liquid triboelectric nanogenerator based on the immiscible interface of an aqueous two-phase system. Nat. Commun. 2022, 13, 5316. [Google Scholar] [CrossRef]
- Long, L.; Liu, W.; Wang, Z.; He, W.; Li, G.; Tang, Q.; Guo, H.; Pu, X.; Liu, Y.; Hu, C. High performance floating self-excited sliding triboelectric nanogenerator for micro mechanical energy harvesting. Nat. Commun. 2021, 12, 4689. [Google Scholar] [CrossRef]
- Li, C.; Liu, D.; Xu, C.; Wang, Z.; Shu, S.; Sun, Z.; Tang, W.; Wang, Z.L. Sensing of joint and spinal bending or stretching via a retractable and wearable badge reel. Nat. Commun. 2021, 12, 2950. [Google Scholar] [CrossRef]
- Ye, C.Y.; Liu, D.; Chen, P.F.; Cao, L.N.Y.; Li, X.J.; Jiang, T.; Wang, Z.L. An Integrated Solar Panel with a Triboelectric Nanogenerator Array for Synergistic Harvesting of Raindrop and Solar Energy. Adv. Mater. 2023, 35, 2209713. [Google Scholar] [CrossRef]
- Gard, A.S.; Miff, S.C.; Kuo, A.D. Comparison of kinematic and kinetic methods for computing the vertical motion of the body center of mass during walking. Hum. Mov. Sci. 2004, 22, 597–610. [Google Scholar] [CrossRef]
- Kram, R. Carrying Loads with Springy Poles. J. Appl. Physiol. 1991, 71, 1119–1122. [Google Scholar] [CrossRef]
- Rome, L.C.; Flynn, L.; Yoo, T.D. Rubber bands reduce the cost of carrying loads. Nature 2006, 444, 1023–1024. [Google Scholar] [CrossRef]
- Hoover, J.; Meguid, S.A. Performance assessment of the suspended-load backpack. Int. J. Mech. Mater. Des. 2011, 7, 111–121. [Google Scholar] [CrossRef]
- Li, D.; Li, T.; Li, Q.; Liu, T.; Yi, J. A simple model for predicting walking energetics with elastically-suspended backpack. J. Biomech. 2016, 49, 4150–4153. [Google Scholar] [CrossRef]
- Ackerman, J.; Seipel, J. A model of human walking energetics with an elastically-suspended load. J. Biomech. 2014, 47, 1922–1927. [Google Scholar] [CrossRef] [PubMed]
- Yang, Z.; Yang, Y.; Liu, F.; Wang, Z.; Li, Y.; Qiu, J.; Xiao, X.; Li, Z.; Lu, Y.; Ji, L.; et al. Power Backpack for Energy Harvesting and Reduced Load Impact. ACS Nano 2021, 15, 2611–2623. [Google Scholar] [CrossRef]
- Foissac, M.; Millet, G.Y.; Geyssant, A.; Freychat, P.; Belli, A. Characterization of the mechanical properties of backpacks and their influence on the energetics of walking. J. Biomech. 2009, 42, 125–130. [Google Scholar] [CrossRef] [PubMed]
- Harandi, M.H.F.; Loghmani, A.; Attarilar, S. Backpack with a nonlinear suspension system designed for low walking speeds. Arch. Appl. Mech. 2023, 93, 2465–2481. [Google Scholar] [CrossRef]
- Yang, Z.; Huang, L.; Zeng, Z.; Wang, R.; Hu, R.; Xie, L. Evaluation of the Load Reduction Performance Via a Suspended Backpack With Adjustable Stiffness. J. Biomech. Eng. 2021, 144, 051001. [Google Scholar] [CrossRef]
- Fan, K.; Xia, P.; Li, R.; Guo, J.; Tan, Q.; Wei, D. An innovative energy harvesting backpack strategy through a flexible mechanical motion rectifier. Energy Convers. Manag. 2022, 264, 115731. [Google Scholar] [CrossRef]
- Yoshimura, N.; Kumagai, S.; Nishimura, S. Electrical and environmental aging of silicone rubber used in outdoor insulation. IEEE Trans. Dielectr. Electr. Insul. 1999, 6, 632–650. [Google Scholar] [CrossRef]
- Gorur, R.S.; Karady, G.G.; Jagota, A.; Shah, M.; Yates, A.M.; Schneider, H.M.; Detourreil, C.; Mackevich, J.P.; Hoffman, J.W.; Orbeck, T.; et al. Aging in Silicone-Rubber Used for outdoor Insulation. IEEE Trans. Power Deliv. 1992, 7, 525–538. [Google Scholar] [CrossRef]
- Chaudhry, A.; Billingham, N. Characterisation and oxidative degradation of a room-temperature vulcanised poly(dimethylsiloxane) rubber. Polym. Degrad. Stab. 2001, 73, 505–510. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, M.; Guo, L.; Hu, J.; Wang, X.; Yang, Y.; Su, Y. Suspended-Load Backpacks to Reduce the Cost of Carrying Loads with Energy Scavenging Potential—Part 1: Pre-Compression Design. Nanoenergy Adv. 2023, 3, 259-270. https://doi.org/10.3390/nanoenergyadv3030014
Zhang M, Guo L, Hu J, Wang X, Yang Y, Su Y. Suspended-Load Backpacks to Reduce the Cost of Carrying Loads with Energy Scavenging Potential—Part 1: Pre-Compression Design. Nanoenergy Advances. 2023; 3(3):259-270. https://doi.org/10.3390/nanoenergyadv3030014
Chicago/Turabian StyleZhang, Maoyi, Liang Guo, Jihai Hu, Xingquan Wang, Ya Yang, and Yewang Su. 2023. "Suspended-Load Backpacks to Reduce the Cost of Carrying Loads with Energy Scavenging Potential—Part 1: Pre-Compression Design" Nanoenergy Advances 3, no. 3: 259-270. https://doi.org/10.3390/nanoenergyadv3030014
APA StyleZhang, M., Guo, L., Hu, J., Wang, X., Yang, Y., & Su, Y. (2023). Suspended-Load Backpacks to Reduce the Cost of Carrying Loads with Energy Scavenging Potential—Part 1: Pre-Compression Design. Nanoenergy Advances, 3(3), 259-270. https://doi.org/10.3390/nanoenergyadv3030014







