Multi-Charge Storage Layer Model of High-Charge-Density Triboelectric Nanogenerator
Abstract
:1. Introduction
2. Theory and Model
2.1. Basic Equation
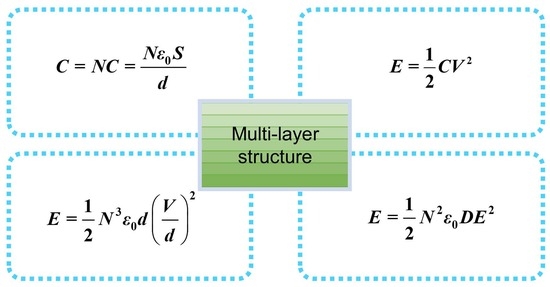
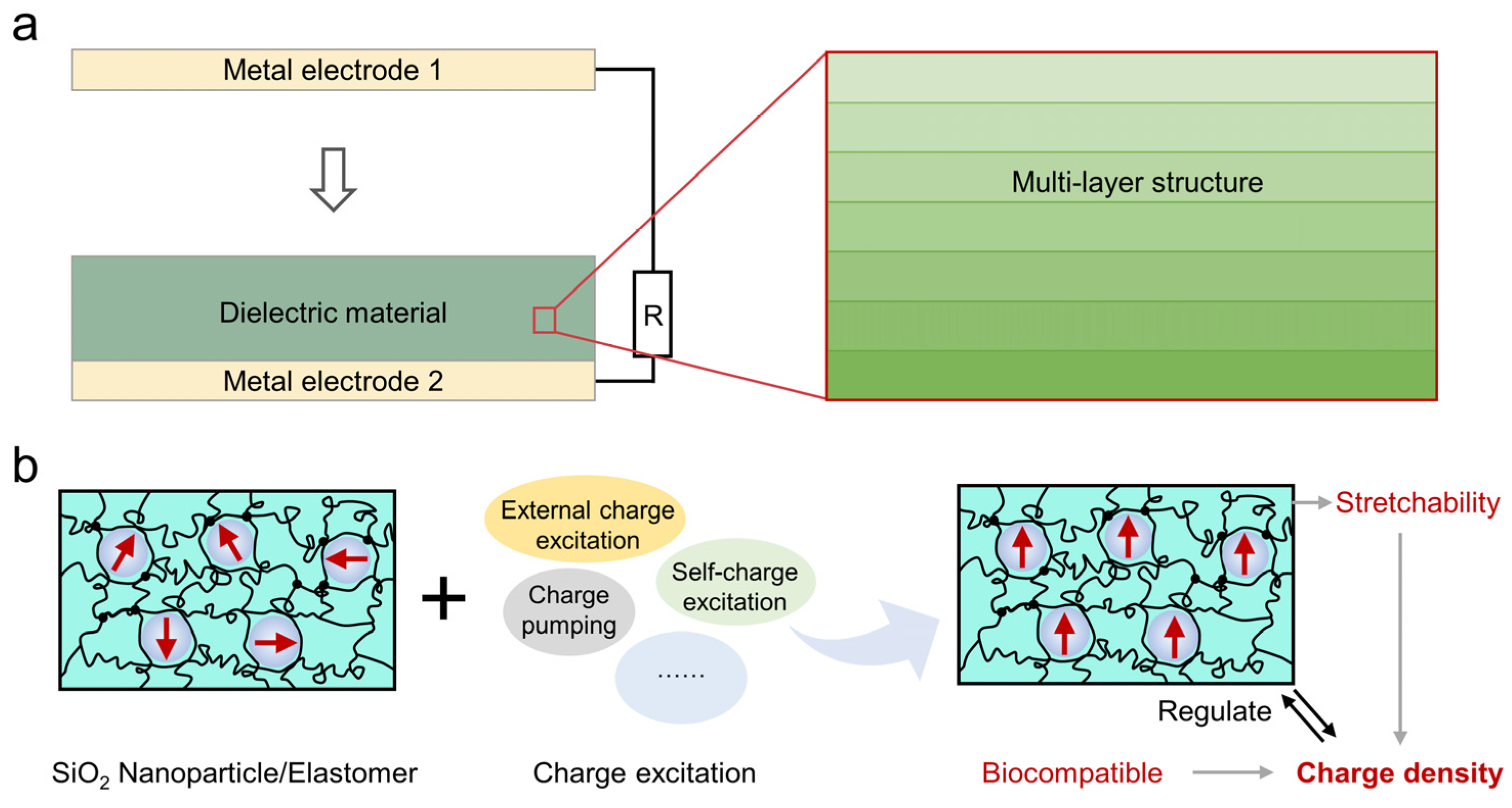
2.2. Triboelectric Nanogenerator Based on Multilayer Triboelectric Materials
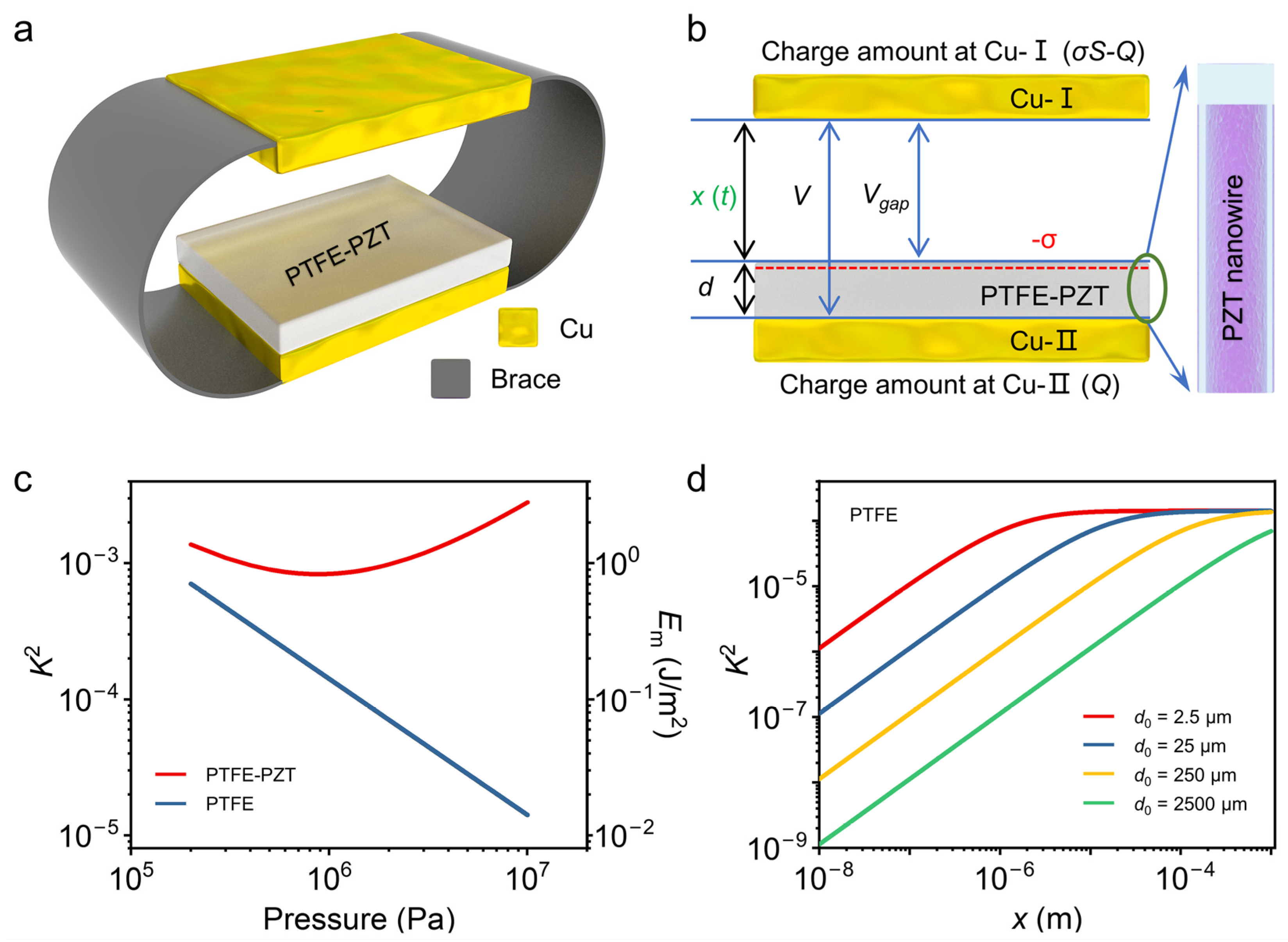
2.3. Electromechanical Coupling Coefficient of the TENG
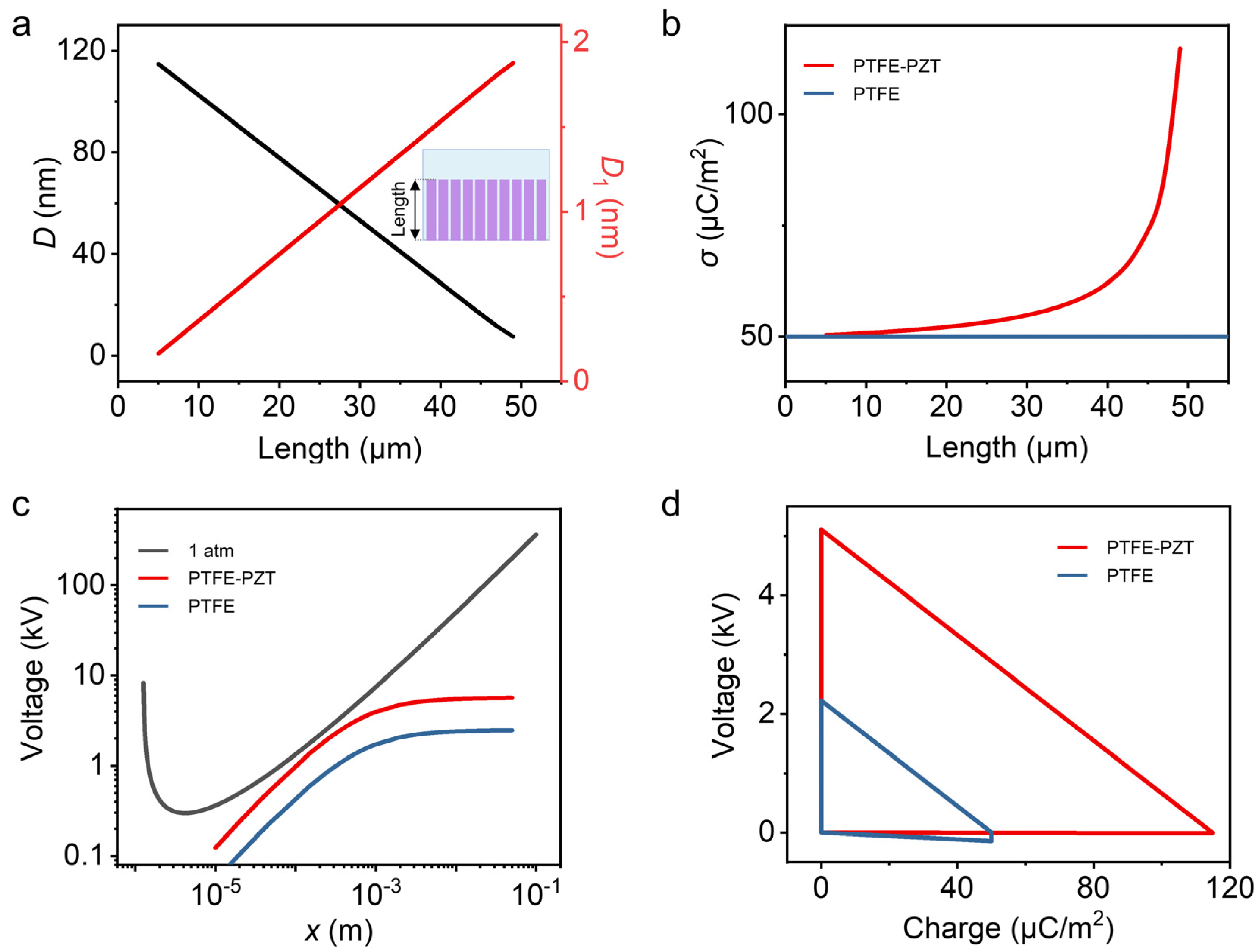
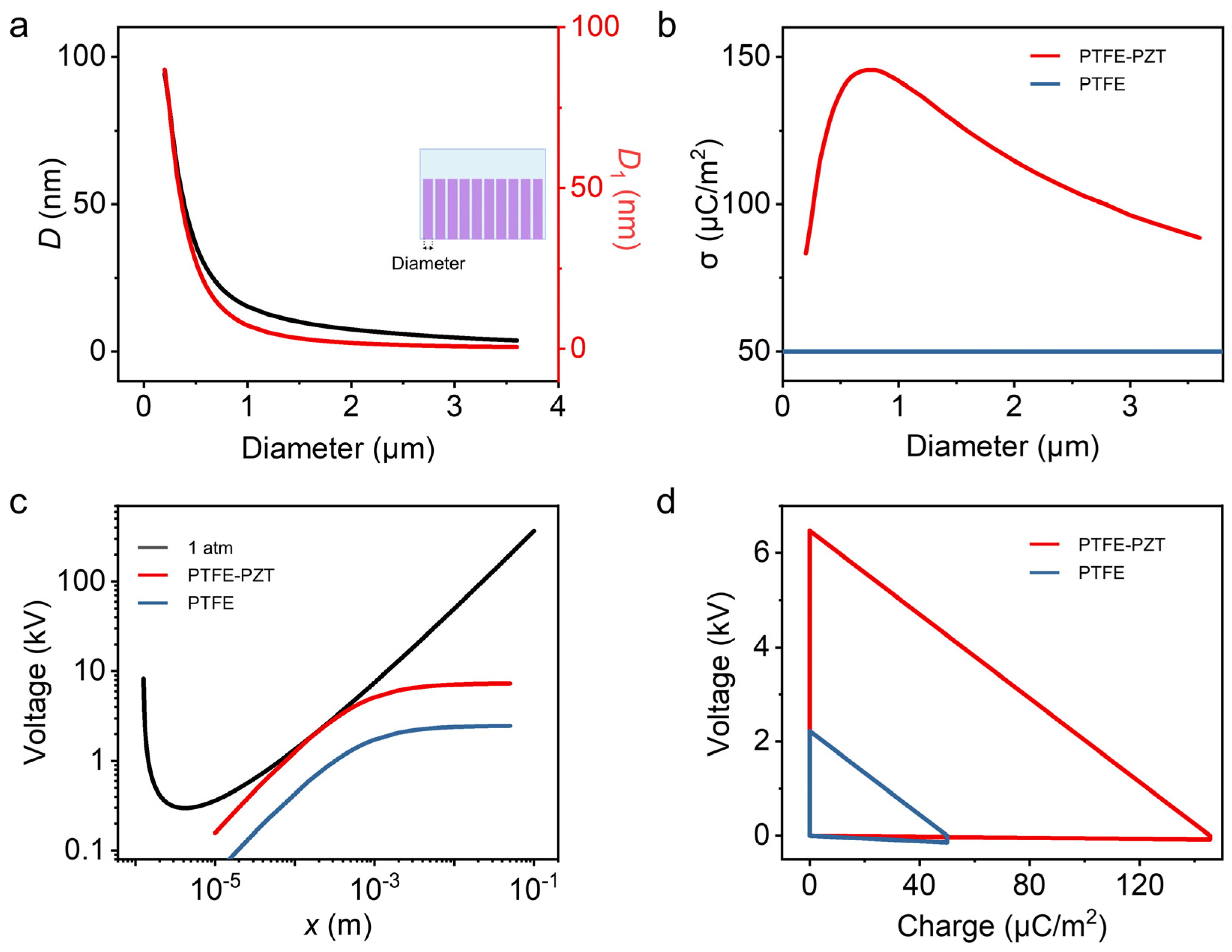
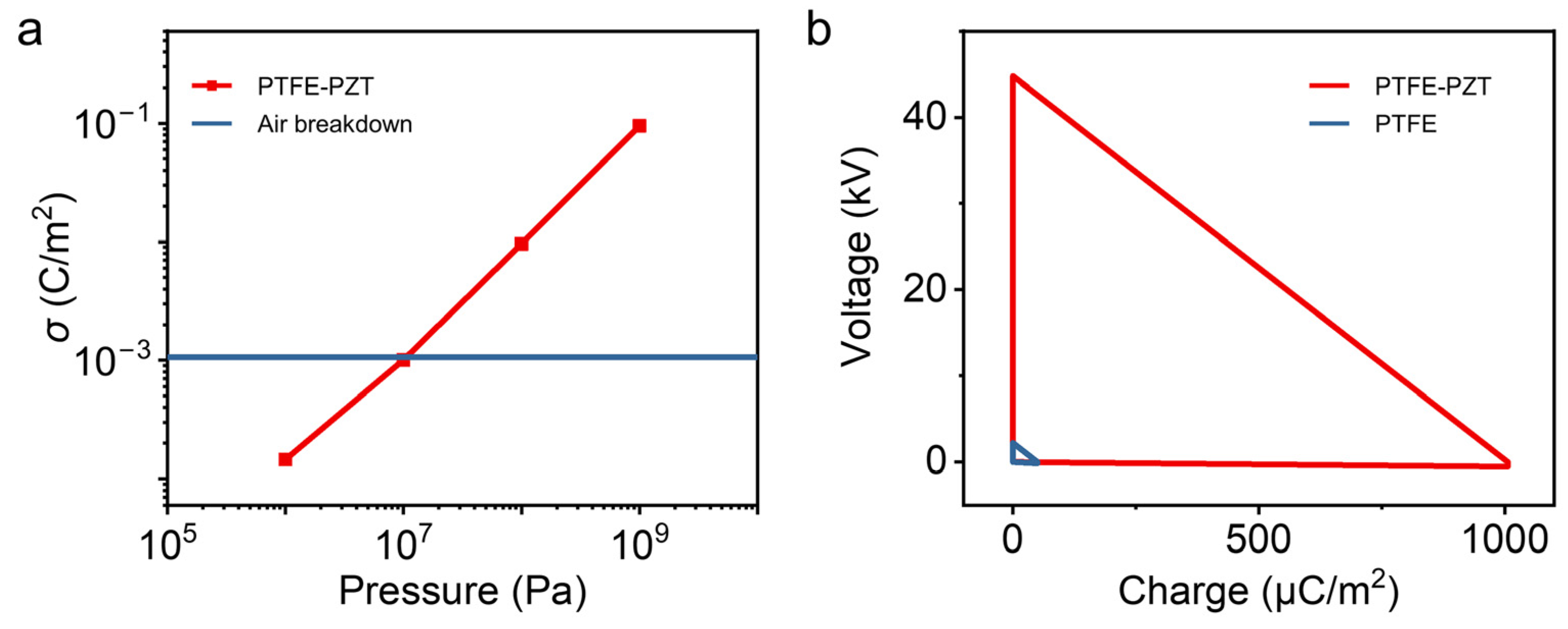
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Cheng, T.; Shao, J.; Wang, Z.L. Triboelectric nanogenerators. Nat. Rev. Methods Primers 2023, 3, 39. [Google Scholar] [CrossRef]
- Won, S.M.; Cai, L.; Gutruf, P.; Rogers, J.A. Wireless and battery-free technologies for neuroengineering. Nat. Biomed. Eng. 2023, 7, 405–423. [Google Scholar] [CrossRef]
- Zheng, Q.; Tang, Q.; Wang, Z.L.; Li, Z. Self-powered cardiovascular electronic devices and systems. Nat. Rev. Cardiol. 2021, 18, 7–21. [Google Scholar] [CrossRef]
- Xu, W.; Zheng, H.; Liu, Y.; Zhou, X.; Zhang, C.; Song, Y.; Deng, X.; Leung, M.; Yang, Z.; Xu, R.X.; et al. A droplet-based electricity generator with high instantaneous power density. Nature 2020, 578, 392–396. [Google Scholar] [CrossRef]
- Zhang, Y.; Lu, Q.; He, J.; Huo, Z.; Zhou, R.; Han, X.; Jia, M.; Pan, C.; Wang, Z.L.; Zhai, J. Localizing strain via micro-cage structure for stretchable pressure sensor arrays with ultralow spatial crosstalk. Nat. Commun. 2023, 14, 1252. [Google Scholar] [CrossRef]
- Qu, X.; Liu, Z.; Tan, P.; Wang, C.; Liu, Y.; Feng, H.; Luo, D.; Li, Z.; Wang, Z.L. Artificial tactile perception smart finger for material identification based on triboelectric sensing. Sci. Adv. 2022, 8, eabq2521. [Google Scholar] [CrossRef]
- Hinchet, R.; Yoon, H.-J.; Ryu, H.; Kim, M.-K.; Choi, E.-K.; Kim, D.-S.; Kim, S.-W. Transcutaneous ultrasound energy harvesting using capacitive triboelectric technology. Science 2019, 365, 491–494. [Google Scholar] [CrossRef]
- Choi, D.; Lee, Y.; Lin, Z.-H.; Cho, S.; Kim, M.; Ao, C.K.; Soh, S.; Sohn, C.; Jeong, C.K.; Lee, J.; et al. Recent Advances in Triboelectric Nanogenerators: From Technological Progress to Commercial Applications. ACS Nano 2023, 17, 11087–11219. [Google Scholar] [CrossRef]
- Zhao, Z.; Dai, Y.; Liu, D.; Zhou, L.; Li, S.; Wang, Z.L.; Wang, J. Rationally patterned electrode of direct-current triboelectric nanogenerators for ultrahigh effective surface charge density. Nat. Commun. 2020, 11, 6186. [Google Scholar] [CrossRef]
- Liu, D.; Gao, Y.; Zhou, L.; Wang, J.; Wang, Z.L. Recent advances in high-performance triboelectric nanogenerators. Nano Res. 2023, 1–20. [Google Scholar] [CrossRef]
- Li, W.; Torres, D.; Díaz, R.; Wang, Z.; Wu, C.; Wang, C.; Lin Wang, Z.; Sepúlveda, N. Nanogenerator-based dual-functional and self-powered thin patch loudspeaker or microphone for flexible electronics. Nat. Commun. 2017, 8, 15310. [Google Scholar] [CrossRef]
- Wang, J.; Li, S.; Yi, F.; Zi, Y.; Lin, J.; Wang, X.; Xu, Y.; Wang, Z.L. Sustainably powering wearable electronics solely by biomechanical energy. Nat. Commun. 2016, 7, 12744. [Google Scholar] [CrossRef]
- Liu, J.X.; Liu, G.; Guo, Z.H.; Hu, W.; Zhang, C.; Pu, X. Electret elastomer-based stretchable triboelectric nanogenerators with autonomously managed power supplies for self-charging systems. Chem. Eng. J. 2023, 462, 142167. [Google Scholar] [CrossRef]
- Zhang, S.; Wang, Y.; Yao, X.; Le Floch, P.; Yang, X.; Liu, J.; Suo, Z. Stretchable Electrets: Nanoparticle–Elastomer Composites. Nano Lett. 2020, 20, 4580–4587. [Google Scholar] [CrossRef]
- Xu, L.; Bu, T.Z.; Yang, X.D.; Zhang, C.; Wang, Z.L. Ultrahigh charge density realized by charge pumping at ambient conditions for triboelectric nanogenerators. Nano Energy 2018, 49, 625–633. [Google Scholar] [CrossRef]
- Liu, W.; Wang, Z.; Wang, G.; Liu, G.; Chen, J.; Pu, X.; Xi, Y.; Wang, X.; Guo, H.; Hu, C.; et al. Integrated charge excitation triboelectric nanogenerator. Nat. Commun. 2019, 10, 1426. [Google Scholar] [CrossRef]
- Cheng, L.; Xu, Q.; Zheng, Y.; Jia, X.; Qin, Y. A self-improving triboelectric nanogenerator with improved charge density and increased charge accumulation speed. Nat. Commun. 2018, 9, 3773. [Google Scholar] [CrossRef]
- Xie, X.; Chen, X.; Zhao, C.; Liu, Y.; Sun, X.; Zhao, C.; Wen, Z. Intermediate layer for enhanced triboelectric nanogenerator. Nano Energy 2021, 79, 105439. [Google Scholar] [CrossRef]
- Cui, N.; Gu, L.; Lei, Y.; Liu, J.; Qin, Y.; Ma, X.; Hao, Y.; Wang, Z.L. Dynamic Behavior of the Triboelectric Charges and Structural Optimization of the Friction Layer for a Triboelectric Nanogenerator. ACS Nano 2016, 10, 6131–6138. [Google Scholar] [CrossRef]
- Lai, M.; Du, B.; Guo, H.; Xi, Y.; Yang, H.; Hu, C.; Wang, J.; Wang, Z.L. Enhancing the Output Charge Density of TENG via Building Longitudinal Paths of Electrostatic Charges in the Contacting Layers. ACS Appl. Mater. Interfaces 2018, 10, 2158–2165. [Google Scholar] [CrossRef]
- Gao, S.; Wang, R.; Ma, C.; Chen, Z.; Wang, Y.; Wu, M.; Tang, Z.; Bao, N.; Ding, D.; Wu, W.; et al. Wearable high-dielectric-constant polymers with core-shell liquid metal inclusions for biomechanical energy harvesting and a self-powered user interface. J. Mater. Chem. A 2019, 7, 7109–7117. [Google Scholar] [CrossRef]
- Xia, X.; Chen, J.; Liu, G.; Javed, M.S.; Wang, X.; Hu, C. Aligning graphene sheets in PDMS for improving output performance of triboelectric nanogenerator. Carbon 2017, 111, 569–576. [Google Scholar] [CrossRef]
- Wang, J.; Wu, C.; Dai, Y.; Zhao, Z.; Wang, A.; Zhang, T.; Wang, Z.L. Achieving ultrahigh triboelectric charge density for efficient energy harvesting. Nat. Commun. 2017, 8, 88. [Google Scholar] [CrossRef] [PubMed]
- Zi, Y.; Niu, S.; Wang, J.; Wen, Z.; Tang, W.; Wang, Z.L. Standards and figure-of-merits for quantifying the performance of triboelectric nanogenerators. Nat. Commun. 2015, 6, 8376. [Google Scholar] [CrossRef]
- Wang, Z.; Cheng, L.; Zheng, Y.; Qin, Y.; Wang, Z.L. Enhancing the performance of triboelectric nanogenerator through prior-charge injection and its application on self-powered anticorrosion. Nano Energy 2014, 10, 37–43. [Google Scholar] [CrossRef]
- Wang, S.; Xie, Y.; Niu, S.; Lin, L.; Liu, C.; Zhou, Y.S.; Wang, Z.L. Maximum surface charge density for triboelectric nanogenerators achieved by ionized-air injection: Methodology and theoretical understanding. Adv. Mater. 2014, 26, 6720–6728. [Google Scholar] [CrossRef]
- Wang, S.; Zi, Y.; Zhou, Y.S.; Li, S.; Fan, F.; Lin, L.; Wang, Z.L. Molecular surface functionalization to enhance the power output of triboelectric nanogenerators. J. Mater. Chem. A 2016, 4, 3728–3734. [Google Scholar] [CrossRef]
- Jeong, C.K.; Baek, K.M.; Niu, S.; Nam, T.W.; Hur, Y.H.; Park, D.Y.; Hwang, G.T.; Byun, M.; Wang, Z.L.; Jung, Y.S.; et al. Topographically-designed triboelectric nanogenerator via block copolymer self-assembly. Nano Lett. 2014, 14, 7031–7038. [Google Scholar] [CrossRef]
- Wang, S.; Lin, L.; Wang, Z.L. Nanoscale triboelectric-effect-enabled energy conversion for sustainably powering portable electronics. Nano Lett. 2012, 12, 6339–6346. [Google Scholar] [CrossRef]
- Tang, W.; Meng, B.; Zhang, H.X. Investigation of power generation based on stacked triboelectric nanogenerator. Nano Energy 2013, 2, 1164–1171. [Google Scholar] [CrossRef]
- Kim, S.; Gupta, M.K.; Lee, K.Y.; Sohn, A.; Kim, T.Y.; Shin, K.S.; Kim, D.; Kim, S.K.; Lee, K.H.; Shin, H.J.; et al. Transparent flexible graphene triboelectric nanogenerators. Adv. Mater. 2014, 26, 3918–3925. [Google Scholar] [CrossRef] [PubMed]
- Lee, K.Y.; Chun, J.; Lee, J.H.; Kim, K.N.; Kang, N.R.; Kim, J.Y.; Kim, M.H.; Shin, K.S.; Gupta, M.K.; Baik, J.M.; et al. Hydrophobic sponge structure-based triboelectric nanogenerator. Adv. Mater. 2014, 26, 5037–5042. [Google Scholar] [CrossRef] [PubMed]
- Mariello, M. Recent Advances on Hybrid Piezo-Triboelectric Bio-Nanogenerators: Materials, Architectures and Circuitry. Nanoenergy Adv. 2022, 2, 64–109. [Google Scholar] [CrossRef]
- Wang, S.; Tong, W.; Li, Y.; Zhang, P.; Liu, Y.; Chen, Y.; Zhang, Y. Contributions of piezoelectricity and triboelectricity to a hydroxyapatite/PVDF–HFP fiber-film nanogenerator. Nano Energy 2023, 105, 108026. [Google Scholar] [CrossRef]
- Lee, L.-H. Dual mechanism for metal-polymer contact electrification. J. Electrost. 1994, 32, 1–29. [Google Scholar] [CrossRef]
- Saurenbach, F.; Wollmann, D.; Terris, B.D.; Diaz, A.F. Force microscopy of ion-containing polymer surfaces: Morphology and charge structure. Langmuir 1992, 8, 1199–1203. [Google Scholar] [CrossRef]
- Niu, S.; Wang, S.; Lin, L.; Liu, Y.; Zhou, Y.S.; Hu, Y.; Wang, Z.L. Theoretical study of contact-mode triboelectric nanogenerators as an effective power source. Energy Environ. Sci. 2013, 6, 3576–3583. [Google Scholar] [CrossRef]
- Wang, J.; Li, G.; Xu, S.; Wu, H.; Fu, S.; Shan, C.; He, W.; Zhao, Q.; Li, K.; Hu, C. Remarkably Enhanced Charge Density of Inorganic Material Via Regulating Contact Barrier Difference and Charge Trapping for Triboelectric Nanogenerator. Adv. Funct. Mater. 2023, 2304221. [Google Scholar] [CrossRef]
- Wu, H.; Wang, J.; He, W.; Shan, C.; Fu, S.; Li, G.; Zhao, Q.; Liu, W.; Hu, C. Ultrahigh output charge density achieved by charge trapping failure of dielectric polymers. Energy Environ. Sci. 2023, 16, 2274–2283. [Google Scholar] [CrossRef]
- Wang, J.; Wu, H.; Wang, Z.; He, W.; Shan, C.; Fu, S.; Du, Y.; Liu, H.; Hu, C. An Ultrafast Self-Polarization Effect in Barium Titanate Filled Poly(Vinylidene Fluoride) Composite Film Enabled by Self-Charge Excitation Triboelectric Nanogenerator. Adv. Funct. Mater. 2022, 32, 2204322. [Google Scholar] [CrossRef]
- Qi, Y.; McAlpine, M.C. Nanotechnology-enabled flexible and biocompatible energy harvesting. Energy Environ. Sci. 2010, 3, 1275–1285. [Google Scholar] [CrossRef]
- Hinchet, R.; Lee, S.; Ardila, G.; Montès, L.; Mouis, M.; Wang, Z.L. Performance Optimization of Vertical Nanowire-based Piezoelectric Nanogenerators. Adv. Funct. Mater. 2014, 24, 971–977. [Google Scholar] [CrossRef]
- Cui, X.; Ni, X.; Zhang, Y. Theoretical study of output of piezoelectric nanogenerator based on composite of PZT nanowires and polymers. J. Alloys Compd. 2016, 675, 306–310. [Google Scholar] [CrossRef]
- Niu, S.; Liu, Y.; Wang, S.; Lin, L.; Zhou, Y.S.; Hu, Y.; Wang, Z.L. Theory of sliding-mode triboelectric nanogenerators. Adv. Mater. 2013, 25, 6184–6193. [Google Scholar] [CrossRef] [PubMed]
- Hu, C.; Cheng, L.; Wang, Z.; Zheng, Y.; Bai, S.; Qin, Y. A Transparent Antipeep Piezoelectric Nanogenerator to Harvest Tapping Energy on Screen. Small 2016, 12, 1315–1321. [Google Scholar] [CrossRef]
- Husain, E.; Nema, R.S. Analysis of Paschen Curves for air, N2 and SF6 Using the Townsend Breakdown Equation. ITEI 1982, 4, 350–353. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cui, X.; Zhang, Y.; Zhang, Y. Multi-Charge Storage Layer Model of High-Charge-Density Triboelectric Nanogenerator. Nanoenergy Adv. 2023, 3, 247-258. https://doi.org/10.3390/nanoenergyadv3030013
Cui X, Zhang Y, Zhang Y. Multi-Charge Storage Layer Model of High-Charge-Density Triboelectric Nanogenerator. Nanoenergy Advances. 2023; 3(3):247-258. https://doi.org/10.3390/nanoenergyadv3030013
Chicago/Turabian StyleCui, Xin, Yaming Zhang, and Yan Zhang. 2023. "Multi-Charge Storage Layer Model of High-Charge-Density Triboelectric Nanogenerator" Nanoenergy Advances 3, no. 3: 247-258. https://doi.org/10.3390/nanoenergyadv3030013
APA StyleCui, X., Zhang, Y., & Zhang, Y. (2023). Multi-Charge Storage Layer Model of High-Charge-Density Triboelectric Nanogenerator. Nanoenergy Advances, 3(3), 247-258. https://doi.org/10.3390/nanoenergyadv3030013