Use of Local Resources in Plant-Based Concretes: Exploring Thermal Performance Through Multi-Scale Modeling
Abstract
:1. Introduction
- -
- -
- The stabilization time in various environments combined with varying thermal conductivities depending on the formulation and external conditions (temperature and ambient relative humidity), make characterization challenging [21].
- -
- -
- Optimizing formulations is complex because reducing material density improves insulation but degrades mechanical properties [29].
- The variability of both the aggregate and the binder to easily study and compare different formulations even before the manufacturing stage.
- A relevant and fast-calculating modeling method to complete and guide time-consuming characterization processes.
- The anisotropic thermal behavior both at the particle and the plant-based concretes scales.
- Different models to link experiment and morphology data to the thermal conductivity of a stabilized plant-based concretes.
2. Materials and Methods
2.1. A Homogenization-Based Effective Thermal Conductivity
2.1.1. Thermal Conductivity Tensor
- Isotropic
- Transversely isotropic
- Orthotropic
2.1.2. Homogenization Schemes
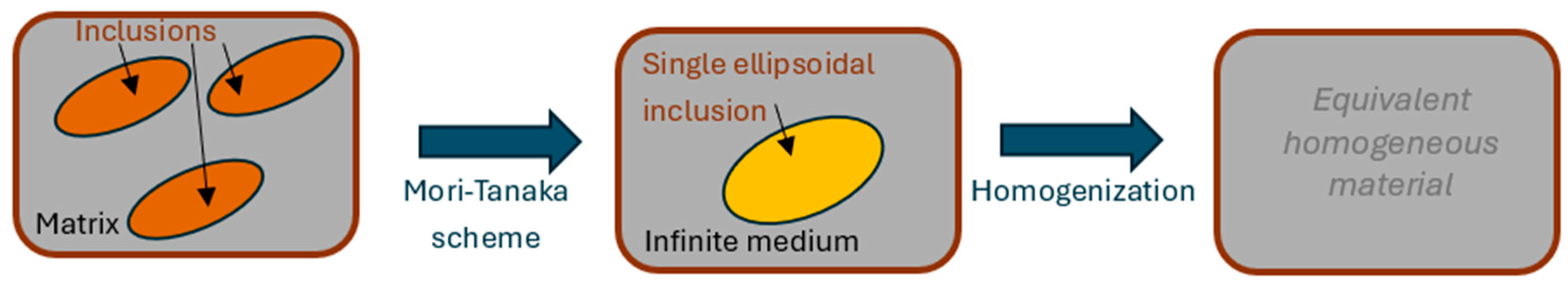
- Mori–Tanaka scheme
- Double inclusion scheme
2.1.3. Representative Volume Equivalent (RVE)
2.1.4. Microstructural Data
- Particulate shape
- Particulate orientation
- Particulate thermal conductivity
2.1.5. Effect of Temperature and Relative Humidity
2.2. Model Validation
2.2.1. Experimental Data
2.2.2. Modeling Input Data
- Particulate thermal conductivity
- Binder thermal conductivity
2.3. Optimization Factors of Effective Thermal Conductivity
2.3.1. Type of Plant Aggregate
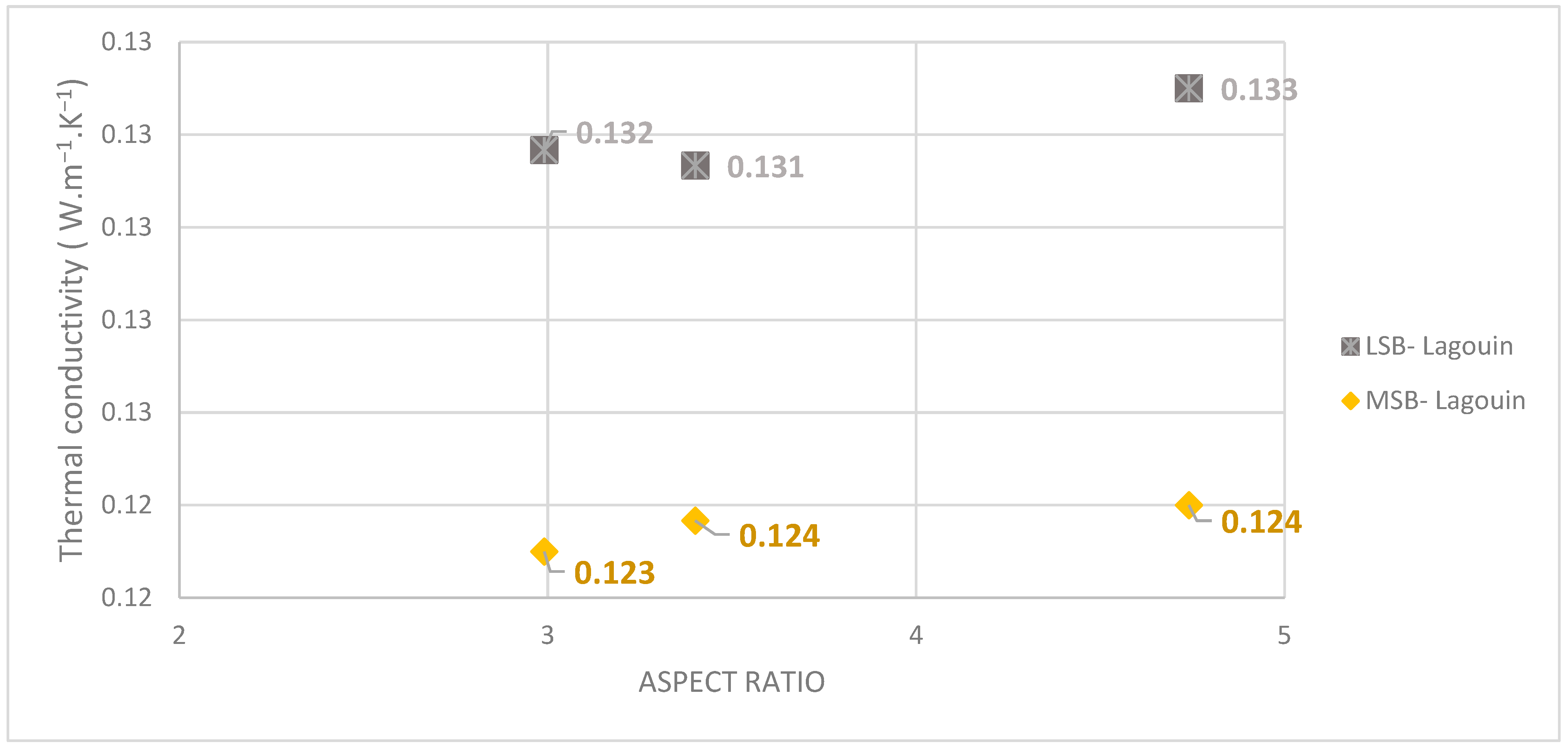
2.3.2. Aspect Ratio
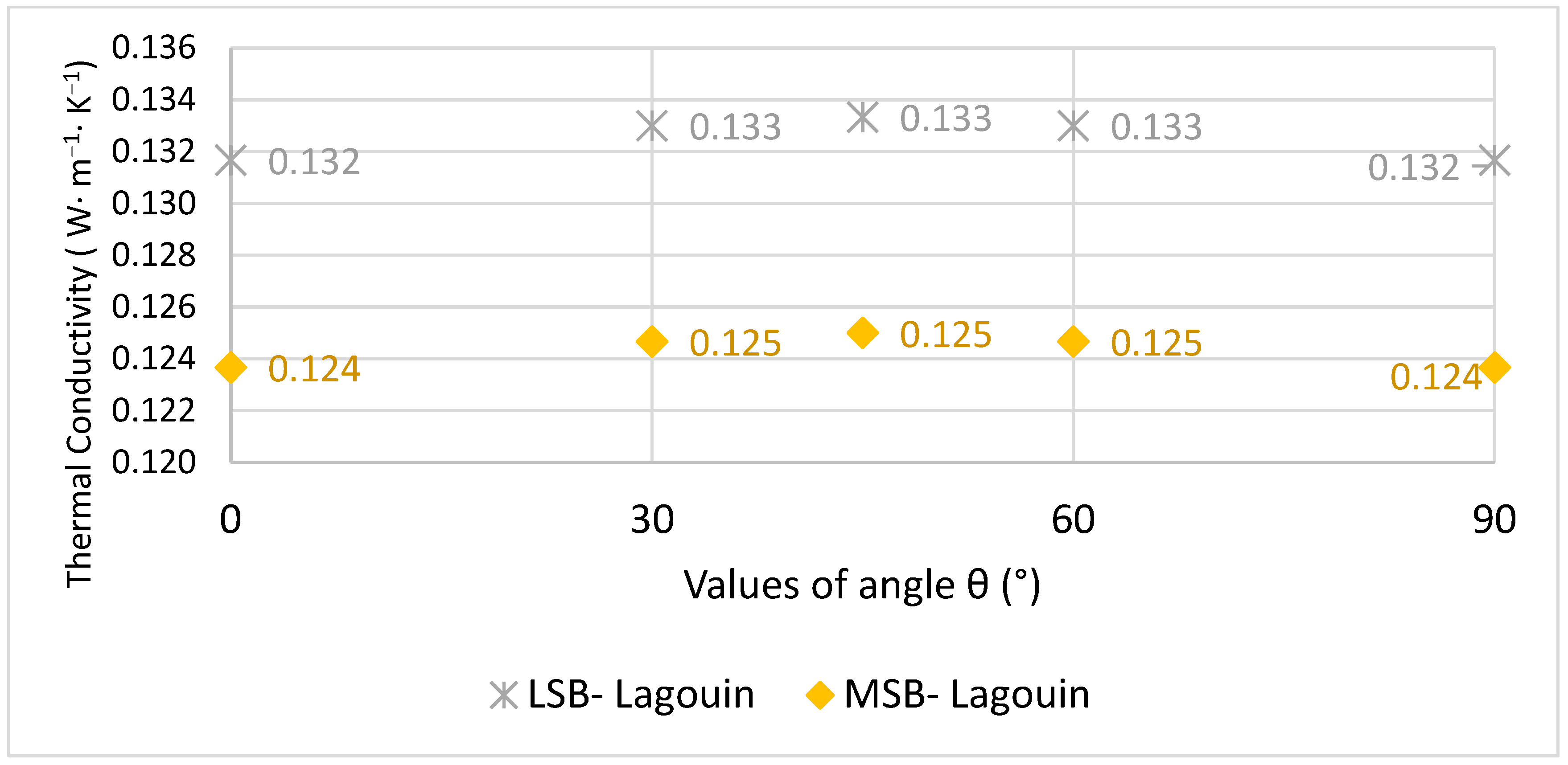
2.3.3. Preferential Orientation
2.3.4. Multi-Criteria Optimization Parameters
- Mechanical aspect
- Hygroscopic aspect
- Resource availability criteria
3. Results and Discussion
3.1. Effective Thermal Conductivity of Plant-Based Concretes Concretes
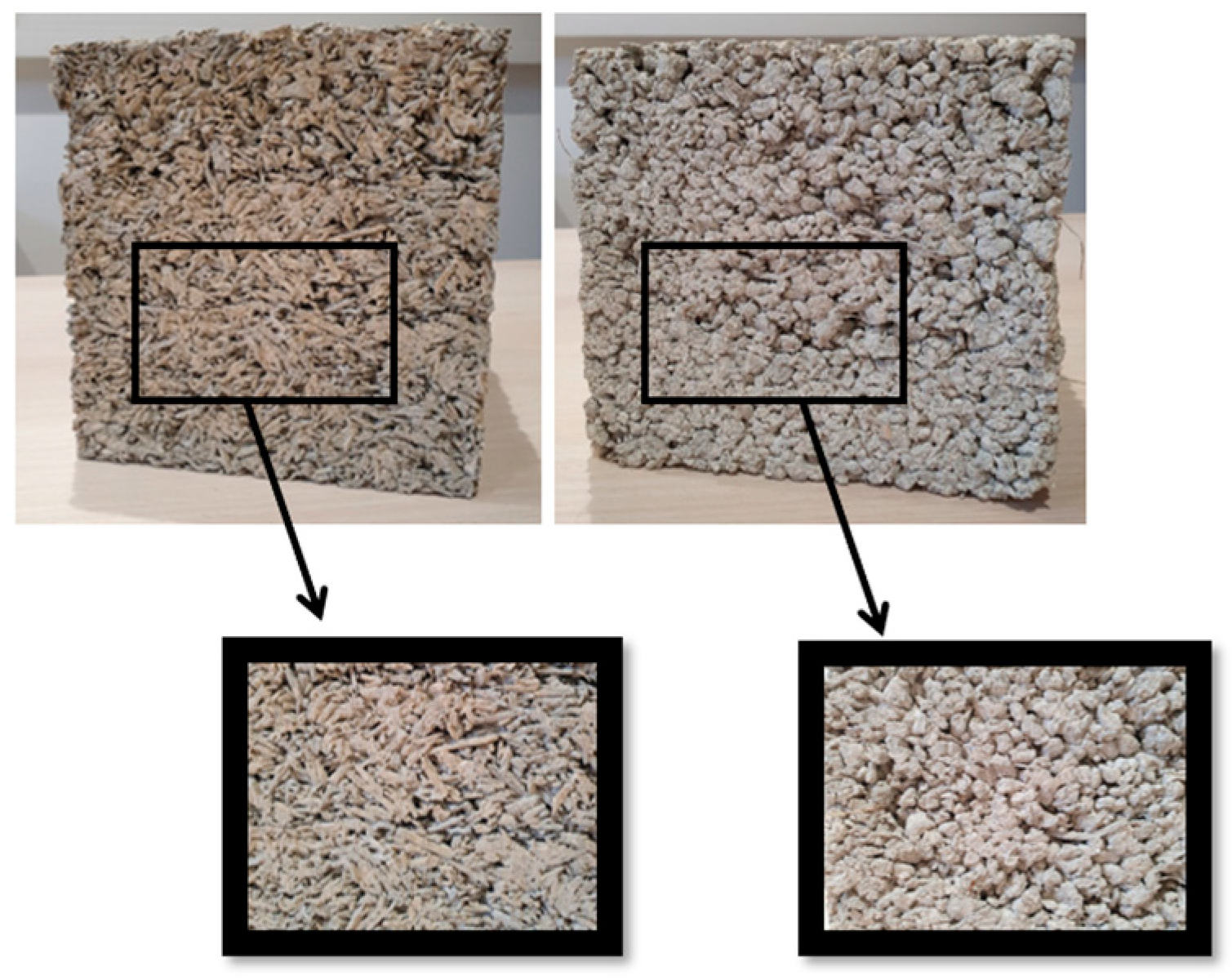
3.1.1. Lime-Based Composite
- -
- RVE HB-1 models: models without additional porosity show a significant relative deviation of over 40% from the experimental target value, indicating their limited relevance.
- -
- RVE HB-2 models: these models exhibit better results with a deviation of around 20% from the experiment value but it follows the trend of experimental data (i.e., predicted data are systematically below the experimental data). This could indicate a bias in the model.
- -
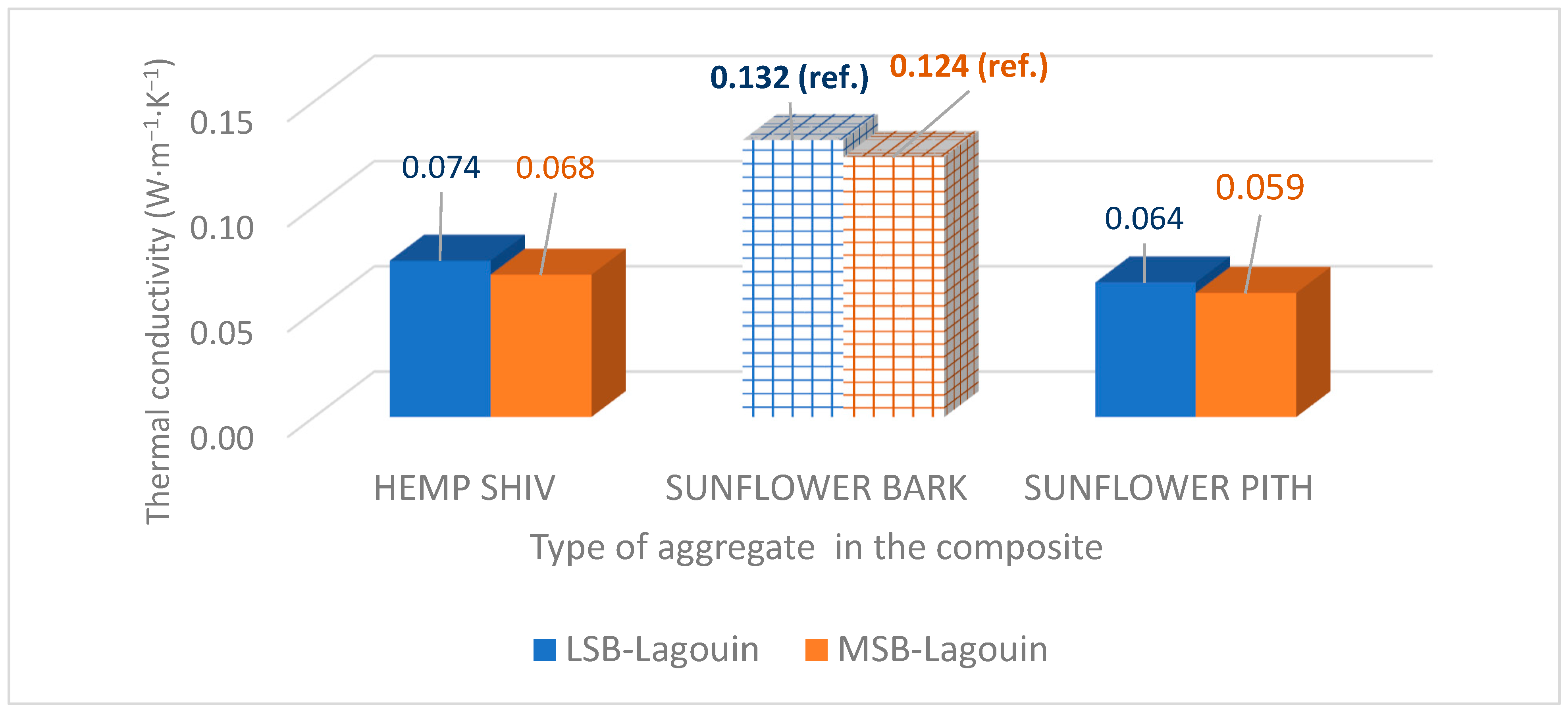
- Double inclusion scheme (HB-3): the most relevant results are obtained with homogenization considering the double inclusion scheme, with a deviation of less than 15% across all components. Given the measurement uncertainty, the thermal conductivity of this composite is estimated between 0.119 W·m−1·K−1 and 0.135 W·m−1·K−1. The predicted values of 0.132 W·m−1·K−1 1 by both the HB-3-3D and HB-3-2D models (averaged) fit within this range.
3.1.2. Metakaolin-Based Composite
- -
- RVE HB-1 models are irrelevant with a relative deviation of over 60% from the experimental target value.
- -
- Composites modeled by RVE HB-2 give unsatisfactory results with a deviation of around 40%, which is significantly below the experimental value.
- -
- Better results are obtained with homogenization using the double inclusion scheme, where both the HB-3-3D and HB-3-2D models show a deviation of less than 5% (considering the average value). Given the measurement uncertainty, this composite has a thermal conductivity value between 0.119 W·m−1·K−1 and 0.137 W·m−1·K−1. So, the values 0.123 and 0.124 W·m−1·K−1 predicted by the MSB-Lagouin-HB-3 models are therefore appropriate. The LMB-Lagouin-HB-3 is consequently retained for the optimization process. Similar conclusions can be drawn for those of the LSB composite concerning the alignment between the chosen model and the material structure, explaining the excellent fit between model and real composite behavior.
3.2. Optimization of Thermal Conductivity
3.2.1. Reference Values and Models
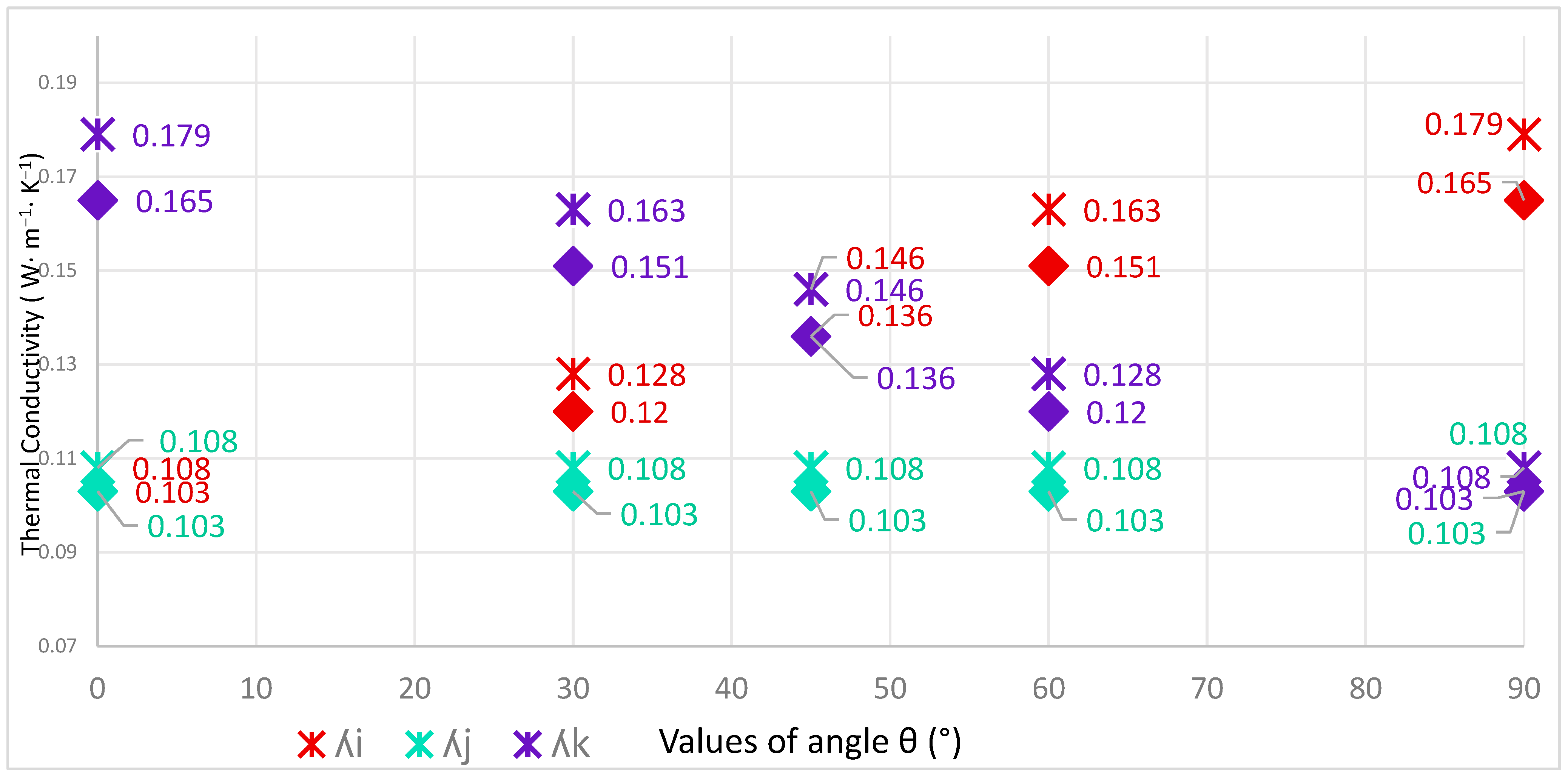
3.2.2. Influence of Aggregate Type
3.2.3. Influence of Aggregate Shape
3.2.4. Influence of Aggregate Orientation
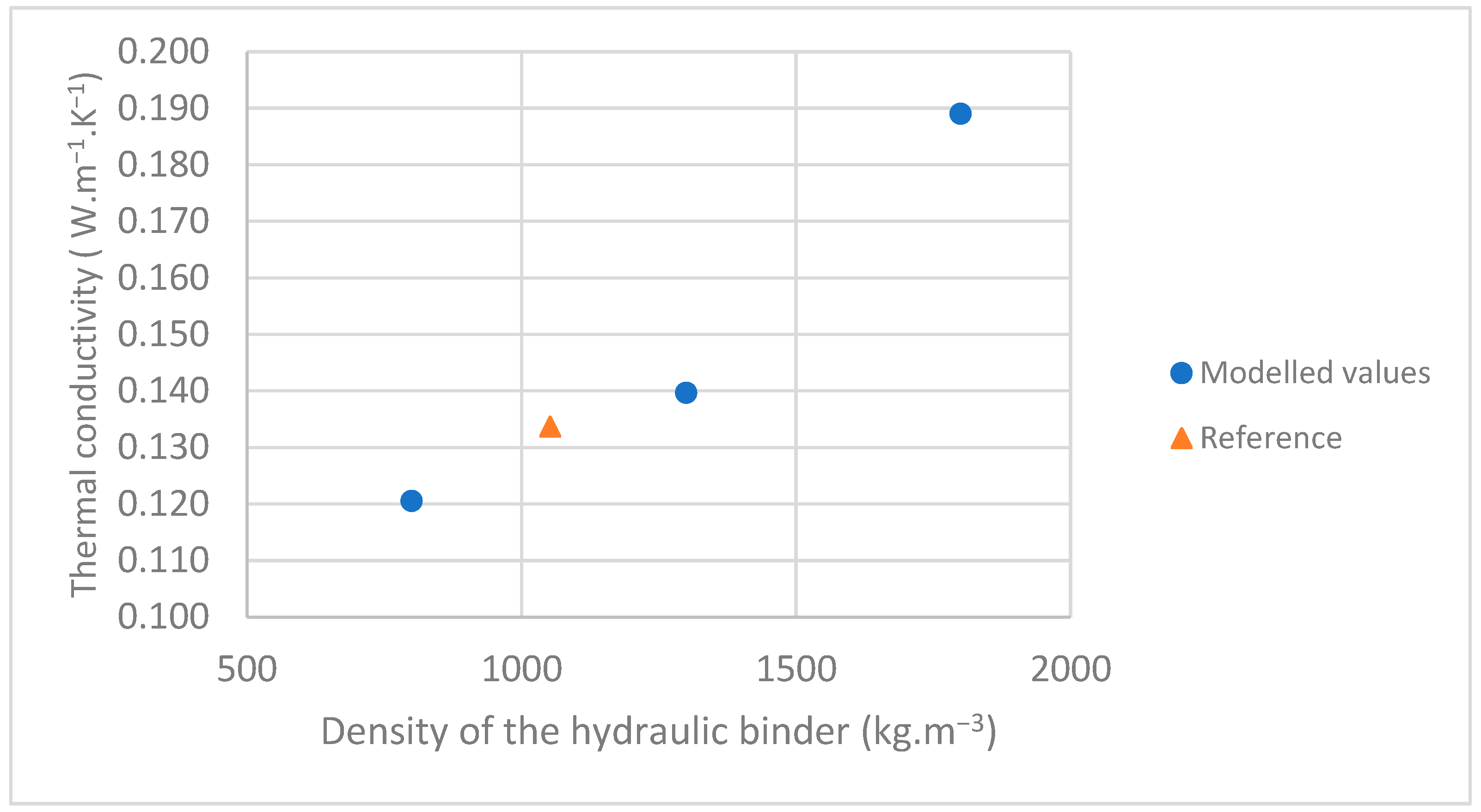
3.2.5. Influence of Binder
3.3. Multi-Criteria Optimization of the Formulation
4. Conclusions
- (i)
- The nature of the aggregate is a fundamental criterion. Replacing sunflower bark with sunflower pith can halve the thermal conductivity of a bio-based composite, especially when the aggregate volume ratio is particularly significant as in plant-based concretes.
- (ii)
- The aggregate shape has a minor impact on the composite thermal conductivity on a macroscopic scale for the same type of aggregate.
- (iii)
- The orientation of plant particles has a significant impact on the thermal conductivity of the plant-based concrete. The difference between normal and tangential components (along or perpendicular to the compaction axis) can be as high as 60%. These results underline that appropriate processing techniques can make a major contribution to optimizing the thermal performance of bio-based materials.
- (iv)
- The impact of a binder change is limited as long as it remains within the same density range, since it has been revealed (from the experimental values) that a polynomial relationship exists between the thermal conductivity of the binder and its density.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Onat, N.C.; Kucukvar, M. Carbon footprint of construction industry: A global review and supply chain analysis. Renew. Sustain. Energy Rev. 2020, 124, 109783. [Google Scholar] [CrossRef]
- Kuittinen, M.; Winter, S. Carbon Footprint of Transitional Shelters. Int. J. Disaster Risk Sci. 2015, 6, 226–237. [Google Scholar] [CrossRef]
- Mouton, L.; Allacker, K.; Röck, M. Bio-based building material solutions for environmental benefits over conventional construction products—Life cycle assessment of regenerative design strategies (1/2). Energy Build. 2022, 282, 112767. [Google Scholar] [CrossRef]
- Dams, B.; Maskell, D.; Shea, A.; Allen, S.; Cascione, V.; Walker, P. Upscaling bio-based construction: Challenges and opportunities. Build. Res. Inf. 2023, 51, 764–782. [Google Scholar] [CrossRef]
- Nguyen, T.T. Contribution à L’étude de la Formulation et du Procédé de Fabrication D’éléments de Construction en Béton de Chanvre. Ph.D. Thesis, Université de Bretagne Sud, Lorient, France, 2010. [Google Scholar]
- Prud’homme, E.; Delhomme, F.; Julliot, C.; Corvalan, L.; Amziane, S.; Toussaint, E.; Marceau, S. A New Experimental Setup to Characterize Binder–Vegetal Particle Compatibility in Plant-Based Concrete. Buildings 2024, 14, 1000. [Google Scholar] [CrossRef]
- Walker, R.; Pavía, S. Moisture transfer and thermal properties of hemp–lime concretes. Constr. Build. Mater. 2014, 64, 270–276. [Google Scholar] [CrossRef]
- Abbas, M.S.; Gourdon, E.; Glé, P.; McGregor, F.; Ferroukhi, M.Y.; Fabbri, A. Relationship between hygrothermal and acoustical behavior of hemp and sunflower composites. Build. Environ. 2021, 188, 107462. [Google Scholar] [CrossRef]
- Seng, B.; Lorente, S.; Magniont, C. Scale analysis of heat and moisture transfer through bio-based materials—Application to hemp concrete. Energy Build. 2017, 155, 546–558. [Google Scholar] [CrossRef]
- Raja, P.; Murugan, V.; Ravichandran, S.; Behera, L.; Mensah, R.A.; Mani, S.; Kasi, A.; Balasubramanian, K.B.N.; Sas, G.; Vahabi, H.; et al. A Review of Sustainable Bio-Based Insulation Materials for Energy-Efficient Buildings. Macromol. Mater. Eng. 2023, 308, 2300086. [Google Scholar] [CrossRef]
- Lagouin, M.; Magniont, C.; Sénéchal, P.; Moonen, P.; Aubert, J.-E.; Laborel-Préneron, A. Influence of types of binder and plant aggregates on hygrothermal and mechanical properties of vegetal concretes. Constr. Build. Mater. 2019, 222, 852–871. [Google Scholar] [CrossRef]
- Glé, P.; Lecompte, T.; de Ménibus, A.H.; Lenormand, H.; Arufe, S.; Chateau, C.; Fierro, V.; Celzard, A. Densities of hemp shiv for building: From multiscale characterisation to application. Ind. Crop. Prod. 2021, 164, 113390. [Google Scholar] [CrossRef]
- Lagouin, M.; Sénéchal, P.; Moonen, P.; Magniont, C.; Aubert, J.-E.E.; Laborel-Préneron, A. Potential of X-ray tomography for the exploration of vegetal concretes’ porous structure. In Proceedings of the 3rd International Conference on Bio-Based Building Materials (ICBBM), Belfast, UK, 26–28 June 2019; Available online: https://hal.science/hal-02175817 (accessed on 3 April 2023).
- Gacoin, A.; Li, A. Optimal composition of a starch-hemp agro-composite materials. Constr. Build. Mater. 2023, 400, 132711. [Google Scholar] [CrossRef]
- Latapie, S.R.; Lagouin, M.; Douk, N.; Sabathier, V.; Abou-Chakra, A. Multiscale Modelling of Bio-composites: Towards Prediction of Their Thermal Conductivity Based on Adequate Knowledge of Their Constituents. In Bio-Based Building Materials; Amziane, S., Merta, I., Page, J., Eds.; RILEM Bookseries; Springer Nature: Cham, Switzerland, 2023; pp. 841–858. [Google Scholar] [CrossRef]
- Gourlay, E.; Arnaud, L. Comportement hygrothermique des murs de béton de Chanvre. In Proceedings of the Actes du Congrès SFT, Le Touquet, France, May 2010. [Google Scholar]
- Abbas, M.S. Caractérisations Multi-Physiques des Mortiers Bio-Sourcés Isolants et Modélisation de Leurs Impacts sur les Transferts Hygrothermiques à L’échelle des Parois: Application aux Bétons de Moelles Végétales. Ph.D. Thesis, Université de Lyon, Lyon, France, 2021. [Google Scholar]
- Seng, B.; Magniont, C.; Lorente, S. Characterization of a precast hemp concrete. Part I: Physical and thermal properties. J. Build. Eng. 2019, 24, 100540. [Google Scholar] [CrossRef]
- Collet, F.; Pretot, S. Thermal conductivity of hemp concretes: Variation with formulation, density and water content. Constr. Build. Mater. 2014, 65, 612–619. [Google Scholar] [CrossRef]
- Laborel-Préneron, A.; Magniont, C.; Aubert, J.-E. Hygrothermal properties of unfired earth bricks: Effect of barley straw, hemp shiv and corn cob addition. Energy Build. 2018, 178, 265–278. [Google Scholar] [CrossRef]
- Frantz, N.; Dutra, L.F.; Nguyen, D.M.; Almeida, G.; Perré, P. Effects of phase ratios, density and particle shapes on directional thermal conductivity of vegetable concrete: A predictive model. Constr. Build. Mater. 2023, 410, 134238. [Google Scholar] [CrossRef]
- Tran-Le, A.D.; Nguyen, S.-T.; Langlet, T. A novel anisotropic analytical model for effective thermal conductivity tensor of dry lime-hemp concrete with preferred spatial distributions. Energy Build. 2018, 182, 75–87. [Google Scholar] [CrossRef]
- Huang, G.; Abou-Chakra, A.; Geoffroy, S.; Absi, J. A Multi-Scale Numerical Simulation on Thermal Conductivity of Bio-Based Construction Materials. Constr. Mater. 2022, 2, 148–165. [Google Scholar] [CrossRef]
- Avellaneda, A.; Evon, P.; Haurie, L.; Laborel-Préneron, A.; Lagouin, M.; Magniont, C.; Navarro, A.; Palumbo, M.; Torres, A. Evaluation of the Potential of Plant Aggregates from Corn and Sunflower Stalks for the Design of Building Materials. In Bio-Based Building Materials; Amziane, S., Merta, I., Page, J., Eds.; RILEM Bookseries; Springer Nature: Cham, Switzerland, 2023; pp. 70–86. [Google Scholar] [CrossRef]
- Collet, F. Matériaux Bio-Sourcés à Hautes Performances Hygrothermiques: Caractérisation Expérimentale de L’échelle du Matériau à L’échelle de la Paroi. HDR Thesis, Université de Rennes, Rennes, France, 2018. [Google Scholar]
- Pierre, T.; Carin, M.; Courtois, M.; Carré, P. Transient infrared thermography to characterise thermal properties of millimetre-sized hemp shiv. Quant. Infrared Thermogr. J. 2019, 17, 63–77. [Google Scholar] [CrossRef]
- Dartois, S.; Mom, S.; Dumontet, H.; Ben Hamida, A. An iterative micromechanical modeling to estimate the thermal and mechanical properties of polydisperse composites with platy particles: Application to anisotropic hemp and lime concretes. Constr. Build. Mater. 2017, 152, 661–671. [Google Scholar] [CrossRef]
- Jami, T.; Karade, S.R.; Singh, L.P. A review of the properties of hemp concrete for green building applications. J. Clean. Prod. 2019, 239, 117852. [Google Scholar] [CrossRef]
- Hussain, A.; Calabria-Holley, J.; Lawrence, M.; Jiang, Y. Hygrothermal and mechanical characterisation of novel hemp shiv based thermal insulation composites. Constr. Build. Mater. 2019, 212, 561–568. [Google Scholar] [CrossRef]
- Latapie, S.R.; Lagouin, M.; Sabathier, V.; Abou-Chakra, A. From aggregate to particleboard: A new multi-scale model approach to thermal conductivity in bio-based materials. J. Build. Eng. 2023, 78, 107664. [Google Scholar] [CrossRef]
- Nguyen, S.; Tran-Le, A.; Vu, M.; To, Q.; Douzane, O.; Langlet, T. Modeling thermal conductivity of hemp insulation material: A multi-scale homogenization approach. Build. Environ. 2016, 107, 127–134. [Google Scholar] [CrossRef]
- Collet, F.; Chamoin, J.; Pretot, S.; Lanos, C. Comparison of the hygric behaviour of three hemp concretes. Energy Build. 2013, 62, 294–303. [Google Scholar] [CrossRef]
- Bennai, F.; El Hachem, C.; Abahri, K.; Belarbi, R. Microscopic hydric characterization of hemp concrete by X-ray microtomography and digital volume correlation. Constr. Build. Mater. 2018, 188, 983–994. [Google Scholar] [CrossRef]
- Eshelby, J.D. The determination of the elastic field of an ellipsoidal inclusion, and related problems. Proc. R. Soc. Lond. Ser. A. Math. Phys. Sci. 1957, 241, 376–396. [Google Scholar] [CrossRef]
- Huang, G.; Abou-Chakra, A.; Geoffroy, S.; Absi, J. A multiscale homogenization model on thermal conductivity of bio-based building composite considering anisotropy, imperfect interface and moisture. Constr. Build. Mater. 2023, 377, 131156. [Google Scholar] [CrossRef]
- Laborel-Préneron, A.; Aubert, J.-E.; Magniont, C.; Maillard, P.; Poirier, C. Effect of Plant Aggregates on Mechanical Properties of Earth Bricks. J. Mater. Civ. Eng. 2017, 29, 04017244. [Google Scholar] [CrossRef]
- Latapie, S.R.; Sabathier, V.; Abou-Chakra, A. Bio-based building materials: A prediction of insulating properties for a wide range of agricultural by-products. J. Build. Eng. 2024, 86, 108867. [Google Scholar] [CrossRef]
- Hori, M.; Nemat-Nasser, S. Double-inclusion model and overall moduli of multi-phase composites. Mech. Mater. 1993, 14, 189–206. [Google Scholar] [CrossRef]
- Lu, P.; Leong, Y.; Pallathadka, P.; He, C. Effective moduli of nanoparticle reinforced composites considering interphase effect by extended double-inclusion model—Theory and explicit expressions. Int. J. Eng. Sci. 2013, 73, 33–55. [Google Scholar] [CrossRef]
- Chabriac, P.; Gourdon, E.; Gle, P.; Fabbri, A.; Lenormand, H. Agricultural by-products for building insulation: Acoustical characterization and modeling to predict micro-structural parameters. Constr. Build. Mater. 2016, 112, 158–167. [Google Scholar] [CrossRef]
- Magniont, C.; Escadeillas, G.; Coutand, M.; Oms-Multon, C. Use of plant aggregates in building ecomaterials. Eur. J. Environ. Civ. Eng. 2012, 16, s17–s33. [Google Scholar] [CrossRef]
- Arufe, S.; de Menibus, A.H.; Leblanc, N.; Lenormand, H. Physico-chemical characterisation of plant particles with potential to produce biobased building materials. Ind. Crop. Prod. 2021, 171, 113901. [Google Scholar] [CrossRef]
- Ratsimbazafy, H.H. Évaluation du Potentiel de Co-Produits Agricoles Locaux Valorisables dans le Domaine des Matériaux de Construction (PALOMAC). Ph.D. Thesis, Paul Sabatier University, Toulouse, France, 2022. [Google Scholar]
- Samri, D. Analyse Physique et Caractérisation Hygrothermique des Matériaux de Construction: Approche Expérimentale et Modélisation Numérique. Ph.D. Thesis, Lyon INSA, Villeurbanne, France, 2008. [Google Scholar]
- Cérézo, V. Propriétés Mécaniques, Thermiques et Acoustiques d’un Matériau à Base de Particules Végétales: Approche Expérimentale et Modélisation Théorique. Ph.D. Thesis, Lyon INSA, Lyon, France, 2005. [Google Scholar]
- Černý, R.; Kunca, A.; Tydlitát, V.; Drchalová, J.; Rovnaníková, P. Effect of pozzolanic admixtures on mechanical, thermal and hygric properties of lime plasters. Constr. Build. Mater. 2005, 20, 849–857. [Google Scholar] [CrossRef]
- Magniont, C. Contribution à la Formulation et à la Caractérisation d’un Écomatériau de Construction à Base D’agroressources. Ph.D. Thesis, Paul Sabatier University, Toulouse, France, 2010. [Google Scholar]
- Vejmelková, E.; Keppert, M.; Rovnaníková, P.; Keršner, Z.; Černý, R. Application of burnt clay shale as pozzolan addition to lime mortar. Cem. Concr. Compos. 2012, 34, 486–492. [Google Scholar] [CrossRef]
- Dinh, T.M. Contribution au Développement de Béton de Chanvre Préfabriqué Utilisant un Liant Pouzzolanique Innovant. Ph.D. Thesis, Paul Sabatier University, Toulouse, France, 2014. [Google Scholar]
- Fehrmann, J.; Belleville, B.; Ozarska, B.; Gutowski, W.S.; Wilson, D. Influence of particle granulometry and panel composition on the physico-mechanical properties of ultra-low-density hemp hurd particleboard. Polym. Compos. 2023, 44, 7363–7383. [Google Scholar] [CrossRef]
- Williams, J.; Lawrence, M.; Walker, P. The influence of constituents on the properties of the bio-aggregate composite hemp-lime. Constr. Build. Mater. 2018, 159, 9–17. [Google Scholar] [CrossRef]
- de Toulouse, I.U.; Ratsimbazafy, H.H.; Laborel-Préneron, A.; Magniont, C.; Evon, P. A Review of the Multi-Physical Characteristics of Plant Aggregates and Their Effects on the Properties of Plant-Based Concrete. Recent Prog. Mater. 2021, 3, 1–69. [Google Scholar] [CrossRef]
- Amziane, S.; da Gloria, M.Y.R. Mechanical behavior of bio-aggregates based buildings materials. RILEM Tech. Lett. 2024, 9, 87–92. [Google Scholar] [CrossRef]
- Laborel-Preneron, A. Formulation and characterization of unfired clay bricks with plant aggregates. Ph.D. Thesis, Paul Sabatier University, Toulouse, France, 2017. [Google Scholar]
- Sáez-Pérez, M.; Brümmer, M.; Durán-Suárez, J. A review of the factors affecting the properties and performance of hemp aggregate concretes. J. Build. Eng. 2020, 31, 101323. [Google Scholar] [CrossRef]
- Ntimugura, F.; Vinai, R.; Harper, A.; Walker, P. Mechanical, thermal, hygroscopic and acoustic properties of bio-aggregates—Lime and alkali—Activated insulating composite materials: A review of current status and prospects for miscanthus as an innovative resource in the South West of England. Sustain. Mater. Technol. 2020, 26, e00211. [Google Scholar] [CrossRef]
- Stevulova, N.; Kidalova, L.; Cigasova, J.; Junak, J.; Sicakova, A.; Terpakova, E. Lightweight Composites Containing Hemp Hurds. Procedia Eng. 2013, 65, 69–74. [Google Scholar] [CrossRef]
- Majstorović, F.; Sebera, V.; Mrak, M.; Dolenec, S.; Wolf, M.; Marrot, L. Impact of metakaolin on mechanical performance of flax textile-reinforced cement-based composites. Cem. Concr. Compos. 2022, 126, 104367. [Google Scholar] [CrossRef]
- Mary, F.S.; Nithambigai, G.; Rameshwaran, P. Effect of binders and hemp fibre in concrete. Mater. Today Proc. 2021, 46, 3791–3794. [Google Scholar] [CrossRef]
- Rahim, M.; Douzane, O.; Tran Le, A.; Promis, G.; Langlet, T. Characterization and comparison of hygric properties of rape straw concrete and hemp concrete. Constr. Build. Mater. 2016, 102, 679–687. [Google Scholar] [CrossRef]
- Prétot, F.C.S. Effect of coating on moisture buffering of hemp concrete. In Proceedings of the Second International Conference on Building Energy and Environment, Boulder, CO, USA, 1–4 August 2012; pp. 878–885. [Google Scholar]
- Abbas, M.S.; McGregor, F.; Fabbri, A.; Ferroukhi, M.Y. The use of pith in the formulation of lightweight bio-based composites: Impact on mechanical and hygrothermal properties. Constr. Build. Mater. 2020, 259, 120573. [Google Scholar] [CrossRef]
- Lenormand, H.; Mahieu, A.; Leblanc, N.; Vivet, A. Nouvelles agroressources pour panneaux de particules 100% biosourcés. In Proceedings of the Conférence Matériaux 2014-Colloque Ecomatériau, Montpellier, France, 24–28 November 2014. [Google Scholar]
- Meglin, R.; Kytzia, S.; Habert, G. Regional circular economy of building materials: Environmental and economic assessment combining Material Flow Analysis, Input-Output Analyses, and Life Cycle Assessment. J. Ind. Ecol. 2022, 26, 562–576. [Google Scholar] [CrossRef]
- Debaeke, P.; Casadebaig, P.; Flenet, F.; Langlade, N. Sunflower crop and climate change: Vulnerability, adaptation, and mitigation potential from case-studies in Europe. OCL Oilseeds Fats Crops Lipids 2017, 24, D102. [Google Scholar] [CrossRef]
- Carus, M.; Karst, S.; Kauffmann, A.; Hobson, J.; Bertucelli, S. The european hemp industry: Cultivation, processing and applications for fibres, shives and seeds. Eur. Ind. Hemp Assoc. 2013, 2013, 1–9. [Google Scholar]
- van Dortmont, D.A. The Potential of Nature-inclusive Cultivation as a Source of Biobased Materials. Master’s Thesis, TU Delft, Delft, The Netherlands, 2024. [Google Scholar]
- FAO. World Food and Agriculture—Statistical Yearbook 2021; FAO: Rome, Italy, 2021. [Google Scholar] [CrossRef]
- Schils, R.; Olesen, J.E.; Kersebaum, K.-C.; Rijk, B.; Oberforster, M.; Kalyada, V.; Khitrykau, M.; Gobin, A.; Kirchev, H.; Manolova, V.; et al. Cereal yield gaps across Europe. Eur. J. Agron. 2018, 101, 109–120. [Google Scholar] [CrossRef]
- Sun, S.; Mathias, J.-D.; Toussaint, E.; Grédiac, M. Hygromechanical characterization of sunflower stems. Ind. Crop. Prod. 2013, 46, 50–59. [Google Scholar] [CrossRef]
- Huang, G.; Abou-Chakra, A.; Absi, J.; Geoffroy, S. Optimization of mechanical properties in anisotropic bio-based building materials by a multiscale homogenization model. J. Build. Eng. 2022, 57, 104890. [Google Scholar] [CrossRef]
- Dinh, T.M.; Magniont, C.; Coutand, M.; Escadeillas, G. Hemp concrete using innovative pozzolanic binder. Acad. J. Civ. Eng. 2015, 33, 265–270. [Google Scholar] [CrossRef]
- Nozahic, V.; Amziane, S.; Torrent, G.; Saïdi, K.; De Baynast, H. Design of green concrete made of plant-derived aggregates and a pumice–lime binder. Cem. Concr. Compos. 2012, 34, 231–241. [Google Scholar] [CrossRef]
- Nguyen, T.T.; Picandet, V.; Carre, P.; Lecompte, T.; Amziane, S.; Baley, C. Effect of compaction on mechanical and thermal properties of hemp concrete. Eur. J. Environ. Civ. Eng. 2010, 14, 545–560. [Google Scholar] [CrossRef]
- Younes, A.Y.; Youssef, A. Prise en Compte des Apports Mécaniques du Béton de Chanvre Pour le Calcul de Structure Bois/Béton de Chanvre et Métal/Béton de Chanvre. Ph.D. Thesis, Université de Bretagne Sud, Lorient, France, 2017. [Google Scholar]
- Williams, J.; Lawrence, M.; Walker, P. The influence of the casting process on the internal structure and physical properties of hemp-lime. Mater. Struct. 2016, 50, 108. [Google Scholar] [CrossRef]
- Niyigena, C.; Amziane, S.; Chateauneuf, A. Etude de la variabilité des caractéristiques de granulats de chanvre. In Proceedings of the Rencontres Universitaires de Génie Civil, Bayonne, France, 26 May 2015. [Google Scholar]
- Achour, C.; Remond, S.; Belayachi, N. Swelling and shrinkage of plant aggregates: Experimental and treatment effect. Ind. Crop. Prod. 2023, 203, 117173. [Google Scholar] [CrossRef]
- Biedermann, A.; Taroni, F. Bayesian networks and probabilistic reasoning about scientific evidence when there is a lack of data. Forensic Sci. Int. 2006, 157, 163–167. [Google Scholar] [CrossRef]



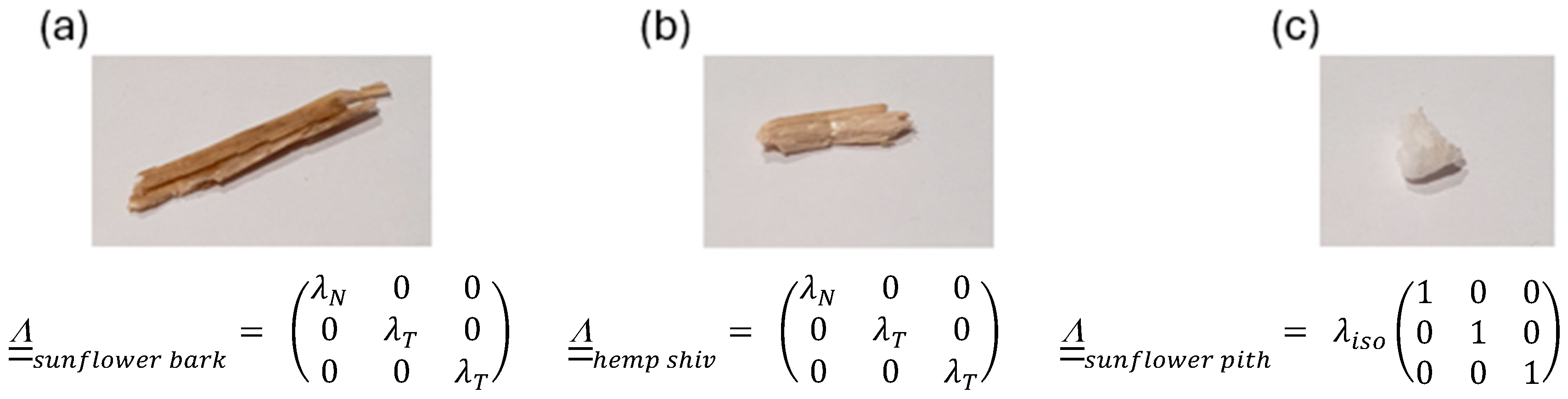




| Aggregate | Aspect Ratio | Assumption | Reference | Shape in Analytical Models |
|---|---|---|---|---|
| Hemp shiv | 3.3 | Transversely isotropic | Laborel-Preneron et al., 2018 [22] |  |
| Sunflower bark | 3.4 | Transversely isotropic | Lagouin et al., 2019 [11] |  |
| Sunflower pith | 1 | Isotropic | Magniont et al., 2012 [41] Arufe et al., 2021 [42] Rosa Latapie et al., 2023 [30] |  |
| Plant-Based Concretes | Reference | Binder/Aggregate | Density (kg·m−3) | Open Porosity (%) | Thermal Conductivity (W·m−1·K−1) | Measurement Conditions |
|---|---|---|---|---|---|---|
| LSB-Lagouin | Lagouin et al., 2019 [11] | Lime/sunflower bark | 539.64 ± 51.56 | 68.6 ± 1.7 | 0.127 ± 0.008 | 25 °C, 50% RH |
| MSB-Lagouin | Metakaolin/sunflower bark | 511.07 ± 61.86 | 71.5 ± 5.3 | 0.128 ± 0.009 |
| Plant-Based Concretes | Binder (kg) | Aggregate (kg) | Water (kg) | Water/Binder | Aggregate/Binder |
|---|---|---|---|---|---|
| LSB-Lagouin | 374.3 | 161.9 | 390.4 | 1.11 | 0.43 |
| MSB-Lagouin | 374.3 | 161.9 | 371.7 | 0.99 | 0.43 |
| Reference | Type of Aggregate | Calculation Method | Modeled Particulate Thermal Conductivity (W·m−1·K−1) | |
|---|---|---|---|---|
| λT | λN | |||
| Lagouin et al., 2019 [11] | Sunflower Bark | From intra-particle porosity | 0.139 | 0.208 |
| Laborel et al., 2018 [22] | Hemp shiv | From measurement on bulk particles | 0.044 | 0.066 |
| Rosa Latapie e al., 2023 [30] | Sunflower pith | From intra-particle porosity | λiso = 0.04 | |
| Hydraulic Binder | Aggregate | ||||||
|---|---|---|---|---|---|---|---|
| Composite | Density (kg·m−3) | Thermal Conductivity (W·m−1·K−1) | Particulate Density (kg·m−3) | Particulate Thermal Conductivity (W·m−1·K−1) | Volume Fraction | Aspect Ratio | |
| λT | λN | ||||||
| LSB-Lagouin | 1052 | 0.366 | 425 | 0.139 | 0.209 | 0.82 | 3.4 |
| MSB-Lagouin | 1079 | 0.277 | 0.87 | ||||
| Aggregate | Range of Aspect Ratio |
|---|---|
| Hemp shiv | 2.28–8.75 |
| Sunflower bark | 2.99–4.74 |
| Sunflower pith | 1–1.50 |
| Raw Material | Cultivated Area in Europe (ha) | Annual European Production (t) | Reference | Rate of Return (t/ha) | Proportion Compared to European Total Cereal Production (%) |
|---|---|---|---|---|---|
| Hemp | 15,700 | 85,000 | Carus et al., 2016 [66] | 5.4 | 0.01 |
| Sunflower | 18,073,170 | 68,655,795 | Debaeke et al., 2017 [65] | 3.8 | 12.6 |
| Plant-Based Concretes | ||
|---|---|---|
| Composite | LSB-Lagouin | MSB-Lagouin |
| Model type | HB-3-2D | HB-3-2D |
| Calibrated value | 20% coating | 20% coating |
| λcomposite [W·m−1·K−1] | 0.127 | 0.128 |
| [W·m−1·K−1] | 0.132 | 0.124 |
| Relative deviation | 4% | 4% |
| Plant-Based Concretes | ||
|---|---|---|
| Composite (λ in W·m−1·K−1) | LSB-Lagouin | MSB-Lagouin |
| λmax/λmin | 1.7 | 1.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Latapie, S.R.; Abou-Chakra, A.; Sabathier, V. Use of Local Resources in Plant-Based Concretes: Exploring Thermal Performance Through Multi-Scale Modeling. Constr. Mater. 2025, 5, 27. https://doi.org/10.3390/constrmater5020027
Latapie SR, Abou-Chakra A, Sabathier V. Use of Local Resources in Plant-Based Concretes: Exploring Thermal Performance Through Multi-Scale Modeling. Construction Materials. 2025; 5(2):27. https://doi.org/10.3390/constrmater5020027
Chicago/Turabian StyleLatapie, Séverine Rosa, Ariane Abou-Chakra, and Vincent Sabathier. 2025. "Use of Local Resources in Plant-Based Concretes: Exploring Thermal Performance Through Multi-Scale Modeling" Construction Materials 5, no. 2: 27. https://doi.org/10.3390/constrmater5020027
APA StyleLatapie, S. R., Abou-Chakra, A., & Sabathier, V. (2025). Use of Local Resources in Plant-Based Concretes: Exploring Thermal Performance Through Multi-Scale Modeling. Construction Materials, 5(2), 27. https://doi.org/10.3390/constrmater5020027





