Computational Aspects of Carbon and Boron Nanotubes
Abstract
:1. Introduction
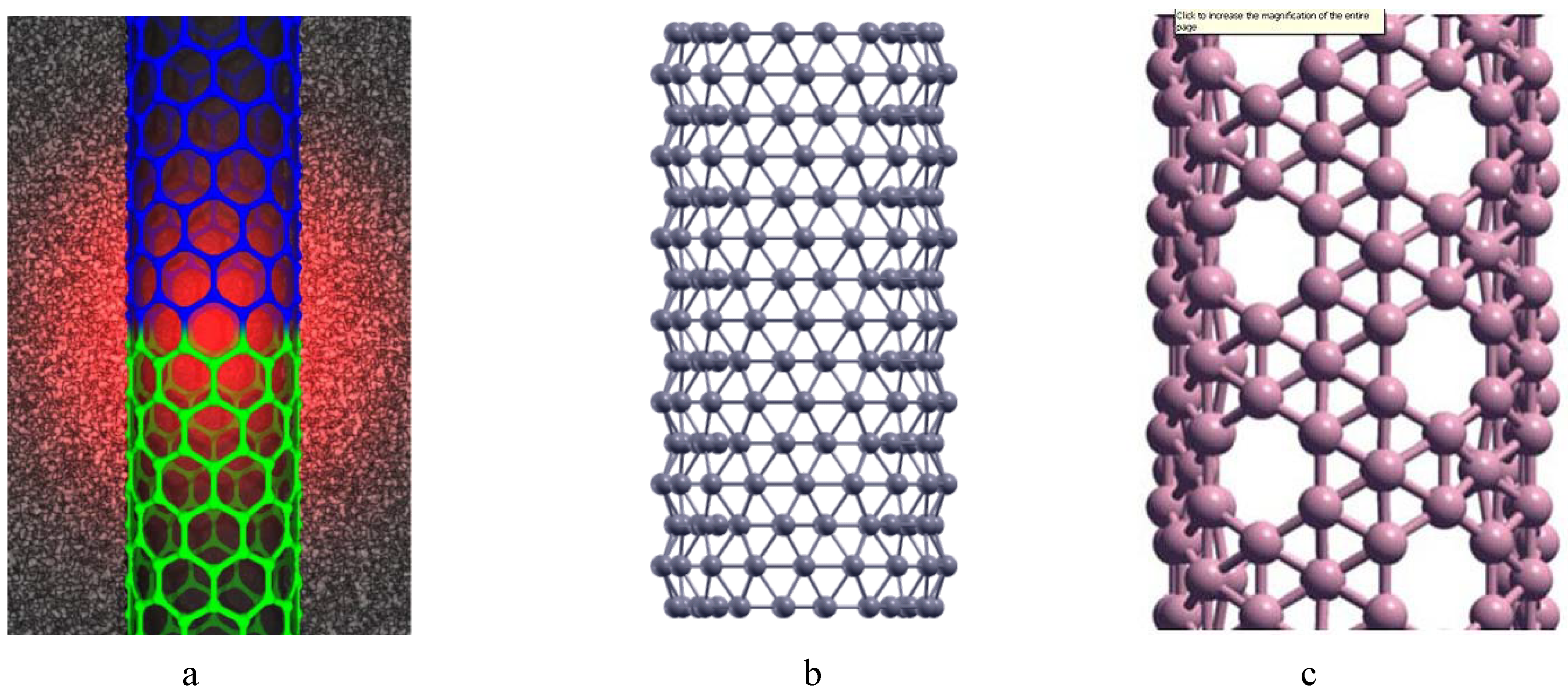
2. Basic Properties of Nanotubes of Armchair Model
- (1)
- A carbon hexagonal nanotube of order n × m has nm vertices and m(3n–2)/2 edges.
- (2)
- A boron triangular nanotube of order n × m has 3nm/2 vertices and 3m(3n–2)/2 edges.
- (3)
- A boron α-nanotube of order n × m has 4 nm/3vertices and m(7n–4)/2 edges when n is a multiple of 3.
3. Independent Set of Three Nanotubes
4. Perfect Matching and Matching Ratio
5. Broadcasting Problem of Carbon and Boron Nanotubes
5.1. Broadcasting Algorithm for Carbon Hexagonal Nanotubes
- Step 1:
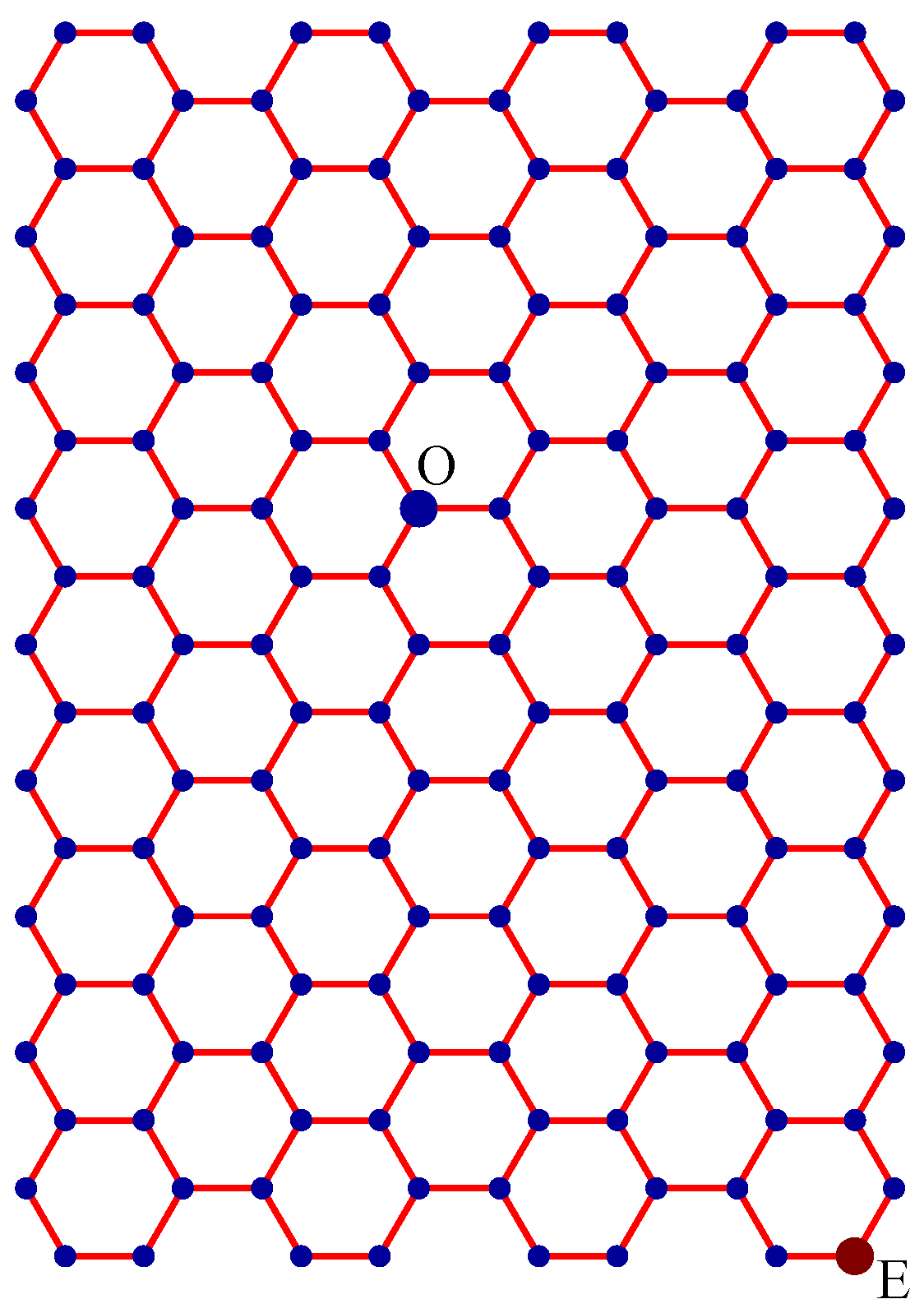
- Let O be the source node with the message and E denote the eccentric node of O. Unfold the carbon hexagonal nanotube into a rectangular sheet in such a way that the eccentric vertex E lies on the perimeter of the rectangular sheet. See Figure 6.
- Step 2:
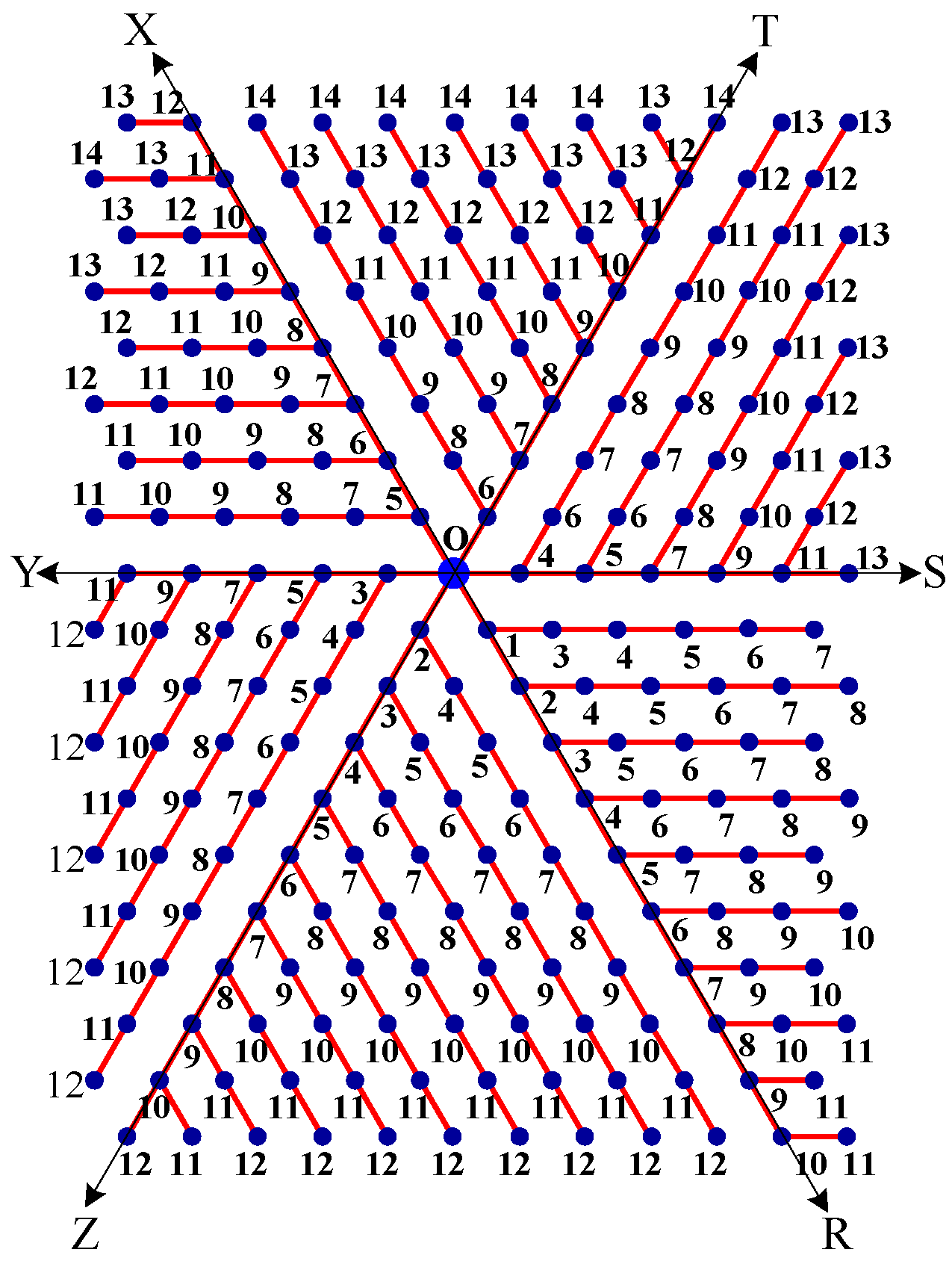
- Draw lines TOZ (at angle 30°), ROX (vertical), and SOY (at angle 150°). These lines create six zones, namely, zones ROS, SOT, TOX, XOY, YOZ and ZOR. See Figure 7.
- Step 3:
- Delete all the edges of zone ROS which are perpendicular to OS. Similarly, delete all the edges perpendicular to OT in zone SOT, edges perpendicular to OX in zone TOX, edges perpendicular to OY in zone XOY, edges perpendicular to OZ in zone YOZ, edges perpendicular to OR in zone ZOR. The resulting tree is the broadcasting tree of the carbon hexagonal nanotubes. See Figure 8.
- Step 4:
- Message is disseminated from source O based on farthest-distance-first protocol where a node with the message chooses an uninformed adjacent node which leads to longest path in the tree. If a node has label i, it means that the node receives the message from its neighbor at ith time unit. See Figure 9.
5.2. Broadcasting Problem of Boron Triangular Nanotubes
- Step 1:
- Let O be the source node with the message and E denote the eccentric node of O. Unfold the boron triangular nanotube into a rectangular sheet in such a way that the eccentric vertex E lies on the perimeter of the rectangular sheet. See Figure 10.
- Step 2:
- Draw lines SOY (horizontal), TOZ (at angle 60°), and XOR (at angle 120°). These lines create six zones, namely, zones ROS, SOT, TOX, XOY, YOZ and ZOR. See Figure 11.
- Step 3:
- Delete all the edges of zone ROS except the edges parallel to OS. Similarly retain only the edges parallel to OT in zone SOT, edges parallel to OX in zone TOX, edges parallel to OY in zone XOY, edges parallel to OZ in zone YOZ, and edges parallel to OR in zone ZOR. The resulting tree is the broadcasting tree of the boron triangular nanotube. See Figure 12.
- Step 4:
- The message is disseminated from source O based on farthest-distance-first protocol where a node with the message chooses an uninformed adjacent node which leads to longest path in the tree. If a node has label i, it means that the node receives the message from its neighbor at ith time unit. See Figure 13.
Conclusions
Acknowledgements
References
- Halford, B. Unusual properties of nanotubes made from inorganic materials offer intriguing possibilities for applications. Chem. Eng. News 2005, 83, 30–33. [Google Scholar]
- Berger, M. Nanotechnology in space. Nanowerk Nanotechnology Spotlight. Available online: http://www.nanowerk.com/spotlight (accessed on 29 June 2007).
- Perlet, J. The Global Market for Carbon Nanotubes to 2015: A Realistic Assessment; Nanoposts: Dublin, Ireland, 2010. [Google Scholar]
- Battersby, S. Boron nanotubes could outperform carbon. New Scientist. Available online: http://www.newscientist.com (accessed on 4 January 2008).
- Kunstmann, J.; Quandt, A. Broad boron sheets and boron nanotubes: An ab initio study of structural, electronic, and mechanical properties. Phys. Rev. B 2006, 74, 354–362. [Google Scholar] [CrossRef]
- Miller, P. Boron nanotubes beat carbon at its own game. Engadget. Available online: http://www.engadget.com (accessed on 6 January 2008).
- Tang, H.; Ismail-Beigi, S. Novel precursors for boron nanotubes: the competition of two-center and three-center bonding in boron sheets. Phys. Rev. Lett. 2007, 99, 115501–115504. [Google Scholar] [CrossRef] [PubMed]
- Yu, J.; Yu, D.; Chen, Y.; Lin, M.Y.; Li, J.; Duan, W. Narrowed bandgaps and stronger excitonic effects from small boron nitride nanotubes. Chem. Phys. Lett. 2009, 476, 240–243. [Google Scholar] [CrossRef]
- Tang, H.; Ismail-Beigi, S. Self-doping in boron sheets from first principles: A route to structural design of metal boride nanostructures. Phys. Rev. B 2009, 80, 134113–134115. [Google Scholar] [CrossRef]
- Clark, K.; Hassanien, A.; Khan, S.; Braun, K.F.; Tanaka, H.; Hla, S.W. Superconductivity in just four pairs of (BETS)2GaCl4 molecules. Nat. Nanotechnol. 2010, 5, 261–265. [Google Scholar] [CrossRef] [PubMed]
- Lee, R.K.F.; Cox, B.J.; Hill, J.M. Ideal Polyhedral Model for Boron Nanotubes with Distinct Bond Lengths. J. Phys. Chem. C 2009, 113, 19794–19805. [Google Scholar] [CrossRef]
- Yang, X.; Ding, Y.; Ni, J. Ab initio prediction of stable boron sheets and boron nanotubes: Structure, stability and electronic properties. Phys. Rev. B 2008, 77, 041402(R). [Google Scholar] [CrossRef]
- Tian, F.Y.; Wang, Y.X.; Lo, V.C.; Sheng, J. An ab initio investigation of boron nanotube in ring like cluster form. Appl. Phys. Lett. 2010, 96, 131901–131903. [Google Scholar] [CrossRef]
- Chen, Y.R.; Weng, C.; Sun, S.J. Electronic properties of zigzag and armchair carbon nanotubes under uniaxial strain. Journal of Applied Physics 2008, 104, 114310–114317. [Google Scholar] [CrossRef]
- Harris, P.J.F. Carbon Nanotube Science: Synthesis, Properties and Applications; Cambridge University Press: Cambridge, UK, 2009. [Google Scholar]
- Manuel, P.; Guizani, M. Broadcasting algorithms of carbon nanotubes. J. Comput. Theor. Nanosci. 2010, in press. [Google Scholar] [CrossRef]
- Madhavan, C.E.V. Approximation algorithm for maximum independent set in planar triangle-free graphs. Lect. Note. Comput. Sci. 1984, 181, 381–392. [Google Scholar]
- Jian, T. An O(20.304n) Algorithm for Solving Maximum Independent Set Problem. IEEE Trans. Comput. 1986, C-35, 847–851. [Google Scholar] [CrossRef]
- Soares, J.; Stefanes, M.A. Algorithms for Maximum Independent Set in Convex Bipartite Graphs. Algorithmica 2009, 53, 35–49. [Google Scholar] [CrossRef]
- Alekseev, V.E.; Lozin, V.; Malyshev, D.; Milanič, M. The Maximum independent set Problem in Planar Graphs. Lect. Note. Comput. Sci. 2008, 5162, 96–107. [Google Scholar]
- Brandstadt, A.; Klembt, T.; Lozin, V.V.; Mosca, R. Independent sets of maximum weight in apple-free graphs. Lect. Note. Comput. Sci. 2008, 5369, 848–858. [Google Scholar]
- Brodal, G.S.; Georgiadis, L.; Hansen, K.A.; Katriel, I. Dynamic Matchings in Convex Bipartite Graphs. Lect. Note. Comput. Sci. 2007, 4708, 406–417. [Google Scholar]
- Fajtlowicz, S.; John, P.E.; Sachs, H. On Maximum Matchings and Eigen values of Benzenoid Graphs. Croat. Chem. Acta 2005, 78, 195–201. [Google Scholar]
- Hansen, P.; Zheng, M. A linear algorithm for perfect matching in hexagonal systems. Discrete Math. 1993, 122, 179–196. [Google Scholar] [CrossRef]
- Klavžar, S.; Salem, K.; Taranenko, A. Maximum cardinality resonant sets and maximal alternating sets of hexagonal systems. Comput. Math. Appl. 2010, 59, 506–513. [Google Scholar] [CrossRef]
- Horton, R.; Moran, L.A.; Scrimgeour, K.G.; Perry, M.D.; Rawn, J.D. Principles of Biochemistry, 4th ed.; Pearson/Prentice Hall: New York, NY, USA, 2006. [Google Scholar]
- Middendorf, M. Minimum broadcast time is NP-complete for 3-regular planar graphs and deadline 2. Inf. Process. Lett. 1993, 46, 281–287. [Google Scholar] [CrossRef]
- Tang, Y.; Zhou, M. A Novel Broadcasting Algorithm for Wireless Sensor Networks. Lect. Note. Comput. Sci. 2006, 4330, 474–484. [Google Scholar]
- Chepoi, V.; Dragan, F.F.; Vaxès, Y. Addressing, distances and routing in triangular systems with applications in cellular networks. Wirel. Netw. 2006, 12, 671–679. [Google Scholar] [CrossRef]
- Su, Y.H.; Lin, C.C.; Lee, D.T. Broadcasting in Heterogeneous Tree Networks. Lect. Note. Comput. Sci. 2010, 6196, 368–377. [Google Scholar]
- Stojmenović, I. Honeycomb Networks: Topological Properties and Communication Algorithms. IEEE Trans. Parall. Distrib. Sys. 1997, 8, 1036–1042. [Google Scholar] [CrossRef]
- García, F.; Solano, J.; Stojmenovic, I.; Stojmenovic, M. Higher dimensional hexagonal networks. J. Parall. Distrib. Comput. 2003, 63, 1164–1172. [Google Scholar] [CrossRef]
- Shen, Z. A generalized broadcasting schema for the mesh structures. Appl. Math. Comput. 2007, 186, 1293–1310. [Google Scholar] [CrossRef]
- Li, J.; Chen, M.; Xiang, Y.; Yao, S. Optimum Broadcasting Algorithms in (n, k)-Star Graphs Using Spanning Trees. Lect. Note. Comput. Sci. 2010, 4672, 220–230. [Google Scholar]
- Nguyen, N.C.; Vo, N.M.D.; Lee, S. Efficient Routing and Broadcasting Algorithm in de Bruijn Networks. Lect. Note. Comput. Sci. 2005, 3358, 677–687. [Google Scholar]
- Kouvatsos, D.D.; Mkwawa, I.M. Broadcasting schemes for hypercubes with background traffic. J. Sys. Software 2004, 73, 3–14. [Google Scholar] [CrossRef]
Sample Availability: Contact the authors. |










© 2010 by the author; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Manuel, P. Computational Aspects of Carbon and Boron Nanotubes. Molecules 2010, 15, 8709-8722. https://doi.org/10.3390/molecules15128709
Manuel P. Computational Aspects of Carbon and Boron Nanotubes. Molecules. 2010; 15(12):8709-8722. https://doi.org/10.3390/molecules15128709
Chicago/Turabian StyleManuel, Paul. 2010. "Computational Aspects of Carbon and Boron Nanotubes" Molecules 15, no. 12: 8709-8722. https://doi.org/10.3390/molecules15128709
APA StyleManuel, P. (2010). Computational Aspects of Carbon and Boron Nanotubes. Molecules, 15(12), 8709-8722. https://doi.org/10.3390/molecules15128709



