Impact of Energy Losses Due to Failures on Photovoltaic Plant Energy Balance

Abstract

Photovoltaic (PV) plant failures have a significant influence on PV plant security, reliability, and energy balance. Energy losses produced by a PV plant are due to two large causes: failures and inefficiencies. Knowing the relative influence of energy losses due to failures and energy losses due to inefficiencies on the PV plant energy balance contribute to the optimization of its design, commissioning, and maintenance tasks. This paper estimates the failure rates, grouped by components, and the relative impact of the failures on the PV plant energy balance through real operation and maintenance follow-up data of 15 PV plants in Spain and Italy for 15 months. Results show that the influence of failures in energy losses of all analysed PV plants is low, reaching a maximum value of 0.96% of the net energy yield. Solar field energy losses only represent 4.26% of all failure energy losses. On the other hand, energy losses due to inefficiencies have represented between 22.34% and 27.58% of the net energy yield.

1. Introduction

As the photovoltaic market is growing rapidly based on improvements in photovoltaic (PV) modules, manufacturing advances, economies of scale and cost reduction [1], reliability, failures, and their associated energy losses, more questions are beginning to be asked.

According to the project report, Technical Risks in PV Projects [2], failures can be categorised into components (modules, inverters, mounting structure, connection and distribution boxes, cabling, potential equalization and grounding, lightning and protection system, weather station, communication and monitoring, transformer station, infrastructure and environmental influence, storage system, and miscellaneous) and phases (product testing, photovoltaic (PV) plant planning/development, installation/transportation, operation/maintenance, and decommissioning) of the value chain of a PV project.

During the operation/maintenance phase, failures can be found in the PV array such as snail trail [3], hot spot, diode failure, EVA discoloration, glass breakage, delamination with breaks in the ribbons and solder bonds [4], light induced degradation [5], low irradiance losses [6], potential induced degradation [7], shading effect [8], soiling effect [9], sun tracking system misalignments [10], wiring losses [11], mismatching effect in solar array [12], and other failures such as ground faults, line-to-line faults, and arc faults; although there have not been many such failures, a recent fire suggests the need for improvements to avoid them [13].

The failures types of PV modules are highly dependent on the design and technology of the PV module and on the environmental conditions in which the module is deployed. Hasselbrink et al. summarized data for returns from a fleet of more than 3 million modules, from 20 manufacturers [14]. The study found that 0.44% of the modules were returned after an average deployment of 5 years, with the vast majority of the returns associated with failures that can usually be identified visually, though there could be bias in this data, since modules with no visual defects would be harder to identify by the customer. A 2017 report from the National Renewable Energy Laboratory [15] examined 54,500 PV systems installed between 2005 and 2015. They found a median failure rate of just 5 out of 10,000 modules annually, which comes out to a 0.05% failure rate in all photovoltaic modules. In general, reported degradation percentages appear to have decreased appreciably in newer installations that were deployed after the year 2000. The report highlighted that modules in hot and humid climates show considerably higher degradation modes than those in desert and moderate climates, which warrants further investigation. Delamination and diode/j-box issues are also more frequent in hot and humid climates than in other climates. The highest concerns of systems installed in the last 10 years appear to be hot spots followed by internal circuitry discoloration. Encapsulant discoloration was the most common degradation mode, particularly in older systems. In newer systems, encapsulant discoloration appears in hotter climates, but to a lesser degree. Thin-film degradation modes are dominated by glass breakage and absorber corrosion, although the breadth of information for thin-film modules is much smaller than for x-Si.

In addition to failures, there are also other technical risks during operation phase due to degradation. For instance, Jordan et al. reviewed potential degradation rates of PV modules and systems reported in published literature from field testing throughout the last 40 years. Nearly 2000 potential degradation rates, measured on individual modules or entire systems, have been assembled from the literature, showing a median value of 0.5%/year [16].

Apart from PV modules, PV plants use inverters in a wide array of sizes and topologies, which complicates the evaluation of inverter failure rates. The majority of inverter failure studies do not treat the inverter as one black box. The reliability of PV inverter depends on the performance of each component. Inverters have failures such as failure in the insulated-gate bipolar transistor [17], surge protection, maximum power point tracker failure [18], alternating current (AC) or direct current (DC) contactors, electrolytic capacitors [19] fuses, control software, monitoring system, and inefficiency due to overheating. In particular, in grid-connected PV systems, a PV inverter may handle a high level of power flow and operate in a high temperature environment, which degrades the inverter reliability and increases the risk of component aging failures. According to Flicker et al., the failure modes that mostly affect PV inverters are related to units exposed to high thermal and electrical stress, as well as to the thermal management system itself [20]. Chang et al. investigated different circuit topologies of the single-phase PV inverters [21]. Results indicate that failures often occur in the switching stage, and temperature is the most likely cause of failure. Peng Zhang et al. analysed the reliability of the three main components: power electronic switches with a failure rate dominated by thermal overstress; capacitors with a failure rate that is dependent on the applied DC voltage, ripple current, and ambient conditions (temperature, airflow, and heat sinking); and inverter topologies in which results show that higher system reliability can be achieved by using module-integrated inverters [22].

Besides, PV plants are connected to an electrical grid that can present failures such as shutdown and overvoltage [23]. Moreover, many PV plants include transformer stations that also can presents failures such as electrical protection setting failure and overheating [24].

There are others failures in PV plants such as weather stations failures (pyranometer, calibrated reference cells, temperature sensors failures), fixed structure (rust on bolt and structure), ground (drainage and tracks damage), perimeter fence damages, and security system and monitoring system failures, but they do not directly affect energy losses and the electrical grid.

Extracting accurate trends of failure rates and their impact is difficult. There is a wide variety of equipment with unknown manufacturing and installation quality, PV array and inverter configurations, PV modules tracker systems, equipment technology, climate, electrical grid quality, equipment operation time, inspection methodologies, how the inspection sheet has been reported, and even what is the definition of the failure rate. Despite this, the overarching failure rates are usually used by reliability analysis methods, such as the Markov method [25], Fault Tree Analysis [26], Monte Carlo simulation [27], Pareto analysis [28], and the state enumeration method [29].

Moreover, it is even more difficult to know the true influence of failure on the PV plant energy balance, because there are many circumstances that affect them. For instance, one failure in a PV module could affect the energy yield of other PV modules and even could affect the inverter efficiency. Consequently, both failures and the energy losses associated with them are significant and must be clear in order to improve of PV market. Energy losses due to failure are an essential form of data that can be used to calculate associated costs.

With regard to energy balance, the performance ratio (PR) is the main rate used to know the impact of all energy losses on the PV plant energy balance without taking into account the PV module efficiency. It is the ratio of utilizable AC electricity at the feed-in meter to the amount of energy, which could be generated in case modules that were operated under Standard Test Conditions (STC), (1000 W/m², 25 °C, AM 1.5) continuously and without any further losses in the system. It is defined in the standard IEC 61724-1:2017. PR could reach a value of more than 0.8 for recent PV plants [30]. Nevertheless, with the PR it is impossible to know clearly the energy losses related to failure with respect to the energy losses related to inefficiencies in the PV plant. If the PV plant would include batteries, the energy balance could also be affected by the control strategies for energy store [31].

In addition to failures, inefficiencies of the PV plant components and their influence on the energy losses have been studied individually. For instance, in the PV array, the influence of a non-uniform of solar radiation on the module plane has always been one of the major concern for PV plants, so the effects of shading, snowing, and soiling on the output power have been extensively studied [9,32,33]. Another significant effect is the temperature influence according to PV technology. For instance, for the temperature, effect negative temperature coefficients are found for the crystalline module. On the contrary, for the amorphous module, positive power temperature coefficients can even be found, due to light soaking and thermal annealing effects. This shows that the data sheet temperature parameters do not reflect the annual thermal response of the module, and no reliable results using such coefficients could be achieved [34]. Another difference is the seasonal performance behaviour among PV technologies. PV technologies have a different response to temperature and the level of irradiance and its characteristics, mainly the angle of incidence and spectral effects. All of these effects should be taken into account to estimate their performance [35]. Amorphous thin film technology seems more suitable for building-integrated photovoltaic plants in cloudy or hot sites, while crystalline technology is suitable for open rack installation in sunny and cold locations [36].

In the inverter, the effect of the inverter temperature and maximum power point tracking are another significant concern of inefficiency. The temperature effect on the inverter efficiency, output power, and failure probability has also been analysed [37], and several maximum power point tracking methodologies have been proposed [38].

However, the influence of all PV plant failures and their relative impact on the whole PV plant energy balance has not been sufficiently researched. Gabriele et al. realize a study of reliability for PV plants between 2.5 kWp and 100 kWp up to the entry of the transformer station. To achieve more reliability, they conclude with the necessity of a high preventive maintenance and monitoring of the PV field (module and string protections) and a normal preventive maintenance of inverters. We propose only monitoring for the rest of components of the PV plant (AC circuit breaker, grid protection, DC Switch, AC Switch, differential circuit breaker and connector) [39]. Anastasios makes a thorough analysis of the failures of a great number of PV arrays and inverters, at two levels: one level is named the failure area that contains information about the item which exhibited an issue; and the second level is named the root cause that tracks the reason behind the issue. He highlights that the limited capability for deep failure analysis may dampen the value of the collected information [40]. In the same vein, Hasselbrink et al. indicate that collecting the failure data in a systematic format greatly simplifies the subsequent analysis [14].

To analyse the economic consequences of these risks, the Solar Bankability project [2] implemented a cost-based failure modes and effects analysis to the PV sector and defined a methodology for the estimation of economic losses due to planning failures, system downtime, the substitution/repair of components, and the assessment of the effectiveness of mitigation measures. The methodology is based on statistical analysis. The quality of the analysis depends on the amount of failure data available and on the assumptions taken for the calculation of a Cost Priority Number (CPN). This methodology is being enhanced by other authors [41] and paves the way to a desirable standardisation.

No studies that include the relative influence of energy losses due to failures on the global energy balance of the PV plant based in real data have been found. Only a comparison of the energy losses related to a single PV module failure for a base and worst-case scenario has been found [2]. In this case, it is not considered that in a PV array, the energy losses due to a PV module failure are strongly influenced by the energy yield of other modules without failure connected in series or parallel to it. Most PV plant simulation software estimates that PV plant energy losses are associated with inefficiencies but not due to failures [42,43]. In many cases, there is a significant difference between predicted and measured energy yield [44].

The current study, which focuses on the operation phase of a PV system, contributes to the analysis of the relative impact of energy losses due to failures in the PV plant energy balance, in 15 PV plants, located in Spain and Northern of Italy, with different PV module technologies, mounting configurations, and power. All of these PV plants have been in operation since 2010–2011. The knowledge of the relative impact of energy losses due to failures on the PV plant energy balance is useful to better estimate the energy losses cost and to improve maintenance and mitigation measures.

2. Methodology to Assess Energy Losses Due to Failure

The maximum electrical output energy of a photovoltaic array (ME) really is impossible to achieve in a PV plant performance, because it is affected by energy losses produced by failure energy losses (FEL) and energy losses produced by performance energy inefficiencies (PEL). The IEC 60050-191 defines failure as “the termination of the ability of an item to perform a required function” [45]. In this study failure is considered the total or partial malfunction of one or more equipment of the PV plant being unavailable for production.

According to the above definition, failures necessarily require corrective maintenance. Within the group of failures that affect FEL has been included PV module failure, which has led to it being replaced according to the warranty or performance conditions, such as glass broken, major delamination and hot spots, internal circuitry failure, solder bond failure and diode failure, inverter failure, or transformer failure such as shutdown or disconnection from the electrical grid.

PEL are the sum of the following energy losses. The energy losses produced by evitable inefficiencies are due to a not optimal performance of one or more equipment of the PV plant; in these cases, the equipment operates but at a level below expectations. In many cases, inefficient performance does not require immediate corrective action. There are multiple causes of these types of energy losses such as module temperature effect, bubbles, discoloration, chalking, potential induced degradation, shading and soiling effect, PV modules degradation, sun tracking system misalignments, wiring losses, mismatching effect in solar array, and inverter overheating. In some of these cases, such as mismatching effect, maximum power point tracker losses, PV module degradation, wiring losses, and shading phenomena are difficult to avoid when the PV plant is finished and in operation. Other failures such as derating effect, soiling effect, and sun tracking system misalignment may be corrected. Failures associated with these evitable inefficiencies usually take longer to manifest and require more sophisticated analytics to identify the corrective action than failures associated with FEL [40].

The energy losses produced by inevitable inefficiencies are due to the expected performance of one type of equipment, according to the nominal operation conditions described by the manufacturer. For instance, an inverter always has energy losses produced during its performance reflected by the manufacturer by its efficiency curve with the conditions set. These energy losses are inevitable for a given equipment and performance conditions. Global energy losses due to inefficiencies PEL are the sum of both inevitable and evitable inefficiencies.

So, based on these definitions, a PV plant in real operating conditions produces an electrical energy output at the feed-in meter, (RE), significantly lower than ME, related to both types of losses, with FEL and PEL using the energy balance shown in Equation (1).

$$ME=RE+PEL+FEL$$

(1)

FEL of a PV plant can be calculated according to equations shown in Appendix A, and ME can be calculated according to Equation (2) [46].

$$ME=P_n·{\displaystyle \sum }_{t=1}^{t=h}\frac{GT{I}_t}{1000}·(1-\mathsf{\gamma }·\Delta T_{cell\_t})$$

(2)

in which

• $P_n\left(\mathrm{kWp}\right)$ is the peak power of the PV plant in Standard Test Conditions (STC).
• $\mathsf{\gamma }$ (%/K) temperature coefficient for power (negative in sign) that corresponds to the installed modules.
• ΔT_{cell_t} (K) is the difference between the average hourly temperature of the PV reference module of the PV plant at hour t and 298.15 K.
• h is the hour number of the analysed period.
• $GT{I}_t$ (W/m²) is the global tilted irradiance in the hour t on the module plane.

Energy losses due to inefficiencies of a generic PV plant, PEL could be calculated as:

$$PEL=ME-RE-FEL$$

(3)

FEL and PEL are related to the PV plant hourly correct performance ratio $P{R}_{corr}$ according to Equation (4).

$$PEL+FEL=RE·\left(1-P{R}_{corr}\right)/P{R}_{corr}$$

(4)

in which $P{R}_{corr}$ is the hourly corrected performance ratio, determined according to Equation (5) [46]. Highlight that with this definition of $P{R}_{corr}$; PEL does not include the energy loss due to the PV module performances at module temperature instead of at module temperature in STC (29,815 K).

$$P{R}_{corr}=RE/(P_n·{\displaystyle \sum }_{t=1}^{t=h}\frac{GT{I}_t}{1000}·(1-\mathsf{\gamma }·\Delta T_{cell\_t})$$

(5)

Using these equations, it is possible to estimate the relative influence of FEL and PEL separately on ME and RE by assuming in this paper the following hypotheses:

• In the calculation of energy losses caused by a failure, the failure time considered includes the lapse of time between the hour in which the failures has been detected, (t_d), until the hour in which the failure has been repaired (t_r). We do not consider the lapse of time between the real occurrence of the failure until it is detected because it is not known.
• The performance ratio during the failure time is considered the average performance ratio of the PV plant in the 15 months defined by Equation (5). In this equation, we have utilized the hourly measured data of energy production, solar radiation on the plane of the modules measured by a pyranometer, and module temperature.
• In the quantification of the energy losses associated with a failure, we take into account the equipment that failed and the remaining equipment that is affected upstream of the one that failed, taking into account the global hourly radiation on the level of the PV array, the average hourly temperature of the panel during the dwell time, the average performance ratio of the PV plant during the 15 months, and the dwell time, from the t_d hour in which the failure is detected until the t_r hour that the failure has been repaired.
• When a failure takes place in a PV module its associated energy losses have been calculated assuming that in the time period between when the failure is detected and is replaced, the module there has not produced electric power in the complete string that the PV module belongs.
• Energy losses due to the transformation of solar radiation into electricity by a PV module in STC according to its datasheet have not been considered in PEL estimation.
• The PV plant energy losses due to a failure produced in the period from the moment when the failure took place and the instant in which it has been detected have been included in PEL estimation.

To estimate the influence of equipment failure and technology involved in the energy losses, the following criteria has been defined:

Criterion 1: Failures have been grouped depending on the affected equipment. Total failures, (TF), have been broken down into failures in the solar field, (TF_SF), inverter, (TF_I), transformer station, (TF_ST), electrical grid, (TF_G), and monitoring system, (TF_MS), according to Figure 1.

Within the solar field, failures have been broken down into failures that have led to the replacement of the photovoltaic module, failures in the DC wiring, and failures in the junction box, included fault in protections (not right performance of protection for fault in other equipment).

The inverter failures have been broken down into operation failures, failures of start-up and stopping, and failures in its monitoring system. Within the transformer station, failures have been broken down into failures in the transformer protections due to operation causes (not right performance of protection for fault in other part of the equipment) and failures resulting from extreme weather situations. Within the electrical grid, failures have been broken down into operation inherent grid failures and failures resulting from extreme weather situations.

Failures are not broken down into a more detailed root cause classification, because although the issue is logged in the database the true root cause was unknown, or it was inappropriately described or annotated in a wrong way by the professional supervision service in some cases. For this reason, we have limited the aim of the article according to the quality of the data set [36]. For instance, an inverter shutdown may be caused by different issues: insulated-gate bipolar transistor, surge protection, overheating, AC or DC contactors, electrolytic capacitors, fuses, control software, and power supply. Sometimes, the true root cause was not professionally described. For this reason, according to the aim of this article, all of them have been grouped into operation failures associated with the inverter.

Criterion 2: This classification is based on the technology linked to the failure, distributed in photovoltaic, electrical, electronic, and telecommunication technologies according to the Figure 2.

Failures occurred in the PV module such as discoloration, browning, delamination, glass, and cell breakage corresponding to replacement of the photovoltaic module have been assigned to PV technology failures.

In the group of electrical technology, failures have been included: failures related to electrical grid, transformer stations, wirings, and junction boxes.

In the group of electronic technology, failures have been included in the operation, start up, and stopping of the inverter failures. In the group of telecommunication technology, failures have included failures in the global communication system, the data acquisition equipment, and the monitoring system of the inverter.

Failure rates calculated for each PV plant have been defined as:

Annual failure rate in the solar field of the PV plant per module of the PV plant, FRSF (failures/year·module):

FRSF = TF_SF·12/(15 Module number)

(6)

Annual failure rate in the inverter of the PV plant per inverter in the PV plant, FRI_in. (failures/year·inverter):

FRI = TF_I·12/(15·Inverter number)

(7)

Annual failure rate in the station transformation of the PV plant, FRST, (failures/year):

FRST = TF_ST·12/15

(8)

Annual failure rate in the electrical grid of the PV plant, FRG, (failures/year):

FRG = TF_G·12/15

(9)

Annual failure rate in the monitoring system of the PV plant, FRM, (failures/year):

FRM = TF_M·12/15

(10)

Annual total failures rate of the PV plant, TFR, (failures/year):

TFR = TF·12/15

(11)

3. Application and Results

The methodology has been applied to 153 PV installations of 15 PV plants located in Spain and Northern Italy as shown in Figure 3 in a 15-months period, from January 2014 to March 2015. All these plants were put into operation in the period of 2010–2011 so they had already been more than three years of operation and adjustments of their set-up. All analysed PV plants are grid-connected, without storage systems and sun tracking systems.

Table 1. Main characteristics of PV plants analysed. (Spain and Italy).

PV Parks Characteristics
Location			Type	Solar Field			Inverter		ST	Plant
PV Park	PV Plant	Province	Ground/Roof	N° PV Modules	Material	Peak Power (Wp)	N° Inverters	Nominal Power (kW)	N° STs	Peak Power (kWp)	Nominal Power (kW)
A	A-1	Mallorca	Ground	44,928	CdTe	75/77.5	2	500	3	3,425.8	3160
							4	540
B	B-1	Castellón	Roof	3465	Si MC	238	7	100	1	824.7	700
	B-2		Roof	2590	Si MC	305	7	100	1	789.9	700
C	C1	Zamora	Ground	57,888	CdTe	75	4	1000	4	4,341.6	4000
D	D-1	Cremona	Ground	16,200	CdTe	77.5	2	630	1	1,255.5	1260
F	F-1	Lérida	Roof	3754	Si PC	235	8	100	1	880.1	800
MA	MA-1	Murcia	Ground	25,812	CdTe	80	4	500	3	2,065.0	2000
MB	MB-1	Murcia	Ground	9540	Si PC	235	4	500	4	2,241.9	2000
MC	MC-1	Murcia	Ground	13,680	Si PC	230	6	500	3	3,146.4	3000
PP	PP-1	Palazzo P.	Ground	46,200	CdTe	77.5	2	800	3	3,580.5	3490
							3	630
P2	P2-1	Parmense	Ground	20,520	CdTe	77.5	2	800	1	1,590.3	1600
P3	P3-1	Parmense	Ground	16,680	CdTe	77.5	2	630	1	1,292.7	1260
S	S-1	Valencia	Roof	3266	Si PC	230	1	630	1	751.2	630
	S-2		Roof	3266	Si PC	230	1	630	1	751.2	630
T	T-1	Tarragona	Roof	5374	Si PC	215-235	10	100	1	1,218.6	1000
TA	TA-1	Lérida	Roof	520	Si PC	220	20	5	1	114.4	100
	TA-2		Roof	520	Si PC	220/225	14	5	1	81.3	70
	TA-3		Roof	5139	CdTe	77.5	4	100	1	398.0	400
TS	TS-1	Lérida	Roof	1587	Si PC	215-235	22	15	1	354.6	330
	TS-2		Roof	1560	Si PC	235	24	15	1	366.1	360
Total
15	20	-	Ground	282,489	Si PC	29.627	153	MAX	34	29.470	27.490
			9		17.4%	(MWp)		1000		(MWp)	(MW)
			Roof		CdTe			MIN
			6		82.6%			5

shows the location, typology, and configurations of the analysed plants.

The failures have been obtained from real data and provided by the responsible companies for the operation and maintenance of the PV systems. These failures have been mainly detected through the analysis of climatological and electrical data obtained from the monitoring system of the PV plant, the alarm system, the monitoring from the inverter, the field thermography measurements, and the visual inspection of the plants. All plants have had a daily professional supervision service. No failures were detected in alternating current wiring.

3.1. Failure Rates

Table 2. Failures of the PV plants.

Failures
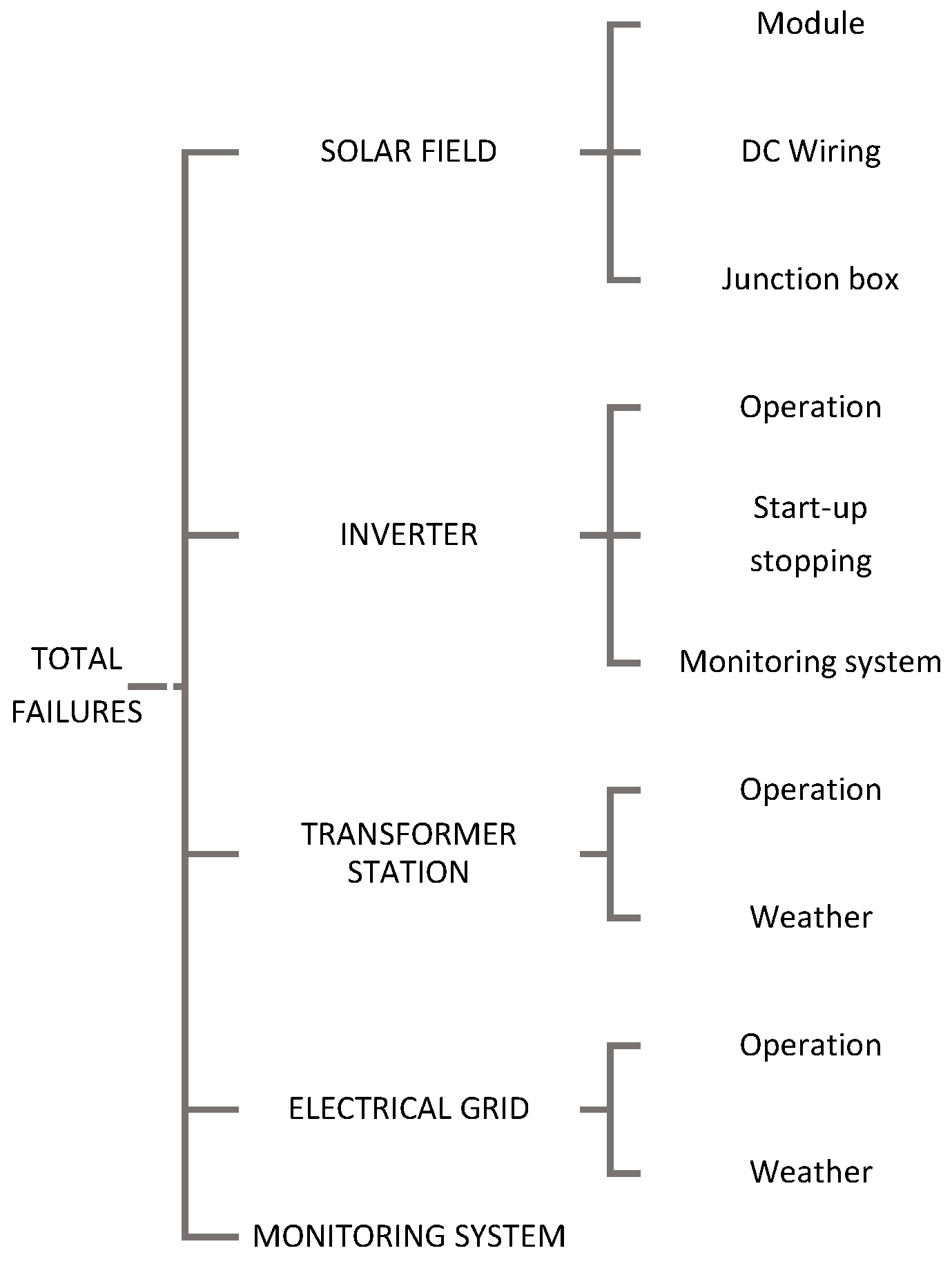
PV Park	Solar Field				Inverter				ST			Electrical Grid			Monitoring System	TF
	Modules	DC Wiring	Junction Box	TFSF	Operation	Start up and Stopping	Monitoring	TFI	Operation	Weather	TFST	Operation	Weather	TFG	TFMS
A	24	0	1	25	7	2	9	18	3	1	4	0	1	1	3	54
B	0	2	0	2	2	0	0	2	0	0	0	0	0	0	34	38
C	13	0	1	14	1	0	2	3	4	0	4	2	0	2	4	31
D	8	0	0	8	0	0	0	0	0	0	0	1	0	1	11	20
F	0	0	0	0	0	0	2	2	0	0	0	0	0	0	20	22
MA	0	0	0	0	0	1	0	1	0	0	0	1	0	1	2	4
MB	0	0	1	1	3	0	0	3	0	0	0	2	0	2	4	10
MC	0	0	1	1	0	2	0	2	0	0	0	0	0	0	2	5
PP	45	0	0	45	1	4	0	5	4	1	5	1	0	1	3	63
P2	12	0	1	13	1	0	2	3	2	1	3	1	1	2	5	28
P3	11	0	0	11	0	0	0	0	2	3	5	0	0	0	4	22
S	0	0	0	0	0	0	0	0	0	0	0	0	0	0	4	4
T	0	0	0	0	2	1	0	3	0	0	0	0	0	0	26	29
TA	0	0	0	0	3	17	2	22	0	0	0	0	0	0	24	46
TS	0	0	0	0	38	30	2	70	0	0	0	0	0	0	47	117
Total	113	2	5	120	58	57	19	134	15	6	21	8	2	10	193	478

shows a breakdown of the total failures in each PV plant according to the previous parameters.

Table 2 shows the distribution of failure rates in each plant associated with the different elements.

As

Table 3. Failure rates of the PV plants.

Failure Rates
PV Parks	FRSF	FRI	FRST	FRG	FRM	TFR	TFRp
A	0.0004452	2.4	1.067	0.8	2.4	43.2	0.01367
B	0.0002642	0.114	0	0	27.2	30.4	0.02171
C	0.0001935	0.6	0.8	1.6	3.2	24.8	0.0062
D	0.0003951	0	0	0.8	8.8	16	0.0127
F	0	0.2	0	0	16	17.6	0.022
MA	0	0.2	0	0.8	1.6	3.2	0.0016
MB	0.0000839	0.6	0	1.6	3.2	8	0.004
MC	0.0000585	0.267	0	0	1.6	4	0.00133
PP	0.0007792	0.8	1.333	0.8	2.4	51.2	0.01467
P2	0.0005068	1.2	2.4	1.6	4	22.4	0.014
P3	0.0005276	0	4	0	3.2	17.6	0.01397
S	0	0	0	0	3.2	3.2	0.00254
T	0	0.24	0	0	20.8	23.2	0.0232
TA	0	0.463	0	0	19.2	36.8	0.06456
TS	0	1.217	0	0	37.6	93.6	0.13565
Total	0.00034081	0.701	0.494	0.53	10.4	25.49	0.01739

shows, as the failure rates change significantly from one plant to another and from one element to another, it is difficult to assign an average value for failure rates.

The number of failures per year rate in a PV plant, TFR, changes between 3.2 and 93.60 failures/year, with a global average value of 25.49 failures/year. This rate is highly influenced by the size of the PV plant.

Per unit of installed equipment, the equipment with the highest failure rate is the monitoring system with an average failure rate of FRM = 10.4 failures/(year·monitoring system), (with a range between 1.6 and 37.6), followed by the inverter with an average failure rate of FRI_in = 0.701 failures/(year·inverter), the electrical grid with 0.53 failures/year, the transformer station with 0.494 failures/(year·transformer station), and the minor failure rate of the solar field, 0.00034081 failures/(year·module).

There are PV plants such as the TS with a high number of failures, 117, which represents 0.136 failures per year and kW. This is a roof PV plant, with Si-PC module, with 46 inverters of 15 kW. On the other side, there are PV plants such as S with very few failures, four, which represents 0.00256 failures per year and kW. This is a roof PV plant with Si-PC module too, but with two inverters of 630 kW. This shows that the relative number of failures have a great dependence on the size and configuration of each PV plant.

3.2. Failures and Energy Losses

3.2.1. Failures and Energy Losses under Criterion 1

Figure 4 shows the distribution of the total failures, TF, and the energy losses associated, FEL, in which TF_SF, TF_I, TF_ST, TF_G, and TF_MS are the total failures in the solar field, inverter, transformer station, electrical grid, and monitoring system, respectively, for all PV plants.

We wish to highlight that the monitoring system is the responsible of most of the failures, but those failures do not have a significant impact on the energy production. It is necessary to emphasize that over 54% of failures in the monitoring system had a duration of less than 1 h. In addition, in most cases, failures were automatically repaired by their own monitoring system.

However, despite the minimum percentage, 6.48%, that the electrical grid and the transformer station failures represent, the energy losses associated with that equipment suppose, approximately, 68% of the total energy losses.

Figure 5 shows the distribution of the 120 failures in the solar field and their influence on the energy losses, FEL.

Notice how most of the failures are caused due to the replacement of modules. This percentage would seem to be high a priori, but it should not be considered in that way taking into account that there are 282,489 PV modules between all the photovoltaic plants. Actually, the energy losses by replacing modules represent only the 20% of the energy losses in the solar field, as the influence of the non-production of one module is low compared to the influence on the energy losses of the wiring and junction boxes failures.

Figure 6 shows the distribution of the 134 failures of the inverters and their influence on the energy losses, FEL.

According to Figure 6, approximately 14% of the failures are produced by the monitoring system that does not affect the energy production. Failures named operation failures of the inverter have a greater impact on production, as it is shown in this figure.

Table 4. Failures and energy losses according to the inverter power.

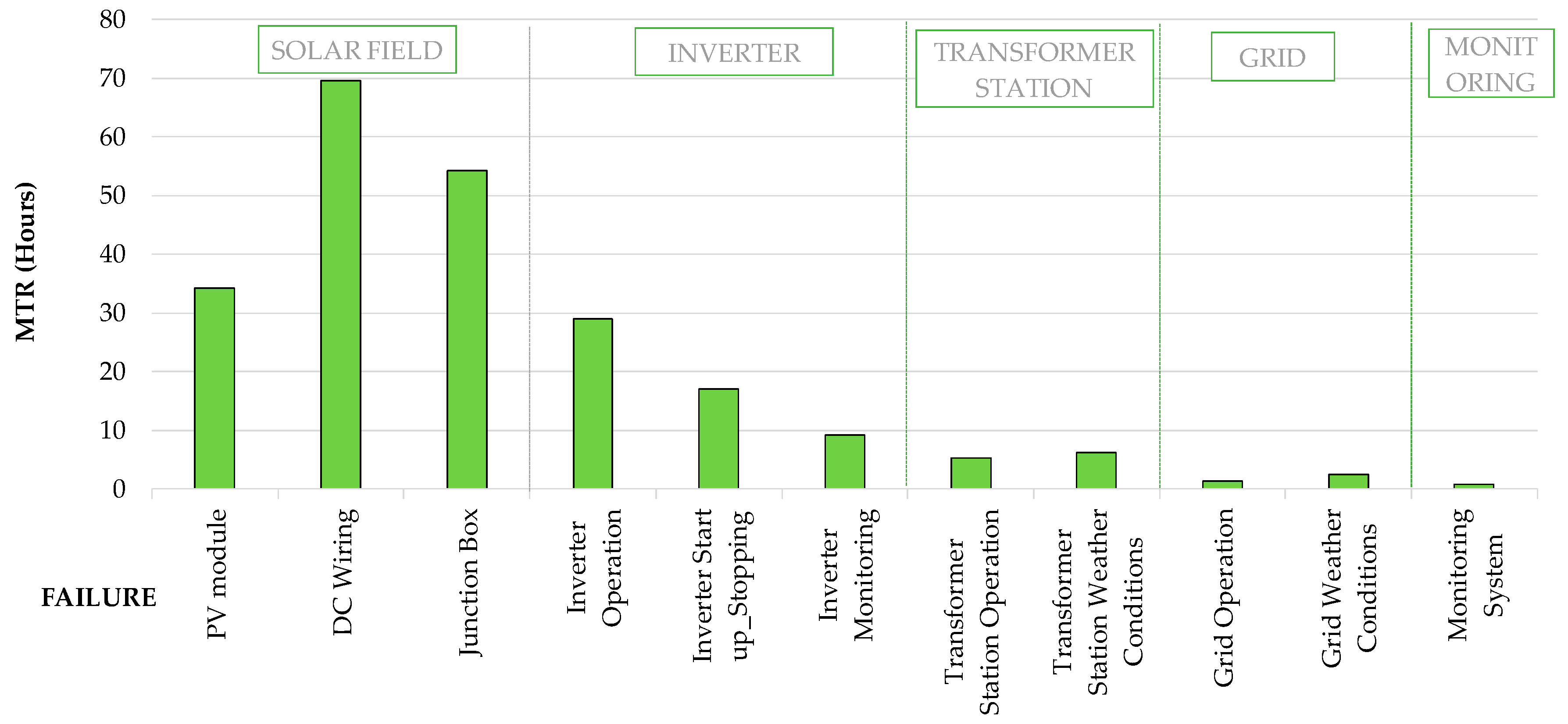
Power (kW)	Number of Inverters	Percentage of Failured Inverters	Operations Failures	Start-up and Stopping Failures	FELIO (kWh)	FELStSp (kWh)	FELI (kWh)	FELI/FEL
5	34	29.41%	1	12	3	2	5	0.01%
15	46	50.00%	38	30	1654	274	1928	1.21%
100	36	25.00%	5	8	2214	96	2310	1.45%
500	17	47.06%	7	4	12,243	2	12,244	7.68%
540	4	75.00%	4	2	20,249	1038	21,287	13.35%
630	8	12.50%	1	1	424	1664	2088	1.40%
800	4	25.00%	1	0	308	0	308	0.19%
1000	4	25.00%	1	0	3,595	0	3595	2.26%
Total	153	-	58	57	40,689	3077	43,766	27.55%

shows a breakdown of failures of operation, start up, and stopping depending on the inverter power.

The results provided in Table 4 show that the inverters of power less or equal to 15 kW are the ones that have presented the highest number of failures, but their incidence in the energy losses do not exceed 1.5%.

Figure 5 shows the probability of an inverter having a number of failures P_I(F).

Figure 7 shows that approximately 60% of the inverters have not presented any failure and that there are no inverters with more than 8 failures recorded in the analysed period.

Figure 8 shows the distribution of the 23 failures of the transformer stations, TF_ST, and their influence on the energy losses, FEL_ST.

According to Figure 8, approximately 76% of the failures are caused by the action of the electrical protection setting. The relationship between the failures and the energy losses are practically the same.

Figure 9 shows the distribution of the 8 failures of the electrical grids, TF_EG.

According to Figure 9, over the 76% of the failures are caused due by electrical grid quality and exhibit, approximately, the same behaviour as the transformer station.

3.2.2. Failure and Energy Losses under Criterion 2

Figure 10 shows the distribution of failures and energy losses depending on the technology for all PV plants.

Notice how TF_TT is the one presenting most failures, 42.05%, while TF_PVT represents 24.62% despite the large number of existing photovoltaic module units (282,489 modules). The percentage of failures associated with the photovoltaic technology only represents approximately 1% of energy losses, whereas the others technologies (excluding telecommunication) include the rest of energy losses with a slightly higher percentage.

3.3. Relative Impact of Failure and Inefficiencies on Energy Balance

Figure 11 shows the relative influence of FEL with respect to RE of each PV plant.

FEL are not significant in all PV plants analysed and have not exceeded 1% of the energy produced. In addition, Figure 9 shows that this percentage varies from one photovoltaic plant to another, being null in the PV plants F and S, and being the maximum percentage, 0.96%, in the PP photovoltaic plant.

Figure 12 shows the percentage of PEL with respect to RE of each PV plant.

It is observed that PEL represent between 22.34% and 27.58% of the RE and are much higher than those associated with FEL that have been registered over the analysed period, as shown in Figure 11. Reducing PEL without modifying the design of the PV plants or changing the equipment is only possible by moving shadows and cleaning the solar field and improving the ventilation system of the inverter. Other failures that affect PEL such as mismatching losses, wiring losses, inverter, and transformer efficiency are difficult to improve.

Figure 13 shows a criticality matrix of the PV plant by combining the frequency of failures with energy losses in each element of the PV plant.

From the point of view of energy losses, according to Figure 13, the most critical types of equipment are the transformer station and the electrical grid, because they represent 68% of the energy losses in only 31 failures, while 32% of the remaining FEL are associated with the solar fields and inverters that had registered 254 failures. Energy losses associated with the solar field are very low. To complement the analysis, Figure 14 illustrates the ratio FEL/TF depending on the failure.

Figure 14 shows effectively that the average energy losses associated with every failure are higher in the case of the electrical grid or transformer station than in inverters or solar field.

Figure 15 shows the mean time to repair (MTR), calculated as the average time from the instant of detection of the failure, t_d, and the instant of repair, t_r, depending on the failure, for all analysed PV plants.

The average time to repair is indicative of the efficiency of the corrective maintenance tasks. In PV systems, time to repair has a non-uniform influence on the energy losses. There would be, necessarily, an analysis of the value of global solar radiation during the time to repair. This value is very linked to the season of the year and especially the hour of the day. In latitudes like Spain and North of Italy, a great difference exists between the diurnal hours and the solar radiation on the module plane depending on the season. Its means that failures in winter would have minor repercussion on energy losses that the same failures in summer time. So, to evaluate energy losses, more important than time to repair is the global solar radiation on the module plane during time to repair as it has been included in Appendix A equations.

Figure 15 shows that monitoring system has the lowest mean time to repair. This is because in many cases the monitoring system failure can be solved automatically or very quickly. Mean time to repair of the electrical grid has also been low due to the fact that electric utilities have experience in its supervision and tele-operation.

Although the major average time to repair has taken place in the solar field, solar field has the lowest energy losses, after monitoring system. It is due to the lower power that it is affected by a failure in the solar field.

4. Conclusions

This paper has shown the failures and energy balance of several real PV plants for 15 months, according to the indicated hypothesis. It has been observed that PEL have represented between 22.34% and 27.58% of RE. The influence of FEL on RE has been low in all analysed PV plants, with values between 0% and 0.96%. The influence of FEL on the energy balance of the PV plant has been much lower than PEL.

To avoid PV plant energy losses, the focus should be on PEL instead of FEL. Preventive maintenance to avoid FEL should be focused on inverters, the station transformer, and the electrical grid plant.

Solar field energy losses only represent 4.26% of all FEL. So, in accordance with the Anastasio study [33], high-resolution monitoring on the DC side does not seem to be critical, as most of the lost energy can be attributed to outages in the other subsystems such as the inverter and the AC subsystem. An alternative to high-resolution monitoring in the solar field may be to carry out thorough, preventive maintenance in the PV modules to detect the different kinds of failures in each period (perhaps five years or according to the warranty conditions over time).

Analysed PV plants are quite reliable, with an annual average number of failures of 25.49 failures/year. According to Table 3, failure rates have a great dependence on the characteristic of each PV plant (size and configuration).

Form the point of view of technology analysis, photovoltaic technology has been the most reliable technology of all.