A Multiscale Analysis on the Superelasticity Behavior of Architected Shape Memory Alloy Materials
Abstract
:1. Introduction
2. Cellular Response
2.1. SMA Constitutive Model
- Transformation driving force related to f:where the definition of is introduced here.
- Orientation force related to :where denotes the deviatoric part of the stress tensor .
- Twin accommodation related to :
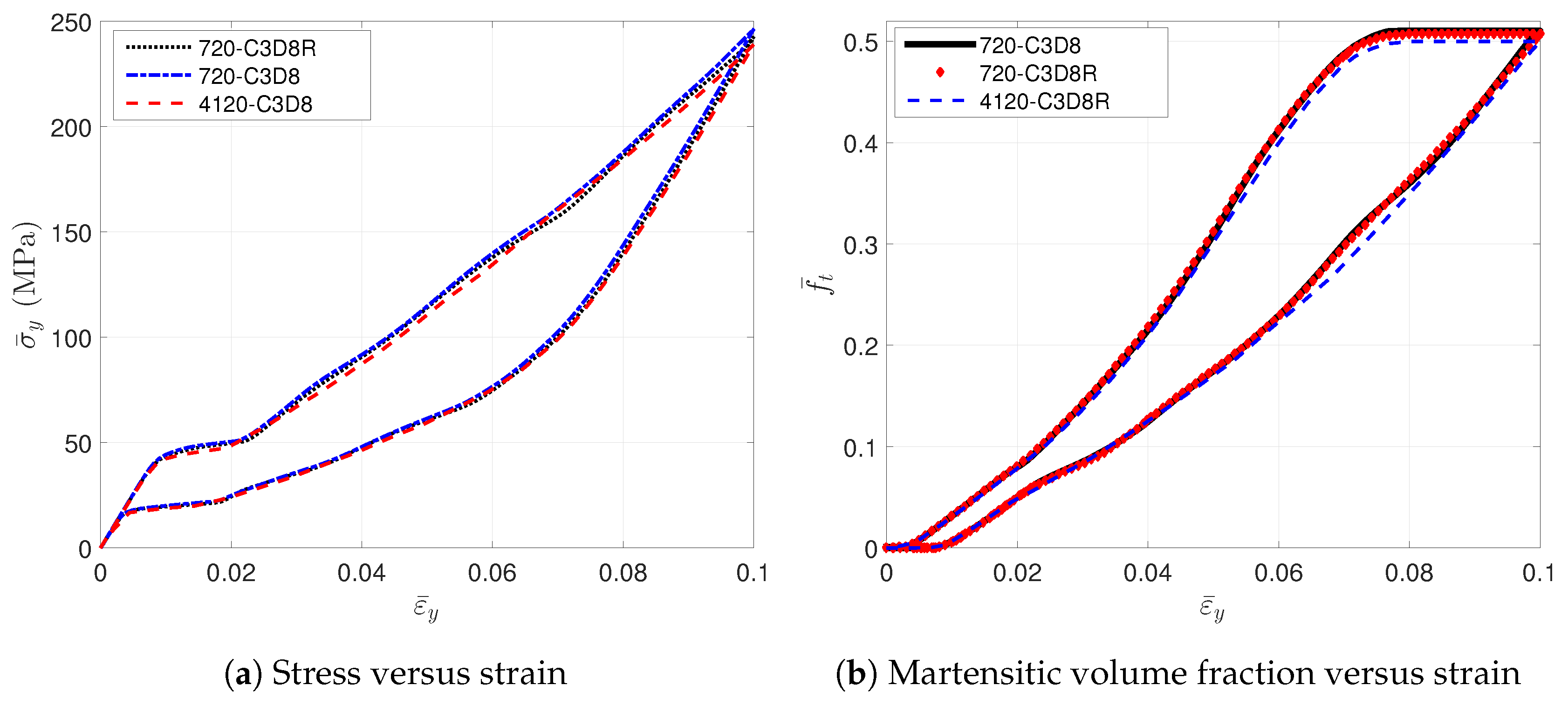
2.2. Convergence Analysis for the RVE Mesh
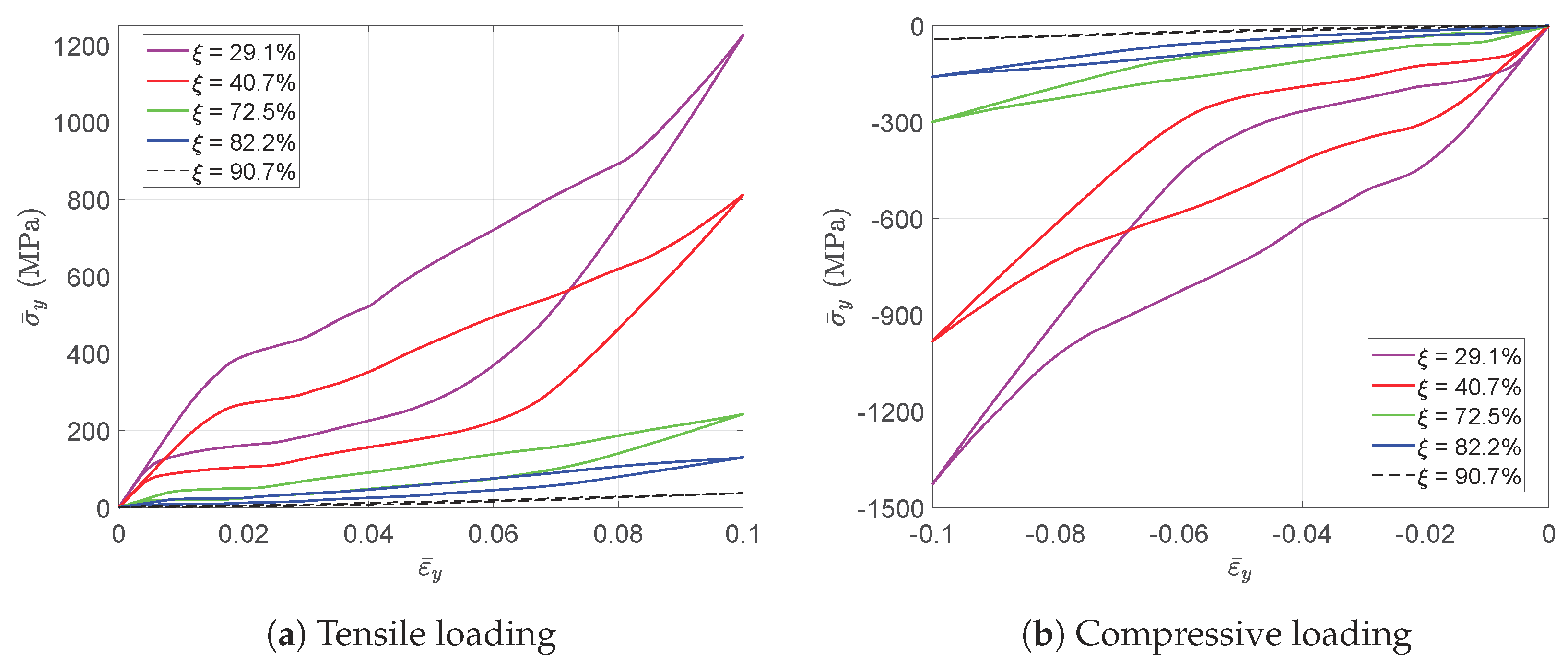
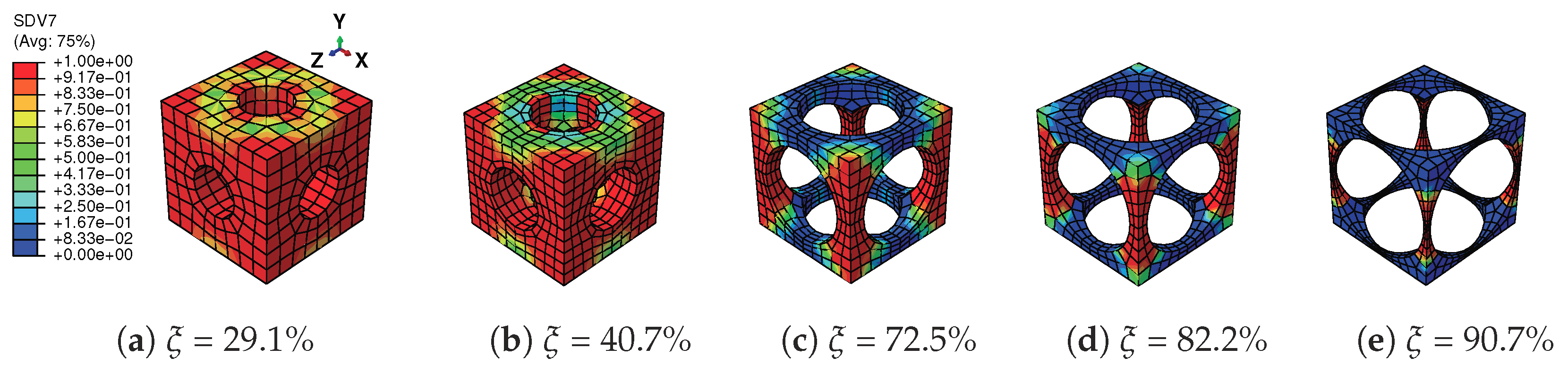
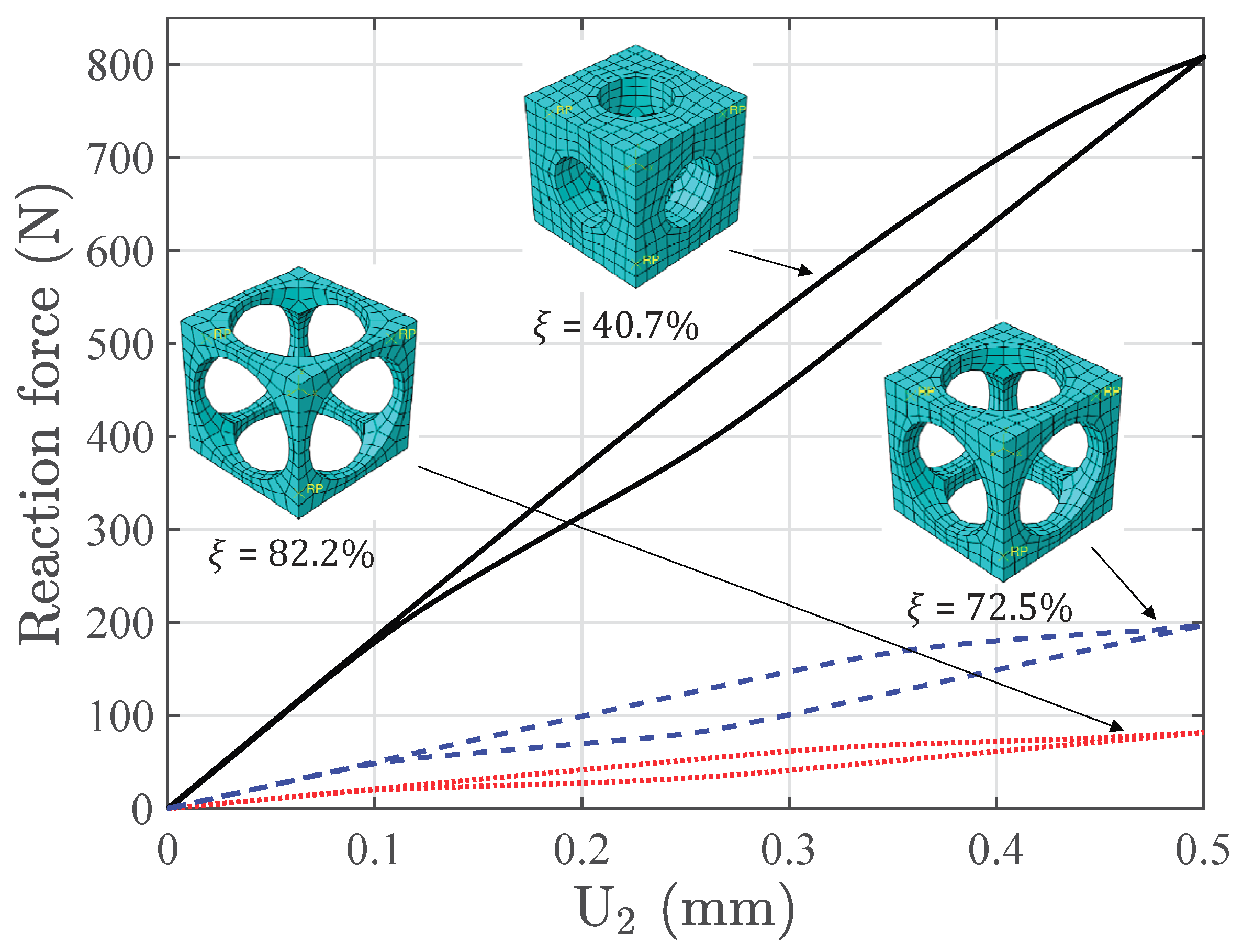
2.3. Cells with Different Geometries
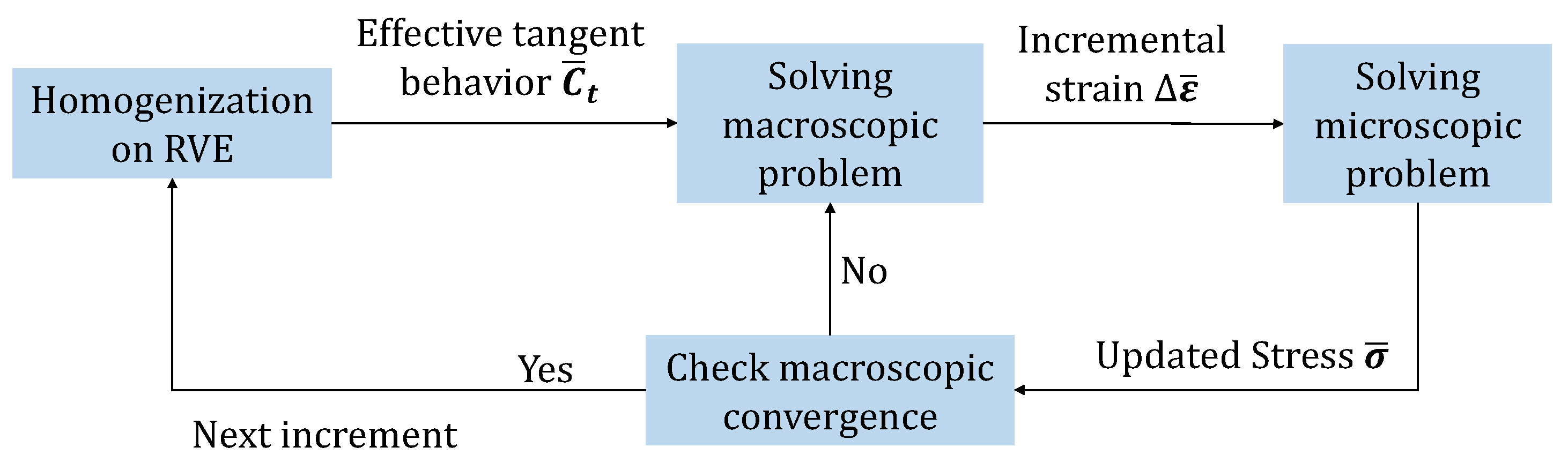
3. Structural Response
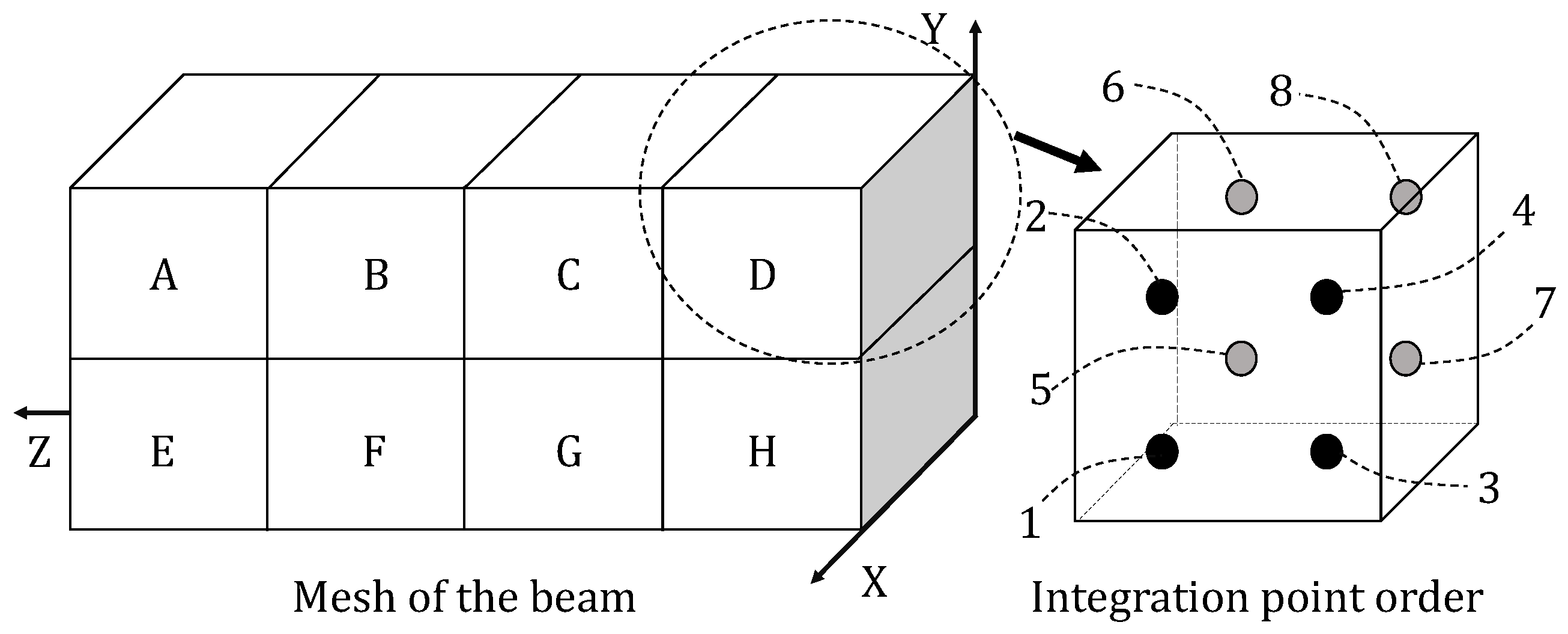
3.1. FE Formulation
3.2. Beam with Three Kinds of Cells Subjected to Three-Point Bending
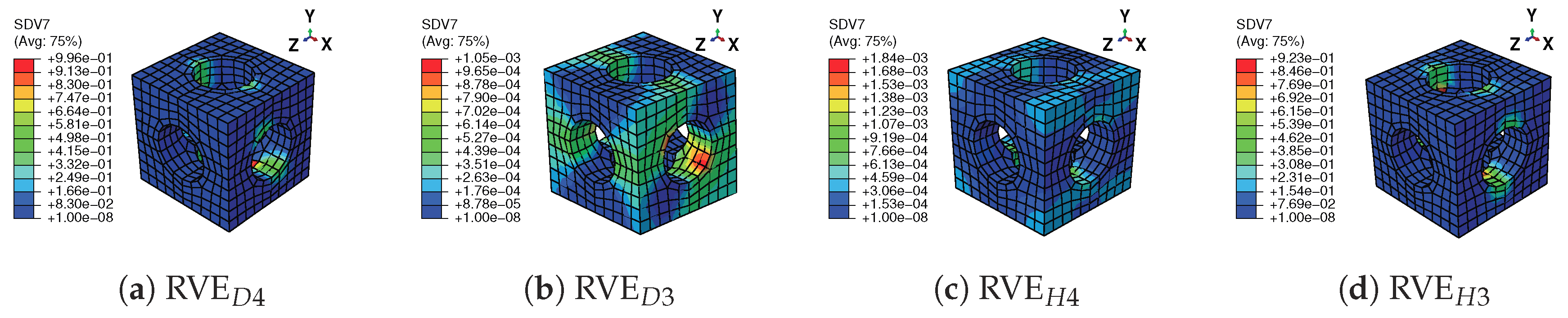
3.3. Stress Distributions at the Macroscopic and Microscopic Levels
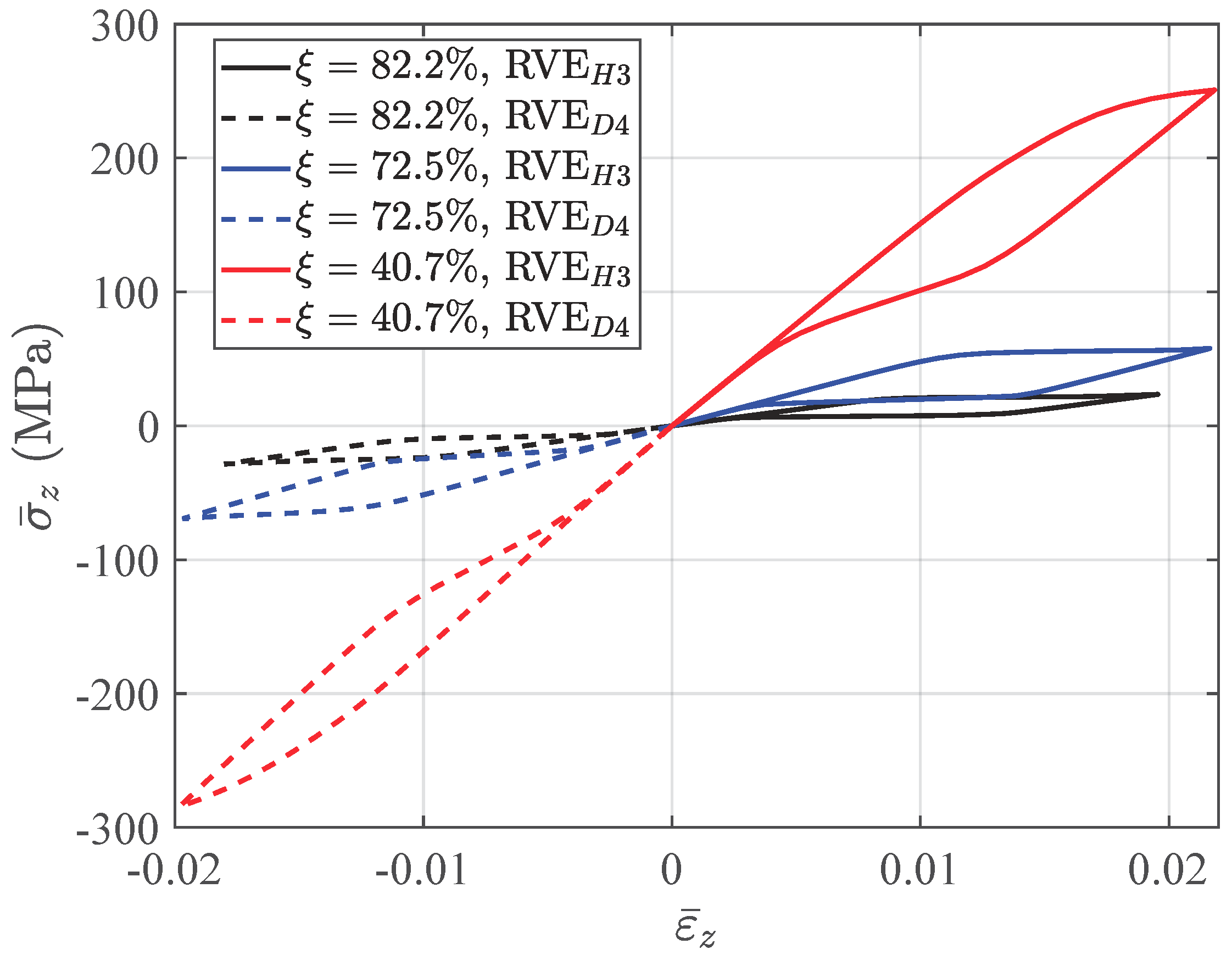
3.4. Evolution of the Loading
3.5. Structural Response with Different Microscopic Structures
3.6. Comments on the Computational Efficiency
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| SMA | Shape memory alloy |
| RVE | Representative volume element |
| FE | Multilevel finite element method |
| FEM | Finite element method |
| UMAT | User-defined materials |
| MPCs | Multi-point constraints |
| RP | Reference point |
| C3D8 | Continuum 3D solid element with full integration |
| C3D8R | Continuum 3D solid element with reduced integration |
| C3D8I | Continuum 3D solid element with incompatible modes |
References
- Gibson, L.J.; Ashby, M.F. Cellular Solids: Structure and Properties; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar]
- Ashby, M.F.; Evans, T.; Fleck, N.A.; Hutchinson, J.; Wadley, H.; Gibson, L. Metal Foams: A Design Guide; Elsevier: Amsterdam, The Netherland, 2000. [Google Scholar]
- Yazdani Sarvestani, H.; Akbarzadeh, A.H.; Niknam, H.; Hermenean, K. 3D printed architected polymeric sandwich panels: Energy absorption and structural performance. Compos. Struct. 2018, 200, 886–909. [Google Scholar] [CrossRef]
- Garcia-Moreno, F. Commercial applications of metal foams: Their properties and production. Materials 2016, 9, 85. [Google Scholar] [CrossRef] [PubMed]
- Hangai, Y.; Nakano, Y.; Koyama, S.; Kuwazuru, O.; Kitahara, S.; Yoshikawa, N. Fabrication of aluminum tubes filled with aluminum alloy foam by frictionwelding. Materials 2015, 8, 7180–7190. [Google Scholar] [CrossRef] [PubMed]
- Strano, M.; Marra, A.; Mussi, V.; Goletti, M.; Bocher, P. Endurance of damping properties of foam-filled tubes. Materials 2015, 8, 4061–4079. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rashed, M.G.; Ashraf, M.; Mines, R.A.W.; Hazell, P.J. Metallic microlattice materials: A current state of the art on manufacturing, mechanical properties and applications. Mater. Des. 2016, 95, 518–533. [Google Scholar] [CrossRef]
- Taherishargh, M.; Belova, I.V.; Murch, G.E.; Fiedler, T. Pumice/aluminium syntactic foam. Mater. Sci. Eng. A 2015, 635, 102–108. [Google Scholar] [CrossRef]
- Taherishargh, M.; Sulong, M.A.; Belova, I.V.; Murch, G.E.; Fiedler, T. On the particle size effect in expanded perlite aluminium syntactic foam. Mater. Des. 2015, 66, 294–303. [Google Scholar] [CrossRef]
- Taherishargh, M.; Belova, I.V.; Murch, G.E.; Fiedler, T. The effect of particle shape on mechanical properties of perlite/metal syntactic foam. J. Alloys Compd. 2017, 693, 55–60. [Google Scholar] [CrossRef]
- Broxtermann, S.; Taherishargh, M.; Belova, I.V.; Murch, G.E.; Fiedler, T. On the compressive behaviour of high porosity expanded Perlite-Metal Syntactic Foam (P-MSF). J. Alloys Compd. 2017, 691, 690–697. [Google Scholar] [CrossRef]
- Linul, E.; Movahedi, N.; Marsavina, L. On the Lateral Compressive Behavior of Empty and Ex-Situ Aluminum Foam-Filled Tubes at High Temperature. Materials 2018, 11, 554. [Google Scholar] [CrossRef] [PubMed]
- Luong, D.; Lehmhus, D.; Gupta, N.; Weise, J.; Bayoumi, M. Structure and compressive properties of invar-cenosphere syntactic foams. Materials 2016, 9, 115. [Google Scholar] [CrossRef] [PubMed]
- Pingle, S.M.; Fleck, N.A.; Deshpande, V.S.; Wadley, H.N.G. Collapse mechanism maps for the hollow pyramidal core of a sandwich panel under transverse shear. Int. J. Solids Struct. 2011, 95, 3417–3430. [Google Scholar] [CrossRef]
- Schaedler, T.A.; Ro, C.J.; Sorensen, A.E.; Eckel, Z.; Yang, S.S.; Carter, W.B.; Jacobsen, A.J. Designing metallic microlattices for energy absorber applications. Adv. Eng. Mater. 2014, 16, 3. [Google Scholar] [CrossRef]
- Schaedler, T.A.; Carter, W.B. Architected cellular materials. Annu. Rev. Mater. Res. 2016, 46, 187–210. [Google Scholar] [CrossRef]
- Lehmhus, D.; Vesenjak, M.; Schampheleire, S.; Fiedler, T. From stochastic foam to designed structure: Balancing cost and performance of cellular metals. Materials 2017, 10, 922. [Google Scholar] [CrossRef] [PubMed]
- Ngo, T.D.; Kashani, A.; Imbalzano, G.; Nguyen, K.T.Q.; Hui, D. Additive manufacturing (3D printing): A review of materials, methods, applications and challenges. Compos. Part B Eng. 2018, 143, 172–196. [Google Scholar] [CrossRef]
- Mostafaei, A.; Vecchis, P.R.D.; Stevens, E.L.; Chmielus, M. Sintering regimes and resulting microstructure and properties of binder jet 3D printed Ni-Mn-Ga magnetic shape memory alloys. Acta Mater. 2018, 154, 355–364. [Google Scholar] [CrossRef]
- Mehrpouya, M.; Gisario, A.; Elahinia, M. Laser welding of NiTi shape memory alloy: A review. J. Manuf. Process. 2018, 31, 162–186. [Google Scholar] [CrossRef]
- Lagoudas, D.C. Shape Memory Alloys: Modeling and Engineering Applications; Springer: New York, NY, USA, 2008. [Google Scholar]
- Patoor, E.; Lagoudas, D.C.; Entchev, P.B.; Catherine Brinson, L.; Gao, X. Shape memory alloys, Part I: General properties and modeling of single crystals. Mech. Mater. 2006, 38, 391–429. [Google Scholar] [CrossRef]
- Lagoudas, D.C.; Entchev, P.B.; Popov, P.; Patoor, E.; Catherine Brinson, L.; Gao, X. Shape memory alloys, Part II: Modeling of polycrystals. Mech. Mater. 2006, 38, 430–462. [Google Scholar] [CrossRef]
- Tobushi, H.; Hayashi, S.; Sugimoto, Y.; Date, K. Two-way bending properties of shape memory composite with SMA and SMP. Materials 2009, 2, 1180–1192. [Google Scholar] [CrossRef]
- Cisse, C.; Zaki, W.; Ben Zineb, T. A review of constitutive models and modeling techniques for shape memory alloys. Int. J. Plast. 2016, 76, 244–284. [Google Scholar] [CrossRef]
- Machado, G.; Louche, H.; Alonso, T.; Favier, D. Superelastic cellular NiTi tube-based materials: Fabrication, experiments and modeling. Mater. Des. 2015, 65, 212–220. [Google Scholar] [CrossRef] [Green Version]
- Ravari Karamooz, M.R.; Nasr Esfahani, S.; Taheri Andani, M.; Kadkhodaei, M.; Ghaei, A.; Karaca, H.; Elahinia, M. On the effects of geometry, defects, and material asymmetry on the mechanical response of shape memory alloy cellular lattice structures. Smart Mater. Struct. 2016, 25, 025008. [Google Scholar] [CrossRef]
- Ashrafi, M.J.; Amerinatanzi, A.; Saebi, Z.; Moghaddam, N.S.; Mehrabi, R.; Karaca, H.; Elahinia, M. Shape memory response of cellular lattice structures: Unit cell finite element prediction. Mech. Mater. 2018, 125, 26–34. [Google Scholar] [CrossRef]
- Kanouté, P.; Boso, D.P.; Chaboche, J.L.; Schrefler, B.A. Multiscale methods for composites: A review. Arch. Comput. Method E 2009, 16, 31–75. [Google Scholar] [CrossRef]
- Geers, M.G.D.; Kouznetsova, V.G.; Brekelmans, W.A.M. Multi-scale computational homogenization: Trends and challenges. J. Comput. Appl. Math. 2010, 234, 2175–2182. [Google Scholar] [CrossRef]
- El Hachemi, M.; Koutsawa, Y.; Nasser, H.; Giunta, G.; Daouadji, A.; Daya, E.M.; Belouettar, S. An intuitive computational multi-scale methodology and tool for the dynamic modeling of viscoelastic composites and structures. Compos. Struct. 2016, 144, 131–137. [Google Scholar] [CrossRef]
- Kinvi-Dossou, G.; Matadi Boumbimba, R.; Bonfoh, N.; Koutsawa, Y.; Eccli, D.; Gerard, P. A numerical homogenization of E-glass/acrylic woven composite laminates: Application to low velocity impact. Compos. Struct. 2018, 200, 540–554. [Google Scholar] [CrossRef]
- Feyel, F. A multilevel finite element method (FE2) to describe the response of highly non-linear structures using generalized continua. Comput. Methods Appl. Mech. Eng. 2003, 192, 3233–3244. [Google Scholar] [CrossRef]
- Nezamabadi, S.; Potier-Ferry, M.; Zahrouni, H.; Yvonnet, J. Compressive failure of composites: A computational homogenization approach. Compos. Struct. 2015, 127, 60–68. [Google Scholar] [CrossRef] [Green Version]
- Cong, Y.; Nezamabadi, S.; Zahrouni, H.; Yvonnet, J. Multiscale computational homogenization of heterogeneous shells at small strains with extensions to finite displacements and buckling. Int. J. Numer. Meth. Eng. 2015, 104, 235–259. [Google Scholar] [CrossRef] [Green Version]
- Tikarrouchine, E.; Chatzigeorgiou, G.; Praud, F.; Piotrowski, B.; Chemisky, Y.; Meraghni, F. Three-dimensional FE2 method for the simulation of non-linear, ratedependent response of composite structures. Compos. Struct. 2018, 193, 165–179. [Google Scholar] [CrossRef]
- Kohlhaas, B.; Klinkel, S. An FE2 model for the analysis of shape memory alloy fiber-composites. Comput. Mech. 2014, 55, 421–437. [Google Scholar] [CrossRef]
- Chatzigeorgiou, G.; Chemisky, Y.; Meraghni, F. Computational micro to macro transitions for shape memory alloy composites using periodic homogenization. Smart Mater. Struct. 2015, 24, 035009. [Google Scholar] [CrossRef] [Green Version]
- Xu, R.; Bouby, C.; Zahrouni, H.; Ben Zineb, T.; Hu, H.; Potier-Ferry, M. 3D modeling of shape memory alloy fiber reinforced composites by multiscale finite element method. Compos. Struct. 2018, 200, 408–419. [Google Scholar] [CrossRef]
- Chemisky, Y.; Duval, A.; Patoor, E.; Ben Zineb, T. Constitutive model for shape memory alloys including phase transformation, martensitic reorientation and twins accommodation. Mech. Mater. 2011, 43, 361–376. [Google Scholar] [CrossRef]
- Peultier, B.; Ben Zineb, T.; Patoor, E. Macroscopic constitutive law of shape memory alloy thermomechanical behaviour. Application to structure computation by FEM. Mech. Mater. 2006, 38, 510–524. [Google Scholar] [CrossRef]
- Duval, A.; Haboussi, M.; Ben Zineb, T. Modelling of localization and propagation of phase transformation in superelastic SMA by a gradient nonlocal approach. Int. J. Solids Struct. 2011, 13, 1879–1893. [Google Scholar] [CrossRef]
- Sittner, P.; Heller, L.; Pilch, J.; Sedlak, P.; Frost, M.; Chemisky, Y.; Duval, A.; Piotrowski, B.; Zineb, T.B.; Patoor, E.; et al. Roundrobin SMA modeling. In Proceedings of the ESOMAT 2009, Prague, Czech Republic, 7–11 September 2009. [Google Scholar]
- Chatzigeorgiou, G.; Charalambakis, N.; Chemisky, Y.; Meraghni, F. Periodic homogenization for fully coupled thermomechanical modeling of dissipative generalized standard materials. Int. J. Plast. 2016, 81, 18–39. [Google Scholar] [CrossRef]
- Fatemi Dehaghani, P.; Hatefi Ardakani, S.; Bayesteh, H.; Mohammadi, S. 3D hierarchical multiscale analysis of heterogeneous SMA based materials. Int. J. Solids Struct. 2017, 118–119, 24–40. [Google Scholar] [CrossRef]
- Yvonnet, J.; Zahrouni, H.; Potier-Ferry, M. A model reduction method for the post-buckling analysis of cellular microstructures. Comput. Methods Appl. Mech. Eng. 2007, 197, 265–280. [Google Scholar] [CrossRef]
- Ammar, A.; Chinesta, F.; Cueto, E.; Doblaré, M. Proper generalized decomposition of time-multiscale models. Int. J. Numer. Meth. Eng. 2012, 90, 569–596. [Google Scholar] [CrossRef] [Green Version]
- Kpogan, K.; Tri, A.; Sogah, A.; Mathieu, N.; Zahrouni, H.; Potier-Ferry, M. Combining MFS and PGD methods to solve transient heat equation. Numer. Methods Part. Differ. Equ. 2018, 34, 257–273. [Google Scholar] [CrossRef]
- Nezamabadi, S.; Yvonnet, J.; Zahrouni, H.; Potier-Ferry, M. A multilevel computational strategy for handling microscopic and macroscopic instabilities. Comput. Methods Appl. Mech. Eng. 2009, 198, 2099–2110. [Google Scholar] [CrossRef]
- Assidi, M.; Zahrouni, H.; Damil, N.; Potier-Ferry, M. Regularization and perturbation technique to solve plasticity problems. Int. J. Mater. Form 2009, 2, 1–14. [Google Scholar] [CrossRef]
- Aggoune, W.; Zahrouni, H.; Potier-Ferry, M. Asymptotic numerical methods for unilateral contact. Int. J. Numer. Meth. Eng. 2006, 68, 605–631. [Google Scholar] [CrossRef]
- Hu, H.; Damil, N.; Potier-Ferry, M. A bridging technique to analyze the influence of boundary conditions on instability patterns. J. Comput. Phys. 2011, 230, 3753–3764. [Google Scholar] [CrossRef]
- Yu, K.; Hu, H.; Chen, S.; Belouettar, S.; Potier-Ferry, M. Multi-scale techniques to analyze instabilities in sandwich structures. Compos. Struct. 2013, 96, 751–762. [Google Scholar] [CrossRef]













| Strain mechanisms: |
| Thermodynamical potential: |
| Clausius–Duhem inequality: |
| Thermo-elastic balance conditions: |
| Thermodynamic forces: |
| Criterion functions: |
| Physical limitations: |
| . |
| E (MPa) | 39,500 | (MPa °C) | 7 | (MPa) | 2 |
| (MPa °C) | 7 | (MPa) | 1635 | ||
| (°C) | (MPa) | 25,000 | |||
| (°C) | (MPa) | ||||
| (MPa) | 220 |
| Radius (mm) | 0.2 | 0.24 | 0.38 | 0.42 | 0.47 |
|---|---|---|---|---|---|
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, R.; Bouby, C.; Zahrouni, H.; Ben Zineb, T.; Hu, H.; Potier-Ferry, M. A Multiscale Analysis on the Superelasticity Behavior of Architected Shape Memory Alloy Materials. Materials 2018, 11, 1746. https://doi.org/10.3390/ma11091746
Xu R, Bouby C, Zahrouni H, Ben Zineb T, Hu H, Potier-Ferry M. A Multiscale Analysis on the Superelasticity Behavior of Architected Shape Memory Alloy Materials. Materials. 2018; 11(9):1746. https://doi.org/10.3390/ma11091746
Chicago/Turabian StyleXu, Rui, Céline Bouby, Hamid Zahrouni, Tarak Ben Zineb, Heng Hu, and Michel Potier-Ferry. 2018. "A Multiscale Analysis on the Superelasticity Behavior of Architected Shape Memory Alloy Materials" Materials 11, no. 9: 1746. https://doi.org/10.3390/ma11091746





