Numerical Simulation and In-Situ Measurement of Ground-Borne Vibration Due to Subway System
Abstract
1. Introduction
2. Numerical Methods of Subway Induced Environmental Vibration
2.1. Basic Principles and Realization of Infinite Element Method in Programs
2.2. Verification of Infinite Element Method in Programs
2.3. The Realization of Infinite Element Method in the Frequency Domain
- Step 1.
- The coupled finite element–infinite element boundary simulation model is built based on the commercial software ABAQUS.
- Step 2.
- The harmonic analyses are conducted based on the commercial software ABAQUS to obtain the amplitude and phase data of the evaluation measures on the ground in the subway induced vibration.
- Step 3.
- The numerical results are saved as dat file type in ABAQUS, and then read automatically by the developed interface programs to MATLAB.
- Step 4.
- Frequency domain analysis is performed based on MATLAB platform and the responses, such as acceleration and displacement time-histories of the evaluation points are obtained.
3. Experimental Verification of the Coupled Model for Subway Induced Vibrations
3.1. Vibration Measurement
3.2. The Coupled Finite Element–Infinite Element Boundary Model
3.3. Numerical Results
3.4. Simplified Prediction Formula of Ground Vibration Level
4. Parametric Study of Coupled Finite Element-Infinite Element Boundary Method in the Subway Induced Vibration
4.1. The Effect of Model Depths on the Simulation Results
4.2. The Effect of Model Lateral Sides with Infinite Element Boundary on the Simulation Results
4.3. The Effect of Model Base with Infinite Element Boundary on the Simulation Results
5. Conclusions
- The coupled simulation method is applicable for the practical engineering projects of subway induced vibration on the soft site in Shanghai, and the proposed formula for the vibration levels is reasonable for the prediction in the subway induced vibration.
- There exists a vibration amplifying zone a certain distance from the tunnel under high frequency loads due to wave propagation and reflection, and the amplification has relationship with the spectrum of the applied excitation accelerations.
- The finite element model dimensions can be reduced 50% and 66.67% effectively in the widths and depth, respectively, with the coupled method, applying the infinite element boundary to the lateral and base sides of the finite element model.
- The model dimensions have great influence on the numerical results as the excited frequency is close to the model’s vertical fundamental frequency, and the depth of the model should be designed seriously to reduce the numerical errors.
Author Contributions
Funding
Conflicts of Interest
References
- Xia, H.; Cao, Y.M. Problem of railway traffic induced vibrations of environments. J. Railw. Sci. Eng. 2004, 1, 44–51. (In Chinese) [Google Scholar]
- Gupta, S.; Degrande, G.; Lombaert, G. Experimental validation of a numerical model for subway induced vibrations. J. Sound Vib. 2009, 321, 786–812. [Google Scholar] [CrossRef]
- Gupta, S.; Liu, W.F.; Degrande, G.; Lombaert, G.; Liu, W.N. Prediction of vibrations induced by underground railway traffic in Beijing. J. Sound Vib. 2008, 310, 608–630. [Google Scholar] [CrossRef]
- Lopez-Mendoza, D.; Romero, A.; Connolly, D.P.; Galvin, P. Scoping assessment of building vibration induced by railway traffic. Soil Dyn. Earthq. Eng. 2017, 93, 147–161. [Google Scholar] [CrossRef]
- Zhong, H.; Qu, D.; Lin, G.; Li, H.J. Application of sensor networks to the measurement of subway-induced ground-borne vibration near the station. Int. J. Distrib. Sens. 2014. [Google Scholar] [CrossRef]
- Sun, K.; Zhang, W.; Ding, H.; Kim, R.E.; Spencer, B.F. Autonomous evaluation of ambient vibration of underground spaces induced by adjacent subway trains using high-sensitivity wireless smart sensors. Smart Struct. Syst. 2017, 19, 1–10. [Google Scholar] [CrossRef]
- Takemiya, H. Analysis of wave field from high-speed train on viaduct at shallow/deep soft grounds. J. Sound Vib. 2008, 310, 631–649. [Google Scholar] [CrossRef]
- Liu, C.; Wei, J.H.; Zhang, Z.X.; Liang, J.S.; Ren, T.Q.; Xu, H.Q. Design and evaluation of a remote measurement system for the online monitoring of rail vibration signals. Proc. Mech. Eng. Part F 2016, 230, 724–733. [Google Scholar]
- Li, X.H.; Long, Y.; Ji, C.; Zhong, M.S.; Zhao, H.B. Study on the vibration effect on operation subway induced by blasting of an adjacent cross tunnel and the reducing vibration techniques. J. Vibroeng. 2013, 15, 1454–1462. [Google Scholar]
- Ling, X.Z.; Chen, S.J.; Zhu, Z.Y.; Zhang, F.; Wang, L.N.; Zou, Z.Y. Field monitoring on the train-induced vibration responses of track structure in the Beiluhe permafrost region along Qinghai-Tibet railway in China. Cold Reg. Sci. Technol. 2010, 60, 75–83. [Google Scholar] [CrossRef]
- Kouroussis, G.; Florentin, J.; Verlinden, O. Ground vibration induced by intercity/inter region trains: A numerical prediction based on the multibody/finite element modeling approach. J. Vib. Control 2016, 22, 4192–4210. [Google Scholar] [CrossRef]
- Kaewunruen, S.; Remennikov, A.M. Sensitivity analysis of free vibration characteristics of an in situ railway concrete sleeper to variations of rail pad parameters. J. Sound Vib. 2006, 298, 453–461. [Google Scholar] [CrossRef]
- Connolly, D.; Giannopoulos, A.; Fan, W.; Woodward, P.K.; Forde, M.C. Optimising low acoustic impedance back-fill material wave barrier dimensions to shield structures from ground borne high speed rail vibrations. Constr. Build. Mater. 2013, 44, 557–564. [Google Scholar] [CrossRef]
- Kaewunruen, S.; Remennikov, A.M. Current state of practice in railway track vibration isolation: An Australian overview. Aust. J. Civil Eng. 2016, 14, 63–71. [Google Scholar] [CrossRef]
- Indraratna, B.; Sun, Q.D.; Heitor, A.; Grant, J. Performance of rubber tire-confined capping layer under cyclic loading for railroad conditions. J. Mater. Civil Eng. 2018, 30. [Google Scholar] [CrossRef]
- Navaratnarajahl, S.K.; Indraratna, B. Use of rubber mats to improve the deformation and degradation behavior of rail ballast under cyclic loading. J. Geotech. Geoenviron. 2017, 143. [Google Scholar] [CrossRef]
- Hussaini, S.K.K.; Indraratna, B.; Vinod, J.S. A laboratory investigation to assess the functioning of railway ballast with and without geogrids. Transp. Geotech. 2016, 6, 45–54. [Google Scholar] [CrossRef]
- Kaewunruen, S.; Remennikov, A.M. Dynamic crack propagations in prestressed concrete sleepers in railway track systems subjected to severe impact loads. J. Struct. Eng 2010, 136, 749–754. [Google Scholar] [CrossRef]
- Remennikov, A.M.; Kaewunruen, S. Experimental load rating of aged railway concrete sleepers. Eng. Struct. 2014, 76, 147–162. [Google Scholar] [CrossRef]
- Lu, Z.; Wang, Z.X.; Zhou, Y.; Lu, X.L. Nonlinear dissipative devices in structural vibration control: A review. J. Sound Vib. 2018, 6, 18–49. [Google Scholar] [CrossRef]
- Dai, K.S.; Wang, J.Z.; Mao, R.F.; Lu, Z.; Chen, S.E. Experimental investigation on dynamic characterization and seismic control performance of a TLPD system. Struct. Des. Tall Spec. 2017, 26, e1350. [Google Scholar] [CrossRef]
- Lu, Z.; Huang, B.; Zhang, Q.; Lu, X.L. Experimental and analytical study on vibration control effects of eddy-current tuned mass dampers under seismic excitations. J. Sound Vib. 2018, 421, 153–165. [Google Scholar] [CrossRef]
- Lu, Z.; Chen, X.Y.; Li, X.W.; Li, P.Z. Optimization and application of multiple tuned mass dampers in the vibration control of pedestrian bridges. Struct. Eng. Mech. 2017, 62, 55–64. [Google Scholar] [CrossRef]
- Lu, Z.; Chen, X.Y.; Zhang, D.C.; Dai, K.S. Experimental and analytical study on the performance of particle tuned mass dampers under seismic excitation. Earthq. Eng. Struct. D 2017, 46, 697–714. [Google Scholar] [CrossRef]
- Lu, Z.; Chen, X.Y.; Zhou, Y. An equivalent method for optimization of particle tuned mass damper based on experimental parametric study. J. Sound Vib. 2018, 419, 571–584. [Google Scholar] [CrossRef]
- Lu, X.L.; Liu, Z.P.; Lu, Z. Optimization design and experimental verification of track nonlinear energy sink for vibration control under seismic excitation. Struct. Control Health Monit. 2017, 24, e2033. [Google Scholar] [CrossRef]
- Lu, Z.; Yang, Y.L.; Lu, X.L.; Liu, C.Q. Preliminary study on the damping effect of a lateral damping buffer under a debris flow load. Appl. Sci. 2017, 7, 201. [Google Scholar] [CrossRef]
- Lu, Z.; Wang, Z.X.; Masri, S.F.; Lu, X.L. Particle Impact Dampers: Past, Present, and Future. Struct. Control Health Monit. 2018, 25, e2058. [Google Scholar] [CrossRef]
- Lu, Z.; Lu, X.L.; Masri, S.F. Studies of the performance of particle dampers under dynamic loads. J. Sound Vib. 2010, 329, 5415–5433. [Google Scholar] [CrossRef]
- Lu, Z.; Huang, B.; Zhou, Y. Theoretical study and experimental validation on the energy dissipation mechanism of particle dampers. Struct. Control Health Monit. 2018, 25, e2125. [Google Scholar] [CrossRef]
- Gupta, S.; Hussein, M.F.M.; Degrande, G.; Hunt, H.E.M.; Clouteau, D. A comparison of two numerical models for the prediction of vibrations from underground railway traffic. Soil Dyn. Earthq. Eng. 2007, 27, 608–624. [Google Scholar] [CrossRef]
- Sun, X.J.; Liu, W.N.; Zhai, H. Study on low-frequency vibration isolation performance of steel spring floating slab track. Environ. Vib. Predict. Monit. Mitig. Eval. 2009, 1–2, 429–434. [Google Scholar]
- Galvin, P.; Francois, S.; Schevenels, M.; Bongini, E.; Degrande, G.; Lombaert, G. A 2.5D coupled FE-BE model for the prediction of railway induced vibrations. Soil Dyn. Earthq. Eng. 2010, 30, 1500–1512. [Google Scholar] [CrossRef]
- Wolf, S. Potential low frequency ground vibration (<6.3 Hz) impacts from underground LRT operations. J. Sound Vib. 2003, 267, 651–661. [Google Scholar]
- Hung, H.H.; Yang, Y.B. Analysis of ground vibrations due to underground trains by 2.5D finite/infinite element approach. Earthq. Eng. Eng. Vib. 2010, 9, 327–335. [Google Scholar]
- Costa, P.A.; Calcada, R.; Cardoso, A.S. Track-ground vibrations induced by railway traffic: In-situ measurements and validation of a 2.5D FEM-BEM model. Soil Dyn. Earthq. Eng. 2012, 32, 111–128. [Google Scholar] [CrossRef]
- Yuan, Z.H.; Bostrom, A.; Cai, Y.Q. Benchmark solution for vibrations from a moving point source in a tunnel embedded in a half-space. J. Sound Vib. 2017, 387, 177–193. [Google Scholar] [CrossRef]
- Lopes, P.; Costa, P.A.; Ferraz, M.; Calcada, R.; Cardoso, A. Numerical modeling of vibrations induced by railway traffic in tunnels: From the source to the nearby buildings. Soil Dyn. Earthq. Eng. 2014, 61–62, 269–285. [Google Scholar] [CrossRef]
- Chiacchiari, L.; Loprencipe, G. Measurement methods and analysis tools for rail irregularities: A case study for urban tram track. J. Modern Transp. 2015, 23, 137–147. [Google Scholar] [CrossRef]
- Kaynia, A.M.; Madshus, C.; Zackrisson, P. Ground vibration from high-speed trains: Prediction and countermeasure. J. Geotech. Geoenviron. 2000, 126, 531–537. [Google Scholar] [CrossRef]
- Huang, H.H.; Chen, G.H.; Yang, Y.B. Effect of railway roughness on soil vibrations due to moving trains by 2.5D finite/infinite element approach. Eng. Struct. 2013, 57, 254–266. [Google Scholar] [CrossRef]
- Jiang, T.; Yue, J.Y. Analysis of environmental vibration induced by subway in Shanghai and Chengdu by using thin layer method. In Proceedings of the 5th International Symposium on Environmental Vibration, Chengdu, China, 20–22 October 2011; pp. 113–119. [Google Scholar]
- Zou, C.; Wang, Y.M.; Guo, J.X. Measurement of ground and nearby building vibration and noise induced by trains in a metro depot. Sci. Total Environ. 2015, 536, 761–773. [Google Scholar] [CrossRef] [PubMed]
- Ju, S.H. Three-dimensional analyses of wave barriers for reduction of train-induced vibrations. J. Geotech. Geoenviron. 2004, 130, 740–748. [Google Scholar] [CrossRef]
- Sanayei, M.; Maurya, P.; Moore, J.A. Measurement of building foundation and ground-borne vibrations due to surface trains and subways. Eng. Struct. 2013, 53, 102–111. [Google Scholar] [CrossRef]
- Andersen, L.; Jones, C.J.C. Coupled boundary and finite element analysis of vibration from railway tunnels—A comparison of two and three-dimensional models. J. Sound Vib. 2006, 293, 611–625. [Google Scholar] [CrossRef]


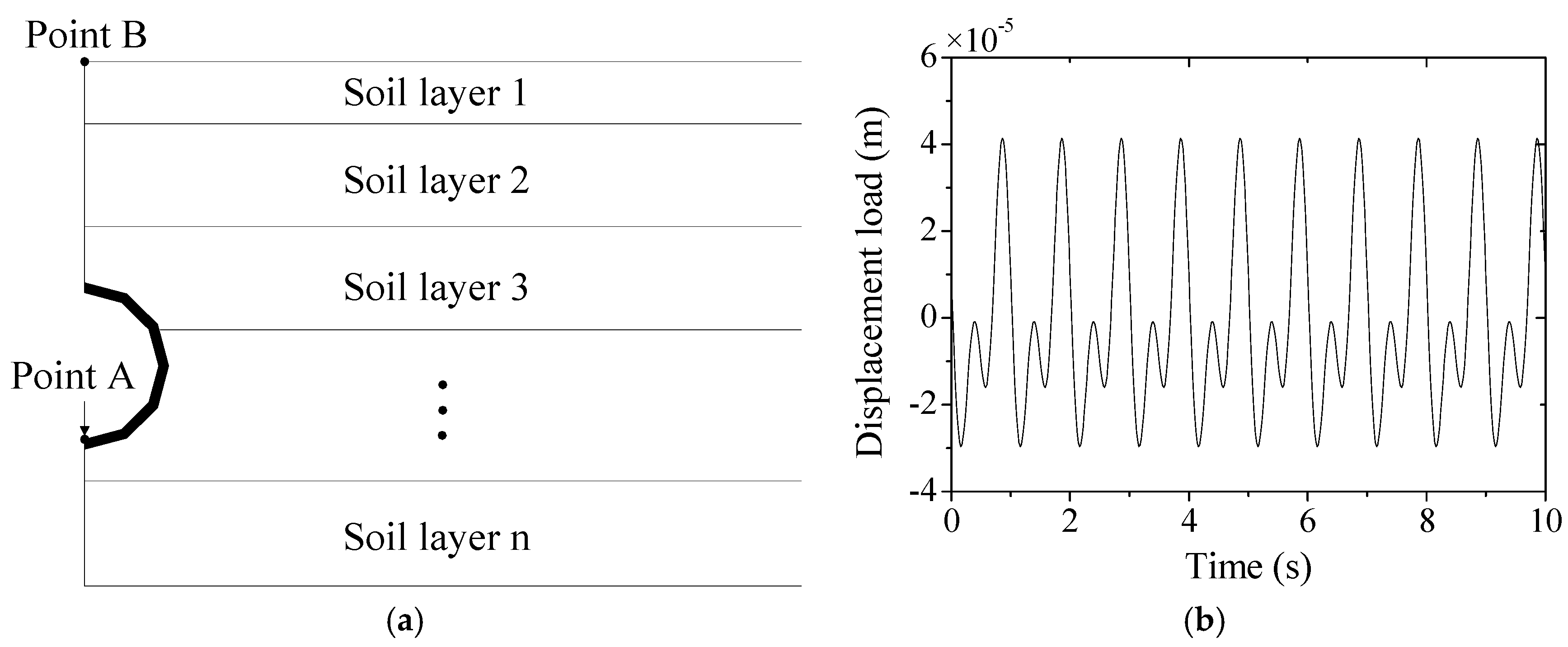












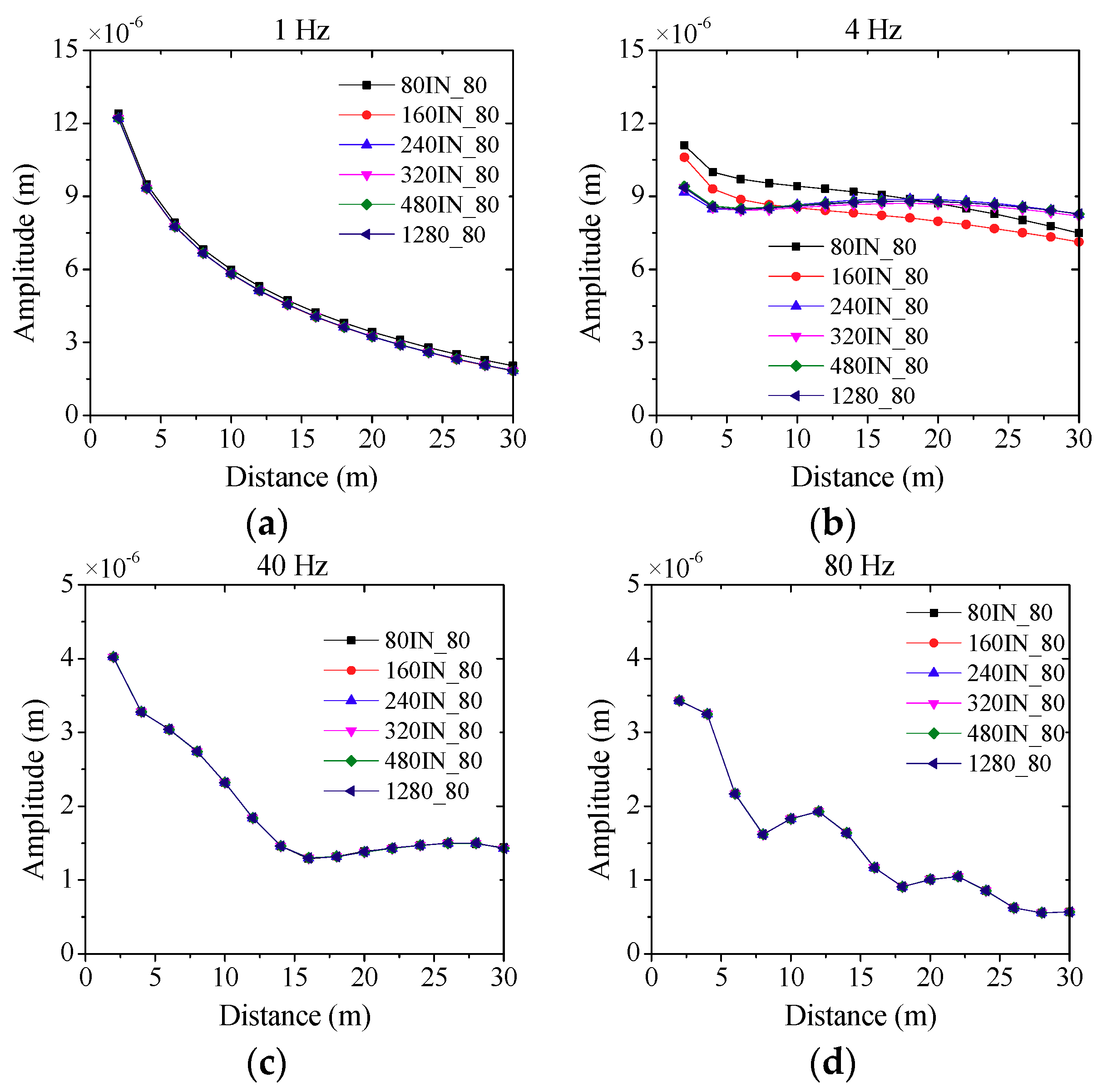
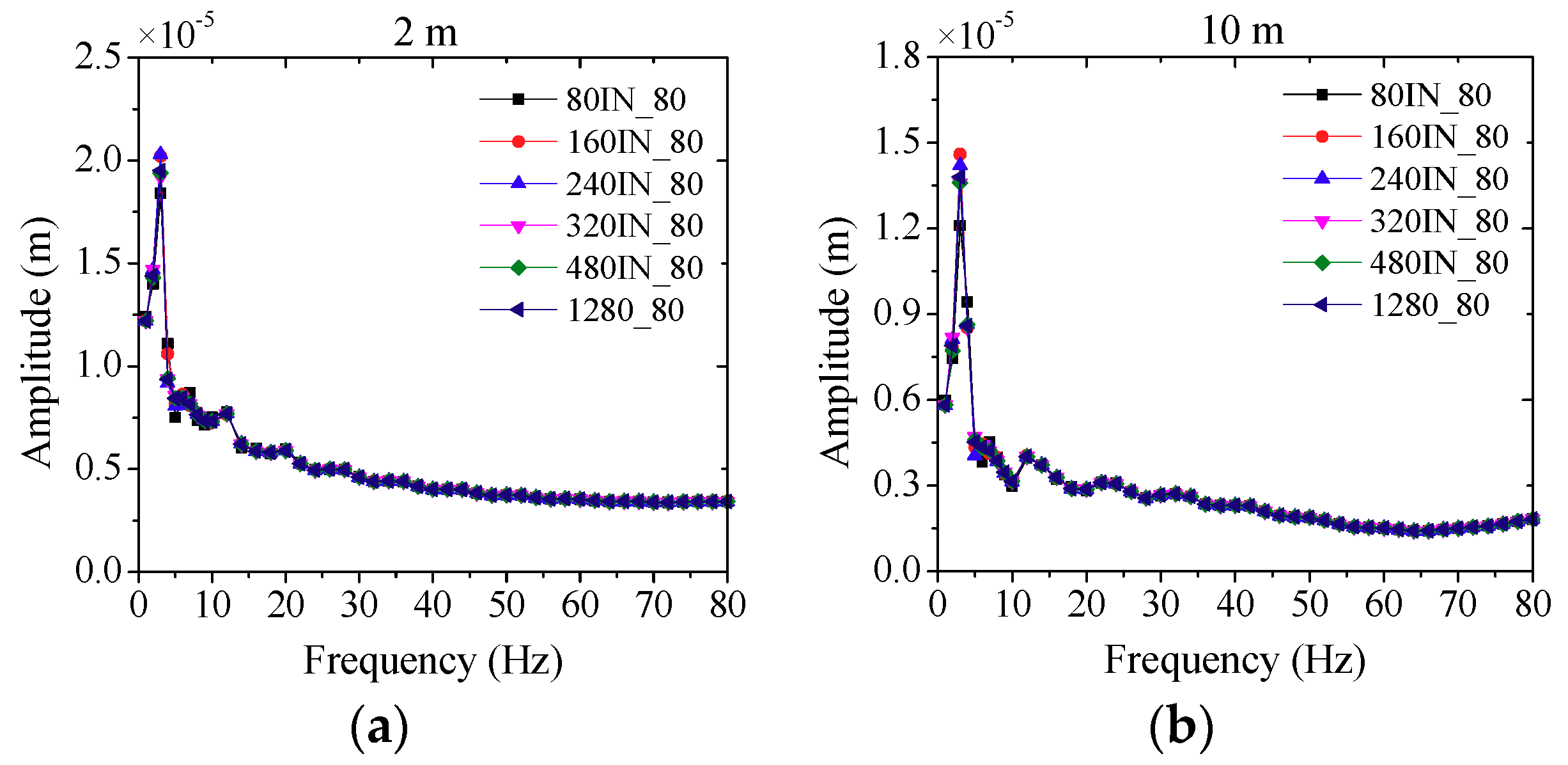




| Soil Layer | Thickness (m) | Depth (m) | Density (kg/m3) | Shear Wave Velocity (m/s) | Poisson’s Ratio |
|---|---|---|---|---|---|
| 1 | 1.00 | 1.00 | 1890 | 74 | 0.35 |
| 2 | 3.30 | 4.30 | 1850 | 89 | 0.30 |
| 3 | 2.10 | 6.40 | 1850 | 85 | 0.30 |
| 4 | 11.26 | 17.66 | 1790 | 108 | 0.30 |
| 5 | 26.40 | 44.06 | 1820 | 220 | 0.25 |
| 6 | 2.00 | 46.06 | 1930 | 189 | 0.25 |
| 7 | 2.00 | 48.06 | 1940 | 191 | 0.25 |
| 8 | 3.40 | 51.46 | 2040 | 195 | 0.25 |
| 9 | 8.05 | 59.51 | 1920 | 230 | 0.25 |
| 10 | 16.96 | 76.47 | 1950 | 220 | 0.25 |
| 11 | 2.20 | 78.67 | 1920 | 263 | 0.25 |
| 12 | 3.78 | 82.45 | 1960 | 267 | 0.25 |
| 13 | 2.99 | 85.44 | 1920 | 272 | 0.25 |
| 14 | 6.65 | 92.09 | 1940 | 279 | 0.25 |
| 15 | 4.36 | 96.45 | 1930 | 287 | 0.25 |
| 16 | 3.55 | 100.00 | 1920 | 296 | 0.25 |
| Case | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|
| L × H (m × m) | 240 × 80 | 240 × 160 | 240 × 320 | 240 × 640 | 240 × 1280 |
| Expression | 240IN_80 | 240IN_160 | 240IN_320 | 240IN_640 | 240IN_1280 |
| Case | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|
| L × H (m × m) | 80 × 80 | 160 × 80 | 240 × 80 | 320 × 80 | 480 × 80 |
| Expression | 80IN_80 | 160IN_80 | 240IN_80 | 320IN_80 | 480IN_80 |
| Case | 1 | 2 | 3 |
|---|---|---|---|
| L × H (m × m) | 240 × 80 | 240 × 160 | 240 × 240 |
| Expression | 240IN_80IN | 240IN _160IN | 240IN_240IN |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, J.; Li, P.; Lu, Z. Numerical Simulation and In-Situ Measurement of Ground-Borne Vibration Due to Subway System. Sustainability 2018, 10, 2439. https://doi.org/10.3390/su10072439
Yang J, Li P, Lu Z. Numerical Simulation and In-Situ Measurement of Ground-Borne Vibration Due to Subway System. Sustainability. 2018; 10(7):2439. https://doi.org/10.3390/su10072439
Chicago/Turabian StyleYang, Jinping, Peizhen Li, and Zheng Lu. 2018. "Numerical Simulation and In-Situ Measurement of Ground-Borne Vibration Due to Subway System" Sustainability 10, no. 7: 2439. https://doi.org/10.3390/su10072439
APA StyleYang, J., Li, P., & Lu, Z. (2018). Numerical Simulation and In-Situ Measurement of Ground-Borne Vibration Due to Subway System. Sustainability, 10(7), 2439. https://doi.org/10.3390/su10072439






