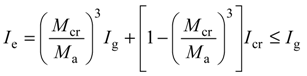Long-Term Flexural Behaviors of GFRP Reinforced Concrete Beams Exposed to Accelerated Aging Exposure Conditions
Abstract
:1. Introduction
2. Experimental Program
2.1. Materials
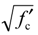 equation of ACI 318-11 [27].
equation of ACI 318-11 [27].
| FRP Bar Type | Bar Size | Nominal Diameter (mm) | Nominal Area (mm2) | Guaranteed Tensile Strength (ffu *: MPa) | Tensile Modulus of Elasticity (Ef *: GPa) | Tensile Strain (εu *: %) |
|---|---|---|---|---|---|---|
| GA series (Type A) | #4 | 13 | 126.7 | 690 | 40.8 | 1.50 |
| #5 | 16 | 197.9 | 655 | 40.8 | 1.50 | |
| GH series (Type B) | #4 | 13 | 126.7 | 1300 | 60.0 | 2.42 |
| #5 | 16 | 197.9 | 1259 | 64.1 | 2.24 | |
| Steel (Grade 60) | #4 | 12.7 | 129.0 | 620 (415: yield) | 200 | 9.00 |
| #5 | 15.875 | 200.0 | 620 (415: yield) | 200 | 9.00 |
2.2. Test Matrix and Design of Specimens for Fabrication

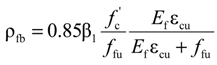

2.3. Sustained Loading and Accelerated Aging Method in the Chamber

2.4. Four-Point Bending Test

3. Test Results
3.1. Moment-Deflection Response

| Specimen I.D. | ρf/ρfb 1 or ρs/ρsb 2 | f'c (MPa) | Mu-th (kN·m) | Mu-exp (kN·m) | Δu-exp (mm) | Failure Mode 3 | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | Avg. | 1 | 2 | 3 | Avg. | |||||
| GA2-4-0 | 1.242 1 | 29.30 | 50.2 | 64.8 | 60.9 | 62.1 | 62.6 | 24.49 | 26.67 | 22.88 | 24.68 | C.C. |
| GA2-4-300 | 0.997 1 | 36.06 | 51.8 | 58.6 | 56.2 | 55.8 | 56.9 | 29.66 | 30.84 | 28.43 | 29.64 | B |
| GA3-5-0 | 2.157 1 | 29.30 | 68.1 | 93.4 | 88.2 | 92.1 | 91.2 | 17.89 | 20.81 | 19.43 | 19.38 | C.C. |
| GA3-5-300 | 1.678 1 | 36.47 | 72.9 | 86.1 | 79.4 | 82.6 | 82.7 | 21.31 | 22.45 | 23.36 | 22.37 | C.C. |
| GH2-4-0 | 2.273 1 | 29.92 | 52.6 | 70.1 | 66.7 | 68.9 | 68.6 | 23.14 | 25.31 | 23.76 | 24.07 | C.C. |
| GH2-4-300 | 1.776 1 | 36.47 | 59.9 | 69.9 | 68.1 | 66.9 | 68.3 | 28.93 | 26.43 | 25.76 | 27.04 | C.C. |
| GH3-5-0 | 4.761 1 | 29.92 | 74.8 | 101.4 | 98.3 | 99.4 | 99.7 | 17.22 | 21.09 | 20.36 | 19.56 | C.C. |
| GH3-5-300 | 3.578 1 | 36.61 | 85.2 | 96.1 | 94.0 | – | 95.1 | 20.75 | 23.41 | – | 22.08 | C.C. |
| S2-4-0 | 0.155 2 | 29.10 | 27.3 | 45.9 | 41.7 | 43.7 | 43.8 | 31.17 | 30.83 | 33.14 | 31.71 | S.Y. + C.C. |
| S2-4-300 | 0.119 2 | 36.06 | 27.8 | 34.6 | 33.9 | – | 34.3 | 35.14 | 35.29 | – | 35.22 | S.Y. + C.C. |
| S3-5-0 | 0.352 2 | 29.92 | 60.1 | 84.6 | 82.1 | 80.6 | 82.4 | 25.29 | 24.80 | 27.16 | 25.75 | S.Y. + C.C. |
| S3-5-300 | 0.267 2 | 36.75 | 61.8 | 75.1 | 72.6 | – | 73.9 | 21.34 | 20.09 | – | 20.72 | S.Y. + C.C. |
3.2. Deflection
| Loading and support condition |  | |
| Theoretical deflection |  | (3) |
| ACI 440 1.R-06 [7] | 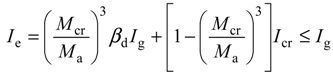 where βd = 0.2(ρf/ρfb) ≤ 1.0 where βd = 0.2(ρf/ρfb) ≤ 1.0 | (4) |
| Toutanji and Saffi [29] | For (Ef/Es) ρf < 0.3, 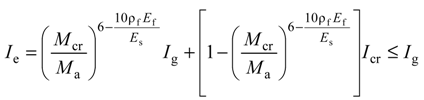 | (5a) |
| Bischoff and Gross [30] | 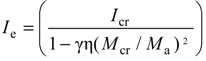 where Ma = PL/6, η = 1−Icr/Ig where Ma = PL/6, η = 1−Icr/IgFor considering tension stiffening, γ = 1.72–0.72Mcr/Ma For considering no tension stiffening in the cracked region, γ = 0.35Mcr/Ma | (6) |

| Specimen I.D. | Reinforcement Ratio (Af/bd) | Deflection (mm) |  |  |  | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| Exp. * | Eq. (4) (CE = 1.0) | Eq. (5) | Eq. (6) | |||||||
| GA2-4-0 | 0.0045 (0.45%) | 24.488 | 21.699 | 16.969 | 23.404 | 0.886 | 0.693 | 0.956 | ||
| GA2-4-300 | 29.655 | 21.699 | 16.969 | 23.404 | 0.732 | 0.572 | 0.789 | |||
| GA3-5-0 | 0.0109 (1.09%) | 17.882 | 16.318 | 13.257 | 19.870 | 0.913 | 0.741 | 1.111 | ||
| GA3-5-300 | 21.311 | 16.318 | 13.257 | 19.870 | 0.766 | 0.622 | 0.932 | |||
| GH2-4-0 | 0.0045 (0.45%) | 23.146 | 17.672 | 14.552 | 20.359 | 0.764 | 0.629 | 0.880 | ||
| GH2-4-300 | 28.931 | 17.672 | 14.552 | 20.359 | 0.611 | 0.503 | 0.704 | |||
| GH3-5-0 | 0.0109 (1.09%) | 17.223 | 15.160 | 12.866 | 17.509 | 0.880 | 0.747 | 1.017 | ||
| GH3-5-300 | 20.752 | 15.160 | 12.866 | 17.509 | 0.731 | 0.620 | 0.844 | |||
| 0 day | Mean | – | – | – | – | – | 0.86 | 0.71 | 0.99 | |
| Std. | – | – | – | – | – | 0.07 | 0.06 | 0.09 | ||
| 300 days | Mean | – | – | – | – | – | 0.71 | 0.58 | 0.82 | |
| Std. | – | – | – | – | – | 0.07 | 0.06 | 0.09 | ||
3.3. Failure Mode and Cracks

3.4. Ductility
| Approach Type | Deformability Index | ||
|---|---|---|---|
Energy-based approach | Naaman and Jeong [33] |  | (7a) |
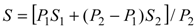 | (7b) | ||
| Grace et al. [31] | 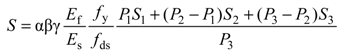 | (8) | |
| α: stirrup factor (GFRP: 0.95) β: failure mode factor (compression and flexure: 1.0) γ: reinforcement factor (GFRP: 4.0) | |||
| Moment and deformation-based approach | Jaeger et al. [32] | 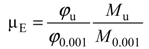 | (9) |
| Zou [34] | 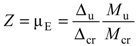 | (10) | |
Deflection-based approach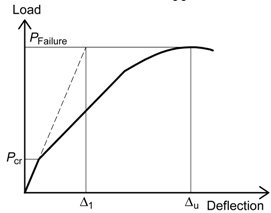 | Abdelrahman et al. [35] | 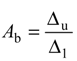 | (11) |
| Specimen I.D. | M0.001 (kN·m) | Mcr (kN·m) | Mu (kN·m) | φ0.001 (rad/mm) | φu (rad/mm) | Δcr (mm) | Δu (mm) | Δu/Δcr | Δ1 (mm) |
|---|---|---|---|---|---|---|---|---|---|
| GA2-4-0 | 22.07 | 16.10 | 64.82 | 0.000024 | 0.000048 | 1.245 | 24.488 | 19.67 | 4.064 |
| GA2-4-300 | 24.23 | 15.23 | 58.57 | 0.000031 | 0.000071 | 1.372 | 29.655 | 21.61 | 4.826 |
| GA3-5-0 | 29.65 | 16.03 | 93.40 | 0.000016 | 0.000054 | 0.737 | 17.881 | 24.26 | 3.302 |
| GA3-5-300 | 27.19 | 15.58 | 86.07 | 0.000018 | 0.000059 | 0.991 | 21.311 | 21.51 | 4.572 |
| GH2-4-0 | 25.47 | 14.68 | 70.12 | 0.000016 | 0.000059 | 1.194 | 23.146 | 19.38 | 4.572 |
| GH2-4-300 | 22.24 | 14.26 | 69.85 | 0.000021 | 0.000067 | 1.092 | 28.932 | 26.49 | 5.334 |
| GH3-5-0 | 33.00 | 16.33 | 101.39 | 0.000013 | 0.000044 | 0.533 | 17.223 | 32.31 | 3.302 |
| GH3-5-300 | 27.84 | 15.70 | 96.07 | 0.000016 | 0.000048 | 0.787 | 20.752 | 26.37 | 5.334 |
| S2-4-0 | 31.21 | 15.01 | 45.92 | 0.000010 | 0.000079 | 0.787 | 31.173 | 39.61 | 2.667 |
| S2-4-300 | 28.74 | 12.02 | 34.60 | 0.000012 | 0.000096 | 0.533 | 35.143 | 65.93 | 2.159 |
| S3-5-0 | 34.03 | 15.13 | 84.62 | 0.000008 | 0.000071 | 1.143 | 25.287 | 22.12 | 3.175 |
| S3-5-300 | 32.56 | 12.02 | 75.05 | 0.000009 | 0.000083 | 0.787 | 21.343 | 27.12 | 2.413 |
| Specimen I.D. | Deformability Index | Normalized Deformability | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Naaman [33] | Grace [31] | Zou [34] | Jaeger [32] | Abdelrahman [35] | Naaman [33] | Grace [31] | Zou [34] | Jaeger [32] | Abdelrahman [35] | |
| GA2-4-0 | 2.13 | 1.31 | 79.19 | 5.87 | 6.03 | 1.00 | 1.00 | 1.00 | 1.00 | 1.00 |
| GA2-4-300 | 1.51 | 0.99 | 83.12 | 5.54 | 6.14 | 0.71 | 0.76 | 1.04 | 0.93 | 1.02 |
| GA3-5-0 | 1.12 | 0.98 | 141.36 | 10.63 | 5.42 | 1.00 | 1.00 | 1.00 | 1.00 | 1.00 |
| GA3-5-300 | 0.98 | 1.11 | 118.80 | 10.38 | 4.66 | 0.88 | 1.13 | 0.84 | 0.98 | 0.86 |
| GH2-4-0 | 1.35 | 1.13 | 92.59 | 10.15 | 5.07 | 1.00 | 1.00 | 1.00 | 1.00 | 1.00 |
| GH2-4-300 | 1.04 | 1.29 | 129.78 | 10.02 | 5.42 | 0.77 | 1.14 | 1.40 | 0.99 | 1.07 |
| GH3-5-0 | 1.28 | 1.11 | 200.63 | 10.40 | 5.22 | 1.00 | 1.00 | 1.00 | 1.00 | 1.00 |
| GH3-5-300 | 1.32 | 1.03 | 161.35 | 10.25 | 3.89 | 1.03 | 0.93 | 0.80 | 0.99 | 0.75 |
| S2-4-0 | 6.22 | 4.16 | 121.18 | 11.62 | 11.63 | 1.00 | 1.00 | 1.00 | 1.00 | 1.00 |
| S2-4-300 | 7.51 | 5.69 | 189.78 | 9.63 | 16.28 | 1.21 | 1.37 | 1.57 | 0.83 | 1.40 |
| S3-5-0 | 2.93 | 1.96 | 123.73 | 22.07 | 7.96 | 1.00 | 1.00 | 1.00 | 1.00 | 1.00 |
| S3-5-300 | 4.57 | 3.06 | 169.33 | 21.26 | 8.85 | 1.56 | 1.56 | 1.37 | 0.96 | 1.11 |
4. Conclusions
- The substitution of GFRP reinforcement for steel bars in the concrete beams affected the load-deflection response with higher strength and less deflection at the failure stage due to the non-ductile behavior of FRP reinforcement. Flexural GFRP reinforcement in the concrete led to a significant improvement in the flexural strength.
- Environmental conditioning has an effect on the flexural strength degradation of both RC-steel and RC-GFRP beams. The test results confirm that steel reinforcement had a higher reduction in flexural strength than GFRP reinforcement. It can be concluded that the substitution of GFRP reinforcement for steel reinforcement in the flexural members affected the durability positively.
- The rate of flexural strength degradation of concrete beams reinforced with Type A GFRP reinforcement (i.e., GA series) was greater than those with Type B GFRP reinforcement (i.e., GH series). This can be attributed to the coating materials: the Type B GFRP bars with a sand-coated surface seem to have better protection against moisture and temperature.
- For both unaged and aged specimens, the predictions of flexural deflection by the ACI 440.1R-06 [7], Toutanji and Saafi [15] and Bischoff and Gross [30] models were in close agreement with the experimental data at the service load level. However, as the applied load and aging duration increased, the ACI 440.1R-06 [7] and Toutanji and Saafi [29] models tend to underestimate deflections. The Bischoff and Gross model [30] provided the smallest error and is the best predictor of deflections before and after accelerated aging.
- If an environmental strength reduction factor (CE) of 0.7 was used for the flexure design of a member failing in compression with GFRP reinforcement, the ACI 440.1R-06 model [7] was still conservative under the accelerated environmental aging, with a 47 °C temperature and 80% relative humidity. Therefore, the CE factor is appropriate for estimating the reduced capacity of GFRP reinforcement until 300 days of accelerated environmental aging.
- Except for the Jaeger index [32], there is no general trend related to the aging duration (days). Rationally, the Jaeger index [32] represents the degradation of ductility due to the aging conditions. However, the deformability indices attained from the Jaeger index were higher than the recommended value of four in ISIS-Canada. Finally, there is still no general agreement on how much degradation of deformability is enough for the aged structures. Further investigation is necessary to determine the requirement of deformability.
Acknowledgments
Conflicts of Interest
References
- Almusallam, T.H.; Al-Salloum, Y.A.; Alsayed, S.H.; Mosallam, A.S. Durability and long-term behavior of reinforced concrete beams strengthened with FRP composites. In Proceedings of International Conference on FRP Composites in Civil Engineering (CICE 2001), Hong Kong, China, 12–15 December 2001; pp. 1579–1588.
- Micelli, F.; Nanni, A. Durability of FRP rods for concrete structures. Constr. Build. Mater. 2004, 18, 491–503. [Google Scholar] [CrossRef]
- Böer, P.; Holliday, L.; Kang, T.H.-K. Independent environmental effects on durability of fiber-reinforced polymer wraps in civil applications: A review. Constr. Build. Mater. 2013, 48, 360–370. [Google Scholar] [CrossRef]
- Böer, P.; Holliday, L.; Kang, T.H.-K. Interaction of environmental factors on fiber-reinforced polymer composites and their inspection & maintenance: A review. Constr. Build. Mater. 2014, 50, 209–218. [Google Scholar] [CrossRef]
- Choi, D.-U.; Kang, T.H.-K.; Ha, S.-S.; Kim, K.-H.; Kim, W. Flexural and bond behavior of concrete beams strengthened with hybrid carbon-glass fiber-reinforced polymer sheets. ACI Struct. J. 2011, 108, 90–98. [Google Scholar]
- American Association of State Highway and Transportation Officials (AASHTO). AASHTO LRFD Bridge Design Guide Specifications for GFRP-Reinforced Concrete Bridge Decks and Traffic Railings; AASHTO: Washington, DC, USA, 2009; p. 68. [Google Scholar]
- American Concrete Institute (ACI). Guide for the Design and Construction of Structural Concrete Reinforced with FRP Bars; ACI 440.1R-06; ACI: Farmington Hills, MI, USA, 2006. [Google Scholar]
- Branson, D.E.; Metz, G.A. Instantaneous and Time-Dependent Deflections of Simple and Continuous Reinforced Concrete Beams; Alabama Highway Department, Bureau of Public Roads: Montgomery, AL, USA, 1965. [Google Scholar]
- Razaqpur, A.G.; Isgor, O.B. Rational method for calculating deflection of continuous FRP R/C beams. ACI Spec. Publ. 2003, 210, 191–208. [Google Scholar]
- Gao, D.; Benmokrane, B.; Masmoudi, R. A Calculating Method of Flexural Properties of FRP-Reinforced Concrete Beam: Part 1–Crack Width and Deflection; Department of Civil Engineering, University of Sherbrooke: Sherbrooke, QC, Canada, 1998. [Google Scholar]
- Au, F.T.K.; Du, J.S. Deformability of concrete beams with unbonded FRP tendons. Eng. Struct. 2008, 30, 3764–3770. [Google Scholar] [CrossRef]
- Jeong, S. Evaluation of Ductility in Prestressed Concrete Beams Using Fiber Reinforced Plastic Tendons. Ph.D. Thesis, University of Michigan, Ann Arbor, MI, USA, January 1994. [Google Scholar]
- Robert, M.; Benmokrane, B. Combined effects of saline solution and moist concrete on long-term durability of gfrp reinforcing bars. Constr. Build. Mater. 2013, 38, 274–284. [Google Scholar] [CrossRef]
- Trejo, D.; Aguiniga, F.; Yuan, R.; James, R.W.; Keating, P.B. Characterization of Design Parameters for Fiber Reinforced Polymer Composite Reinforced Concrete Systems; Texas Transportation Institute Research Report; The Texas A&M University System: College Station, TX, USA, 2005. [Google Scholar]
- Tannous, F.E.; Saadatmanesh, H. Environmental effects on the mechanical properties of E-glass frp rebars. ACI Mater. J. 1998, 95, 87–100. [Google Scholar]
- Mukherjee, A.; Arwikar, S.J. Performance of glass fiber-reinforced polymer reinforcing bars in tropical environments—Part I: Structural scale tests. ACI Struct. J. 2005, 102, 745–753. [Google Scholar]
- Bakis, C.E.; Nanni, A.; Terosky, J.A.; Koehler, S.W. Self-monitoring, pseudo-ductility, hybrid FRP reinforcement rods for concrete applications. Compos. Sci. Technol. 2001, 61, 815–823. [Google Scholar] [CrossRef]
- Mufti, A.; Banthia, N.; Benmokrane, B.; Boulfiza, M.; Newhook, J. Durability of GFRP composite rods. Am. Concr. Inst. Concr. Int. 2007, 37–42. [Google Scholar]
- Mufti, A.; Onofrei, M.; Benmokrane, B.; Banthia, N.; Boulfiza, M.; Newhook, J.P.; Bakht, B.; Tadros, G.S.; Brett, P. Field study of glass-fibre-reinforced polymer durability in concrete. Can. J. Civ. Eng. 2007, 34, 355–366. [Google Scholar] [CrossRef]
- Benmokrane, B.; Cousin, P. University of Sherbrook GFRP Durability Study Report; Intelligent Sensing for Innovative Structures (ISIS): Winnipeg, MB, Canada, 2005. [Google Scholar]
- Kamal, A.S.M.; Boulfiza, M. Durability of GFRP rebars in simulated concrete solutions under accelerated aging conditions. ASCE J. Compos. Constr. 2012, 15, 473–481. [Google Scholar] [CrossRef]
- Benmokrane, B.; Wnag, P.; Pavate, T.; Robert, M. Durability of FRP Composites for Civil Infrastructure Applications; Whittles Publishing: Dunbeath, Scotland, 2006. [Google Scholar]
- American Society for Testing and Materials (ASTM). Standard Test Method for Ignition Loss of Cured Reinforced Resins; ASTM D 2584–13; ASTM: West Conshohocken, PA, USA, 2013. [Google Scholar]
- Trejo, D.; Gardoni, P.; Kim, J.J. Long-term performance of GFRP reinforcement embedded in concrete. ACI Mater. J. 2011, 108, 605–613. [Google Scholar]
- Gardoni, P.; Trejo, D.; Kim, Y.H. Time-variant capacity model for GFRP bars embedded in concrete. ASCE J. Eng. Mech. 2013, 139, 1435–1445. [Google Scholar] [CrossRef]
- Kim, Y.H.; Trejo, D.; Gardoni, P. Time-variant capacity and reliability of GFRP-reinforced bridge decks. ASCE J. Compos. Constr. 2012, 16, 359–370. [Google Scholar] [CrossRef]
- American Concrete Institute (ACI). Building Code Requirements for Structural Concrete and Commentary; ACI 318, 318–11; ACI: Farmington Hills, MI, USA, 2011. [Google Scholar]
- Park, Y. Long-Term Performance of GFRP Reinforced Concrete Beams and Bars Subjected to Aggressive Environments. Ph.D. Thesis, The University of Texas at Arlington, Arlington, TX, USA, May 2012. [Google Scholar]
- Toutanji, H.A.; Saafi, M. Flexural behavior of concrete beams reinforced with glass fiber-reinforced polymer (GFRP) bars. ACI Struct. J. 2000, 97, 712–719. [Google Scholar]
- Bischoff, P.; Gross, S. Equivalent moment of inertia based on integration of curvature. ASCE J. Compos. Constr. 2011, 15, 263–273. [Google Scholar] [CrossRef]
- Grace, N.; Soliman, A.; Abdel-Sayed, G.; Saleh, K. Behavior and ductility of simple and continuous frp reinforced beams. ASCE J. Compos. Constr. 1998, 2, 186–194. [Google Scholar]
- Jaeger, L.G.; Mufti, A.A.; Tadros, G. The concept of the overall performance factor in rectangular-section reinforced concrete members. In Proceedings of the 3rd International Symposium on Non-Metallic (FRP) Reinforcement for Concrete Structures, Sapporo, Japan, 14–16 October 1997; pp. 551–559.
- Naaman, A.; Jeong, S.M. Structural ductility of concrete beams prestressed with FRP tendons. In non-metric (FRP) reinforcement for concrete structures. In Proceedings of the 2nd International RILEM Symposium (FRPRCS-2), Ghent, Belgium, 23–25 August 1995; pp. 379–386.
- Zou, P. Flexural behavior and deformability of fiber reinforced polymer prestressed concrete beams. ASCE J. Compos. Constr. 2003, 7, 275–284. [Google Scholar] [CrossRef]
- Abdelrahman, A.; Tadros, G.; Rizkalla, S. Test model for the first canadian smart highway bridge. ACI Struct. J. 1995, 92, 1–9. [Google Scholar]
- Newhook, J.; Svecova, D. Reinforcing Concrete Structures with Fibre Reinforced Polymers; The Canadian Network of Centres of Excellence, ISIS Canada, University of Manitoba: Winnipeg, Canada, 2007. [Google Scholar]
© 2014 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Park, Y.; Kim, Y.H.; Lee, S.-H. Long-Term Flexural Behaviors of GFRP Reinforced Concrete Beams Exposed to Accelerated Aging Exposure Conditions. Polymers 2014, 6, 1773-1793. https://doi.org/10.3390/polym6061773
Park Y, Kim YH, Lee S-H. Long-Term Flexural Behaviors of GFRP Reinforced Concrete Beams Exposed to Accelerated Aging Exposure Conditions. Polymers. 2014; 6(6):1773-1793. https://doi.org/10.3390/polym6061773
Chicago/Turabian StylePark, Yeonho, Young Hoon Kim, and Swoo-Heon Lee. 2014. "Long-Term Flexural Behaviors of GFRP Reinforced Concrete Beams Exposed to Accelerated Aging Exposure Conditions" Polymers 6, no. 6: 1773-1793. https://doi.org/10.3390/polym6061773
APA StylePark, Y., Kim, Y. H., & Lee, S.-H. (2014). Long-Term Flexural Behaviors of GFRP Reinforced Concrete Beams Exposed to Accelerated Aging Exposure Conditions. Polymers, 6(6), 1773-1793. https://doi.org/10.3390/polym6061773