Influence of Sudden Stratospheric Warmings on the Migrating Diurnal Tide in the Equatorial Middle Atmosphere Observed by Aura/Microwave Limb Sounder
Abstract
:1. Introduction
2. Dataset and Data Analysis
3. Results
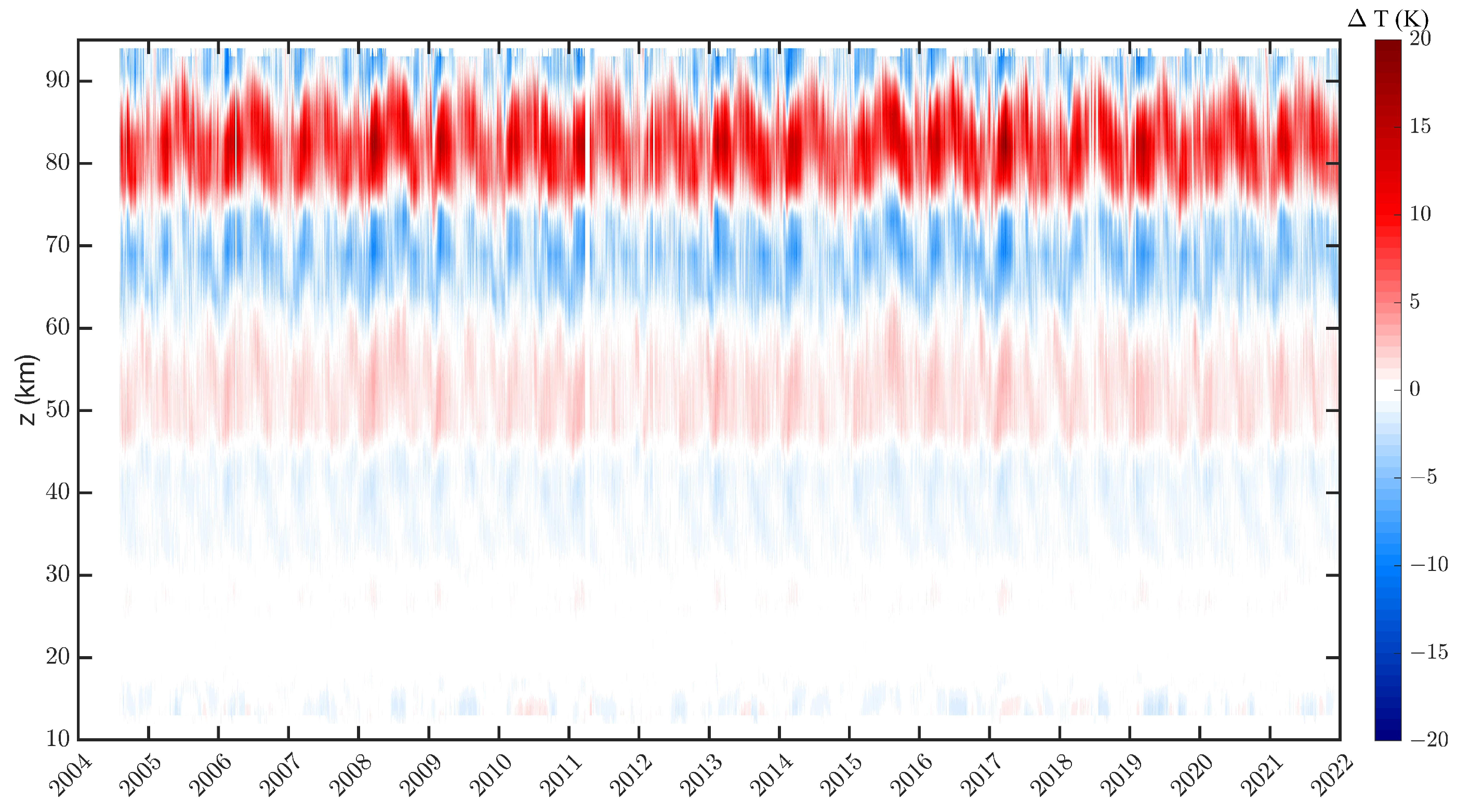
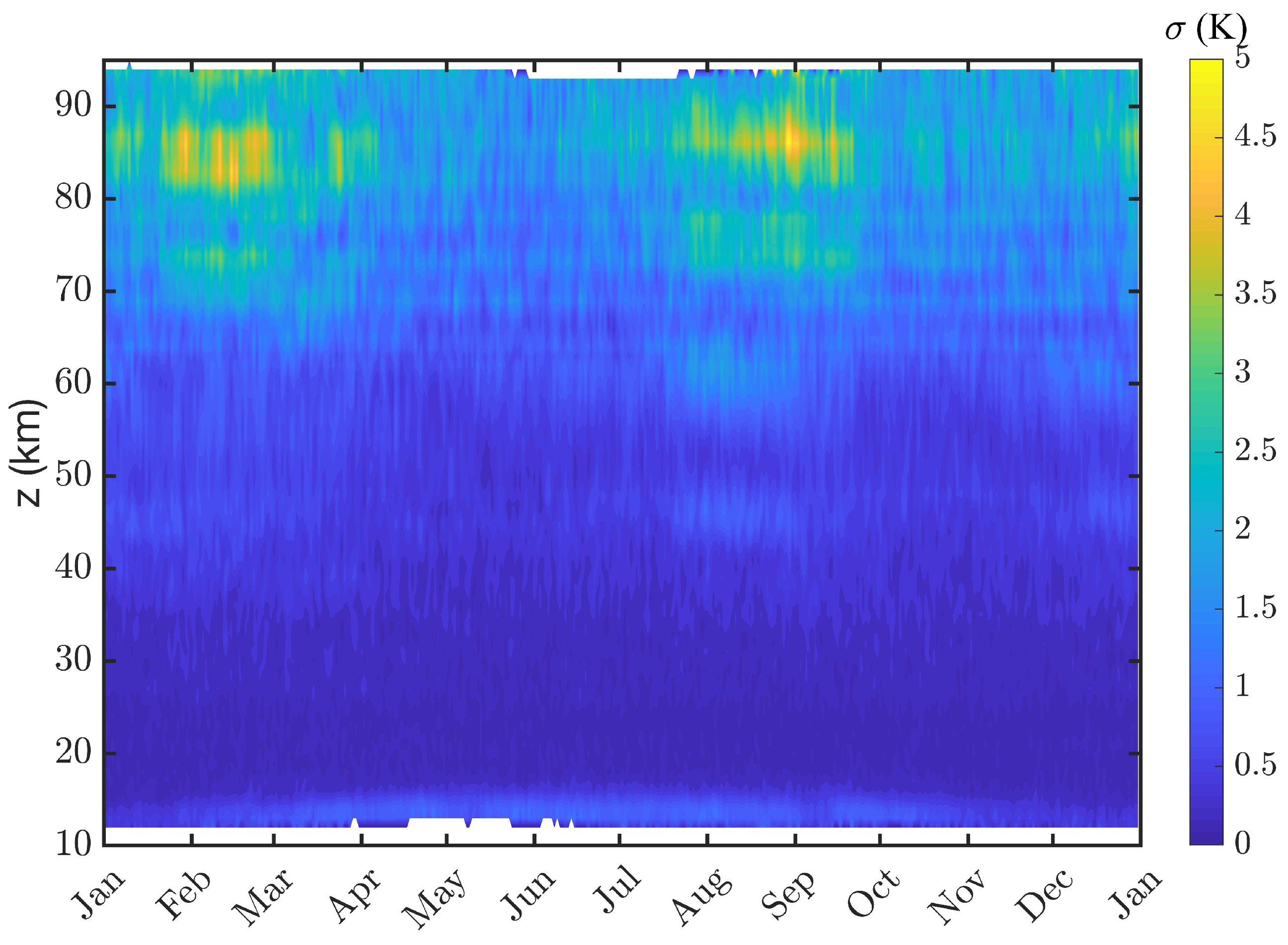
3.1. Characteristics of the Equatorial DW1 Proxy
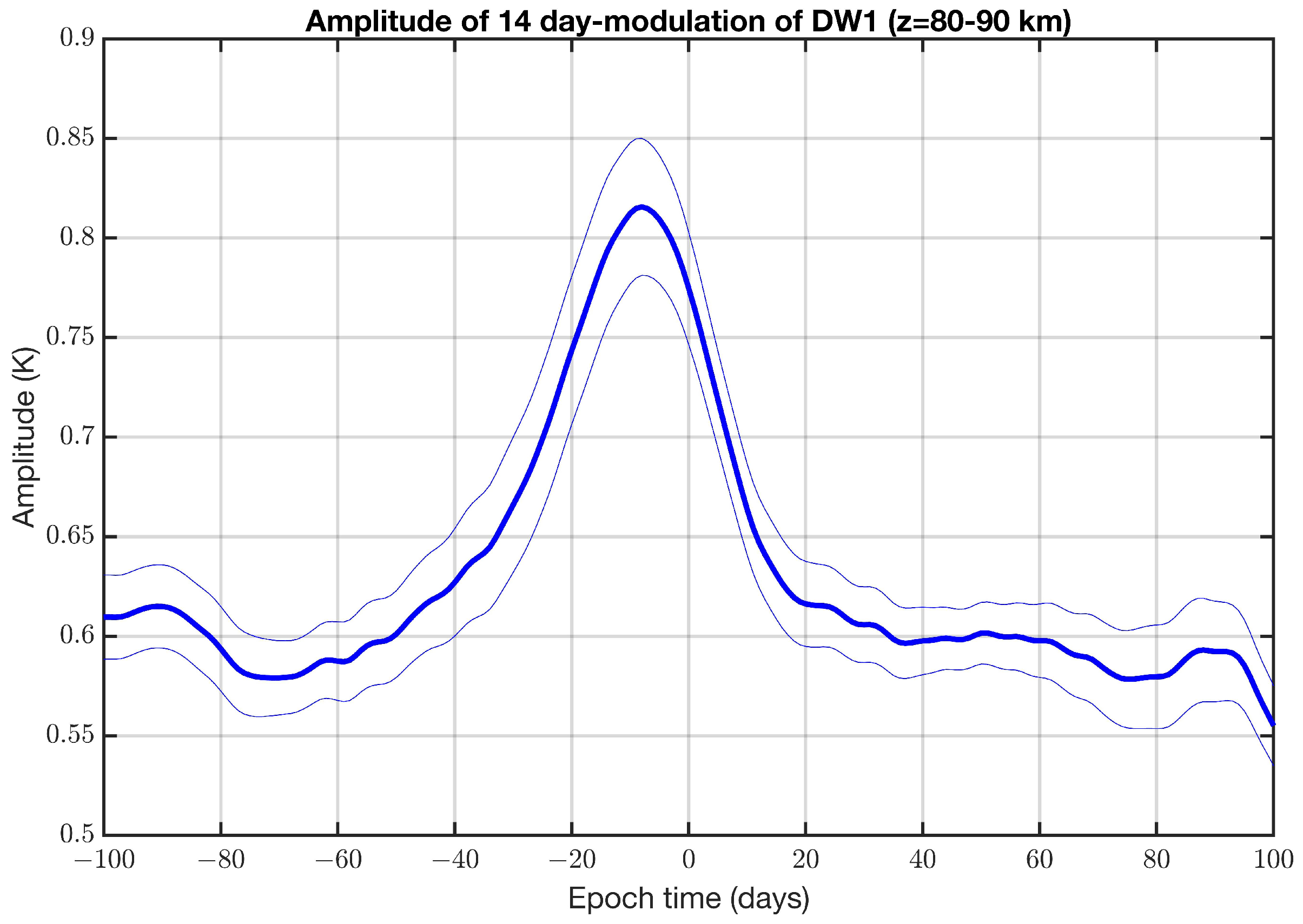
3.2. Composite Analysis of the Equatorial DW1 Behaviour during Major SSWs
4. Discussion
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dieminger, W.; Hartmann, G.K.; Leitinger, R. (Eds.) Atmospheric Tides. In The Upper Atmosphere: Data Analysis and Interpretation; Springer: Berlin/Heidelberg, Germany, 1996; pp. 97–109. [Google Scholar] [CrossRef]
- Alexander, S.P.; Tsuda, T. Observations of the diurnal tide during seven intensive radiosonde campaigns in Australia and Indonesia. J. Geophys. Res. Atmos. 2008, 113, D04109. [Google Scholar] [CrossRef]
- Sakazaki, T.; Fujiwara, M.; Zhang, X.; Hagan, M.E.; Forbes, J.M. Diurnal tides from the troposphere to the lower mesosphere as deduced from TIMED/SABER satellite data and six global reanalysis data sets. J. Geophys. Res. Atmos. 2012, 117, D13108. [Google Scholar] [CrossRef]
- Garcia, R.R. On the Structure and Variability of the Migrating Diurnal Temperature Tide Observed by SABER. J. Atmos. Sci. 2023, 80, 687–704. [Google Scholar] [CrossRef]
- Lindzen, R.S.; Chapman, S. Atmospheric tides. Space Sci. Rev. 1969, 10, 3–188. [Google Scholar] [CrossRef]
- Forbes, J.M. Atmospheric tides: 1. Model description and results for the solar diurnal component. J. Geophys. Res. Space Phys. 1982, 87, 5222–5240. [Google Scholar] [CrossRef]
- Hagan, M.E.; Forbes, J.M. Migrating and nonmigrating diurnal tides in the middle and upper atmosphere excited by tropospheric latent heat release. J. Geophys. Res. Atmos. 2002, 107, ACL 6-1–ACL 6-15. [Google Scholar] [CrossRef]
- Oberheide, J.; Hagan, M.E.; Roble, R.G. Tidal signatures and aliasing in temperature data from slowly precessing satellites. J. Geophys. Res. Space Phys. 2003, 108, 1055. [Google Scholar] [CrossRef]
- Waters, J.W.; Froidevaux, L.; Harwood, R.S.; Jarnot, R.F.; Pickett, H.M.; Read, W.G.; Siegel, P.H.; Cofield, R.E.; Filipiak, M.J.; Flower, D.A.; et al. The Earth Observing System Microwave Limb Sounder (EOS MLS) on the Aura satellite. IEEE Trans. Geosci. Remote Sens. 2006, 44, 1075–1092. [Google Scholar] [CrossRef]
- Forbes, J.M.; Vincent, R.A. Effects of mean winds and dissipation on the diurnal propagating tide: An analytic approach. Planet. Space Sci. 1989, 37, 197–209. [Google Scholar] [CrossRef]
- Zhou, X.; Wan, W.; Yu, Y.; Ning, B.; Hu, L.; Yue, X. New Approach to Estimate Tidal Climatology From Ground- and Space-Based Observations. J. Geophys. Res. Space Phys. 2018, 123, 5087–5101. [Google Scholar] [CrossRef]
- Salinas, C.C.J.H.; Wu, D.L.; Lee, J.N.; Chang, L.C.; Qian, L.; Liu, H. Aura/MLS observes and SD-WACCM-X simulates the seasonality, quasi-biennial oscillation and El Niño–Southern Oscillation of the migrating diurnal tide driving upper mesospheric CO primarily through vertical advection. Atmos. Chem. Phys. 2023, 23, 1705–1730. [Google Scholar] [CrossRef]
- Xu, J.; Smith, A.K.; Liu, H.L.; Yuan, W.; Wu, Q.; Jiang, G.; Mlynczak, M.G.; Russell, J.M., III; Franke, S.J. Seasonal and quasi-biennial variations in the migrating diurnal tide observed by Thermosphere, Ionosphere, Mesosphere, Energetics and Dynamics (TIMED). J. Geophys. Res. Atmos. 2009, 114, D13107. [Google Scholar] [CrossRef]
- Matsuno, T. Dynamical Model of Stratospheric Sudden Warming. J. Atmos. Sci. 1971, 28, 1479–1494. [Google Scholar] [CrossRef]
- Schoeberl, M.R. Stratospheric Warmings—Observations and Theory. Rev. Geophys. 1978, 16, 521–538. [Google Scholar] [CrossRef]
- McInturff, R. Stratospheric Warmings: Synoptic, Dynamic and General Circulation Aspects; NASA References Publ. NASA-RP-1017; National Meteorological Center: Washington, DC, USA, 1978. [Google Scholar]
- Matthewman, N.J.; Esler, J.G.; Charlton-Perez, A.J.; Polvani, L.M. A New Look at Stratospheric Sudden Warmings. Part III: Polar Vortex Evolution and Vertical Structure. J. Clim. 2009, 22, 1566–1585. [Google Scholar] [CrossRef]
- Pedatella, N.M.; Chau, J.L.; Schmidt, H.; Goncharenko, L.P.; Stolle, C.; Hocke, K.; Harvey, V.; Funke, B.; Siddiqui, T.A. How Sudden stratospheric warmings affect the whole atmosphere. EOS Trans. AGU 2018, 99, 35–38. [Google Scholar] [CrossRef]
- Lossow, S.; McLandress, C.; Jonsson, A.I.; Shepherd, T.G. Influence of the Antarctic ozone hole on the polar mesopause region as simulated by the Canadian Middle Atmosphere Model. J. Atmos. Sol. Terr. Phys. 2012, 74, 111–123. [Google Scholar] [CrossRef]
- Lubis, S.W.; Omrani, N.E.; Matthes, K.; Wahl, S. Impact of the Antarctic Ozone Hole on the Vertical Coupling of the Stratosphere–Mesosphere–Lower Thermosphere System. J. Atmos. Sci. 2016, 73, 2509–2528. [Google Scholar] [CrossRef]
- Zülicke, C.; Becker, E. The structure of the mesosphere during sudden stratospheric warmings in a global circulation model. J. Geophys. Res. Atmos. 2013, 118, 2255–2271. [Google Scholar] [CrossRef]
- Siddiqui, T.A.; Chau, J.L.; Stolle, C.; Yamazaki, Y. Migrating solar diurnal tidal variability during Northern and Southern Hemisphere Sudden Stratospheric Warmings. Earth Planets Space 2022, 74, 101. [Google Scholar] [CrossRef]
- Harvey, V.L.; Randall, C.E.; Bailey, S.M.; Becker, E.; Chau, J.L.; Cullens, C.Y.; Goncharenko, L.P.; Gordley, L.L.; Hindley, N.P.; Lieberman, R.S.; et al. Improving ionospheric predictability requires accurate simulation of the mesospheric polar vortex. Front. Astron. Space Sci. 2022, 9, 1041426. [Google Scholar] [CrossRef]
- Wang, J.; Yi, W.; Wu, J.; Chen, T.; Xue, X.; Zeng, J.; Vincent, R.A.; Reid, I.M.; Batista, P.P.; Buriti, R.A.; et al. Coordinated Observations of Migrating Tides by Multiple Meteor Radars in the Equatorial Mesosphere and Lower Thermosphere. J. Geophys. Res. Space Phys. 2022, 127, e2022JA030678. [Google Scholar] [CrossRef]
- Scheiben, D.; Straub, C.; Hocke, K.; Forkman, P.; Kämpfer, N. Observations of middle atmospheric H2O and O3 during the 2010 major sudden stratospheric warming by a network of microwave radiometers. Atmos. Chem. Phys. 2012, 12, 7753–7765. [Google Scholar] [CrossRef]
- Scheiben, D.; Tschanz, B.; Hocke, K.; Kämpfer, N.; Ka, S.; Oh, J.J. The quasi 16-day wave in mesospheric water vapor during boreal winter 2011/2012. Atmos. Chem. Phys. 2014, 14, 6511–6522. [Google Scholar] [CrossRef]
- Woollings, T.; Li, C.; Drouard, M.; Dunn-Sigouin, E.; Elmestekawy, K.A.; Hell, M.; Hoskins, B.; Mbengue, C.; Patterson, M.; Spengler, T. The role of Rossby waves in polar weather and climate. Weather Clim. Dyn. 2023, 4, 61–80. [Google Scholar] [CrossRef]
- Riggin, D.M.; Lieberman, R.S. Variability of the diurnal tide in the equatorial MLT. J. Atmos. Sol. Terr. Phys. 2013, 102, 198–206. [Google Scholar] [CrossRef]
- Kogure, M.; Liu, H. DW1 Tidal Enhancements in the Equatorial MLT During 2015 El Niño: The Relative Role of Tidal Heating and Propagation. J. Geophys. Res. Space Phys. 2021, 126, e2021JA029342. [Google Scholar] [CrossRef]
- Livesey, N.J.; Read, W.G.; Wagner, P.A.; Froidevaux, L.; Santee, M.L.; Schwartz, M.J.; Lambert, A.; Valle, L.F.M.; Pumphrey, H.C.; Manney, G.L.; et al. Earth Observing System (EOS) Aura Microwave Limb Sounder (MLS) Version 5.0x Level 2 and 3 Data Quality and Description Document. Technical Report, JPL D-105336 Rev. B. 2022. Available online: https://mls.jpl.nasa.gov/data/v5-0_data_quality_document.pdf (accessed on 24 November 2023).
- Schwartz, M.J.; Lambert, A.; Manney, G.L.; Read, W.G.; Livesey, N.J.; Froidevaux, L.; Ao, C.O.; Bernath, P.F.; Boone, C.D.; Cofield, R.E.; et al. Validation of the Aura Microwave Limb Sounder temperature and geopotential height measurements. J. Geophys. Res. Atmos. 2008, 113, D15S11. [Google Scholar] [CrossRef]
- Hagan, M. Global Scale Wave Model. Available online: https://www2.hao.ucar.edu/modeling/gswm-global-scale-wave-model (accessed on 25 October 2023).
- Hagan, M.E.; Chang, J.L.; Avery, S.K. Global-scale wave model estimates of nonmigrating tidal effects. J. Geophys. Res. Atmos. 1997, 102, 16439–16452. [Google Scholar] [CrossRef]
- Tsuda, T.; Ohnishi, K.; Isoda, F.; Nakamura, T.; Vincent, R.A.; Reid, I.M.; Harijono, S.W.B.; Sribimawati, T.; Nuryanto, A.; Wiryosumarto, H. Coordinated radar observations of atmospheric diurnal tides in equatorial regions. Earth Planets Space 1999, 51, 579–592. [Google Scholar] [CrossRef]
- Keckhut, P.; Funatsu, B.M.; Claud, C.; Hauchecorne, A. Tidal effects on stratospheric temperature series derived from successive advanced microwave sounding units. Q. J. R. Meteorol. Soc. 2015, 141, 477–483. [Google Scholar] [CrossRef] [PubMed]
- Hagen, J.; Hocke, K.; Stober, G.; Pfreundschuh, S.; Murk, A.; Kämpfer, N. First measurements of tides in the stratosphere and lower mesosphere by ground-based Doppler microwave wind radiometry. Atmos. Chem. Phys. 2020, 20, 2367–2386. [Google Scholar] [CrossRef]
- Hocke, K.; Lainer, M.; Schanz, A. Composite analysis of a major sudden stratospheric warming. Ann. Geophys. 2015, 33, 783–788. [Google Scholar] [CrossRef]
- Palmeiro, F.M.; García-Serrano, J.; Ruggieri, P.; Batté, L.; Gualdi, S. On the Influence of ENSO on Sudden Stratospheric Warmings. J. Geophys. Res. Atmos. 2023, 128, e2022JD037607. [Google Scholar] [CrossRef]
- Hersbach, H.; Bell, B.; Berrisford, P.; Hirahara, S.; Horanyi, A.; Munoz-Sabater, J.; Nicolas, J.; Peubey, C.; Radu, R.; Schepers, D.; et al. The ERA5 global reanalysis. Q. J. R. Meteorol. Soc. 2020, 146, 1999–2049. [Google Scholar] [CrossRef]
- Butler, A.H.; Sjoberg, J.P.; Seidel, D.J.; Rosenlof, K.H. A sudden stratospheric warming compendium. Earth Syst. Sci. Data 2017, 9, 63–76. [Google Scholar] [CrossRef]
- Studer, S.; Hocke, K.; Kämpfer, N. Intraseasonal oscillations of stratospheric ozone above Switzerland. J. Atmos. Sol. Terr. Phys. 2012, 74, 189–198. [Google Scholar] [CrossRef]
- Forbes, J. Wave Coupling and Nonlinear Interactions in the Atmospheres of Earth and Mars. Q. Phys. Rev. 2017, 3. Available online: https://esmed.org/MRA/qpr/article/view/1439 (accessed on 24 November 2023).
- World Meteorological Organization. 2015 Ozone Hole One of the Largest on Record. Available online: https://public.wmo.int/en/resources/meteoworld/2015-ozone-hole-one-of-largest-record (accessed on 16 November 2023).
- Ramesh, K.; Smith, A.K.; Garcia, R.R.; Marsh, D.R.; Sridharan, S.; Kishore Kumar, K. Long-Term Variability and Tendencies in Migrating Diurnal Tide From WACCM6 Simulations During 1850–2014. J. Geophys. Res. Atmos. 2020, 125, e2020JD033644. [Google Scholar] [CrossRef]
- Fuller-Rowell, T.J.; Fang, T.W.; Wang, H.; Matthias, V.; Hoffmann, P.; Hocke, K.; Studer, S. Impact of migrating tides on electrodynamics during the January 2009 sudden stratospheric warming. In Ionospheric Space Weather; American Geophysical Union (AGU): Washington, DC, USA, 2016; Chapter 14; pp. 163–174. [Google Scholar] [CrossRef]








Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hocke, K. Influence of Sudden Stratospheric Warmings on the Migrating Diurnal Tide in the Equatorial Middle Atmosphere Observed by Aura/Microwave Limb Sounder. Atmosphere 2023, 14, 1743. https://doi.org/10.3390/atmos14121743
Hocke K. Influence of Sudden Stratospheric Warmings on the Migrating Diurnal Tide in the Equatorial Middle Atmosphere Observed by Aura/Microwave Limb Sounder. Atmosphere. 2023; 14(12):1743. https://doi.org/10.3390/atmos14121743
Chicago/Turabian StyleHocke, Klemens. 2023. "Influence of Sudden Stratospheric Warmings on the Migrating Diurnal Tide in the Equatorial Middle Atmosphere Observed by Aura/Microwave Limb Sounder" Atmosphere 14, no. 12: 1743. https://doi.org/10.3390/atmos14121743
APA StyleHocke, K. (2023). Influence of Sudden Stratospheric Warmings on the Migrating Diurnal Tide in the Equatorial Middle Atmosphere Observed by Aura/Microwave Limb Sounder. Atmosphere, 14(12), 1743. https://doi.org/10.3390/atmos14121743






