Anti-Swaying Control Strategy of Ship-Mounted 3-RCU Parallel Platform Based on Dynamic Gravity Compensation
Abstract
:1. Introduction
2. Marine Anti-Swaying Strategy
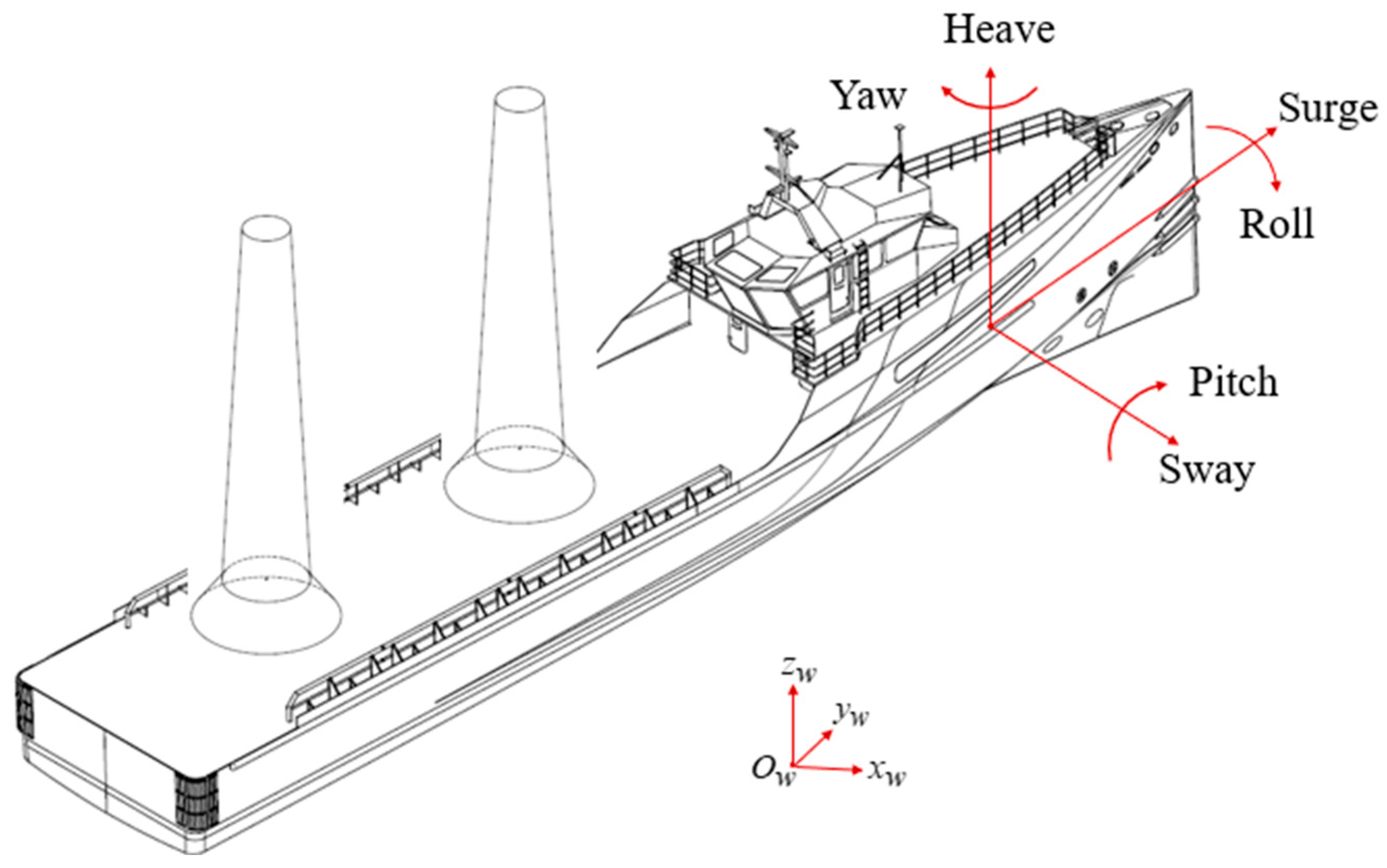
2.1. Application Scenarios
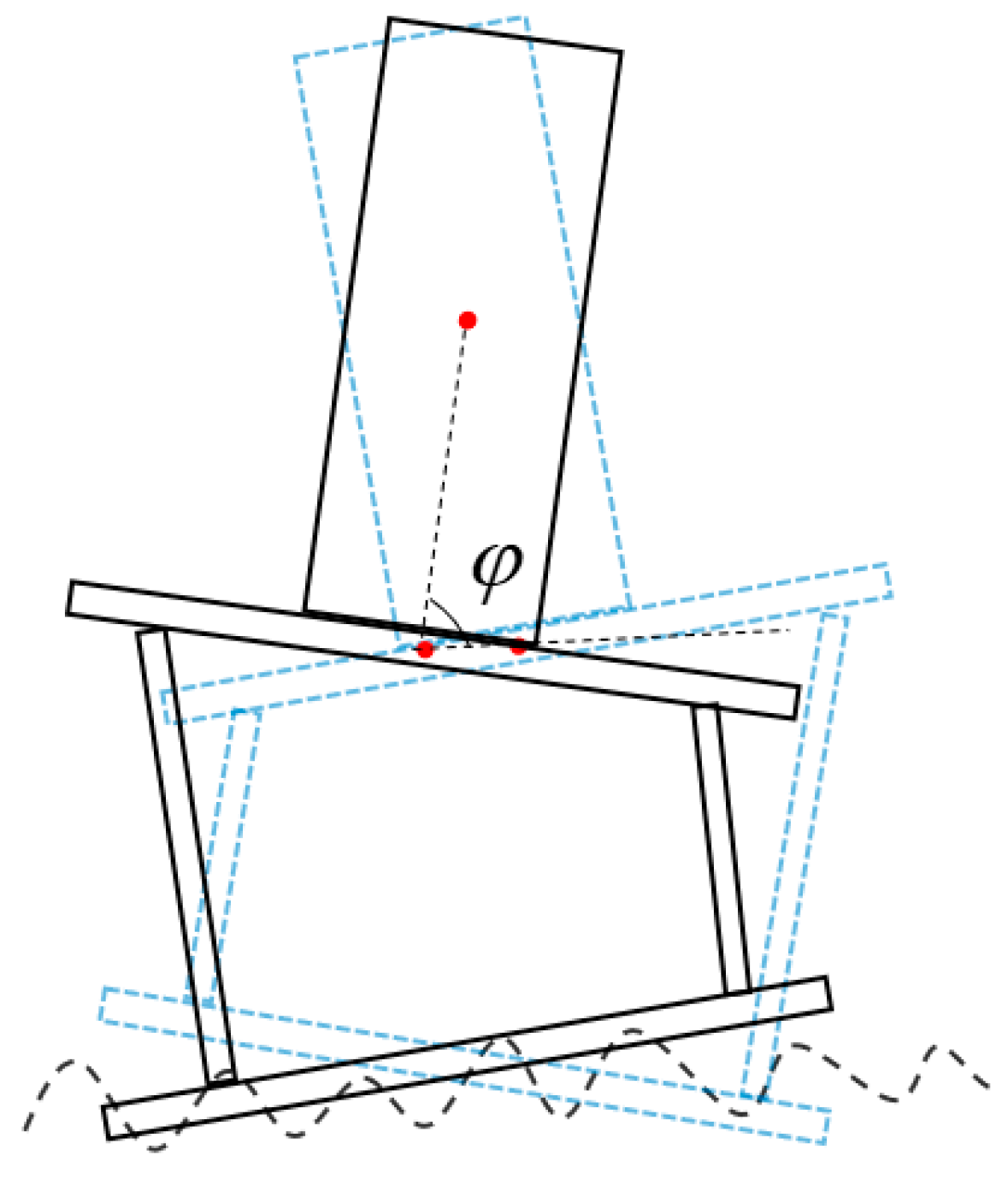
2.2. Anti-Swaying Strategy
3. Marine Anti-Swaying Control Methods
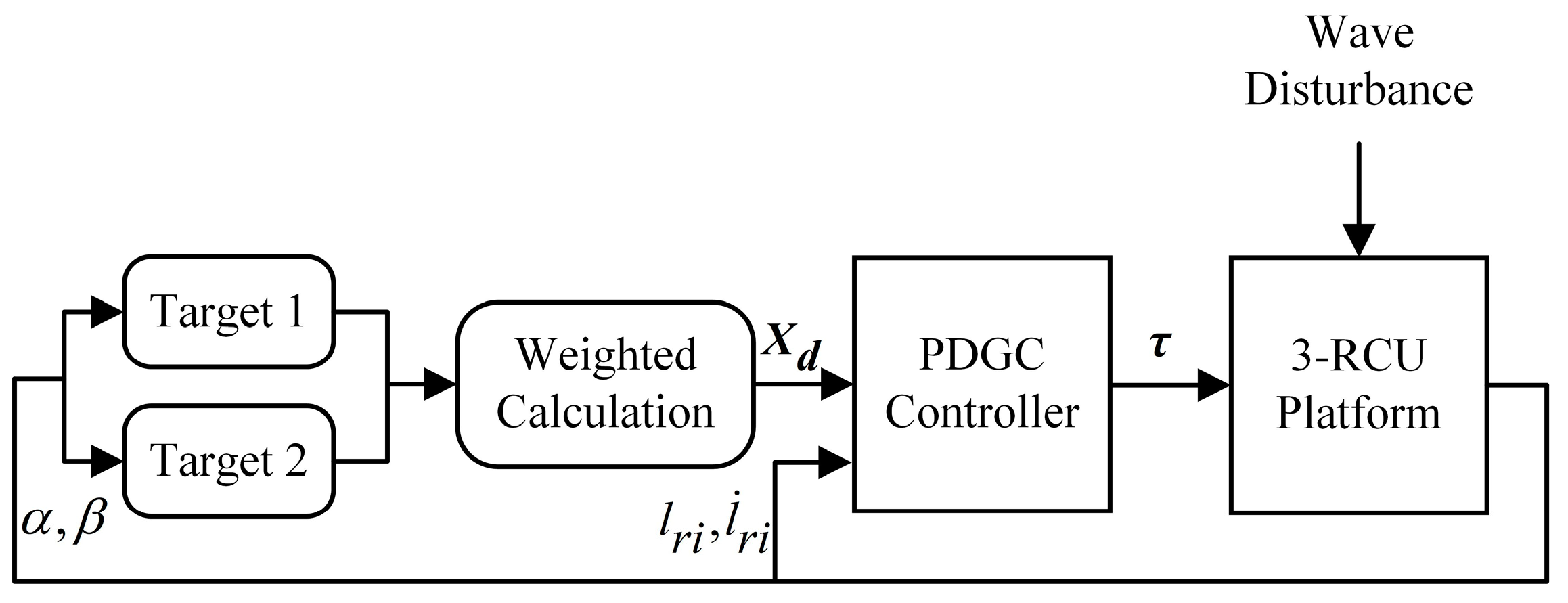
3.1. System Control Strategy
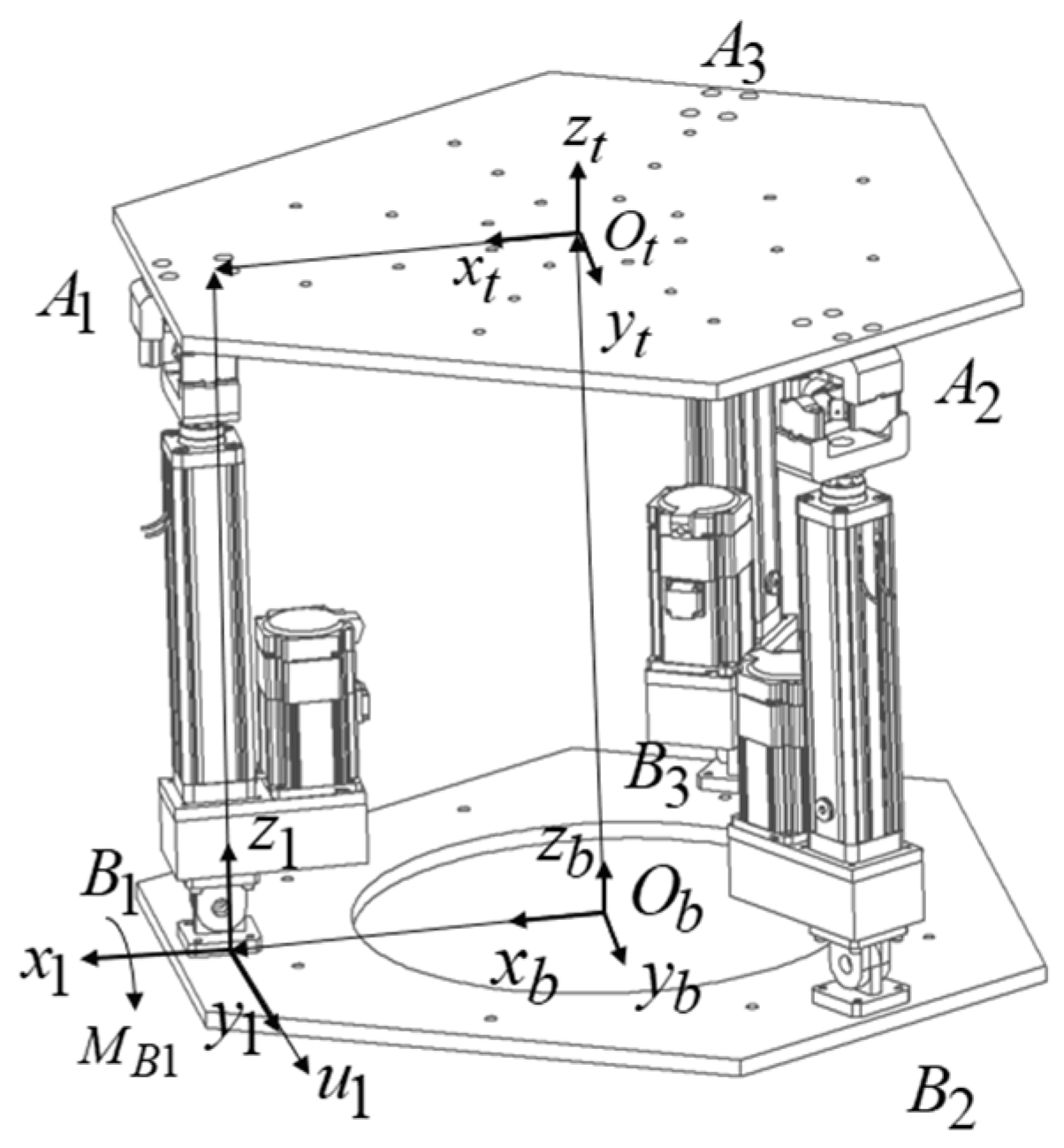
3.2. 3-RCU Platform Analysis
3.3. PDGC Controller
4. Simulation Validation
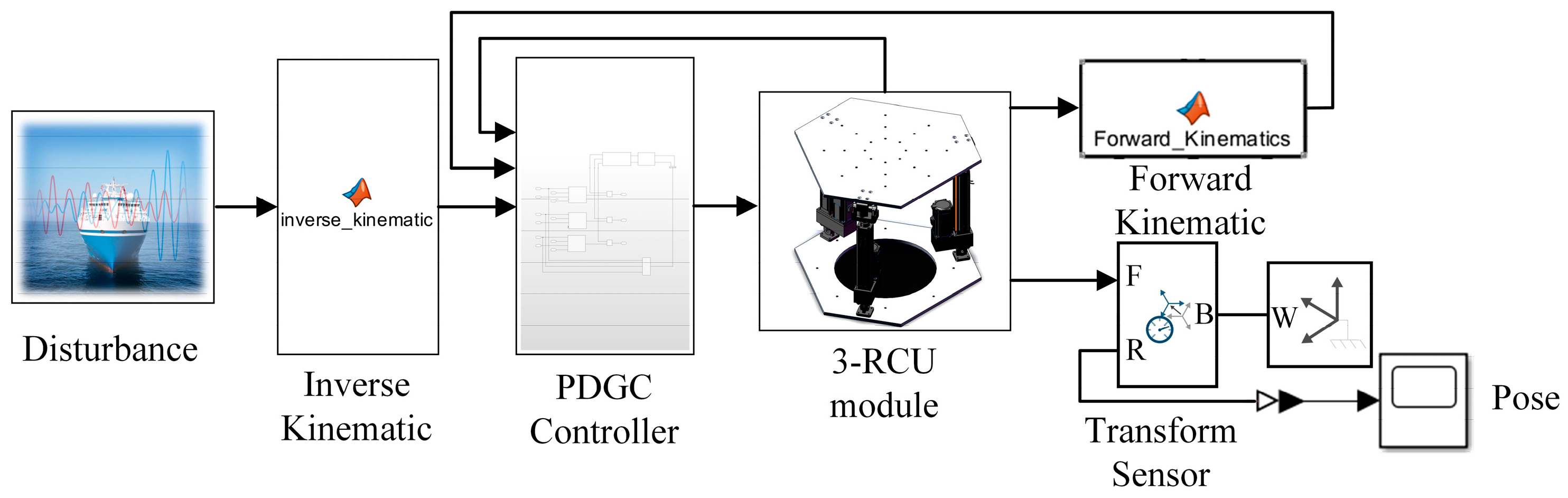
4.1. Description of the Simulation System
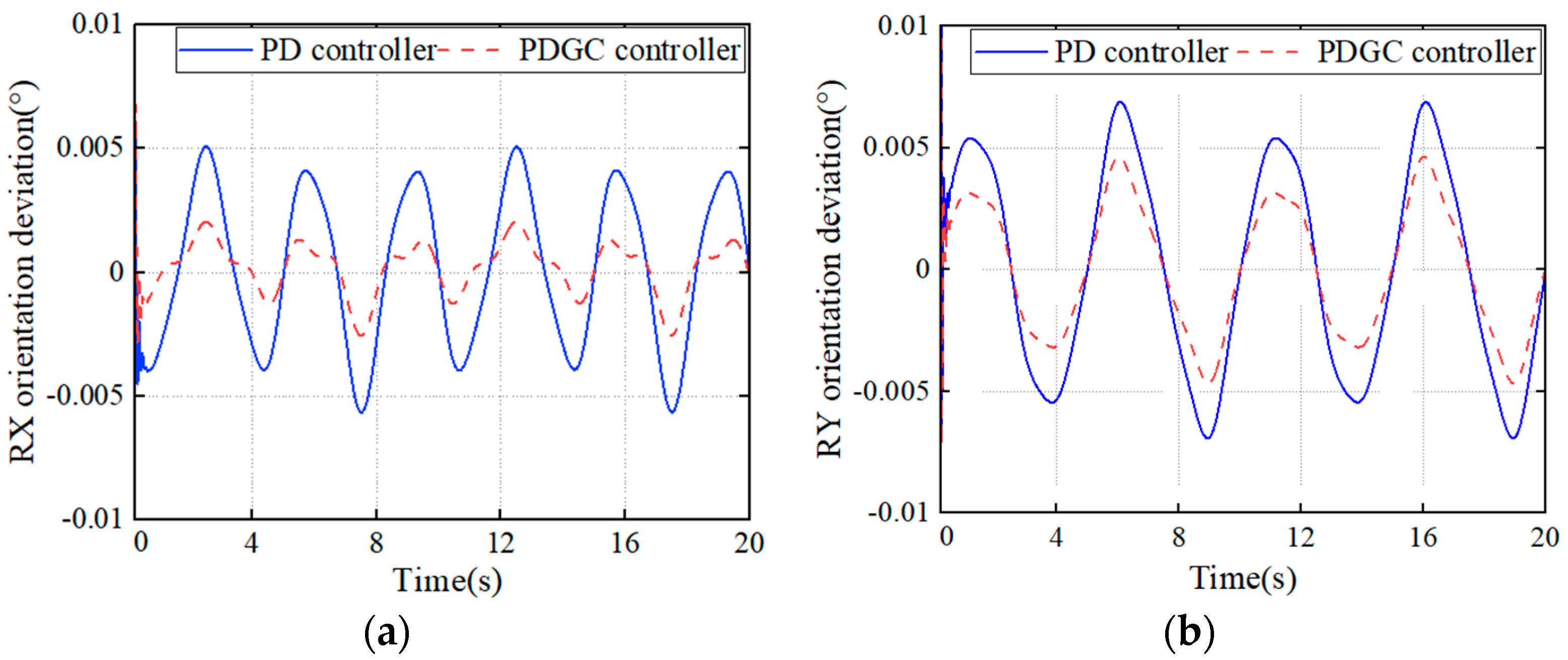
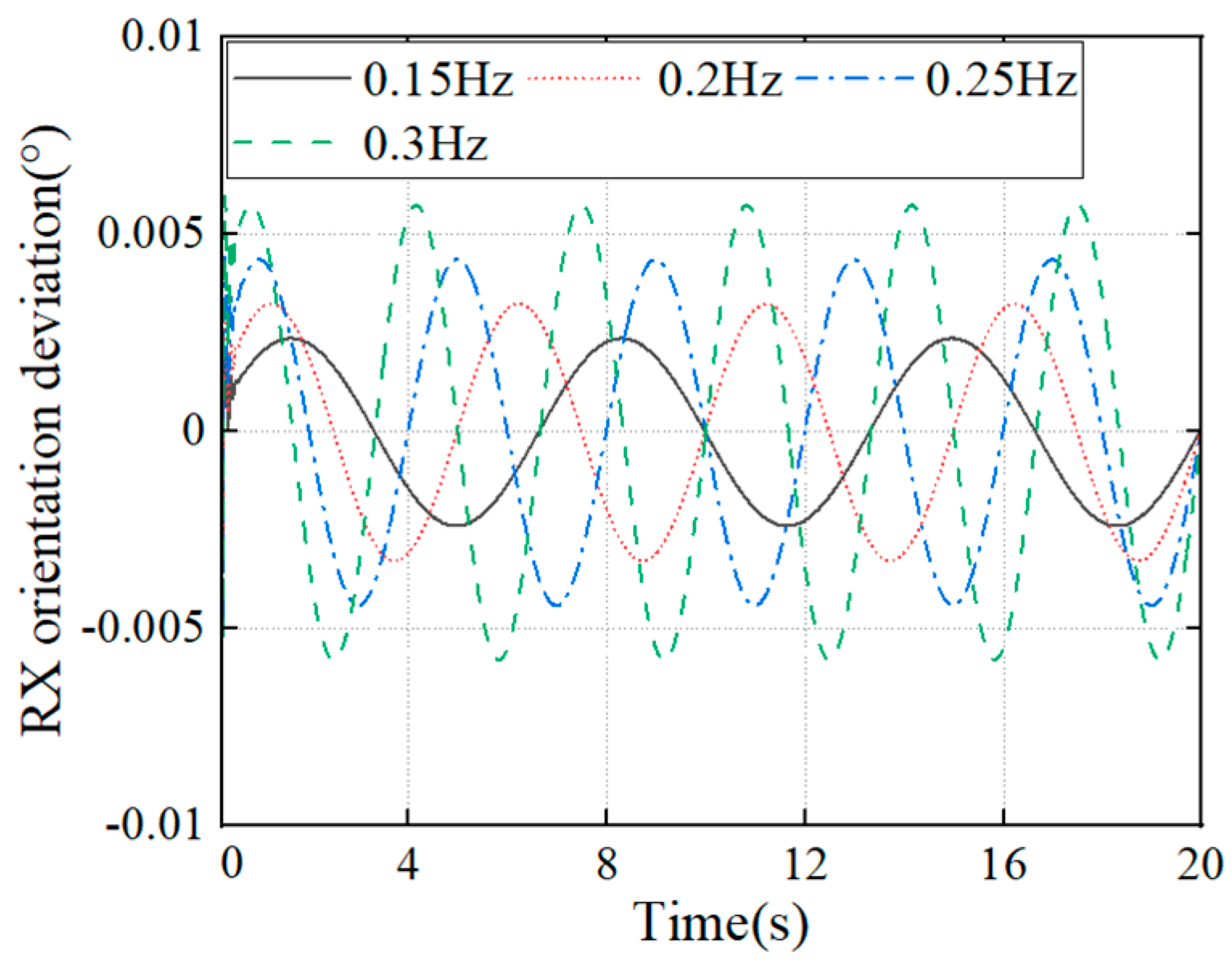
4.2. Performance under Sinusoidal Excitation
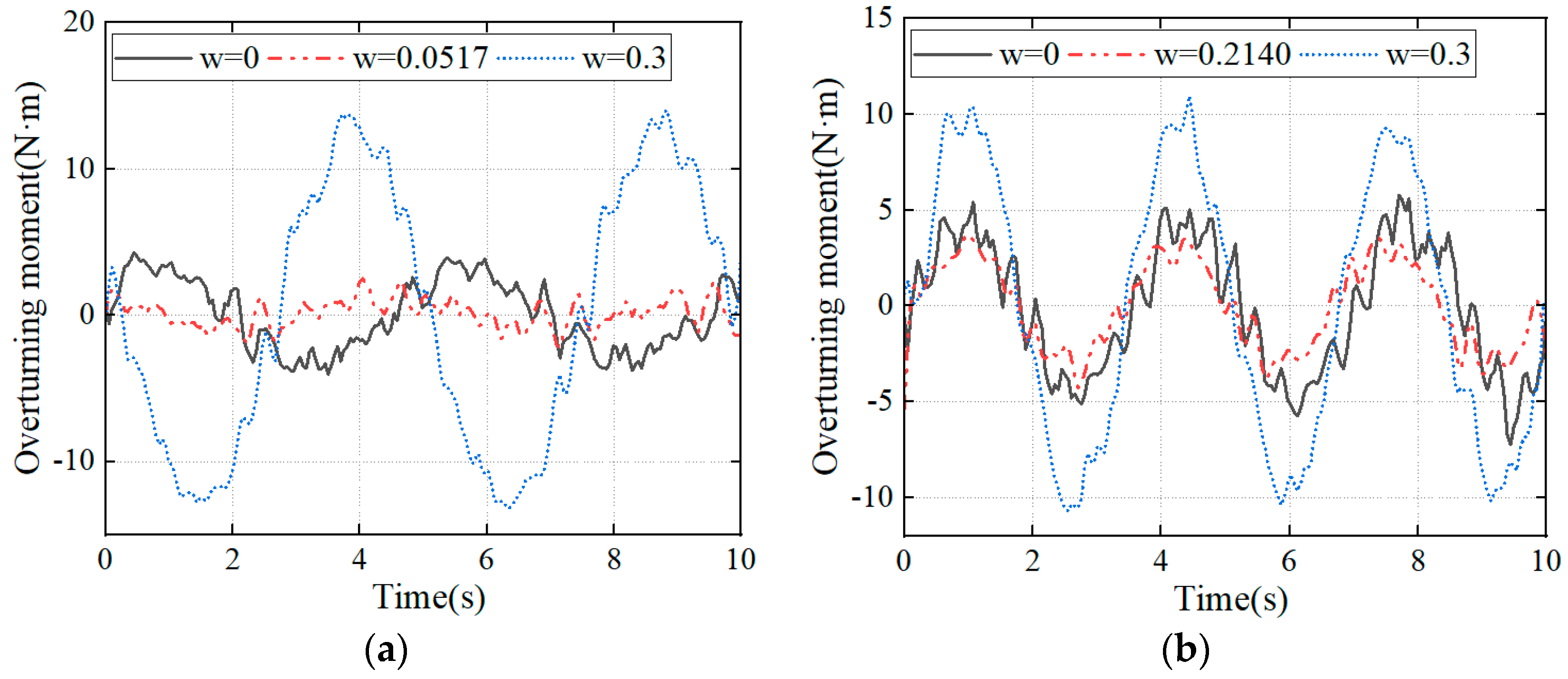
4.3. Optimal Weighting Coefficient
5. Experimental Validation
5.1. Description of the Testing System
5.2. Performance of PDGC Controller under Marine Excitation
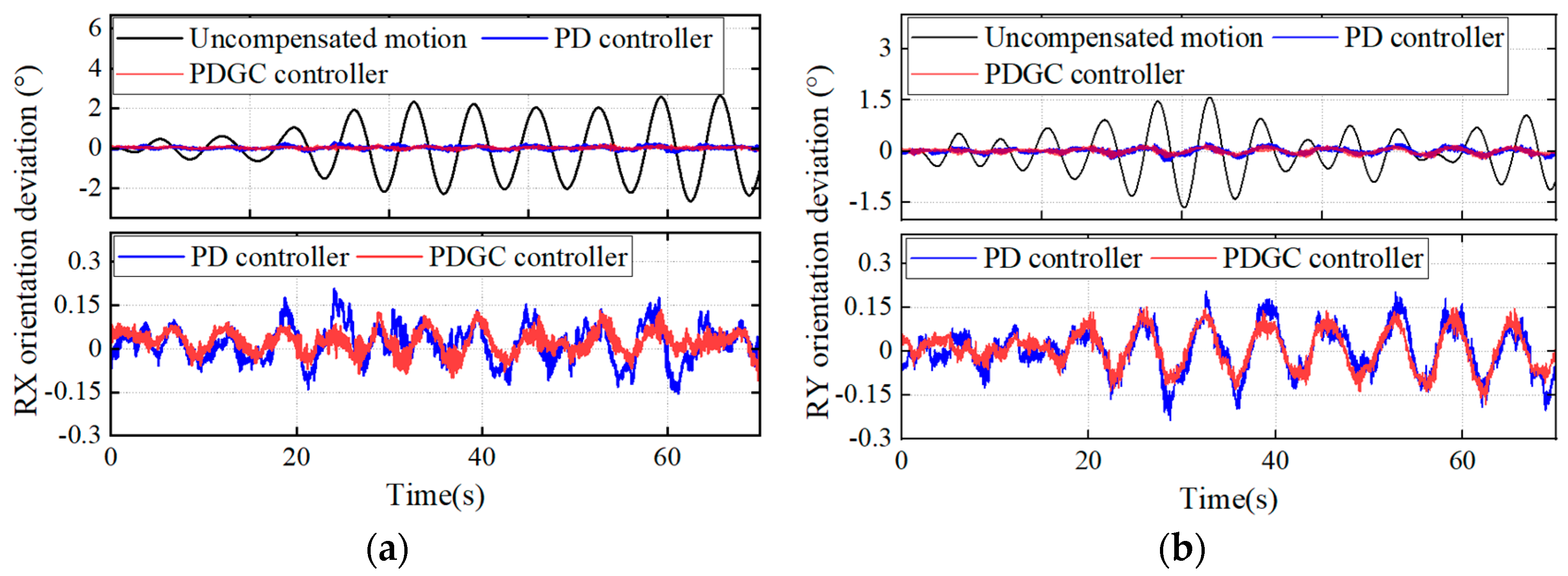
5.3. Validation of Anti-Swaying Strategy
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sanden, S.; Hovland, G. Inverse kinematic control of an industrial robot used in Vessel-to-Vessel Motion Compensation. In Proceedings of the 2017 25th Mediterranean Conference on Control and Automation (MED), Valletta, Malta, 3–6 July 2017; IEEE: Piscataway, NJ, USA; pp. 1392–1397. [Google Scholar]
- Fossen, T.I. Handbook of Marine Craft Hydrodynamics and Motion Control; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Halvorsen, H.S.; Øveraas, H.; Landstad, O.; Smines, V.; Fossen, T.I.; Johansen, T.A. Wave motion compensation in dynamic positioning of small autonomous vessels. J. Mar. Sci. Technol. 2021, 26, 693–712. [Google Scholar] [CrossRef]
- Elbadawy, A.A.; Shehata, M.M. Anti-sway control of marine cranes under the disturbance of a parallel manipulator. Nonlinear Dyn. 2015, 82, 415–434. [Google Scholar] [CrossRef]
- Schlick, E.O.; Wurl, M. Apparatus for Minimizing the Oscillatory Movements of Ships and Other Oscillating Bodies. U.S. Patent No. 944,511, 28 December 1909. [Google Scholar]
- Said, L.; Sheng, L.; Farouk, N.; Latifa, B. Modeling, design and control of a ship carried 3 DOF stabilized platform. Res. J. Appl. Sci. Eng. Technol. 2012, 4, 3843–3851. [Google Scholar]
- Tao, S.; Fei, C.; Xiuzhi, L.; He, L.; Yi, W. Research of the feedforward control system of 3-axis stable platform based on disturbance observer. Syst. Sci. Control Eng. 2018, 6, 324–332. [Google Scholar] [CrossRef]
- Zhang, M.; Guan, Y.; Zhao, W. Adaptive super-twisting sliding mode control for stabilization platform of laser seeker based on extended state observer. Optik 2019, 199, 163337. [Google Scholar] [CrossRef]
- Stewart, D. A platform with six degrees of freedom. Proc. Inst. Mech. Eng. 1965, 180, 371–386. [Google Scholar] [CrossRef]
- Liu, S.; Peng, G.; Gao, H. Dynamic modeling and terminal sliding mode control of a 3-DOF redundantly actuated parallel platform. Mechatronics 2019, 60, 26–33. [Google Scholar] [CrossRef]
- Xu, P.; Cheung, C.F.; Li, B.; Ho, L.T.; Zhang, J.F. Kinematics analysis of a hybrid manipulator for computer controlled ultra-precision freeform polishing. Robot. Comput. Integr. Manuf. 2017, 44, 44–56. [Google Scholar] [CrossRef]
- Jaouen, F.; van den Berg, J.; van der Schaaf, H.; May, E.; Koppenol, J. How does barge-master compensate for the barge motions: Experimental and numerical study. In Proceedings of the ASME 2012 31st International Conference on Offshore Mechanics and Arctic Engineering, Rio de Janeiro, Brazil, 1–6 July 2012; American Society of Mechanical Engineers: New York, NY, USA; Volume 44885, pp. 35–46. [Google Scholar]
- De Zeeuw, W.A. Ship Motion Compensation Platform for High Payloads: Dynamic Analysis and Control. Master’s Thesis, Delft University of Technology, Delft, The Netherlands, August 2012. [Google Scholar]
- Hu, X.; Li, F.; Tang, G. Kinematics analysis of 3UPU_UP coupling parallel platform in the marine environment. IEEE Access 2020, 8, 158142–158151. [Google Scholar] [CrossRef]
- Tang, G.; Lu, P.; Hu, X.; Men, S. Control system research in wave compensation based on particle swarm optimization. Sci. Rep. 2021, 11, 15316. [Google Scholar] [CrossRef] [PubMed]
- Lan-yong, Z.; An, C.; Yi-xuan, D.; Bing, L. Multivariable fuzzy genetic controller for stabilized platform. In Proceedings of the 2015 34th Chinese Control Conference (CCC), Hangzhou, China, 28–30 July 2015; IEEE: Piscataway, NJ, USA; pp. 783–788. [Google Scholar]
- Lv, W.; Tao, L.; Ji, Z. Design and control of cable-drive parallel robot with 6-dof active wave compensation. In Proceedings of the 2017 3rd International Conference on Control, Automation and Robotics (ICCAR), Nagoya, Japan, 22–24 April 2017; IEEE: Piscataway, NJ, USA; pp. 129–133. [Google Scholar]
- Campos, A.; Quintero, J.; Saltaren, R.; Ferre, M.; Aracil, R. An active helideck testbed for floating structures based on a stewart-gough platform. In Proceedings of the 2008 IEEE/RSJ International Conference on Intelligent Robots and Systems, Nice, France, 22–26 September 2008; IEEE: Piscataway, NJ, USA; pp. 3705–3710. [Google Scholar]
- Cai, Y.; Zheng, S.; Liu, W.; Qu, Z.; Zhu, J.; Han, J. Sliding-mode control of ship-mounted Stewart platforms for wave compensation using velocity feedforward. Ocean Eng. 2021, 236, 109477. [Google Scholar] [CrossRef]
- He, Y.; Wu, Y.; Li, W. Sliding mode control for offshore parallel antenna platform with large orientation workspace. ISA Trans. 2022, 128, 90–108. [Google Scholar] [CrossRef]
- Ono, T.; Eto, R.; Yamakawa, J.; Murakami, H. Control Simulations for a Stewart Platform Compensator Mounted on Moving Base. In Proceedings of the ASME International Mechanical Engineering Congress and Exposition, Salt Lake City, UT, USA, 8–14 November 2019; American Society of Mechanical Engineers: New York, NY, USA; Volume 59414, p. V004T05A017. [Google Scholar]
- Liu, J.; Chen, X. Adaptive control based on neural network and beetle antennae search algorithm for an active heave compensation system. Int. J. Control Autom. Syst. 2022, 20, 515–525. [Google Scholar] [CrossRef]
- Chen, W.; Wang, S.; Li, J.; Lin, C.; Yang, Y.; Ren, A.; Wei, L.; Zhao, X.; Zhang, W.; Guo, W.; et al. An ADRC-based triple-loop control strategy of ship-mounted Stewart platform for six-DOF wave compensation. Mech. Mach. Theory 2023, 184, 105289. [Google Scholar] [CrossRef]
- Yu, D.; Chen, W.X. Analysis of erection and transportation of offshore wind power single-pile foundation stabilized pile platform. China Harb. Constr. 2022, 42, 10–14. (In Chinese) [Google Scholar]
- Bingul, Z.; Karahan, O. Dynamic Modeling and Simulation of Stewart Platform; INTECH Open Access Publisher: London, UK, 2012. [Google Scholar]
- Tang, G.; Lei, J.; Li, F.; Zhu, W.; Xu, X.; Yao, B.; Claramunt, C.; Hu, X. A modified 6-DOF hybrid serial–parallel platform for ship wave compensation. Ocean. Eng. 2023, 280, 114336. [Google Scholar] [CrossRef]
- Kizir, S.; Bingul, Z. Position control and trajectory tracking of the Stewart platform. In Serial and Parallel Robot Manipulators-Kinematics, Dynamics, Control and Optimization; InTech: Istanbul, Turkey, 2012; Volume 3, pp. 179–202. [Google Scholar]
- Ren, Z.; Skjetne, R.; Verma, A.S.; Jiang, Z.; Gao, Z.; Halse, K.H. Active heave compensation of floating wind turbine installation using a catamaran construction vessel. Mar. Struct. 2021, 75, 102868. [Google Scholar] [CrossRef]
- Tsai, L.W. The mechanics of serial and parallel manipulators. In Robot Analysis; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 1999. [Google Scholar]
- Wang, Y.; Yu, J.; Pei, X. Fast forward kinematics algorithm for real-time and high-precision control of the 3-RPS parallel mechanism. Front. Mech. Eng. 2018, 13, 368–375. [Google Scholar] [CrossRef]
- Su, Y.X.; Duan, B.Y.; Zheng, C.H. Nonlinear PID control of a six-DOF parallel manipulator. IEE Proc. Control Theory Appl. 2004, 151, 95–102. [Google Scholar] [CrossRef]
- Nguyen, C.C.; Antrazi, S.S.; Zhou, Z.L.; Campbell, C.E., Jr. Adaptive control of a Stewart platform-based manipulator. J. Robot. Syst. 1993, 10, 657–687. [Google Scholar] [CrossRef]





| Parameters | Value |
|---|---|
| Circumradius of base r | 0.345 m |
| Height of load center of gravity h | 1 m |
| Total mass of moving platform and load M | 100 kg |
| Initial height of 3-RCU H | 0.78 m |
| [3 × 105 3 × 105 3 × 105] | |
| [2 × 103 2 × 103 2 × 103] |
| Parameters | Value |
|---|---|
| Population size | 100 |
| Maximum number of function evaluations | 10,000 |
| Probability of crossover | 1 |
| Distribution index of polynomial mutation | 20 |
| (m) | 1 | 1.25 | 1.5 | 1.75 | 2 | |
|---|---|---|---|---|---|---|
| (Hz) | ||||||
| 0.3 | 0.2821 | 0.3232 | 0.3650 | 0.3986 | 0.4348 | |
| 0.275 | 0.2476 | 0.2845 | 0.3222 | 0.3583 | 0.3889 | |
| 0.25 | 0.2114 | 0.2450 | 0.2801 | 0.3150 | 0.3453 | |
| 0.225 | 0.1752 | 0.2091 | 0.2391 | 0.2781 | 0.2978 | |
| 0.2 | 0.1412 | 0.1698 | 0.1985 | 0.2280 | 0.2530 | |
| Evaluation Parameters | Results |
|---|---|
| R-squared | 0.99524 |
| Sum of Squared Errors (SSE) | 0.0006611 |
| Adjusted R-squared | 0.99429 |
| Root Mean Square Error (RMSE) | 0.005749 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lv, Z.; Liu, P.; Ning, D.; Wang, S. Anti-Swaying Control Strategy of Ship-Mounted 3-RCU Parallel Platform Based on Dynamic Gravity Compensation. Machines 2024, 12, 209. https://doi.org/10.3390/machines12030209
Lv Z, Liu P, Ning D, Wang S. Anti-Swaying Control Strategy of Ship-Mounted 3-RCU Parallel Platform Based on Dynamic Gravity Compensation. Machines. 2024; 12(3):209. https://doi.org/10.3390/machines12030209
Chicago/Turabian StyleLv, Zhiyuan, Pengfei Liu, Donghong Ning, and Shuqing Wang. 2024. "Anti-Swaying Control Strategy of Ship-Mounted 3-RCU Parallel Platform Based on Dynamic Gravity Compensation" Machines 12, no. 3: 209. https://doi.org/10.3390/machines12030209





