Complex Use of the Main Marine Diesel Engine High- and Low-Temperature Waste Heat in the Organic Rankine Cycle
Abstract
:1. Introduction
- A study was conducted on the energy efficiency and performance indicators of the WHR cycle, considering variable operational conditions typical for maritime transport while the ship’s power plant operated over a wide range of load conditions. This included numerical studies and comparative analysis evaluations of the impact of three characteristic ORC categories of working fluids. Additionally, it involved studying the principles of heat regeneration and power turbine regulation in the WHR cycle, with a rational adaptation to ship propulsion plant, as well as experimental numerical variation studies. These findings are presented in the authors’ publication [53].
- In the second stage of research, the main goal was related to the formation of an information base to substantiate the rational choice of ORC structure based on energy consumption efficiency indicators within the operational load range, considering the limitations of ship technological systems.
- The results of this research stage are presented in the author’s publication. The authors primarily attribute the scientific novelty and practical significance of the research to the ORC’s applicability in utilizing secondary heat sources of the ship’s power plant under various complex combinations in operating conditions, including 25–100% of the load range of the ship power plant. Moreover, the research determined the relationship between cycle energy performance with cycle structure and outboard water flow rate, which is considered one of the limitations of ORC applicability in ship technological systems.
2. Methodological Aspects of the Research
2.1. Formation, Justification, and Identification of the ORC Research Cycle Structure (Complex form of WHR Cycle with Different Heat Sources)
2.2. Selection of ORC Working Fluid and Formation of Physical and Energetic Indicators
2.3. Selection of the Research Object for ORC
2.4. Mathematical Model of Numerical Studies of Engine Parameters
2.5. Calculation of the Energy Balance during the Operation of the Diesel Engine in the Operational Characteristic Modes
2.6. ORC Energy Efficiency and Its Structure Unit Parameters
- Evaluating how much the effective useful coefficient of the main engine increased with the WHR system cycle, using secondary heat in it to generate electrical energy.
- Evaluating the efficiency of energy use in the WHR cycle itself .
- Exhaust gas secondary heat source;
- Internal cylinder cooling circuit secondary heat source;
- Scavenge air cooling circuit secondary heat source.
3. Results
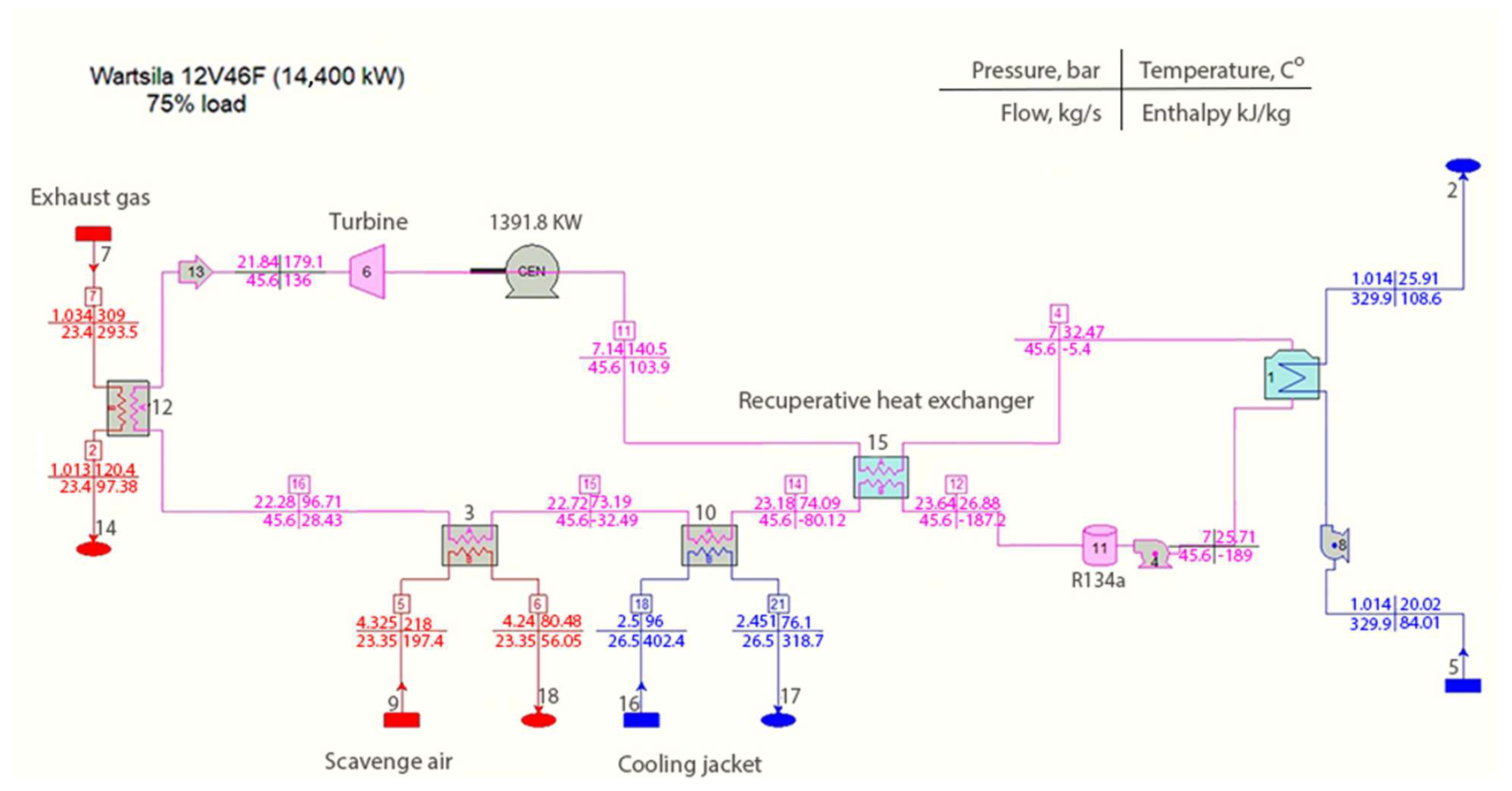
Complex Form WHR Cycle with Different Heat Sources
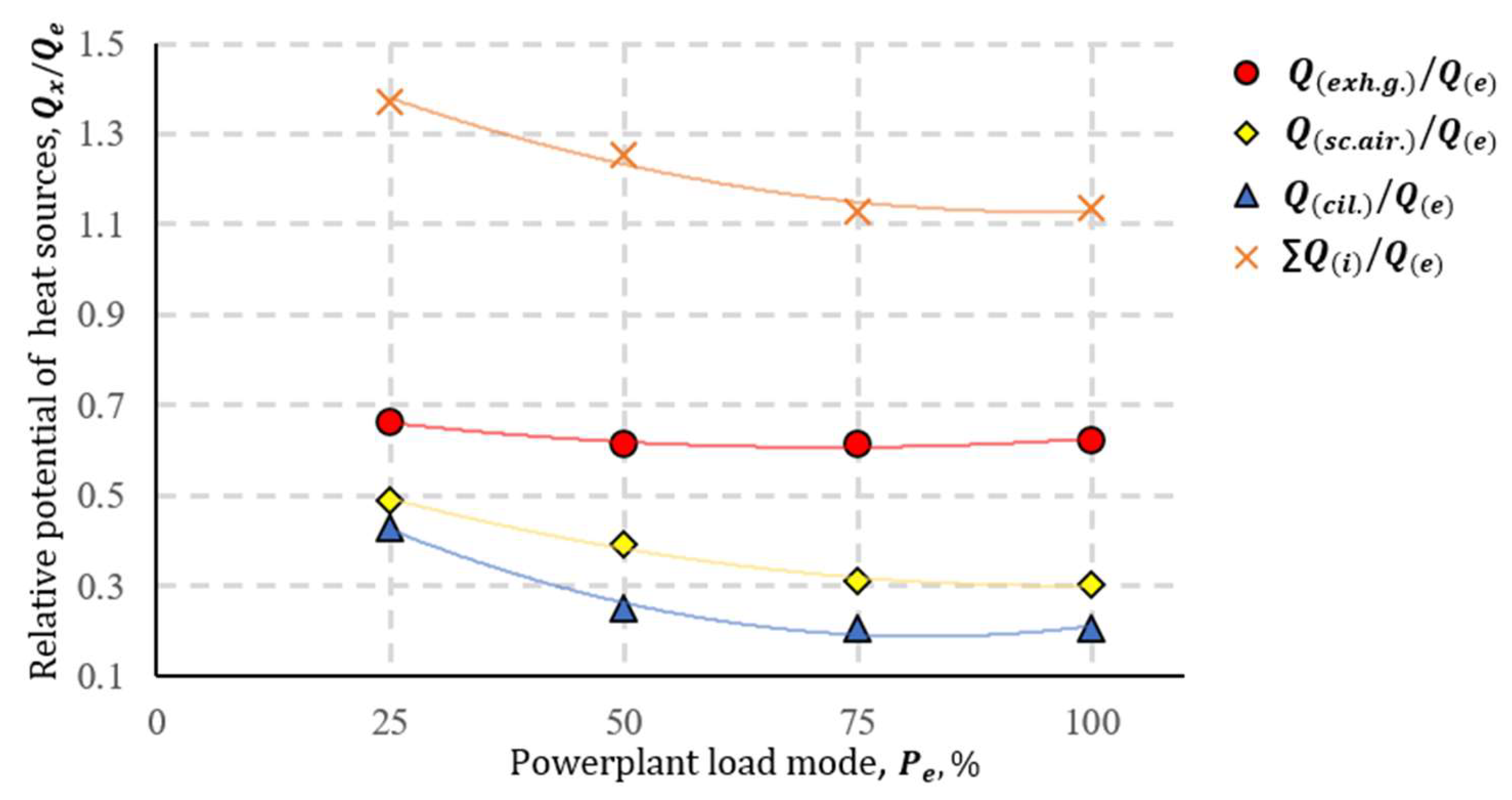
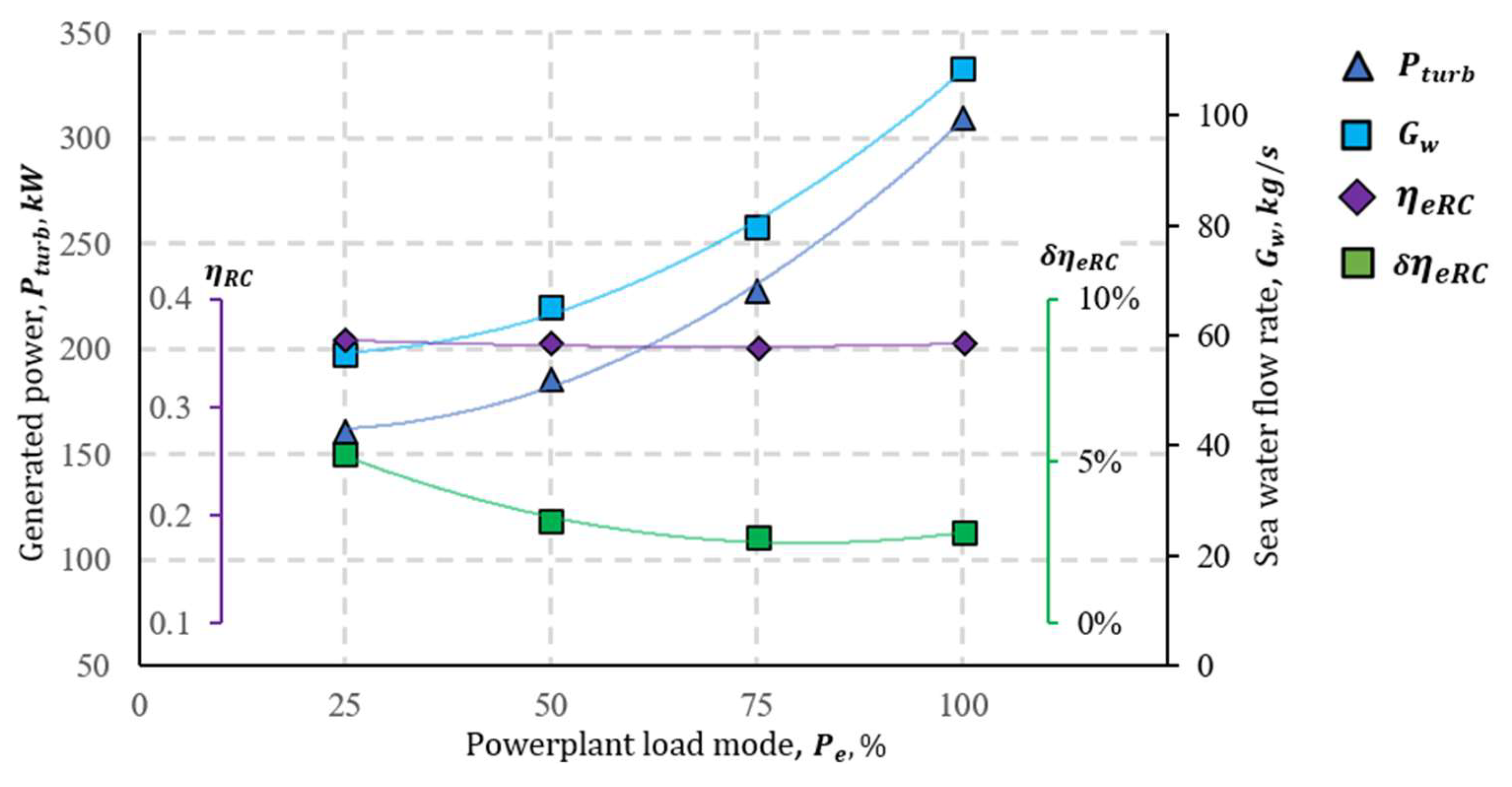
- The power generated by the secondary internal cylinder cooling circuit heat source in the WHR cycle ranges from 160 kW to 310 kW. The seawater flow rate for condensation ranges from 55 kg/s to 108 kg/s, corresponding to load conditions of 25–100% of the engine, at a seawater temperature of 20 °C. Graphically, it can be observed that the most significant change in the WHR system’s useful efficiency coefficient is achieved under low load conditions (Figure 4).
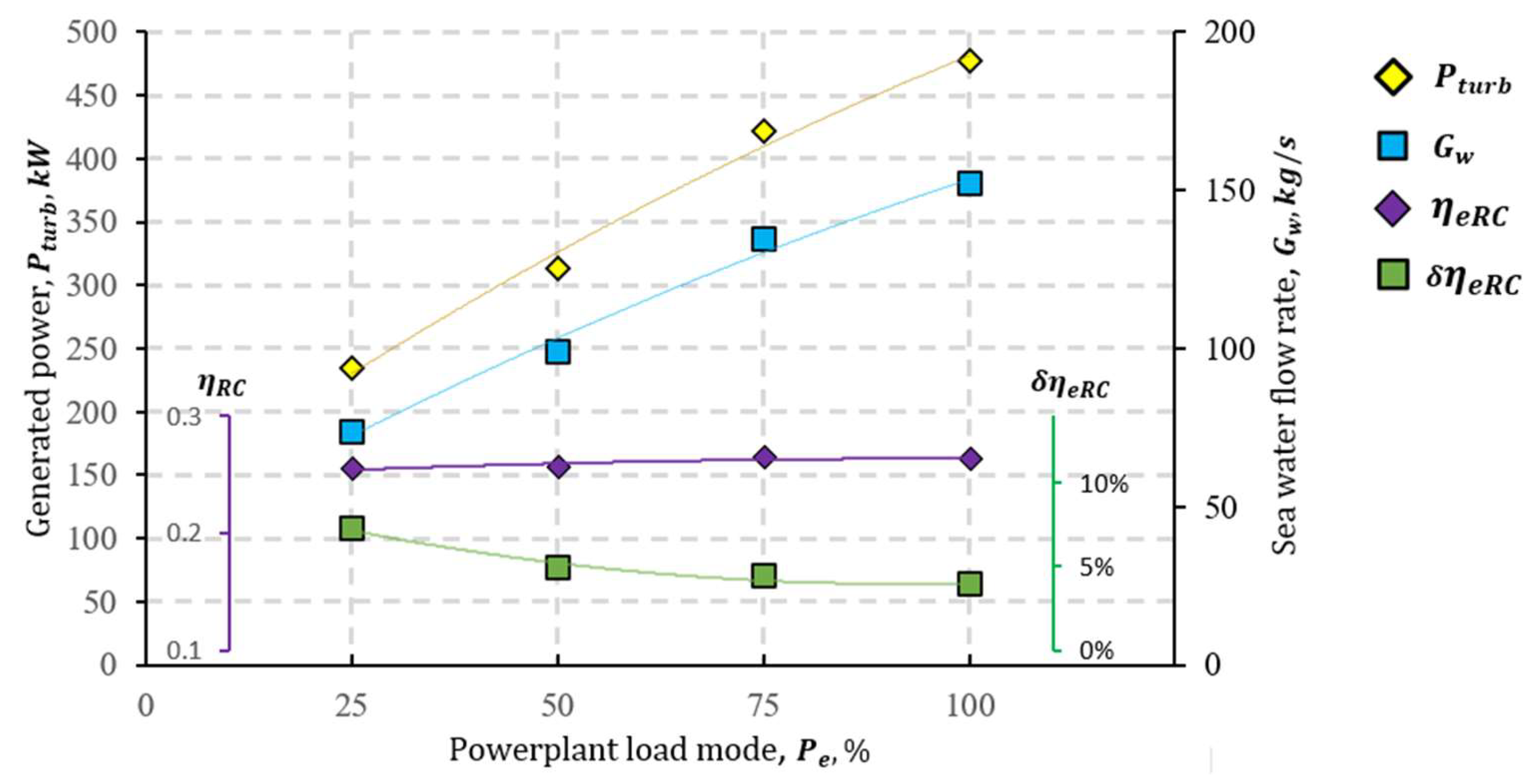
- The power generated by the secondary scavenge air cooling circuit heat source () in the WHR cycle is higher than that of the cylinder cooling circuit, ranging from 234 kW to 477 kW. The seawater flow rate for condensation ranges from 74 kg/s to 152 kg/s, corresponding to load conditions of 25–100% of the engine, at a seawater temperature of 20 °C. Graphically, it can be observed that the most significant change in the WHR system’s useful efficiency coefficient is also achieved under low load conditions (Figure 5).
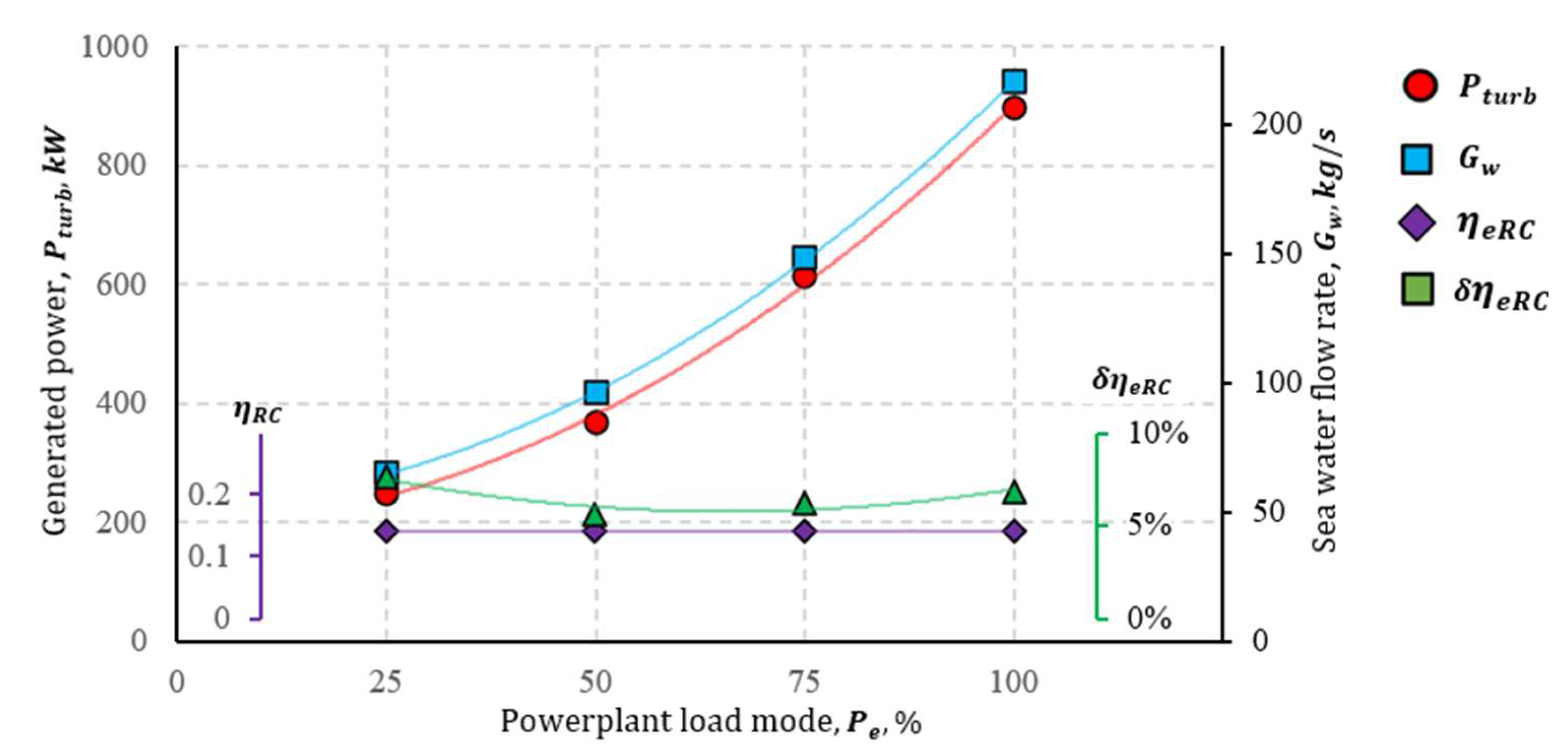
- The power generated by the secondary exhaust gas circuit heat source in the WHR cycle, under low load conditions, is slightly lower than the compressed air source, producing 249 kW. However, when there is a high load, the generated power is almost twice as much as the compressed air source, reaching 898 kW. The seawater flow rate for condensation in the cycle varies from 74 kg/s to 152 kg/s, corresponding to load conditions of 25–100% of the engine, at a seawater temperature of 20 °C. Graphically, it can be observed that the greatest positive change in the useful efficiency coefficient of the WHR system is achieved under low load conditions (Figure 6).
4. Conclusions
- The rational distribution of ORC heat exchangers based on the increasing characteristic temperature of secondary heat sources (operating in the range of 25–100% engine load, engine cooling jacket, scavenge air, and exhaust gas WHR secondary heat cooling circuits) ensures close proximity to the energy potential of the heat sources: internal cylinder cooling circuit—95%; scavenge air cooling circuit—84%; and exhaust gases—99% (with );
- The results indicate that it is rational to use ORC throughout the typical operational range of the engine, as reducing the nominal power from 100% to 25% leads to an improvement in the effective efficiency increase using ORC as follows: WHR for exhaust gases from 6.9% to 7.7%; charge air cooling circuit from 4% to 7.3%; and cylinder block cooling circuit from 2.8% to 5.2%.
- Specifically, the high efficiency of increasing at low engine loads determines the most crucial operational average values for the entire load cycle, respectively, 6.6%; 4.8%; and 3.1%.
- The comprehensive composition of a WHR system with various combinations ensures increase over the operational load cycle ranging from 14.8% (all three secondary heat source WHR cycle) to 3% (only low-temperature WHR cycle).
- Attention is drawn to the relatively high energy efficiency of the implementation of the scavenge air cooling WHR system—the difference in compared to exhaust gas WHR is only about 1.5%: approximately ~5% versus 6.5%, respectively. In combination with a relatively straightforward technical implementation, this allows considering this WHR as one of the effective components of the ship engine’s ORC, both in its standalone and combined applications with other WHR systems.
- ORC variation study data indicate that the application of secondary heat sources in the marine power plant in the operational characteristic range alternatively ensures the total power plant efficiency and improves energy performance. Variations in the complex use of exhaust gases, internal cylinder cooling circuit, and scavenge air cooler heat guarantee , indicator values.
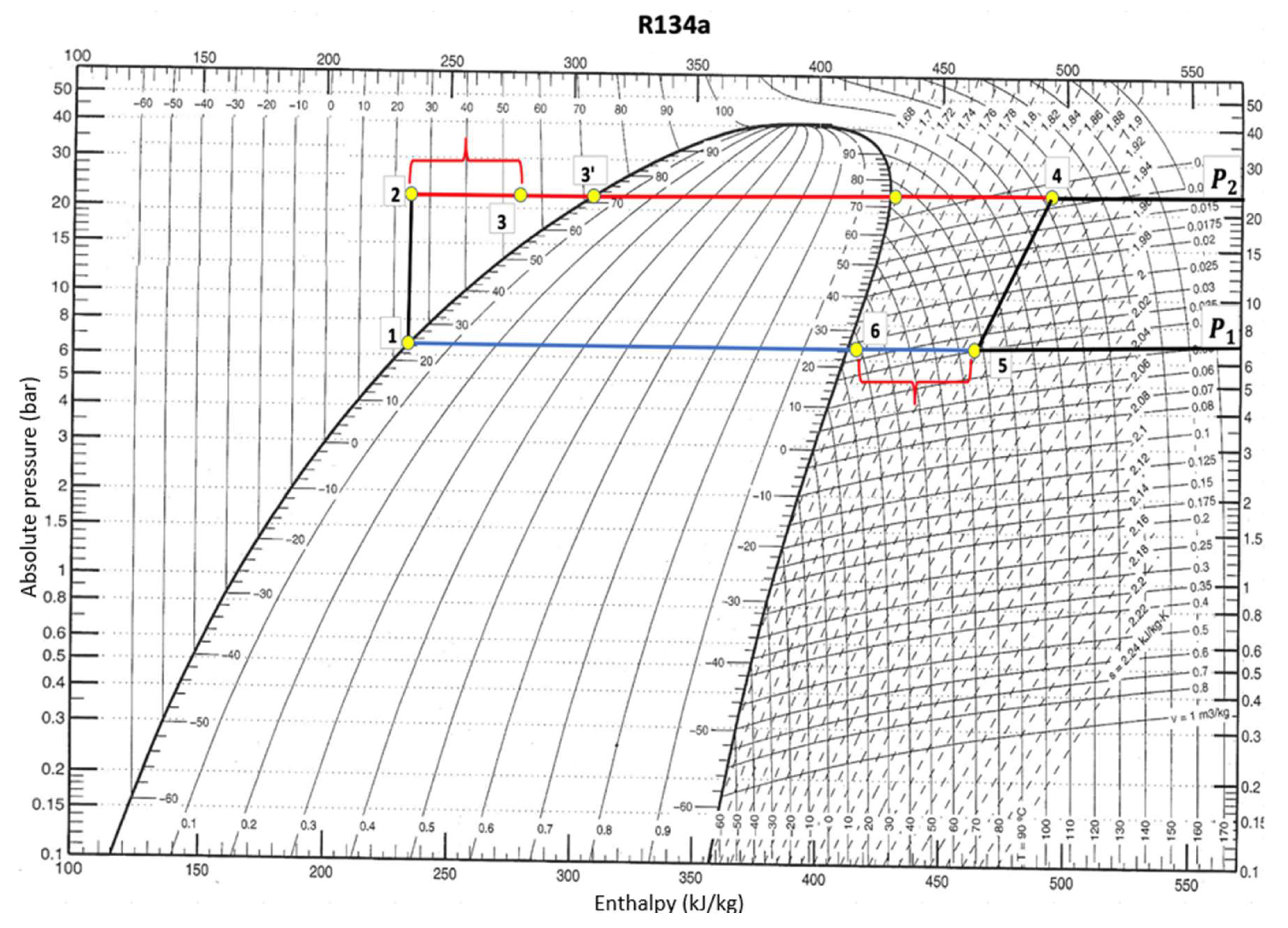
- In pursuit of complex heat source application in the cycle, an experimentally identified and analytically supported linear relationship between the cycle’s energy efficiency and the efficiency of the condensation system’s pump is established. Based on this, the selection of ORC heat source complex utilization strategies is limited by data from one of the sources and is evaluated in terms of the technologically achievable efficiency of pumps in relation to .
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Correction Statement
Nomenclature
| Specific fuel consumption, g/kWh. | |
| Specific isobaric heat of the working fluid, kJ/(kgK). | |
| Charge air flow before entering the engine cylinder, kg/s. | |
| Flow rate of working fluid, kg/s. | |
| Hourly engine fuel consumption, kg/s. | |
| Seawater flow rate, kg/h. | |
| Lower fuel calorific value, kJ/kg. | |
| Enthalpy of the working material before and after the turbogenerator, kJ/kg. | |
| Enthalpy of the working before and after cylinder cooling jacket heat exchanger, kJ/kg. | |
| Enthalpy value which is necessary according to engine manufacturer specification, kJ/kg. | |
| Specific heat ratio. | |
| The cumulative efficiency of the power turbine. | |
| n | revolutions, min1. |
| p | Pressure, Pa |
| Main engine power, kW. | |
| The power generated by the turbogenerator of the WHR system, kW. | |
| The temperature of the working fluid, °C. | |
| Exhaust gas temperature, °C. | |
| Power plant exhaust gas energy part of heat balance, kJ/s. | |
| Total fuel energy, kW. | |
| Power plant scavenges air cooling energy part of heat balance, kJ/s. | |
| Power plant lubricating oil cooling energy part of heat balance, kJ/s. | |
| Power plant cylinder cooling jacket energy part of heat balance, kJ/s. | |
| WHR cycle heat dissipation through overboard water, kJ/s. | |
| Total heat transferred per unit mass of working fluid, kJ/s. | |
| Secondary heat source transferred heat, kJ/s. | |
| Transformed heat in the turbine into mechanical work, kJ/s. | |
| Specific heat of secondary heat sources, kJ/kg. | |
| Heat transferred from the working substance to the condenser, kJ/kg. | |
| Transferred specific heat from secondary heat sources to WF, kJ/kg. | |
| Transferred specific heat from the working material to the overboard water kJ/kg. | |
| Gained heat from seawater cooling. | |
| The degree of pressure drop in the turbine. | |
| Exhaust gas temperature, °C. | |
| Coefficient of performance of the main power plant. | |
| The total coefficient of performance of the ship’s main power plant with a WHR system. | |
| Coefficient of performance of the WHR cycle. | |
| Relative change in ship power plant efficiency with and without ORC. | |
| Ship power plant efficiency with ORC with ISO 8178 operational cycle. | |
| Thermal efficiency coefficient of the secondary heat source exchangers. | |
| Internal (adiabatic) efficiency of the turbogenerator. | |
| Mechanical efficiency of the turbogenerator. | |
| Energy utilization factors of secondary heat sources. | |
| pulse energy input factor. | |
| Temperature of the WF before the turbine, °C. | |
| Abbreviations | |
| CII | Carbon intensity indicator |
| CO2 | Carbon dioxide |
| EE | Efficiency coefficient |
| EEDI | Energy efficiency design index |
| EEXI | Existing energy efficiency index |
| EU | European Union |
| GHG | Greenhouse gases |
| GWP | Global warming potential |
| HCFC | Hydrochlorofluorocarbons |
| IMO | International Maritime Organization |
| LCA | Low-carbon-dioxide-generating fuel |
| MARPOL | International Convention for the Prevention of Pollution from Ships |
| MEPC | Marine Environment Protection Committee |
| ORC | Organic Rankine cycle |
| SRC | Steam Rankine cycle |
| RHE | Recuperative heat exchanger |
| WF | Working fluid |
| WHR | Waste heat recovery |
Appendix A

Appendix B
| Parameter | Data | Dimension |
|---|---|---|
| Manufacturer, type | WÄRTSILA 12V46, trunk type | - |
| Year of manufacture | 2008 | Year |
| Piston stroke | 580 | Mm |
| Average piston speed | 9.7 | m/s |
| Cylinder diameter | 460 | mm |
| Number of cylinders | 12 | vnt. |
| Nominal power | 12,000 | kW |
| Possibility of reversal | Non-reversal | - |
| Type | 4 stroke | - |
| Number of valves | 48 | pcs. |
| Crankshaft revolutions | 350–600 | rpm |
| Type of fuel used | IFO 380 heavy fuel oil, diesel | - |
| Compression pressure | 56 | bar |
| Maximum combustion pressure | 135 | bar |
| Specific fuel consumption | 174 | g/kWh |
| Load Mode % | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 100% | 85% | 75% | 50% | 25% | |||||||
| , cil. kW | 1200 | 1020 | 900 | 600 | 300 | ||||||
| , kg/s | 0.72 | 0.59 | 0.55 | 0.38 | 0.20 * | ||||||
| 0.469 | 0.483 | 0.459 | 0.44 | 0.425 * | |||||||
| 2.5 | 2.68 | 298 | 3.38 | 3.8 * | |||||||
| , kg/s | 26.1 | 23.35 | 23.35 | 18.8 | 14.5 * | ||||||
| , kg/m3 | 4.51 | 4.26 | 4.44 | 4.1 | 3.98 * | ||||||
| , bar | 4.24 | 4.01 | 4.17 | 3.86 | 3.75 | ||||||
| , °C | 366 | 316 | 309 | 273 | 255 * | ||||||
| , bar | 4.45 | 4.2 | 4.38 | 4.06 | 3.93 | ||||||
| , °C | 220 | 211 | 218 | 205 | 200 | ||||||
| 29.344 | 29.324 | 29.34 | 29.311 | 29.3 | |||||||
| , mol | 1.25 | 1.34 | 1.49 | 1.69 | 1.9 | ||||||
| , CO2 kg fuel | 0.0725 | ||||||||||
| , H2O kg fuel | 0.063 | ||||||||||
| , O2 kg fuel | 0.156 | 0.174 | 0.206 | 0.248 | 0.291 | ||||||
| , N2 kg fuel | 0.99 | 1.06 | 1.18 | 1.338 | 1.51 | ||||||
| , mol | 1.28 | 1.37 | 1.52 | 1.72 | 1.996 | ||||||
| , kj/kmolK | 20.795 | ||||||||||
| , kj/kmolK | 29.11 | ||||||||||
| , kj/kmolK | 22.31 | 22.11 | 21.93 | 21.67 | 21.46 | ||||||
| , kj/kmolK | 30.63 | 30.43 | 30.25 | 29.96 | 29.78 | ||||||
| , kW | 8990 | 6622 | 6622 | 4411 | 2387 | ||||||
| , kW | 30,744 | 25,193 | 23,485 | 16,226 | 8540 | ||||||
| , kW | 14,400 | 12,240 | 10,800 | 7200 | 3600 | ||||||
| , kW | 4369 | 3629 | 3851 | 2814 | 1010 | ||||||
| + , kW | 2985 | 2702 | 2212 | 1801 | 1543 | ||||||
| , kW | 420 | Not applicable | |||||||||
| EXHAUST GAS | |||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Working Material | Load, % | Working Fluid Enthalpy (pos. 12), kJ/kg | Exhaust Gas Temperature (pos. 12), C | Working Material Temperature (pos. 6) | Working Fluid Enthalpy (pos. 6), kJ/kg | Working Material Flow, kg/s | Pressure, Bar (pos. 6) | Pressure decrease Ratio (in Turbine, pos. 6) | Power, kW | Scavenge Air Temperature (pos.3) | Scavenge Air Flow, kg/s | Cylinder Cooling Temp. (poz 10) | Cylinder Cooling Flow, kg/s | ||||||||||||||
| R134a | 100 | −86.16 | 132.3 | 364 | 123.2 | 179.5 | 137.3 | 132.3 | 100.5 | 29.5 | 21.84 | 7.14 | 3.059 | 897.7 | N/A | N/A | N/A | N/A | 0.469 | 6.87% | 0.48 | 0.142 | 0.994 | N/A | N/A | ||
| 75 | −86.17 | 132.3 | 309 | 121.5 | 175.9 | 137.3 | 132.3 | 100.5 | 20.3 | 21.84 | 7.14 | 3.059 | 613.6 | 0.459 | 6.25% | 0.142 | 0.994 | ||||||||||
| 50 | −86.18 | 132.3 | 273 | 121.3 | 175.9 | 137.3 | 132.3 | 100.5 | 13.15 | 21.84 | 7.14 | 3.059 | 367.7 | 0.44 | 5.66% | 0.142 | 0.992 | ||||||||||
| 25 | −86.16 | 132.3 | 255 | 121.7 | 175.9 | 137.3 | 132.3 | 100.5 | 8.9 | 21.84 | 7.14 | 3.059 | 248.7 | 0.425 | 7.65% | 0.141 | 0.988 | ||||||||||
| SCAVENGE AIR | |||||||||||||||||||||||||||
| R134a | 100 | N/A | N/A | 103.5 | 61.77 | 47.49 | 23.57 | 21 | 21.84 | 7.14 | 3.059 | 477.3 | 220 | 55.42 | 26.1 | N/A | N/A | 0.469 | 3.93% | 0.47 | 0.263 | N/A | 0.9975 | N/A | |||
| 75 | 103.2 | 61.38 | 47.06 | 23.19 | 18.6 | 21.84 | 7.14 | 3.059 | 422 | 218 | 55.08 | 23.35 | 0.459 | 4.46% | 0.265 | 0.9995 | |||||||||||
| 50 | 105 | 63.37 | 49.27 | 25.17 | 13.7 | 21.84 | 7.14 | 3.059 | 313.8 | 205 | 55.64 | 18.8 | 0.44 | 4.91% | 0.257 | 0.9958 | |||||||||||
| 25 | 105.5 | 63.92 | 49.87 | 25.71 | 10.2 | 21.84 | 7.14 | 3.059 | 234.3 | 200 | 55.72 | 14.5 | 0.425 | 7.25% | 0.255 | 0.9951 | |||||||||||
| CYLINDER COOLING | |||||||||||||||||||||||||||
| R134a | 100 | N/A | N/A | 87.62 | 43.9 | 27.55 | 5.815 | 15 | 21.84 | 7.14 | 3.059 | 309.9 | N/A | N/A | 96 | 75.26 | 35.7 | 0.469 | 2.76% | 0.47 | 0.35889 | N/A | N/A | 0.988 | |||
| 75 | 87.62 | 43.9 | 27.55 | 5.813 | 11 | 21.84 | 7.14 | 3.059 | 227.2 | 96 | 75.51 | 26.5 | 0.459 | 2.65% | 0.35455 | 0.976 | |||||||||||
| 50 | 87.62 | 43.89 | 27.54 | 5.81 | 9 | 21.84 | 7.14 | 3.059 | 185.9 | 96 | 75.33 | 21.5 | 0.44 | 3.13% | 0.35784 | 0.984 | |||||||||||
| 25 | 87.61 | 43.89 | 27.54 | 5.81 | 7.8 | 21.84 | 7.14 | 3.059 | 161.1 | 96 | 75.18 | 18.5 | 0.425 | 5.20% | 0.36139 | 0.994 | |||||||||||
| EXHAUST GAS + SCAVENGE AIR + CYLINDER COOLING | |||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Working Material | Load, % | Working Fluid Enthalpy (pos. 12), kJ/kg | Exhaust Gas Temperature (pos. 12), C | Working Material Temperature (pos. 6) | Working Fluid Enthalpy (pos. 6), kJ/kg | Working Material Flow, kg/s | Pressure, Bar (pos. 6) | Pressure decrease Ratio (in Turbine, pos. 6) | Power, kW | Scavenge Air Temperature (pos.3) | Scavenge Air Flow, kg/s | Cylinder Cooling Temp. (poz 10) | Cylinder Cooling Flow, kg/s | ||||||||||||||
| Before | After | Before | After | Before | After | Before | After | Before | After | Before | After | Before | After | ||||||||||||||
| R134a | 100 | 28.76 | 135.7 | 364 | 120.5 | 178.8 | 140.3 | 135.7 | 103.6 | 60.4 | 21.84 | 7.14 | 3.059 | 1842.2 | 220 | 80.59 | 26.1 | 96 | 76.09 | 35.7 | 0.469 | 0.520 | 13.46% | 0.132 | 0.996 | 0.846 | 0.948 |
| 75 | 38.43 | 136 | 309 | 120.4 | 179.1 | 140.5 | 136 | 103.9 | 45.6 | 21.84 | 7.14 | 1391.8 | 218 | 80.48 | 23.35 | 96 | 76.1 | 26.5 | 0.459 | 13.49% | 0.131 | 0.994 | 0.845 | 0.948 | |||
| 50 | 47.19 | 136.3 | 273 | 120.1 | 179.3 | 140.7 | 136.3 | 104.2 | 32.5 | 21.84 | 7.14 | 992.7 | 205 | 79.82 | 18.8 | 96 | 76.1 | 21.5 | 0.44 | 14.39% | 0.130 | 0.993 | 0.835 | 0.948 | |||
| 25 | 55.55 | 136.5 | 255 | 120.6 | 179.5 | 140.9 | 136.5 | 104.4 | 24.2 | 21.84 | 7.14 | 739.6 | 200 | 79.57 | 14.5 | 96 | 76.09 | 18.5 | 0.425 | 21.39% | 0.132 | 0.991 | 0.831 | 0.948 | |||
| EXHAUST GAS + SCAVENGE AIR | |||||||||||||||||||||||||||
| R134a | 100 | 72.29 | 178 | 364 | 120.3 | 178 | 139.4 | 134.8 | 102.8 | 46.9 | 21.84 | 7.14 | 3.059 | 1426.9 | 220 | 80.59 | 26.1 | N/A | 0.469 | 0.504 | 10.56% | 0.133 | 0.996 | 0.846 | N/A | ||
| 75 | 75.79 | 178.3 | 309 | 120.1 | 178.3 | 139.8 | 135.2 | 103.1 | 35.6 | 21.84 | 7.14 | 3.059 | 1084.2 | 218 | 80.48 | 23.35 | 0.459 | 10.63% | 0.131 | 0.994 | 0.845 | ||||||
| 50 | 80.37 | 178.5 | 273 | 120.8 | 178.5 | 139.9 | 135.4 | 103.3 | 24.3 | 21.84 | 7.14 | 3.059 | 740.5 | 205 | 79.82 | 18.8 | 0.44 | 10.87% | 0.130 | 0.993 | 0.835 | ||||||
| 25 | 83.79 | 178.6 | 255 | 120.5 | 178.6 | 140.1 | 135.5 | 103.4 | 17.2 | 21.84 | 7.14 | 3.059 | 524.3 | 200 | 79.57 | 14.5 | 0.425 | 15.36% | 0.131 | 0.991 | 0.831 | ||||||
| EXHAUST GAS + CYLINDER COOLING | |||||||||||||||||||||||||||
| R134a | 100 | −12.1 | 134.5 | 364 | 120.3 | 177.8 | 139.2 | 134.5 | 102.5 | 44.1 | 21.84 | 7.14 | 3.059 | 1340.8 | 96 | 75.19 | 35.7 | 0.469 | 0.500 | 9.96% | 0.141084 | 0.999 | N/A | 0.991 | |||
| 75 | −8.334 | 134.6 | 309 | 120.6 | 177.9 | 139.3 | 134.6 | 102.6 | 31.1 | 21.84 | 7.14 | 3.059 | 945.8 | 96 | 75.19 | 26.5 | 0.459 | 9.34% | 0.140796 | 0.997 | 0.991 | ||||||
| 50 | 3.048 | 135 | 273 | 120.5 | 178.2 | 139.6 | 135 | 102.9 | 21.9 | 21.84 | 7.14 | 3.059 | 666.6 | 96 | 75.19 | 21.5 | 0.44 | 9.84% | 0.140583 | 0.997 | 0.991 | ||||||
| 25 | 15.9 | 135.3 | 255 | 120.7 | 178.5 | 139.9 | 135.3 | 103.3 | 16.4 | 21.84 | 7.14 | 3.059 | 499.7 | 96 | 75.2 | 18.5 | 0.425 | 14.68% | 0.1402 | 0.995 | 0.991 | ||||||
| SCAVENGE AIR + CYLINDER COOLING | |||||||||||||||||||||||||||
| R134a | 100 | 170.7 | 132 | 126.2 | 94.9 | 31 | 21.84 | 7.14 | 3.059 | 921.9 | 220 | 83.14 | 26.1 | 96 | 75.19 | 35.7 | 0.469 | 0.490 | 7.04% | 0.132168 | N/A | 0.831 | 0.991 | ||||
| 75 | 152.4 | 113.3 | 104.9 | 75.5 | 26 | 21.84 | 7.14 | 3.059 | 727.6 | 218 | 79.63 | 23.35 | 96 | 75.19 | 26.5 | 0.459 | 7.31% | 0.14799 | 0.850 | 0.991 | |||||||
| 50 | 176.8 | 138.2 | 133.4 | 101.5 | 17 | 21.84 | 7.14 | 3.059 | 515.3 | 205 | 79.89 | 18.8 | 96 | 75.19 | 21.5 | 0.44 | 7.73% | 0.112181 | 0.645 | 0.991 | |||||||
| 25 | 177 | 138.4 | 133.6 | 101.7 | 14 | 21.84 | 7.14 | 3.059 | 424.7 | 200 | 79.23 | 14.5 | 96 | 75.19 | 18.5 | 0.425 | 12.58% | 0.124063 | 0.680 | 0.991 | |||||||
References
- United Nations Framework Convention on Climate Change (UNFCCC). World Trade and the Reduction of CO2 Emissions, International Chamber of Shipping. 2014. Available online: https://www.ics-shipping.org/wp-content/uploads/2014/08/shipping-world-trade-and-the-reduction-of-co2-emissions-min.pdf (accessed on 23 November 2023).
- International Maritime Organization (IMO). Third IMO Greenhouse Gas Study 2014. Available online: https://www.imo.org/en/ourwork/environment/pages/greenhouse-gas-studies-2014.aspx (accessed on 9 November 2023).
- Maritime Industry Authority. MARPOL 73/78. 1973. Available online: https://marina.gov.ph/wp-content/uploads/2020/10/MARPOL-73_78.pdf (accessed on 9 November 2023).
- MARPOL Annex VI, Resolution MEPC. 308(73). 2018. Available online: https://wwwcdn.imo.org/localresources/en/Knowledge-Centre/IndexofIMOResolutions/MEPCDocuments/MEPC.308(73).pdf (accessed on 23 November 2023).
- Guidelines on Survey and Certification of the Attained Energy Efficiency Existing Ship Index (EEXI), Resolution MEPC. 351(78). 2022. Available online: https://wwwcdn.imo.org/localresources/en/KnowledgeCentre/IndexofIMOResolutions/MEPCDocuments/MEPC.351(78).pdf (accessed on 23 November 2023).
- Interim Guidelines on Correction Factors and Voyage Adjustments for CII Calculations, Resolution MEPC.355(78). 2022. Available online: https://wwwcdn.imo.org/localresources/en/KnowledgeCentre/IndexofIMOResolutions/MEPCDocuments/MEPC.355(78).pdf (accessed on 23 November 2023).
- EU Monitor. Use of Renewable and Low-Carbon Fuels in Maritime Transport, COM(2021)562. Available online: https://www.eumonitor.eu/9353000/1/j4nvhdfdk3hydzq_j9vvik7m1c3gyxp/vlkimqggznzr (accessed on 23 November 2023).
- European Commision, Monitor. Directive of the European Parliament and of the Council, 2021, COM(2021)551. Available online: https://eur-lex.europa.eu/resource.html?uri=cellar:618e6837-eec6-11eb-a71c-01aa75ed71a1.0001.02/DOC_1&format=PDF (accessed on 1 November 2023).
- International Renewable Energy Agency. A Pathway to Decarbonise the Shipping Sector by 2050; International Renewable Energy Agency: Abu Dhabi, United Arab Emirates, 2021; ISBN 978-92-9260-330-4. [Google Scholar]
- Maersk Mc-Kinney Moller Center for Zero Carbon Shipping. The Shipping Industry’s Fuel Choices on the Path to Net Zero. 2023. Available online: https://www.globalmaritimeforum.org/content/2023/04/the-shipping-industrys-fuel-choices-on-the-path-to-net-zero_final.pdf (accessed on 1 December 2023).
- Theotokatos, G.; Livanos, G.A. Techno-economic analysis of single pressure exhaust gas waste heat recovery systems in marine propulsion plants. Proc. Inst. Mech. Eng. Part M J. Eng. Marit. Environ. 2013, 227, 83–97. [Google Scholar]
- Livanos, G.; Theotokatos, G.; Pagonis, D. Techno-economic investigation of alternative propulsion plants for ferries and roro ships. Energy Convers. Manag. 2014, 79, 640–651. [Google Scholar] [CrossRef]
- Dimopoulos, G.; Georgopoulou, C.; Kakalis, N. Modelling and optimization of an integrated marine combined cycle system. In Proceedings of the 24th International Conference on Energy, Cost, Optimization, Simulation and Environmental Impact of Energy Systems (ECOS), Novi Sad, Serbia, 4–7 July 2011; pp. 1283–1298. [Google Scholar]
- Hountalas, D.T.; Katsanos, C.; Mavropoulos, G.C. Efficiency improvement of large scale 2-stroke diesel engines through the recovery of exhaust gas using a rankine cycle. Procedia-Soc. Behav. Sci. 2012, 48, 1444–1453. [Google Scholar] [CrossRef]
- Maritime Forecast 2050, Energy Transition Outlook, DNV. 2022. Available online: https://www.dnv.com/maritime/publications/maritime-forecast-2023/ (accessed on 10 December 2023).
- Dharmalingam, P.; Kumar, J.N.; Suryanarayanan, R.; Kumar, S.S. Waste heat recovery. In The National Certification Examination for Energy Managers and Energy Auditors, 2nd ed.; Bureau of Energy Efficiency: New Delhi, India, 2004; pp. 1–18. [Google Scholar]
- Woodyard, D. Pounder’s Marine Diesel Engines and Gas Turbines, 9th ed.; Elsevier: Oxford, UK, 2009. [Google Scholar]
- Olaniyi, E.O.; Prause, G. Investment Analysis of Waste Heat Recovery System Installations on Ships’ Engines. J. Mar. Sci. Eng. 2020, 8, 811. [Google Scholar] [CrossRef]
- Baldi, F.; Gabrielii, C. A feasibility analysis of waste heat recovery systems for marine applications. Energy 2015, 80, 654–665. [Google Scholar] [CrossRef]
- Altosole, M. Waste heat recovery systems from marine diesel engines: Comparison between new design and retrofitting solutions. In Maritime Technology and Engineering; CRC Press: Boca Raton, FL, USA, 2014; pp. 735–742. [Google Scholar]
- Díaz-Secades, L.A.; González, R.; Rivera, N.; Montañés, E.; Quevedo, J.R. Waste heat recovery system for marine engines optimized through a preference learning rank function embedded into a Bayesian optimizer. Ocean Eng. 2023, 281, 114747. [Google Scholar] [CrossRef]
- Annual Report to the Congress for 1982 (March 1983) Industrial and Commercial Cogeneration (February 1983). Available online: https://www.princeton.edu/~ota/disk3/1983/9565/9565.PDF (accessed on 3 December 2023).
- Hatchman, J.C. Steam cycles for waste heat recovery: A case study. R&D J. 1991, 7, 32–38. [Google Scholar]
- Korlak, P.K. Comparative analysis of the heat balance results of the selected Tier III-compliant gas-fuelled two-stroke main engines. Combust. Engines 2023, 193, 24–28. [Google Scholar] [CrossRef]
- Tchanche, B.F.; Bertrand, M.; Papadakis, G. Heat resources and organic Rankine cycle machines. Renew. Sustain. Energy Rev. 2014, 39, 1185–1199. [Google Scholar] [CrossRef]
- Tchanche, B.F.; Lambrinos, G.; Frangoudakis, A.; Papadakis, G. Low-grade heat conversion into power using organic Rankine cycles—A review of various applications. Renew. Sustain. Energy Rev. 2011, 15, 3963–3979. [Google Scholar] [CrossRef]
- Tian, H.; Shu, G.; Wei, H.; Liang, X.; Liu, L. Fluids and parameters optimization for the organic Rankine cycles (ORCs) used in exhaust heat recovery of Internal Combustion Engine (ICE). Energy 2012, 47, 125–136. [Google Scholar] [CrossRef]
- Wei, D.; Lu, X.; Lu, Z.; Gu, J. Performance analysis and optimization of organic Rankine cycle (ORC) for waste heat recovery. Energy Convers. Manag. 2007, 48, 1113–1119. [Google Scholar] [CrossRef]
- Konur, O.; Yuksel, O.; Korkmaz, S.A.; Colpan, C.O.; Saatcioglu, O.Y.; Muslu, I. Thermal design and analysis of an organic rankine cycle system utilizing the main engine and cargo oil pump turbine based waste heats in a large tanker ship. J. Clean. Prod. 2022, 368, 133230. [Google Scholar] [CrossRef]
- Díaz-Secades, L.A.; González, R.; Rivera, N. Waste heat recovery from marine engines and their limiting factors: Bibliometric analysis and further systematic review. Clean. Energy Syst. 2023, 6, 100083. [Google Scholar] [CrossRef]
- Song, J.; Song, Y.; Gu, C. Thermodynamic and lysis and performance optimization of an Organic Rankine Cycle (ORC) waste heat recovery system for marine diesel engines. Energy 2015, 82, 976–985. [Google Scholar] [CrossRef]
- Ng, C.W.; Tam, I.C.K.; Wu, D. Study of a Waste Energy Driven Organic Rankine Cycle Using Free Piston Linear Expander for Marine Application; The National Technical University of Athens: Athens, Greece, 2019. [Google Scholar]
- Park, B.-S.; Usman, M.; Imran, M.; Pesyridis, A. Review of Organic Rankine Cycle experimental data trends. Energy Convers. Manag. 2018, 173, 679–691. [Google Scholar] [CrossRef]
- Grljušić, M.; Medica, V.; Radica, G. Calculation of efficiencies of a ship power plant operating with waste heat and power production. Energies 2015, 8, 4273–4299. [Google Scholar] [CrossRef]
- Baldi, F.; Larsen, U.; Gabrielii, C. Comparison of different procedures for the optimisation of a combined diesel engine and organic Rankine cycle system based on ship operational profile. Ocean Eng. 2015, 110, 85–93. [Google Scholar] [CrossRef]
- Necmi, O. Thermodynamic Analysis of Gas Turbine Cogeneration Power Plants. Int. J. Eng. Sci. Res. Technol. 2016, 5, 736–742. [Google Scholar] [CrossRef]
- Konur, O.; Yuksel, O.; Korkmaz, S.A.; Colpan, C.O.; Saatcioglu, O.Y.; Koseoglu, B. Operation-dependent exergetic sustainability assessment and environmental analysis on a large tanker ship utilizing Organic Rankine cycle system. Energy 2023, 262, 125477. [Google Scholar] [CrossRef]
- Konur, O.; Colpan, C.O.; Saatcioglu, O.Y. A comprehensive review on organic Rankine cycle systems used as waste heat recovery technologies for marine applications. Energy Sources Part A Recover. Util. Environ. Eff. 2022, 44, 4083–4122. [Google Scholar] [CrossRef]
- Ng, C. Modelling and Simulation of Organic Rankine Cycle Waste Heat Recovery System with the Operational Profile of a Ship; Newcastle University: Newcastle upon Tyne, UK, 2021. [Google Scholar]
- Ng, C.; Tam, I.C.K.; Wetenhall, B. Waste Heat Source Profiles for Marine Application of Organic Rankine Cycle. J. Mar. Sci. Eng. 2022, 10, 1122. [Google Scholar] [CrossRef]
- Shu, G.; Liu, P.; Tian, H.; Wang, X.; Jing, D. Operational profile based thermal-economic analysis on an Organic Rankine cycle using for harvesting marine engine’s exhaust waste heat. Energy Convers. Manag. 2017, 146, 107–123. [Google Scholar] [CrossRef]
- Qu, J.; Feng, Y.; Zhu, Y.; Zhou, S.; Zhang, W. Design and thermodynamic analysis of a combined system including steam Rankine cycle, organic Rankine cycle, and power turbine for marine low-speed diesel engine waste heat recovery. Energy Convers. Manag. 2021, 245, 114580. [Google Scholar] [CrossRef]
- Baldasso, E.; Mondejar, M.E.; Andreasen, J.G.; Rønnenfelt, K.A.T.; Nielsen, B.; Haglind, F. Design of organic Rankine cycle power systems for maritime applications accounting for engine backpressure effects. Appl. Therm. Eng. 2020, 178, 115527. [Google Scholar] [CrossRef]
- Liu, X.; Nguyen, M.Q.; Chu, J.; Lan, T.; He, M. A novel waste heat recovery system combing steam Rankine cycle and organic Rankine cycle for marine engine. J. Clean. Prod. 2020, 265, 121502. [Google Scholar] [CrossRef]
- Ng, C.; Tam, I.C.K.; Wu, D. Thermo-Economic Performance of an Organic Rankine Cycle System Recovering Waste Heat Onboard an Offshore Service Vessel. J. Mar. Sci. Eng. 2020, 8, 351. [Google Scholar] [CrossRef]
- Sellers, C. Field operation of a 125kW ORC with ship engine jacket water. Energy Procedia 2017, 129, 495–502. [Google Scholar] [CrossRef]
- Mohammed, A.G.; Mosleh, M.; El-Maghlany, W.M.; Ammar, N.R. Performance analysis of supercritical ORC utilizing marine diesel engine waste heat recovery. Alex. Eng. J. 2020, 59, 893–904. [Google Scholar] [CrossRef]
- Ouyang, T.; Su, Z.; Zhao, Z.; Wang, Z.; Huang, H. Advanced exergo-economic schemes and optimization for medium–low grade waste heat recovery of marine dual-fuel engine integrated with accumulator. Energy Convers. Manag. 2020, 226, 113577. [Google Scholar] [CrossRef]
- Ouyang, T.; Su, Z.; Yang, R.; Li, C.; Huang, H.; Wei, Q. A framework for evaluating and optimizing the cascade utilization of medium-low grade waste heat in marine dual-fuel engines. J. Clean. Prod. 2020, 276, 123289. [Google Scholar] [CrossRef]
- Su, Z.; Ouyang, T.; Chen, J.; Xu, P.; Tan, J.; Chen, N.; Huang, H. Green and efficient configuration of integrated waste heat and cold energy recovery for marine natural gas/diesel dual-fuel engine. Energy Convers. Manag. 2020, 209, 112650. [Google Scholar] [CrossRef]
- Wang, X.; Shu, G.-Q.; Tian, H.; Liang, Y.; Wang, X. Simulation and Analysis of an ORC-Desalination Combined System Driven by the Waste Heat of Charge Air at Variable Operation Conditions; SAE Technical Paper 2014-01-1949; SAE International: Warrendale, PA, USA, 2014. [Google Scholar]
- Chen, W.; Fu, B.; Zeng, J.; Luo, W. Research on the Operational Performance of Organic Rankine Cycle System for Waste Heat Recovery from Large Ship Main Engine. Appl. Sci. 2023, 13, 8543. [Google Scholar] [CrossRef]
- Lebedevas, S.; Čepaitis, T. Research of Organic Rankine Cycle Energy Characteristics at Operating Modes of Marine Diesel Engine. J. Mar. Sci. Eng. 2021, 9, 1049. [Google Scholar] [CrossRef]
- Report of the Marine Environment Protection Committee on its Seventy-Sixth Session; MEPC 76/15/ADD.1; MEPC: London, UK, 24 August 2021.
- ES 2022/0099(COD). Proposal for a Regulation of the European Parliament and of the Council on Fluorinated Greenhouse Gases, Amending Directive (EU) 2019/1937 and Repealing Regulation (EU) No 517/2014. 19 October 2023. Available online: https://eur-lex.europa.eu/legal-content/EN/TXT/?uri=celex%3A52022PC0150 (accessed on 9 November 2023).
- Hafner, A.; Gabrielii, C.H.; Widell, K. Refrigeration Units in Marine Vessels—Alternatives to HCFCs and high GWP HFCs; Nordic Council of Ministers: Copenhagen, Denmark, 2019. [Google Scholar]
- Møller-Hansen, T. Alternatives to HCFC as Refrigerant in Shipping Vessels; TemaNord 2000:573; Nordic Council of Ministers: Copenhagen, Denmark, 2000. [Google Scholar]
- Wärtsila “Marine market shares 2022”. 2022. Available online: https://www.wartsila.com/docs/default-source/investors/market-shares/ship-power-market-share/marine-market-share-2022.pdf?sfvrsn=37f13b43_1 (accessed on 28 December 2023).
- Lam JS, L.; Piga, B.; Zengqi, X. Role of Bio-LNG in Shipping Industry Decarbonization; Nanyang Technological University: Singapore, 2022. [Google Scholar]
- BioLNG in Transport: Making Climate Neutrality a Reality A joint White Paper about bioLNG Production and Infrastructure as Enabler for Climate Neutral Road and Maritime Transport. EBA, GIE, NGVA & SEA-LNG. 2020. Available online: https://www.europeanbiogas.eu/wp-content/uploads/2020/11/BioLNG-in-Transport_Making-Climate-Neutrality-a-Reality.pdf (accessed on 28 December 2023).
- Alternative Fuels for Containerships. DNV. 2023. Available online: https://www.dnv.com/maritime/publications/alternative-fuels-for-containerships-methanol-and-ammonia-download/ (accessed on 28 December 2023).
- Lebedev, S.; Nechayev, L. Sovershenstvovaniye Pokazateley Vysokooborotnykh Dizeley Unifitsirovannogo Tiporazmera; Akademiya Transporta RF: Barnaul, Russia, 1999; p. 48. [Google Scholar]
- Lebedev, S.; Lebedeva, G.; Matievskij, D.; Reshetov, V. Formirovanie Konstruktivnogo Rjada Porshnej Dlja Tipaža Vysokooborotnyh Forsirovannyh Dizelej; Akademiya Transporta RF: Barnaul, Russia, 2003; p. 49. [Google Scholar]
- Kreienbaum, J. The Energy Crises of the 1970s as Challenges to the Industrialized World; Historisches Institut, Universität Rostock: Rostock, Germany, 2013. [Google Scholar]
- Grabowski, L.; Wendeker, M.; Pietrykowski, K. AVL Simulation Tools Practical Applications; Lublin University of Technology: Lublin, Poland, 2012. [Google Scholar]
- Wiebe, I. Brennverlauf und Kreisprozesse Vonverbrennungsmotoren; VEB Verlag Technik: Berlin, Germany, 1970; 280p. [Google Scholar]
- Woschni, G. Eine Methode zur Vorausberechnung der Änderung des Brenverlaufs Mittelschnellaufender Dieselmotoren bei Geanderten Betriebsbedigungen; Springer Fachmedien: Wiesbaden, Germany, 1973; pp. 106–110. [Google Scholar]
- Woschni, G. Verbrennungsmotoren; TU Munchen: Munich, Germany, 1988. [Google Scholar]

| Mass Percentage | Boiling Point (°C) | Critical Pressure (kPa) | Critical Temperature (°C) | Chemical Composition | |
|---|---|---|---|---|---|
| R134a | 100 | −26.07 | 4060 | 101.06 | CH2FCF3 |
| Load Modes | Pe, kW | n, rmp | , g/kWh | Gair, kg/s | Gf, kg/s | , °C |
|---|---|---|---|---|---|---|
| 100% | 1200 | 600 | 178.7 | 26.1 | 0.72 | 366 |
| 75% | 900 | 545 | 188.7 | 23.35 | 0.54 | 309 |
| 50% | 600 | 478 | 190.6 | 18.8 | 0.384 | 273 |
| 25% | 300 | 378 | 197.0 | 14.5 | 0.2 | 255 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lebedevas, S.; Čepaitis, T. Complex Use of the Main Marine Diesel Engine High- and Low-Temperature Waste Heat in the Organic Rankine Cycle. J. Mar. Sci. Eng. 2024, 12, 521. https://doi.org/10.3390/jmse12030521
Lebedevas S, Čepaitis T. Complex Use of the Main Marine Diesel Engine High- and Low-Temperature Waste Heat in the Organic Rankine Cycle. Journal of Marine Science and Engineering. 2024; 12(3):521. https://doi.org/10.3390/jmse12030521
Chicago/Turabian StyleLebedevas, Sergejus, and Tomas Čepaitis. 2024. "Complex Use of the Main Marine Diesel Engine High- and Low-Temperature Waste Heat in the Organic Rankine Cycle" Journal of Marine Science and Engineering 12, no. 3: 521. https://doi.org/10.3390/jmse12030521
APA StyleLebedevas, S., & Čepaitis, T. (2024). Complex Use of the Main Marine Diesel Engine High- and Low-Temperature Waste Heat in the Organic Rankine Cycle. Journal of Marine Science and Engineering, 12(3), 521. https://doi.org/10.3390/jmse12030521







