The Four-Dimensional Natural Transform Adomian Decomposition Method and (3+1)-Dimensional Fractional Coupled Burgers’ Equation
Abstract
:1. Introduction
2. Basic Definitions of the Natural Transform Method
3. Analysis of the Four-Dimensional Natural Adomian Decomposition Method
- Step 1: By implementing the four-dimensional natural transform to Equation (9), we obtain
- Step 2: Now, by using the differentiation property of the natural transform, we have
- Step 3: By employing the inverse four-dimensional natural transform for Equation (12), we obtain
- Step 4: The four-dimensional natural Adomian decomposition method assumes series solutions of the functions , , and , which are determined by
- Step 5: After applying the four-dimensional natural Adomian decomposition method, we introduce the recursive relations as follows:
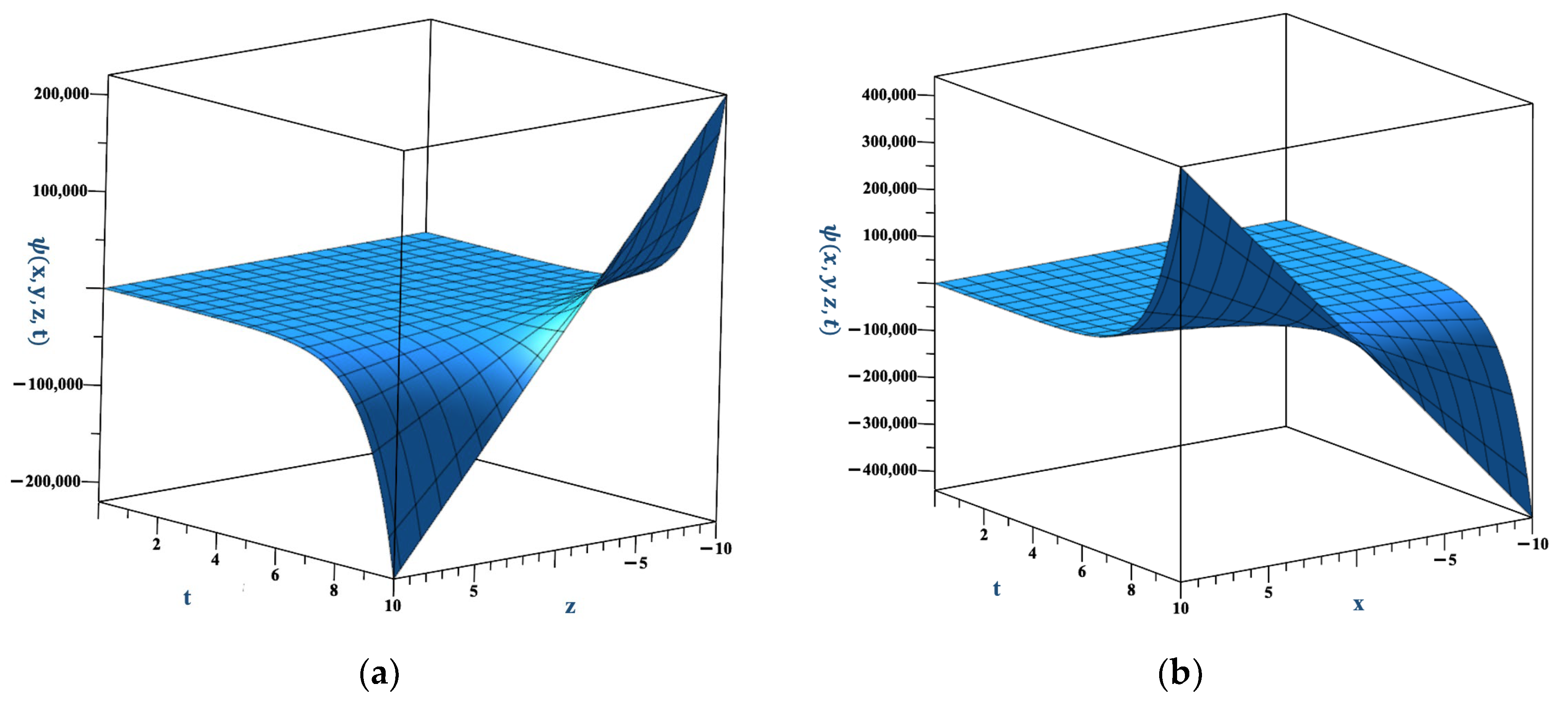
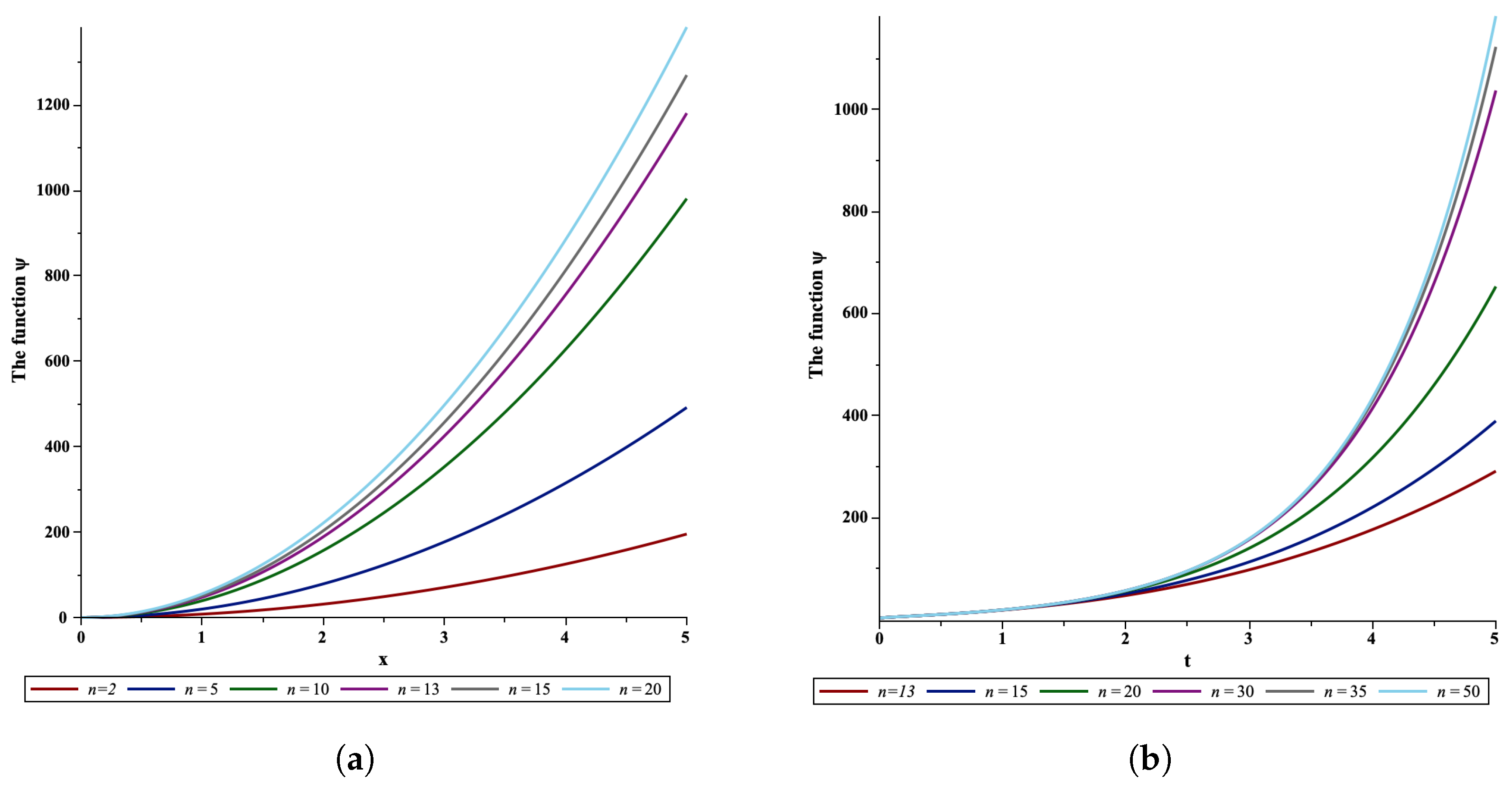
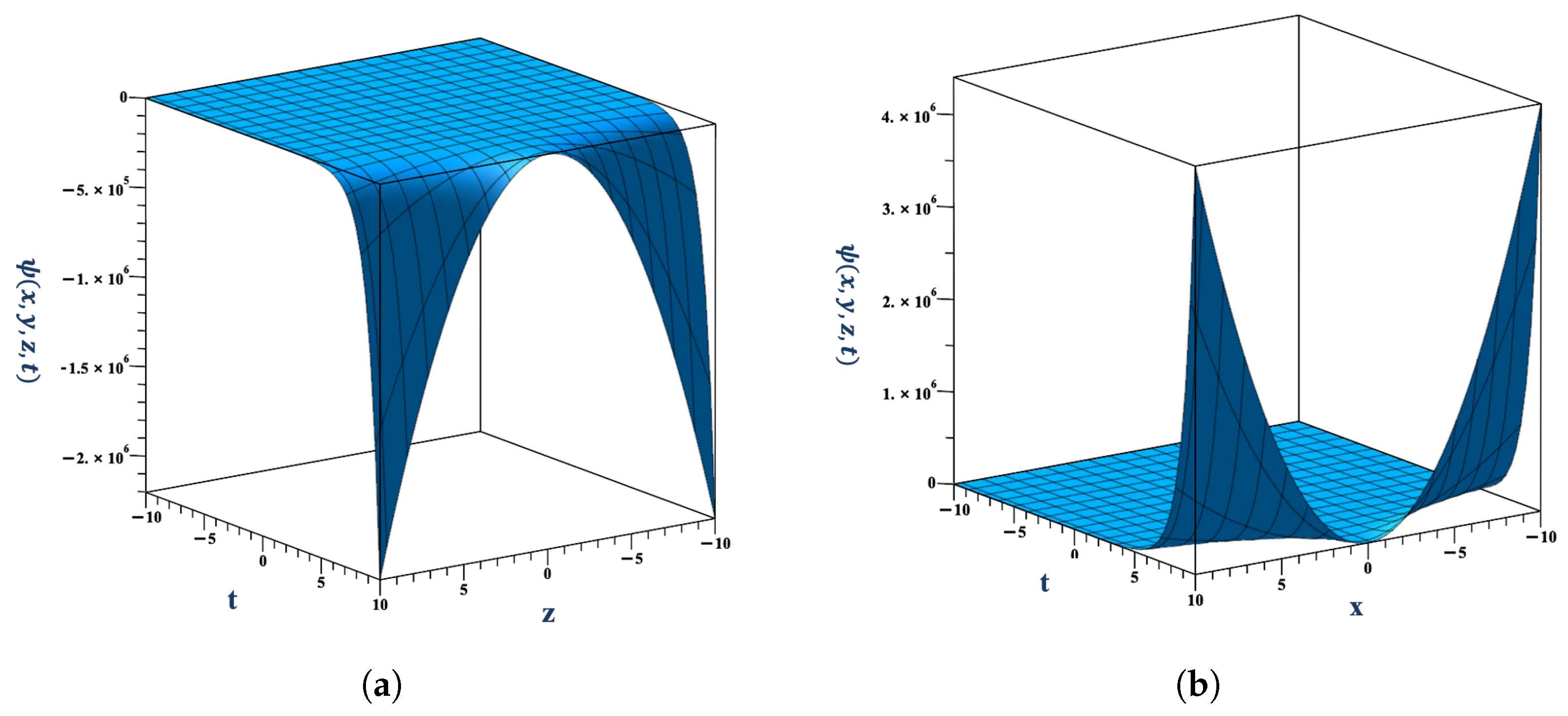
4. Four-Dimensional Natural Adomian Decomposition Method and Singular (3+1)-Dimensional Fractional Coupled Burgers’ Equation
- Step 1: Multiply both sides of Equation (41) by to obtain
- Step 2: Operating the four-dimensional natural transform for both sides of Equation (42) yields
- Step 3: By integrating both sides of Equation (46) from 0 to to and 0 to with respect to , and , respectively, we have
- Step 6: After applying the four-dimensional natural Adomian decomposition method, we introduce the recursive relations as follows:
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Machado, J.T.; Kiryakova, V.; Mainardi, F. Recent history of fractional calculus. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 1140–1153. [Google Scholar] [CrossRef]
- Sabatier, J.A.T.M.J.; Agrawal, O.P.; Machado, J.T. Advances in Fractional Calculus; Springer: Dordrecht, The Netherlands, 2007; Volume 4. [Google Scholar]
- Baleanu, D.; Diethelm, K.; Scalas, E.; Trujillo, J.J. Fractional Calculus: Models and Numerical Methods; World Scientific: Singapore, 2012; Volume 3. [Google Scholar]
- Debnath, L. Recent applications of fractional calculus to science and engineering. Int. J. Math. Math. Sci. 2003, 2003, 3413–3442. [Google Scholar] [CrossRef]
- Hilfer, R. Applications of Fractional Calculus in Physics; World Scientific Publishing Co., Inc.: River Edge, NJ, USA, 2000. [Google Scholar]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; North Holland Mathematics Studies, Elsevier Science B.V.: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Baleanu, D.; Diethelm, K.; Scalas, E.; Trujillo, J.J. Fractional Calculus, Series on Complexity, Nonlinearity and Chaos; World Scientific Publishing Co. Pte. Ltd.: Hackensack, NJ, USA, 2012; Volume 3. [Google Scholar]
- Riemann, B. Versuch einer allgemeinen Auffassung der Integration und Differentiation. In Gesammelte Werke; Dover: New York, NY, USA, 1876; Volume 62, pp. 331–344. [Google Scholar]
- Liouville, J. Mémoire sur le Calcul des Différentielles á Indices Quelconques; Walter de Gruyter: Berlin, Germany; New York, NY, USA, 1832. [Google Scholar]
- Almeida, R. A Caputo fractional derivative of a function with respect to another function. Commun. Nonlinear Sci. Numer. Simul. 2017, 44, 460–481. [Google Scholar] [CrossRef]
- Baleanu, D.; Jajarmi, A.; Mohammadi, H.; Rezapour, S. A new study on the mathematical modeling of human liver with Caputo–Fabrizio fractional derivative. Chaos Solitons Fractals 2020, 134, 109705. [Google Scholar] [CrossRef]
- Yang, Q.; Liu, F.; Turner, I. Numerical methods for fractional partial differential equations with Riesz space fractional derivatives. Appl. Math. Model. 2010, 34, 200–218. [Google Scholar] [CrossRef]
- Sousa, J.V.d.C.; De Oliveira, E.C. On the ψ-Hilfer fractional derivative. Commun. Nonlinear Sci. Numer. Simul. 2018, 60, 72–91. [Google Scholar] [CrossRef]
- Mathai, A.; Haubold, H.J. Erdélyi–Kober Fractional Calculus: From a Statistical Perspective, Inspired by Solar Neutrino Physics; Springer: Berlin/Heidelberg, Germany, 2018; Volume 31. [Google Scholar]
- Jarad, F.; Abdeljawad, T.; Hammouch, Z. On a class of ordinary differential equations in the frame of Atangana–Baleanu fractional derivative. Chaos Solitons Fractals 2018, 117, 16–20. [Google Scholar] [CrossRef]
- Scherer, R.; Kalla, S.L.; Tang, Y.; Huang, J. The Grunwald–Letnikov method for fractional differential equations. Comput. Math. Appl. 2011, 62, 902–917. [Google Scholar] [CrossRef]
- Liao, S.J. Beyond Perturbation: Introduction to the Homotopy Analysis Method; CRC Press: Boca Raton, FL, USA, 2003. [Google Scholar]
- Bayrak, M.A.; Demir, A. A new approach for space-time fractional partial differential equations by residual power series method. Appl. Math. Comput. 2018, 336, 215–230. [Google Scholar] [CrossRef]
- Liu, J.G.; Yang, X.J.; Geng, L.L.; Yu, X.J. On fractional symmetry group scheme to the higher dimensional space and time fractional dissipative Burgers equation. Int. J. Geom. Meth. Moder. Phys. 2022, 19, 2250173. [Google Scholar] [CrossRef]
- Li, X.Y.; Wu, B.Y. Iterative reproducing kernel method for nonlinear variable-order space fractional diffusion equations. Int. J. Comput. Math. 2018, 95, 1210–1221. [Google Scholar] [CrossRef]
- Thabet, H.; Kendre, S.D.; Peters, J.F. Travelling wave solutions for fractional Korteweg-de Vries equations via an approximate-analytical method. AIMS Math. 2019, 4, 1203. [Google Scholar] [CrossRef]
- Aljahdaly, N.H. New application through multistage differential transform method. AIP Conf. Proc. 2020, 2293, 420025. [Google Scholar]
- Aljahdaly, N.H.; El-Tantawy, S.A. On the multistage differential transformation method for analyzing damping Duffing oscillator and its applications to plasma physics. Mathematics 2021, 9, 432. [Google Scholar] [CrossRef]
- Wu, G.C.; Baleanu, D. Variational iteration method for fractional calculus-a universal approach by Laplace transform. Adv. Differ. Equ. 2013, 2013, 18. [Google Scholar] [CrossRef]
- Jleli, M.; Kumar, S.; Kumar, R.; Samet, B. Analytical approach for time fractional wave equations in the sense of Yang-Abdel-Aty- Cattani via the homotopy perturbation transform method. Alex. Eng. J. 2020, 59, 2859–2863. [Google Scholar] [CrossRef]
- Prakash, A.; Goyal, M.; Gupta, S. q-homotopy analysis method for fractional Bloch model arising in nuclear magnetic resonance via the Laplace transform. Indian J. Phys. 2020, 94, 507–520. [Google Scholar] [CrossRef]
- Mirzaee, F.; Samadyar, N. On the numerical solution of stochastic quadratic integral equations via operational matrix method. Math. Methods Appl. Sci. 2018, 41, 4465–4479. [Google Scholar] [CrossRef]
- Sarra, S.A.; Kansa, E.J. Multiquadric radial basis function approximation methods for the numerical solution of partial differential equations. Adv. Comput. Mech. 2009, 2, 220. [Google Scholar]
- Shah, R.; Khan, H.; Baleanu, D. Fractional Whitham-Broer-Kaup equations within modified analytical approaches. Axioms 2019, 8, 125. [Google Scholar] [CrossRef]
- Sohail, A.; Maqbool, K.; Ellahi, R. Stability analysis for fractional-order partial differential equations by means of space spectral time Adams Bashforth Moulton method. Numer. Methods Partial. Differ. Equ. 2018, 34, 19–29. [Google Scholar] [CrossRef]
- Khan, Z.H.; Khan, W.A. N-Transform Properties and Applications. NUST J. Eng. Sci. 2008, 1, 127–133. [Google Scholar]
- Rawashdeh, M.S.; Al-Jammal, H. Theories and Applications of the Inverse Fractional Natural Transform Method. Adv. Differ. Equ. 2018, 2018, 222. [Google Scholar] [CrossRef]
- Belgacem, F.B.M.; Silambarasan, R. Theory of Natural Transform. Mathematics in Engineering. Sci. Aerosp. (MESA) J. 2012, 3, 99–124. [Google Scholar]
- Belgacem, F.B.M.; Silambarasan, R. Advances in the Natural Transform. AIP Conf. Proc. 2012, 1493, 106. [Google Scholar] [CrossRef]
- Rawashdeh, M.S.; Maitama, S. Solving coupled system of nonlinear PDE’s using the natural decomposition method. Int. J. Pure Appl. Math. 2014, 92, 757–776. [Google Scholar] [CrossRef]
- Cherif, M.H.; Ziane, D.; Belghaba, K. Fractional natural decomposition method for solving fractional system of nonlinear equations of unsteady flow of a polytropic gas. Nonlinear Stud. 2018, 25, 753–764. [Google Scholar]
- Eltayeb, H.; Abdalla, Y.T.; Bachar, I.; Khabir, M.H. Fractional telegraph equation and its solution by natural transform decomposition method. Symmetry 2019, 11, 334. [Google Scholar] [CrossRef]
- Elbadri, M.; Ahmed, S.A.; Abdalla, Y.T.; Hdidi, W. A New Solution of Time-Fractional Coupled KdV Equation by Using Natural Decomposition Method. Abstr. Appl. Anal. 2020, 2020, 3950816. [Google Scholar] [CrossRef]
- Khan, H.; Shah, R.; Kumam, P.; Arif, M. Analytical Solutions of Fractional-Order Heat and Wave Equations by the Natural Transform Decomposition Method. Entropy 2019, 21, 597. [Google Scholar] [CrossRef]
- Elbadri, M. The Natural Transform Decomposition Method for Solving Fractional Klein-Gordon Equation. Appl. Math. 2023, 14, 230–243. [Google Scholar] [CrossRef]
- Eltayeb, H. Application of Double Natural Decomposition Method for Solving Singular One Dimensional Boussinesq Equation. Filomat 2018, 32, 4389–4401. [Google Scholar] [CrossRef]
- Bateman, H. Some recent researches on the motion of fluids. Mon. Weath. Rev. 1915, 43, 163–170. [Google Scholar] [CrossRef]
- Burger, J.M. A mathematical model illustrating the theory of turbulence. Adv. Appl. Mech. 1948, 1, 171–199. [Google Scholar] [CrossRef]
- Cole, J.D. On a quasilinear parabolic equations occurring in aerodynamics. Quart. Appl. Math. 1951, 9, 225–236. [Google Scholar] [CrossRef]
- Wazwaz, A.M. Partial Differential Equations: Methods and Applications; CRC Press: Boca Raton, FL, USA, 2002; p. 474. [Google Scholar]
- Moslem, W.M.; Sabry, R. Zakharov-Kuznetsov-Burgers’ equation for dust ion acoustic waves. Chaos Solitons Fractals 2008, 36, 628–634. [Google Scholar] [CrossRef]
- Benton, E.R.; Platzman, G.W. A table of solutions of the one-dimensional Burgers equation. Q. Appl. Math. 1972, 30, 195–212. [Google Scholar] [CrossRef]
- Soliman, A.A. The modified extended tanh-function method for solving Burgers’-type equations. Phys. A Stat. Mech. Appl. 2006, 361, 394–404. [Google Scholar] [CrossRef]
- Biazar, J.; Ghazvini, H. Exact solutions for nonlinear Burgers’ equation by homotopy perturbation method. Numer. Methods Partial Differ. Equ. 2009, 25, 833–842. [Google Scholar] [CrossRef]
- Majeed, A.; Kamran, M.; Iqbal, M.K.; Baleanu, D. Solving time fractional Burgers and Fisher’s equations using cubic B-spline approximation method. Adv. Differ. Equ. 2020, 2020, 175. [Google Scholar] [CrossRef]
- Singh, J.; Kumar, D.; Qurashi, M.A.; Baleanu, D. Analysis of a New Frac- tional Model for Damped Bergers’ Equation. Open Phys. 2017, 15, 35–41. [Google Scholar] [CrossRef]
- Peng, X.; Xu, D.; Qiu, W. Pointwise error estimates of compact difference scheme for mixed-type time-fractional Burgers’ equation. Math. Comput. Simul. 2023, 208, 702–726. [Google Scholar] [CrossRef]
- Qiu, W.; Chen, H.; Zheng, X. An implicit difference scheme and algorithm implementation for the one-dimensional time-fractional Burgers equations. Math. Comput. Simul. 2019, 166, 298–314. [Google Scholar] [CrossRef]
- Johnston, A.J.; Jafari, H.; Moshokoa, S.P.; Ariyan, V.M.; Baleanu, D. Laplace homotopy perturbation method for Burgers’ equation with space- and time-fractional order. Open Phys. 2016, 14, 247–252. [Google Scholar] [CrossRef]
- Kaya, D. An explicit solution of coupled viscous Burgers’ equation by the decomposition method. Int. J. Math. Math. Sci. 2001, 27, 675–680. [Google Scholar] [CrossRef]
- Aminikhah, H. An analytical approximation for coupled viscous Burgers’ equation. Appl. Math. Model. 2013, 37, 5979–5983. [Google Scholar] [CrossRef]
- Shokhanda, R.; Goswami, P.; He, J.H.; Althobaiti, A. An Approximate Solution of the Time-Fractional Two-Mode Coupled Burgers’ Equation. Fractal Fract. 2021, 5, 196. [Google Scholar] [CrossRef]
- Agheli, B.; Darzi, R. Analysis of solution for system of nonlinear fractional Burger differential equations based on multiple fractional power series. Alex. Eng. J. 2017, 56, 271–276. [Google Scholar] [CrossRef]
- Zhu, H.; Shu, H.; Ding, M. Numerical solutions of two-dimensional Burgers’ equations by discrete Adomian decomposition method. Comput. Math. Appl. 2010, 60, 840–848. [Google Scholar] [CrossRef]
- Khan, M. A novel solution technique for two dimensional Burgers’ equation. Alex. Eng. J. 2014, 53, 485–490. [Google Scholar] [CrossRef]
- Eltayeb, H.; Bahar, I. A note on singular two-dimensional fractional coupled Burgers’ equation and triple Laplace Adomian decomposition method. Bound. Value Probl. 2020, 2020, 129. [Google Scholar] [CrossRef]
- Eltayeb, H.; Mesloub, S. Application of Multi-Dimensional of Conformable Sumudu Decomposition Method for Solving Conformable Singular Fractional Coupled Burger’s Equation. Acta Math. Sci. 2021, 41, 1679–1698. [Google Scholar] [CrossRef]
- Srivastava, V.K.; Ashutosh, M.T. Generating Exact Solution of Three-Dimensional Coupled Unsteady Nonlinear Generalized Viscous Burgers’ Equations. Int. J. Math. Sci. 2013, 5, 1–13. [Google Scholar]
- Alhendi, F.; Alderremy, A. Numerical solutions of three-dimensional coupled Burgers’ equations by using some numerical methods. J. Appl. Math. Phys. 2016, 4, 2011–2030. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Luo, M.; Raina, R.K. A new integral transform and its applications. Acta Math. Sci. 2015, 35, 1386–1400. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alsaud, H.; Eltayeb, H. The Four-Dimensional Natural Transform Adomian Decomposition Method and (3+1)-Dimensional Fractional Coupled Burgers’ Equation. Fractal Fract. 2024, 8, 227. https://doi.org/10.3390/fractalfract8040227
Alsaud H, Eltayeb H. The Four-Dimensional Natural Transform Adomian Decomposition Method and (3+1)-Dimensional Fractional Coupled Burgers’ Equation. Fractal and Fractional. 2024; 8(4):227. https://doi.org/10.3390/fractalfract8040227
Chicago/Turabian StyleAlsaud, Huda, and Hassan Eltayeb. 2024. "The Four-Dimensional Natural Transform Adomian Decomposition Method and (3+1)-Dimensional Fractional Coupled Burgers’ Equation" Fractal and Fractional 8, no. 4: 227. https://doi.org/10.3390/fractalfract8040227
APA StyleAlsaud, H., & Eltayeb, H. (2024). The Four-Dimensional Natural Transform Adomian Decomposition Method and (3+1)-Dimensional Fractional Coupled Burgers’ Equation. Fractal and Fractional, 8(4), 227. https://doi.org/10.3390/fractalfract8040227





