Novel Hopf Bifurcation Exploration and Control Strategies in the Fractional-Order FitzHugh–Nagumo Neural Model Incorporating Delay
Abstract
1. Introduction
2. Preliminaries
3. Bifurcation Issue
- (i)
- In view of (21), we obtain the following:
- (ii)
4. Bifurcation Control via the Controller
- (i)
- In view of (49), we obtain the following:
- (ii)
5. Bifurcation Control via Hybrid Controller
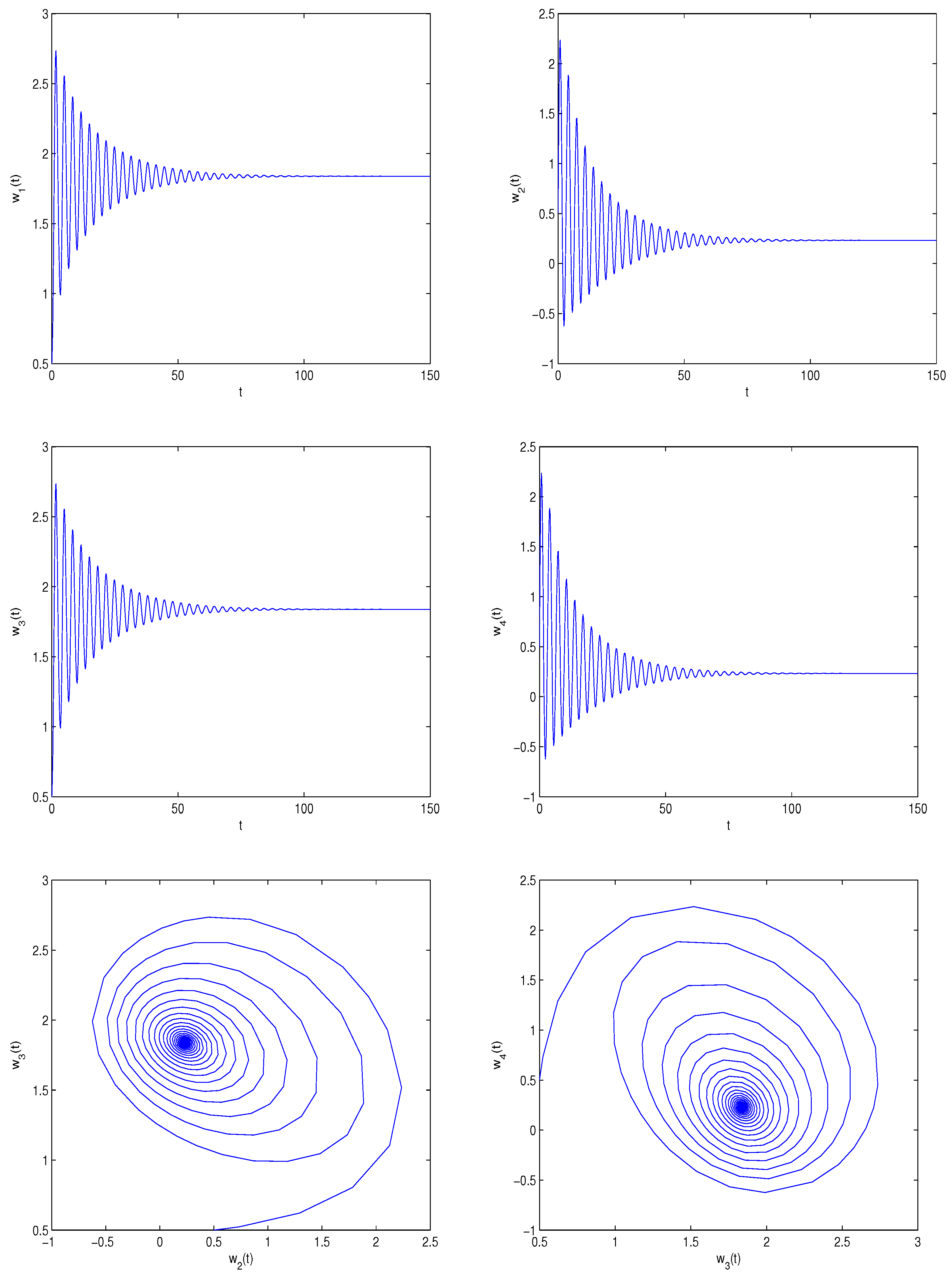
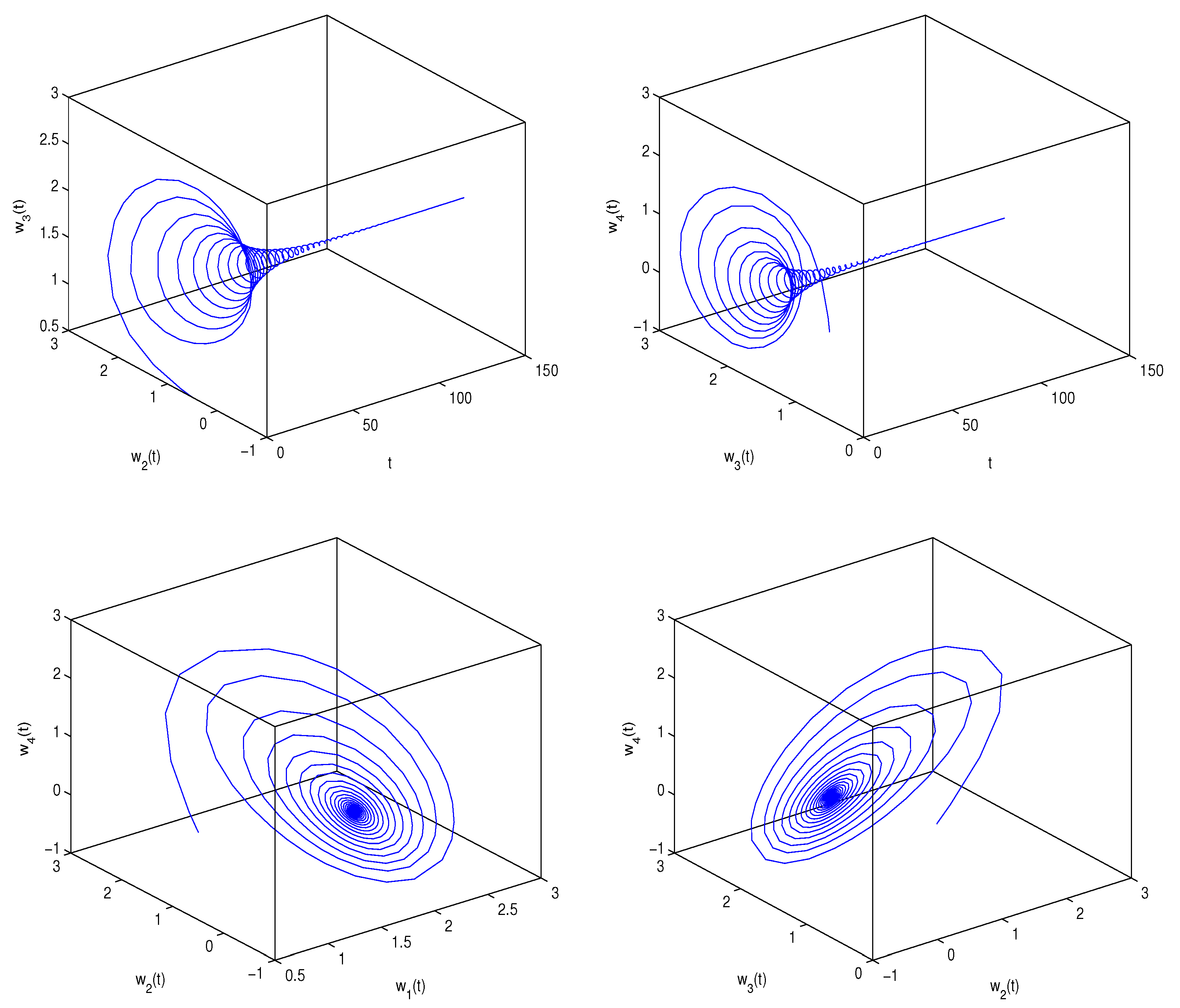
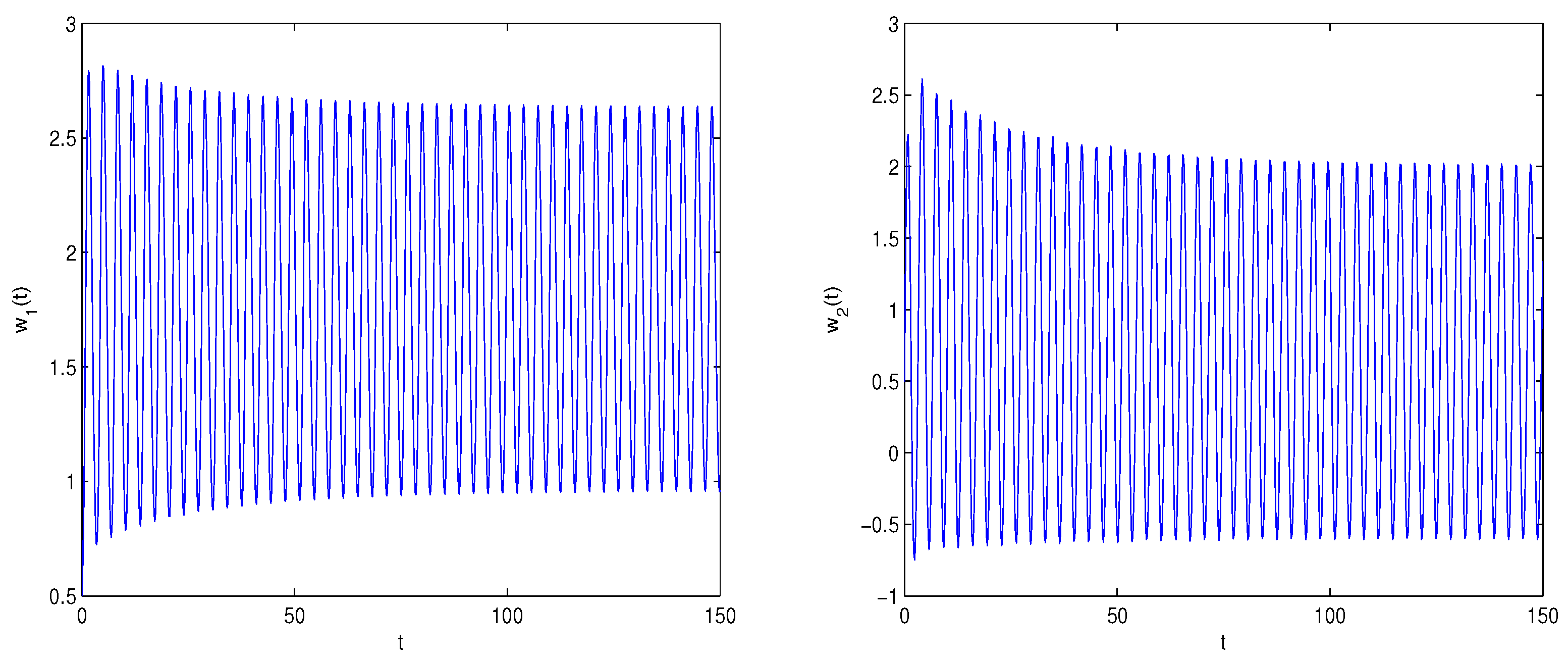
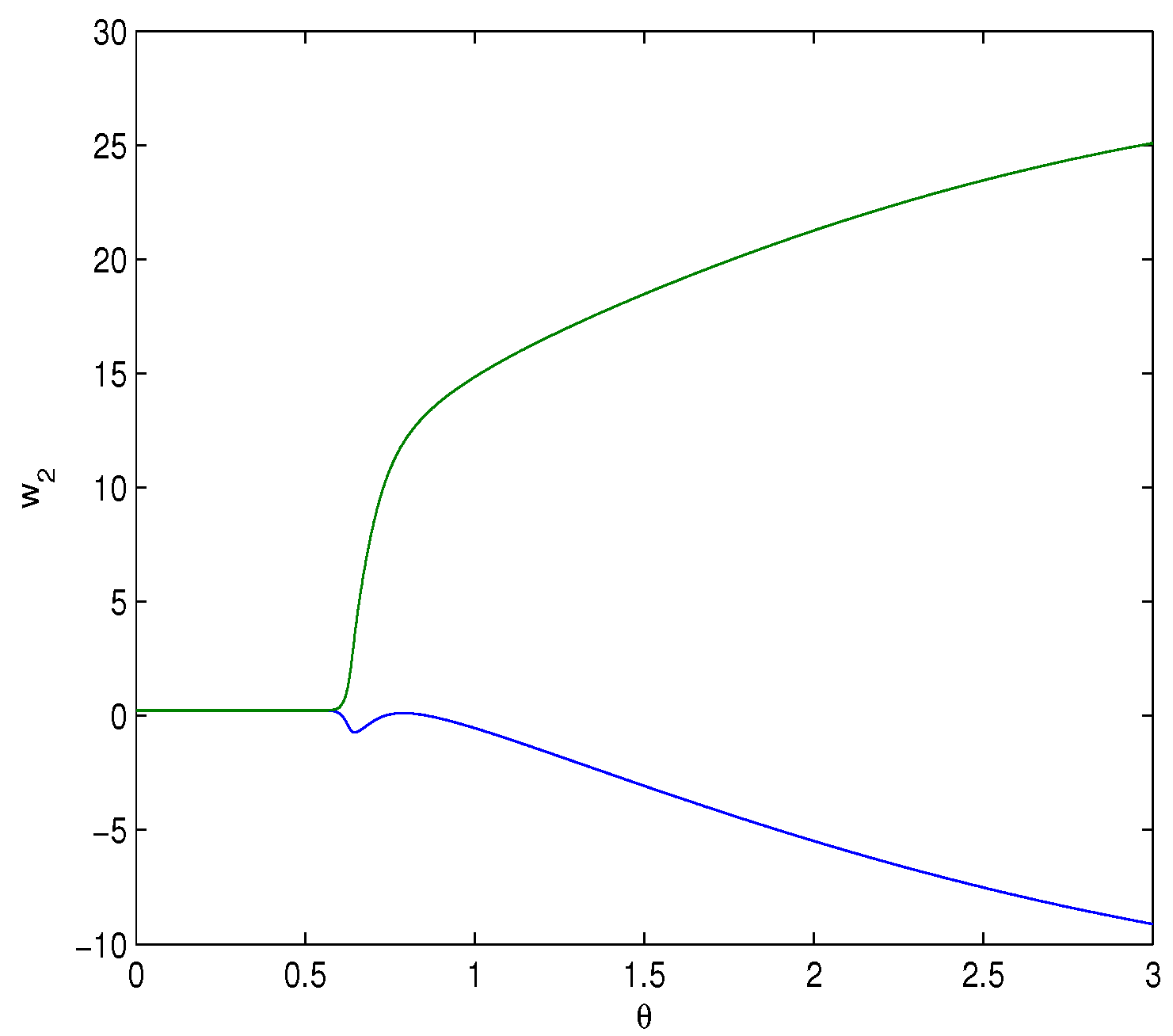
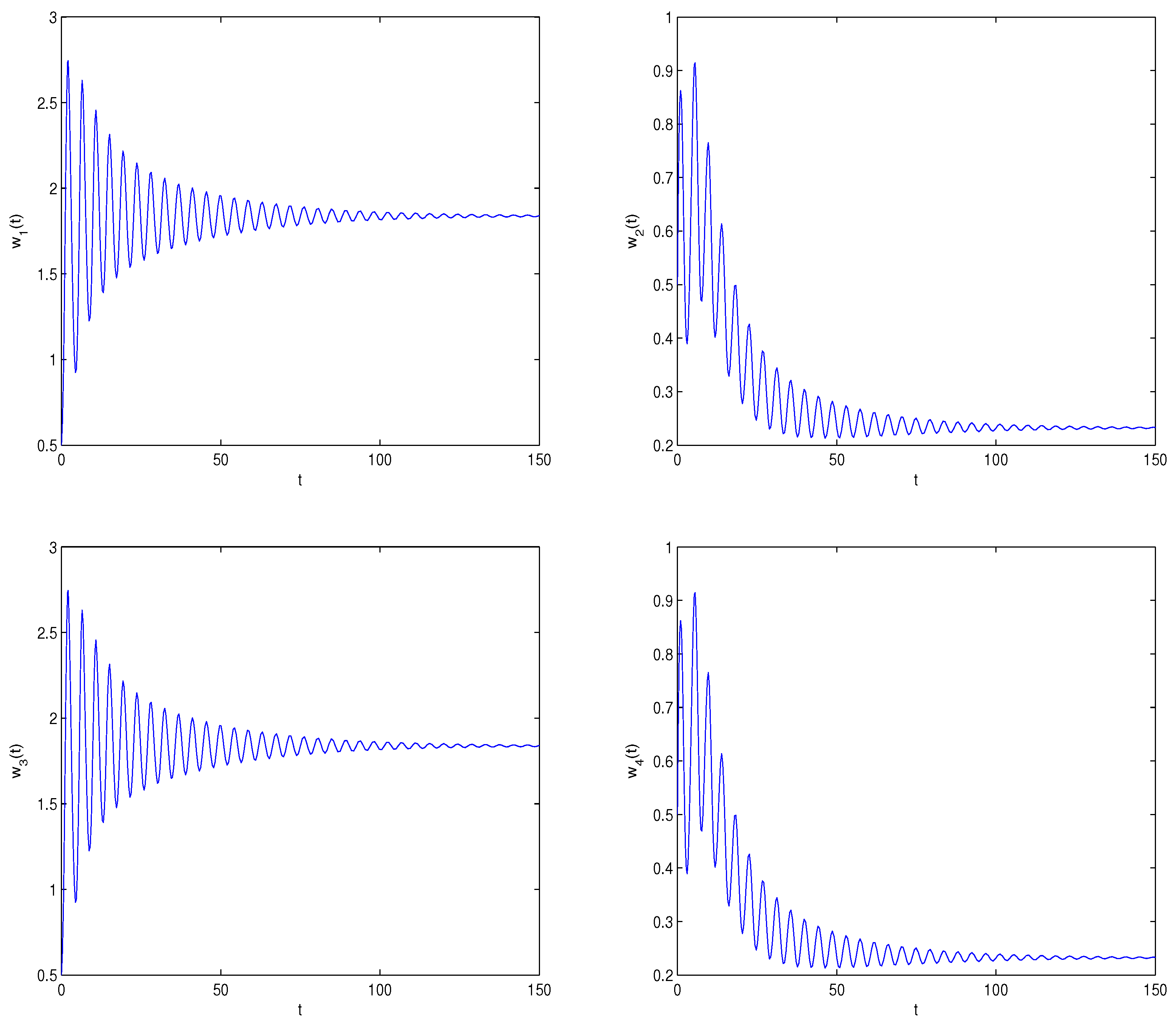
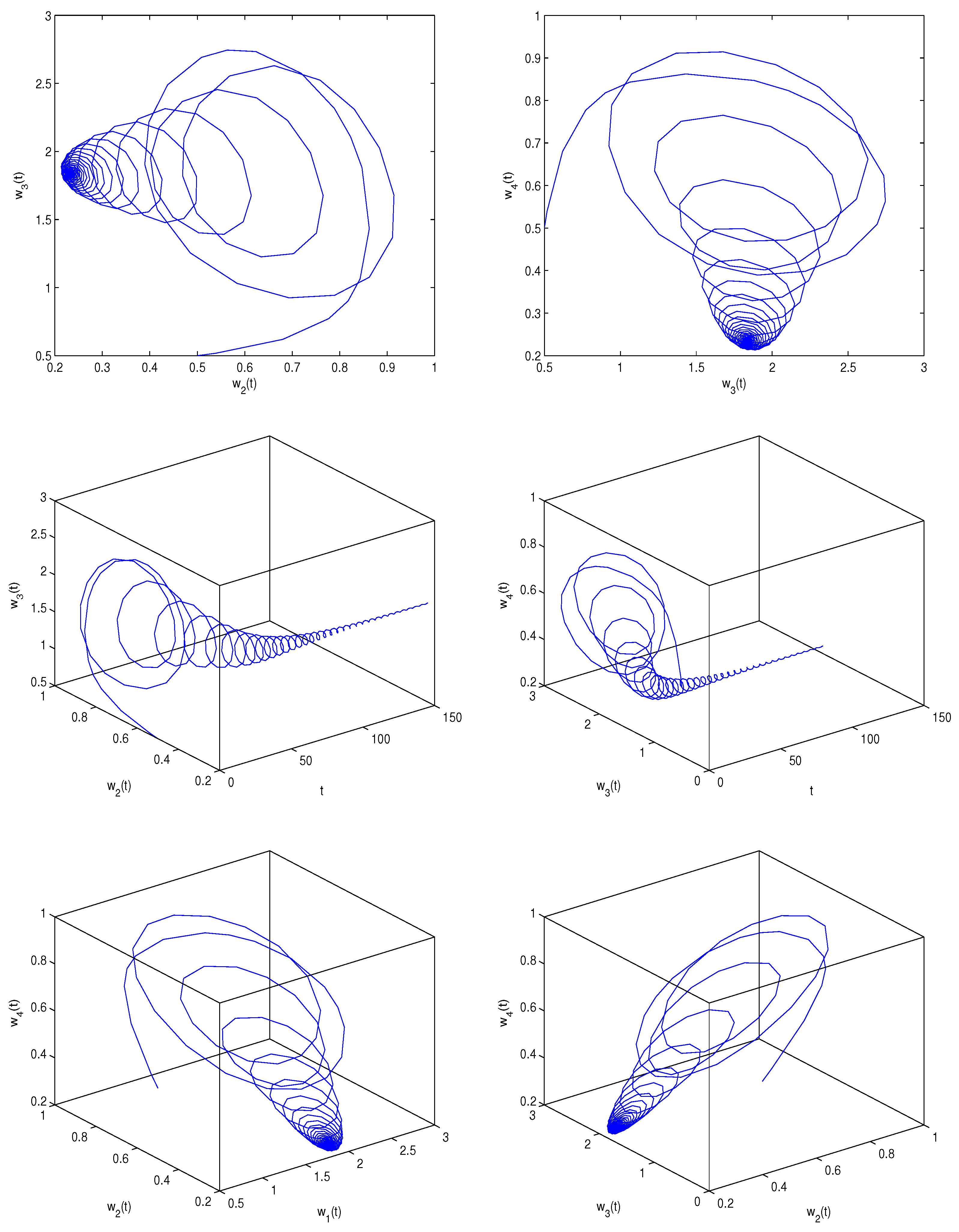
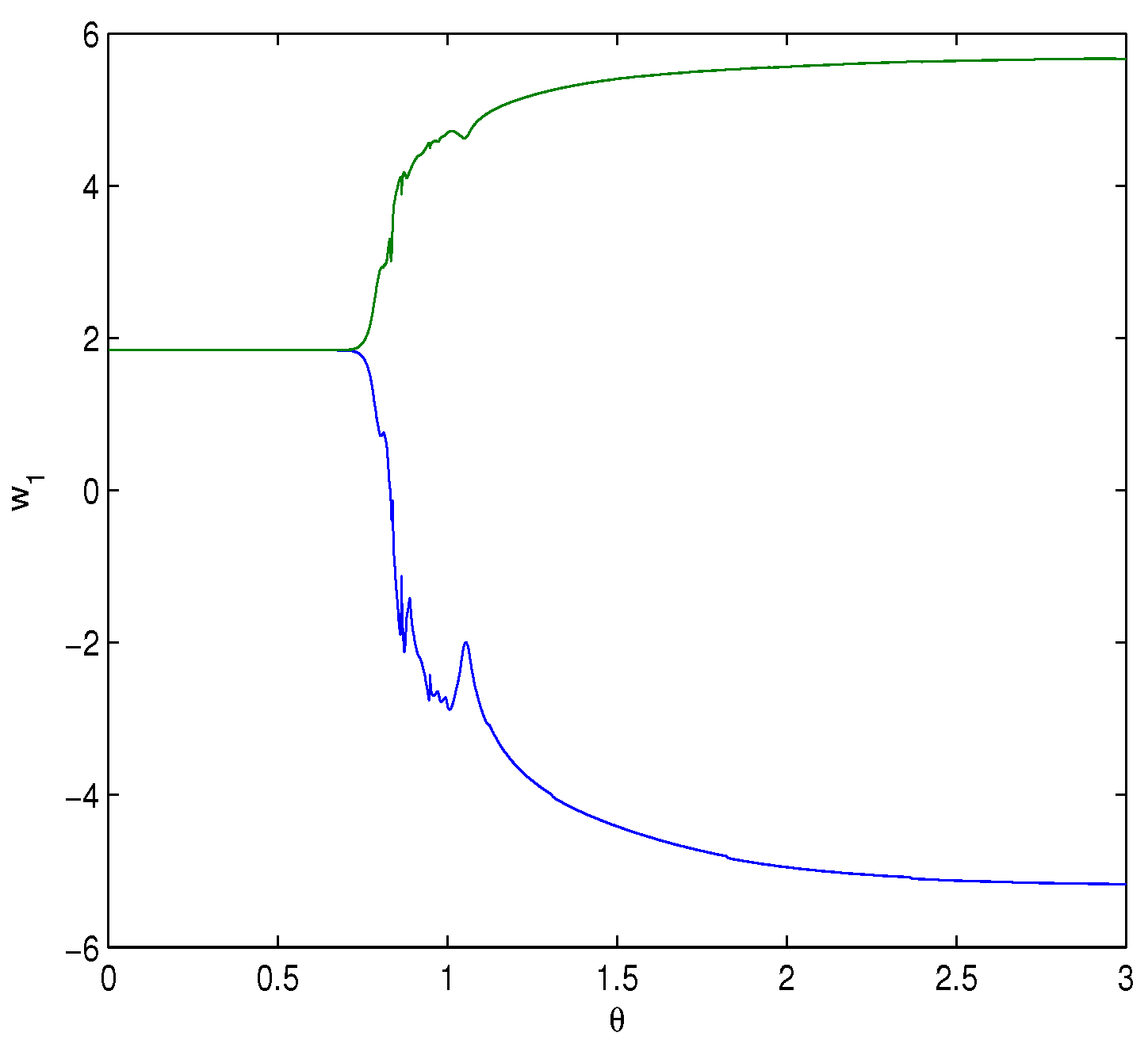
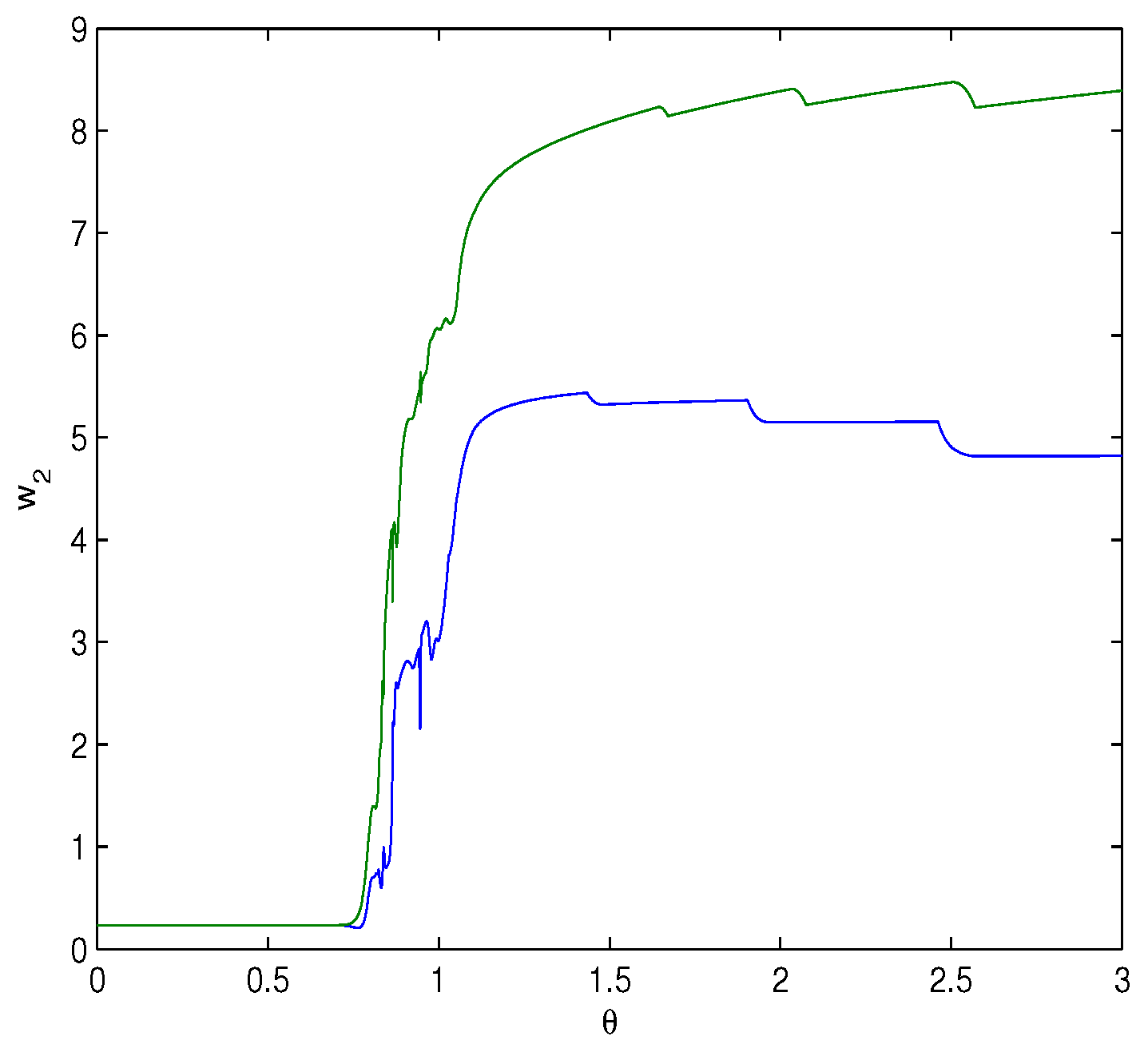
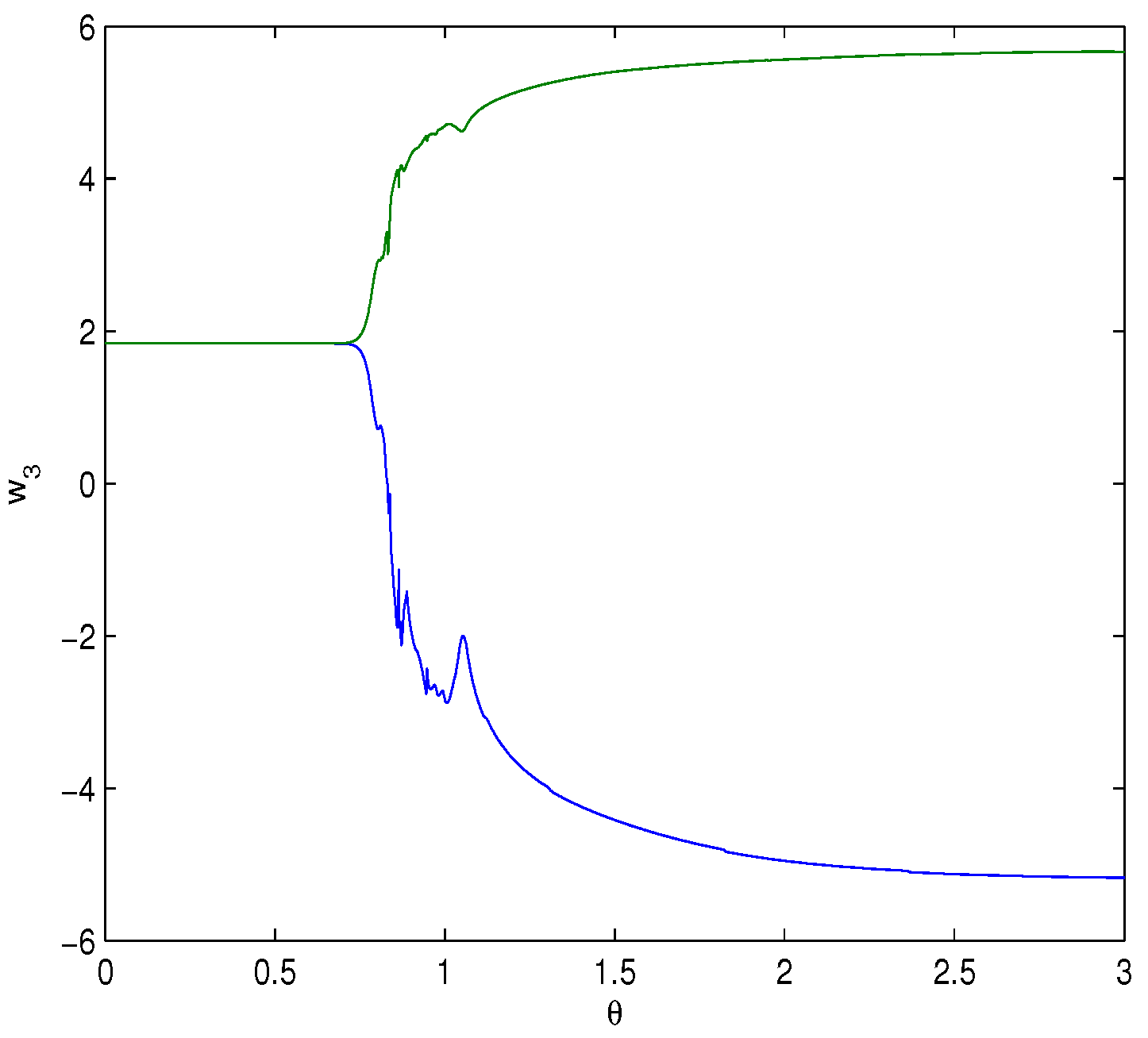
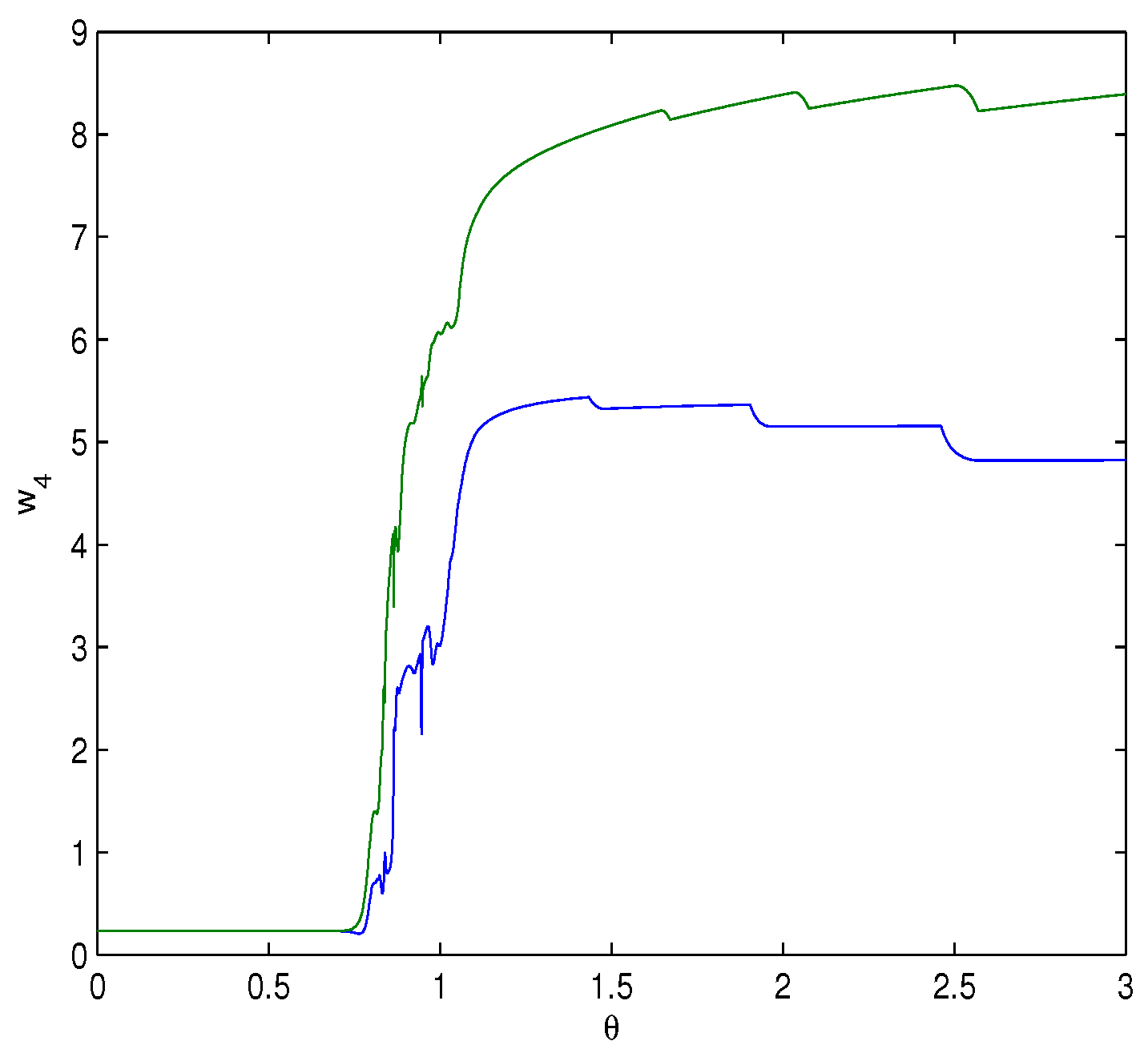
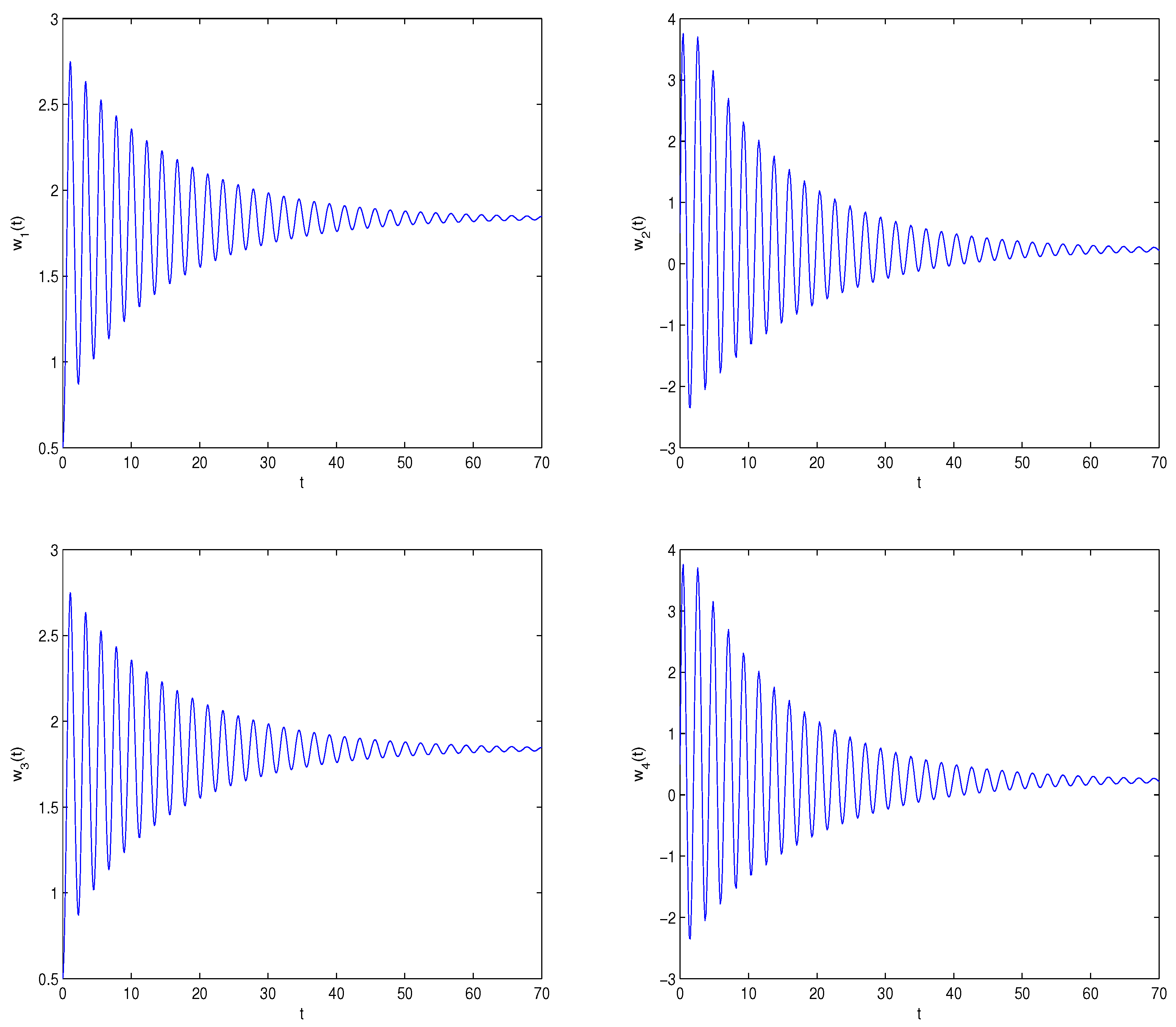
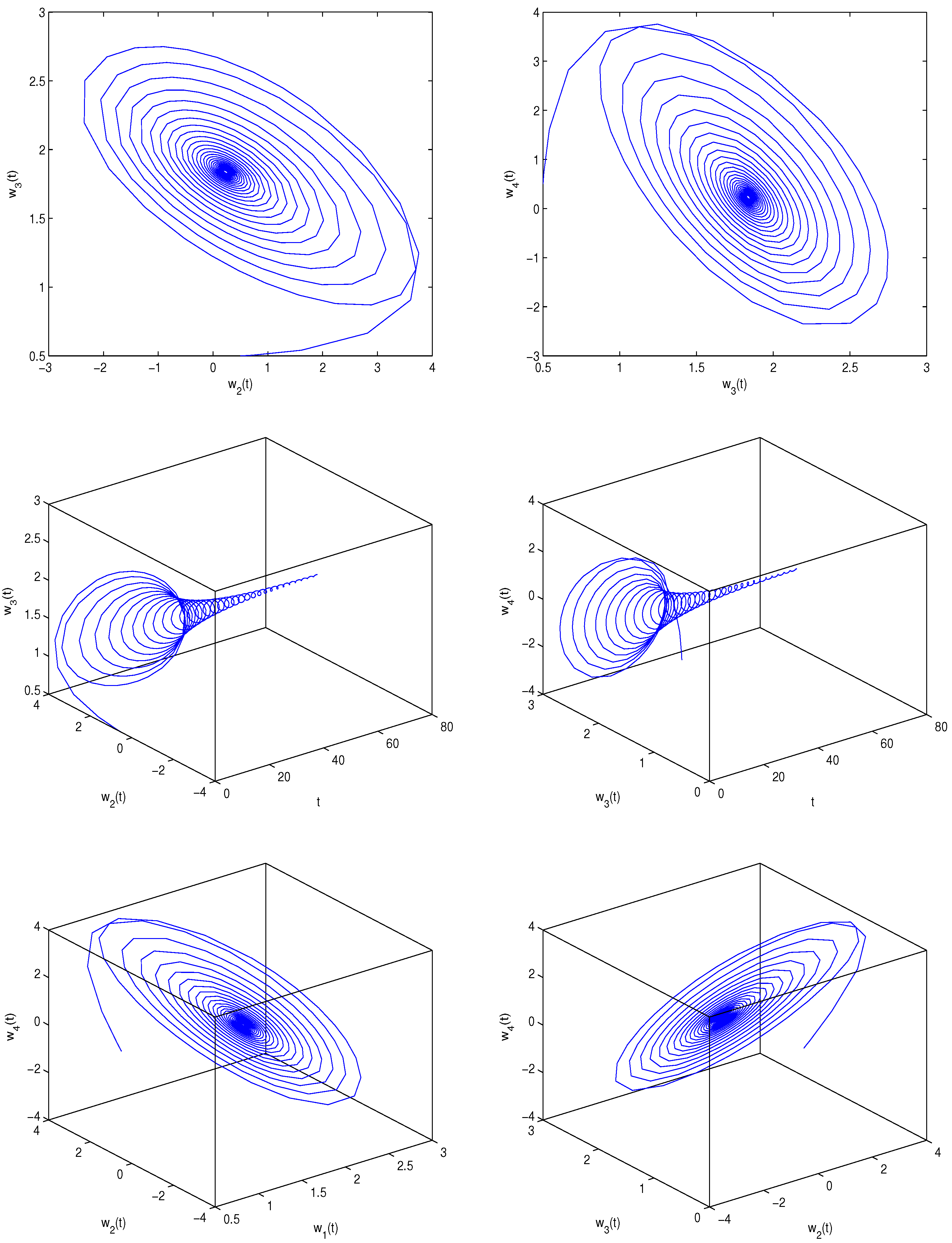
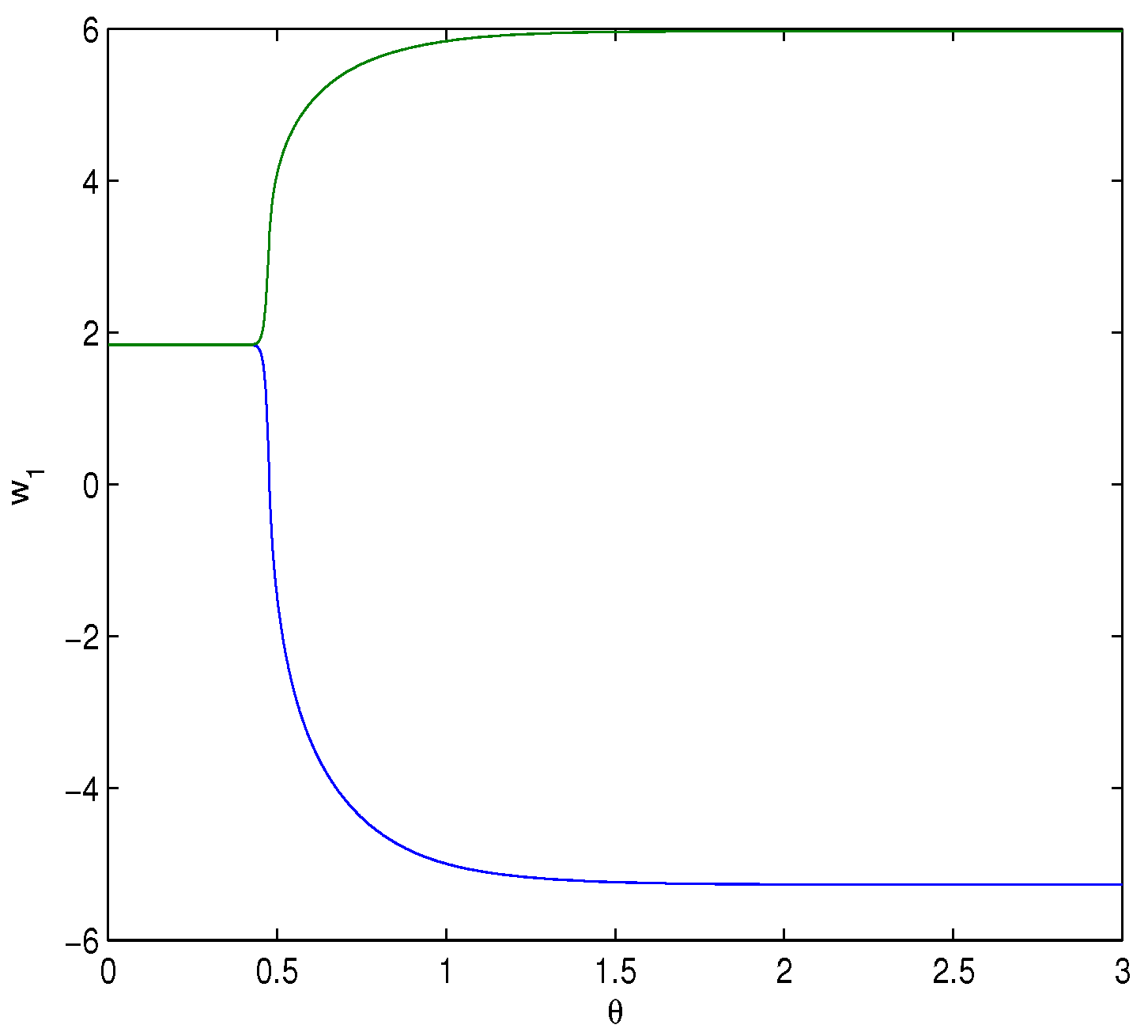
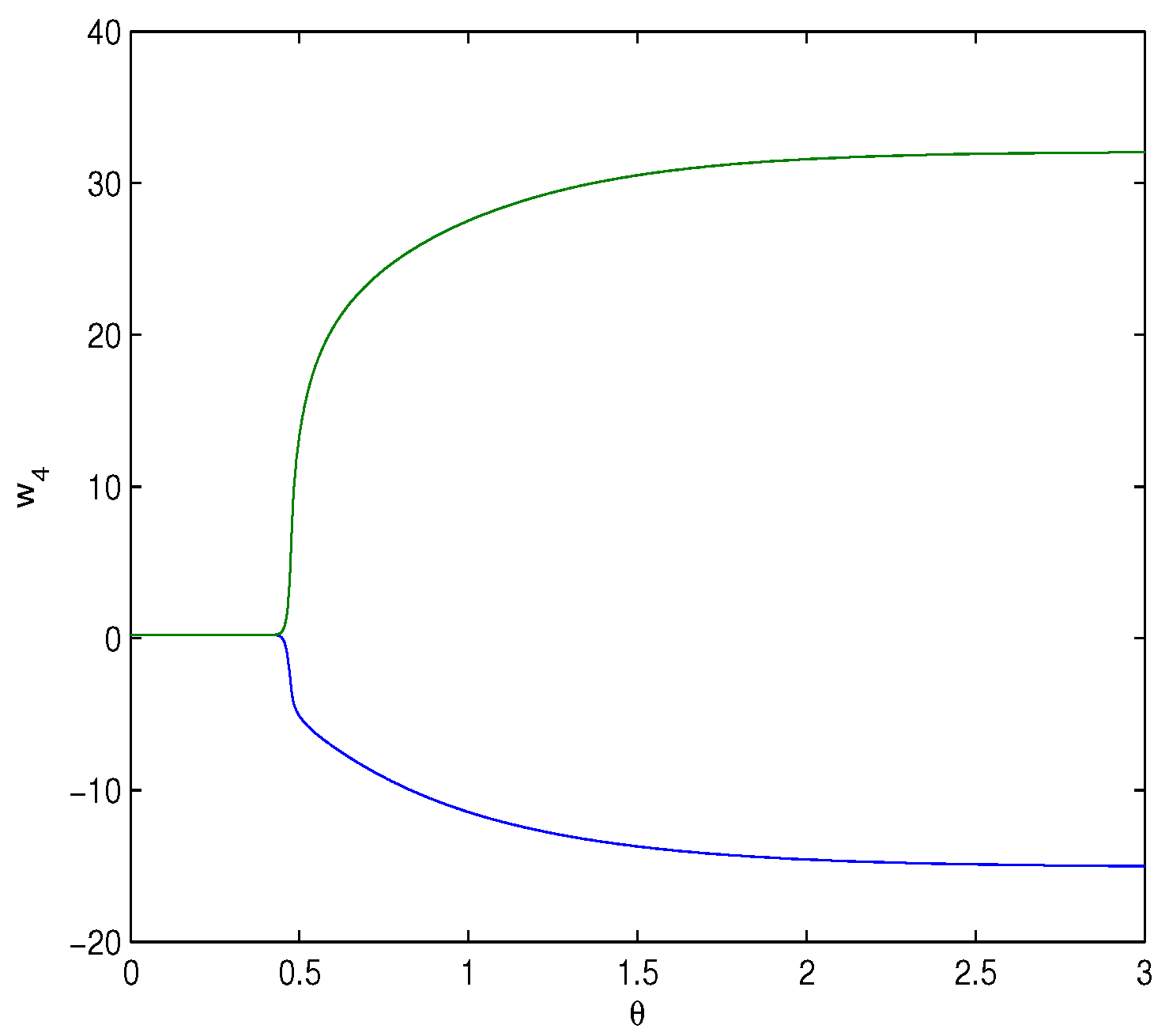
6. Simulation Outcomes
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Goetze, F.; Lai, P.Y. Dynamics of synaptically coupled FitzHugh-Nagumo neurons. Chin. J. Phys. 2022, 77, 1365–1380. [Google Scholar] [CrossRef]
- Hodgkin, A.L.; Huxley, A.F. A quantitative description of membrane current and its application to conduction and excitation in nerve. J. Physiol. 1952, 117, 500–544. [Google Scholar] [CrossRef] [PubMed]
- Morris, C.; Lecar, H. Voltage oscillations in the barnacle giant muscle fiber. Biophys. J. 1981, 35, 193–213. [Google Scholar] [CrossRef] [PubMed]
- Ermentrout, G.B.; Kopell, N. Parabolic bursting in an excitable system coupled with a slow oscillation. SIAM J. Appl. Math. 1986, 46, 233–253. [Google Scholar] [CrossRef]
- FitzHugh, R. Impulses and physiological states in theoretical models of nerve membrane. Biophys. J. 1961, 1, 445–466. [Google Scholar] [CrossRef] [PubMed]
- Touboul, J.; Brette, R. Spiking dynamics of bidimensional integrate-and-fire neurons. SIAM J. Appl. Dyn. Syst. 2009, 8, 1462–1506. [Google Scholar] [CrossRef]
- Ermentrout, B. Type I membranes, phase resetting curves, and synchrony. Neural Comput. 1996, 8, 979–1001. [Google Scholar] [CrossRef]
- Zeng, C.H.; Zeng, C.P.; Gong, A.L.; Nie, L.R. Effect of time delay in FitzHugh-Nagumo neural model with correlations between multiplicative and additive noises. Physica A 2010, 389, 5117–5127. [Google Scholar] [CrossRef]
- Demina, M.V.; Kudryashov, N.A. Meromorphic solutions in the FitzHugh-Nagumo model. Appl. Math. Lett. 2018, 82, 18–23. [Google Scholar] [CrossRef]
- He, Z.L.; Li, C.D.; Chen, L.; Cao, Z.R. Dynamic behaviors of the FitzHugh-Nagumo neuron model with state-dependent impulsive effects. Neural Netw. 2020, 121, 497–511. [Google Scholar] [CrossRef]
- Zheng, Q.Q.; Shen, J.W. Turing instability induced by random network in FitzHugh-Nagumo model. Appl. Math. Comput. 2020, 381, 125304. [Google Scholar] [CrossRef]
- Gani, M.O.; Ogawa, T. Instability of periodic traveling wave solutions in a modified FitzHugh-Nagumo model for excitable media. Appl. Math. Comput. 2015, 256, 968–984. [Google Scholar] [CrossRef]
- Buckwar, E.; Samson, A.; Tamborrino, M.; Tubikanec, I. A splitting method for SDEs with locally Lipschitz drift: Illustration on the FitzHugh-Nagumo model. Appl. Numer. Math. 2022, 179, 191–220. [Google Scholar] [CrossRef]
- Chen, L.; Campbell, S.A. Hysteresis bifurcation and application to delayed FitzHugh-Nagumo neural systems. J. Math. Anal. Appl. 2021, 500, 125151. [Google Scholar] [CrossRef]
- Ciszak, M.; Balle, S.; Piro, O.; Marino, F. Intermittent chaotic spiking in the van der Pol-FitzHugh-Nagumo system with inertia. Chaos Solitons Fractals 2023, 167, 113053. [Google Scholar] [CrossRef]
- Njitacke, Z.T.; Takembo, C.N.; Awrejcewicz, J.; Fouda, H.P.E.; Kengne, J. Hamilton energy, complex dynamical analysis and information patterns of a new memristive FitzHugh-Nagumo neural network. Chaos Solitons Fractals 2022, 160, 112211. [Google Scholar] [CrossRef]
- Achouri, H.; Aouiti, C.; Hamed, B.B. Codimension two bifurcation in a coupled FitzHugh-Nagumo system with multiple delays. Chaos Solitons Fractals 2022, 156, 111824. [Google Scholar] [CrossRef]
- Hoff, A.; Santos, J.V.d.; Manchein, C.; Albuquerque, H.A. Numerical bifurcation analysis of two coupled FitzHugh-Nagumo oscillators. Eur. Phys. J. 2014, 87, 87–151. [Google Scholar] [CrossRef]
- Gan, C.B.; Perc, M.; Wang, Q.Y. Delay-aided stochastic multiresonances on scale-free FitzHugh Nagumo neuronal networks. Chin. Phys. 2010, 19, 040508. [Google Scholar]
- Jia, J.Y.; Liu, H.H.; Xu, C.L.; Yan, F. Dynamic effects of time delay on a coupled FitzHugh-Nagumo neural system. Alex. Eng. J. 2015, 54, 241–250. [Google Scholar] [CrossRef]
- Petras, I. Fractional-Order Nonlinear Systems; Springer: London, UK, 2011. [Google Scholar]
- Xu, C.J.; Liao, M.X.; Li, P.L.; Guo, Y.; Liu, Z.X. Bifurcation properties for fractional order delayed BAM neural networks. Cogn. Comput. 2021, 13, 322–356. [Google Scholar] [CrossRef]
- Xu, C.J.; Mu, D.; Liu, Z.X.; Pang, Y.C.; Liao, M.X.; Li, P.L.; Yao, L.Y.; Qin, Q.W. Comparative exploration on bifurcation behavior for integer-order and fractional-order delayed BAM neural networks. Nonlinear Anal. Model. Control 2022, 27, 1030–1053. [Google Scholar] [CrossRef]
- Li, P.L.; Gao, R.; Xu, C.J.; Shen, J.W.; Ahmad, S.; Li, Y. Exploring the impact of delay on Hopf bifurcation of a type of BAM neural network models concerning three nonidentical delays. Neural Process. Lett. 2023, 55, 5905–5921. [Google Scholar] [CrossRef]
- Xu, C.J.; Zhao, Y.Y.; Lin, J.T.; Pang, Y.C.; Liu, Z.X.; Shen, J.W.; Qin, Y.X.; Farman, M.; Ahmad, S. Mathematical exploration on control of bifurcation for a plankton-oxygen dynamical model owning delay. J. Math. Chem. 2023. [Google Scholar] [CrossRef]
- Ou, W.; Xu, C.J.; Cui, Q.Y.; Pang, Y.C.; Liu, Z.X.; Shen, J.W.; Baber, M.Z.; Farman, M.; Ahmad, S. Hopf bifurcation exploration and control technique in a predator-prey system incorporating delay. Aims Math. 2023, 9, 1622–1651. [Google Scholar] [CrossRef]
- Cui, C.Q.; Xu, C.J.; Ou, W.; Pang, Y.C.; Liu, Z.X.; Li, P.L.; Yao, L.Y. Bifurcation behavior and hybrid controller design of a 2D Lotka-Volterra commensal symbiosis system accompanying delay. Mathematics 2023, 11, 4808. [Google Scholar] [CrossRef]
- Maharajan, C.; Sowmiya, C.; Xu, C.J. Fractional order uncertain BAM neural networks with mixed time delays: An existence and Quasi-uniform stability analysis. J. Intell. Fuzzy Syst. 2023, 46, 4291–4313. [Google Scholar] [CrossRef]
- Xiao, J.Y.; Wen, S.P.; Yang, X.J.; Zhong, S.M. New approach to global Mittag-Leffler synchronization problem of fractional-order quaternion-valued BAM neural networks based on a new inequality. Neural Netw. 2020, 122, 320–337. [Google Scholar] [CrossRef]
- Xu, C.J.; Rahman, M.; Baleanu, D. On fractional-order symmetric oscillator with offset-boosting control. Nonlinear Anal. Control 2022, 27, 994–1008. [Google Scholar] [CrossRef]
- Li, N.; Yan, M.T. Bifurcation control of a delayed fractional-order prey-predator model with cannibalism and disease. Phys. Stat. Mech. Its Appl. 2022, 600, 127600. [Google Scholar] [CrossRef]
- Kao, Y.G.; Li, H. Asymptotic multistability and local S-asymptotic ω-periodicity for the nonautonomous fractional-order neural networks with impulses. Sci. China Inf. Sci. 2021, 64, 112207. [Google Scholar] [CrossRef]
- Luo, R.Z.; Liu, S.; Song, Z.J.; Zhang, F. Fixed-time control of a class of fractional-order chaotic systems via backstepping method. Chaos Solitons Fractals 2023, 167, 113076. [Google Scholar] [CrossRef]
- Jin, X.C.; Lu, J.G.; Zhang, Q.H. Delay-dependent and order-dependent conditions for stability and stabilization of fractional-order memristive neural networks with time-varying delays. Neurocomputing 2023, 522, 53–63. [Google Scholar] [CrossRef]
- Hao, Y.J.; Zhang, M.H.; Cui, Y.H.; Cheng, G.; Xie, J.Q.; Chen, Y.M. Dynamic analysis of variable fractional order cantilever beam based on shifted Legendre polynomials algorithm. J. Comput. Appl. Math. 2023, 423, 114952. [Google Scholar] [CrossRef]
- Hioual, A.; Ouannas, A.; Grassi, G.; Oussaeif, T.E. Nonlinear nabla variable-order fractional discrete systems: Asymptotic stability and application to neural networks. J. Comput. Appl. Math. 2023, 423, 114939. [Google Scholar] [CrossRef]
- Huang, C.D.; Nie, X.B.; Zhao, X.; Song, Q.K.; Tu, Z.W.; Xiao, M.; Cao, J.D. Novel bifurcation results for a delayed fractional-order quaternion-valued neural network. Neural Netw. 2019, 117, 67–93. [Google Scholar] [CrossRef] [PubMed]
- Ali, M.S.; Narayanan, G.; Sevgen, S.; Shekher, V.; Arik, S. Global stability analysis of fractional-order fuzzy BAM neural networks with time delay and impulsive effects. Commun. Nonlinear Sci. Numer. Simul. 2019, 78, 104853. [Google Scholar]
- Arslan, E.; Narayanan, G.; Ali, M.S.; Arik, S.; Saroha, S. Controller design for finite-time and fixed-time stabilization of fractional-order memristive complex-valued BAM neural networks with uncertain parameters and time-varying delays. Neural Networks 2020, 130, 60–74. [Google Scholar] [CrossRef] [PubMed]
- Amine, S.; Hajri, Y.; Allali, K. A delayed fractional-order tumor virotherapy model: Stability and Hopf bifurcation. Chaos Solitons Fractals 2022, 161, 112396. [Google Scholar] [CrossRef]
- Xu, C.J.; Mu, D.; Liu, Z.X.; Pang, Y.C.; Liao, M.X.; Aouiti, C. New insight into bifurcation of fractional-order 4D neural networks incorporating two different time delays. Commun. Nonlinear Sci. Numer. Simul. 2023, 118, 107043. [Google Scholar] [CrossRef]
- Huang, C.D.; Cao, J.D.; Xiao, M.; Alsaedi, A.; Hayat, T. Effects of time delays on stability and Hopf bifurcation in a fractional ring-structured network with arbitrary neurons. Commun. Nonlinear Sci. Numer. Simul. 2018, 57, 1–13. [Google Scholar] [CrossRef]
- Alidousti, J. Stability and bifurcation analysis for a fractional prey-predator scavenger model. Appl. Math. Model. 2020, 81, 342–355. [Google Scholar] [CrossRef]
- Xu, C.J.; Liao, M.X.; Li, P.L.; Yao, L.Y.; Qin, Q.W.; Shang, Y.L. Chaos control for a fractional-order Jerk system via time delay feedback controller and mixed controller. Fractal Fract. 2021, 5, 257. [Google Scholar] [CrossRef]
- Eshaghi, S.; Ghaziani, R.K.; Ansari, A. Hopf bifurcation, chaos control and synchronization of a chaotic fractional-order system with chaos entanglement function. Math. Comput. Simul. 2020, 172, 321–340. [Google Scholar] [CrossRef]
- Zhao, L.Z.; Huang, C.D.; Cao, J.D. Effects of double delays on bifurcation for a fractional-order neural network. Cogn. Neurodyn. 2022, 16, 1189–1201. [Google Scholar] [CrossRef] [PubMed]
- Fleitas, A.; Méndez, J.A.; Nápoles, J.E.; Sigarreta, J.M. On fractional Lienard-type systems. Rev. Mex. Física 2019, 65, 618–625. [Google Scholar] [CrossRef]
- Tang, Y.H.; Xiao, M.; Jiang, G.P.; Lin, J.X.; Cao, J.D.; Zheng, W.X. Fractional-order PD control at Hopf bifurcations in a fractional-ordr congestion control system. Nonlinear Dyn. 2007, 90, 2185–2198. [Google Scholar] [CrossRef]
- Ding, D.W.; Zhang, X.Y.; Cao, J.D.; Wang, N.A.; Liang, D. Bifurcation control of complex networks model via PD controller. Neurocomputing 2016, 175, 1–9. [Google Scholar] [CrossRef]
- Huang, C.D.; Mo, S.S.; Cao, J.D. Detections of bifurcation in a fractional-order Cohen-Grossberg neural network with multiple delays. Cogn. Neurodyn. 2023, 1–18. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations; Academic Press: New York, NY, USA, 1999. [Google Scholar]
- Bandyopadhyay, B.; Kamal, S. Stabliization and Control of Fractional Order Systems: A Sliding Mode Approach; Springer: Heidelberg, Germany, 2015; Volume 317. [Google Scholar]
- Matignon, D. Stability results for fractional differential equations with applications to control processing. Comput. Eng. Syst. Appl. 1996, 2, 963–968. [Google Scholar]
- Wang, X.H.; Wang, Z.; Xia, J.W. Stability and bifurcation control of a delayed fractional-order eco-epidemiological model with incommensurate orders. J. Frankl. Inst. 2019, 356, 8278–8295. [Google Scholar] [CrossRef]
- Deng, W.H.; Li, C.P.; Lü, J.H. Stability analysis of linear fractional differential system with multiple time delays. Nonlinear Dyn. 2007, 48, 409–416. [Google Scholar] [CrossRef]
- Zhang, Z.Z.; Yang, H.Z. Hybrid control of Hopf bifurcation in a two prey one predator system with time delay. In Proceedings of the 33rd Chinese Control Conference, Nanjing, China, 28–30 July 2014; pp. 6895–6900. [Google Scholar]
- Zhang, L.P.; Wang, H.N.; Xu, M. Hybrid control of bifurcation in a predator-prey system with three delays. Acta Phys. Sin. 2011, 60, 010506. [Google Scholar] [CrossRef]











Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Xu, C. Novel Hopf Bifurcation Exploration and Control Strategies in the Fractional-Order FitzHugh–Nagumo Neural Model Incorporating Delay. Fractal Fract. 2024, 8, 229. https://doi.org/10.3390/fractalfract8040229
Zhang Y, Xu C. Novel Hopf Bifurcation Exploration and Control Strategies in the Fractional-Order FitzHugh–Nagumo Neural Model Incorporating Delay. Fractal and Fractional. 2024; 8(4):229. https://doi.org/10.3390/fractalfract8040229
Chicago/Turabian StyleZhang, Yunzhang, and Changjin Xu. 2024. "Novel Hopf Bifurcation Exploration and Control Strategies in the Fractional-Order FitzHugh–Nagumo Neural Model Incorporating Delay" Fractal and Fractional 8, no. 4: 229. https://doi.org/10.3390/fractalfract8040229
APA StyleZhang, Y., & Xu, C. (2024). Novel Hopf Bifurcation Exploration and Control Strategies in the Fractional-Order FitzHugh–Nagumo Neural Model Incorporating Delay. Fractal and Fractional, 8(4), 229. https://doi.org/10.3390/fractalfract8040229






