On Casimir and Helmholtz Fluctuation-Induced Forces in Micro- and Nano-Systems: Survey of Some Basic Results
Abstract
:1. Introduction
2. The QED Casimir Effect
3. The Critical and Thermodynamic Casimir Effects
4. Casimir Force versus Helmholtz Force
4.1. Casimir Force and Grand Canonical Ensemble
4.2. Definition of the Thermodynamic Casimir Force
4.3. Helmholtz Force and Canonical Ensemble
4.4. On the Definition of the Helmholtz Force
5. Some Results for the Helmholtz Force versus the Casimir Force
On the Connection between the Canonical and Grand Canonical Partition Functions
6. Concluding Comments and Discussion
- Additional interest in the QED Casimir effect is being driven by certain theoretical predictions stemming from attempts to construct unified field theories of the fundamental forces. According to these predictions, Newton’s gravitational law might be modified at sub-millimeter distances. By measuring the Casimir force and comparing these data with the predictions of theory, it could be possible to obtain, inter alia, constraints for the parameters characterizing Yukawa-type deviations from Newtonian gravity, which could help to test the validity of such ideas [133,134,135].
- There has been speculation about possible relations of the Casimir effect to topics such as dark matter and cosmology [28,136,137,138,139]. These relations are linked to discussions about the physical meaning of the zero-point energy of quantum fields, the cosmological constant problem, and the physical interpretation of the Casimir effect. There is a considerable body of literature dealing with the physical source of the Casimir force. An extensive discussion of this issue can be found in [7,13], and more recently in [28,137,138,140].
- It has been suggested [141,142] that hypothetical chameleon interactions, which might explain the mechanisms behind dark energy, might be detected through high-precision force measurements. In [142,143], the authors proposed the design, fabrication, and characterization of such a force sensor for chameleon and Casimir force experiments using a parallel-plate configuration. The idea is to measure the total force between two parallel plates as a function of the density of a neutral gas allowed into the cavity. As the density of the gas increases, the mass of the chameleon field in the cavity increases, giving rise to a screening effect of the chameleon interaction.
- Regarding the description of the dielectric properties, especially for the thermal contribution to the Casimir effect, there has been discussion of this topic over the course of more than two decades [144]. We would just mention that the disagreement between theoretical predictions and precise experimental results has placed the focus on the proper account of dissipation in the description of the material optical response; in several experiments on the QED effect between metallic objects, a simple non-dissipative model has provided the best description. The same experiments appear to exclude the account of dissipation provided by the commonly used Drude model [95,145,146]. An additional issue involves the description of the role of free electrons in semiconductors [147], where attempts to date have not reached a unanimous consensus.
Funding
Conflicts of Interest
Abbreviations
| CCF | Critical Casimir Force |
| QED | Quantum Electrodynamics |
| DA | Derjaguin Approximation |
| CF | Casimir Force |
| MEMS/NEMS | Micro/Nanoelectromechanical Systems |
| CC | Critical Casimir |
| HF | Helmholtz Force |
| CCE | Critical Casimir Effect |
| FIF | Fluctuation-Induced Force |
| GCE | Grand Canonical Ensemble |
| PBC | Periodic Boundary Conditions. |
References
- Casimir, H.B. On the Attraction between Two Perfectly Conducting Plates. Proc. K. Ned. Akad. Wet. 1948, 51, 793–796. [Google Scholar]
- Milonni, P.W.; Cook, R.J.; Goggin, M.E. Radiation pressure from the vacuum: Physical interpretation of the Casimir force. Phys. Rev. A 1988, 38, 1621. [Google Scholar] [CrossRef] [PubMed]
- Plunien, G.; Müller, B.; Greiner, W. The Casimir effect. Phys. Rep. 1986, 134, 87–193. [Google Scholar] [CrossRef]
- Mostepanenko, V.M.; Trunov, N.N. The Casimir effect and its applications. Sov. Phys. Uspekhi 1988, 31, 965. [Google Scholar] [CrossRef]
- Levin, F.S.; Micha, D.A. (Eds.) Long-Range Casimir Forces; Finite Systems and Multiparticle Dynamics; Springer: Berlin/Heidelberg, Germany, 1993. [Google Scholar] [CrossRef]
- Mostepanenko, V.M.; Trunov, N.N. The Casimir Effect and Its Applications; Energoatomizdat, Moscow, 1990, in Russian; English Version; Clarendon: New York, NY, USA, 1997. [Google Scholar]
- Milonni, P.W. The Quantum Vacuum; Academic: San Diego, CA, USA, 1994. [Google Scholar]
- Kardar, M.; Golestanian, R. The “friction” of vacuum, and other fluctuation-induced forces. Rev. Mod. Phys. 1999, 71, 1233–1245. [Google Scholar] [CrossRef]
- Bordag, M. (Ed.) The Casimir Effect 50 Years Later; World Scientific: Singapore, 1999; pp. 3–9. [Google Scholar]
- Bordag, M.; Mohideen, U.; Mostepanenko, V.M. New developments in the Casimir Effect. Phys. Rep. 2001, 353, 1–205. [Google Scholar] [CrossRef]
- Milton, K.A. The Casimir Effect: Physical Manifestations of Zero-Point Energy; World Scientific: Singapore, 2001. [Google Scholar]
- Milton, K.A. The Casimir effect: Recent controversies and progress. J. Phys. A Math. Gen. 2004, 37, R209–R277. [Google Scholar] [CrossRef]
- Lamoreaux, S.K. The Casimir force: Background, experiments, and applications. Rep. Prog. Phys. 2005, 68, 201–236. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mostepanenko, V.M. Experiment and theory in the Casimir effect. Contemp. Phys. 2006, 47, 131–144. Available online: http://www.tandfonline.com/doi/pdf/10.1080/00107510600693683 (accessed on 11 January 2006). [CrossRef]
- Buhmann, S.Y.; Welsch, D.G. Dispersion forces in macroscopic quantum electrodynamics. Prog. Quantum Electron. 2007, 31, 51–130. [Google Scholar] [CrossRef]
- Genet, C.; Lambrecht, A.; Reynaud, S. The Casimir effect in the nanoworld. Eur. Phys. J. Spec. Top. 2008, 160, 183–193. [Google Scholar] [CrossRef]
- Bordag, M.; Klimchitskaya, G.L.; Mohideen, U.; Mostepanenko, V.M. Advances in the Casimir Effect; Oxford University Press: Oxford, UK, 2009. [Google Scholar]
- Klimchitskaya, G.L.; Mohideen, U.; Mostepanenko, V.M. The Casimir force between real materials: Experiment and theory. Rev. Mod. Phys. 2009, 81, 1827–1885. [Google Scholar] [CrossRef]
- French, R.H.; Parsegian, V.A.; Podgornik, R.; Rajter, R.F.; Jagota, A.; Luo, J.; Asthagiri, D.; Chaudhury, M.K.; Chiang, Y.M.; Granick, S.; et al. Long range interactions in nanoscale science. Rev. Mod. Phys. 2010, 82, 1887–1944. [Google Scholar] [CrossRef]
- Dalvit, D.; Milonni, P.; Roberts, D.; da Rosa, F. (Eds.) Casimir Physics, 1st ed.; Lecture Notes in Physics; Springer: Berlin, Germany, 2011; Volume 834, p. 460. [Google Scholar]
- Sergey, D.O.; Sáez-Gómez, D.; Xambó-Descamps, S. (Eds.) Cosmology, Quantum Vacuum and Zeta Functions; Number 137 in Springer Proceedings in Physics; Springer: Berlin, Germany, 2011; ISBN 978-3-642-19759-8/978-3-642-19760-4. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mohideen, U.; Mostepanenko, V.M. CONTROL OF THE CASIMIR FORCE USING SEMICONDUCTOR TEST BODIES. Int. J. Mod. Phys. B 2011, 25, 171–230. [Google Scholar] [CrossRef]
- Rodriguez, A.W.; Capasso, F.; Johnson, S.G. The Casimir effect in microstructured geometries. Nat. Photonics 2011, 5, 211–221. [Google Scholar] [CrossRef]
- Milton, K.A.; Abalo, E.K.; Parashar, P.; Pourtolami, N.; Brevik, I.; Ellingsen, S.A. Repulsive Casimir and Casimir—Polder forces. J. Phys. A Math. Gen. 2012, 45, 374006. [Google Scholar] [CrossRef]
- Brevik, I. Casimir theory of the relativistic composite string revisited, and a formally related problem in scalar QFT. J. Phys. A Math. Theor. 2012, 45, 374003. [Google Scholar] [CrossRef]
- Bordag, M. Low temperature expansion in the Lifshitz formula. Adv. Math. Phys. 2014, 2014, 981586. [Google Scholar] [CrossRef]
- Buhmann, S.Y. Dispersion Forces I: Macroscopic Quantum Electrodynamics and Ground-State Casimir, Casimir–Polder and Van Der Waals Forces. In Springer Tracts in Modern Physics; Springer: Heidelberg, Germany, 2012; Volume 247. [Google Scholar] [CrossRef]
- Cugnon, J. The Casimir Effect and the Vacuum Energy: Duality in the Physical Interpretation. Few-Body Syst. 2012, 53, 181–188. [Google Scholar] [CrossRef]
- Robert, A.D.J.; Vivekanand, V.G.; Alexandre, T. Many-body van der Waals interactions in molecules and condensed matter. J. Phys. Condens. Matter 2014, 26, 213202. [Google Scholar]
- Rodriguez, A.W.; Hui, P.C.; Woolf, D.P.; Johnson, S.G.; Lončar, M.; Capasso, F. Classical and fluctuation-induced electromagnetic interactions in micron-scale systems: Designer bonding, antibonding, and Casimir forces. Ann. Phys. 2015, 527, 45–80. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mostepanenko, V.M. Casimir and van der Waals forces: Advances and problems. Proc. Peter Great St. Petersburg Polytech. 2015, N1, 41–65. [Google Scholar] [CrossRef]
- Simpson, W.M.R.; Leonhardt, U. Forces of the Quantum Vacuum: An Introduction to Casimir Physics; World Scientific: Singapore, 2015. [Google Scholar]
- Zhao, R.; Luo, Y.; Pendry, J.B. Transformation optics applied to van der Waals interactions. Sci. Bull. 2016, 61, 59–67. [Google Scholar] [CrossRef]
- Woods, L.M.; Dalvit, D.A.R.; Tkatchenko, A.; Rodriguez-Lopez, P.; Rodriguez, A.W.; Podgornik, R. Materials perspective on Casimir and van der Waals interactions. Rev. Mod. Phys. 2016, 88, 045003. [Google Scholar] [CrossRef]
- Bimonte, G.; Emig, T.; Kardar, M.; Krüger, M. Nonequilibrium Fluctuational Quantum Electrodynamics: Heat Radiation, Heat Transfer, and Force. Ann. Rev. Condens. Matter Phys. 2017, 8, 119–143. [Google Scholar] [CrossRef]
- Woods, L.M.; Krüger, M.; Dodonov, V.V. Perspective on some recent and future developments in Casimir interactions. Appl. Sci. 2021, 11, 293. [Google Scholar] [CrossRef]
- Gong, T.; Corrado, M.R.; Mahbub, A.R.; Shelden, C.; Munday, J.N. Recent progress in engineering the Casimir effect—Applications to nanophotonics, nanomechanics, and chemistry. Nanophotonics 2021, 10, 523–536. [Google Scholar] [CrossRef]
- Bimonte, G.; Emig, T.; Graham, N.; Kardar, M. Something Can Come of Nothing: Surface Approaches to Quantum Fluctuations and the Casimir Force. Ann. Rev. Nuclear Particle Sci. 2022, 72, 93–118. [Google Scholar] [CrossRef]
- Moore, G.T. Quantum Theory of the Electromagnetic Field in a Variable Length One Dimensional Cavity. J. Math. Phys. 1970, 11, 2679. [Google Scholar] [CrossRef]
- Golestanian, R.; Kardar, M. Path-integral approach to the dynamic Casimir effect with fluctuating boundaries. Phys. Rev. A 1998, 58, 1713–1722. [Google Scholar] [CrossRef]
- Johansson, J.R.; Johansson, G.; Wilson, C.M.; Nori, F. Dynamical Casimir Effect in a Superconducting Coplanar Waveguide. Phys. Rev. Lett. 2009, 103, 147003. [Google Scholar] [CrossRef] [PubMed]
- Faccio, D.; Carusotto, I. Dynamical Casimir Effect in optically modulated cavities. EPL 2011, 96, 24006. [Google Scholar] [CrossRef]
- Wilson, C.; Johansson, G.; Pourkabirian, A.; Johansson, J.; Duty, T.; Nori, F.; Delsing, P. Observation of the Dynamical Casimir Effect in a Superconducting Circuit. Nature 2011, 479, 376–379. [Google Scholar] [CrossRef] [PubMed]
- Nation, P.D.; Johansson, J.R.; Blencowe, M.P.; Nori, F. Colloquium: Stimulating uncertainty: Amplifying the quantum vacuum with superconducting circuits. Rev. Mod. Phys. 2012, 84, 1–24. [Google Scholar] [CrossRef]
- Lähteenmäki, P.; Paraoanu, G.S.; Hassel, J.; Hakonen, P.J. Dynamical Casimir effect in a Josephson metamaterial. Proc. Natl. Acad. Sci. USA 2013, 110, 4234–4238. Available online: http://www.pnas.org/content/110/11/4234.full.pdf+html (accessed on 13 August 2012).
- Dodonov, V. Fifty Years of the Dynamical Casimir Effect. Physics 2020, 2, 67–104. [Google Scholar] [CrossRef]
- Antezza, M.; Pitaevskii, L.P.; Stringari, S.; Svetovoy, V.B. Casimir-Lifshitz force out of thermal equilibrium. Phys. Rev. A 2008, 77, 022901. [Google Scholar] [CrossRef]
- Bimonte, G. Scattering approach to Casimir forces and radiative heat transfer for nanostructured surfaces out of thermal equilibrium. Phys. Rev. A 2009, 80, 042102. [Google Scholar] [CrossRef]
- Krüger, M.; Emig, T.; Kardar, M. Nonequilibrium Electromagnetic Fluctuations: Heat Transfer and Interactions. Phys. Rev. Lett. 2011, 106, 210404. [Google Scholar] [CrossRef] [PubMed]
- Krüger, M.; Emig, T.; Bimonte, G.; Kardar, M. Non-equilibrium Casimir forces: Spheres and sphere-plate. EPL 2011, 95, 21002. [Google Scholar] [CrossRef]
- Messina, R.; Antezza, M. Casimir-Lifshitz force out of thermal equilibrium and heat transfer between arbitrary bodies. EPL 2011, 95, 61002. [Google Scholar] [CrossRef]
- Latella, I.; Ben-Abdallah, P.; Biehs, S.A.; Antezza, M.; Messina, R. Radiative heat transfer and non-equilibrium Casimir-Lifshitz force in many-body systems with planar geometry. Phys. Rev. B 2017, 95, 205404. [Google Scholar] [CrossRef]
- Iizuka, H.; Fan, S. Control of non-equilibrium Casimir force. Appl. Phys. Lett. 2021, 118, 144001. [Google Scholar] [CrossRef]
- Farrokhabadi, A.; Abadian, N.; Kanjouri, F.; Abadyan, M. Casimir force-induced instability in freestanding nanotweezers and nanoactuators made of cylindrical nanowires. Int. J. Mod. Phys. B 2014, 28, 1450129. Available online: http://www.worldscientific.com/doi/pdf/10.1142/S021797921450129X (accessed on 3 April 2014). [CrossRef]
- Farrokhabadi, A.; Mokhtari, J.; Rach, R.; Abadyan, M. Modeling the influence of the Casimir force on the pull-in instability of nanowire-fabricated nanotweezers. Int. J. Mod. Phys. B 2015, 29, 1450245. Available online: http://www.worldscientific.com/doi/pdf/10.1142/S0217979214502452 (accessed on 6 June 2014). [CrossRef]
- Fisher, M.E.; de Gennes, P.G. Phénomènes aux parois dans un mélange binaire critique. C. R. Seances Acad. Sci. Paris Ser. B 1978, 287, 207–209. [Google Scholar]
- Dantchev, D.; Dietrich, S. Critical Casimir effect: Exact results. Phys. Rep. 2023, 1005, 1–130. [Google Scholar] [CrossRef]
- Krech, M. The Casimir Effect in Critical Systems; World Scientific: Singapore, 1994. [Google Scholar]
- Brankov, J.G.; Dantchev, D.M.; Tonchev, N.S. The Theory of Critical Phenomena in Finite-Size Systems—Scaling and Quantum Effects; World Scientific: Singapore, 2000. [Google Scholar]
- Maciołek, A.; Dietrich, S. Collective behavior of colloids due to critical Casimir interactions. Rev. Mod. Phys. 2018, 90, 045001. [Google Scholar] [CrossRef]
- Gambassi, A.; Dietrich, S. Critical Casimir forces in soft matter. Soft Matter 2024, 20, 3212–3242. [Google Scholar] [CrossRef]
- Dantchev, D.; Rudnick, J. Exact expressions for the partition function of the one-dimensional Ising model in the fixed-M ensemble. Phys. Rev. E 2022, 106, L042103. [Google Scholar] [CrossRef]
- Dantchev, D.M.; Tonchev, N.S.; Rudnick, J. Casimir versus Helmholtz forces: Exact results. Ann. Phys. 2023, 459, 169533. [Google Scholar] [CrossRef]
- Dantchev, D.; Tonchev, N.; Rudnick, J. Casimir and Helmholtz forces in one-dimensional Ising model with Dirichlet (free) boundary conditions. Ann. Phys. 2024, 464, 169647. [Google Scholar] [CrossRef]
- Dantchev, D.; Tonchev, N. A Brief Survey of Fluctuation-induced Interactions in Micro- and Nano-systems and One Exactly Solvable Model as Example. arXiv 2024, arXiv:2403.17109. [Google Scholar] [CrossRef]
- Lifshitz, E.M. The Theory of Molecular Attractive Forces between Solids. Sov. Phys. 1956, 2, 73. reprinted from Zhur. Eksptl. i Teoret. Fiz. 1955, 29, 94–110. (In Russian). [Google Scholar]
- Barash, Y.S.; Ginzburg, V.L. Electromagnetic fluctuations in the substance and molecular (Van der Waals) forces between bodies. Phys. Usp. 1975, 18, 305. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mohideen, U.; Mostepanenko, V.M. Casimir and van der Waals forces between two plates or a sphere (lens) above a plate made of real metals. Phys. Rev. A 2000, 61, 062107. [Google Scholar] [CrossRef]
- Lambrecht, A.; Reynaud, S. Casimir force between metallic mirrors. Eur. Phys. J. D 2000, 8, 309. [Google Scholar] [CrossRef]
- Bezerra, V.B.; Klimchitskaya, G.L.; Mostepanenko, V.M. Higher-order conductivity corrections to the Casimir force. Phys. Rev. A 2000, 62, 014102. [Google Scholar] [CrossRef]
- Geyer, B.; Klimchitskaya, G.L.; Mostepanenko, V.M. Perturbation approach to the Casimir force between two bodies made of different real metals. Phys. Rev. A 2002, 65, 062109. [Google Scholar] [CrossRef]
- Esquivel, R.; Villarreal, C.; Mochán, W.L. Exact surface impedance formulation of the Casimir force: Application to spatially dispersive metals. Phys. Rev. A 2003, 68, 052103. [Google Scholar] [CrossRef]
- Torgerson, J.R.; Lamoreaux, S.K. Low-frequency character of the Casimir force between metallic films. Phys. Rev. E 2004, 70, 047102. [Google Scholar] [CrossRef]
- Esquivel, R.; Svetovoy, V.B. Correction to the Casimir force due to the anomalous skin effect. Phys. Rev. A 2004, 69, 062102. [Google Scholar] [CrossRef]
- Parsegian, V.A. Van der Waals Forces; Cambridge University Press: New York, NY, USA, 2006. [Google Scholar]
- Castillo-Garza, R.; Chang, C.C.; Jimenez, D.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Experimental approaches to the difference in the Casimir force due to modifications in the optical properties of the boundary surface. Phys. Rev. A 2007, 75, 062114. [Google Scholar] [CrossRef]
- Dzyaloshinskii, I.E.; Lifshitz, E.M.; Pitaevskii, L.P. The general theory of van der Waals forces. Adv. Phys. 1961, 10, 165–209. [Google Scholar] [CrossRef]
- Dzyaloshinskii, I.E.; Lifshitz, E.M.; Pitaevskii, L.P. General theory of van der waals’ forces. Sov. Phys. Usp. 1961, 4, 153–176. reprinted from Usp. Fiz. Nauk 1961, 73, 381. (In Russian). [Google Scholar] [CrossRef]
- Landau, L.D.; Pitaevskii, L.P.; Lifshitz, E.M. Electrodynamics of Continuous Media, 2nd ed.; Elsevier: Amsterdam, The Netherlands, 1984. [Google Scholar]
- Sabisky, E.S.; Anderson, C.H. Verification of the Lifshitz Theory of the van der Waals Potential Using Liquid-Helium Films. Phys. Rev. A 1973, 7, 790–806. [Google Scholar] [CrossRef]
- Dietrich, S. Wetting phenomena. In Phase Transitions and Critical Phenomena; Domb, C., Lebowitz, J.L., Eds.; Academic: New York, NY, USA, 1988; Volume 12, pp. 1–218. [Google Scholar]
- Munday, J.N.; Capasso, F.; Parsegian, V.A. Measured long-range repulsive Casimir–Lifshitz forces. Nature 2009, 457, 170. [Google Scholar] [CrossRef]
- Derjaguin, B. Untersuchungen über die Reibung und Adhäsion. Theorie des Anhaftens kleiner Teilchen. Kolloid Z. 1934, 69, 155–164. [Google Scholar] [CrossRef]
- Schlesener, F.; Hanke, A.; Dietrich, S. Critical Casimir forces in colloidal suspensions. J. Stat. Phys. 2003, 110, 981–1013. [Google Scholar] [CrossRef]
- Butt, H.J.; Kappl, M. Surface and Interfacial Forces; WILEY-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2010. [Google Scholar]
- Dantchev, D.; Valchev, G. Surface integration approach: A new technique for evaluating geometry dependent forces between objects of various geometry and a plate. J. Colloid Interface Sci. 2012, 372, 148–163. [Google Scholar] [CrossRef]
- Rusanov, A.I.; Brodskaya, E.N. Dispersion forces in nanoscience. Russ. Chem. Rev. 2019, 88, 837. [Google Scholar] [CrossRef]
- Brodskaya, E.; Rusanov, A.I. Shape Factors of Nanoparticles Interacting with a Solid Surface. Colloid J. 2019, 81, 84–89. [Google Scholar] [CrossRef]
- Djafri, Y.; Turki, D. Dispersion Adhesion Forces between Macroscopic Objects-Basic Concepts and Modelling Techniques: A Critical Review. In Progress in Adhesion and Adhesives; Wiley: Hoboken, NJ, USA, 2019; Chapter 9; pp. 421–442. [Google Scholar] [CrossRef]
- Lu, D.; Fatehi, P. Interfacial interactions of rough spherical surfaces with random topographies. Colloids Surf. A 2022, 642, 128570. [Google Scholar] [CrossRef]
- Esquivel-Sirvent, R. Finite-Size Effects of Casimir—Van der Waals Forces in the Self-Assembly of Nanoparticles. Physics 2023, 5, 322–330. [Google Scholar] [CrossRef]
- Fosco, C.D.; Lombardo, F.C.; Mazzitelli, F.D. Casimir Physics beyond the Proximity Force Approximation: The Derivative Expansion. Physics 2024, 6, 290–316. [Google Scholar] [CrossRef]
- Maia Neto, P.A.; Lambrecht, A.; Reynaud, S. Casimir energy between a plane and a sphere in electromagnetic vacuum. Phys. Rev. A 2008, 78, 012115. [Google Scholar] [CrossRef]
- Rahi, S.J.; Emig, T.; Graham, N.; Jaffe, R.L.; Kardar, M. Scattering theory approach to electrodynamic Casimir forces. Phys. Rev. D 2009, 80, 085021. [Google Scholar] [CrossRef]
- Bimonte, G.; Spreng, B.; Maia Neto, P.A.; Ingold, G.L.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Decca, R.S. Measurement of the Casimir Force between 0.2 and 8 μm: Experimental Procedures and Comparison with Theory. Universe 2021, 7, 93. [Google Scholar] [CrossRef]
- Chamati, H.; Dantchev, D.M.; Tonchev, N.S. Casimir amplitudes in a quantum spherical model with long-range interaction. Eur. Phys. J. B 2000, 14, 307–316. [Google Scholar] [CrossRef]
- Li, H.; Kardar, M. Fluctuation-induced forces between rough surfaces. Phys. Rev. Lett. 1991, 67, 3275–3278. [Google Scholar] [CrossRef]
- Ajdari, A.; Peliti, L.; Prost, J. Fluctuation-induced long-range forces in liquid crystals. Phys. Rev. Lett. 1991, 66, 1481–1484. [Google Scholar] [CrossRef]
- Li, H.; Kardar, M. Fluctuation-induced forces between manifolds immersed in correlated fluids. Phys. Rev. A 1992, 46, 6490–6500. [Google Scholar] [CrossRef] [PubMed]
- Barber, M.N. Finite-size Scaling. In Phase Transitions and Critical Phenomena; Domb, C., Lebowitz, J.L., Eds.; Academic: London, UK, 1983; Volume 8, Chapter 2; pp. 146–266. [Google Scholar]
- Privman, V. Finite-size scaling theory. In Finite Size Scaling and Numerical Simulations of Statistical Systems; Privman, V., Ed.; World Scientific: Singapore, 1990; pp. 1–98. [Google Scholar]
- Kadanoff, L.P. Critical behavior. Universality and scaling. In Proceedings of the International School of Physics “Enrico Fermi”; Green, M.S., Ed.; Academic: New York, NY, USA, 1971; Volume LI, pp. 101–117. [Google Scholar]
- Evans, R. Microscopic theories of simple fluids and their interfaces. In Liquids at Interfaces; Les Houches Session; Charvolin, J., Joanny, J., Zinn-Justin, J., Eds.; Elsevier: Amsterdam, The Netherlands, 1990; Volume XLVIII. [Google Scholar]
- Cardy, J.L. (Ed.) Finite-Size Scaling; North-Holland: Amsterdam, The Netherlands, 1988. [Google Scholar]
- Privman, V. (Ed.) Finite Size Scaling and Numerical Simulation of Statistical Systems; World Scientific: Singapore, 1990; p. 1. [Google Scholar]
- Krech, M.; Dietrich, S. Free energy and specific heat of critical films and surfaces. Phys. Rev. A 1992, 46, 1886–1921. [Google Scholar] [CrossRef]
- Rohwer, C.M.; Squarcini, A.; Vasilyev, O.; Dietrich, S.; Gross, M. Ensemble dependence of critical Casimir forces in films with Dirichlet boundary conditions. Phys. Rev. E 2019, 99, 062103. [Google Scholar] [CrossRef] [PubMed]
- Gross, M.; Vasilyev, O.; Gambassi, A.; Dietrich, S. Critical adsorption and critical Casimir forces in the canonical ensemble. Phys. Rev. E 2016, 94, 022103. [Google Scholar] [CrossRef]
- Gross, M.; Gambassi, A.; Dietrich, S. Statistical field theory with constraints: Application to critical Casimir forces in the canonical ensemble. Phys. Rev. E 2017, 96, 022135. [Google Scholar] [CrossRef]
- Abramowitz, M.; Stegun, I.A. Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables; Dover: New York, NY, USA, 1970. [Google Scholar]
- Baxter, R.J. Exactly Solved Models in Statistical Mechanics; Academic: London, UK, 1982. [Google Scholar]
- Fedoryuk, M.V. The Method of Steepest Descent; Nauka: Moscow, Russia, 1977. (In Russian) [Google Scholar]
- Fedoryuk, M.V. Asymptotic: Integrals and Series; Nauka: Moscow, Russia, 1987. (In Russian) [Google Scholar]
- Chan, H.B.; Aksyuk, V.A.; Kleiman, R.N.; Bishop, D.J.; Capasso, F. Quantum Mechanical Actuation of Microelectromechanical Systems by the Casimir Force. Science 2001, 291, 1941. [Google Scholar] [CrossRef] [PubMed]
- Delrio, F.W.; de Boer, M.P.; Knapp, J.A.; Reedy, E.D.J.; Clews, P.J.; Dunn, M.L. The role of van der Waals forces in adhesion of micromachined surfaces. Nat. Mater. 2005, 4, 629–634. [Google Scholar] [CrossRef]
- Boström, M.; Ellingsen, S.; Brevik, I.; Dou, M.; Persson, C.; Sernelius, B.E. Casimir attractive-repulsive transition in MEMS. Eur. Phys. J. B 2012, 85, 377. [Google Scholar] [CrossRef]
- Buks, E.; Roukes, M.L. Metastability and the Casimir effect in micromechanical systems. Europhys. Lett. 2001, 54, 220. [Google Scholar] [CrossRef]
- Buks, E.; Roukes, M.L. Stiction, adhesion energy, and the Casimir effect in micromechanical systems. Phys. Rev. B 2001, 63, 033402. [Google Scholar] [CrossRef]
- Chan, H.B.; Aksyuk, V.A.; Kleiman, R.N.; Bishop, D.J.; Capasso, F. Nonlinear Micromechanical Casimir Oscillator. Phys. Rev. Lett. 2001, 87, 211801. [Google Scholar] [CrossRef] [PubMed]
- Cecil, J.; Vasquez, J.; Powell, D. A review of gripping and manipulation techniques for micro-assembly applications. Int. J. Prod. Res. 2005, 43, 819–828. [Google Scholar] [CrossRef]
- Casimir, H.B.G.; Polder, D. The Influence of Retardation on the London-van der Waals Forces. Phys. Rev. 1948, 73, 360–372. [Google Scholar] [CrossRef]
- Kenneth, O.; Klich, I. Opposites Attract: A Theorem about the Casimir Force. Phys. Rev. Lett. 2006, 97, 160401. [Google Scholar] [CrossRef] [PubMed]
- Silveirinha, M.G. Casimir interaction between metal-dielectric metamaterial slabs: Attraction at all macroscopic distances. Phys. Rev. B 2010, 82, 085101. [Google Scholar] [CrossRef]
- Rahi, S.J.; Kardar, M.; Emig, T. Constraints on Stable Equilibria with Fluctuation-Induced (Casimir) Forces. Phys. Rev. Lett. 2010, 105, 070404. [Google Scholar] [CrossRef] [PubMed]
- Lifshitz, E.M.; Pitaevskii, L.P. Statistical Physics, Part II; Pergamon: Oxford, UK, 1980. [Google Scholar]
- Milling, A.; Mulvaney, P.; Larson, I. Direct Measurement of Repulsive van der Waals Interactions Using an Atomic Force Microscope. J. Colloid Interface Sci. 1996, 180, 460–465. [Google Scholar] [CrossRef]
- Meurk, A.; Luckham, P.F.; Bergström, L. Direct Measurement of Repulsive and Attractive van der Waals Forces between Inorganic Materials. Langmuir 1997, 13, 3896–3899. [Google Scholar] [CrossRef]
- Lee, S.W.; Sigmund, W.M. Repulsive van der Waals Forces for Silica and Alumina. J. Colloid Interface Sci. 2001, 243, 365–369. [Google Scholar] [CrossRef]
- Lee, S.W.; Sigmund, W.M. AFM study of repulsive van der Waals forces between Teflon AF(TM) thin film and silica or alumina. Colloids Surf. A 2002, 204, 43–50. [Google Scholar] [CrossRef]
- Ishikawa, M.; Inui, N.; Ichikawa, M.; Miura, K. Repulsive Casimir Force in Liquid. J. Phys. Soc. Jpn. 2011, 80, 114601. [Google Scholar] [CrossRef]
- Valchev, G.; Dantchev, D. Critical and near-critical phase behavior and interplay between the thermodynamic Casimir and van der Waals forces in a confined nonpolar fluid medium with competing surface and substrate potentials. Phys. Rev. E 2015, 92, 012119. [Google Scholar] [CrossRef] [PubMed]
- Valchev, G.; Dantchev, D. Sign change in the net force in sphere-plate and sphere-sphere systems immersed in nonpolar critical fluid due to the interplay between the critical Casimir and dispersion van der Waals forces. Phys. Rev. E 2017, 96, 022107. [Google Scholar] [CrossRef] [PubMed]
- Mostepanenko, V.M.; Novello, M. Constraints on non-Newtonian gravity from the Casimir force measurements between two crossed cylinders. Phys. Rev. D 2001, 63, 115003. [Google Scholar] [CrossRef]
- Decca, R.S.; López, D.; Fischbach, E.; Klimchitskaya, G.L.; Krause, D.E.; Mostepanenko, V.M. Tests of new physics from precise measurements of the Casimir pressure between two gold-coated plates. Phys. Rev. D 2007, 75, 077101. [Google Scholar] [CrossRef]
- Masuda, M.; Sasaki, M. Limits on Nonstandard Forces in the Submicrometer Range. Phys. Rev. Lett. 2009, 102, 171101. [Google Scholar] [CrossRef] [PubMed]
- Adler, R.J.; Casey, B.; Jacob, O.C. Vacuum catastrophe: An elementary exposition of the cosmological constant problem. Am. J. Phys. 1995, 63, 620–626. [Google Scholar] [CrossRef]
- Elizalde, E. Quantum vacuum fluctuations and the cosmological constant. J. Phys. A Math. Gen. 2007, 40, 6647. [Google Scholar] [CrossRef]
- Jaffe, R.L. Casimir effect and the quantum vacuum. Phys. Rev. D 2005, 72, 021301. [Google Scholar] [CrossRef]
- Khoury, J.; Weltman, A. Chameleon cosmology. Phys. Rev. D 2004, 69, 044026. [Google Scholar] [CrossRef]
- Nikolic, H. Proof that Casimir force does not originate from vacuum energy. Phys. Lett. B 2016, 761, 197–202. [Google Scholar] [CrossRef]
- Brax, P.; van de Bruck, C.; Davis, A.C.; Shaw, D.J.; Iannuzzi, D. Tuning the Mass of Chameleon Fields in Casimir Force Experiments. Phys. Rev. Lett. 2010, 104, 241101. [Google Scholar] [CrossRef]
- Haghmoradi, H.; Fischer, H.; Bertolini, A.; Galić, I.; Intravaia, F.; Pitschmann, M.; Schimpl, R.; Sedmik, R.I.P. Force metrology with plane parallel plates: Final design review and outlook. arXiv 2024, arXiv:2403.10998. [Google Scholar] [CrossRef]
- Almasi, A.; Brax, P.; Iannuzzi, D.; Sedmik, R.I.P. Force sensor for chameleon and Casimir force experiments with parallel-plate configuration. Phys. Rev. D 2015, 91, 102002. [Google Scholar] [CrossRef]
- Mostepanenko, V.M. Casimir Puzzle and Casimir Conundrum: Discovery and Search for Resolution. Universe 2021, 7, 84. [Google Scholar] [CrossRef]
- Bimonte, G.; López, D.; Decca, R.S. Isoelectronic determination of the thermal Casimir force. Phys. Rev. B 2016, 93, 184434. [Google Scholar] [CrossRef]
- Liu, M.; Xu, J.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Examining the Casimir puzzle with an upgraded AFM-based technique and advanced surface cleaning. Phys. Rev. B 2019, 100, 081406. [Google Scholar] [CrossRef]
- Chen, F.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Control of the Casimir force by the modification of dielectric properties with light. Phys. Rev. B 2007, 76, 035338. [Google Scholar] [CrossRef]
- Pincus, P.A.; Safran, S.A. Charge fluctuations and membrane attractions. EPL 1998, 42, 103–108. [Google Scholar] [CrossRef]
- Ambaum, M.H.P.; Auerswald, T.; Eaves, R.; Harrison, R.G. Enhanced attraction between drops carrying fluctuating charge distributions. Proc. R. Soc. A 2022, 478, 20210714. Available online: https://royalsocietypublishing.org/doi/pdf/10.1098/rspa.2021.0714 (accessed on 9 September 2021). [CrossRef] [PubMed]
- Kirkwood, J.G.; Shumaker, J.B. Forces between Protein Molecules in Solution Arising from Fluctuations in Proton Charge and Configuration. Proc. Natl. Acad. Sci. USA 1952, 38, 863–871. [Google Scholar] [CrossRef]
- Podgornik, R. Electrostatic correlation forces between surfaces with surface specific ionic interactions. J. Chem. Phys. 1989, 91, 5840–5849. [Google Scholar] [CrossRef]
- Ha, B.Y.; Liu, A.J. Counterion-Mediated Attraction between Two Like-Charged Rods. Phys. Rev. Lett. 1997, 79, 1289–1292. [Google Scholar] [CrossRef]
- Henle, M.L.; Pincus, P.A. Equilibrium bundle size of rodlike polyelectrolytes with counterion-induced attractive interactions. Phys. Rev. E 2005, 71, 060801. [Google Scholar] [CrossRef]
- Naji, A.; Dean, D.S.; Sarabadani, J.; Horgan, R.R.; Podgornik, R. Fluctuation-Induced Interaction between Randomly Charged Dielectrics. Phys. Rev. Lett. 2010, 104, 060601. [Google Scholar] [CrossRef]
- Drosdoff, D.; Bondarev, I.V.; Widom, A.; Podgornik, R.; Woods, L.M. Charge-Induced Fluctuation Forces in Graphitic Nanostructures. Phys. Rev. X 2016, 6, 011004. [Google Scholar] [CrossRef]
- Goulian, M.; Bruinsma, R.; Pincus, P. Long-Range Forces in Heterogeneous Fluid Membranes. EPL 1993, 22, 145–150. [Google Scholar] [CrossRef]
- Bitbol, A.F.; Dommersnes, P.G.; Fournier, J.B. Fluctuations of the Casimir-like force between two membrane inclusions. Phys. Rev. E 2010, 81, 050903. [Google Scholar] [CrossRef] [PubMed]
- Lehle, H.; Oettel, M.; Dietrich, S. Effective forces between colloids at interfaces induced by capillary wavelike fluctuations. Europhys. Lett. 2006, 75, 174–180. [Google Scholar] [CrossRef]
- Oettel, M.; Dietrich, S. Colloidal Interactions at Fluid Interfaces. Langmuir 2008, 24, 1425–1441. [Google Scholar] [CrossRef] [PubMed]
- Bitbol, A.F.; Ronia, K.S.; Fournier, J.B. Universal amplitudes of the Casimir-like interactions between four types of rods in fluid membranes. EPL 2011, 96, 40013. [Google Scholar] [CrossRef]
- Machta, B.B.; Veatch, S.L.; Sethna, J.P. Critical Casimir Forces in Cellular Membranes. Phys. Rev. Lett. 2012, 109, 138101. [Google Scholar] [CrossRef] [PubMed]
- Noruzifar, E.; Wagner, J.; Zandi, R. Scattering approach for fluctuation-induced interactions at fluid interfaces. Phys. Rev. E 2013, 88, 042314. [Google Scholar] [CrossRef] [PubMed]
- Rodin, A. Many-impurity phonon Casimir effect in atomic chains. Phys. Rev. B 2019, 100, 195403. [Google Scholar] [CrossRef]
- Lee, G.; Rodin, A. Phonon Casimir effect in polyatomic systems. Phys. Rev. B 2021, 103, 195434. [Google Scholar] [CrossRef]
- Kirkpatrick, T.R.; Ortiz de Zárate, J.M.; Sengers, J.V. Giant Casimir Effect in Fluids in Nonequilibrium Steady States. Phys. Rev. Lett. 2013, 110, 235902. [Google Scholar] [CrossRef]
- Kirkpatrick, T.R.; Ortiz de Zárate, J.M.; Sengers, J.V. Fluctuation-induced pressures in fluids in thermal nonequilibrium steady states. Phys. Rev. E 2014, 89, 022145. [Google Scholar] [CrossRef]
- Kirkpatrick, T.R.; Ortiz de Zárate, J.M.; Sengers, J.V. Nonequilibrium Casimir-like Forces in Liquid Mixtures. Phys. Rev. Lett. 2015, 115, 035901. [Google Scholar] [CrossRef]
- Kirkpatrick, T.R.; Ortiz de Zárate, J.M.; Sengers, J.V. Nonequilibrium fluctuation-induced Casimir pressures in liquid mixtures. Phys. Rev. E 2016, 93, 032117. [Google Scholar] [CrossRef] [PubMed]
- Kirkpatrick, T.R.; Ortiz de Zárate, J.M.; Sengers, J.V. Physical origin of nonequilibrium fluctuation-induced forces in fluids. Phys. Rev. E 2016, 93, 012148. [Google Scholar] [CrossRef]
- Aminov, A.; Kafri, Y.; Kardar, M. Fluctuation-Induced Forces in Nonequilibrium Diffusive Dynamics. Phys. Rev. Lett. 2015, 114, 230602. [Google Scholar] [CrossRef]
- Rohwer, C.M.; Kardar, M.; Krüger, M. Transient Casimir Forces from Quenches in Thermal and Active Matter. Phys. Rev. Lett. 2017, 118, 015702. [Google Scholar] [CrossRef]
- Rohwer, C.M.; Solon, A.; Kardar, M.; Krüger, M. Nonequilibrium forces following quenches in active and thermal matter. Phys. Rev. E 2018, 97, 032125. [Google Scholar] [CrossRef]
- Cattuto, C.; Brito, R.; Marconi, U.M.B.; Nori, F.; Soto, R. Fluctuation-Induced Casimir Forces in Granular Fluids. Phys. Rev. Lett. 2006, 96, 178001. [Google Scholar] [CrossRef]
- Ajdari, A.; Duplantier, B.; Hone, D.; Peliti, L.; Prost, J. “Pseudo-Casimir” effect in liquid crystals. J. Phys. II Fr. 1992, 2, 487–501. [Google Scholar] [CrossRef]
- Lyra, M.L.; Kardar, M.; Svaiter, N.F. Effects of surface enhancement on fluctuation-induced interactions. Phys. Rev. E 1993, 47, 3456–3462. [Google Scholar] [CrossRef]
- Ziherl, P.; Žumer, S. Fluctuations in Confined Liquid Crystals above Nematic-Isotropic Phase Transition Temperature. Phys. Rev. Lett. 1997, 78, 682–685. [Google Scholar] [CrossRef]
- Ziherl, P.; Podgornik, R.; Žumer, S. Wetting-Driven Casimir Force in Nematic Liquid Crystals. Phys. Rev. Lett. 1999, 82, 1189–1192. [Google Scholar] [CrossRef]
- Haddadan, F.K.P.; Schlesener, F.; Dietrich, S. Liquid-crystalline Casimir effect in the presence of a patterned substrate. Phys. Rev. E 2004, 70, 041701. [Google Scholar] [CrossRef] [PubMed]
- Karimi Pour Haddadan, F.; Schlesener, F.; Dietrich, S. Publisher’s Note: Liquid-crystalline Casimir effect in the presence of a patterned substrate [Phys. Rev. E 70, 041701 (2004)]. Phys. Rev. E 2005, 71, 019902. [Google Scholar] [CrossRef]
- Karimi Pour Haddadan, F.; Dietrich, S. Lateral and normal forces between patterned substrates induced by nematic fluctuations. Phys. Rev. E 2006, 73, 051708. [Google Scholar] [CrossRef]
- Ray, D.; Reichhardt, C.; Reichhardt, C.J.O. Casimir effect in active matter systems. Phys. Rev. E 2014, 90, 013019. [Google Scholar] [CrossRef]
- Kjeldbjerg, C.M.; Brady, J.F. Theory for the Casimir effect and the partitioning of active matter. Soft Matter 2021, 17, 523–530. [Google Scholar] [CrossRef]
- Tayar, A.M.; Caballaro, F.; Anderberg, T.; Saleh, O.A.; Marchetti, M.C.; Dogic, Z. Controlling liquid-liquid phase behavior with an active fluid. arXiv, 2022; arXiv:2208.12769. [Google Scholar]
- Balda, A.B.; Argun, A.; Callegari, A.; Volpe, G. Playing with Active Matter. arXiv 2022, arXiv:2209.04168. [Google Scholar]
- Fava, G.; Gambassi, A.; Ginelli, F. Strong Casimir-like Forces in Flocking Active Matter. arXiv 2022, arXiv:2211.02644. [Google Scholar]
- Einstein, A. Über die Gültigkeitsgrenze des Satzes vom thermodynamischen Gleichgewicht und über die Möglichkeit einer neuen Bestimmung der Elementarquanta. Ann. Phys. 1907, 327, 569–572. [Google Scholar] [CrossRef]
- Johnson, J.B. Thermal Agitation of Electricity in Conductors. Phys. Rev. 1928, 32, 97–109. [Google Scholar] [CrossRef]
- Nyquist, H. Thermal Agitation of Electric Charge in Conductors. Phys. Rev. 1928, 32, 110–113. [Google Scholar] [CrossRef]
- Rodriguez, A.W.; Reid, M.T.H.; Johnson, S.G. Fluctuating-surface-current formulation of radiative heat transfer: Theory and applications. Phys. Rev. B 2013, 88, 054305. [Google Scholar] [CrossRef]
- Imboden, M.; Morrison, J.; Campbell, D.K.; Bishop, D.J. Design of a Casimir-driven parametric amplifier. J. Appl. Phys. 2014, 116, 134504. [Google Scholar] [CrossRef]
- Ye, Y.; Hu, Q.; Zhao, Q.; Meng, Y. Casimir repulsive-attractive transition between liquid-separated dielectric metamaterial and metal. Phys. Rev. B 2018, 98, 035410. [Google Scholar] [CrossRef]
- Palasantzas, G.; Sedighi, M.; Svetovoy, V.B. Applications of Casimir forces: Nanoscale actuation and adhesion. Appl. Phys. Lett. 2020, 117, 120501. [Google Scholar] [CrossRef]
- Munkhbat, B.; Canales, A.; Küçüköz, B.; Baranov, D.G.; Shegai, T.O. Tunable self-assembled Casimir microcavities and polaritons. Nature 2021, 597, 214–219. [Google Scholar] [CrossRef] [PubMed]
- Xu, Z.; Gao, X.; Bang, J.; Jacob, Z.; Li, T. Non-reciprocal energy transfer through the Casimir effect. Nat. Nanotechnol. 2022, 17, 148–152. [Google Scholar] [CrossRef] [PubMed]
- Schmidt, F.; Callegari, A.; Daddi-Moussa-Ider, A.; Munkhbat, B.; Verre, R.; Shegai, T.; Käll, M.; Löwen, H.; Gambassi, A.; Volpe, G. Tunable critical Casimir forces counteract Casimir–Lifshitz attraction. Nat. Phys. 2023, 19, 271–278. [Google Scholar] [CrossRef]
- Iannuzzi, D.; Munday, J.; Capasso, F. Ultra-Low Friction Configuration. U.S. Patent No. US 2007/0066494 A1, 22 March 2007. [Google Scholar]
- Tröndle, M.; Zvyagolskaya, O.; Gambassi, A.; Vogt, D.; Harnau, L.; Bechinger, C.; Dietrich, S. Trapping colloids near chemical stripes via critical Casimir forces. Mol. Phys. 2011, 109, 1169–1185. [Google Scholar] [CrossRef]
- Dean, D.S.; Lu, B.S.; Maggs, A.C.; Podgornik, R. Nonequilibrium Tuning of the Thermal Casimir Effect. Phys. Rev. Lett. 2016, 116, 240602. [Google Scholar] [CrossRef] [PubMed]
- Nguyen, T.A.; Newton, A.; Veen, S.J.; Kraft, D.J.; Bolhuis, P.G.; Schall, P. Switching Colloidal Superstructures by Critical Casimir Forces. Adv. Mater. 2017, 29, 1700819. [Google Scholar] [CrossRef]
- Guo, H.; Stan, G.; Liu, Y. Nanoparticle separation based on size-dependent aggregation of nanoparticles due to the critical Casimir effect. Soft Matter 2018, 14, 1311–1318. [Google Scholar] [CrossRef]
- Marino, E.; Balazs, D.M.; Crisp, R.W.; Hermida-Merino, D.; Loi, M.A.; Kodger, T.E.; Schall, P. Controlling Superstructure-Property Relationships via Critical Casimir Assembly of Quantum Dots. J. Phys. Chem. C 2019, 23, 13451–13457. [Google Scholar] [CrossRef]
- Magazzù, A.; Callegari, A.; Staforelli, J.P.; Gambassi, A.; Dietrich, S.; Volpe, G. Controlling the dynamics of colloidal particles by critical Casimir forces. Soft Matter 2019, 15, 2152–2162. [Google Scholar] [CrossRef]
- Vasilyev, O.; Marino, E.; Kluft, B.B.; Schall, P.; Kondrat, S. Debye vs Casimir: Controlling the structure of charged nanoparticles deposited on a substrate. Nanoscale 2021, 13, 6475–6488. [Google Scholar] [CrossRef]
- Stuij, S.; Rouwhorst, J.; Jonas, H.J.; Ruffino, N.; Gong, Z.; Sacanna, S.; Bolhuis, P.G.; Schall, P. Revealing Polymerization Kinetics with Colloidal Dipatch Particles. Phys. Rev. Lett. 2021, 127, 108001. [Google Scholar] [CrossRef]
- Xi, Y.; Lankone, R.S.; Sung, L.P.; Liu, Y. Tunable thermo-reversible bicontinuous nanoparticle gel driven by the binary solvent segregation. Nat. Commun. 2021, 12, 910. [Google Scholar] [CrossRef]
- Valencia, J.R.V.; Guo, H.; Castaneda-Priego, R.; Liu, Y. Concentration and size effects on the size-selective particle purification method using the critical Casimir force. Phys. Chem. Chem. Phys. 2021, 23, 4404–4412. [Google Scholar] [CrossRef] [PubMed]
- Wang, G.; Nowakowski, P.; Bafi, N.F.; Midtvedt, B.; Schmidt, F.; Verre, R.; Käll, M.; Dietrich, S.; Kondrat, S.; Volpe, G. Nanoalignment by Critical Casimir Torques. arXiv 2024, arXiv:2401.06260. [Google Scholar] [CrossRef]





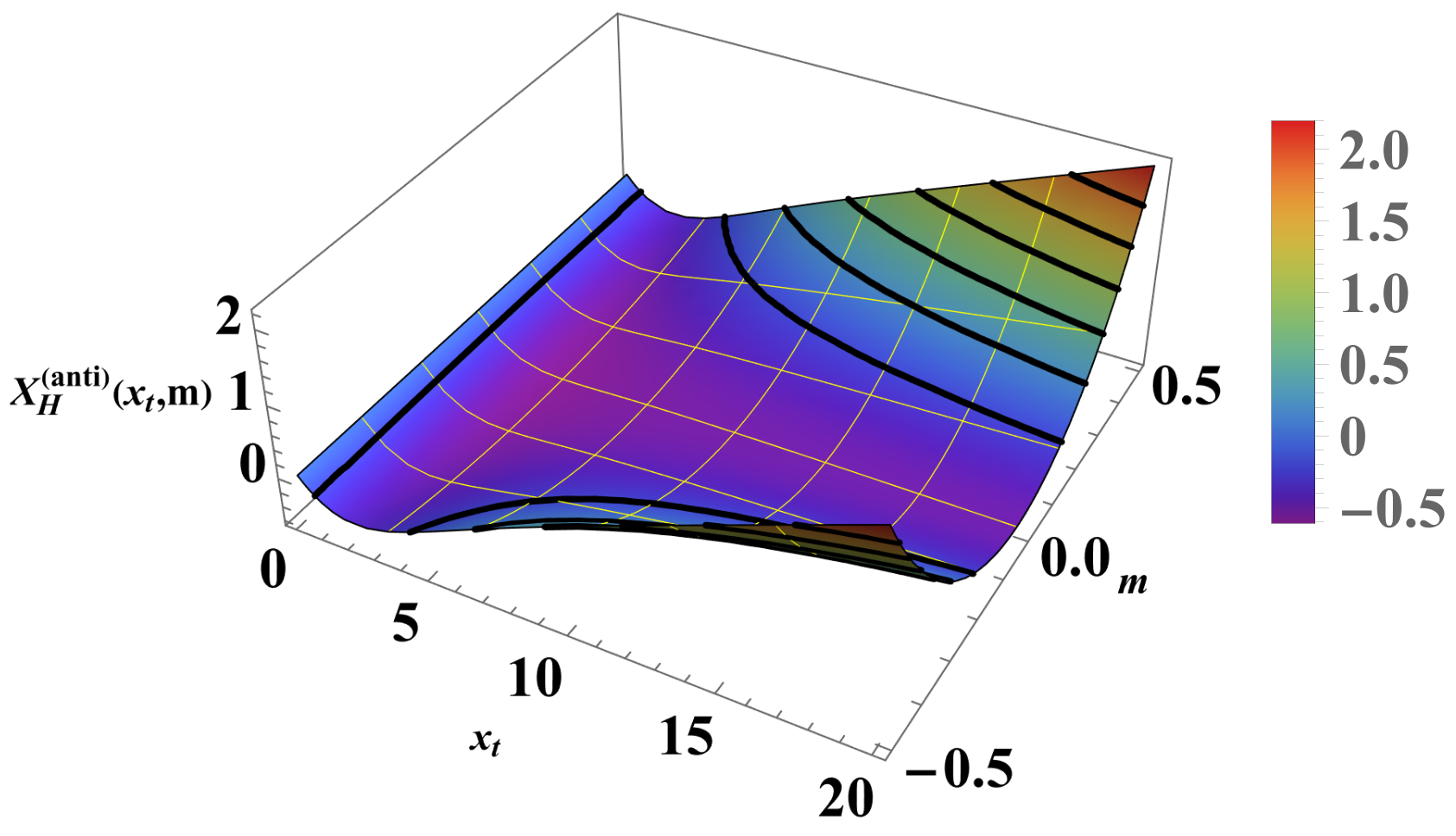
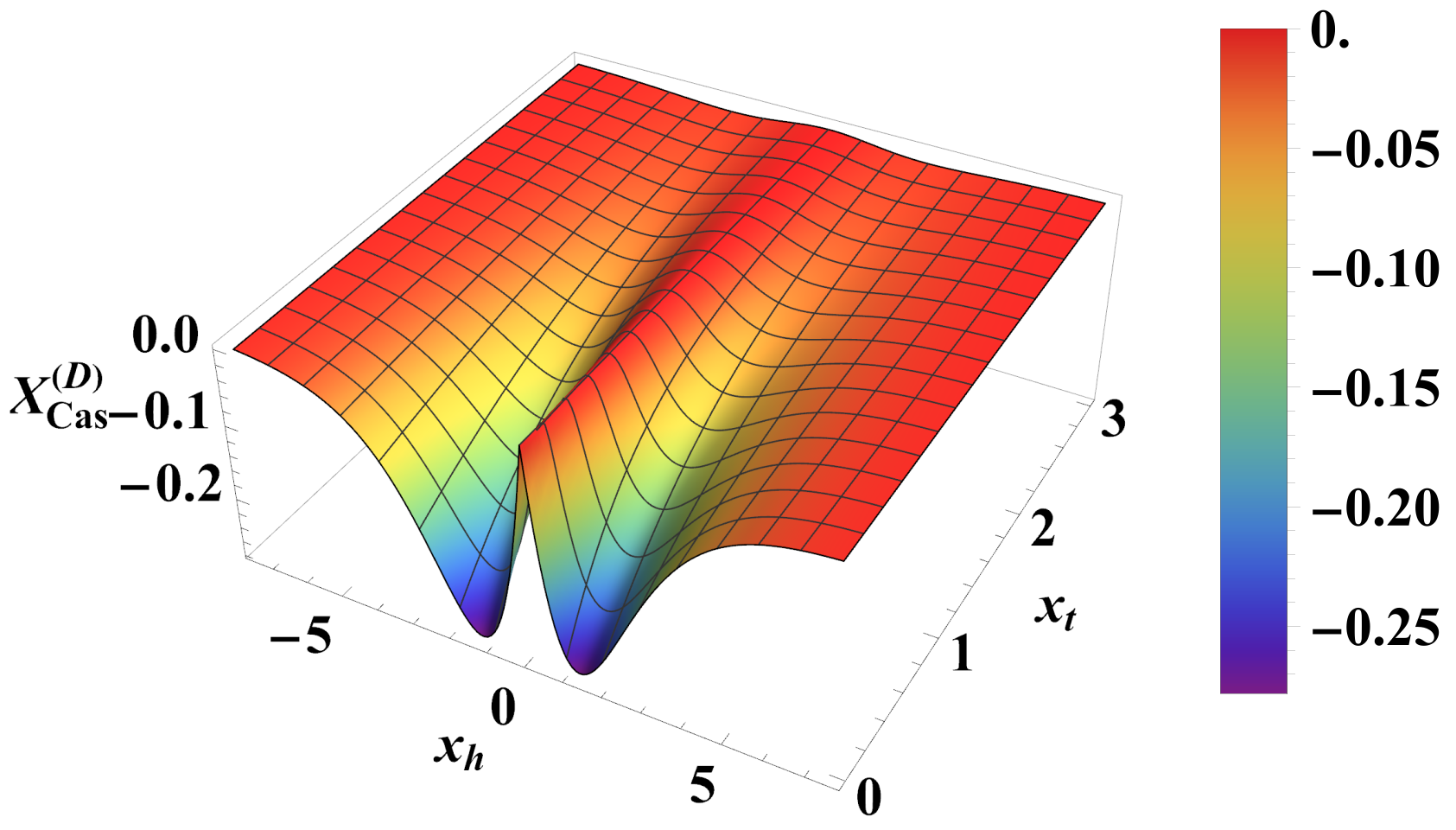
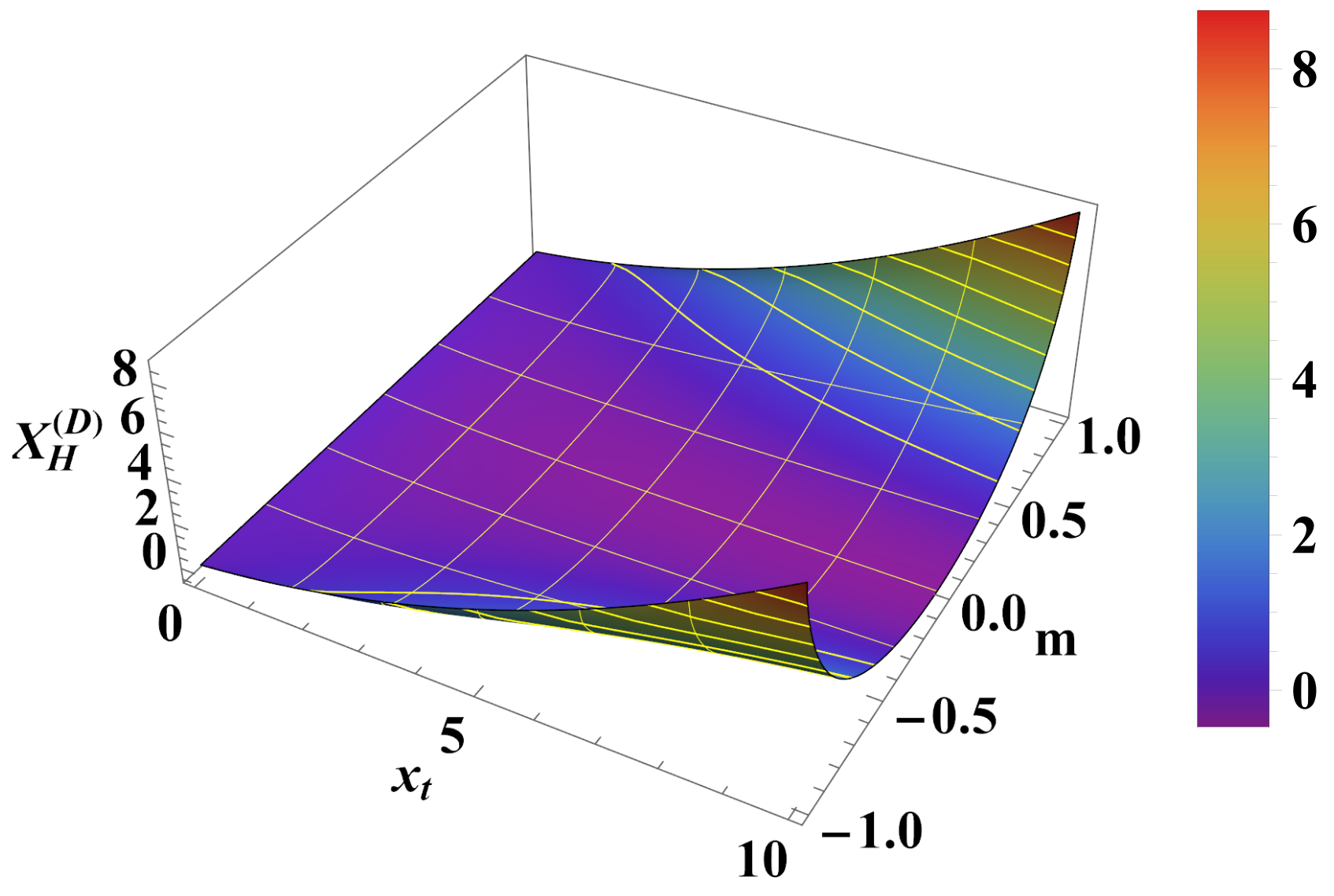
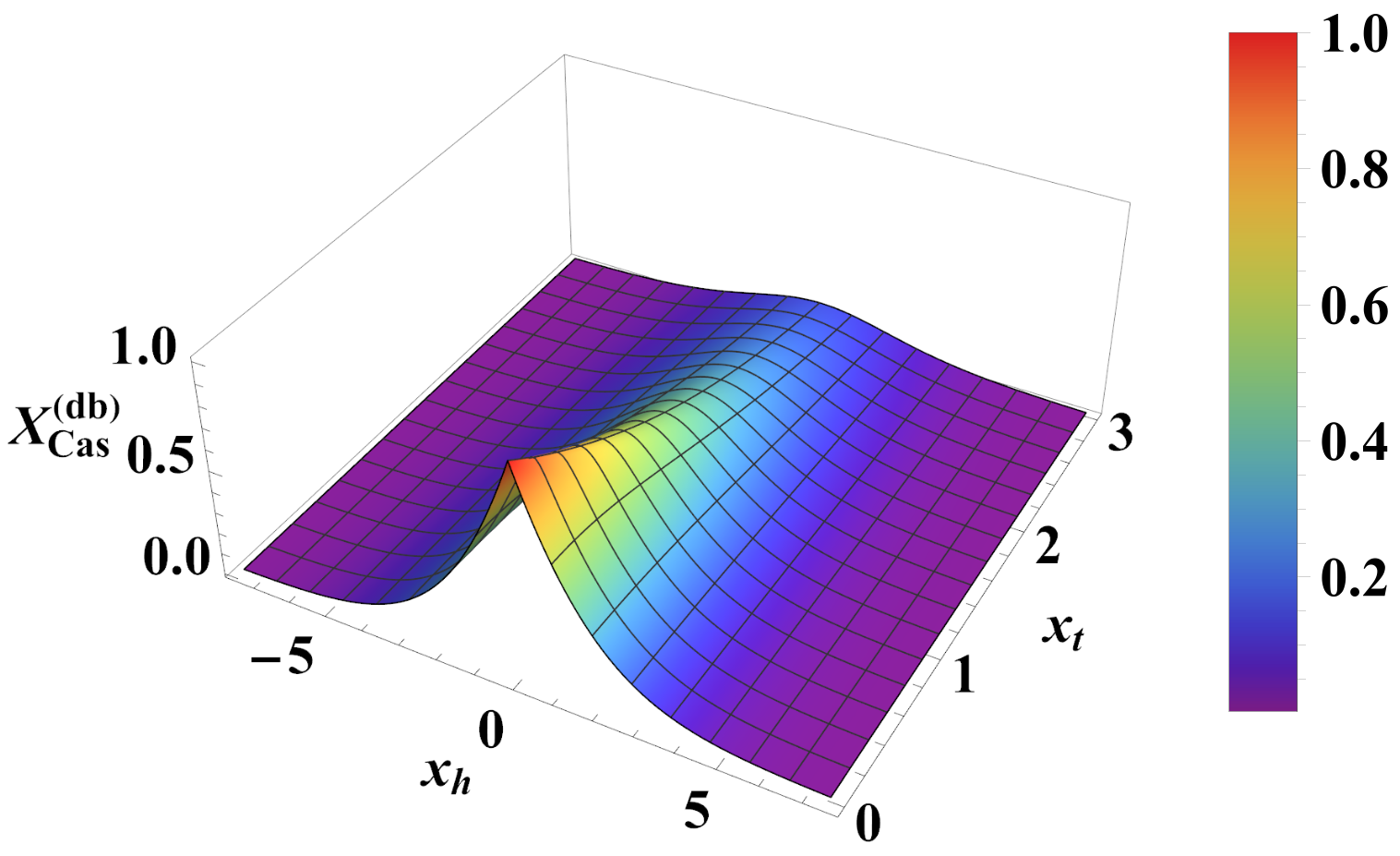

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dantchev, D. On Casimir and Helmholtz Fluctuation-Induced Forces in Micro- and Nano-Systems: Survey of Some Basic Results. Entropy 2024, 26, 499. https://doi.org/10.3390/e26060499
Dantchev D. On Casimir and Helmholtz Fluctuation-Induced Forces in Micro- and Nano-Systems: Survey of Some Basic Results. Entropy. 2024; 26(6):499. https://doi.org/10.3390/e26060499
Chicago/Turabian StyleDantchev, Daniel. 2024. "On Casimir and Helmholtz Fluctuation-Induced Forces in Micro- and Nano-Systems: Survey of Some Basic Results" Entropy 26, no. 6: 499. https://doi.org/10.3390/e26060499
APA StyleDantchev, D. (2024). On Casimir and Helmholtz Fluctuation-Induced Forces in Micro- and Nano-Systems: Survey of Some Basic Results. Entropy, 26(6), 499. https://doi.org/10.3390/e26060499





