Forced Friends: Why the Free Energy Principle Is Not the New Hamilton’s Principle
Abstract
:1. Introduction
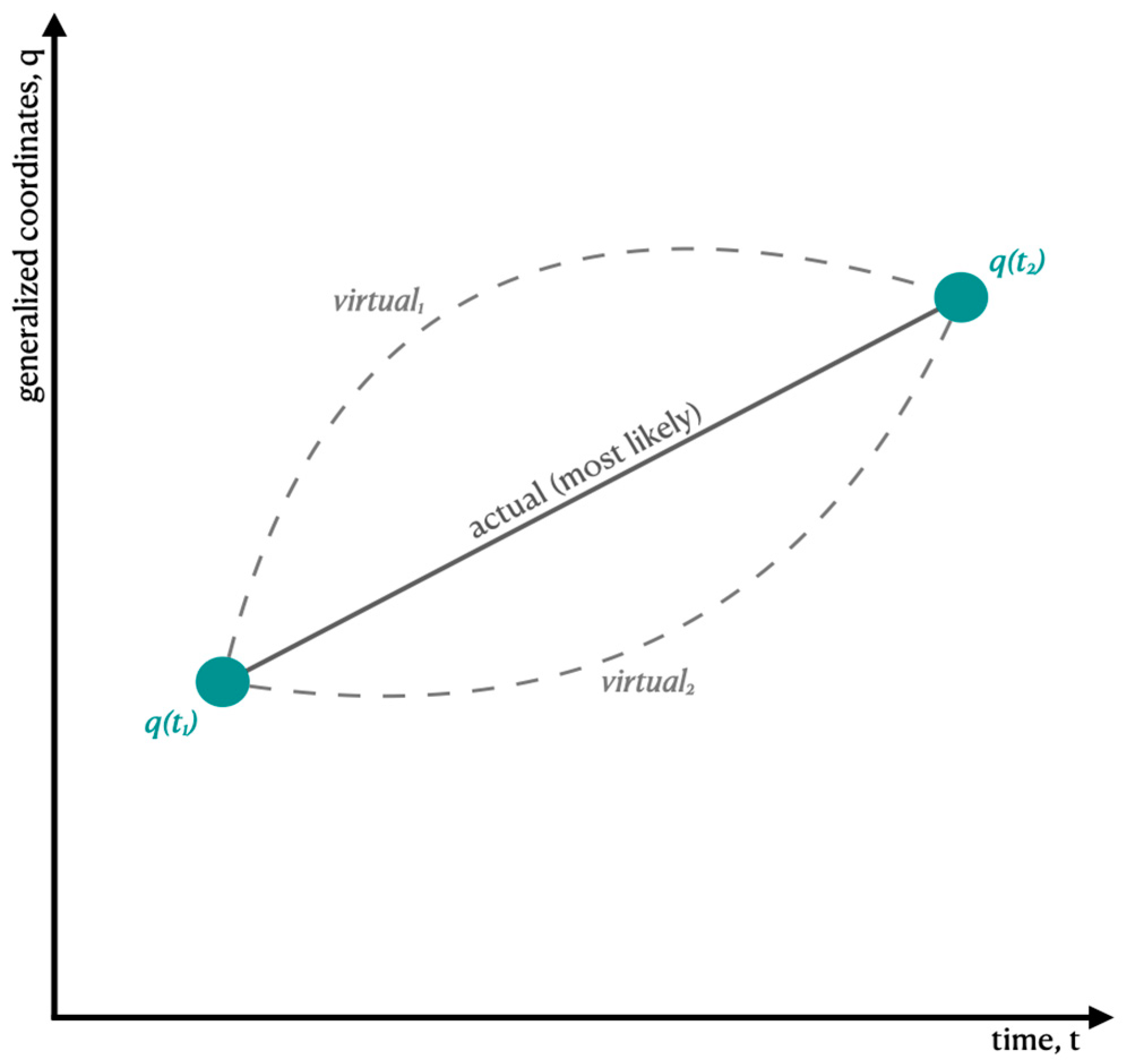
2. Hamilton’s Principle of Stationary Action
3. The Free Energy Principle as a Principle of Least Action
3.1. The Free Energy Principle
3.2. Model Inversion
3.3. Morphogenesis
4. Interpreting the Relationship between the Free Energy Principle and Hamilton’s Principle
4.1. Strong Reading
Dilemma for the Strong Reading
- The scope of application of the FEP includes all biological systems;
- The scope of application of HP does not include biological systems;
- The scopes of application of theoretical constructs are equivalent iff the theoretical constructs are logically equivalent;
- The FEP and HP are logically equivalent.
4.2. Weak Reading
Assessing the Analogies between the Free Energy Principle and Hamilton’s Principle
5. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Friston, K. The Variational Principles of Action. In Geometric and Numerical Foundations of Movements; Laumond, J.-P., Mansard, N., Lasserre, J.-B., Eds.; Springer International Publishing: Cham, Switzerland, 2017; pp. 207–235. ISBN 978-3-319-51547-2. [Google Scholar]
- Ramstead, M.J.D.; Badcock, P.B.; Friston, K.J. Answering Schrödinger’s Question: A Free-Energy Formulation. Phys. Life Rev. 2018, 24, 1–16. [Google Scholar] [CrossRef] [PubMed]
- Da Costa, L.; Friston, K.; Heins, C.; Pavliotis, G.A. Bayesian Mechanics for Stationary Processes. arXiv 2021, arXiv:210613830. [Google Scholar] [CrossRef]
- Fortier, M.; Friedman, D.; Friston, K. Of Woodlice and Men: A Bayesian Account of Cognition, Life and Consciousness. An Interview with Karl Friston. ALIUS Bull. 2018, 2, 17–43. [Google Scholar]
- Kim, C.S. Bayesian Mechanics of Perceptual Inference and Motor Control in the Brain. Biol. Cybern. 2021, 115, 87–102. [Google Scholar] [CrossRef] [PubMed]
- Berdichevsky, V. Variational Principles. In Variational Principles of Continuum Mechanics; Springer: Berlin/Heidelberg, Germany, 2009; pp. 3–44. ISBN 978-3-540-88466-8. [Google Scholar]
- dell’Isola, F.; Placidi, L. Variational Principles Are a Powerful Tool Also for Formulating Field Theories. In Variational Models and Methods in Solid and Fluid Mechanics; Pfeiffer, F., Rammerstorfer, F.G., Salençon, J., Schrefler, B., Serafini, P., dell’Isola, F., Gavrilyuk, S., Eds.; Springer: Vienna, Austria, 2011; Volume 535, pp. 1–15. ISBN 978-3-7091-0982-3. [Google Scholar]
- Ván, P.; Kovács, R. Variational Principles and Nonequilibrium Thermodynamics. Philos. Trans. R. Soc. Math. Phys. Eng. Sci. 2020, 378, 20190178. [Google Scholar] [CrossRef]
- Helrich, C. Is There a Basis for Teleology in Physics? Zygon 2007, 42, 97–110. [Google Scholar] [CrossRef]
- Escamilla-Rivera, C.; Fabris, J.C. The Possibility of a Non-Lagrangian Theory of Gravity. Universe 2021, 7, 230. [Google Scholar] [CrossRef]
- Rao, J.S. Energy Methods. In History of Rotating Machinery Dynamics; Rao, J.S., Ed.; History of Mechanism and Machine Science; Springer: Dordrecht, The Netherlands, 2011; pp. 49–98. ISBN 978-94-007-1165-5. [Google Scholar]
- Feynman, R. The Feynman Lectures on Physics. Volume II, Mainly Electromagnetism and Matter; The New Millen, Ed.; California Institute of Technology: Pasadena, CA, USA, 2013. [Google Scholar]
- Badur, J.; Badur, J. An Introduction to Variational Derivation of the Pseudomomentum Conservation in Thermohydrodynamics. In Variational and Extremum Principles in Macroscopic Systems; Sieniutycz, S., Farkas, H., Eds.; Elsevier: Oxford, UK, 2005; pp. 157–185. ISBN 978-0-08-044488-8. [Google Scholar]
- Longo, G.; Montévil, M.; Kauffman, S. No Entailing Laws, but Enablement in the Evolution of the Biosphere. In Proceedings of the 14th Annual Conference Companion on Genetic and Evolutionary Computation, Philadelphia, PA, USA, 7–11 July 2012; ACM: New York, NY, USA, 2012; pp. 1379–1392. [Google Scholar]
- Kondepudi, D.; Prigogine, I. Modern Thermodynamics: From Heat Engines to Dissipative Structures; CourseSmart Series; John Wiley & Sons: Hoboken, NJ, USA, 2014; ISBN 978-1-118-37181-7. [Google Scholar]
- Dodin, I.Y.; Zhmoginov, A.I.; Ruiz, D.E. Variational Principles for Dissipative (Sub)Systems, with Applications to the Theory of Linear Dispersion and Geometrical Optics. Phys. Lett. A 2017, 381, 1411–1430. [Google Scholar] [CrossRef]
- Lazo, M.J.; Krumreich, C.E. The Action Principle for Dissipative Systems. J. Math. Phys. 2014, 55, 122902. [Google Scholar] [CrossRef]
- Friston, K.; FitzGerald, T.; Rigoli, F.; Schwartenbeck, P.; Pezzulo, G. Active Inference: A Process Theory. Neural Comput. 2017, 29, 1–49. [Google Scholar] [CrossRef]
- Friston, K. Hierarchical Models in the Brain. PLoS Comput. Biol. 2008, 4, e1000211. [Google Scholar] [CrossRef]
- Friston, K. The Free-Energy Principle: A Rough Guide to the Brain? Trends Cogn. Sci. 2009, 13, 293–301. [Google Scholar] [CrossRef]
- Friston, K. A Free Energy Principle for Biological Systems. Entropy 2012, 14, 2100–2121. [Google Scholar] [CrossRef]
- Friston, K.; Stephan, K.E. Free-Energy and the Brain. Synthese 2007, 159, 417–458. [Google Scholar] [CrossRef]
- Friston, K.; Kiebel, S. Predictive Coding under the Free-Energy Principle. Philos. Trans. R. Soc. Lond. B Biol. Sci. 2009, 364, 1211–1221. [Google Scholar] [CrossRef]
- Friston, K.; Daunizeau, J.; Kilner, J.; Kiebel, S.J. Action and Behavior: A Free-Energy Formulation. Biol. Cybern. 2010, 102, 227–260. [Google Scholar] [CrossRef]
- Calvo, P.; Friston, K. Predicting Green: Really Radical (Plant) Predictive Processing. J. R. Soc. Interface 2017, 14, 20170096. [Google Scholar] [CrossRef]
- Ramstead, M.; Kirchhoff, M.; Friston, K. A Tale of Two Densities: Active Inference Is Enactive Inference. Adapt. Behav. 2019, 28, 225–239. [Google Scholar] [CrossRef]
- Parr, T.; Pezzulo, G.; Friston, K.J. Active Inference: The Free Energy Principle in Mind, Brain, and Behavior; The MIT Press: Cambridge, MA, USA, 2022; ISBN 978-0-262-04535-3. [Google Scholar]
- Ramstead, M.J.D.; Constant, A.; Badcock, P.B.; Friston, K.J. Variational Ecology and the Physics of Sentient Systems. Phys. Life Rev. 2019, 31, 188–205. [Google Scholar] [CrossRef] [PubMed]
- Friston, K. A Theory of Cortical Responses. Philos. Trans. R. Soc. Lond. B Biol. Sci. 2005, 360, 815–836. [Google Scholar] [CrossRef] [PubMed]
- Mann, S.F.; Pain, R.; Kirchhoff, M.D. Free Energy: A User’s Guide. Biol. Philos. 2022, 37, 33. [Google Scholar] [CrossRef]
- Buckley, C.; Kim, C.S.; McGregor, S.; Seth, A. The Free Energy Principle for Action and Perception: A Mathematical Review. J. Math. Psychol. 2017, 81, 55–79. [Google Scholar] [CrossRef]
- Bruineberg, J.; Dolega, K.; Dewhurst, J.; Baltieri, M. The Emperor’s New Markov Blankets. Behav. Brain Sci. 2021, 45, e183. [Google Scholar] [CrossRef]
- Millidge, B.; Seth, A.; Buckley, C.L. A Mathematical Walkthrough and Discussion of the Free Energy Principle. arXiv 2021, arXiv:210813343. [Google Scholar]
- Bogacz, R. A Tutorial on the Free-Energy Framework for Modelling Perception and Learning. J. Math. Psychol. 2017, 76, 198–211. [Google Scholar] [CrossRef]
- Friston, K.J.; Daunizeau, J.; Kiebel, S.J. Reinforcement Learning or Active Inference? PLoS ONE 2009, 4, e6421. [Google Scholar] [CrossRef]
- Hesp, C.; Smith, R.; Parr, T.; Allen, M.; Friston, K.J.; Ramstead, M.J.D. Deeply Felt Affect: The Emergence of Valence in Deep Active Inference. Neural Comput. 2021, 33, 398–446. [Google Scholar] [CrossRef]
- Friston, K. A Free Energy Principle for a Particular Physics. arXiv 2019, arXiv:190610184. [Google Scholar]
- Aguilera, M.; Millidge, B.; Tschantz, A.; Buckley, C.L. How Particular Is the Physics of the Free Energy Principle? Phys. Life Rev. 2022, 40, 24. [Google Scholar] [CrossRef]
- Webb, S.; Goliński, A.; Zinkov, R.; Siddharth, N.; Rainforth, T.; Teh, Y.W.; Wood, F. Faithful Inversion of Generative Models for Effective Amortized Inference. In Advances in Neural Information Processing Systems 31 (NeurIPS 2018), Proceedings of the 32nd International Conference on Neural Information Processing Systems, Montréal, QC, Canada, 3–8 December 2018; Curran Associates Inc.: New York, NY, USA, 2018; pp. 3074–3084. [Google Scholar]
- Clark, A. Whatever next? Predictive Brains, Situated Agents, and the Future of Cognitive Science. Behav. Brain Sci. 2013, 36, 181–204. [Google Scholar] [CrossRef]
- Rao, R.; Ballard, D. Predictive Coding in the Visual Cortex: A Functional Interpretation of Some Extra-Classical Receptive-Field Effects. Nat. Neurosci. 1999, 2, 79–87. [Google Scholar] [CrossRef]
- Encyclopedia Britannica (Ed.) Morphogenesis; Encyclopedia Britannica: Edinburgh, UK, 2016. [Google Scholar]
- Kuchling, F.; Friston, K.; Georgiev, G.; Levin, M. Morphogenesis as Bayesian Inference: A Variational Approach to Pattern Formation and Control in Complex Biological Systems. Phys. Life Rev. 2020, 33, 88–108. [Google Scholar] [CrossRef]
- Cline, D.; Sarkis, M. Variational Principles in Classical Mechanics; University of Rochester River Campus Librarie: Rochester, NY, USA, 2017; ISBN 978-0-9988372-4-6. [Google Scholar]
- Friston, K.; Michael, L.; Biswa, S.; Giovanni, P. Knowing One’s Place: A Free-Energy Approach to Pattern Regulation. J. R. Soc. Interface 2015, 12, 20141383. [Google Scholar] [CrossRef]
- Nesbet, R.K. Variational Principles and Methods in Theoretical Physics and Chemistry; Cambridge University Press: Cambridge, UK, 2005; ISBN 978-0-521-80391-5. [Google Scholar]
- Strutt, J.W. Some General Theorems Relating to Vibrations. Proc. Lond. Math. Soc. 1873, 4, 357–368. [Google Scholar] [CrossRef]
- Galley, C.R. Classical Mechanics of Nonconservative Systems. Phys. Rev. Lett. 2013, 110, 174301. [Google Scholar] [CrossRef]
- Georgiev, G.; Georgiev, I. The Least Action and the Metric of an Organized System. Open Syst. Inf. Dyn. 2002, 9, 371–380. [Google Scholar] [CrossRef]
- de Oliveira, C.; Werlang, T. Ergodic Hypothesis in Classical Statistical Mechanics. Rev. Bras. Ensino Física 2007, 29, 189–201. [Google Scholar] [CrossRef]
- Lombardi, O.; Fortin, S.; López, C.; Holik, F. Quantum Worlds: Perspectives on the Ontology of Quantum Mechanics; Cambridge University Press: Cambridge, UK, 2019; ISBN 978-1-108-47347-7. [Google Scholar]
- Nicol, M.; Petersen, K. Ergodic Theory: Basic Examples and Constructions BT—Mathematics of Complexity and Dynamical Systems; Meyers, R.A., Ed.; Springer: New York, NY, USA, 2011; pp. 264–288. ISBN 978-1-4614-1806-1. [Google Scholar]
- Annila, A. Chiral Conformity Emerges from the Least-Time Free Energy Consumption. Interface Focus 2023, 13, 20220074. [Google Scholar] [CrossRef]
- Mendelson, E. Introduction to Mathematical Logic, 3rd ed.; Springer: New York, NY, USA, 1987. [Google Scholar]
- Sims, A. A Problem of Scope for the Free Energy Principle as a Theory of Cognition. Philos. Psychol. 2016, 29, 967–980. [Google Scholar] [CrossRef]
- Levins, R. The Strategy of Model Building in Poupation Biology. In Conceptual Issues in Evolutionary Biology; MIT Press: Cambridge, MA, USA, 1966; pp. 18–27. [Google Scholar]
- Matthewson, J.; Weisberg, M. The Structure of Tradeoffs in Model Building. Synthese 2009, 170, 169–190. [Google Scholar] [CrossRef]
- Badcock, P.B.; Ramstead, M.J.D.; Sheikhbahaee, Z.; Constant, A. Applying the Free Energy Principle to Complex Adaptive Systems. Entropy 2022, 24, 689. [Google Scholar] [CrossRef]
- Sleeman, B.D. Complexity in Biological Systems and Hamiltonian Dynamics. Proc. R. Soc. Lond. Math. Phys. Sci. 1989, 425, 17–47. [Google Scholar] [CrossRef]
- Veissière, S.P.L.; Constant, A.; Ramstead, M.J.D.; Friston, K.J.; Kirmayer, L.J. Thinking through Other Minds: A Variational Approach to Cognition and Culture. Behav. Brain Sci. 2020, 43, e90. [Google Scholar] [CrossRef]
- Hesp, C.; Ramstead, M.; Constant, A.; Badcock, P.; Kirchhoff, M.; Friston, K. A Multi-Scale View of the Emergent Complexity of Life: A Free-Energy Proposal. In Evolution, Development and Complexity; Georgiev, G.Y., Smart, J.M., Flores Martinez, C.L., Price, M.E., Eds.; Springer International Publishing: Cham, Switzerland, 2019; pp. 195–227. ISBN 978-3-030-00074-5. [Google Scholar]
- Wang, Q.A.; Wang, R. A True Least Action Principle for Damped Motion. J. Phys. Conf. Ser. 2018, 1113, 012003. [Google Scholar] [CrossRef]
- Bartha, P. Analogy and Analogical Reasoning. In The Stanford Encyclopedia of Philosophy; Zalta, E.N., Ed.; Metaphysics Research Lab, Stanford University: Stanford, CA, USA, 2022. [Google Scholar]
- Hesse, M. Models in Physics. Br. J. Philos. Sci. 1953, 15, 198–214. [Google Scholar] [CrossRef]
- Frigg, R.; Hartmann, S. Models in Science. In The Stanford Encyclopedia of Philosophy; Zalta, E.N., Ed.; Metaphysics Research Lab, Stanford University: Stanford, CA, USA, 2020. [Google Scholar]
- Toon, A. Similarity and Scientific Representation. Int. Stud. Philos. Sci. 2012, 26, 241–257. [Google Scholar] [CrossRef]
- Hesse, M. Models and Analogies in Science, 2nd ed.; Notre Dame Press: Notre Dame, IN, USA, 1963. [Google Scholar]
- Sacksteder, W. The Logic of Analogy. Philos. Rhetor. 1974, 7, 234–252. [Google Scholar]
- Hopfield, J.J. Neural Networks and Physical Systems with Emergent Collective Computational Abilities. Proc. Natl. Acad. Sci. USA 1982, 79, 2554–2558. [Google Scholar] [CrossRef]
- Sherrington, D.; Kirkpatrick, S. Solvable Model of a Spin-Glass. Phys. Rev. Lett. 1975, 35, 1792–1796. [Google Scholar] [CrossRef]
- Churchland, P.; Sejnowski, T. The Computational Brain, 1st ed.; MIT Press: Cambridge, MA, USA, 1992; ISBN 978-0-262-53120-7. [Google Scholar]
- Keynes, J.M. A Treatise on Probability; Dover Publications: New York, NY, USA, 1921. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Radomski, B.M.; Dołęga, K. Forced Friends: Why the Free Energy Principle Is Not the New Hamilton’s Principle. Entropy 2024, 26, 797. https://doi.org/10.3390/e26090797
Radomski BM, Dołęga K. Forced Friends: Why the Free Energy Principle Is Not the New Hamilton’s Principle. Entropy. 2024; 26(9):797. https://doi.org/10.3390/e26090797
Chicago/Turabian StyleRadomski, Bartosz Michał, and Krzysztof Dołęga. 2024. "Forced Friends: Why the Free Energy Principle Is Not the New Hamilton’s Principle" Entropy 26, no. 9: 797. https://doi.org/10.3390/e26090797
APA StyleRadomski, B. M., & Dołęga, K. (2024). Forced Friends: Why the Free Energy Principle Is Not the New Hamilton’s Principle. Entropy, 26(9), 797. https://doi.org/10.3390/e26090797







