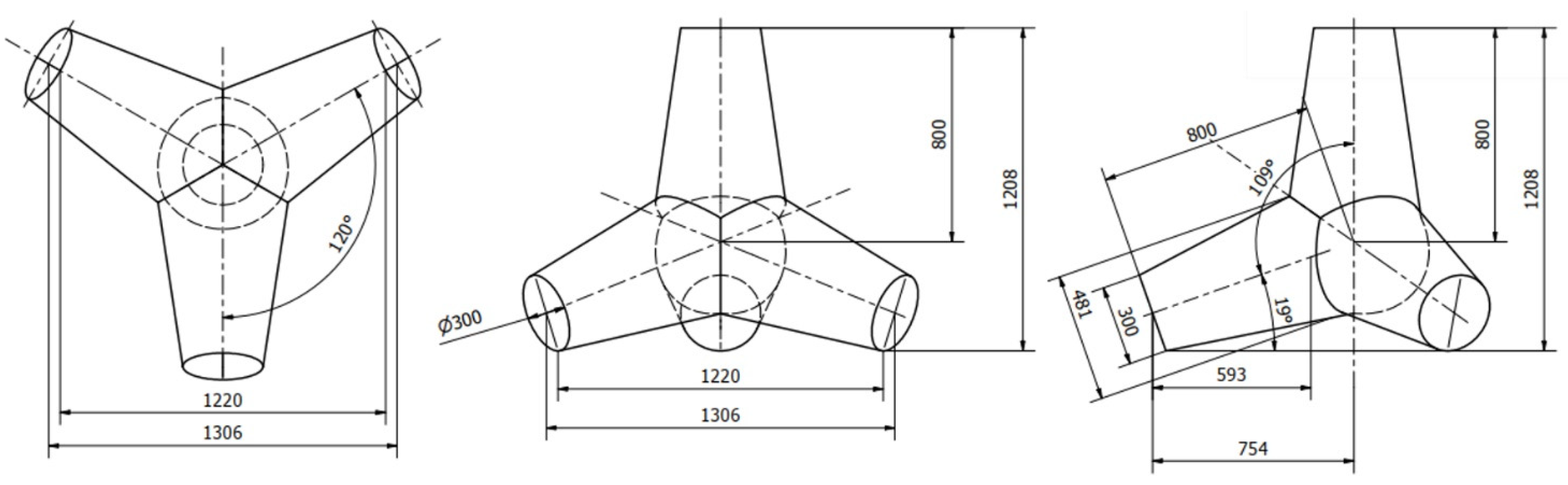
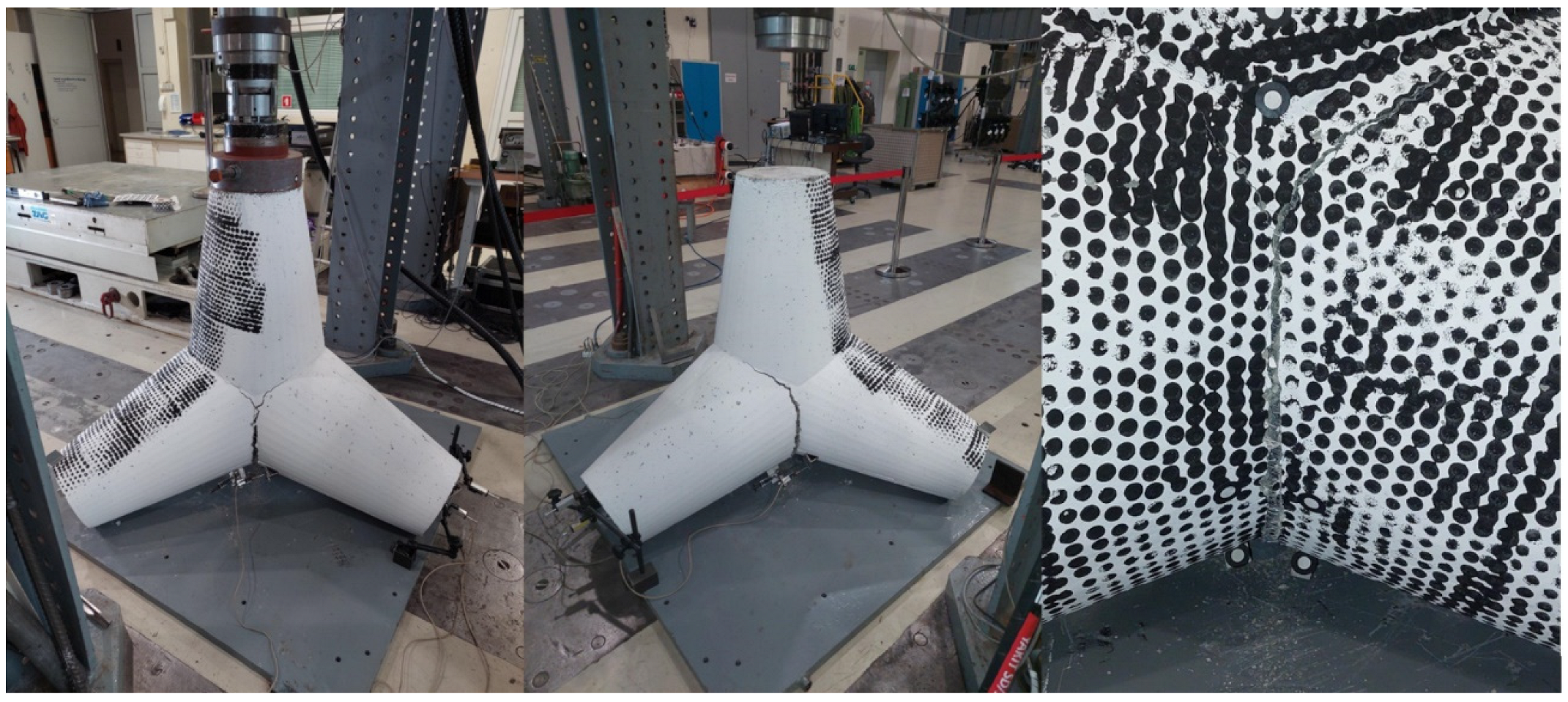
The evaluation of the tetrapod load-bearing capacity is based on the idealization of the tetrapod as a linear structure. The tetrapod legs’ center-lines are considered beams with variable cross-sections. Rotational symmetry is considered, so the static-calculation model is limited to one leg of the tetrapod. It is further assumed that the load (force) is distributed uniformly to the three supported legs. The critical cross-section (the section where the crack occurs) is determined according to the observations from the load tests. As can be seen from
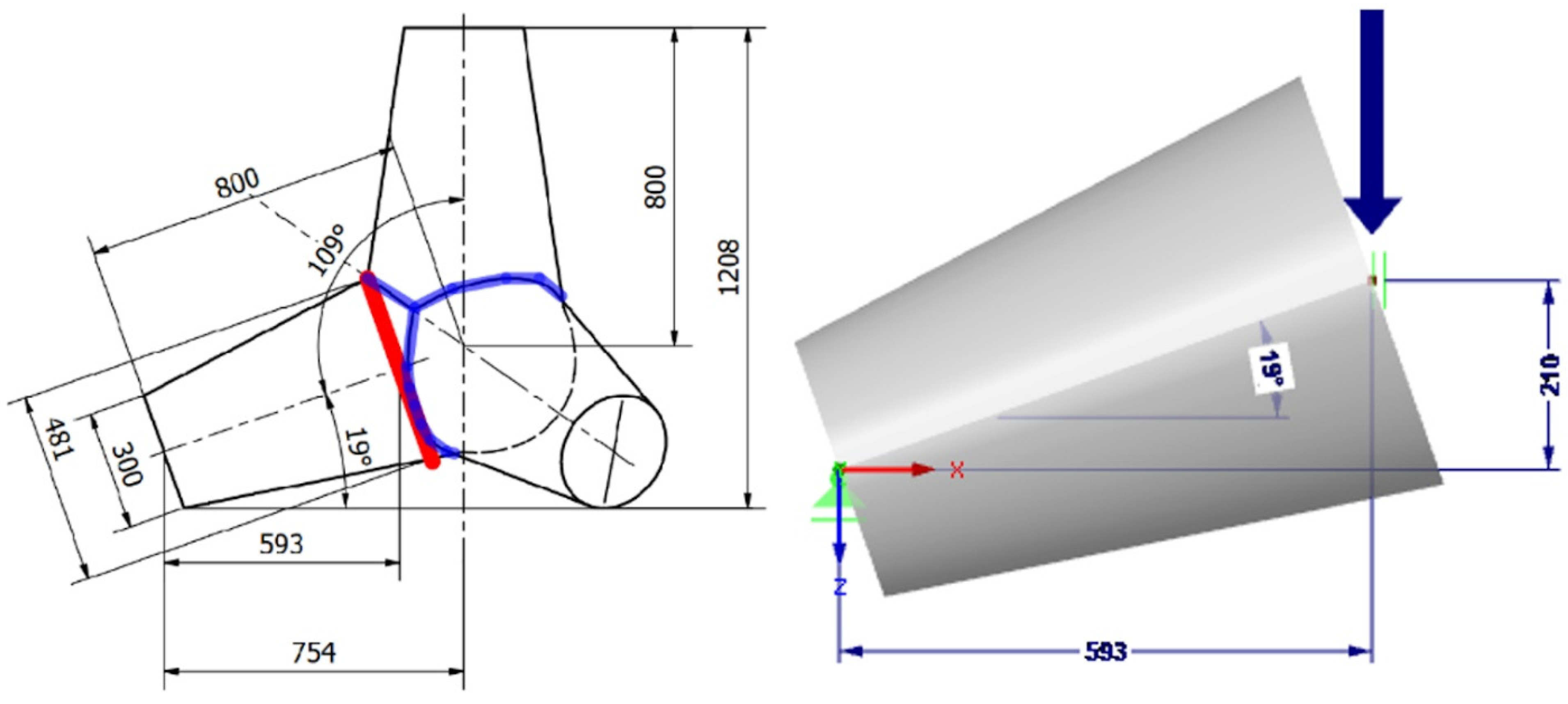
Figure 11, left, the critical cross-section considered in the static-calculation model (marked with a red color) agrees with the actual crack (marked with a blue color) and its location observed during the load tests, especially for small crack-widths, which are the center of interest regarding the residual-load-bearing capacity. The static-calculation model (
Figure 11, right) includes only the part of the leg up to the critical cross-section, while the remaining part, between the critical cross-section and the geometric center of the tetrapod, is considered a non-deformable core.
The left support in
Figure 11, right, is movable in the horizontal direction, while the right support is fixed in the horizontal direction and movable in the vertical direction, and is also prevented from rotating (symmetry conditions). In addition, the frictional force in the left support is considered. The coefficient of friction (
) is taken to be 0.05, which represents an intermediate value for friction between smooth-concrete surfaces and steel surfaces (friction coefficient 0.1) and between smooth-concrete surfaces and painted steel-surfaces (friction coefficient 0.0). The data on friction coefficients are taken from the standard BS 5975 [
21]. For the relationship between the load
(force) and bending moment
of the critical cross-section, the relationship is thus:
where angle α is equal to 19° and length
is equal to 593 mm. The relationship between the shear force,
, and the load is:
Two computational models regarding the constitutive law of (fiber-reinforced) concrete were considered, to calculate the load-bearing capacity at the cross-section level of the tetrapods. The presented equations were based on mechanics, as opposed to those based solely on experimental databases, as were presented in the study [
22], where the axial capacity of fiber-reinforced polymer-bar-reinforced concrete columns was predicted using data-driven machine learning algorithms. The exact data on the effect of the STRUX
® 75/32 fibers on the residual strength of concrete of strength-class C25/30 was unavailable, so both calculations were performed using varying specific parameters for the calculated load-bearing capacities, to match the experimentally determined load-bearing capacities. Thus, the calculation was an inverse analysis (or back-calculation), where the structural resistance is known and material-properties are sought, using a structural model. This definition is given in the fib Model Code for Concrete Structures 2010 [
23]. These parameters were the concrete’s tensile strength and the fiber-reinforced concrete’s residual strength. All other material properties of concrete strength-class C25/30 were taken from the fib Model Code for Concrete Structures 2010 [
23] and the Eurocode 2 [
20]. The back-calculated parameters were then compared with the available data on the material properties, i.e., the tensile strength of concrete of strength-class C25/30 and the estimated residual-strength of concrete of strength-class C25/30 with STRUX
® 75/32 synthetic-polymer fibers. Therefore, if the back-calculated strength values exceed the standard tensile-strength of the used concrete C25/30 and the literature data on the residual strength of concrete C25/30, reinforced with the STRUX
® 75/32 synthetic-polymer fibers, the calculations are conservative—the calculation with the standard/literature tensile and residual-strength data would underestimate the load-bearing capacities.
4.1. Simplified Calculation
To calculate the tensile strength of concrete,
, it was assumed that the stresses vary linearly across the section and that the maximum load-bearing capacity of the tetrapod is reached when, at the lowest point of the cross-section, the tensile stress is equal to the tensile strength of the concrete. In this case, it is necessary to solve the equation:
where
is the tetrapod-leg critical cross-section radius. The simplified residual-load-bearing capacity calculation is based on the assumption of constant tensile stresses in the tension zone of the cross-section (in the fib Model Code for Concrete Structures 2010 [
23], referred to as the “rigid-plastic model”) and on the assumption of constant compressive stresses in the compressive zone of the cross-section (following fib Model Code for Concrete Structures 2010 [
23] and the Eurocode 2 [
20]).
To calculate the residual-strength,
the conditions for the equilibrium of forces (5) and moments (6) in the cross-section must be satisfied:
where the moment equilibrium is set at the lower edge of the cross-section, and
is the resultant of the compressive stresses in the section,
is the resultant of the tensile stresses in the section,
is the lever arm of the resultant of the compressive stresses to the lower edge of the cross-section, and
the lever arm of the tensile-stress resultant to the cross-section bottom edge.
For the resultant of the compressive stresses, the following expression is considered:
where
is the effective compression-zone height-factor equal to 0.8,
is the effective strength-factor equal to 0.9 (reduced by 10%, due to the reduction of the compression zone towards the most compressed edge of the cross-section),
is the mean compressive-strength of the concrete, and x is the height of the compression zone. For the resultant of the tensile stresses, the following expression applies:
For the lever arm of the resultant of the compressive stresses, the following expression is used:
The following expression has been derived for the tensile-stress resultant lever-arm:
Using expressions (1) through (10), we can calculate the unknowns, x and
. The back-calculated results of the calculation can be found in
Table 2. For the “rigid-plastic model” the following equality is considered from the fib Model Code for Concrete Structures 2010 [
23]:
, where
is the residual-strength value at 2.5 mm crack width as defined in the European standard EN 14651 [
24].
4.2. Detailed Calculation
The detailed calculation is based on the fiber-reinforced concrete constitutive-model, as given in the fib Model Code for Concrete Structures 2010 [
23]. It is assumed that fibers do not influence concrete behavior in compression. It is also considered that fibers only start to affect the load-bearing behavior at relatively large tensile-strains. In order to construct a constitutive law for the pre- and post-cracking phase, the residual strengths of concrete with fibers at different crack widths have to be converted into stress–strain relationships. In the fib Model Code for Concrete Structures 2010 [
23], a recommendation is made that, for fiber-reinforced concrete elements which show strain-softening behavior (relaxation of the fiber-reinforced concrete, parallel to the increase of the crack width), the characteristic structural length,
, can be taken as the height of the cross-section, i.e., in the present case, the diameter of the critical cross-section of the tetrapod leg. For the relation between the strain, ε, and the crack width, w, the expression
is valid. For calculation, the tensile-strain limit,
, at which the influence of the fibers begins to be taken into account, at least computationally, is, according to the fib Model Code for Concrete Structures 2010 [
23], calculated with the expression:
where
is the residual strength at a crack width of 0.5 mm,
the residual strength at a crack width of 2.5 mm,
the tensile strain of the concrete just before (macro) crack formation equal to
,
the strain at crack width 0.5 mm,
the strain at crack width 2.5 mm, and
the strain of the concrete without fibers after crack formation at a stress equal to 0.2 times the tensile strength of the concrete. Examples of constitutive laws for the fiber-reinforced (specimen L22033_2_1) and unreinforced (specimen L22058_2_1) concrete, are shown in
Figure 12.
The constitutive laws of the fiber-reinforced (specimen L22033_2_1) and unreinforced (specimen L22058_2_1) concrete shown in
Figure 12 clearly depict the fact that the fibers do not affect (as regards the calculation) the compressive behavior of concrete (
Figure 12a); however, it is evident from
Figure 12b that the fibers affect the residual tensile strength of concrete, which is nonexistent for large strains and approximately larger than 1‰ for the given case, as can be seen in
Figure 12c.
The method of dividing the cross-section into layers was employed. It was decided to divide the cross-section into 1500 layers. The thickness of each layer (of the critical cross-section) is:
where
is the diameter of the critical cross-section of the tetrapod leg. The width of each layer is:
where
is the center of gravity of each layer measured from the bottom edge of the cross-section, and is calculated by:
where i is the index of each layer, (i are numbers between 1 and 1500).
The relationship between the curvature of the cross-section and the load-bearing capacity of each tetrapod was calculated. The detailed calculation included the bisection method, which was employed to calculate the strain at the bottom edge of the cross-section at different values of cross-section curvature. The corresponding loads (forces) were calculated for the different curvatures. The expressions (1–3) and the equations of equilibrium of forces and moments in the cross-section were used:
where
is the stress in the i-th layer of the cross-section (read for the deformation of the i-th layer,
, from the stress–strain diagram,
Figure 12). The calculation was carried out as explained above, by looking for the tensile strength of the concrete,
, the residual strength at a crack width of 0.5 mm,
and the residual strength at a crack width of 2.5 mm,
. The calculation of the stress–strain relationship was repeated until the experimental and calculated maximum load-bearing capacities, the residual-load capacities at a crack width of 0.5 mm, and the residual-load-bearing capacities at a crack width of 2.5 mm, were in agreement. The back-calculated strength-value results of the calculation are given in
Table 3, while the corresponding bending-moment-versus-curvature relations are given in
Figure 13.
To calculate the residual strengths,
and
in
Table 3, the relationships presented in [
25] were applied, as the corresponding relationships in the fib Model Code for Concrete Structures 2010 [
23] are very simplified and, in the case at hand, when
and
are calculated from
and
the calculation returns inappropriate results. The expressions used are:
4.3. Estimated Residual-Strength Values of Concrete C25/30 Reinforced with STRUX® 75/32 Fibers
No specific values for the residual strengths of concrete of strength-class C25/30 reinforced with STRUX
® 75/32 fibers were found. However, the results of bending tests (in line with the European standard EN 14889-2 [
18] and EN 14651 [
24]) of concrete strength-class C40/50 beams with different fiber dosages (four beams per dosage) of STRUX
® 75/32 fibers were available in [
26]. Furthermore, indicative values of the equivalent residual-strengths (according to the standard ASTM C 1018-97 [
27]) of concretes of different strength-classes and with different dosages of STRUX
® 90/40 fibers were also available in [
28]. It was assumed that the effect of the concrete strength-class on the differently defined residual-strengths (equivalent or at specific crack-width) is similar. Furthermore, STRUX
® 75/32 and STRUX
® 90/40 fibers are both made of the same material, but have different lengths (32 mm and 40 mm) and similar effective-diameters, as seen from the document [
29]. It was thus also assumed that the relationships of residual strengths to concrete-strength classes of concretes reinforced with STRUX
® 90/40 fibers are similar to the relationships of residual strengths to concrete strength-classes of concretes reinforced with STRUX
® 75/32 fibers. The estimated residual-strengths of concrete of strength-class C25/30 with STRUX
® 75/32 synthetic-polymer fibers were calculated using linear interpolation. The STRUX
® 75/32 data (for concrete strength-class C40/50) was used to interpolate the estimated strengths according to the chosen fiber-dosage, and the STRUX
® 90/40 equivalent residual-strength data was used to interpolate the estimated values according to the strength-class of the concrete. Interpolated estimated residual-strength values
(at 0.5 mm crack width) and
(at 2.5 mm crack width) as defined by the European standard EN 14651 [
24], are summarized in
Table 4. It should be noted that the residual strengths
, according to the European standard EN 14651 [
24], are fictitious strengths, since they are calculated on the assumption of a linear stress-distribution in the cross-section of the beams.
4.4. Comparison of the Calculated (Based on the Load Tests) Strength Values with Strength Values Available from the Literature
The tetrapods were made of concrete of strength-class C25/30, for which (according to Eurocode 2 [
20]) the 5% fractile-tensile strength is equal to 1.8 MPa, the mean tensile-strength equal to 2.6 MPa, and the 95% fractile-tensile strength equal to 3.3 MPa. The comparison with the back-calculated tensile strengths of the simplified calculation (results in
Table 2) shows that the simplified calculation is rather conservative—tensile-strength values close to or even higher than the 95% fractile-tensile strength of concrete C25/30 are needed for the calculated maximum load-bearing capacity to match the experimentally determined maximum load-bearing capacity. Comparing the concrete C25/30 tensile-strength values with the back-calculated tensile-strength values of the detailed calculation (results in
Table 3) shows that the detailed calculation gives more accurate results. In order for the calculated maximum load-bearing capacity to match the experimentally determined maximum load-bearing capacity, a tensile strength close to the 5% fractile-tensile strength of the concrete strength-class C25/30 is needed, except for specimen L22033_2_2, for which a value close to the 95% fractile-tensile strength of the concrete strength-class C25/30 is needed. The comparison of the (from the literature) estimated residual-strengths
and
(
Table 4) with the calculated ones shows that both the simplified and the detailed calculations are conservative, since residual-strength values of fiber-reinforced concrete of strength-class C25/30 close to or higher than the estimated residual-strength values
and
from
Table 4 are needed for the calculated residual load-bearing capacities
and
to match the experimentally observed residual load-bearing capacities. Furthermore, the comparison of the calculated residual-strength values
from
Table 2 and
Table 3, indicates that the rigid-plastic constitutive model (
Section 4.1) and the consideration of the more realistic constitutive model (
Section 4.2) of fiber-reinforced concrete, give very similar results (differences of less than 3%). It means that the rigid-plastic model can be applied for calculating the residual-load-bearing capacities of fiber-reinforced concrete circular cross-sections without loss of accuracy, compared to a calculation with more realistic stress-distributions in the cross-section.
4.5. Load-Displacement Calculation and Comparison with Load Test Results
The load-displacement behavior of the tetrapod L22033_2_1 was calculated using the same static model as for the load-bearing-capacity calculation (
Figure 11, right). A numeric algorithm was written where, in the first step, the tetrapod leg was divided into 100 segments and where for each segment cross-section, the bending-moment-versus-curvature relation was calculated (as for the critical cross-section in
Section 4.2). The total deflection was calculated as the sum of the deflection coming from the flexural, shear, and axial deformation of the tetrapod leg and the axial deformation of the vertical (axially loaded) tetrapod leg.
The flexural deflection was calculated by numerically integrating (the trapezoidal rule) the curvature along the tetrapod leg with virtual bending-moments resulting from a virtual unit-force at the location, and oriented in the direction of the actual tetrapod-loading during the load tests. For each calculated curvature and the corresponding bending moment of the critical cross-section, the corresponding curvatures along the tetrapod leg were calculated, taking into consideration the fact that the bending moments vary linearly along the tetrapod leg and are a function of the segment location along the tetrapod leg. The shear deflections were calculated by integrating the shear forces with virtual shear-forces and considering the shear correction-factor (κ
S) as equal to 0.9 (as per [
30], for example). The deflections resulting from axial forces were calculated by integrating the axial forces with the virtual axial-forces. Variation of the cross-section area along the tetrapod leg was considered. The following equation was used for calculating the deflection Δ (for different loads):
where κ
fl,i are curvatures at different segment cross-sections along the tetrapod leg. Index 0 denotes the cross-section at the beginning of the tetrapod leg, and index 100 (99+1) denotes the critical cross-section of the tetrapod leg.
are virtual bending-moments at different segment cross-sections, L
seg is the length of the segments, L
leg is the length of the tetrapod leg, N is the axial force in the tetrapod leg,
is the virtual axial-force in the tetrapod leg, E
cm is the mean elastic-modulus of the used concrete-strength-class, A(x) is the tetrapod-leg cross-section area at location x, V is the shear force in the tetrapod leg,
is the virtual shear-force in the tetrapod leg, ν is the Poisson’s ratio for concrete (0.2), and F
pre the different load (force) steps.
This procedure was, however, only appropriate up to curvatures of the critical cross-section corresponding to a bending-moment less than or equal to the maximum bending-moment from the bending-moment-versus-curvature relation from
Figure 13. For larger curvatures, the plastic-hinge approach was applied. Many studies state different values for the appropriate plastic-hinge lengths, as shown in [
31], where different studies’ plastic-hinge lengths (also called characteristic or structural-characteristic length) range from half of the cross-section height to twice the cross-section height for steel-fiber-reinforced concrete. As steel-fiber-reinforced-concrete design procedures are commonly also applied to polymer-fiber-reinforced concrete, the plastic-hinge length applied for the deflection calculation in this study was chosen as equal to the diameter of the critical cross-section. After all, this value was already used for transforming the stress-versus-crack-width relation into the stress-versus-strain relation in
Section 4.2. However, for the present case of three bent tetrapod-legs (the fourth is only axially loaded) joined at one location, the plastic-hinge length for beams translates to half of the chosen plastic-hinge length at the end of each of the three bent tetrapod-legs.
For the tetrapod leg-segments inside the plastic-hinge length, a constant curvature equal to the curvature of the critical cross-section, was considered. According to [
32], this is a valid assumption for fiber-reinforced concrete elements with tensile-softening behavior, as was the case in this study. For tetrapod leg-segments outside the plastic hinge, the curvature for a specific bending-moment acting on the segment cross-section was read (from
Figure 13) from the quasi-elastic part of the bending-moment-versus-curvature relation curve (part of the curve from zero curvature up to curvature corresponding to the maximum bending-moment of the segment cross-section).
After reaching the maximum load-bearing capacity of the tetrapod, or for loading after reaching the curvature corresponding to the maximum bending-moment of the critical cross-section, the shear deflection consisted of two parts: shear deflection of the tetrapod leg outside the plastic-hinge (calculated as before) and shear deflection of the plastic-hinge part. A shear area equal to the uncracked critical cross-section area multiplied by the shear correction-factor (κ
S) was considered for the integration of the shear forces with virtual shear-forces for the plastic-hinge part. The crack depth was calculated based on the assumption that the layers of the critical cross-section with tensile strains larger than 0.15‰ are cracked. The portion of the total deflection resulting from flexural and shear deformations (Δ
fl+s) of the plastic-hinge part was calculated with:
where L
P is the plastic-hinge length,
and
the virtual bending-moments at the beginning and the end of the plastic-hinge part, κ
fl.
cs the curvature of the critical cross-section (considered as uniform curvature along the plastic-hinge length), and A
cs.
uncr. the uncracked area of the critical cross-section. The deflection resulting from the tetrapod parts outside the plastic-hinge length is calculated according to Equation (19), but with accordingly lower integration-lengths. The deflection calculations with Equations (19) and (20) were performed at each load (force) step.
In order to compare the experimental and calculated load-deflection relations, the displacements of the supports had to be subtracted from the total deflection (deflection of point T1 in
Figure 3). Only the support displacements of two tetrapod legs were monitored (points T9 and T11 in
Figure 3). However, as the displacements were measured in three dimensions, the measured displacements were used to estimate the unmonitored leg-support-point displacement (point T12 in
Figure 14). It was assumed that the displacement of point T1 in the vertical direction was mainly the result of the support-point displacements (displacements of points T9, T11, T12) and that the displacements of point T1 result from rotations about the three axes going through the ground-contact points (G9, G11, and G12), as shown in
Figure 14.
By knowing the coordinates of the monitored points and the unmonitored support-point (given in
Table 5), an algorithm was written to estimate the displacements of the support-point T12. The Rodrigues’ rotation matrix (
) formula [
33] was applied:
where θ denotes the rotation angle, and ῶ denotes the antisymmetric matrix:
ω
x, ω
y, and ω
z are components of the unit vector, which specifies the axis of rotation. For each step of the measurement data (measured displacements of point T1 for each load stage), the rotation angles (θ
1, θ
2, θ
3) about the three axes going through the support points were calculated by applying the equality condition of the measured displacements being the same as the calculated displacements of point T1 (with coordinates from
Table 5), due to the three rotations θ
1, θ
2, and θ
3. The rotation-axes unit vectors were calculated from the coordinates of points G9, G11, and G12, from
Table 5. The three rotation angles and Rodrigues’-rotation-matrix formula were then used to calculate the estimated displacement of the unmonitored support-point T12 (based on the coordinates of point T12, from
Table 5).
The average of the vertical displacements of the three support points was then subtracted from the measured vertical-displacement of point T1, to extract the deflection portion resulting from the flexural, shear, and axial deformations of the tetrapod legs. The comparison of the measured and calculated load-deflection response can be seen in
Figure 15.
A comparison of the load-displacement relations of the tetrapod L22033_2_1 in
Figure 15, shows reasonable agreement between the experimental and calculated results. The most significant difference is in the region of displacements less than or equal to the displacement corresponding to the maximum load-bearing capacity. This difference is most likely the result of considering standard material-properties of the specific concrete-strength-class as input parameters for the calculation. However, the post-maximum load-bearing-capacity deflection region (residual load-bearing region of the load-displacement behavior) shows much better agreement between the experimental and calculated results. The latter is another indication of adequate evaluation of the residual strengths of the fiber-reinforced concrete at different crack widths. In future research, the load-displacement results can be used in combination with numerical tools considering fracture and dynamic effects, such as the combined finite–discrete element method (FDEM) in the studies [
34,
35], where the FDEM method was applied to different structural problems.