Accurate Computation of Mutual Inductance of Non Coaxial Pancake Coils
Abstract
1. Introduction
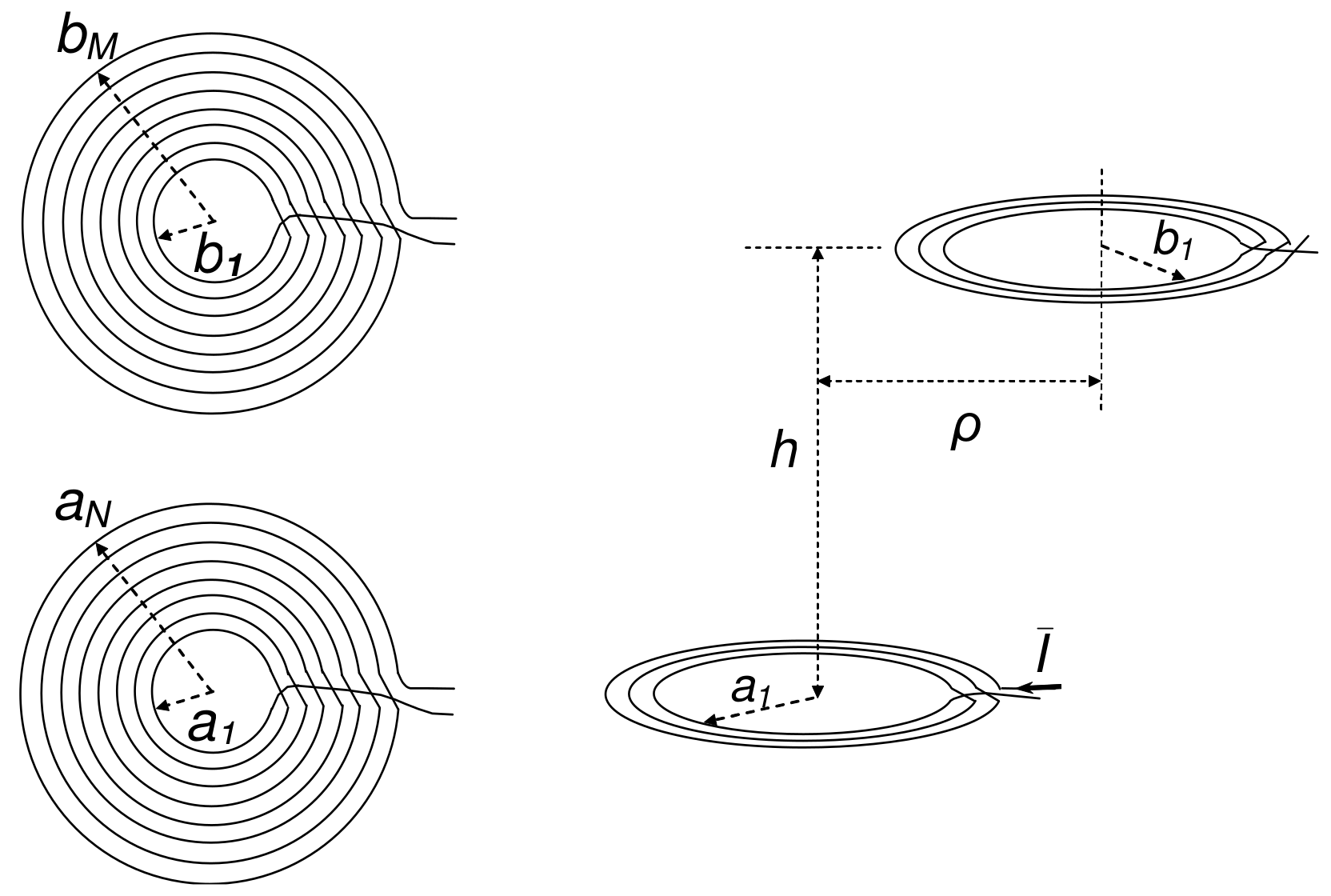
2. Theory
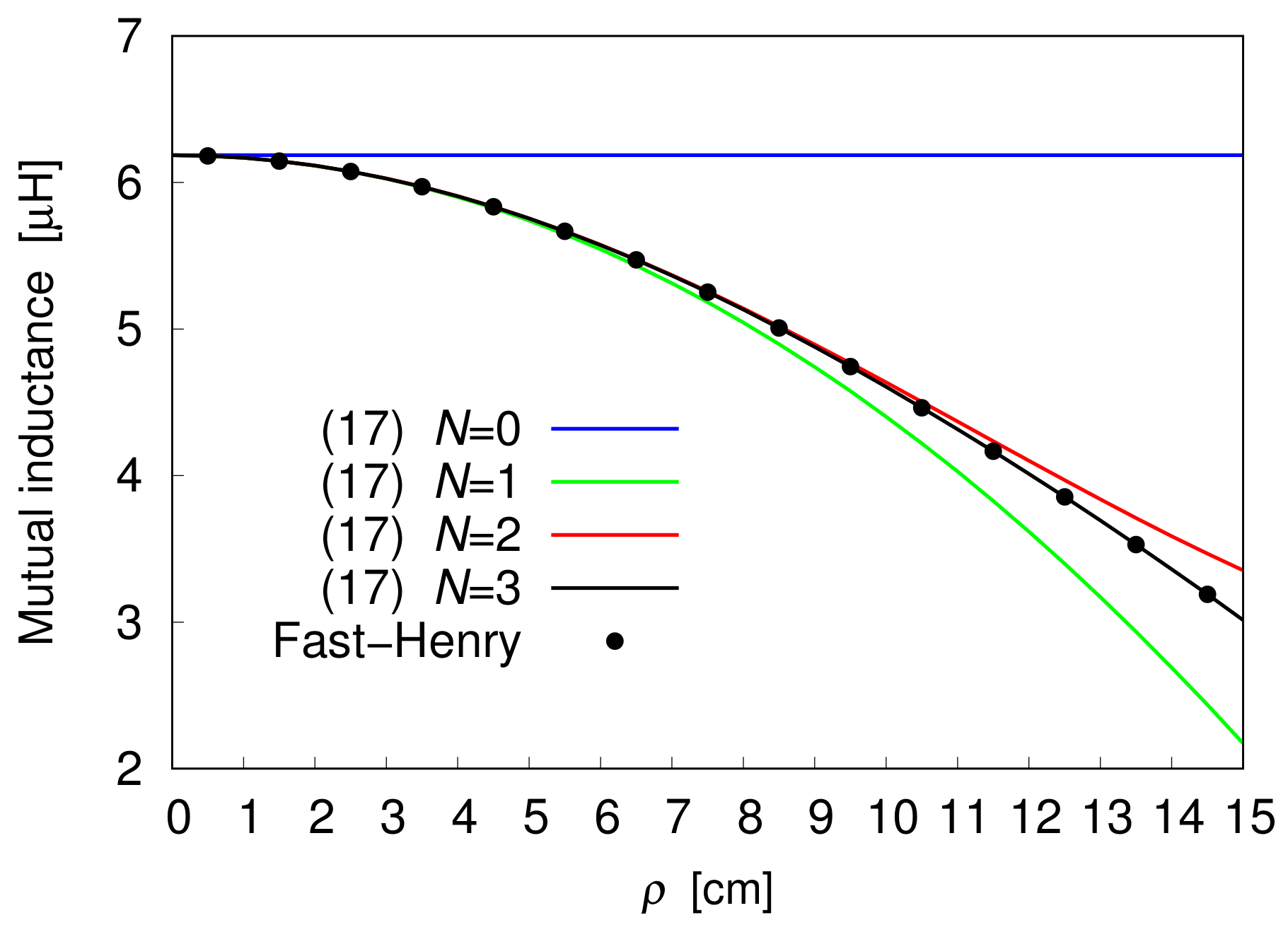
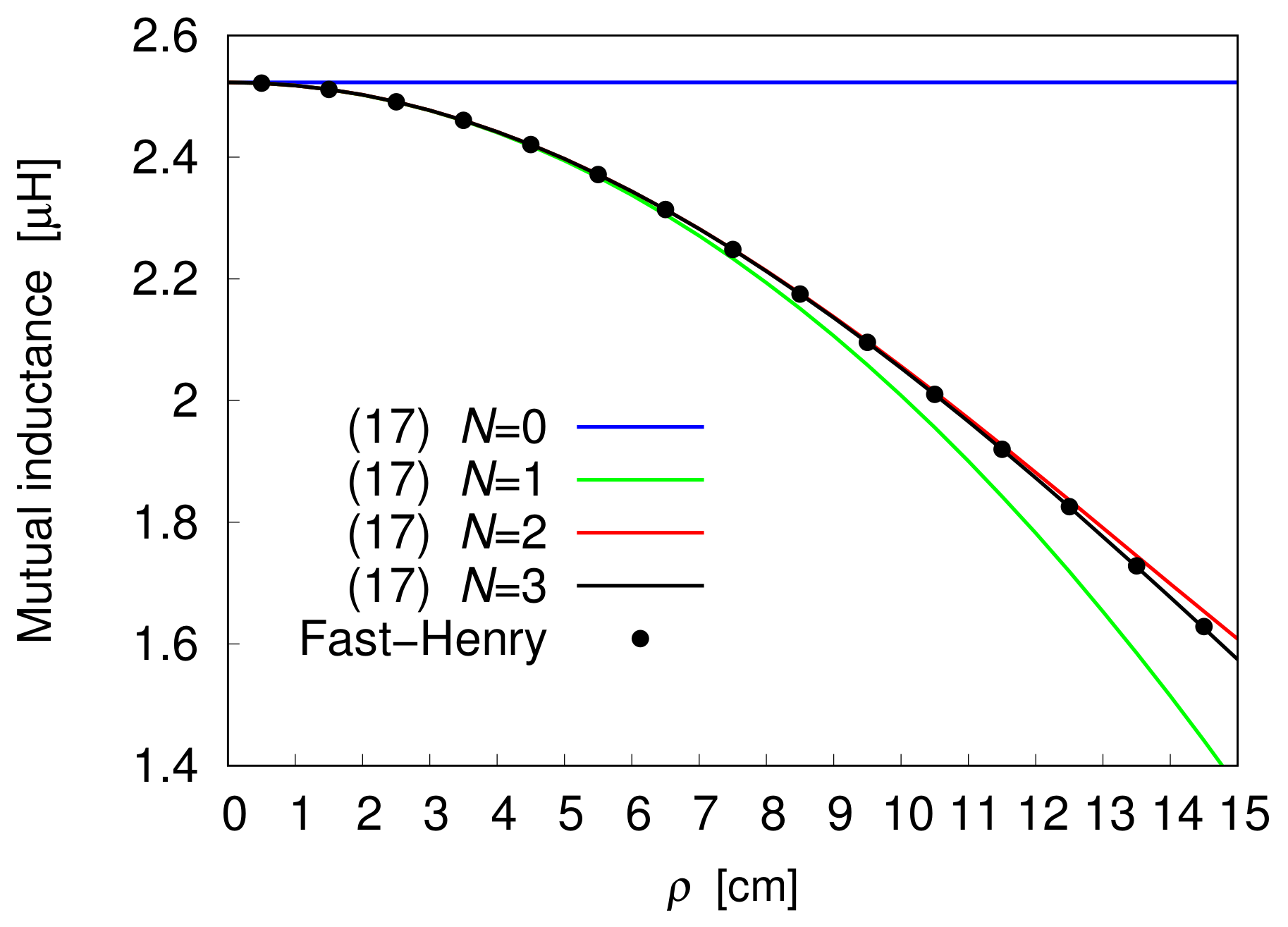
3. Results and Discussion
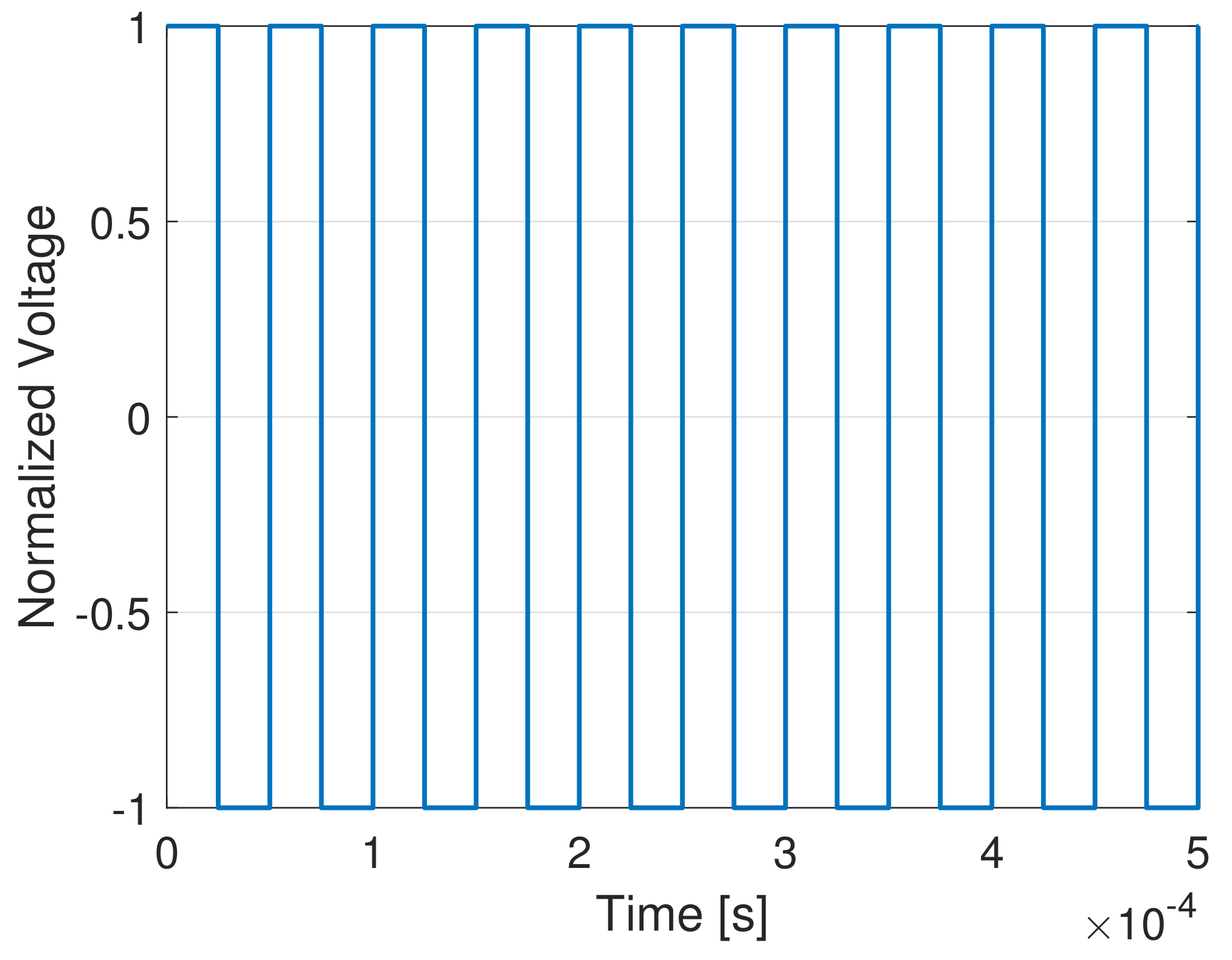
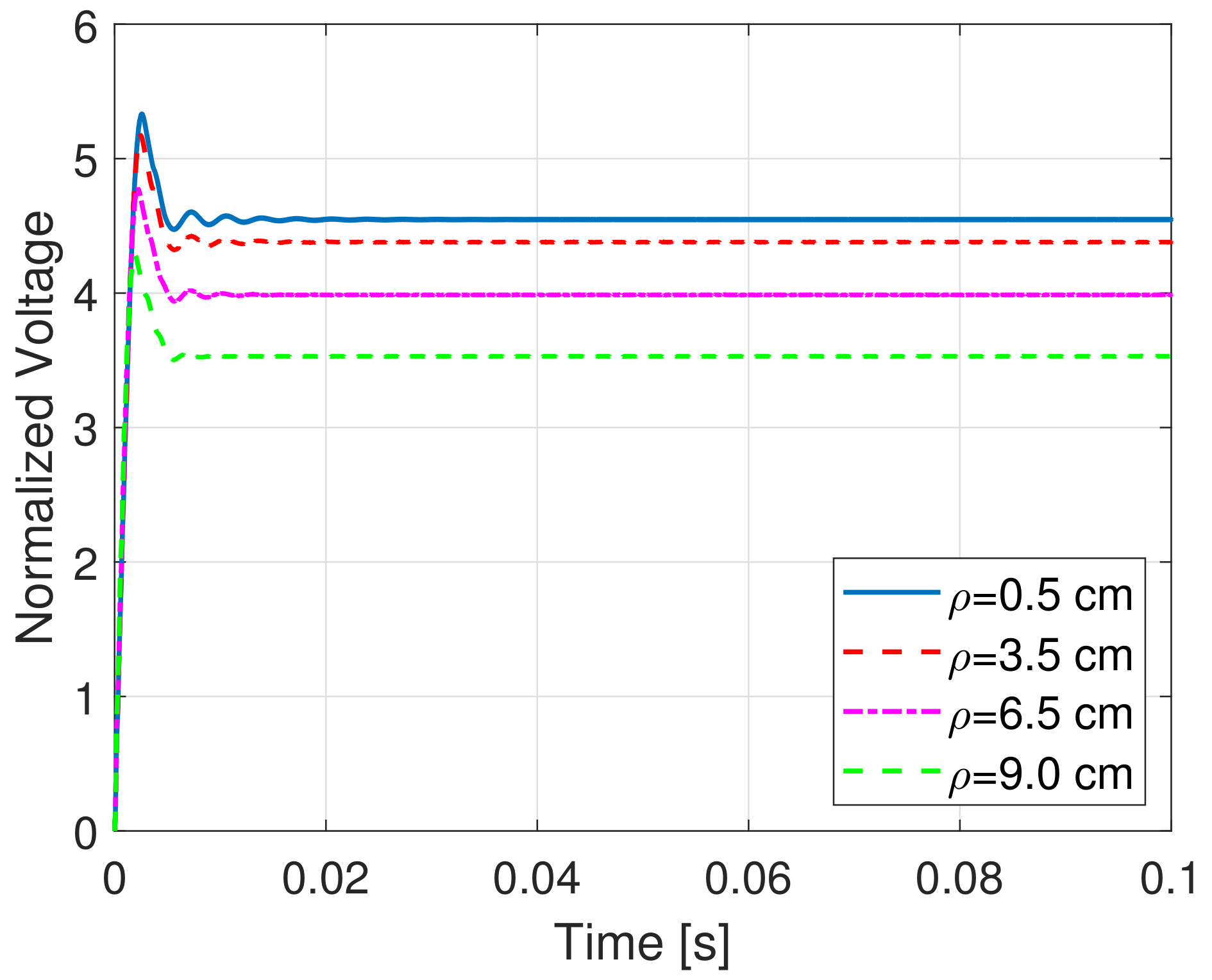
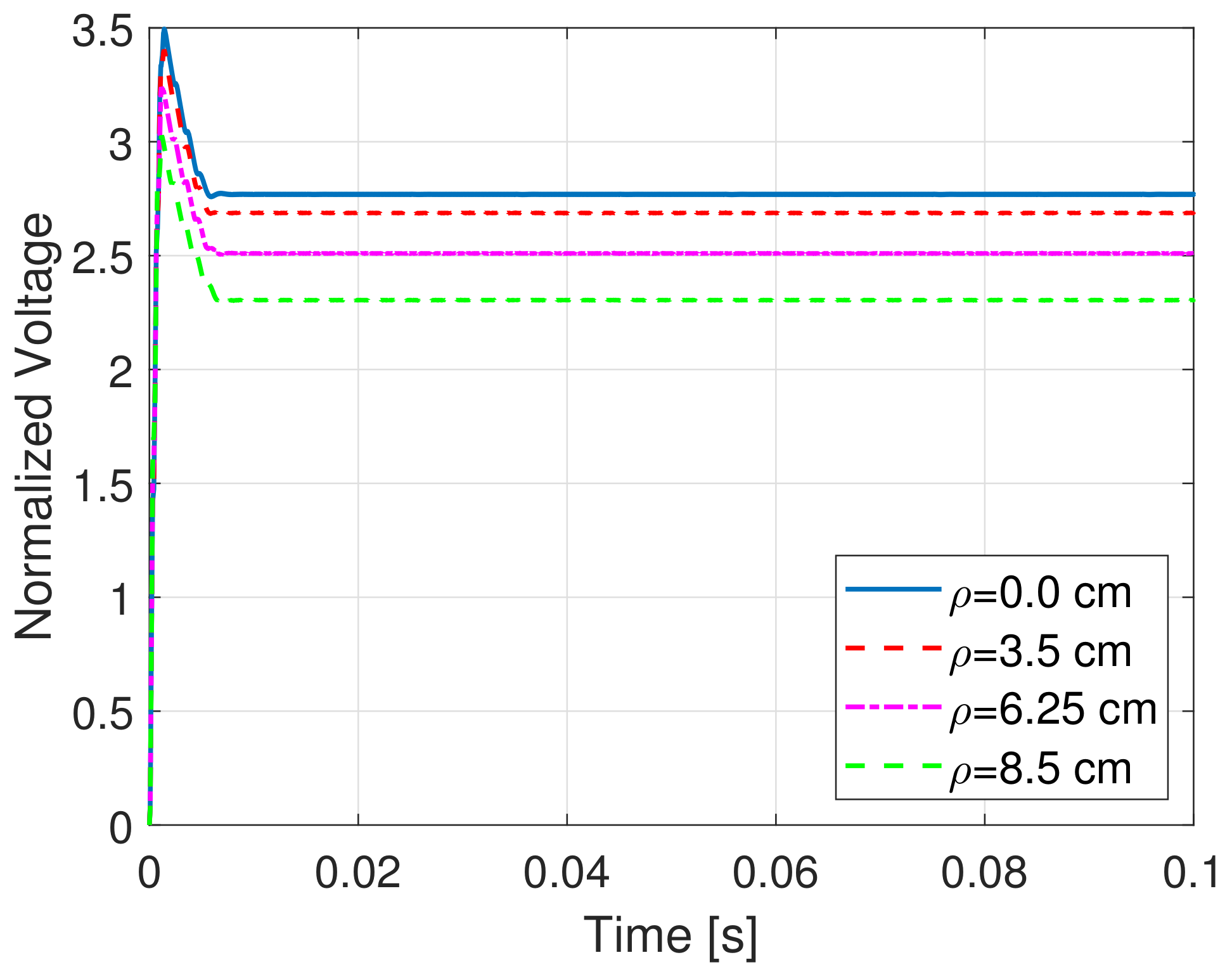
4. WPT System with a Full Bridge Inverter
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| MDPI | Multidisciplinary Digital Publishing Institute |
| DOAJ | Directory of open access journals |
| EM | Electromagnetic |
| PE | Power electronics |
| PES | Power electronic systems |
| HF | High Frequency |
| LF | Low Frequency |
| SiC | Silicon Carbide |
| PEEC | Partial Element Equivalent Circuit |
| FEM | Finite Element |
| 3-D | Three-dimensional |
References
- Maxwell, J.C. A Treatise on Electricity and Magnetism; Dover: New York, NY, USA, 1954. [Google Scholar]
- Butterworth, S. On the coefficients of mutual induction of eccentric coils. Philos. Mag. J. Sci. 1916, 31, 443–454. [Google Scholar] [CrossRef]
- Snow, C. Formulas for Computing Capacitance and Inductance; Bureau of Standards Circular 544: Washington, DC, USA, 1954. [Google Scholar]
- Babic, S.; Sirois, F.; Akyel, C.; Girardi, C. Mutual Inductance Calculation Between Circular Filaments Arbitrarily Positioned in Space: Alternative to Grover’s Formula. IEEE Trans. Magn. 2010, 46, 3591–3600. [Google Scholar] [CrossRef]
- Babic, S.; Akyel, C. New analytic-numerical solutions for the mutual inductance of two coaxial circular coils with rectangular cross section in air. IEEE Trans. Magn. 2006, 42, 1661–1669. [Google Scholar] [CrossRef]
- Babic, S.I.; Akyel, C. Calculating Mutual Inductance Between Circular Coils With Inclined Axes in Air. IEEE Trans. Magn. 2008, 44, 1743–1750. [Google Scholar] [CrossRef]
- Conway, J.T. Inductance calculations for noncoaxial coils using Bessel functions. IEEE Trans. Magn. 2007, 43, 1023–1034. [Google Scholar] [CrossRef]
- Conway, J.T. Noncoaxial Inductance Calculations Without the Vector Potential for Axisymmetric Coils and Planar Coils. IEEE Trans. Magn. 2008, 44, 453–462. [Google Scholar] [CrossRef]
- Engel, T.; Rohe, S. A comparison of single-layer coaxial coil mutual inductance calculations using finite-element and tabulated methods. IEEE Trans. Magn. 2006, 42, 2159–2163. [Google Scholar] [CrossRef]
- Cirimele, V.; Torchio, R.; Virgillito, A.; Freschi, F.; Alotto, P. Challenges in the Electromagnetic Modeling of Road Embedded Wireless Power Transfer. Energies 2019, 12, 2677. [Google Scholar] [CrossRef]
- Su, Y.P.; Liu, X.; Hui, S.Y.R. Mutual Inductance Calculation of Movable Planar Coils on Parallel Surfaces. IEEE Trans. Power Electron. 2009, 24, 1115–1123. [Google Scholar] [CrossRef]
- Sonntag, C.L.W.; Lomonova, E.A.; Duarte, J.L. Implementation of the Neumann formula for calculating the mutual inductance between planar PCB inductors. In Proceedings of the 2008 18th International Conference on Electrical Machines, Vilamoura, Portugal, 6–9 September 2008; pp. 1–6. [Google Scholar] [CrossRef]
- Tang, S.; Hui, S.; Chung, H. A low-profile wide-band three-port isolation amplifier with coreless printed-circuit-board (PCB) transformers. IEEE Trans. Ind. Electron. 2001, 48, 1180–1187. [Google Scholar] [CrossRef]
- Ravaud, R.; Lemarquand, G.; Lemarquand, V. Force and Stiffness of Passive Magnetic Bearings Using Permanent Magnets. Part 1: Axial Magnetization. IEEE Trans. Magn. 2009, 45, 2996–3002. [Google Scholar] [CrossRef]
- Ravaud, R.; Lemarquand, G.; Lemarquand, V. Force and Stiffness of Passive Magnetic Bearings Using Permanent Magnets. Part 2: Radial Magnetization. IEEE Trans. Magn. 2009, 45, 3334–3342. [Google Scholar] [CrossRef]
- Keyi, Z.; Bin, L.; Zhiyuan, L.; Shukang, C.; Ruiping, Z. Inductance Computation Consideration of Induction Coil Launcher. IEEE Trans. Magn. 2009, 45, 336–340. [Google Scholar] [CrossRef]
- Engel, T.G.; Mueller, D.W. High-Speed and High-Accuracy Method of Mutual-Inductance Calculations. IEEE Trans. Plasma Sci. 2009, 37, 683–692. [Google Scholar] [CrossRef]
- Kajikawa, K.; Yokoo, R.; Tomachi, K.; Enpuku, K.; Funaki, K.; Hayashi, H.; Fujishiro, H. Numerical Evaluation of Pulsed Field Magnetization in a Bulk Superconductor Using Energy Minimization Technique. IEEE Trans. Appl. Supercond. 2008, 18, 1557–1560. [Google Scholar] [CrossRef]
- Babic, S.; Martinez, J.; Akyel, C.; Babic, B. Mutual Inductance Calculation between Misalignment Coils for Wireless Power Transfer of Energy. Prog. Electromagn. Res. M 2014, 38, 91–102. [Google Scholar] [CrossRef]
- Luo, Z.; Wei, X. Mutual Inductance Analysis of Planar Coils with Misalignment for Wireless Power Transfer Systems in Electric Vehicle. In Proceedings of the 2016 IEEE Vehicle Power and Propulsion Conference (VPPC), Hangzhou, China, 17–20 October 2016; pp. 1–6. [Google Scholar] [CrossRef]
- Liu, S.; Su, J.; Lai, J. Accurate Expressions of Mutual Inductance and Their Calculation of Archimedean Spiral Coils. Energies 2019, 12, 2017. [Google Scholar] [CrossRef]
- Zierhofer, C.M.; Hochmair, E.S. Geometric approach for coupling enhancement of magnetically coupled coils. IEEE Trans. Biomed. Eng. 1996, 43, 708–714. [Google Scholar] [CrossRef]
- Parise, M.; Antonini, G.; Romano, D. On the Flux Linkage between Pancake Coils in Resonance-Type Wireless Power Transfer Systems. Int. J. Antennas Propag. 2020, 2020, 1–6. [Google Scholar] [CrossRef]
- Simonazzi, M.; Sandrolini, L.; Zarri, L.; Reggiani, U.; Alberto, J. Model of Misalignment Tolerant Inductive Power Transfer System for EV Charging. In Proceedings of the 2020 IEEE 29th International Symposium on Industrial Electronics (ISIE), Delft, The Netherlands, 17–19 June 2020; pp. 1617–1622. [Google Scholar] [CrossRef]
- Fontana, N.; Brizi, D.; Barmada, S.; Monorchio, A. A Methodology for Efficiency Recovering in Wireless Power Transfer Applications with Misalignment. In Proceedings of the 2020 XXXIIIrd General Assembly and Scientific Symposium of the International Union of Radio Science, Rome, Italy, 29 August–5 September 2020. [Google Scholar] [CrossRef]
- ElGhanam, E.; Hassan, M.; Osman, A.; Kabalan, H. Design and Performance Analysis of Misalignment Tolerant Charging Coils for Wireless Electric Vehicle Charging Systems. World Electr. Veh. J. 2021, 12, 89. [Google Scholar] [CrossRef]
- Steckiewicz, A.; Stankiewicz, J.M.; Choroszucho, A. Numerical and Circuit Modeling of the Low-Power Periodic WPT Systems. Energies 2020, 13, 2651. [Google Scholar] [CrossRef]
- Tal, N.; Morag, Y.; Levron, Y. Magnetic Induction Antenna Arrays for MIMO and Multiple-Frequency Communication Systems. Prog. Electromagn. Res. C 2017, 75, 155–167. [Google Scholar] [CrossRef][Green Version]
- Miller, J.M.; Onar, O.C.; Chinthavali, M. Primary-Side Power Flow Control of Wireless Power Transfer for Electric Vehicle Charging. IEEE J. Emerg. Sel. Top. Power Electron. 2015, 3, 147–162. [Google Scholar] [CrossRef]
- Kamon, M.; Tsuk, M.J.; White, J.K. FASTHENRY: A multipole-accelerated 3-D inductance extraction program. IEEE Trans. Microw. Theory Tech. 1994, 42, 1750–1758. [Google Scholar] [CrossRef]
- Abramowitz, M.; Stegun, I.A. Handbook of Mathematical Functions: With Formulas, Graphs, and Mathematical Tables; Courier Corporation: Washington, DC, USA, 1964; Volume 55. [Google Scholar]
- Watson, G.N. A Treatise on the Theory of Bessel Functions; Cambridge mathematical library, Cambridge University Press: Cambridge, UK, 1944. [Google Scholar]
- Erdelyi, A. Tables of Integral Transforms; McGraw-Hill: New York, NY, USA, 1954; Volume 1. [Google Scholar]
- Gradshteyn, I.S.; Ryzhik, I.M. Table of Integrals, Series, and Products; Academic Press: New York, NY, USA, 2007. [Google Scholar]
- Paul, C.R. Inductance: Loop and Partial; John Wiley & Sons: Hoboken, NJ, USA, 2010. [Google Scholar]
- Rim, C.T.; Mi, C. Wireless Power Transfer for Electric Vehicles and Mobile Devices; JohnWiley & Sons: Hoboken, NJ, USA, 2017. [Google Scholar]




| Switching frequency | kHz |
| Primary capacitor | F |
| Primary resistance | m |
| Secondary capacitor | F |
| Secondary resistance | m |
| Self inductances | H |
| Load capacitor | F |
| Load resistance | |
| Conductors radius | mm |
| Distance h [cm] | Misalignment [cm] | [H] |
|---|---|---|
| 10 | ||
| 10 | ||
| 10 | ||
| 10 | 9 | |
| 15 | 0 | |
| 15 | ||
| 15 | ||
| 15 | ||
| 20 | 0 | |
| 20 | ||
| 20 | 6 | |
| 20 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Parise, M.; Loreto, F.; Romano, D.; Antonini, G.; Ekman, J. Accurate Computation of Mutual Inductance of Non Coaxial Pancake Coils. Energies 2021, 14, 4907. https://doi.org/10.3390/en14164907
Parise M, Loreto F, Romano D, Antonini G, Ekman J. Accurate Computation of Mutual Inductance of Non Coaxial Pancake Coils. Energies. 2021; 14(16):4907. https://doi.org/10.3390/en14164907
Chicago/Turabian StyleParise, Mauro, Fabrizio Loreto, Daniele Romano, Giulio Antonini, and Jonas Ekman. 2021. "Accurate Computation of Mutual Inductance of Non Coaxial Pancake Coils" Energies 14, no. 16: 4907. https://doi.org/10.3390/en14164907
APA StyleParise, M., Loreto, F., Romano, D., Antonini, G., & Ekman, J. (2021). Accurate Computation of Mutual Inductance of Non Coaxial Pancake Coils. Energies, 14(16), 4907. https://doi.org/10.3390/en14164907







