3.1. FEM Simulation Results
Figure 5 shows exemplary flux density simulation results obtained with the model.
It is important to note that the used steel saturates rapidly above approximately 1.25 T (see
Figure 4). The first field plot (
Figure 5a) shows the state when no currents are fed into the windings. In this state, the magnetic circuit is saturated at magnet sides. This is a necessary feature of every interior permanent magnet synchronous machine (IPMSM) design [
1], as it provides very high reluctance to the leakage flux path around magnet sides and therefore forces the magnetic flux out of the rotor through the air gap. It is worth mentioning that even in this current-less state the rotor yoke is saturated at a flux return path (which indicates relatively high core material utilization). The second plot (
Figure 5b) shows the state when currents resulting in the peak torque of the drive are fed into the windings (it is explained later in this paper how the values of these currents were obtained). It can be seen that these currents cause significant saturation in the stator yoke, the stator teeth, as well as bulk rotor saturation.
The FEM simulation was carried out for many different combinations of the
d- and
q-axis currents and for one full electrical period. It should be taken into consideration that the rotor of this machine is segmented in six parts in the axial direction (see the report [
19]). These segments are rotated consecutively by one mechanical degree providing rotor skewing, which helps to partly eliminate adverse spatial harmonics in torque and induced voltage. It was considered in the analysis in such a way that the simulation was carried out for six different initial rotor positions and the results were averaged.
Please note that all the results (after averaging due to rotor skewing) are provided to the reader in
Supplementary Materials attached to this paper. The attached spreadsheet includes the following signals as functions of mechanical angle: machine torque,
d-axis flux linkage,
q-axis flux linkage and induced voltages in all three phases.
The following part of the analysis focuses mainly on torque vs. speed curve calculation. From this point of view only average torque values are important and the spatial harmonics should be neglected. Hence, the torque and flux linkage values were averaged over one electrical period. The obtained numerical values are given in
Appendix A providing the reader with the possibility to reproduce all the following results. The
d- and
q-axis flux linkage values can be found in
Table A4 and
Table A5, respectively. Torque values can be found in
Table A6. The peak to peak torque ripple values were calculated as well (see
Table A7). Values for intermediate current operation points were obtained via cubic interpolation and plotted in the form of three dimensional surfaces in
Figure 6.
Severe magnetic circuit saturation due to an increase of the
q-axis current component can be clearly seen in the
q-axis flux linkage surface (
Figure 6b). On the other hand, the
d-axis flux linkage surface is relatively linear (
Figure 6a), although it bends slightly downwards with the rising
q-axis flux linkage value in a low
d-axis current range. This is due to the so called cross-saturation effect caused by the fact that both
d- and
q-axis fluxes share some common path in the magnetic circuit [
18]. This effect is also visible in the
q-axis flux linkage surface, as the
d-axis de-magnetization (i.e., rise in the de-magnetizing
d-axis current magnitude) causes a slight increase in the
q-axis flux linkage value. All the above findings indicate that the modeled machine exhibits significant iron core saturation in its feasible operation range. Hence, it has proven to be a very good candidate for comparison with the linearized model, since the obtained discrepancy between the results calculated with these two models can be expected to be close to its realistic extreme values.
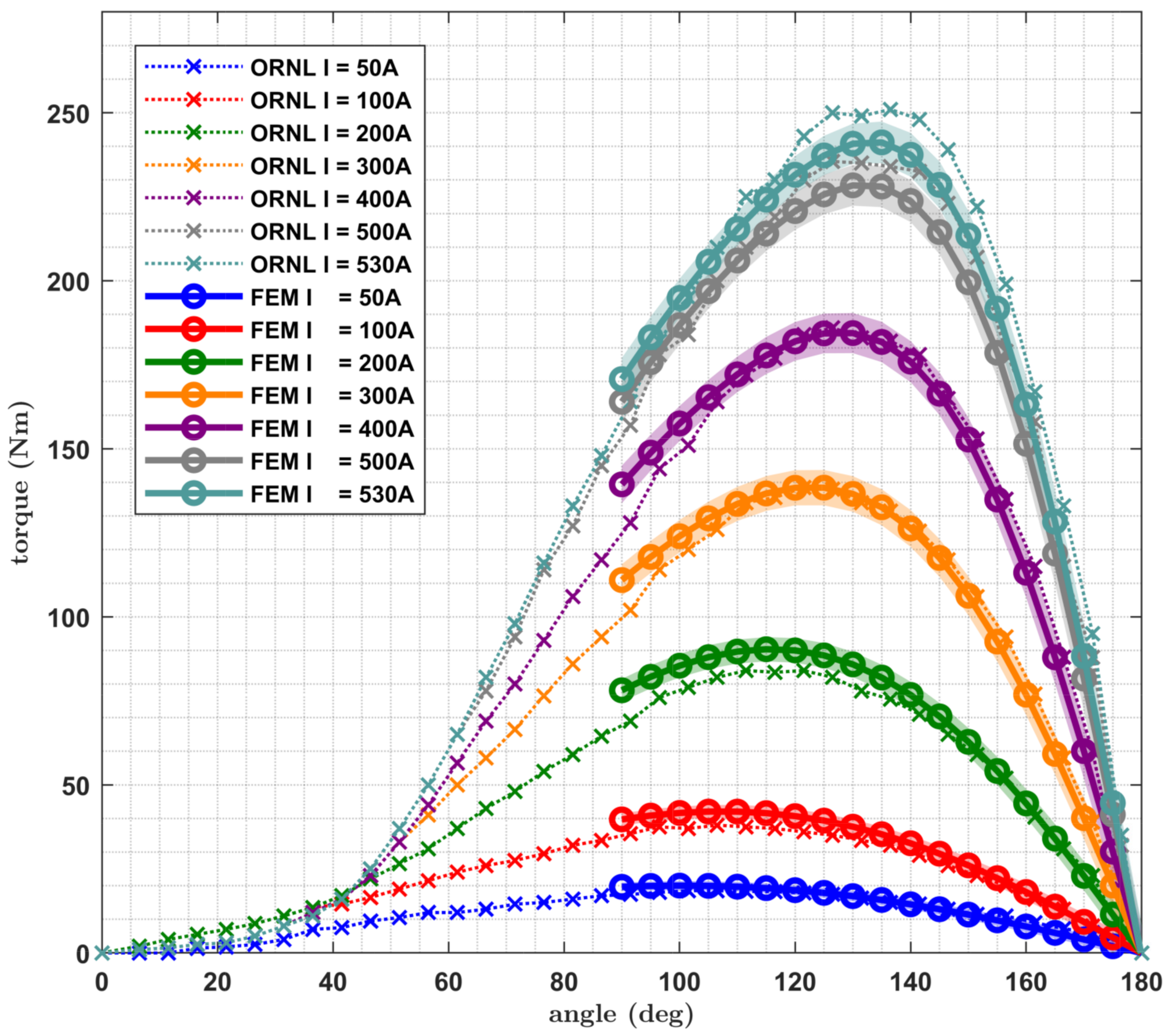
The model was validated based on the locked rotor test results published in the ORNL report [
19] (see
Figure 7). During such a test the rotor is locked and currents of constant amplitude and various space-vector angles (with respect to
d-axis) are fed into the windings. Importantly, the machine torque contains spatial harmonics and thus the value measured in the locked rotor test depends on the position in which the rotor has been locked. In order to take it into consideration, the simulation values used for the comparison in
Figure 7 are the values averaged over one electrical period and the expected torque ripple is shown in the form of shaded areas. For this validation the torque and the torque ripple values presented in
Figure 6c,d were used. The values corresponding to the measurement points were calculated using cubic interpolation.
It should be considered that the rotor geometry of the machine is relatively complex and it was reconstructed based only on a photograph. Hence, it is obvious that some inaccuracies of the model should be expected. A difference between torque values calculated with the FEM model and the measurement results is especially visible at angles around 90 degrees (i.e., for low d-axis current). In the authors’ opinion, the obtained accuracy is sufficient to positively validate the presented model.
3.2. Calculations Based on the Non-Linear Model
The torque vs. speed curve can now be calculated using the following non-linear steady-state model [
18]:
where
and
are
d- and
q-axis terminal voltage space-vector components (V);
and
are
d- and
q-axis current space-vector components (A);
R is stator phase resistance (
);
is electrical rotor speed (rad/s);
and
are
d- and
q-axis flux linkages (Wb), which are non-linear functions of the current space-vector components (i.e.,
and
);
p is the number of pole pairs;
is machine torque (Nm).
The torque maximizing operation points were calculated based on (1) and the values listed in
Table 1 using a numerical search algorithm created in MATLAB (R2017b) software [
33]. The results are shown in
Figure 8a, where the filled dots represent the obtained operation points for the consecutively rising speed. The same points can be identified on the torque vs. speed curve shown in
Figure 8b. The first one corresponds to the peak torque in the base operation speed range and lies in a place where the possibly uppermost torque iso-line (blue lines in
Figure 8a) is tangential to the current limitation circle (red circle in
Figure 8a). The flux density plot in
Figure 5b was obtained for exactly this operation point, which can be described with the following current space-vector component values:
It is common knowledge that there exist two general drive classes regarding their behavior during field-weakening operation. The first is the finite maximal speed drive class. In such a case, there is only one field-weakening operating mode, i.e., current and voltage limited operating mode. During such operation, the current space-vector locus follows a current limitation circle up to the maximal speed of the drive. The second class is the infinite maximal speed drive class. In this case, there exists an additional operating mode above some threshold speed. This is a voltage limited operation according to a so-called maximal torque per voltage (MTPV) control strategy. During such an operation, the current space-vector locus follows a path from the current limitation circle into the so-called machine characteristic current point. All these characteristics are explained in detail in [
6,
10].
It can be identified in
Figure 8a that the analyzed drive exhibits only one field-weakening operation mode, i.e., current and voltage limited operation along the current limitation circle path. This operation mode is maintained up to the maximal speed of this drive (i.e., 11,400 rpm) and the MTPV operation region has not been identified (which means that this is the finite maximal speed drive according to [
6,
10]).
3.3. Calculations Based on the Linearized Model
The goal of this paper was to quantify the influence of iron core saturation and voltage drop across the winding resistance on the results obtained with the following simplified model (for the details of this model please refer to [
6,
10]):
where
and
are
d- and
q-axis inductances calculated for some particular linearization point (H);
is permanent magnet flux linkage calculated for some particular linearization point (Wb). In order to do so, the torque vs. speed curve for the BMW i3 traction drive should be now calculated with analytical formulas derived using this model, which can be found in [
6]. The results can be then compared with these obtained using the previously introduced more complicated model (1). For convenience, in the course of this paper the following names are going to be used, in order to distinguish between the two models:
‘Non-linear model’—the space-vector model in steady-state defined with Equation (1), which includes voltage drop across stator resistance and non-linear flux linkage surfaces.
‘Linearized model’—the simplified model defined with Equation (3), which neglects voltage drop across stator resistance and assumes constant machine parameters (hence the term ‘linearized’).
As the linearized model uses constant machine parameter values, the non-linear flux linkage surfaces obtained in FEM simulation (see
Figure 6) should be linearized at some operation point. In the presented analysis, the peak torque operation point (2) was chosen. The linearized machine parameters can be calculated from the flux linkages definition:
as follows:
The obtained linearized system parameters have been summarized in
Table 2. These data follow the nomenclature introduced in [
6]:
where
is the machine saliency factor;
is the drive characteristic factor;
is the maximal phase current of the drive (A);
is a machine characteristic current, i.e.,
d-axis current magnitude needed to reduce the
d-axis flux linkage to zero (A).
It is a well-known fact that the field-weakening performance of an inverter-fed PMSM drive depends on the relationship between the motor characteristic current
and the maximal current of the drive
[
8,
10,
13]. In [
6], the authors proposed to describe this relationship with a single variable, i.e., the drive characteristic factor
. It was also derived that the value of this factor can deliver information about the necessity to over-size the drive inverter power rating.
For , the drive has a finite maximal speed and the ratio of this speed to the base speed rises with the value of this factor. The peak power value of drives from this class is equal to the volt-ampere rating of the inverter needed to operate these drives within their specifications. It means that for this class the power rating of the power electronic converters does not need to be over-sized.
For
, the drive has an infinite maximal speed and the true constant power speed region rises when this factor is increased. Unfortunately, together with the value of
the ratio between the volt-ampere rating of the power electronics inverter needed to operate the drive and the peak power at the machine shaft also rises. Hence, in this case the inverter power rating needs to be over-sized. A detailed discussion regarding this topic can be found in [
6].
For the reasons explained above, it is common practice to design the drives in such a way that the characteristic factor has a value possibly close to the unity. It provides a good trade-off between field-weakening performance and power converter sizing. The analyzed BMW i3 drive is a perfect example of this design trend. Please note that the obtained drive characteristic factor value for this drive (see
Table 2) is slightly smaller than the unity, but very close to it. As the drive belongs to the finite maximum speed class (i.e.,
), there should be no MTPV operation region, which matches the results obtained with the non-linear model (see
Figure 8).
Now, the torque vs. speed curve can be calculated based on the linearized model using analytical formulas. Please note, that different equivalent forms of these equations can be found in the literature [
6,
8,
10]. The solution derived in [
6] is going to be rewritten here for the reader’s convenience. It bases on the following normalized quantities in a per-unit system:
where the superscript (
) denotes the per-unit quantity (p.u.);
i is current (A);
is electrical angular speed (rad/s);
T is torque (Nm);
is the maximal phase voltage (V);
is the base electrical angular speed for the normalization (rad/s);
is the base torque for the normalization (Nm). The following formulas allow to calculate per-unit values of currents, torque and speed, which can be converted into physical quantities with (7).
The per-unit peak torque in the base speed region can be calculated with:
The per-unit transition speed between the base speed region and the field-weakening speed region equals:
The per-unit torque in the field-weakening speed operation range can be expressed as a function of the per-unit speed as:
3.4. Comparison of the Results—Maximal Torque
The results of the maximal torque vs. speed calculations for both methods are shown in
Figure 9 (all data for the non-linear model are marked with black and for the linearized model with gray).
It can be seen that both torque surfaces diverge significantly from each other in the high
q-axis current region (see
Figure 9a). On the other hand, matching between the surfaces is relatively good in the low
q-axis current region. It can be observed, that operation points in the field-weakening speed range (i.e., above ca. 4000 rpm) lie in this quasi linear region, hence the torque vs. speed curves obtained with the two methods match very well in this speed range (see
Figure 9b). On the other hand, a vast part of the current limitation boundaries (solid quasi quarter-circular curves on the surfaces) lies in the saturated region of the torque surface (see
Figure 9a). It is a well-known fact [
6,
7,
8,
9,
10,
11,
12] that the peak torque operation point lies somewhere on this boundary and since both surfaces diverge significantly in this region, completely different peak torque operation points were identified with both methods. As a result, there is a relatively big difference in the peak torque value obtained with both models: 279.7 Nm with the linearized model vs. 258.2 Nm with the non-linear model.