On the Quantum Confinement Effects in Ultrathin PdO Films by Experiment and Theory
Abstract
:1. Introduction
2. Materials and Methods
2.1. Experimental Details
2.2. Theory
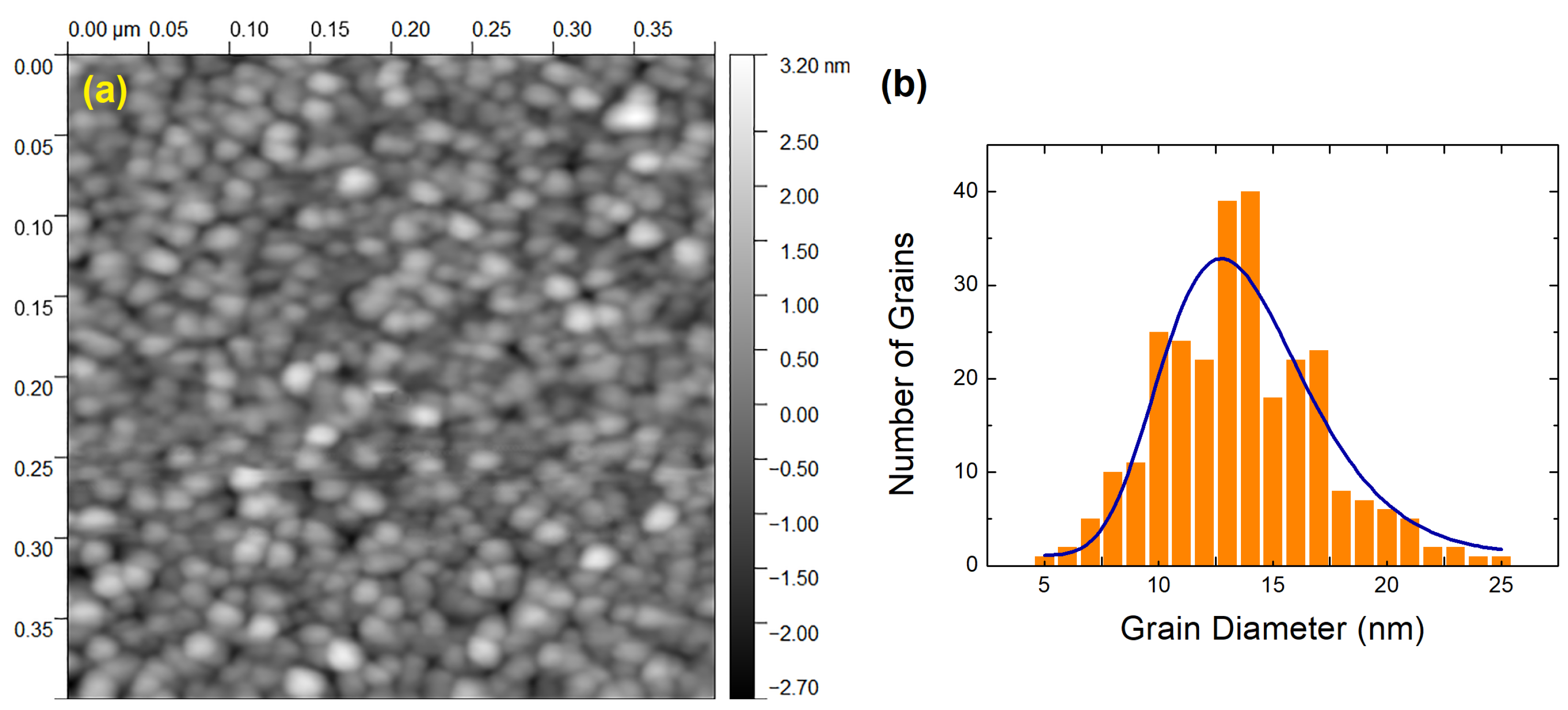
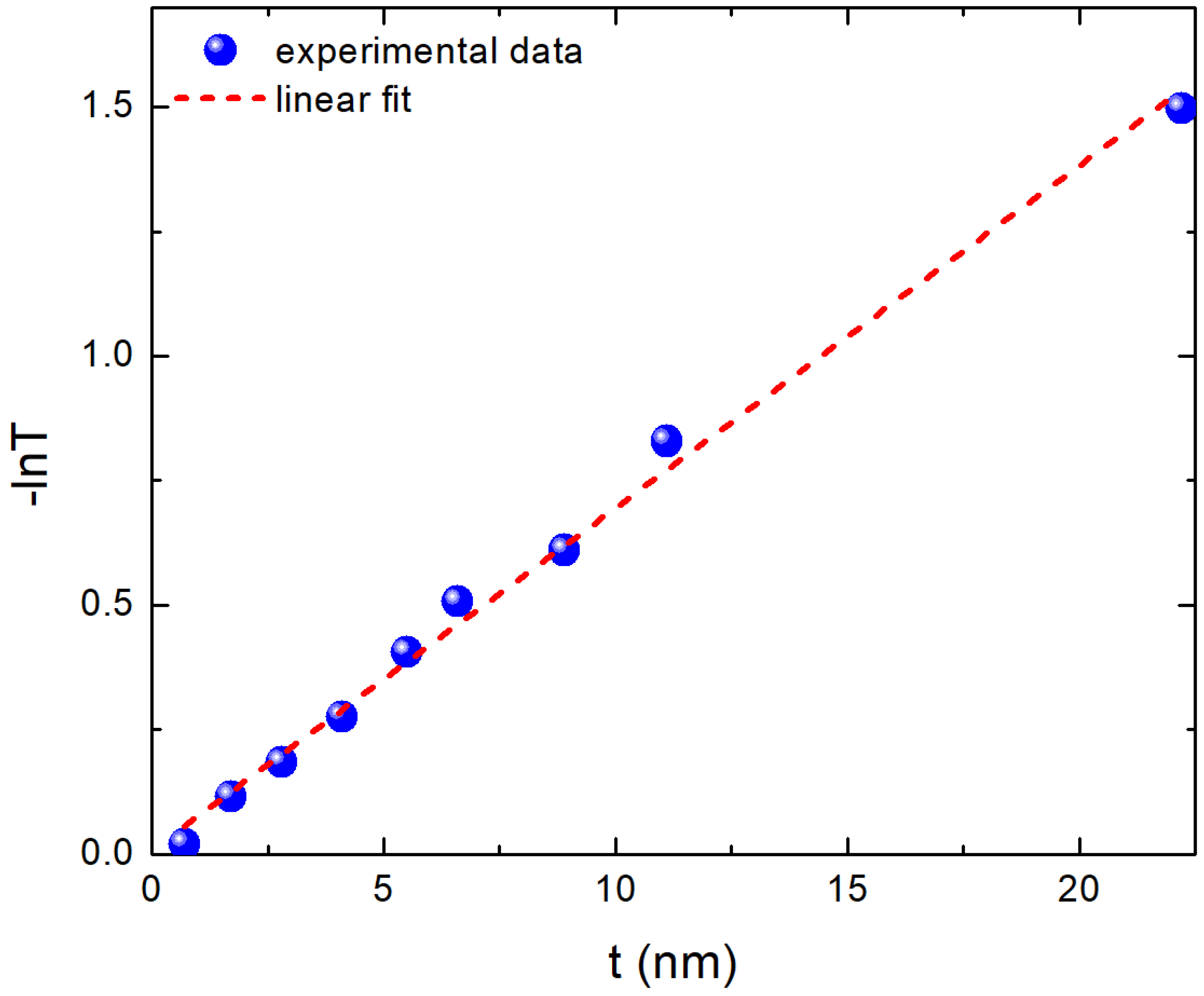
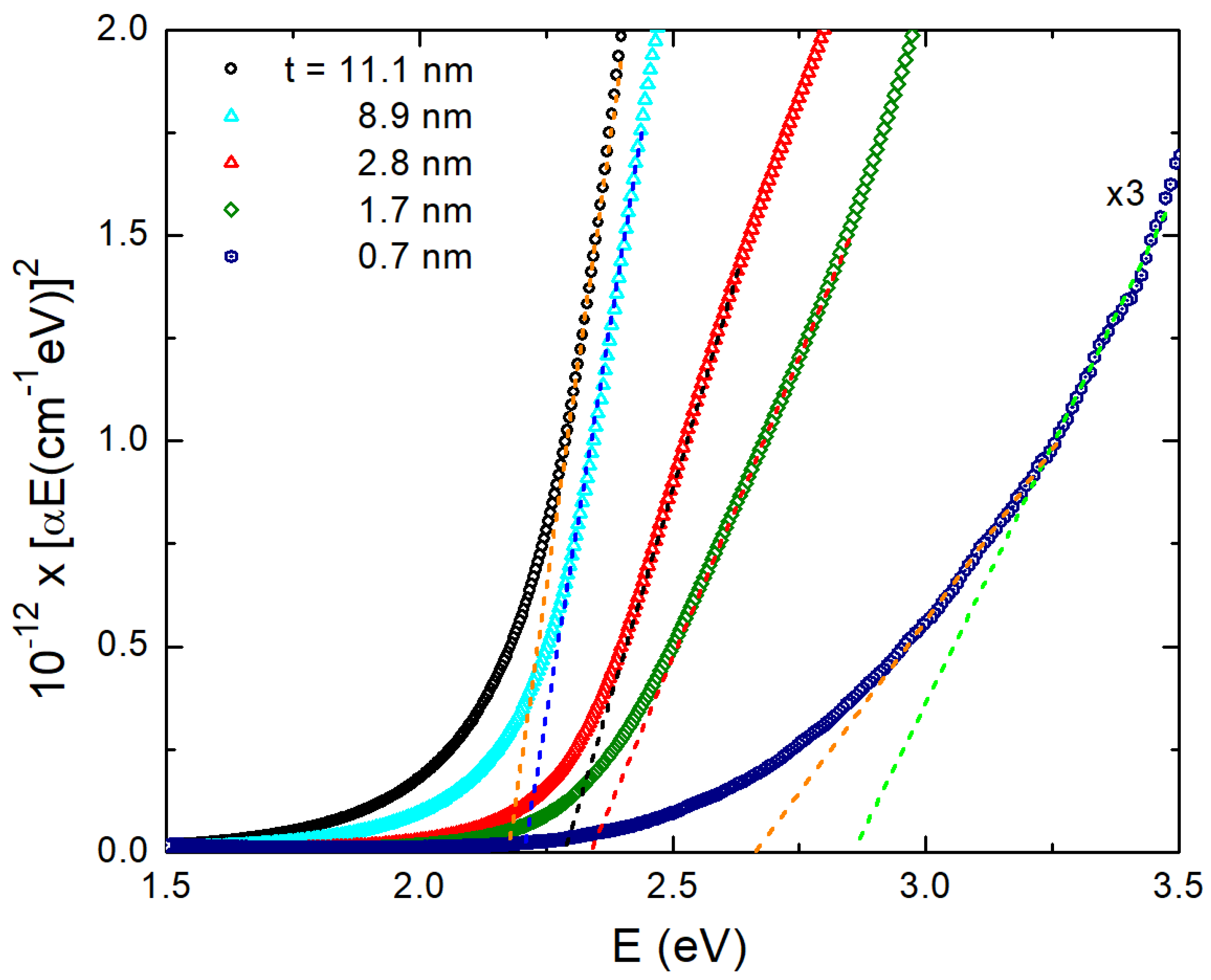
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Park, K.T.; Novikov, D.L.; Gubanov, V.A.; Freeman, A.J. Electronic structure of noble-metal monoxides: PdO, PtO, and AgO. Phys. Rev. B 1994, 49, 4425–4431. [Google Scholar] [CrossRef] [PubMed]
- Lee, Y.T.; Lee, J.M.; Kim, Y.J.; Joe, J.H.; Lee, W. Hydrogen gas sensing properties of PdO thin films with nano-sized cracks. Nanotechnology 2010, 21, 165503. [Google Scholar] [CrossRef] [PubMed]
- Chiang, Y.J.; Pan, F.M. PdO Nanoflake Thin Films for CO Gas Sensing at Low Temperatures. J. Phys. Chem. C 2013, 117, 15593–15601. [Google Scholar] [CrossRef]
- Ryabtsev, S.; Ievlev, V.; Samoylov, A.; Kuschev, S.; Soldatenko, S. Microstructure and electrical properties of palladium oxide thin films for oxidizing gases detection. Thin Solid Film. 2017, 636, 751–759. [Google Scholar] [CrossRef]
- Corso, A.J.; Tessarolo, E.; Guidolin, M.; Della Gaspera, E.; Martucci, A.; Angiola, M.; Donazzan, A.; Pelizzo, M.G. Room-temperature optical detection of hydrogen gas using palladium nano-islands. Int. J. Hydrogen Energy 2018, 43, 5783–5792. [Google Scholar] [CrossRef]
- Arora, K.; Puri, N.K. Electrophoretically deposited nanostructured PdO thin film for room temperature amperometric H2 sensing. Vacuum 2018, 154, 302–308. [Google Scholar] [CrossRef]
- Ievlev, V.; Ryabtsev, S.; Samoylov, A.; Shaposhnik, A.; Kuschev, S.; Sinelnikov, A. Thin and ultrathin films of palladium oxide for oxidizing gases detection. Sens. Actuators B Chem. 2018, 255, 1335–1342. [Google Scholar] [CrossRef]
- Agarwal, S.; Ahemad, M.J.; Kumar, S.; Van Dung, D.; Rai, P.; Kumar, M.; Awasthi, K.; Yu, Y.T. Enhanced hydrogen sensing performances of PdO nanoparticles-decorated ZnO flower-like nanostructures. J. Alloys Compd. 2022, 900, 163545. [Google Scholar] [CrossRef]
- Yang, S.; Chen, G.; Zheng, F.; Yu, Y.; Ye, S.; Wang, T.; Fu, Y.; Zhang, X. Pd-decorated PdO nanoparticle nanonetworks: A low-cost eye-readable H2 indicator with reactivation ability. Sens. Actuators B Chem. 2022, 368, 132242. [Google Scholar] [CrossRef]
- Huang, C.J.; Pan, F.M.; Chen, H.Y.; Chang, L. Growth and photoresponse study of PdO nanoflakes reactive-sputter deposited on SiO2. J. Appl. Phys. 2010, 108, 053105. [Google Scholar] [CrossRef]
- Omotunde, O.; Okoronkwo, A.; Aiyesanmi, A.; Gurgur, E. Photocatalytic behavior of mixed oxide NiO/PdO nanoparticles toward degradation of methyl red in water. J. Photochem. Photobiol. A Chem. 2018, 365, 145–150. [Google Scholar] [CrossRef]
- Styopkin, V.; Rudenko, V.; Brodyn, M.; Liakhovetskyi, V. Characteristics of Plasmon Resonance of Gold Nanoparticles in Three-Layer Systems AuNP-Al2O3-PdO(Pd). Plasmonics 2022, 17, 859–867. [Google Scholar] [CrossRef]
- Bruska, M.K.; Czekaj, I.; Delley, B.; Mantzaras, J.; Wokaun, A. Electronic structure and oxygen vacancies in PdO and ZnO: Validation of DFT models. Phys. Chem. Chem. Phys. 2011, 13, 15947–15954. [Google Scholar] [CrossRef] [PubMed]
- Rey, E.; Kamal, M.R.; Miles, R.B.; Royce, B.S.H. The semiconductivity and stability of palladium oxide. J. Mater. Sci. 1978, 13, 812–816. [Google Scholar] [CrossRef]
- Nilsson, P.O. Optical properties of PdO in the range of 0.5–5.4 eV. J. Phys. C Solid State Phys. 1979, 12, 1423. [Google Scholar] [CrossRef]
- Garoufalis, C.S.; Barnasas, A.; Stamatelatos, A.; Karoutsos, V.; Grammatikopoulos, S.; Poulopoulos, P.; Baskoutas, S. A Study of Quantum Confinement Effects in Ultrathin NiO Films Performed by Experiment and Theory. Materials 2018, 11, 949. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Barnasas, A.; Garoufalis, C.S.; Anyfantis, D.I.; Bouropoulos, N.; Poulopoulos, P.; Hayrapetyan, D.B.; Baskoutas, S. Quantum Confinement Effects of Thin Co3O4 Films. Atoms 2021, 9, 70. [Google Scholar] [CrossRef]
- Barnasas, A.; Kanistras, N.; Ntagkas, A.; Anyfantis, D.; Stamatelatos, A.; Kapaklis, V.; Bouropoulos, N.; Mystiridou, E.; Poulopoulos, P.; Garoufalis, C.; et al. Quantum confinement effects of thin ZnO films by experiment and theory. Phys. E Low-Dimens. Syst. Nanostruct. 2020, 120, 114072. [Google Scholar] [CrossRef]
- Karoutsos, V. Scanning probe microscopy: Instrumentation and applications on thin films and magnetic multilayers. J. Nanosci. Nanotechnol. 2009, 9, 6783. [Google Scholar] [CrossRef] [PubMed]
- Kapaklis, V.; Poulopoulos, P.; Karoutsos, V.; Manouras, T.; Politis, C. Growth of thin Ag films produced by radio frequency magnetron sputtering. Thin Solid Film. 2006, 510, 138–142. [Google Scholar] [CrossRef]
- Garoufalis, C.; Poulopoulos, P.; Bouropoulos, N.; Barnasas, A.; Baskoutas, S. Growth and optical properties of Fe2O3 thin films: A study of quantum confinement effects by experiment and theory. Phys. E Low-Dimens. Syst. Nanostruct. 2017, 89, 67–71. [Google Scholar] [CrossRef]
- Poulopoulos, P.; Baskoutas, S.; Pappas, S.D.; Garoufalis, C.S.; Droulias, S.A.; Zamani, A.; Kapaklis, V. Intense Quantum Confinement Effects in Cu2O Thin Films. J. Phys. Chem. C 2011, 115, 14839–14843. [Google Scholar] [CrossRef]
- Baskoutas, S.; Terzis, A.F. Size-dependent band gap of colloidal quantum dots. J. Appl. Phys. 2006, 99, 013708. [Google Scholar] [CrossRef]
- Baskoutas, S. Novel formulation of the Hartree–Fock approximation: Effective band gap calculation of InAs nanorods. Phys. Lett. A 2005, 341, 303–307. [Google Scholar] [CrossRef]
- Rieth, M.; Schommers, W.; Baskoutas, S. Exact numerical solution of Schrödinger’s equation for a particle in an interaction potential of general shape. Int. J. Mod. Phys. B 2002, 16, 4081–4092. [Google Scholar] [CrossRef]
- Poulopoulos, P.; Angelakeris, M.; Kehagias, T.; Niarchos, D.; Flevaris, N. Improved growth and perpendicular anisotropy in Pd–Co multilayers with intentionally alloyed layers. Thin Solid Film. 2000, 371, 225–230. [Google Scholar] [CrossRef]
- Escobar, M.A.; Magaña, L.F.; Valenzuela, R. Effect of the grain size distribution on the magnetization curve. J. Appl. Phys. 1985, 57, 2142–2146. [Google Scholar] [CrossRef]
- Tsuji, Y.; Saito, M.; Yoshizawa, K. Dynamics and Energetics of Methane on the Surfaces of Transition Metal Oxides. In Direct Hydroxylation of Methane: Interplay Between Theory and Experiment; Yoshizawa, K., Ed.; Springer Singapore: Singapore, 2020; pp. 101–133. [Google Scholar]
- Fox, M. Optical Properties of Solids, 2nd ed.; Oxford University Press: New York, NY, USA, 2010. [Google Scholar]
- Barnasas, A.; Diamantopoulos, N.C.; Anyfantis, D.I.; Bouropoulos, N.; Constantin, P.; Poulopoulos, P. Growth and Optical Properties of MoO3 thin Films. Nano Hybrids Compos. 2022, 36, 1–12. [Google Scholar] [CrossRef]
- Sobolev, V.V.; Merzlyakov, D.A.; Sobolev, V.V. Optical Properties and Electronic Structure of CaO. J. Appl. Spectrosc. 2016, 83, 567–572. [Google Scholar] [CrossRef]
- Weber, W.H.; Remillard, J.T.; McBride, J.R.; Aspnes, D.E. Optical dielectric response of PdO. Phys. Rev. B 1992, 46, 15085–15091. [Google Scholar] [CrossRef]
- Dovesi, R.; Saunders, V.R.; Roetti, C.; Orlando, R.; Zicovich-Wilson, C.M.; Pascale, F.; Civalleri, B.; Doll, K.; Harrison, N.M.; Bush, I.J.; et al. CRYSTAL06 User’s Manual; University of Torino: Torino, Italy, 2006. [Google Scholar]
- Stephens, P.J.; Devlin, F.J.; Chabalowski, C.F.; Frisch, M.J. Ab Initio Calculation of Vibrational Absorption and Circular Dichroism Spectra Using Density Functional Force Fields. J. Phys. Chem. 1994, 98, 11623–11627. [Google Scholar] [CrossRef]
- Laun, J.; Vilela Oliveira, D.; Bredow, T. Consistent gaussian basis sets of double- and triple-zeta valence with polarization quality of the fifth period for solid-state calculations. J. Comput. Chem. 2018, 39, 1285–1290. [Google Scholar] [CrossRef]
- Vilela Oliveira, D.; Laun, J.; Peintinger, M.F.; Bredow, T. BSSE-correction scheme for consistent gaussian basis sets of double- and triple-zeta valence with polarization quality for solid-state calculations. J. Comput. Chem. 2019, 40, 2364–2376. [Google Scholar] [CrossRef] [PubMed]
- Zeng, Z.; Garoufalis, C.S.; Baskoutas, S.; Bester, G. Excitonic optical properties of wurtzite ZnS quantum dots under pressure. J. Chem. Phys. 2015, 142, 114305. [Google Scholar] [CrossRef] [Green Version]
- Ma, X.; Min, J.; Zeng, Z.; Garoufalis, C.S.; Baskoutas, S.; Jia, Y.; Du, Z. Excitons in InP, GaP, and GaxIn1-xP quantum dots: Insights from time-dependent density functional theory. Phys. Rev. B 2019, 100, 245404. [Google Scholar] [CrossRef] [Green Version]
- Han, P.; Min, J.; Zeng, Z.; Garoufalis, C.S.; Baskoutas, S.; Jia, Y.; Du, Z. Excitonic characteristics of blue-emitting quantum dot materials in group II-VI using hybrid time-dependent density functional theory. Phys. Rev. B 2021, 104, 045404. [Google Scholar] [CrossRef]
- Min, J.; Zhang, Y.; Zhou, Y.; Xu, D.; Garoufalis, C.S.; Zeng, Z.; Shen, H.; Baskoutas, S.; Jia, Y.; Du, Z. Size Engineering of Trap Effects in Oxidized and Hydroxylated ZnSe Quantum Dots. Nano Lett. 2022, 22, 3604–3611. [Google Scholar] [CrossRef]
- Zhou, Y.; Garoufalis, C.S.; Baskoutas, S.; Zeng, Z.; Jia, Y. Twisting Enabled Charge Transfer Excitons in Epitaxially Fused Quantum Dot Molecules. Nano Lett. 2022, 22, 4912–4918. [Google Scholar] [CrossRef]
- Hass, K.C.; Carlsson, A.E. Band structures of nonmagnetic transition-metal oxides: PdO and PtO. Phys. Rev. B 1992, 46, 4246–4249. [Google Scholar] [CrossRef]
- Frank, M.; Wolter, K.; Magg, N.; Heemeier, M.; Kühnemuth, R.; Bäumer, M.; Freund, H.J. Phonons of clean and metal-modified oxide films: An infrared and HREELS study. Surf. Sci. 2001, 492, 270–284. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barnasas, A.; Garoufalis, C.S.; Anyfantis, D.I.; Poulopoulos, P.; Baskoutas, S. On the Quantum Confinement Effects in Ultrathin PdO Films by Experiment and Theory. Materials 2022, 15, 8700. https://doi.org/10.3390/ma15238700
Barnasas A, Garoufalis CS, Anyfantis DI, Poulopoulos P, Baskoutas S. On the Quantum Confinement Effects in Ultrathin PdO Films by Experiment and Theory. Materials. 2022; 15(23):8700. https://doi.org/10.3390/ma15238700
Chicago/Turabian StyleBarnasas, Alexandros, Christos S. Garoufalis, Dimitrios I. Anyfantis, Panagiotis Poulopoulos, and Sotirios Baskoutas. 2022. "On the Quantum Confinement Effects in Ultrathin PdO Films by Experiment and Theory" Materials 15, no. 23: 8700. https://doi.org/10.3390/ma15238700
APA StyleBarnasas, A., Garoufalis, C. S., Anyfantis, D. I., Poulopoulos, P., & Baskoutas, S. (2022). On the Quantum Confinement Effects in Ultrathin PdO Films by Experiment and Theory. Materials, 15(23), 8700. https://doi.org/10.3390/ma15238700











