Overlooked Ionic Contribution of a Chiral Dopant in Cholesteric Liquid Crystals
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Preparation of LC Cell Samples
2.3. Phase Transition Temperature Measurement by Dielectric Spectroscopy of LCs
2.4. Thermo-Optical Transmission Measurement of CLCs
2.5. Measurement of Ionic Properties of CLCs
3. Results and Discussion
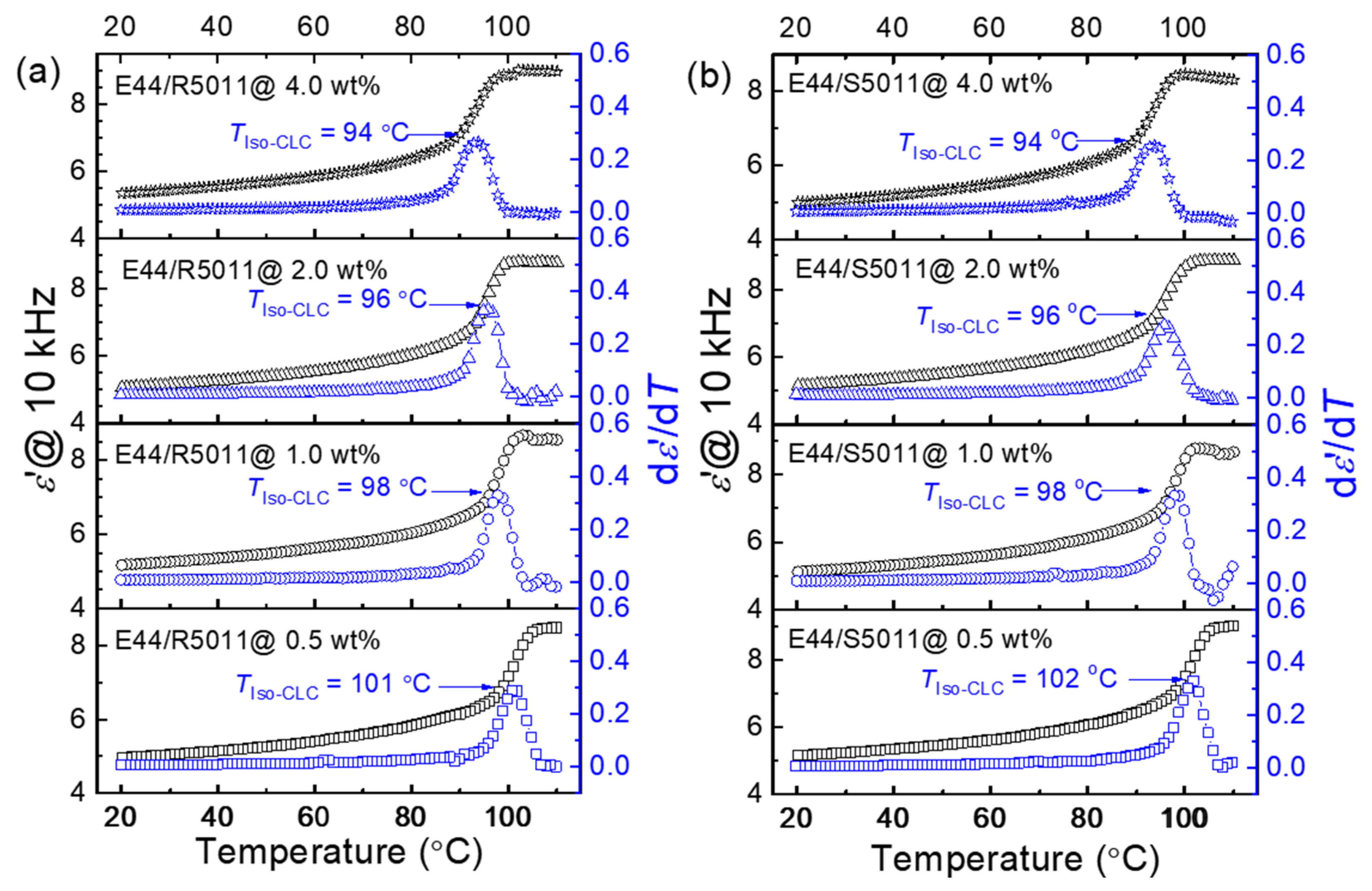
3.1. Influence of the Chiral Dopant Concentration on CLC Phase Transition Temperature
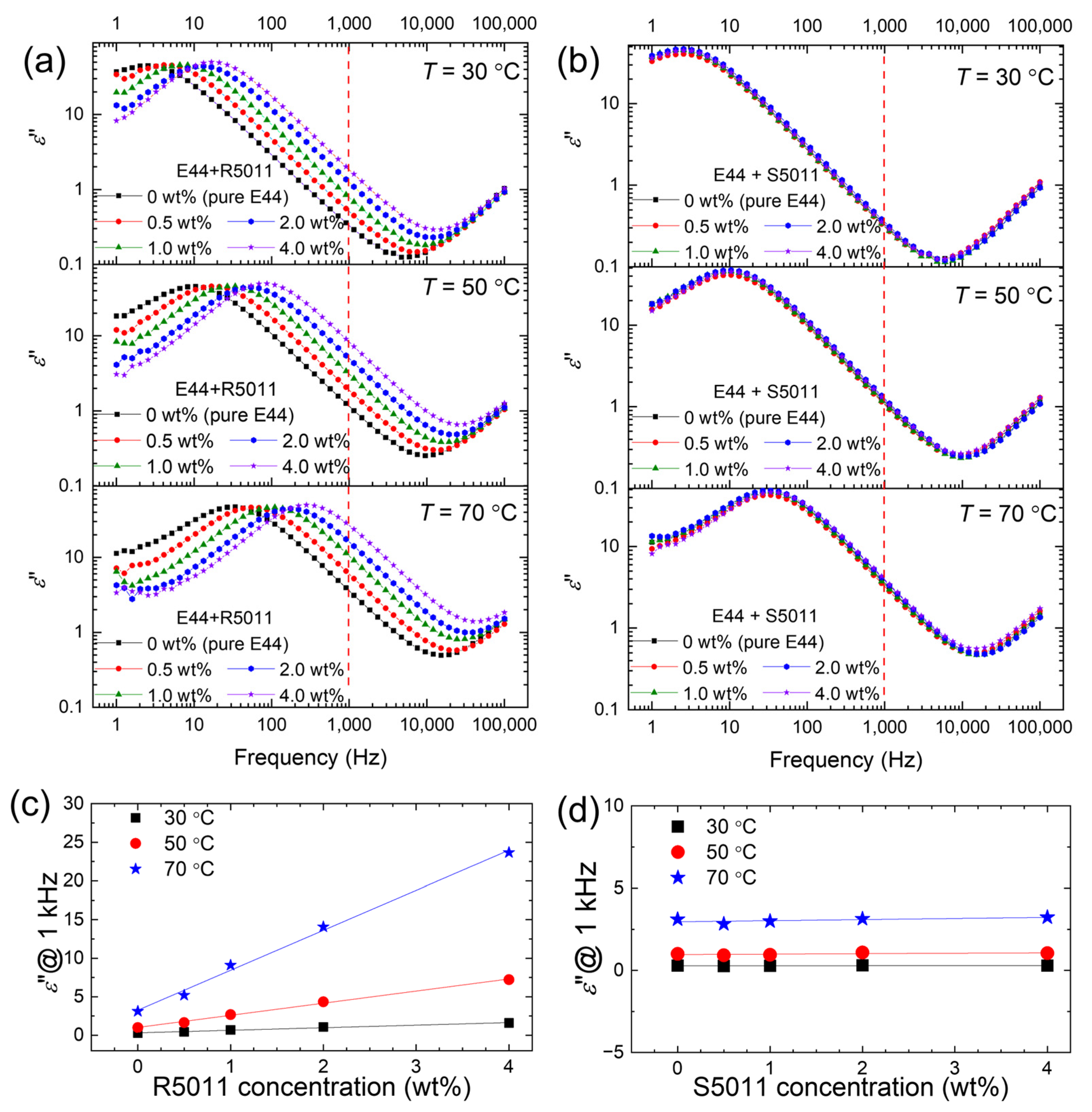
3.2. Thermal Behavior of Ionic Responses of Oppositely Handed CLCs
3.3. Thermal Behavior of Ionic Responses of LCs with Binary Doping of Two Racemic Chiral Dopants
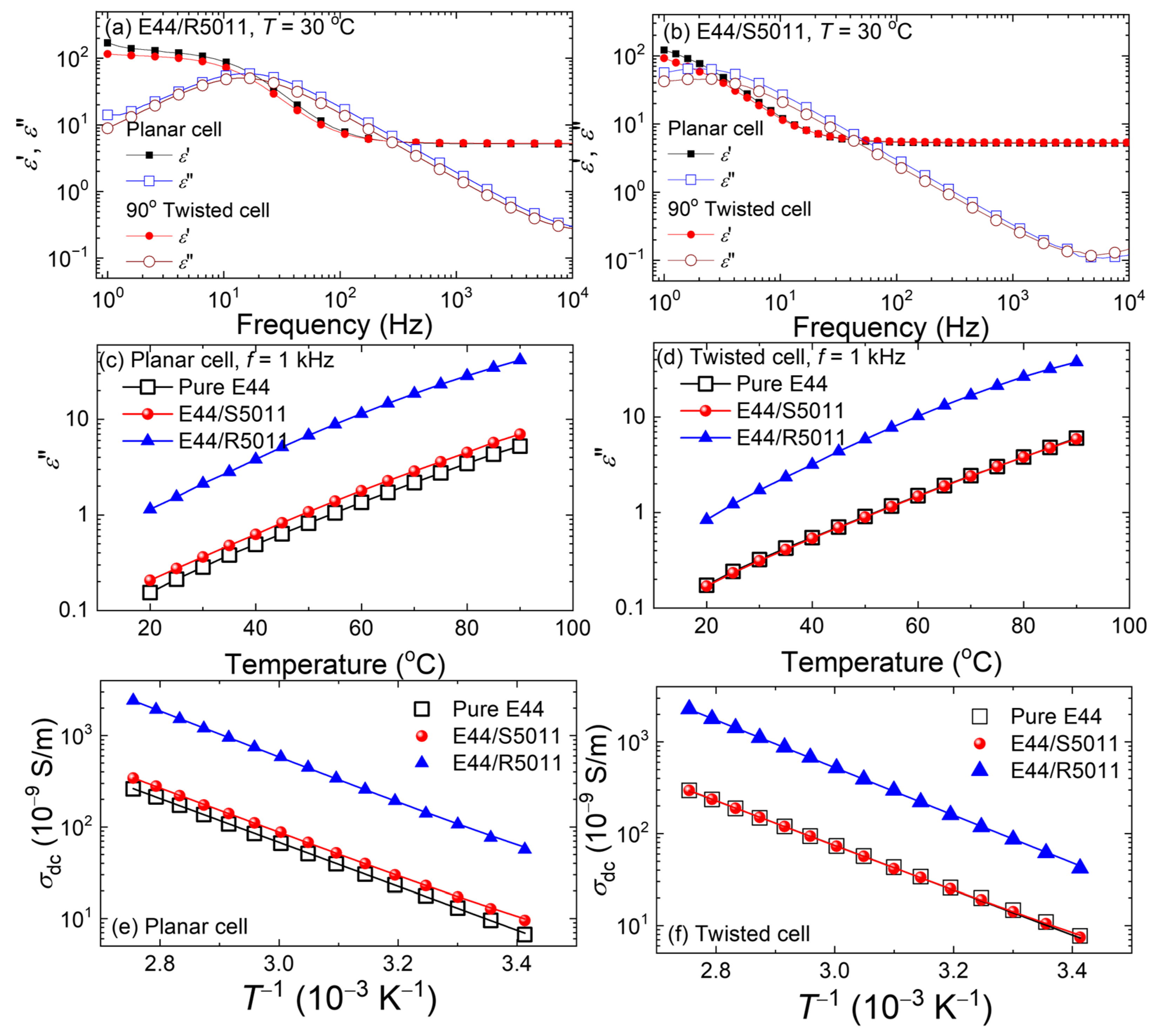
3.4. Dielectric Properties of CLCs in Antiparallel and 90°-Twisted LC Cells
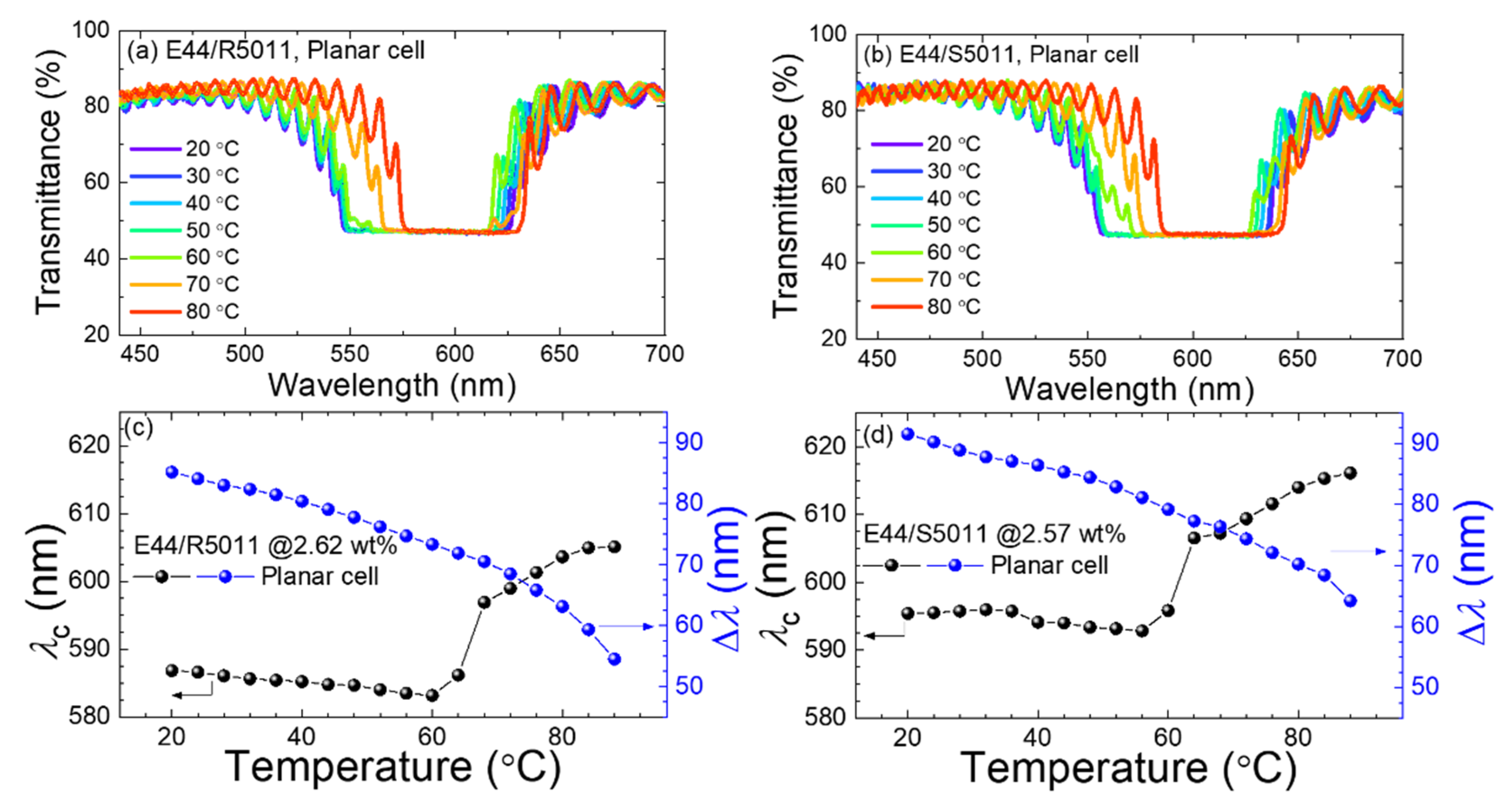
3.5. Optical Characteristics of CLCs in Antiparallel and 90°-Twisted LC Cells
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lee, Y.-H.; Huang, K.-C.; Lee, W.; Chen, C.-Y. Low-power displays with dye-doped bistable chiral-tilted homeotropic nematic liquid crystals. J. Disp. Technol. 2014, 10, 1106–1109. [Google Scholar] [CrossRef]
- Hsiao, Y.-C.; Yeh, E.-R.; Lee, W. Advanced color-reflective dual-frequency cholesteric liquid crystal displays and the driving matrix. Mol. Cryst. Liq. Cryst. 2017, 644, 12–18. [Google Scholar] [CrossRef]
- Lin, M.-Y.; Xu, W.-H.; Bikbaev, R.G.; Yang, J.-H.; Li, C.-R.; Timofeev, I.V.; Lee, W.; Chen, K.-P. Chiral-Selective Tamm Plasmon Polaritons. Materials 2021, 14, 2788. [Google Scholar] [CrossRef]
- Rudakova, N.V.; Timofeev, I.V.; Bikbaev, R.G.; Pyatnov, M.V.; Vetrov, S.Y.; Lee, W. Chiral optical Tamm states at the interface between an all-dielectric polarization-preserving anisotropic mirror and a cholesteric liquid crystal. Crystals 2019, 9, 502. [Google Scholar] [CrossRef]
- Hsiao, Y.-C.; Wu, C.-Y.; Chen, C.-H.; Zyryanov, V.Y.; Lee, W. Electro-optical device based on photonic structure with a dual-frequency cholesteric liquid crystal. Opt. Lett. 2011, 36, 2632–2634. [Google Scholar] [CrossRef]
- Xu, M.; Yang, D.-K. Dual frequency cholesteric light shutters. Appl. Phys. Lett. 1997, 70, 720–722. [Google Scholar] [CrossRef]
- Bao, R.; Liu, C.-M.; Yang, D.-K. Smart bistable polymer stabilized cholesteric texture light shutter. Appl. Phys. Express 2009, 2, 112401. [Google Scholar] [CrossRef]
- Huang, C.-Y.; Fu, K.-Y.; Lo, K.-Y.; Tsai, M.-S. Bistable transflective cholesteric light shutters. Opt. Express 2003, 11, 560–565. [Google Scholar] [CrossRef]
- Hwang, J.; Song, M.H.; Park, B.; Nishimura, S.; Toyooka, T.; Wu, J.; Takanishi, Y.; Ishikawa, K.; Takezoe, H. Electro-tunable optical diode based on photonic bandgap liquid-crystal heterojunctions. Nat. Mater. 2005, 4, 383–387. [Google Scholar] [CrossRef]
- Gevorgyan, A. Tunable reflectance of a two-defect-layer cholesteric liquid crystal. Phys. Rev. E 2011, 83, 011702. [Google Scholar] [CrossRef]
- Hsiao, Y.-C.; Tang, C.-Y.; Lee, W. Fast-switching bistable cholesteric intensity modulator. Opt. Express 2011, 19, 9744–9749. [Google Scholar] [CrossRef]
- Kopp, V.I.; Zhang, Z.-Q.; Genack, A.Z. Lasing in chiral photonic structures. Prog. Quant. Electron. 2003, 27, 369–416. [Google Scholar] [CrossRef]
- Sung, G.-F.; Wu, P.-C.; Zyryanov, V.Y.; Lee, W. Electrically active and thermally passive liquid-crystal device toward smart glass. Photonics Res. 2021, 9, 2288–2295. [Google Scholar] [CrossRef]
- Berreman, D.; Heffner, W. New bistable cholesteric liquid-crystal display. Appl. Phys. Lett. 1980, 37, 109–111. [Google Scholar] [CrossRef]
- Lin, F.-C.; Lee, W. Color-reflective dual-frequency cholesteric liquid crystal displays and their drive schemes. Appl. Phys. Express 2011, 4, 112201. [Google Scholar] [CrossRef]
- Garner, S.M.; Wu, K.-W.; Liao, Y.C.; Shiu, J.W.; Tsai, Y.S.; Chen, K.T.; Lai, Y.C.; Lai, C.-C.; Lee, Y.-Z.; Lin, J.C. Cholesteric liquid crystal display with flexible glass substrates. J. Disp. Technol. 2013, 9, 644–650. [Google Scholar] [CrossRef]
- Berreman, D.W.; Scheffer, T.J. Bragg reflection of light from single-domain cholesteric liquid-crystal films. Phys. Rev. Lett. 1970, 25, 577. [Google Scholar] [CrossRef]
- Yeh, M.-C.; Yang, S.-H.; Lee, W. Color tuning in thermo-sensitive chiral photonic liquid crystals based on the pseudo-dielectric heating effect. J. Mol. Liq. 2019, 296, 112082. [Google Scholar] [CrossRef]
- Wen, C.-H.; Gauza, S.; Wu, S.-T. Ultraviolet stability of liquid crystals containing cyano and isothiocyanato terminal groups. Liq. Cryst. 2004, 31, 1479–1485. [Google Scholar] [CrossRef]
- Hung, H.-Y.; Lu, C.-W.; Lee, C.-Y.; Hsu, C.-S.; Hsieh, Y.-Z. Analysis of metal ion impurities in liquid crystals using high resolution inductively coupled plasma mass spectrometry. Anal. Methods-UK 2012, 4, 3631–3637. [Google Scholar] [CrossRef]
- Liu, H.-H.; Lee, W. Time-varying ionic properties of a liquid-crystal cell. Appl. Phys. Lett. 2010, 97, 023510. [Google Scholar] [CrossRef]
- Huang, C.-T.; Liao, K.-T.; Lin, C.-H.; Hsu, J.-S.; Lee, W. Improved electric properties of degraded liquid crystal using metal–organic frameworks. Appl. Phys. Express 2013, 6, 121701. [Google Scholar] [CrossRef]
- Rahman, M.; Lee, W. Scientific duo of carbon nanotubes and nematic liquid crystals. J. Phys. D Appl. Phys. 2009, 42, 063001. [Google Scholar] [CrossRef]
- Liu, H.-H.; Lee, W. Ionic properties of liquid crystals dispersed with carbon nanotubes and montmorillonite nanoplatelets. Appl. Phys. Lett. 2010, 97, 173501. [Google Scholar] [CrossRef]
- Jian, B.-R.; Tang, C.-Y.; Lee, W. Temperature-dependent electrical properties of dilute suspensions of carbon nanotubes in nematic liquid crystals. Carbon 2011, 49, 910–914. [Google Scholar] [CrossRef]
- Lee, W.; Wang, C.-Y.; Shih, Y.-C. Effects of carbon nanosolids on the electro-optical properties of a twisted nematic liquid-crystal host. Appl. Phys. Lett. 2004, 85, 513–515. [Google Scholar] [CrossRef]
- Wu, P.-C.; Lee, W. Phase and dielectric behaviors of a polymorphic liquid crystal doped with graphene nanoplatelets. Appl. Phys. Lett. 2013, 102, 162904. [Google Scholar] [CrossRef]
- Tang, C.-Y.; Huang, S.-M.; Lee, W. Electrical properties of nematic liquid crystals doped with anatase TiO2 nanoparticles. J. Phys. D Appl. Phys. 2011, 44, 355102. [Google Scholar] [CrossRef]
- Liao, S.-W.; Hsieh, C.-T.; Kuo, C.-C.; Huang, C.-Y. Voltage-assisted ion reduction in liquid crystal-silica nanoparticle dispersions. Appl. Phys. Lett. 2012, 101, 161906. [Google Scholar] [CrossRef]
- Chandran, A.; Prakash, J.; Ganguly, P.; Biradar, A.M. Zirconia nanoparticles/ferroelectric liquid crystal composites for ionic impurity-free memory applications. RSC Adv. 2013, 3, 17166–17173. [Google Scholar] [CrossRef]
- Basu, R.; Garvey, A. Effects of ferroelectric nanoparticles on ion transport in a liquid crystal. Appl. Phys. Lett. 2014, 105, 151905. [Google Scholar] [CrossRef]
- Helfrich, W. Electrohydrodynamic and dielectric instabilities of cholesteric liquid crystals. J. Chem. Phys. 1971, 55, 839–842. [Google Scholar] [CrossRef]
- Rondelez, F.; Arnould, H.; Gerritsma, C. Electrohydrodynamic effects in cholesteric liquid crystals under ac electric fields. Phys. Rev. Lett. 1972, 28, 735. [Google Scholar] [CrossRef]
- Lu, H.; Xu, W.; Song, Z.; Zhang, S.; Qiu, L.; Wang, X.; Zhang, G.; Hu, J.; Lv, G. Electrically switchable multi-stable cholesteric liquid crystal based on chiral ionic liquid. Opt. Lett. 2014, 39, 6795–6798. [Google Scholar] [CrossRef]
- Hu, W.; Zhao, H.; Song, L.; Yang, Z.; Cao, H.; Cheng, Z.; Liu, Q.; Yang, H. Electrically controllable selective reflection of chiral nematic liquid crystal/chiral ionic liquid composites. Adv. Mater. 2010, 22, 468–472. [Google Scholar] [CrossRef]
- Tondiglia, V.; Natarajan, L.; Bailey, C.; Duning, M.; Sutherland, R.; Ke-Yang, D.; Voevodin, A.; White, T.; Bunning, T. Electrically induced bandwidth broadening in polymer stabilized cholesteric liquid crystals. J. Appl. Phys. 2011, 110, 053109. [Google Scholar] [CrossRef]
- Tondiglia, V.P.; Natarajan, L.V.; Bailey, C.A.; McConney, M.E.; Lee, K.M.; Bunning, T.J.; Zola, R.; Nemati, H.; Yang, D.-K.; White, T.J. Bandwidth broadening induced by ionic interactions in polymer stabilized cholesteric liquid crystals. Opt. Mater. Express 2014, 4, 1465–1472. [Google Scholar] [CrossRef]
- Lin, T.-H.; Jau, H.-C.; Chen, C.-H.; Chen, Y.-J.; Wei, T.-H.; Chen, C.-W.; Fuh, A.Y.-G. Electrically controllable laser based on cholesteric liquid crystal with negative dielectric anisotropy. Appl. Phys. Lett. 2006, 88, 061122. [Google Scholar] [CrossRef]
- Chen, S.-C.; Wu, P.-C.; Lee, W. Dielectric and phase behaviors of blue-phase liquid crystals. Opt. Mater. Express 2014, 4, 2392–2400. [Google Scholar] [CrossRef]
- Fan, Y.-H.; Lin, Y.-H.; Ren, H.; Gauza, S.; Wu, S.-T. Fast-response and scattering-free polymer network liquid crystals for infrared light modulators. Appl. Phys. Lett. 2004, 84, 1233–1235. [Google Scholar] [CrossRef]
- Perkowski, P. Dielectric spectroscopy of liquid crystals. Theoretical model of ITO electrodes influence on dielectric measurements. Opto-Electron. Rev. 2009, 17, 180–186. [Google Scholar] [CrossRef]
- Shaban, H.; Wu, P.-C.; Lee, J.-H.; Lee, W. Dielectric and electro-optical responses of a dielectrically negative nematic liquid crystal doped with cationic surfactant. Opt. Mater. Express 2021, 11, 3208–3222. [Google Scholar] [CrossRef]
- Nian, Y.-L.; Wu, P.-C.; Lee, W. Optimized frequency regime for the electrohydrodynamic induction of a uniformly lying helix structure. Photonics Res. 2016, 4, 227–232. [Google Scholar] [CrossRef]
- Hsiao, Y.-C.; Lee, W. Polymer stabilization of electrohydrodynamic instability in non-iridescent cholesteric thin films. Opt. Express 2015, 23, 22636–22642. [Google Scholar] [CrossRef] [PubMed]
- Yu, C.-H.; Wu, P.-C.; Lee, W. Polymer Stabilization of Uniform Lying Helix Texture in a Bimesogen-Doped Cholesteric Liquid Crystal for Frequency-Modulated Electro-Optic Responses. Materials 2022, 15, 771. [Google Scholar] [CrossRef]
- Yu, C.-H.; Wu, P.-C.; Lee, W. Electro-thermal formation of uniform lying helix alignment in a cholesteric liquid crystal cell. Crystals 2019, 9, 183. [Google Scholar] [CrossRef]
- Liu, Y.-J.; Wu, P.-C.; Lee, W. Spectral variations in selective reflection in cholesteric liquid crystals containing opposite-handed chiral dopants. Mol. Cryst. Liq. Cryst. 2014, 596, 37–44. [Google Scholar] [CrossRef]
- Yang, Z.-H.; Hsiao, Y.-C.; Shen, D.; Lee, W. A thermally tunable narrowband selector based on a chiral nematic containing a binary thermosensitive chiral dopant mixture. Mol. Cryst. Liq. Cryst. 2017, 644, 19–25. [Google Scholar] [CrossRef]
- Li, J.; Gauza, S.; Wu, S.-T. Temperature effect on liquid crystal refractive indices. J. Appl. Phys. 2004, 96, 19–24. [Google Scholar] [CrossRef]
- Shim, K.S.; Heo, J.U.; Jo, S.I.; Lee, Y.-J.; Kim, H.-R.; Kim, J.-H.; Yu, C.-J. Temperature-independent pitch invariance in cholesteric liquid crystal. Opt. Express 2014, 22, 15467–15472. [Google Scholar] [CrossRef]





| Dopant Concentration (wt%) | Ea (eV) | ||
|---|---|---|---|
| E44/R5011 | E44/S5011 | E44/R5011/S5011 | |
| 0 | 0.577 | 0.584 | 0.577 |
| 1 | 0.602 | 0.590 | 0.599 |
| 2 | 0.620 | 0.579 | 0.604 |
| 4 | 0.642 | 0.586 | 0.655 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shaban, H.; Wu, P.-C.; Jia, Y.-F.; Lee, W. Overlooked Ionic Contribution of a Chiral Dopant in Cholesteric Liquid Crystals. Materials 2024, 17, 5080. https://doi.org/10.3390/ma17205080
Shaban H, Wu P-C, Jia Y-F, Lee W. Overlooked Ionic Contribution of a Chiral Dopant in Cholesteric Liquid Crystals. Materials. 2024; 17(20):5080. https://doi.org/10.3390/ma17205080
Chicago/Turabian StyleShaban, Hassanein, Po-Chang Wu, Yi-Fei Jia, and Wei Lee. 2024. "Overlooked Ionic Contribution of a Chiral Dopant in Cholesteric Liquid Crystals" Materials 17, no. 20: 5080. https://doi.org/10.3390/ma17205080
APA StyleShaban, H., Wu, P.-C., Jia, Y.-F., & Lee, W. (2024). Overlooked Ionic Contribution of a Chiral Dopant in Cholesteric Liquid Crystals. Materials, 17(20), 5080. https://doi.org/10.3390/ma17205080








