An Intensity Measure for the Rocking Fragility Analysis of Rigid Blocks Subjected to Floor Motions
Abstract
:1. Introduction
2. Rocking Seismic Response Analysis
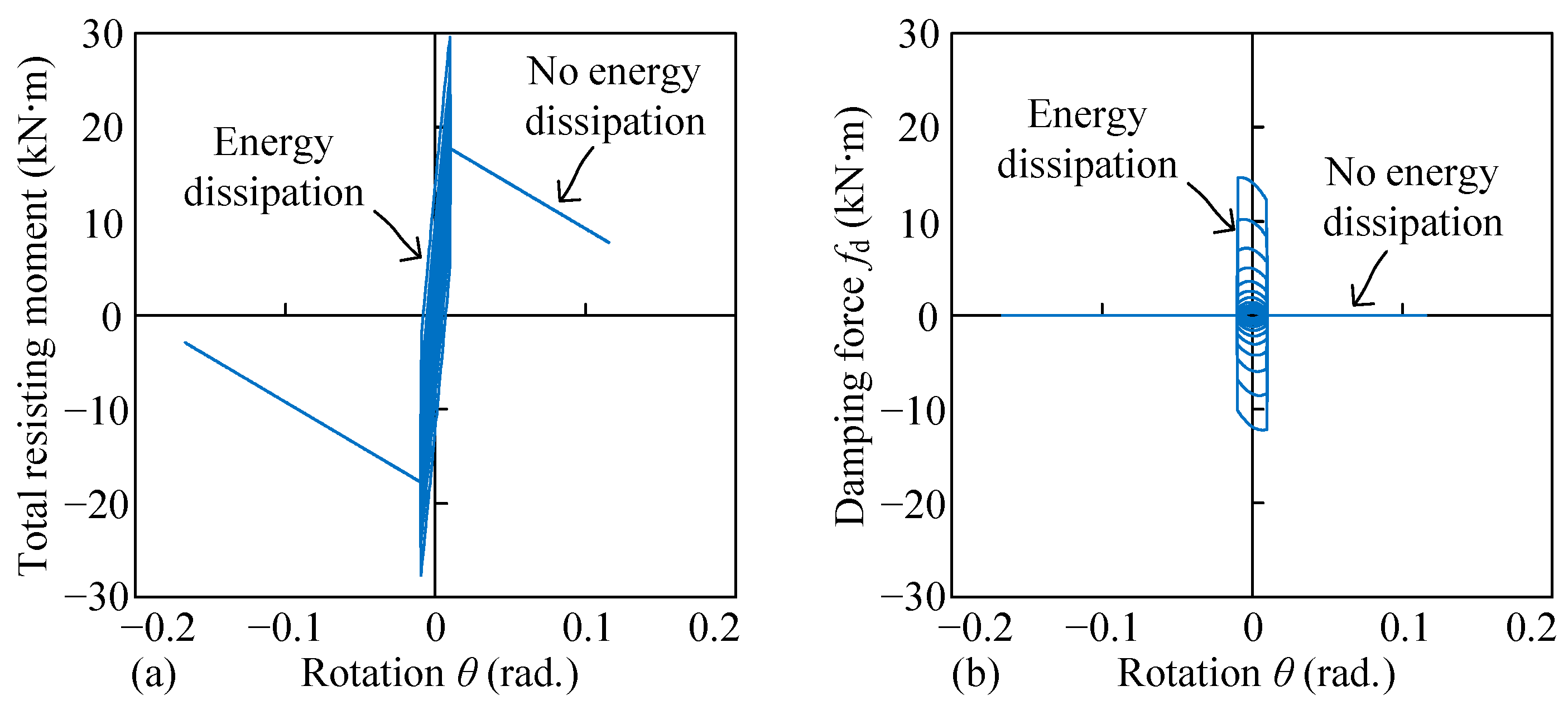
2.1. Numerical Model of the Rocking Block
2.2. Experimental Verifications
3. Rocking Spectra
4. Rocking Fragility Analysis
4.1. Damage Measure and Limit States
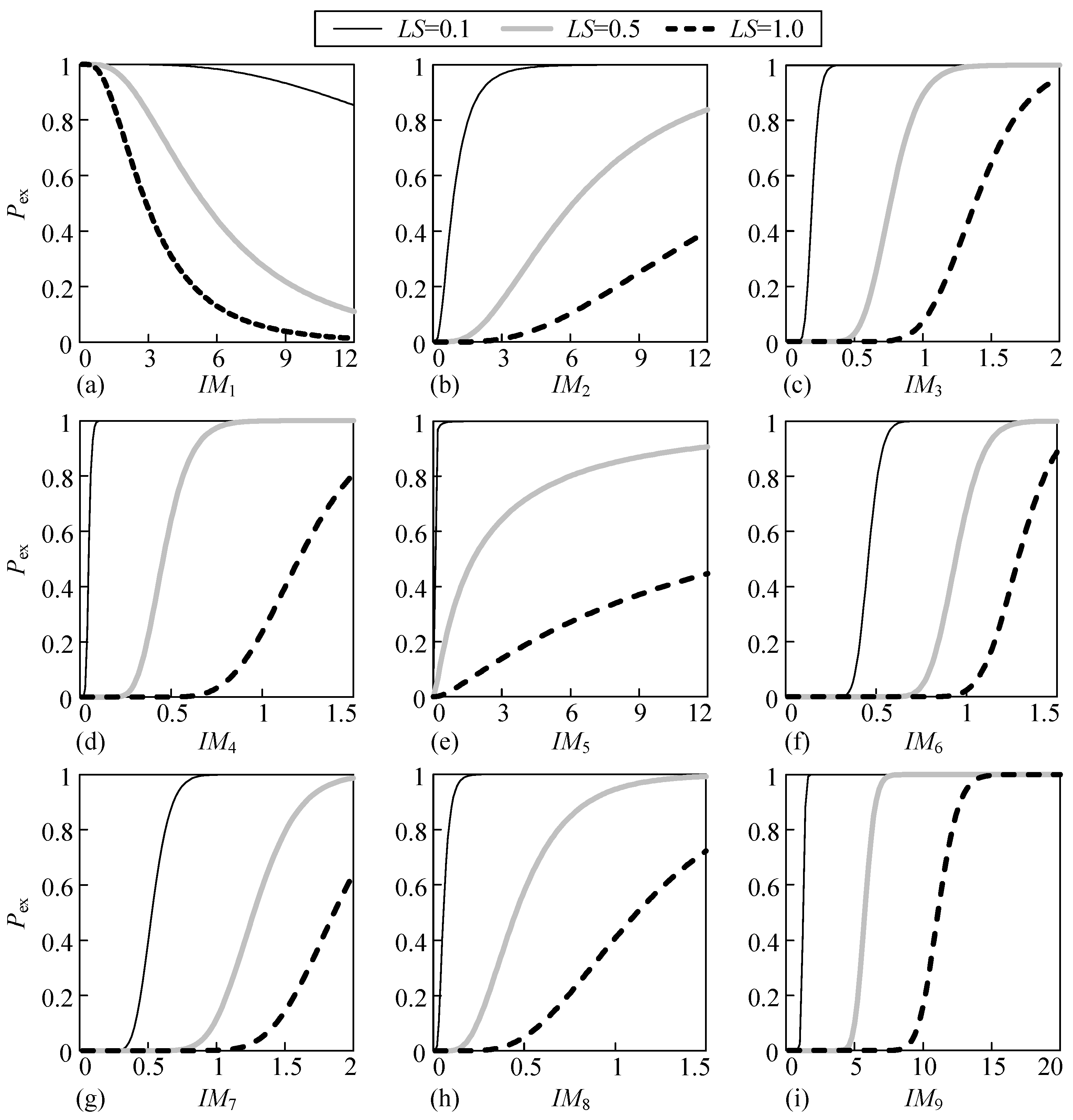
4.2. Intensity Measures
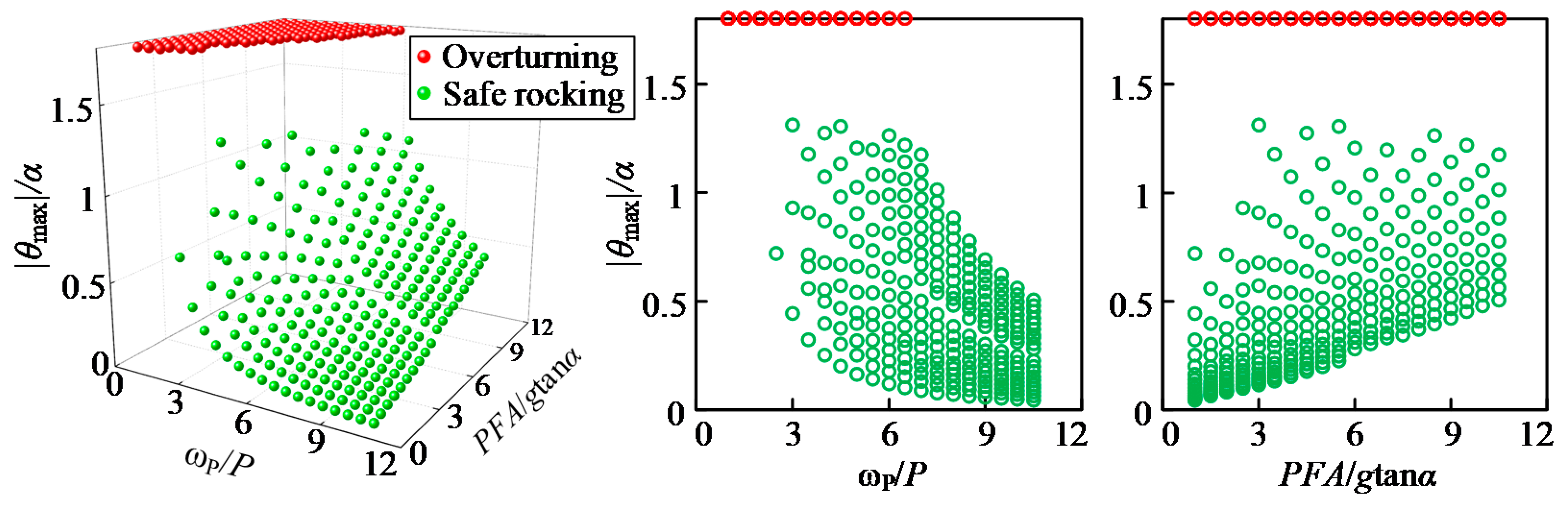
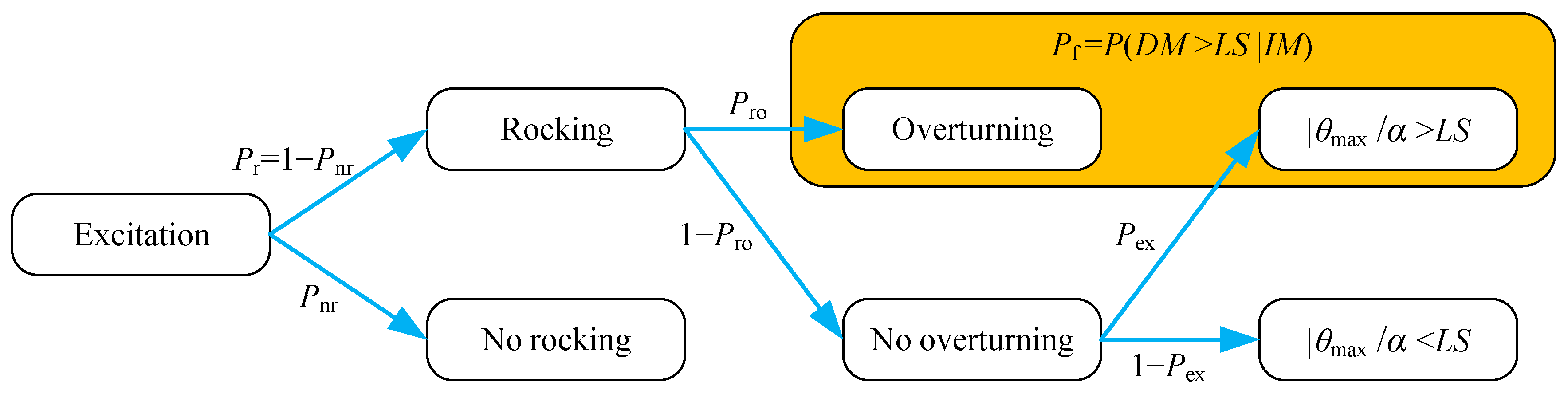
4.3. Probability of Limit State Exceedance during Safe Rocking
5. Conclusions
- An effective IM should take into account not only the excitation characteristics (magnitude PFA, frequency ωP) but also the geometric parameters of the rigid blocks (size parameter R, slenderness parameter α);
- The dimensionless peak floor velocity performs better among the univariate IMs commonly used in rocking fragility analysis. Bivariate IMs perform better overall, but require more computation;
- A novel IM explicitly including excitation characteristics and geometric parameters of the rigid blocks is proposed in this paper. The proposed IM exhibits a much stronger correlation with the DM in logarithmic space; consequently, the proposed IM yields the smallest β in linear regression analysis, which results in the best-performing fragility curves;
- Future studies should aim at evaluating the overturning fragility, as well as the rocking behavior subject to excitations in the real world.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Symbol | Definition |
|---|---|
| 2b | Width |
| 2h | Height |
| Size parameter | |
| Slenderness parameter | |
| Moment of inertia | |
| and | Rotation angle and rotational angular acceleration |
| Horizontal excitation | |
| and | Vertical and horizontal transient distances |
| g | Gravity acceleration |
| M | Restoring moment |
| M0 | Maximum restoring moment |
| and | Angular velocities before and after impacts |
| Restitution coefficient | |
| Rigid-body restitution coefficient | |
| Damping force | |
| Tangent stiffness | |
| Additional moment of inertia | |
| Initial stiffness | |
| Negative stiffness | |
| A large number | |
| δα | Small range around initial position |
| Discrete viscous damping coefficient | |
| θmax | Peak rocking rotation |
| T p | Period of pulse excitation |
| ωP | Circular frequency of pulse excitation |
| P | Block frequency parameter |
| PFA | Peak floor acceleration |
| PFV | Peak floor velocity |
| IM | Intensity measure |
| DM | Damage measure |
| LS | Limit state |
| Conditional probability | |
| Overturning probability | |
| Probability for DM exceeding LS within safe rocking | |
| x | IM value |
| μ | Median value of |
| a and b | Linear regression parameters |
| β | Dispersion |
| R2 | Coefficient of determination |
References
- Taghavi, S.; Miranda, M.M. Response Assessment of Nonstructural Building Elements; Pacific Earthquake Engineering Research Center; University of California: Berkeley, CA, USA, 2003. [Google Scholar]
- Majdalaweyh, S.; Pang, W. Empirical seismic fragility assessment and optimal risk mitigation of building contents. Eng. Struct. 2022, 259, 114183. [Google Scholar] [CrossRef]
- Shenton, H.W., III. Criteria for initiation of slide, rock, and slide-rock rigid-body modes. J. Eng. Mech. 1996, 122, 690–693. [Google Scholar] [CrossRef]
- Newmark, N.M. Effects of earthquakes on dams and embankments. Géotechnique 1965, 15, 139–160. [Google Scholar] [CrossRef] [Green Version]
- Lopez Garcia, D.; Soong, T.T. Sliding fragility of block-type nonstructural components. Part 1: Freestanding components. Earthq. Eng. Struct. Dyn. 2003, 32, 111–129. [Google Scholar] [CrossRef]
- Chaudhuri, S.R.; Hutchinson, T.C. Characterizing frictional behavior for use in predicting the seismic response of unattached equipment. Soil Dyn. Earthq. Eng. 2005, 25, 591–604. [Google Scholar] [CrossRef]
- Konstantinidis, D.; Makris, N. Experimental and analytical studies on the response of freestanding laboratory equipment to earthquake shake. Earthq. Eng. Struct. Dyn. 2009, 38, 827–848. [Google Scholar] [CrossRef]
- Gazetas, G.; Garini, E.; Berrill, J.B.; Apostolou, M. Sliding and overturning potential of Christchurch 2011 earthquake records. Earthq. Eng. Struct. Dyn. 2012, 41, 1921–1944. [Google Scholar] [CrossRef]
- Nagao, T.; Kagano, H.; Hamaguchi, K. Full-Scale Shake Table Test on Furnitures Subjected to Long-Period Earthquake Motions. In Proceedings of the 15th World Conference on Earthquake Engineering, Lisbon, Portugal, 24–28 September 2012. [Google Scholar]
- Konstantinidis, D.; Nikfar, F. Seismic response of sliding equipment and contents in base-isolated buildings subjected to broadband ground motions. Earthq. Eng. Struct. Dyn. 2015, 44, 865–887. [Google Scholar] [CrossRef]
- Nikfar, F.; Konstantinidis, D. Peak sliding demands on unanchored equipment and contents in base-isolated buildings under pulse exitation. J. Struct. Eng. 2017, 143, 04017086. [Google Scholar] [CrossRef]
- Housner, G.W. The behavior of inverted pendulum structures during earthquakes. Bull. Seismol. Soc. Am. 1963, 53, 403–417. [Google Scholar] [CrossRef]
- Filiatrault, A.; Kuan, S.; Tremblay, R. Shake table testing of bookcase–partition wall systems. Can. J. Civ. Eng. 2004, 31, 664–676. [Google Scholar] [CrossRef]
- Arredondo, C.; Jaimes, M.A.; Reinoso, E. A simplified model to evaluate the dynamic rocking behavior of irregular free-standing rigid bodies calibrated with experimental shaking-table tests. J. Earthq. Eng. 2019, 23, 46–71. [Google Scholar] [CrossRef]
- Berto, L.; Meroi, E.; Rocca, I.; Saetta, A. Rocking activation of free standing elements in real conditions: A safe experimentally-based acceleration limit. Eng. Struct. 2021, 226, 111331. [Google Scholar] [CrossRef]
- Peña, F.; Prieto, F.; Lourenço, P.B.; Costa, A.C.; Lemos, J.V. On the dynamics of rocking motion of single rigid-block structures. Earthq. Eng. Struct. Dyn. 2007, 36, 2383–2399. [Google Scholar] [CrossRef] [Green Version]
- Kuo, K.C.; Suzuki, Y.; Katsuragi, S.; Yao, G.C. Shake table tests on clutter levels of typical medicine shelves and contents subjected to earthquakes. Earthq. Eng. Struct. Dyn. 2011, 40, 1367–1386. [Google Scholar] [CrossRef]
- Konstantinidis, D.; Makris, N. Experimental and analytical studies on the response of 1/4-scale models of freestanding laboratory equipment subjected to strong earthquake shake. Bull. Earthq. Eng. 2010, 8, 1457–1477. [Google Scholar] [CrossRef] [Green Version]
- Bachmann, J.A.; Blöchlinger, P.; Wellauer, M.; Vassiliou, M.F.; Stojadinovic, B. Experimental investigation of the seismic response of a column rocking and rolling on a concave base. In Proceedings of the ECCOMAS Congress, VII European Congress on Computational Methods in Applied Sciences and Engineering, Crete Island, Greece, 5–10 June 2016; pp. 5023–5062. [Google Scholar]
- Huang, B.; Pan, Q.; Lu, W.; Shen, F. Free-rocking tests of a freestanding object with variation of center of gravity. Earthq. Eng. Struct. Dynamics. 2021, 50, 3015–3040. [Google Scholar] [CrossRef]
- Vassiliou, M.F.; Broccardo, M.; Cengiz, C.; Dietz, M.; Dihoru, L.; Gunay, S.; Stojadinovic, B. Shake table testing of a rocking podium: Results of a blind prediction contest. Earthq. Eng. Struct. Dyn. 2021, 50, 1043–1062. [Google Scholar] [CrossRef]
- Di Sarno, L.; Magliulo, G.; D’Angela, D. Experimental assessment of the seismic performance of hospital cabinets using shake table testing. Earthq. Eng. Struct. Dyn. 2019, 48, 103–123. [Google Scholar] [CrossRef] [Green Version]
- Vassiliou, M.F.; Cengiz, C.; Dietz, M.; Dihoru, L.; Broccardo, M.; Mylonakis, G.; Stojadinovic, B. Data set from shake table tests of free-standing rocking bodies. Earthq. Spectra 2021, 37, 2971–2987. [Google Scholar] [CrossRef]
- Liu, P.; Xue, W.; Pang, H.; Zhang, Y.M.; Chen, H.T.; Yang, W.G. Seismic overturning fragility analysis for freestanding building contents subjected to horizontal bidirectional floor motions. Soil Dyn. Earthq. Eng. 2022, 161, 107414. [Google Scholar] [CrossRef]
- Ishiyama, Y. Motions of rigid bodies and criteria for overturning by earthquake excitations. Earthq. Eng. Struct. Dyn. 1982, 10, 635–650. [Google Scholar] [CrossRef]
- Thiers-Moggia, R.; Málaga-Chuquitaype, C. Dynamic response of post-tensioned rocking structures with inerters. Int. J. Mech. Sci. 2020, 187, 105927. [Google Scholar] [CrossRef]
- Chae, Y.B.; Kim, J.K. Implementation of configuration dependent stiffness proportional damping for the dynamics of rigid multi-block systems. Earthq. Eng. Eng. Vib. 2003, 2, 87–97. [Google Scholar] [CrossRef]
- Di Egidio, A.; Zulli, D.; Contento, A. Comparison between the seismic response of 2D and 3D models of rigid blocks. Earthq. Eng. Eng. Vib. 2014, 13, 151–162. [Google Scholar] [CrossRef]
- Vetr, M.G.; Nouri, A.R.; Kalantari, A. Seismic evaluation of rocking structures through performance assessment and fragility analysis. Earthq. Eng. Eng. Vib. 2016, 15, 115–127. [Google Scholar] [CrossRef]
- Fragiadakis, M.; Kolokytha, M. Diamantopoulos, S. Seismic risk assessment of rocking building contents of multistorey buildings. Procedia Eng. 2017, 199, 3534–3539. [Google Scholar] [CrossRef]
- Gesualdo, A.; Iannuzzo, A.; Minutolo, V.; Monaco, M. Rocking of freestanding objects: Theoretical and experimental comparisons. J. Theor. Appl. Mech. 2018, 56, 977–991. [Google Scholar] [CrossRef]
- Kasinos, S.; Lombardo, M.; Makris, N.; Palmeri, A. Dynamic response analysis of nonlinear secondary oscillators to idealised seismic pulses. Earthq. Eng. Struct. Dyn. 2020, 49, 1473–1495. [Google Scholar] [CrossRef]
- Reggiani Manzo, N.; Vassiliou, M.F. Simplified analysis of bilinear elastic systems exhibiting negative stiffness behavior. Earthq. Eng. Struct. Dyn. 2021, 50, 580–600. [Google Scholar] [CrossRef]
- D’Angela, D.; Magliulo, G.; Cosenza, E. Towards a reliable seismic assessment of rocking components. Eng. Struct. 2021, 230, 111673. [Google Scholar] [CrossRef]
- Aghagholizadeh, M. A finite element model for seismic response analysis of vertically-damped rocking-columns. Eng. Struct. 2020, 219, 110894. [Google Scholar] [CrossRef]
- Vanin, F.; Penna, A.; Beyer, K. A three-dimensional macroelement for modelling the in-plane and out-of-plane response of masonry walls. Earthq. Eng. Struct. Dyn. 2020, 49, 1365–1387. [Google Scholar] [CrossRef]
- Galvez, F.; Sorrentino, L.; Dizhur, D.; Ingham, J.M. Seismic rocking simulation of unreinforced masonry parapets and façades using the discrete element method. Earthq. Eng. Struct. Dyn. 2022, 51, 1840–1856. [Google Scholar] [CrossRef]
- Scattarreggia, N.; Malomo, D.; DeJong, M.J. A new Distinct Element meso-model for simulating the rocking-dominated seismic response of RC columns. Earthq. Eng. Struct. Dyn. 2022, 1–11. [Google Scholar] [CrossRef]
- Vlachakis, G.; Giouvanidis, A.I.; Mehrotra, A.; Lourenço, P.B. Numerical block-based simulation of rocking structures using a novel universal viscous damping model. J. Eng. Mech. 2021, 147, 04021089. [Google Scholar] [CrossRef]
- Aslam, M.; Godden, W.G.; Scalise, D.T. Earthquake rocking response of rigid bodies. ASCE J. Struct. Div. 1980, 106, 377–392. [Google Scholar] [CrossRef]
- Yim, C.; Chopra, A.K.; Penzien, J. Rocking response of rigid blocks to earthquakes. Earthq. Eng. Struct. Dyn. 1980, 8, 565–587. [Google Scholar] [CrossRef]
- Ibarra, L. Seismic Performance of Dry Casks Storage for Long-term Exposure. Final Report; University of Utah: Salt Lake City, UT, USA, 2016. [Google Scholar]
- Bachmann, J.A.; Strand, M.; Vassiliou, M.F.; Broccardo, M.; Stojadinović, B. Is rocking motion predictable? Earthq. Eng. Struct. Dyn. 2018, 47, 535–552. [Google Scholar] [CrossRef]
- Giannini, R.; Masiani, R. Risposta in frequenza del blocco rigido: Stabilita delle soluzioni. In Proceedings of the 10th Conference of the Italian Association of Theoretical and Applied Mechanics, Pisa, Italy, 2–6 July 1990. [Google Scholar]
- Acikgoz, S.; Ma, Q.; Palermo, A.; DeJong, M.J. Experimental identification of the dynamic characteristics of a flexible rocking structure. J. Earthq. Eng. 2016, 20, 1199–1221. [Google Scholar] [CrossRef]
- Roh, H.; Cimellaro, G.P. Seismic fragility evaluation of RC frame structures retrofitted with controlled concrete rocking column and damping technique. J. Earthq. Eng. 2011, 15, 1069–1082. [Google Scholar] [CrossRef]
- Deng, L.; Kutter, B.L.; Kunnath, S.K. Probabilistic seismic performance of rocking-foundation and hinging-column bridges. Earthq. Spectra 2012, 28, 1423–1446. [Google Scholar] [CrossRef] [Green Version]
- Cosenza, E.; Di Sarno, L.; Maddaloni, G.; Magliulo, G.; Petrone, C.; Prota, A. Shake table tests for the seismic fragility evaluation of hospital rooms. Earthq. Eng. Struct. Dyn. 2015, 44, 23–40. [Google Scholar] [CrossRef]
- Feng, M.Q.; Shinozuka, M.; Kim, H.K.; Kim, S.H. Statistical analysis of fragility curves. J. Eng. Mech. 2000, 126, 1224–1231. [Google Scholar]
- Dimitrakopoulos, E.G.; Paraskeva, T.S. Dimensionless fragility curves for rocking response to near-fault excitations. Earthq. Eng. Struct. Dyn. 2015, 44, 2015–2033. [Google Scholar] [CrossRef]
- Petrone, C.; Di Sarno, L.; Magliulo, G.; Cosenza, E. Numerical modelling and fragility assessment of typical freestanding building contents. Bull. Earthq. Eng. 2017, 15, 1609–1633. [Google Scholar] [CrossRef] [Green Version]
- Sieber, M.; Vassiliou, M.F.; Anastasopoulos, I. Intensity measures, fragility analysis and dimensionality reduction of rocking under far-field ground motions. Earthq. Eng. Struct. Dyn. 2022, 51, 3639–3657. [Google Scholar] [CrossRef]
- Liu, H.; Huang, Y.; Qu, Z. A discretely damped SDOF model for the rocking response of freestanding blocks. Earthq. Eng. Eng. Vib. 2022, 21, 729–740. [Google Scholar] [CrossRef]
- Zhang, J.; Makris, N. Rocking response of freestanding blocks under cycloidal pulses. J. Eng. Mech. 2001, 127, 473–483. [Google Scholar]
- Weir, G.; McGavin, P. The coefficient of restitution for the idealized impact of a spherical, nano-scale particle on a rigid plane. Proc. R. Soc. A Math. Phys. Eng. Sci. 2008, 464, 1295–1307. [Google Scholar] [CrossRef]
- Čeh, N.; Jelenić, G.; Bićanić, N. Analysis of restitution in rocking of single rigid blocks. Acta Mech. 2018, 229, 4623–4642. [Google Scholar] [CrossRef]
- Diamantopoulos, S.; Fragiadakis, M. Seismic response assessment of rocking systems using single degree-of-freedom oscillators. Earthq. Eng. Struct. Dyn. 2019, 48, 689–708. [Google Scholar] [CrossRef]
- Makris, N.; Konstantinidis, D. The rocking spectrum and the limitations of practical design methodologies. Earthq Eng Struct Dyn. 2003, 32, 265–289. [Google Scholar] [CrossRef]
- Vassiliou, M.F.; Mackie, K.R.; Stojadinović, B. Dynamic response analysis of solitary flexible rocking bodies: Modeling and behavior under pulse-like ground excitation. Earthq. Eng. Struct. Dyn. 2014, 43, 1463–1481. [Google Scholar] [CrossRef]
- McKenna, F. OpenSees: A Framework for Earthquake Engineering Simulation. Comput. Sci. Eng. 2011, 13, 58–66. [Google Scholar] [CrossRef]
- Nasi, K.T.J. Stability of Rocking Structures. Master’s thesis, Purdue University, West Lafayette, IN, USA, 2011. [Google Scholar]
- Klaboe, K.; Pujol, S.; Laughery, L. Stability of Rocking Structures. Purdue Univ. Res. Repos. 2017. [Google Scholar] [CrossRef]
- Klaboe, K.; Pujol, S.; Laughery, L. Seismic Response of Rocking Blocks. Earthq. Spectra 2018, 34, 1051–1063. [Google Scholar] [CrossRef]
- Linde, S.A.; Konstantinidis, D.; Tait, M.J. Rocking response of unanchored building contents considering horizontal and vertical excitation. J. Struct. Eng. 2020, 146, 04020175. [Google Scholar] [CrossRef]





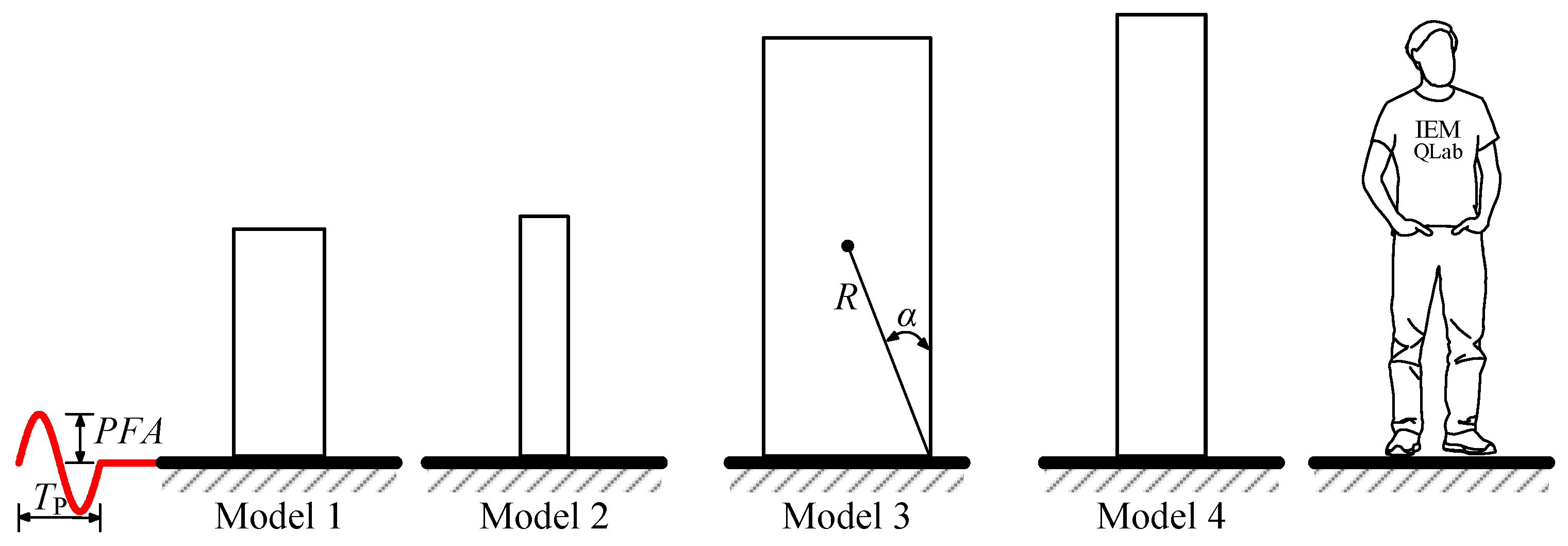


| 2b (m) | 2h (m) | R (m) | α | P | |
|---|---|---|---|---|---|
| Model 1 | 0.3785 | 0.9462 | 0.5095 | 0.3805 | 3.7981 |
| Model 2 | 0.1999 | 0.9993 | 0.5095 | 0.1974 | 3.7981 |
| Model 3 | 0.6971 | 1.7427 | 0.9385 | 0.3805 | 2.7986 |
| Model 4 | 0.3681 | 1.8405 | 0.9385 | 0.1974 | 2.7986 |
| IM | a | b | β | R2 |
|---|---|---|---|---|
| IM1 | 1.1730 | −1.0999 | 0.7083 | 0.1846 |
| IM2 | −2.1786 | 0.8195 | 0.5597 | 0.4908 |
| IM3 | −0.3761 | 1.1643 | 0.2569 | 0.8928 |
| IM4 | −0.1313 | 0.7151 | 0.1827 | 0.9457 |
| IM5 | −0.8771 | 0.3274 | 0.4765 | 0.6310 |
| IM6 | −0.5569 | 2.2191 | 0.2829 | 0.8699 |
| IM7 | −1.1339 | 1.8261 | 0.3689 | 0.7788 |
| IM8 | −0.0879 | 0.7671 | 0.3782 | 0.7675 |
| IM9 | −2.5232 | 1.0484 | 0.1113 | 0.9799 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, H.; Huang, Y.; Liu, X. An Intensity Measure for the Rocking Fragility Analysis of Rigid Blocks Subjected to Floor Motions. Sustainability 2023, 15, 2418. https://doi.org/10.3390/su15032418
Liu H, Huang Y, Liu X. An Intensity Measure for the Rocking Fragility Analysis of Rigid Blocks Subjected to Floor Motions. Sustainability. 2023; 15(3):2418. https://doi.org/10.3390/su15032418
Chicago/Turabian StyleLiu, Hanquan, Yong Huang, and Xiaohui Liu. 2023. "An Intensity Measure for the Rocking Fragility Analysis of Rigid Blocks Subjected to Floor Motions" Sustainability 15, no. 3: 2418. https://doi.org/10.3390/su15032418




