Pre-Compensation of Thermal Error for Laser-Assisted Diamond Turning
Abstract
:1. Introduction
2. Theoretical Approaches
2.1. Tool-Setting Error Influence
2.2. Structural Thermal Expansion
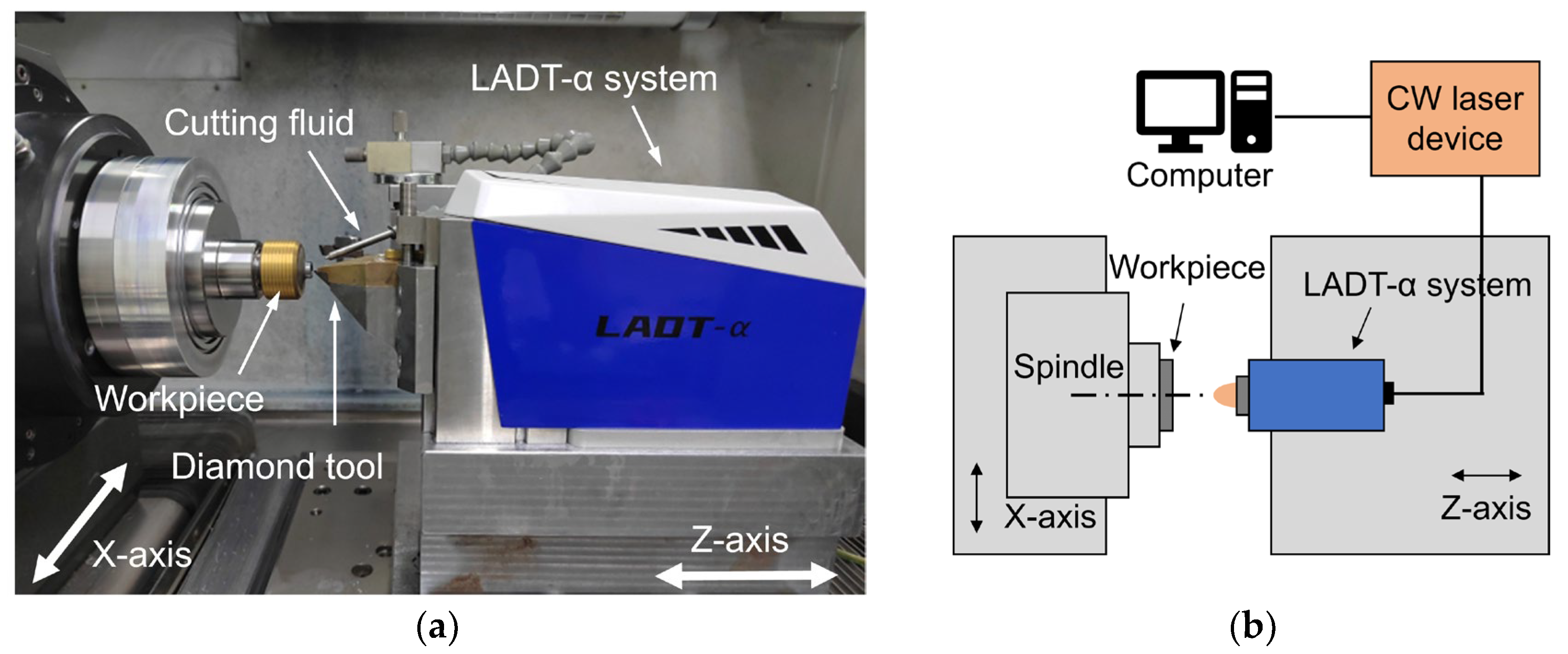
3. Experimental Setup
4. Results and Discussion
5. Conclusions
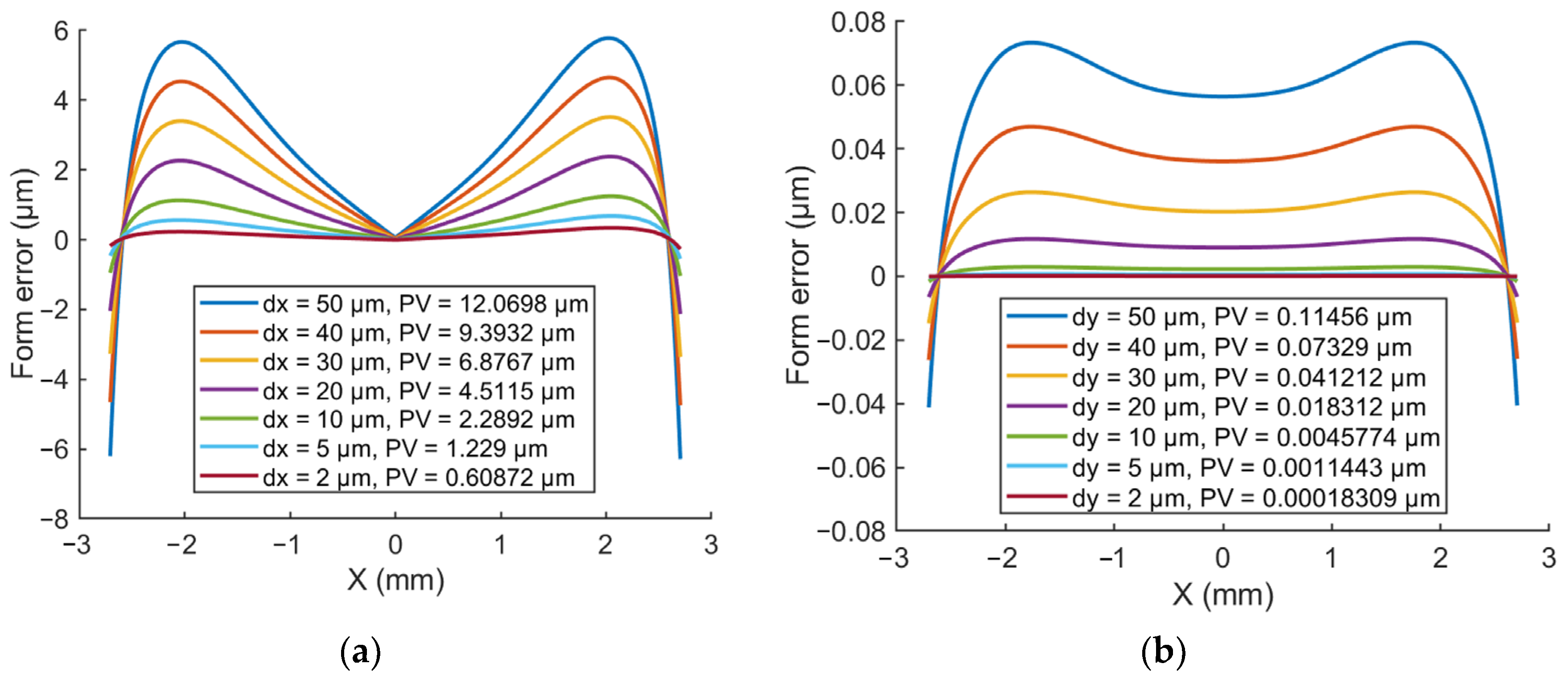
- The tool shank and tool holder will be heated to 384 K, resulting in large thermal drifts in the diamond tool tip of 10.9 and 11.7 μm in the Y-axis and Z-axis directions, respectively.
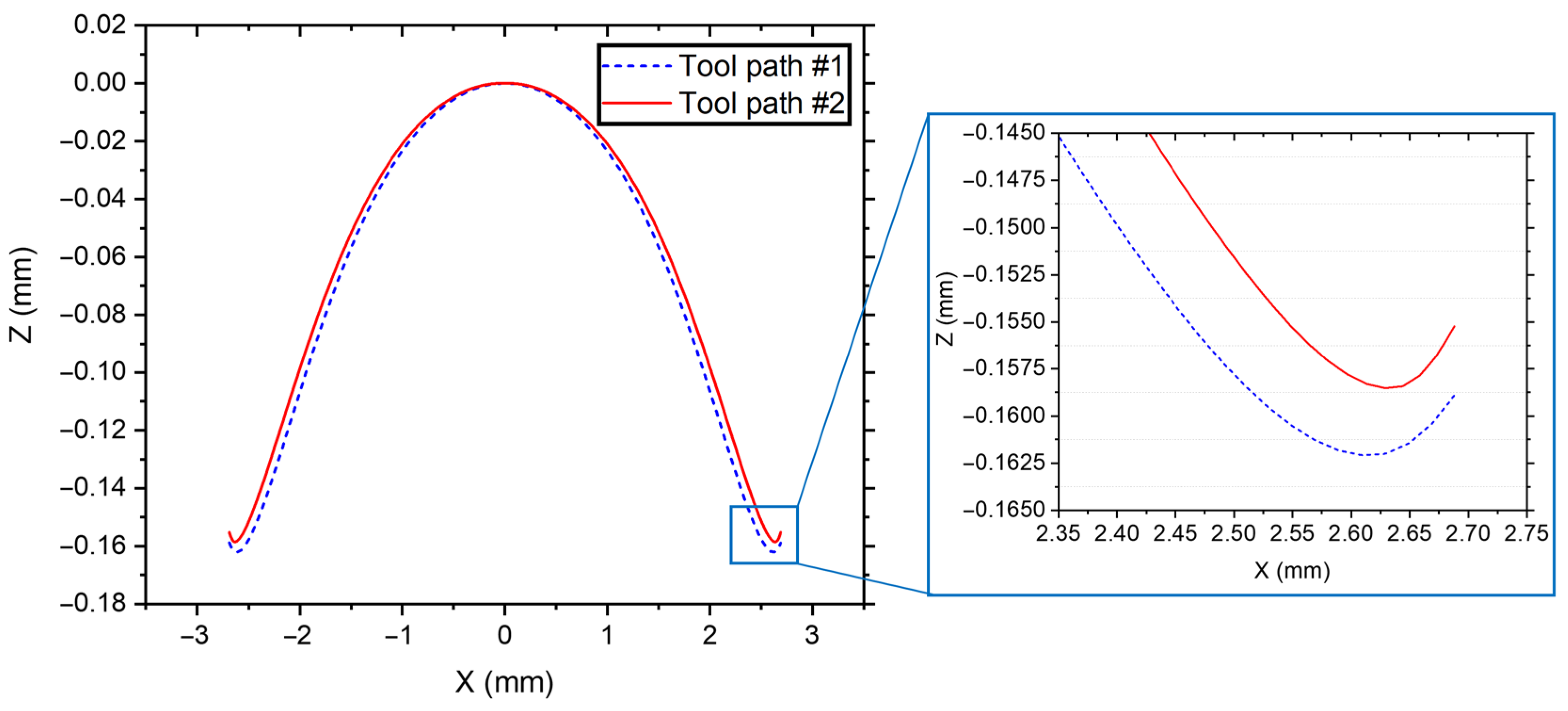
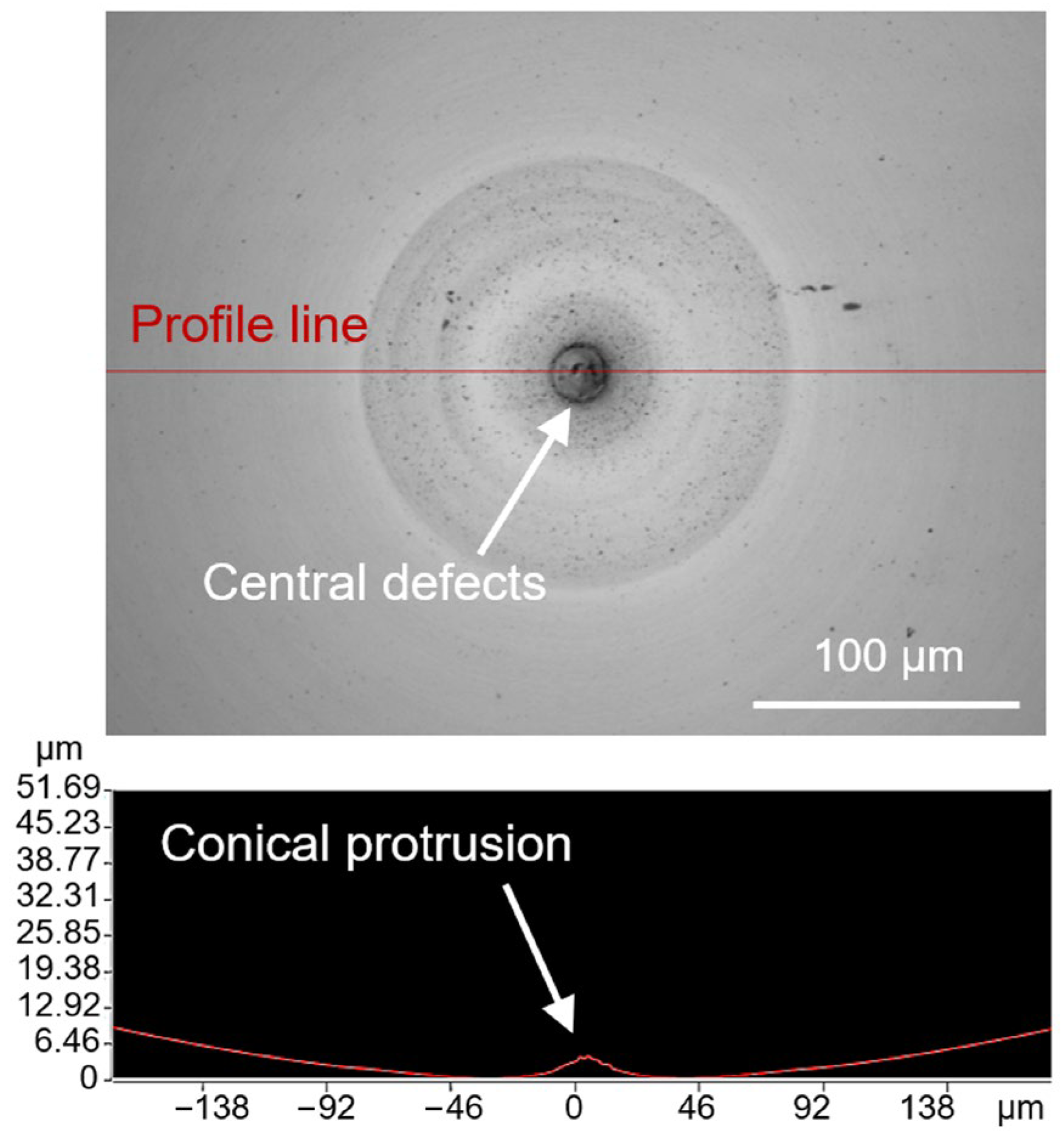
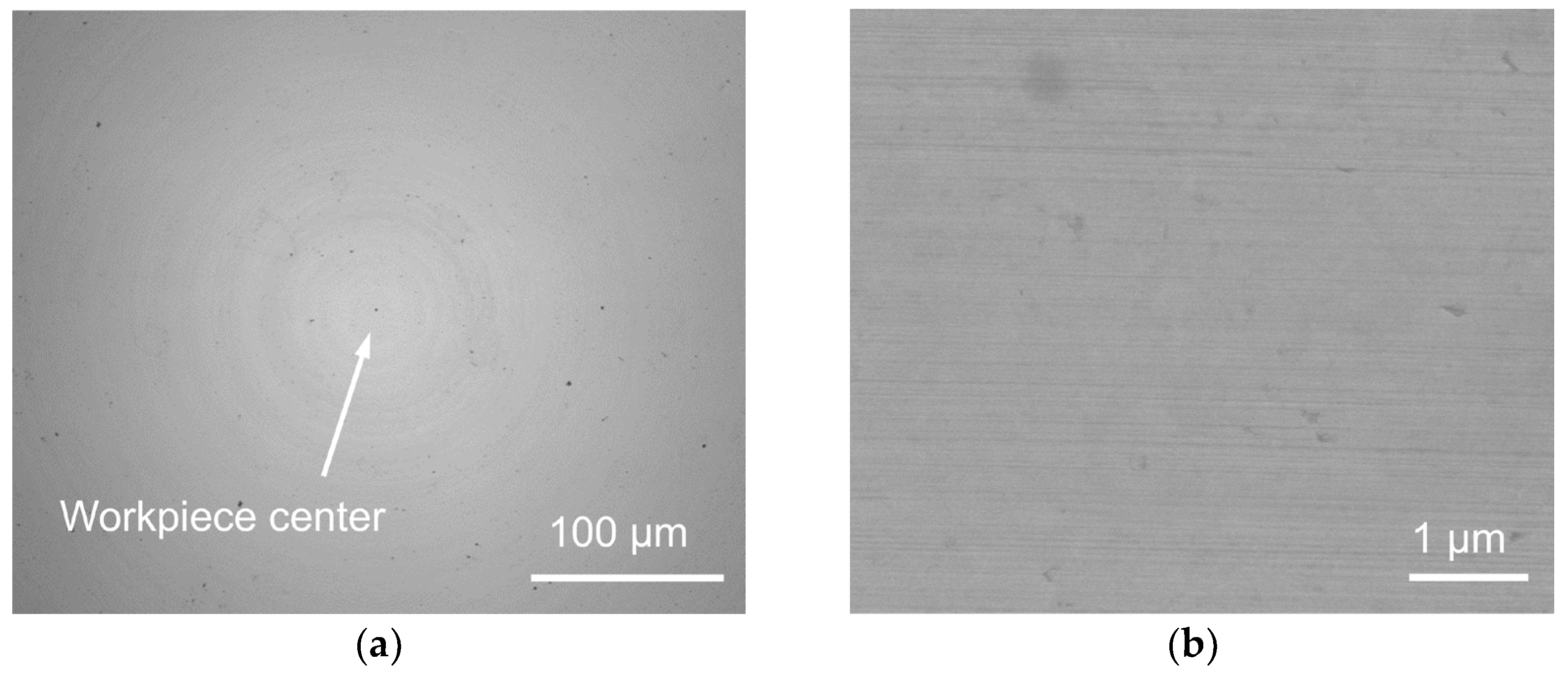
- The workpiece central conical protrusion defects can be effectively eliminated by tool-setting height pre-compensation according to the FEA simulation results.
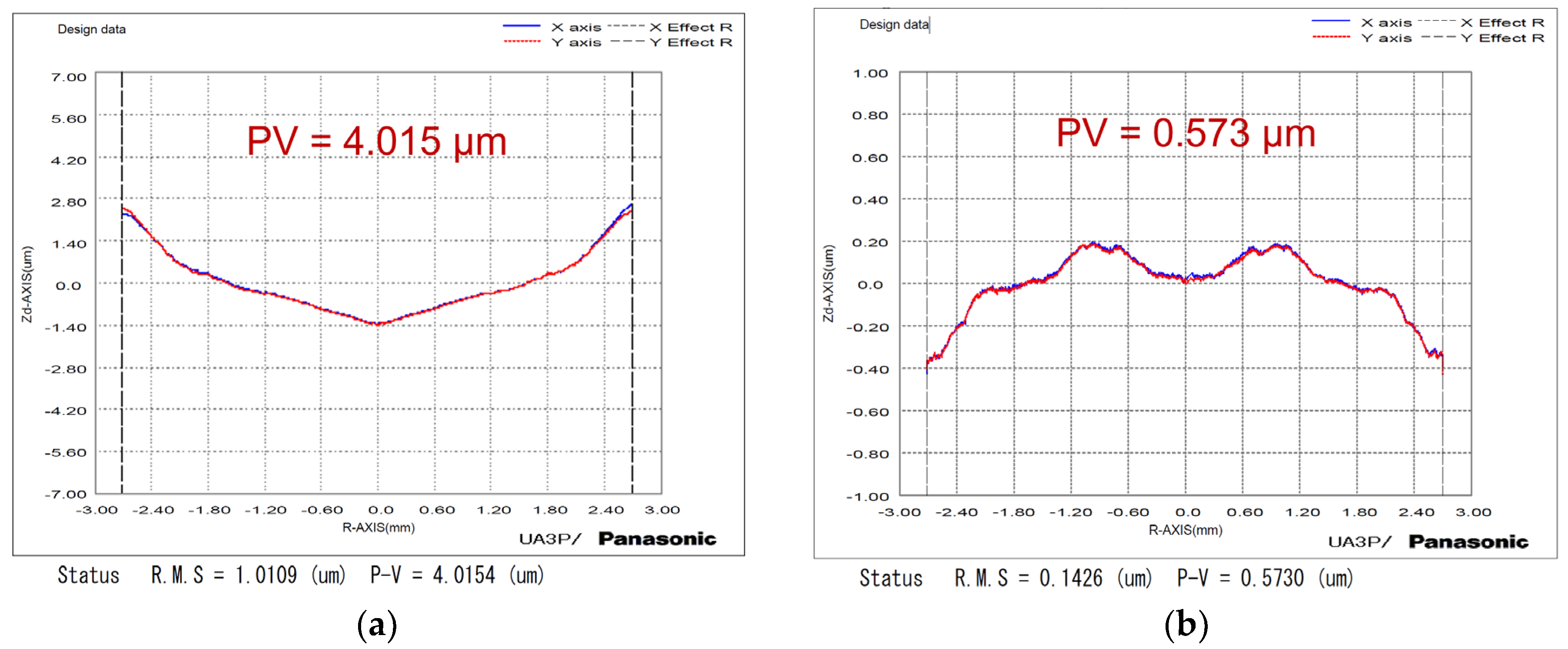
- The form accuracy of the machined aspherical workpiece is effectively improved by 85.7% to a PV of 0.573 μm using the pre-compensated tool path #2.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhang, X.J.; Hu, H.X.; Wang, X.K.; Luo, X.; Zhang, G.; Zhao, W.; Wang, X.; Liu, Z.; Xiong, L.; Qi, E.; et al. Challenges and strategies in high-accuracy manufacturing of the world’s largest SiC aspheric mirror. Light Sci. Appl. 2022, 11, 2873–2885. [Google Scholar] [CrossRef] [PubMed]
- Lukman, N.A.; Khaled, A.E.H.; Abubakar, I.J.; Odedeyi, P.B.; Liman, M.M.; Olaniyan, T.A. Ultra-precision diamond turning of optical silicon—A review. Int. J. Adv. Manuf. Technol. 2018, 96, 173–208. [Google Scholar]
- Zhao, H.H.; Gain, A.K.; Li, Z.; Zhang, L. Wear of mold surfaces: Interfacial adhesion in precision glass molding. Wear 2023, 204847, 524–525. [Google Scholar] [CrossRef]
- Guo, B.; Zhao, Q.L.; Li, H.L. Ultra-precision grinding of binderless tungsten carbide aspheric mold. J. Mech. Eng. 2014, 50, 190–195. [Google Scholar] [CrossRef]
- Doetz, M.; Dambon, O.; Klocke, F.; Vogt, C.; Rascher, R.; Faehnle, O. Ductile mode single point diamond turning (SPDT) of binderless tungsten carbide molds. In Proceedings of the SPIE 10742, Optical Manufacturing and Testing XII, San Diego, CA, USA, 20–22 August 2018; Volume 107420E. [Google Scholar]
- Huang, H.; Li, X.L.; Mu, D.K. Science and art of ductile grinding of brittle solids. Int. J. Mach. Tools Manuf. 2021, 161, 103675. [Google Scholar] [CrossRef]
- Yu, S.M.; Yao, P.; Xu, J.M.; Wang, W.; Li, Y.; Chu, D.; Qu, S.; Huang, C. Profile error compensation in ultra-precision grinding of aspherical-cylindrical lens array based on the real-time profile of wheel and normal residual error. J. Mater. Process. Technol. 2023, 312, 117849. [Google Scholar] [CrossRef]
- Zhang, L.; Yi, A.Y.; Yan, J.W. Flexible fabrication of Fresnel micro-lens array by off-spindle-axis diamond turning and precision glass molding. Precis. Eng. 2022, 74, 186–194. [Google Scholar] [CrossRef]
- Kode, S.K.; Ellis, J.D.; Mohammadi, H. Laser assisted diamond turning of tungsten carbide and the material properties required to obtain optical surface finish suitable for lens molds. Polym. Opt. Molded Glass Opt. Des. Fabr. Mater. 2022, 1221906, 28–32. [Google Scholar]
- Mohammadi, H.; Ravindra, D.; Kode, S.K.; Patten, J.A. Experimental work on micro laser-assisted diamond turning of silicon (111). J. Manuf. Process. 2015, 19, 125–128. [Google Scholar] [CrossRef]
- Geng, R.W.; Xie, Q.M.; Yang, X.J.; Zhang, R.; Zhang, W.; Qiu, H.; You, J.; Jiao, S. Experimental study of the feasibility of micro-laser-assisted machining in polycrystal ZnSe ceramics. Eighth Symp. Nov. Photoelectron. Detect. Technol. Appl. 2022, 12169, 3350–3360. [Google Scholar]
- You, K.Y.; Fang, F.Z. High effective laser assisted diamond turning of binderless tungsten carbide. J. Mater. Process. Technol. 2022, 302, 117505. [Google Scholar] [CrossRef]
- Xu, J.F.; Huang, K.; Zheng, Z.D.; Lin, C.T.; Zhang, J.G.; Xiao, J.F.; Chen, X. Review of field-assisted ultraprecision machining difficult-to-machine materials. Sci. Sin. Technol. 2022, 52, 829–853. [Google Scholar] [CrossRef]
- Jiang, Z.D.; Li, C.S.; Sun, L.; Duan, R.Z.; Kang, C.W.; Chen, S.S.; Lin, Q.J.; Yang, S.M. Ultra-Precision Machining Technology and Equipment for High-End Optical Elements. Strateg. Study Chin. Acad. Eng. 2023, 25, 131–141. [Google Scholar] [CrossRef]
- Bauer, A.; Schiesser, E.M.; Rolland, J.P. Starting geometry creation and design method for freeform optics. Nat. Commun. 2018, 9, 1756. [Google Scholar] [CrossRef]
- Bos, A.; Henselmans, R.; Rosielle, P.C.J.N. Nanometre-accurate form measurement machine for E-ELT M1 segments. Precis. Eng. 2015, 40, 14–25. [Google Scholar] [CrossRef]
- O’Toole, L.; Fang, F.Z. Optimal tool design in micro-milling of difficult-to-machine materials. Adv. Manuf. 2022, 30, 40413–40424. [Google Scholar] [CrossRef]
- Wang, Z.Y.; Chen, Z.Z.; Zhang, X.Q. Profile Compensation for Single-Point Diamond Turning of Microlens Array. Nanomanuf. Metrol. 2022, 5, 403–411. [Google Scholar] [CrossRef]
- Sato, Y.; Yan, J.W. Toolpath generation and optimization for freeform surface diamond turning based on an independently controlled fast tool servo. Int. J. Extrem. Manuf. 2022, 4, 025102. [Google Scholar] [CrossRef]
- Mayr, J.; Jedrzejewski, J.; Uhlmann, E. Thermal issues in machine tools. CIRP Ann.-Manuf. Technol. 2012, 61, 771–791. [Google Scholar] [CrossRef]
- Gong, H.; Wang, Y.; Song, L.; Fang, F.Z. Spiral tool path generation for diamond turning optical freeform surfaces of quasi-revolution. Comput.-Aided Des. 2015, 59, 15–22. [Google Scholar] [CrossRef]
- Fang, F.Z.; Zhang, X.D.; Hu, X.T. Cylindrical coordinate machining of optical freeform surfaces. Opt. Express 2008, 16, 7323–7329. [Google Scholar] [CrossRef]
- Guo, P.; Liu, M.Y.; Zhou, Y.P.; Xiong, Z.W.; Zhang, S.J.; To, S. Two crucial suggestions on tool paths in slow-tool-servo diamond cutting of micro-structured functional surfaces. J. Manuf. Process. 2023, 95, 415–420. [Google Scholar] [CrossRef]
- Zhou, T.F.; He, Y.P.; Wang, T.X.; Zhu, Z.; Xu, R.; Yu, Q.; Zhao, B.; Zhao, W.; Liu, P.; Wang, X. A review of the techniques for the mold manufacturing of micro/nanostructures for precision glass molding. Int. J. Extrem. Manuf. 2021, 3, 042002. [Google Scholar] [CrossRef]
- Peixoto, C.; Valentim, P.T.; Sousa, P.C.; Dias, D.; Araújo, C.; Pereira, D.; Machado, C.F.; Pontes, A.J.; Santos, H.; Cruz, S. Injection molding of high-precision optical lenses: A review. Precis. Eng. 2022, 76, 29–51. [Google Scholar] [CrossRef]
- Su, L.J.; Wang, F.; He, P.; Dambon, O.; Klocke, F.; Yi, A.Y. An integrated solution for mold shape modification in precision glass molding to compensate refractive index change and geometric deviation. Opt. Lasers Eng. 2014, 53, 98–103. [Google Scholar] [CrossRef]
- Zhang, Y.; You, K.Y.; Fang, F.Z. Pre-Compensation of Mold in Precision Glass Molding Based on Mathematical Analysis. Micromachine 2020, 11, 1069. [Google Scholar] [CrossRef]
- Guo, J. Ultra-precision finishing of micro-aspheric mold using a magnetostrictive vibrating polisher. CIRP Ann.-Manuf. Technol. 2019, 61, 371–374. [Google Scholar] [CrossRef]
- Ke, X.L.; Wu, W.; Shi, C.C.; Li, K.; Yu, Y.H.; Wang, T.Y.; Zhong, B.; Wang, Z.Z.; Guo, J.; Cheung, C.F.; et al. Theoretical and experimental investigation of material removal in semi-rigid bonnet polishing of binderless tungsten carbide. J. Mater. Res. Technol. 2023, 24, 1597–1611. [Google Scholar] [CrossRef]


| Parameters | Value |
|---|---|
| Tool shank material | WC |
| Tool shank thermal expansivity | 4.5 × 10−6 1/K |
| Tool holder material | Stainless steel |
| Tool holder thermal expansivity | 12.6 × 10−6 1/K |
| Initial temperature | 293 K |
| Laser power | 18 W |
| Laser beam diameter | 170 μm |
| Emissivity | 0.8 |
| Convection coefficient | 10 W/(m2·K) |
| Parameters | Value |
|---|---|
| Workpiece material | Binderless WC |
| Workpiece aperture | 10 mm |
| Surface form | Aspherical (Convex with edge inversion) |
| Tool nose radius | 0.3 mm |
| Tool rake angle | −35° |
| Feedrate | 2 mm/min |
| Laser power | 10 W |
| Cutting direction | Edge-to-center |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
You, K.; Liu, G.; Yan, G.; Fang, F.; Wang, W.; Du, L.; Ding, J. Pre-Compensation of Thermal Error for Laser-Assisted Diamond Turning. Micromachines 2023, 14, 1843. https://doi.org/10.3390/mi14101843
You K, Liu G, Yan G, Fang F, Wang W, Du L, Ding J. Pre-Compensation of Thermal Error for Laser-Assisted Diamond Turning. Micromachines. 2023; 14(10):1843. https://doi.org/10.3390/mi14101843
Chicago/Turabian StyleYou, Kaiyuan, Guangyu Liu, Guangpeng Yan, Fengzhou Fang, Wei Wang, Li Du, and Jiexiong Ding. 2023. "Pre-Compensation of Thermal Error for Laser-Assisted Diamond Turning" Micromachines 14, no. 10: 1843. https://doi.org/10.3390/mi14101843
APA StyleYou, K., Liu, G., Yan, G., Fang, F., Wang, W., Du, L., & Ding, J. (2023). Pre-Compensation of Thermal Error for Laser-Assisted Diamond Turning. Micromachines, 14(10), 1843. https://doi.org/10.3390/mi14101843







