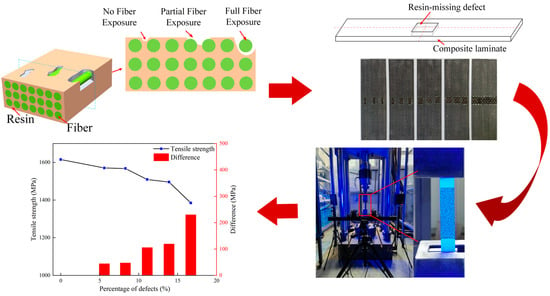
Effect of Resin-Missing Defects on Tensile Behavior of Carbon Fiber/Epoxy Composites
Abstract
1. Introduction
2. Experiment
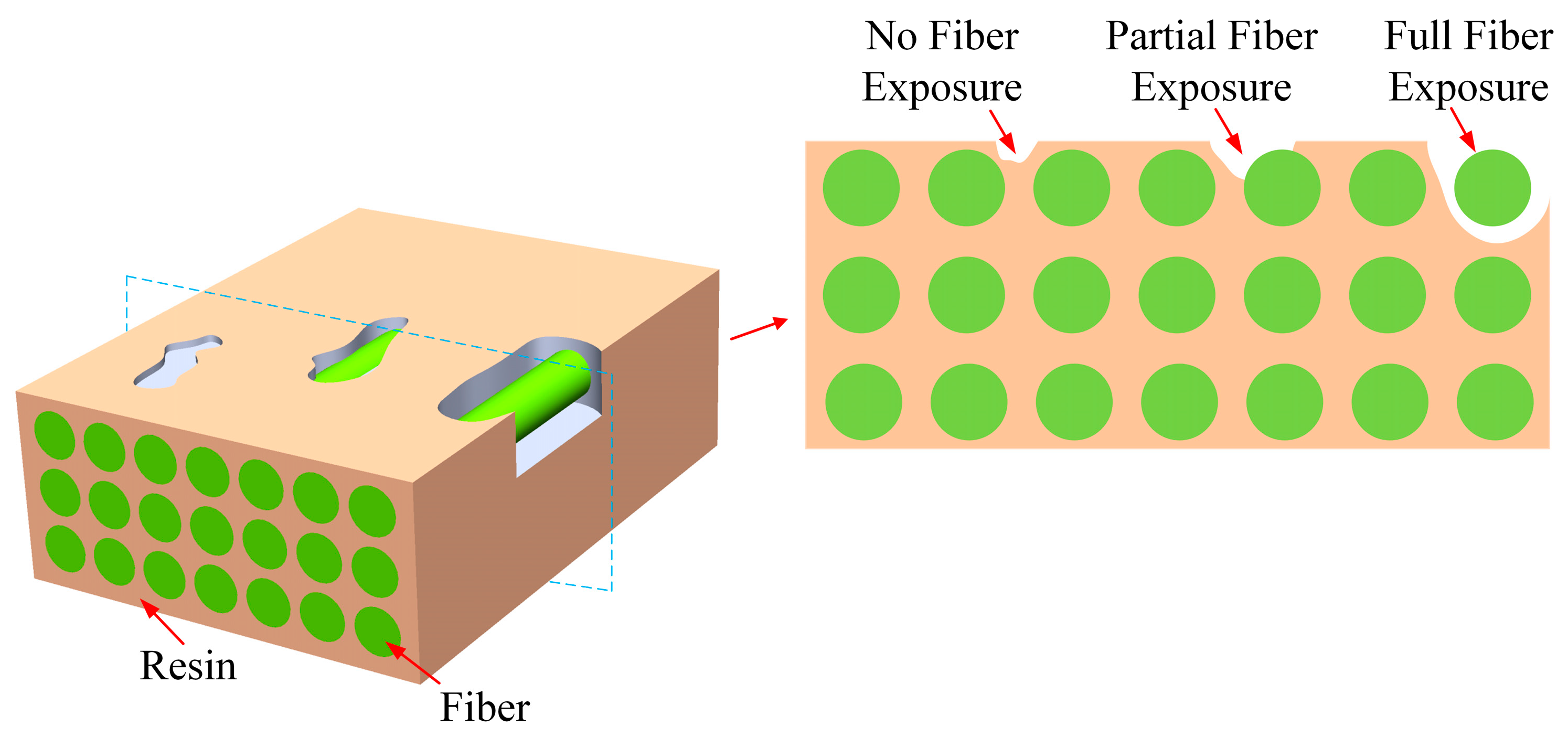
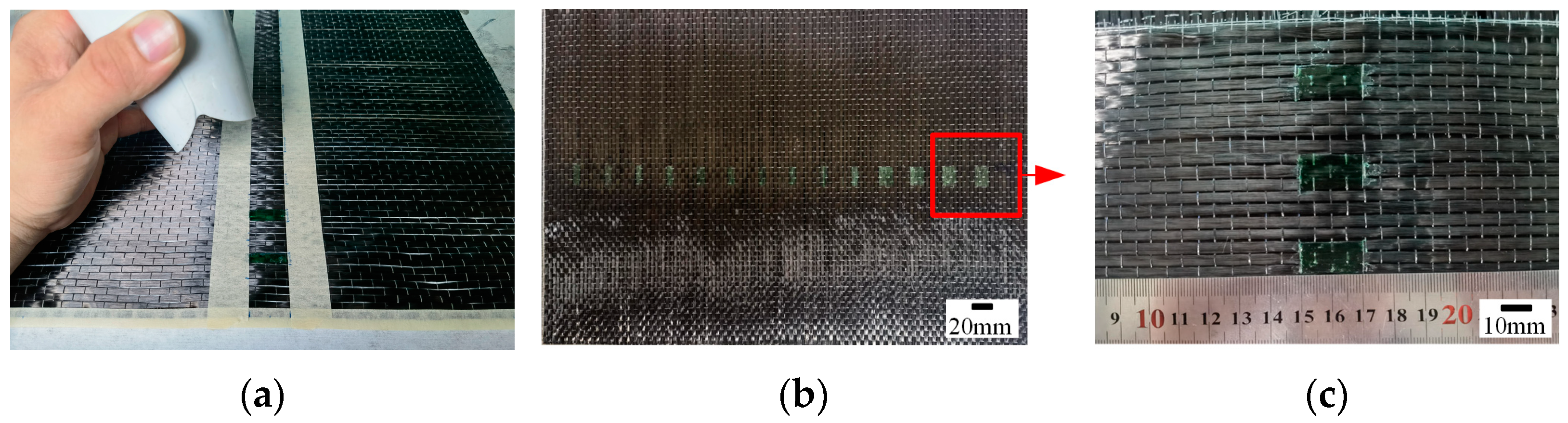
2.1. Specimen Preparation
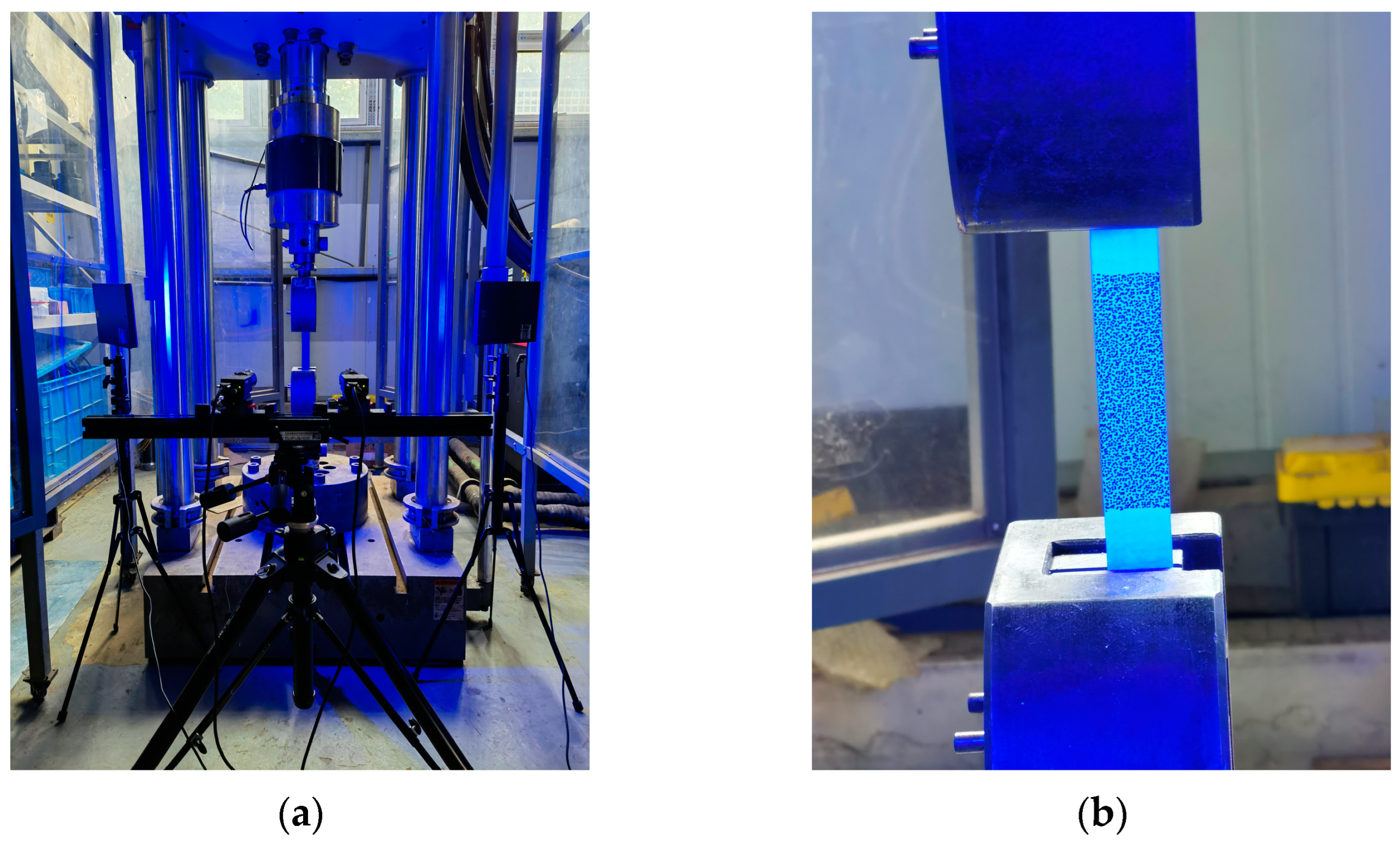
2.2. Experimental Method
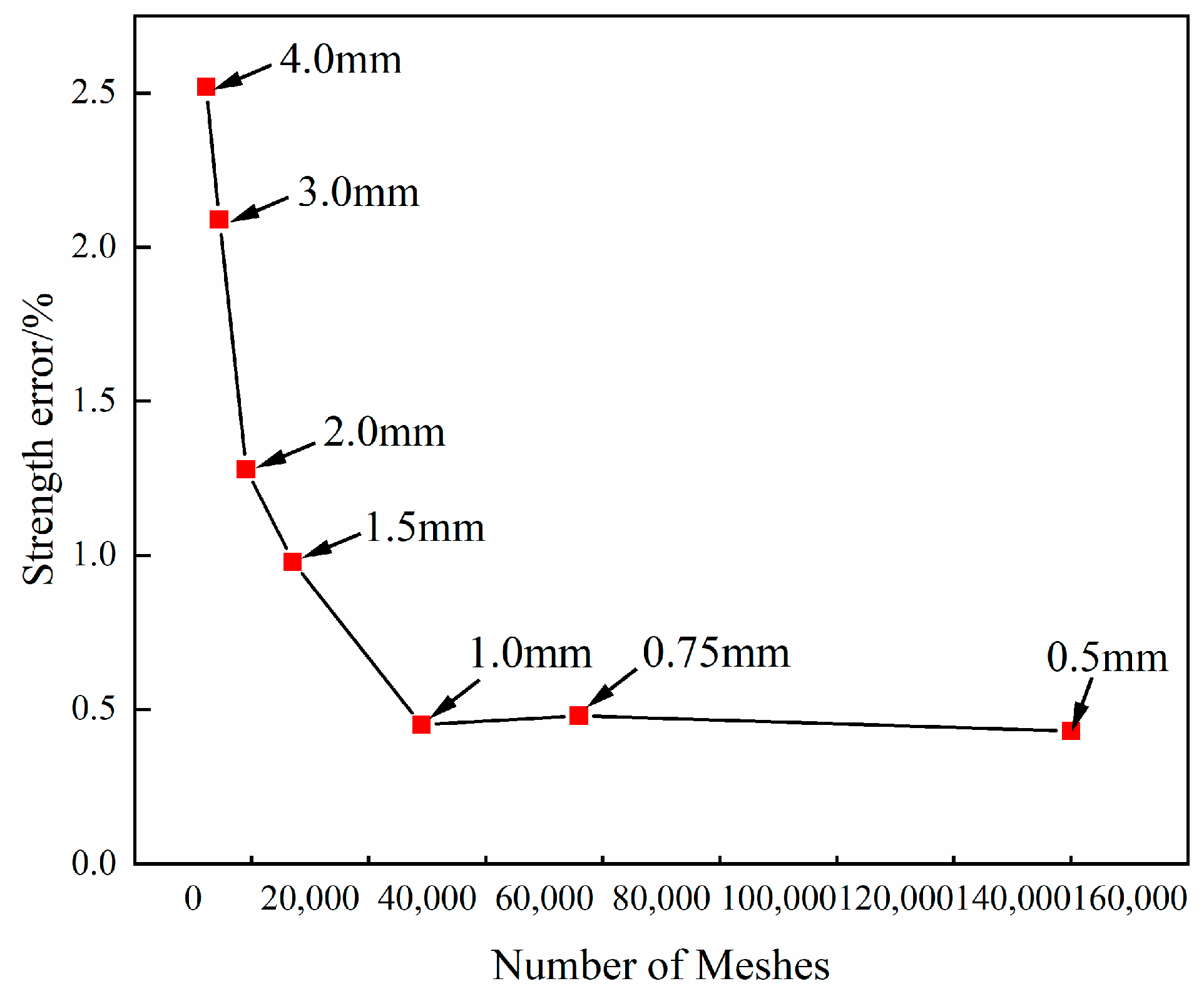
3. Finite Element Analysis
4. Results and Discussion
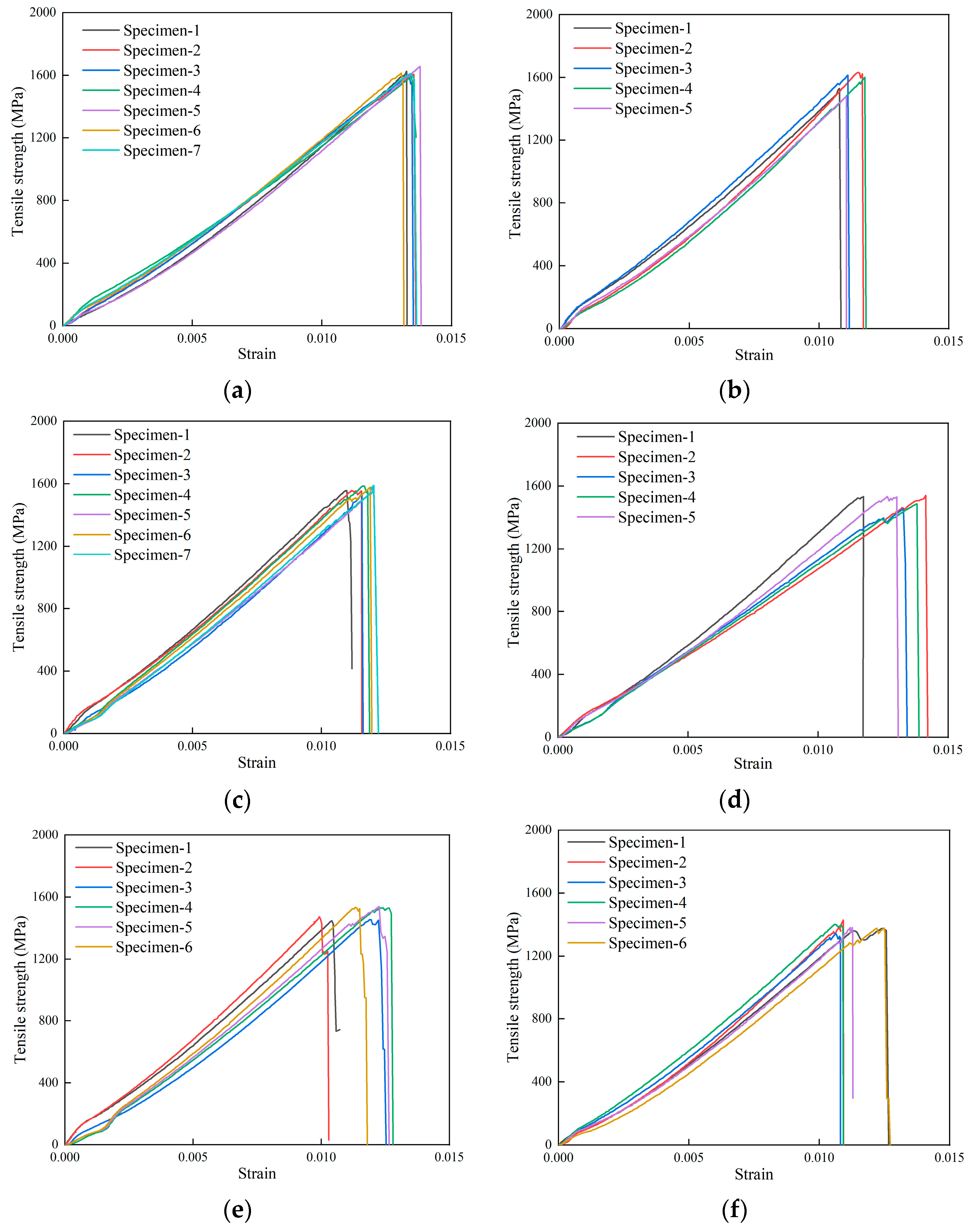
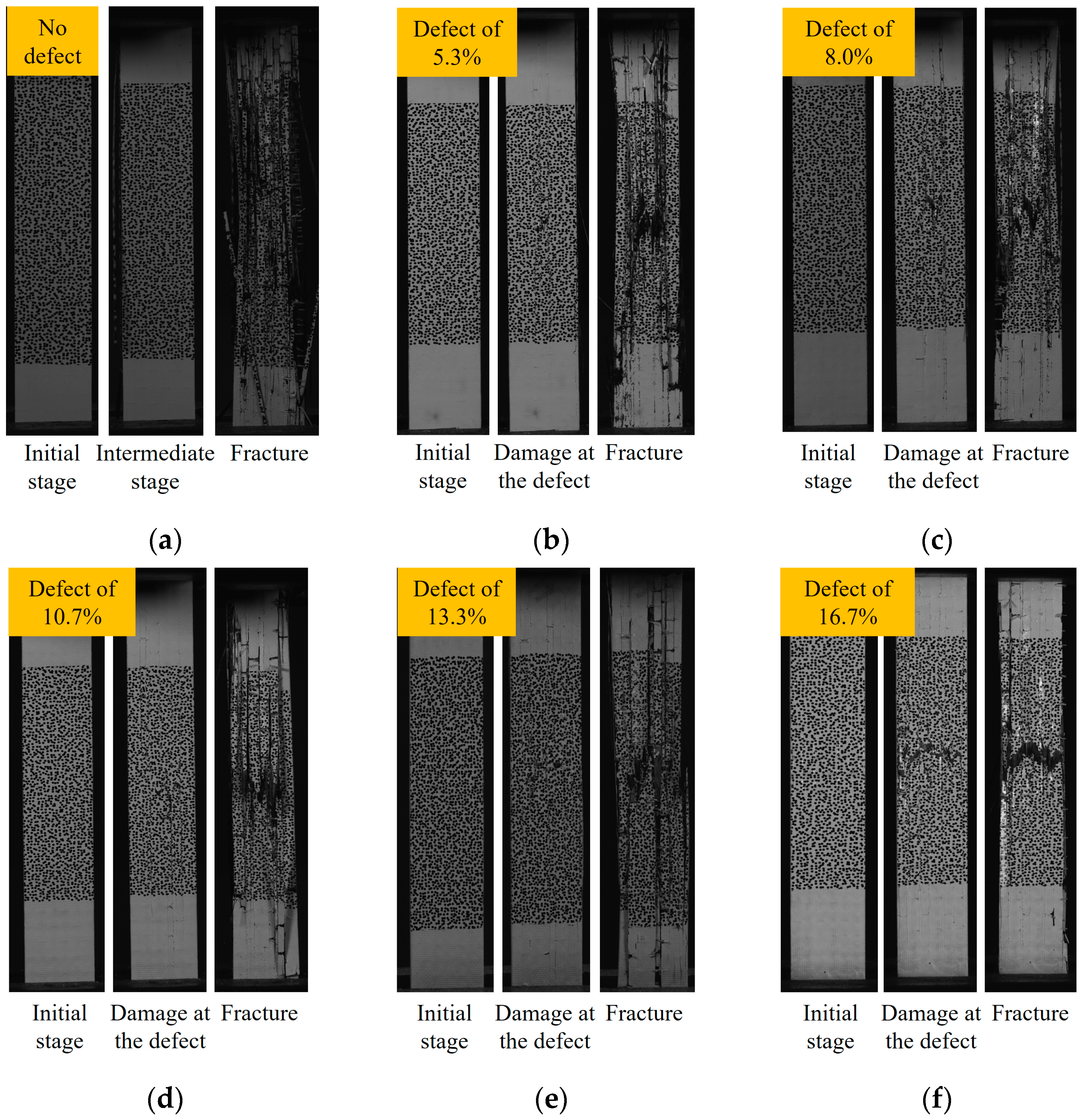
4.1. Experiment Results
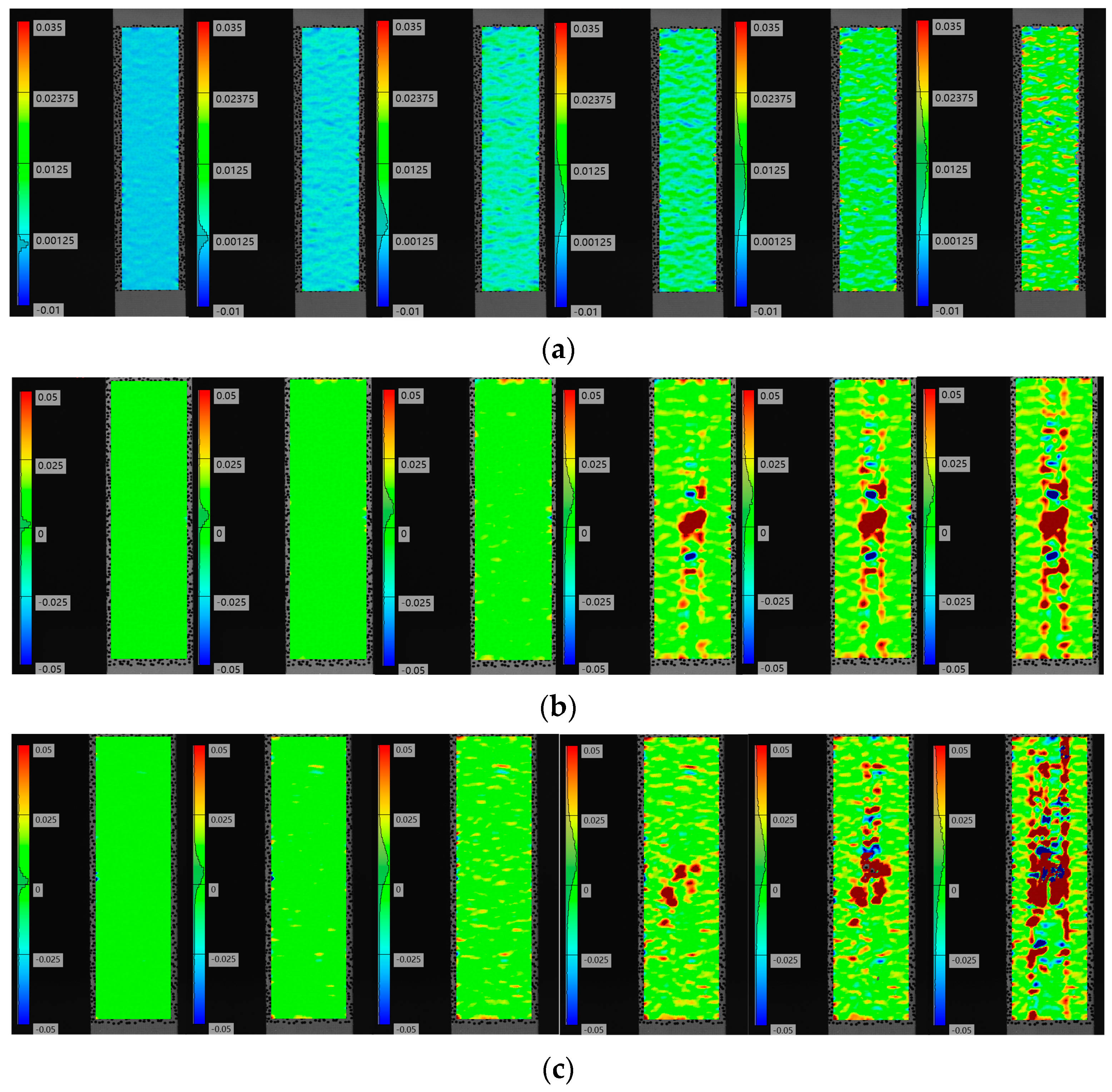
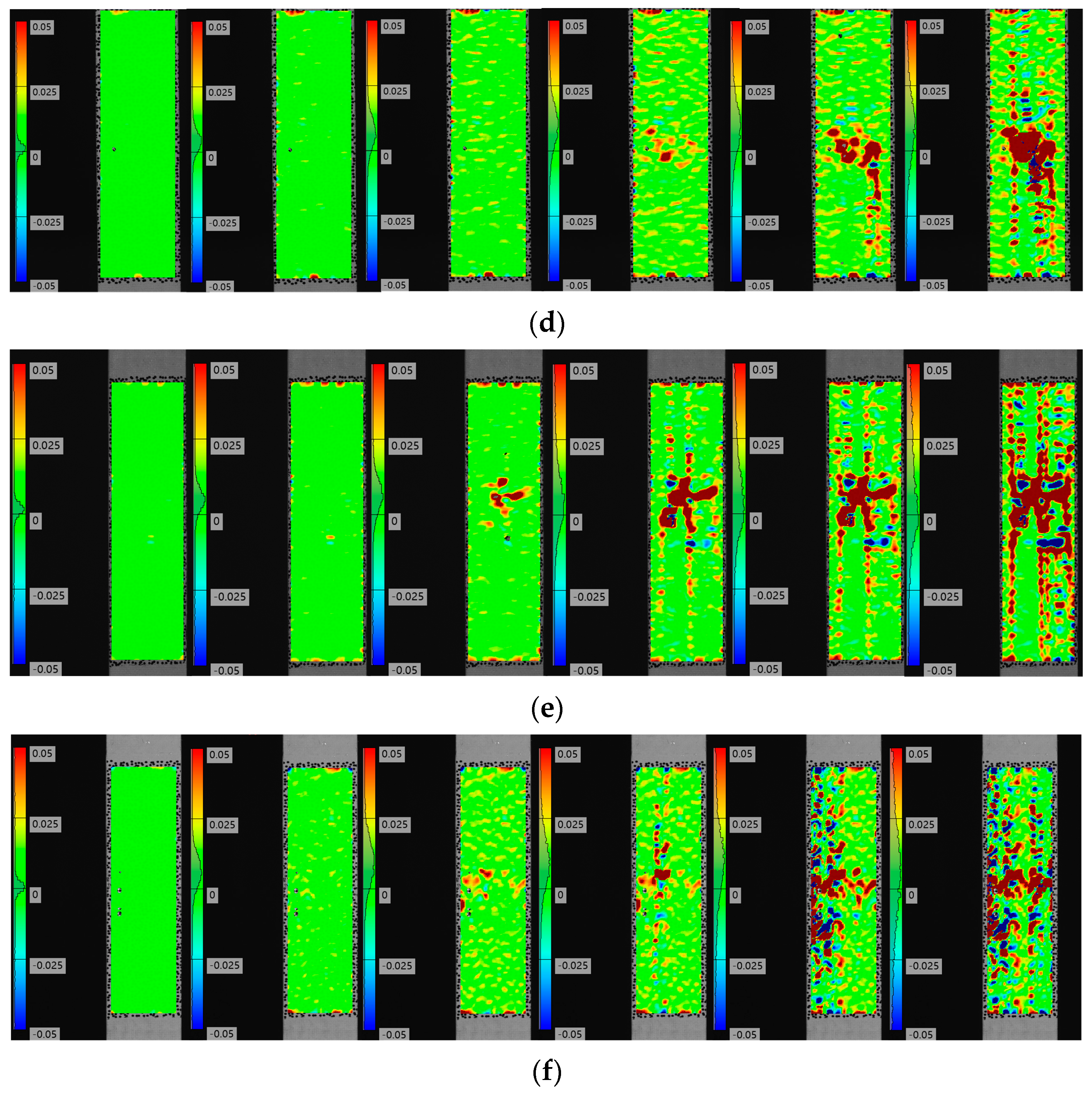
4.2. Strain Analysis Based on DIC
4.3. Numerical Results
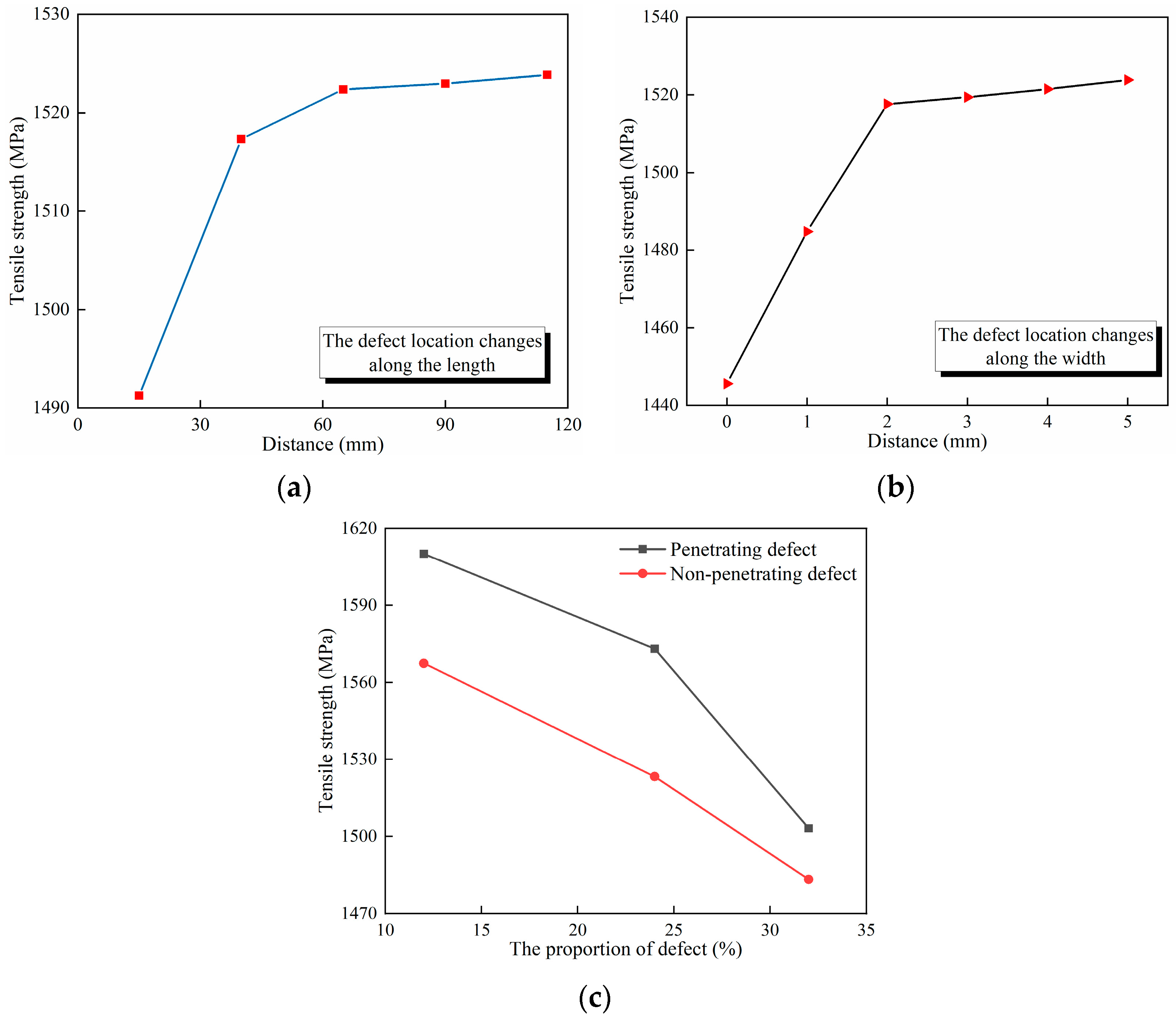
4.4. Effect of Defect Location Variation on Tensile Properties of Composite Laminates
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, H.; Tu, S.; Liu, Y.; Lu, X.; Zhu, X. Mechanical Properties of L-Joint with Composite Sandwich Structure. Compos. Struct. 2019, 217, 165–174. [Google Scholar] [CrossRef]
- Tretiak, I.; Kawashita, L.F.; Hallett, S.R. Predicting Short Beam Shear Strength Reduction in Carbon/Epoxy Laminates Containing Voids. Compos. Struct. 2022, 290, 115472. [Google Scholar] [CrossRef]
- Facciotto, S.; Simacek, P.; Advani, S.G.; Pickett, A.; Middendorf, P. Modeling Formation and Evolution of Voids in Unsaturated Dual Scale Preforms in Resin Transfer Molding Processes. Compos. Part A Appl. Sci. Manuf. 2023, 173, 107675. [Google Scholar] [CrossRef]
- Yalcinkaya, M.A.; Sozer, E.M.; Altan, M.C. Effect of External Pressure and Resin Flushing on Reduction of Process-Induced Voids and Enhancement of Laminate Quality in Heated-VARTM. Compos. Part A Appl. Sci. Manuf. 2019, 121, 353–364. [Google Scholar] [CrossRef]
- Dong, C. Effects of Process-Induced Voids on the Properties of Fibre Reinforced Composites. J. Mater. Sci. Technol. 2016, 32, 597–604. [Google Scholar] [CrossRef]
- Maragoni, L.; Carraro, P.A.; Peron, M.; Quaresimin, M. Fatigue Behaviour of Glass/Epoxy Laminates in the Presence of Voids. Int. J. Fatigue 2017, 95, 18–28. [Google Scholar] [CrossRef]
- Chen, L.; Miao, L.; Xu, Q.; Yang, Q.; Zhu, W.; Ke, Y. Damage and Failure Mechanisms of CFRP Due to Manufacturing Induced Wrinkling Defects. Compos. Struct. 2023, 326, 117624. [Google Scholar] [CrossRef]
- Calvo, J.V.; Quiñonero-Moya, A.R.; Feito, N.; Miguélez, M.H.; Giner, E. Influence of Distributed Out-of-Plane Waviness Defects on the Mechanical Behavior of CFRP Laminates. Compos. Struct. 2023, 323, 117498. [Google Scholar] [CrossRef]
- Mendonça, H.G.; Mikkelsen, L.P.; Zhang, B.; Allegri, G.; Hallett, S.R. Fatigue Delaminations in Composites for Wind Turbine Blades with Artificial Wrinkle Defects. Int. J. Fatigue 2023, 175, 107822. [Google Scholar] [CrossRef]
- Zhang, Y.; Huang, K.; Sun, R.; Liao, F.; Guo, L.; Zhang, L. Effect of Embedded Delamination on the Compression Performance of Carbon Fiber Reinforced Composites. Compos. Struct. 2022, 281, 115063. [Google Scholar] [CrossRef]
- Samal, S. Interface Failure and Delamination Resistance of Fiber-Reinforced Geopolymer Composite by Simulation and Experimental Method. Cem. Concr. Compos. 2022, 128, 104420. [Google Scholar] [CrossRef]
- Li, Y.; Wang, B.; Zhou, L. Study on the Effect of Delamination Defects on the Mechanical Properties of CFRP Composites. Eng. Fail. Anal. 2023, 153, 107576. [Google Scholar] [CrossRef]
- Pang, X.; Chen, X.; He, Y.; Zhang, S.; Wang, Y.; Fan, Z.; Li, X. Effect of Resin-Rich Areas on Failure Behavior of Unidirectional CFRP under Transverse Pure Shear. Comput. Mater. Sci. 2023, 219, 112023. [Google Scholar] [CrossRef]
- Ghayoor, H.; Marsden, C.C.; Hoa, S.V.; Melro, A.R. Numerical Analysis of Resin-Rich Areas and Their Effects on Failure Initiation of Composites. Compos. Part A Appl. Sci. Manuf. 2019, 117, 125–133. [Google Scholar] [CrossRef]
- Rezasefat, M.; Mostafavi, Y.; Ma, D.; Manes, A. A Hybrid Micro-Macro Mechanical Damage Model to Consider the Influence of Resin-Rich Zones on the Transverse Tensile Behaviour of Unidirectional Composites. Compos. Struct. 2023, 308, 116714. [Google Scholar] [CrossRef]
- Mehdikhani, M.; Straumit, I.; Gorbatikh, L.; Lomov, S.V. Detailed Characterization of Voids in Multidirectional Carbon Fiber/Epoxy Composite Laminates Using X-ray Micro-Computed Tomography. Compos. Part A Appl. Sci. Manuf. 2019, 125, 105532. [Google Scholar] [CrossRef]
- Fu, Y.; Yao, X.; Gao, X. Micro-Mesoscopic Prediction of Void Defect in 3D Braided Composites. Compos. Part A Appl. Sci. Manuf. 2021, 147, 106450. [Google Scholar] [CrossRef]
- Huang, H.S. Influence of Cracks and Voids on the Responses of Composites. Compos. Struct. 2021, 272, 114172. [Google Scholar] [CrossRef]
- Fu, Y.; Yao, X. A Review on Manufacturing Defects and Their Detection of Fiber Reinforced Resin Matrix Composites. Compos. Part C Open Access 2022, 8, 100276. [Google Scholar] [CrossRef]
- Staffan Lundström, T.; Frishfelds, V.; Jakovics, A. Bubble Formation and Motion in Non-Crimp Fabrics with Perturbed Bundle Geometry. Compos. Part A Appl. Sci. Manuf. 2010, 41, 83–92. [Google Scholar] [CrossRef]
- Lawrence, J.M.; Neacsu, V.; Advani, S.G. Modeling the Impact of Capillary Pressure and Air Entrapment on Fiber Tow Saturation during Resin Infusion in LCM. Compos. Part A Appl. Sci. Manuf. 2009, 40, 1053–1064. [Google Scholar] [CrossRef]
- Fisher, B.; Eaton, M.; Pullin, R. A Novel Multi-Scale Modelling Approach to Predict the Reduction of Transverse Strength Due to Porosity in Composite Materials. Compos. Struct. 2023, 312, 116861. [Google Scholar] [CrossRef]
- Turteltaub, S.; De Jong, G. Multiscale Modeling of the Effect of Sub-Ply Voids on the Failure of Composite Materials. Int. J. Solids Struct. 2019, 165, 63–74. [Google Scholar] [CrossRef]
- Dong, J.; Huo, N. A Two-Scale Method for Predicting the Mechanical Properties of 3D Braided Composites with Internal Defects. Compos. Struct. 2016, 152, 1–10. [Google Scholar] [CrossRef]
- Zhang, J.; Xie, J.; Zhao, X.; Chen, J.; Li, Z. Influence of Void Defects on Impact Properties of CFRP Laminates Based on Multi-Scale Simulation Method. Int. J. Impact Eng. 2023, 180, 104706. [Google Scholar] [CrossRef]
- Gao, X.; Yuan, L.; Fu, Y.; Yao, X.; Yang, H. Prediction of Mechanical Properties on 3D Braided Composites with Void Defects. Compos. Part B Eng. 2020, 197, 108164. [Google Scholar] [CrossRef]
- Ge, L.; Li, H.; Zheng, H.; Zhang, C.; Fang, D. Two-Scale Damage Failure Analysis of 3D Braided Composites Considering Pore Defects. Compos. Struct. 2021, 260, 113556. [Google Scholar] [CrossRef]
- Huang, H.; Shan, Z.; Liu, J.; Sun, Z.; Guo, Z.; Gong, H.; Xia, H.; Jin, P.; Chen, C. A Unified Trans-Scale Mechanical Properties Prediction Method of 3D Composites with Void Defects. Compos. Struct. 2023, 306, 116574. [Google Scholar] [CrossRef]
- Ge, L.; Li, H.; Zhong, J.; Zhang, C.; Fang, D. Micro-CT Based Trans-Scale Damage Analysis of 3D Braided Composites with Pore Defects. Compos. Sci. Technol. 2021, 211, 108830. [Google Scholar] [CrossRef]
- Wang, P.; Lei, H.; Zhu, X.; Chen, H.; Wang, C.; Fang, D. Effect of Manufacturing Defect on Mechanical Performance of Plain Weave Carbon/Epoxy Composite Based on 3D Geometrical Reconstruction. Compos. Struct. 2018, 199, 38–52. [Google Scholar] [CrossRef]
- Shigang, A.; Daining, F.; Rujie, H.; Yongmao, P. Effect of Manufacturing Defects on Mechanical Properties and Failure Features of 3D Orthogonal Woven C/C Composites. Compos. Part B Eng. 2015, 71, 113–121. [Google Scholar] [CrossRef]
- Guo, J.; Ke, Y.; Wu, Y.; Gu, B.; Sun, B. Effects of Defect Sizes at Different Locations on Compressive Behaviors of 3D Braided Composites. Thin-Walled Struct. 2022, 179, 109563. [Google Scholar] [CrossRef]
- Tang, Z.; Guo, L.; Li, Z.; Huang, K.; Zheng, T.; Sun, R. A Comparative Study of Void Characteristics on the Mechanical Response of Unidirectional Composites. Mech. Mater. 2022, 174, 104456. [Google Scholar] [CrossRef]
- ASTM D3039/DM. 3039; Standard Test Method for Tensile Properties of Polymer Matrix Composite Materials. ASTM: West Conshohocken, PA, USA, 2008. [CrossRef]
- Hashin, Z. Fatigue Failure Criteria for Unidirectional Fiber Composites. J. Appl. Mech. 1981, 48, 846–852. [Google Scholar] [CrossRef]
- Camanho, P.P.; Matthews, F.L. A Progressive Damage Model for Mechanically Fastened Joints in Composite Laminates. J. Compos. Mater. 1999, 33, 2248–2280. [Google Scholar] [CrossRef]
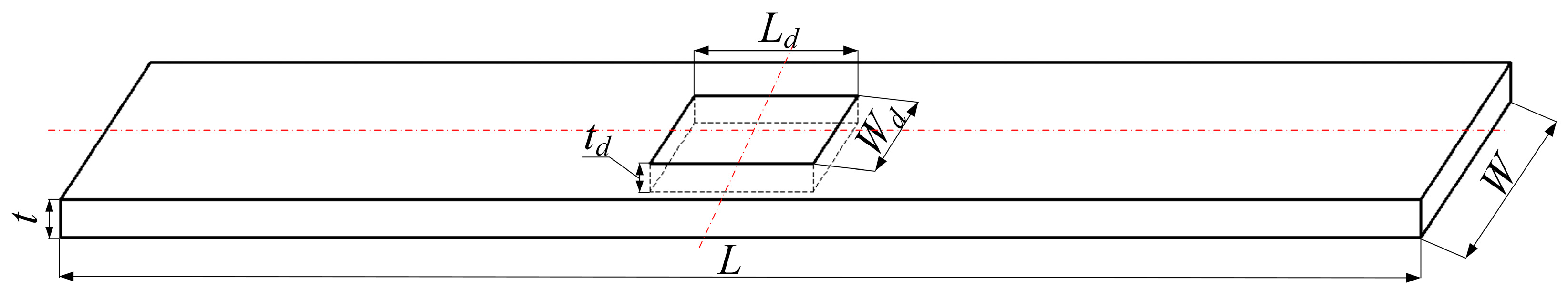
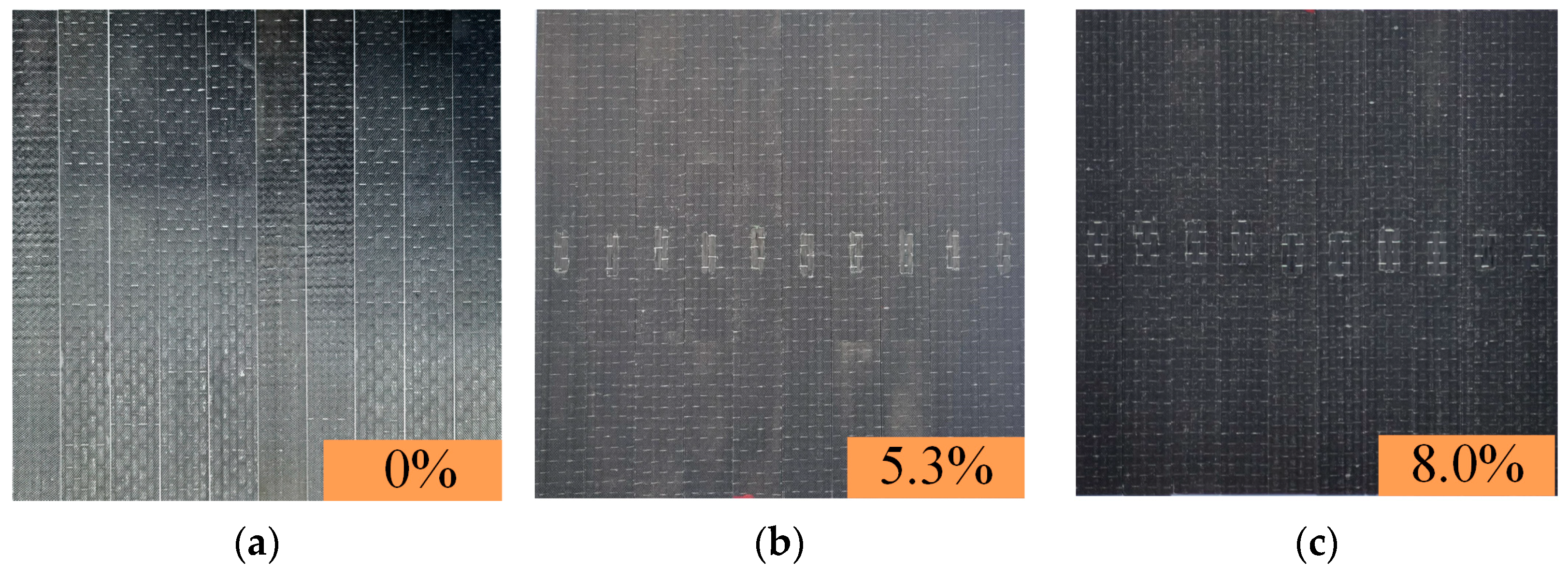
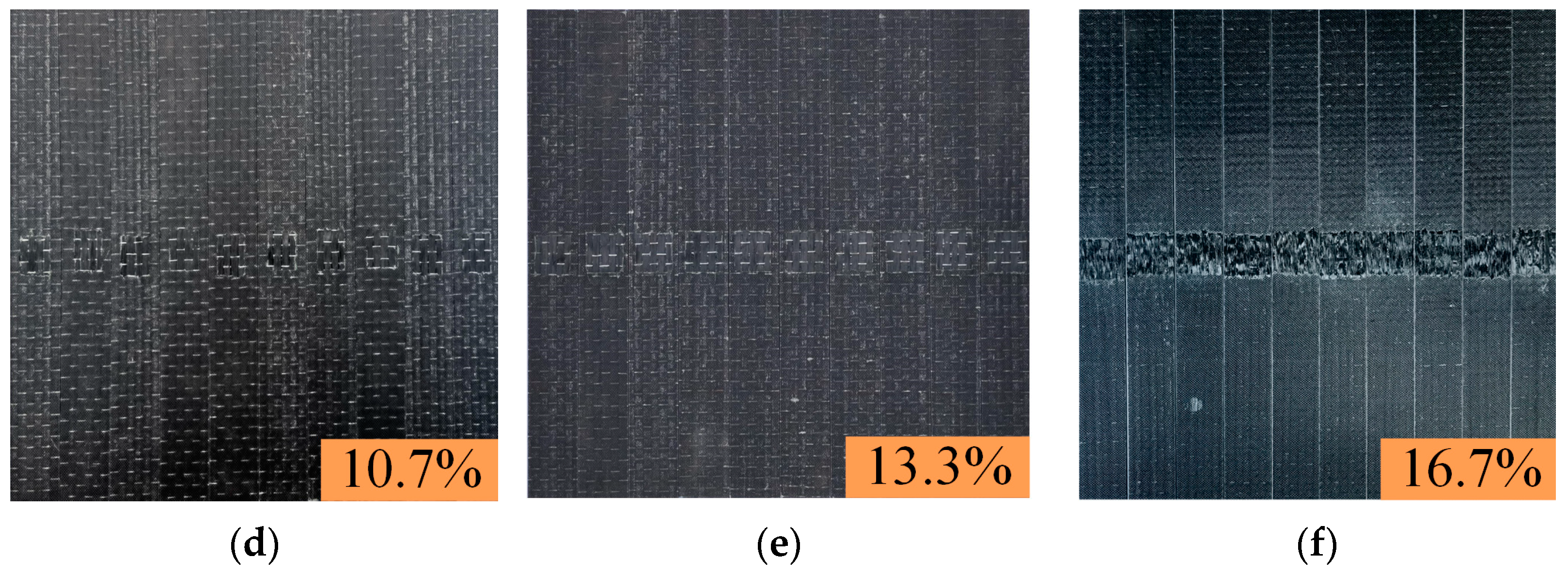



| L (mm) | W (mm) | t (mm) | Ld (mm) | Wd (mm) | td (mm) | Percentage of Defect Area (%) |
|---|---|---|---|---|---|---|
| 250 | 25 | 1.35 | 20 | 8 | 0.225 | 5.3 |
| 12 | 8.0 | |||||
| 16 | 10.7 | |||||
| 20 | 13.3 | |||||
| 25 | 16.7 |
| Elastic Modulus (GPa) | Poisson’s Ratio | Shear Modulus (GPa) | |||
|---|---|---|---|---|---|
| E11 | E22 = E33 | μ12 = μ13 | μ23 | G12 = G13 | G23 |
| 125 | 8.193 | 0.3 | 0.4 | 3.307 | 3.151 |
| Tensile strength (MPa) | Comprehensive strength (MPa) | Shear strength (MPa) | |||
| XT | YT = ZT | XC | YC = ZC | S12 = S13 | S23 |
| 1630 | 25 | 592 | 98 | 53 | 38 |
| Elastic Modulus (GPa) | Poisson’s Ratio | Tensile Strength (MPa) | |||
|---|---|---|---|---|---|
| E11 | E22 = E33 | μ12 = μ13 | μ23 | XT | YT = ZT |
| 95.88 | 1 × 10−5 | 0.3 | 0.2 | 1367 | 1 × 10−5 |
| No Defect | Defect −5.3% | Defect −8.0% | Defect −10.7% | Defect −13.3% | Defect −16.7% | |
|---|---|---|---|---|---|---|
| Tensile strength (MPa) | 1615.01 | 1570.46 | 1567.56 | 1509.19 | 1495.71 | 1384.72 |
| Reducing ratio (%) | 2.76 | 2.94 | 6.55 | 7.39 | 14.26 |
| No Defect | Percentage of Defect Area (%) | |||||
|---|---|---|---|---|---|---|
| 5.3 | 8.0 | 10.7 | 13.3 | 16.7 | ||
| Experiment (MPa) | 1615.01 | 1570.46 | 1567.56 | 1509.19 | 1495.71 | 1384.72 |
| FEM (MPa) | 1612.58 | 1563.34 | 1539.09 | 1522.7 | 1511.94 | 1427.11 |
| Error (%) | 0.15 | 0.45 | 1.82 | 0.90 | 1.09 | 3.06 |
| Width (mm) | Thickness (mm) | Defect Area (mm2) | The Proportion of Defect (%) | Distance (mm) | |
|---|---|---|---|---|---|
| The defect location changes along the length | 15 | 0.225 | 3.375 | 10 | 15 |
| 40 | |||||
| 65 | |||||
| 90 | |||||
| 115 | |||||
| The defect location changes along the width | 15 | 0.225 | 3.375 | 10 | 0 |
| 1 | |||||
| 2 | |||||
| 3 | |||||
| 4 | |||||
| 5 | |||||
| The penetrating and non-penetrating defects | 3 | 1.35 | 4.05 | 12 | P |
| 9 | 0.45 | NP | |||
| 6 | 1.35 | 8.1 | 24 | P | |
| 18 | 0.45 | NP | |||
| 8 | 1.35 | 10.8 | 32 | P | |
| 24 | 0.45 | NP |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, H.; Li, F.; Zhu, L. Effect of Resin-Missing Defects on Tensile Behavior of Carbon Fiber/Epoxy Composites. Polymers 2024, 16, 348. https://doi.org/10.3390/polym16030348
Li H, Li F, Zhu L. Effect of Resin-Missing Defects on Tensile Behavior of Carbon Fiber/Epoxy Composites. Polymers. 2024; 16(3):348. https://doi.org/10.3390/polym16030348
Chicago/Turabian StyleLi, Hongfeng, Feng Li, and Lingxue Zhu. 2024. "Effect of Resin-Missing Defects on Tensile Behavior of Carbon Fiber/Epoxy Composites" Polymers 16, no. 3: 348. https://doi.org/10.3390/polym16030348
APA StyleLi, H., Li, F., & Zhu, L. (2024). Effect of Resin-Missing Defects on Tensile Behavior of Carbon Fiber/Epoxy Composites. Polymers, 16(3), 348. https://doi.org/10.3390/polym16030348