The Effect of Notch and Molecular Weight on the Impact Fracture Behavior of Polycarbonate
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Methods
2.2.1. Sample Preparation
2.2.2. Orthogonal Experimental Design
2.2.3. Notch Processing
2.2.4. Charpy Impact Test
2.2.5. Fracture Morphology Diagram
2.2.6. Residual Stress Testing
3. Results and Discussion
3.1. Orthogonal Experiment of Charpy Impact
3.2. Single-Factor Test of Charpy Impact
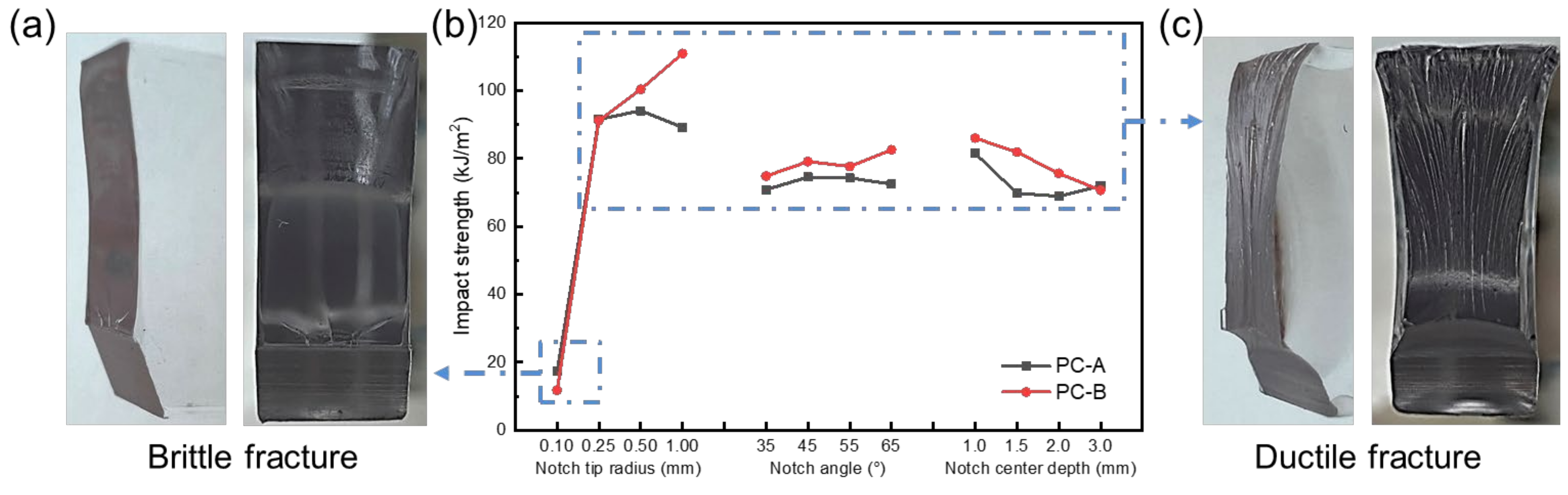
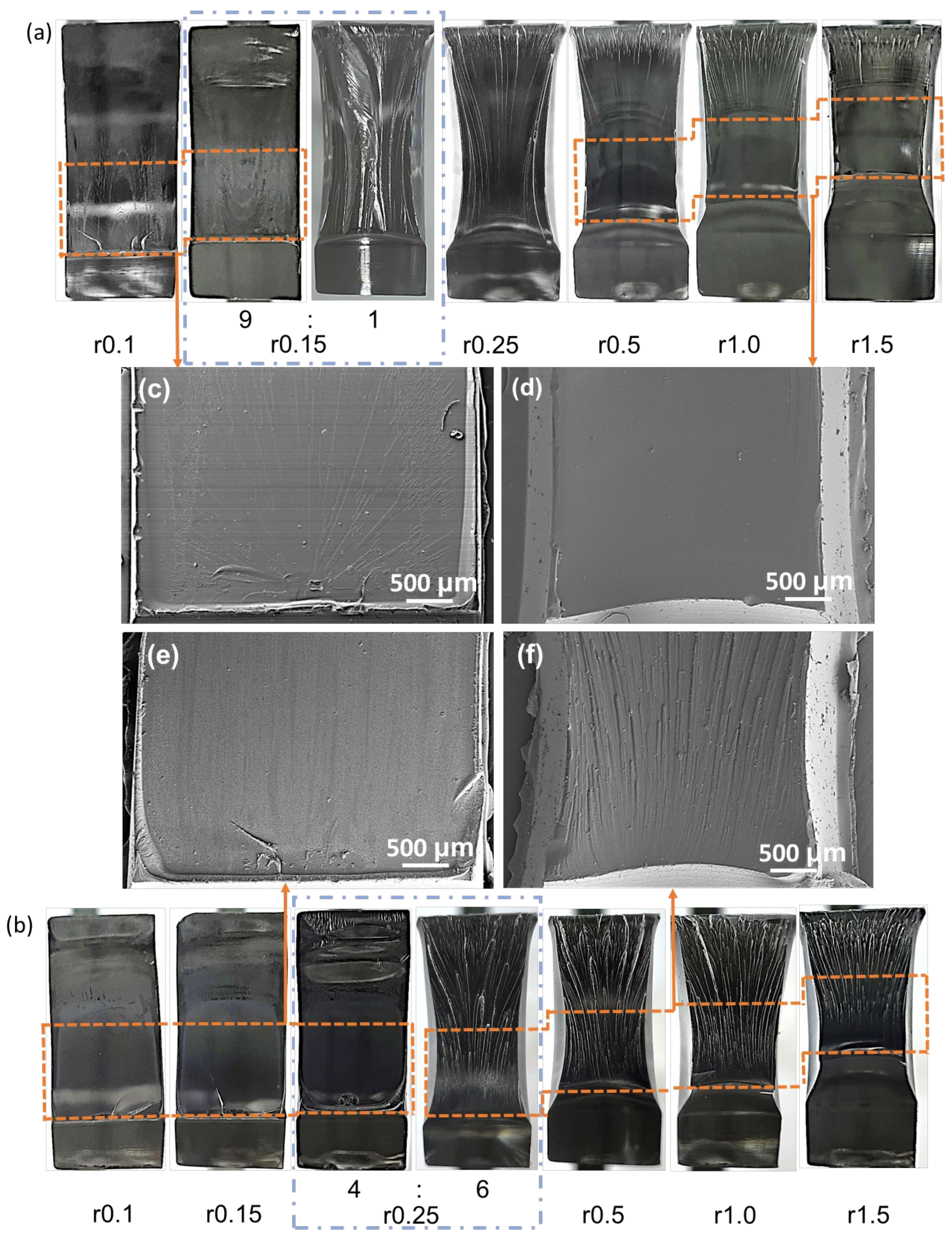
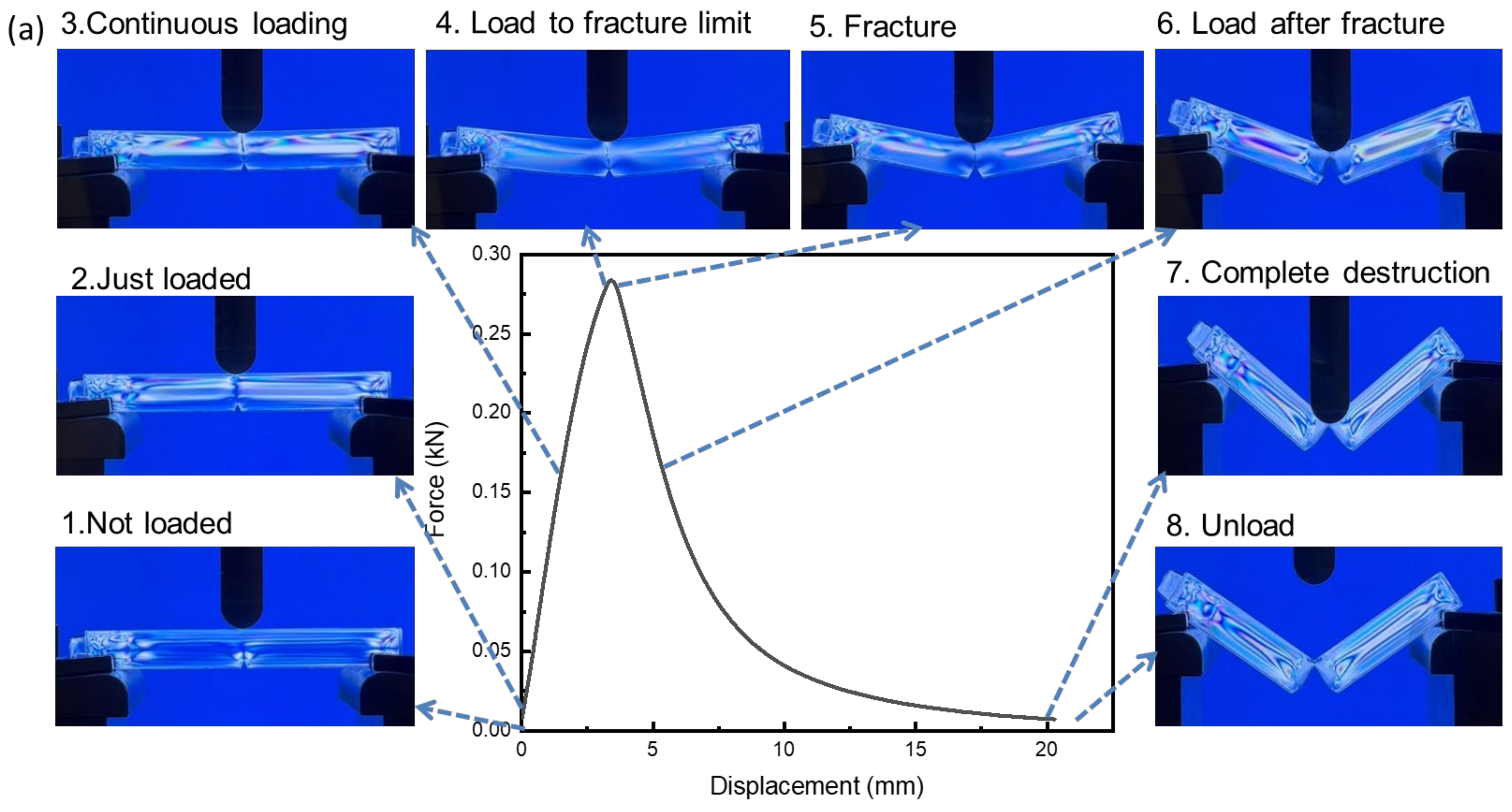
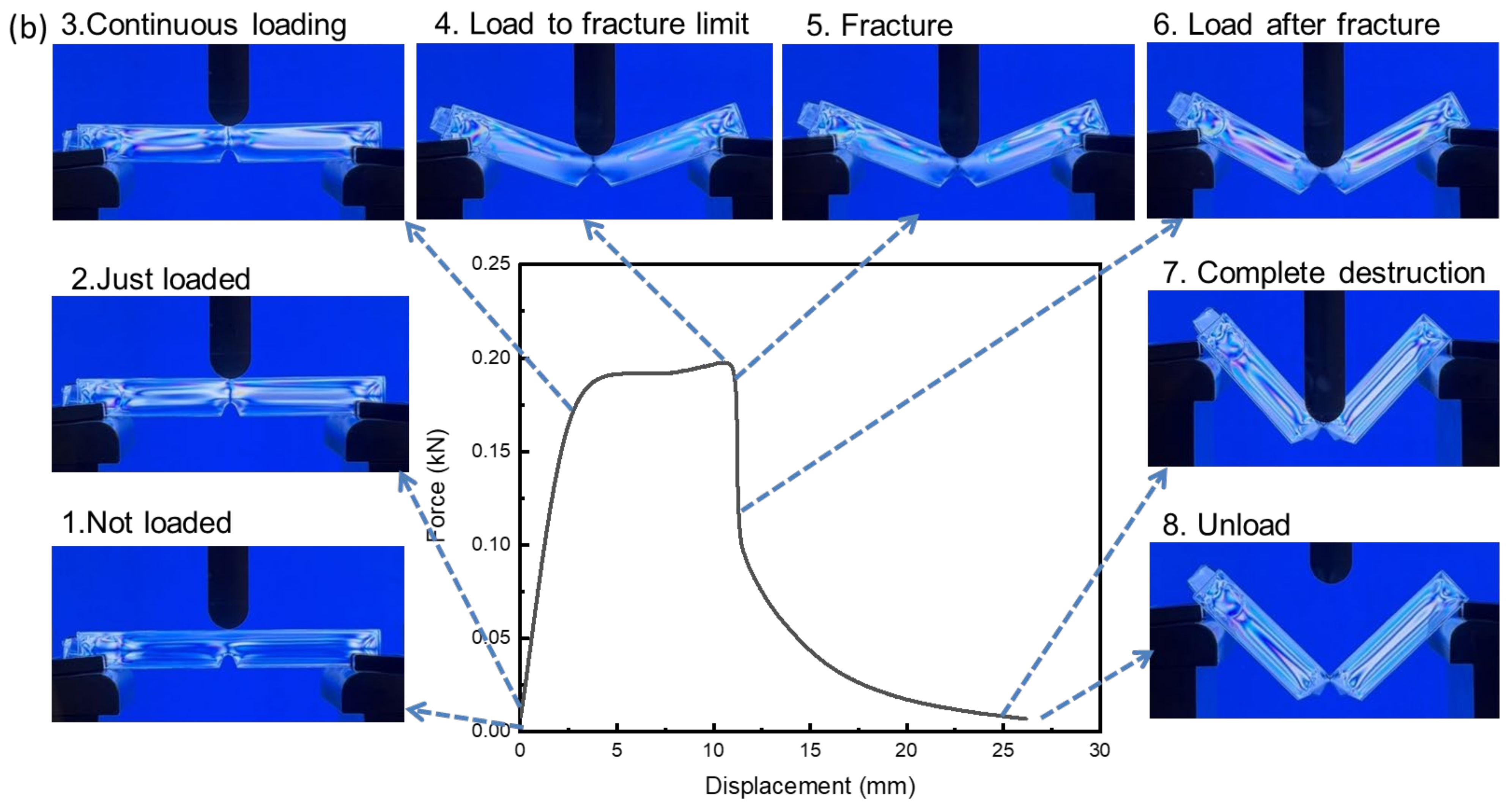
3.2.1. Charpy Impact Test Results and Cross-Sectional Morphology Analysis
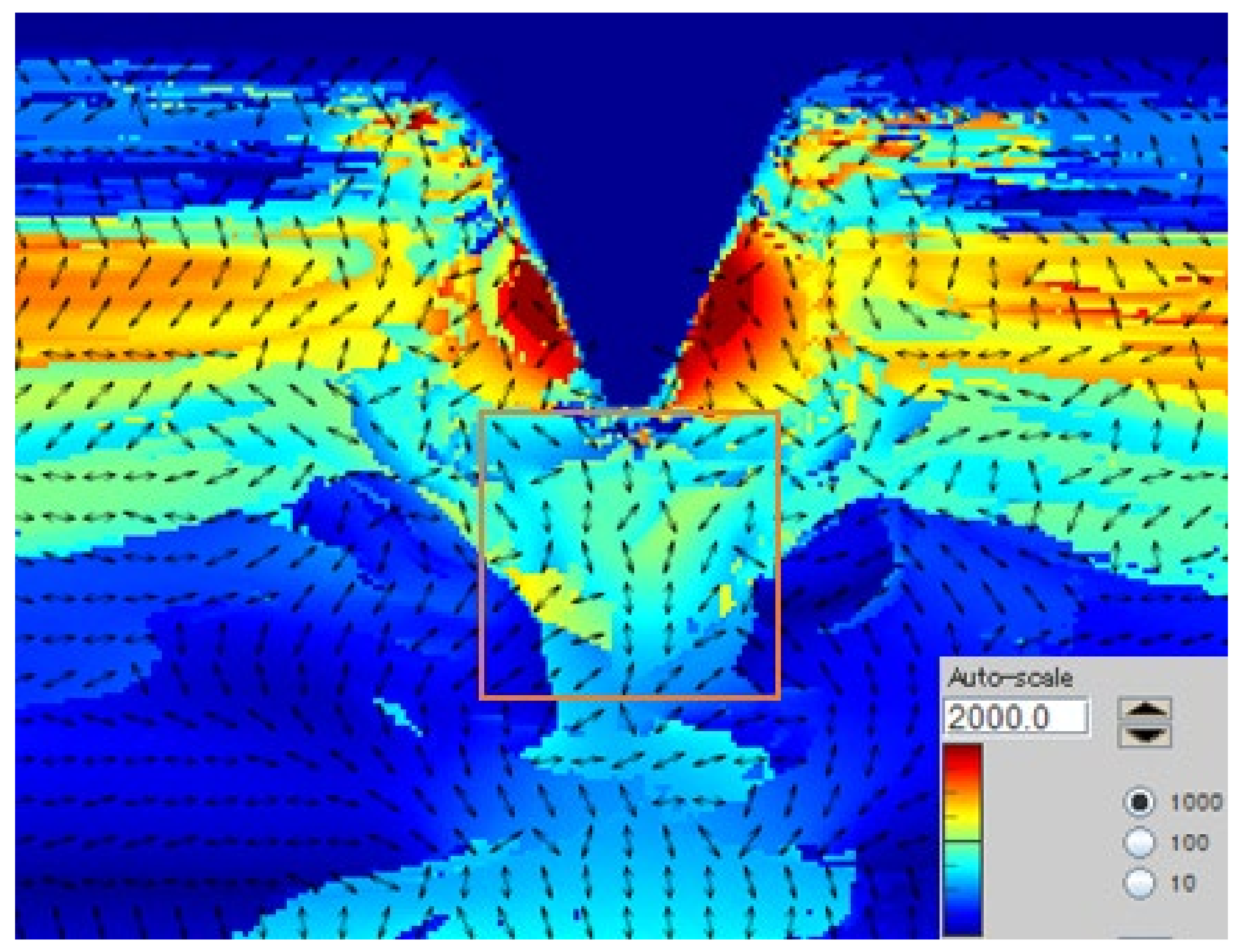
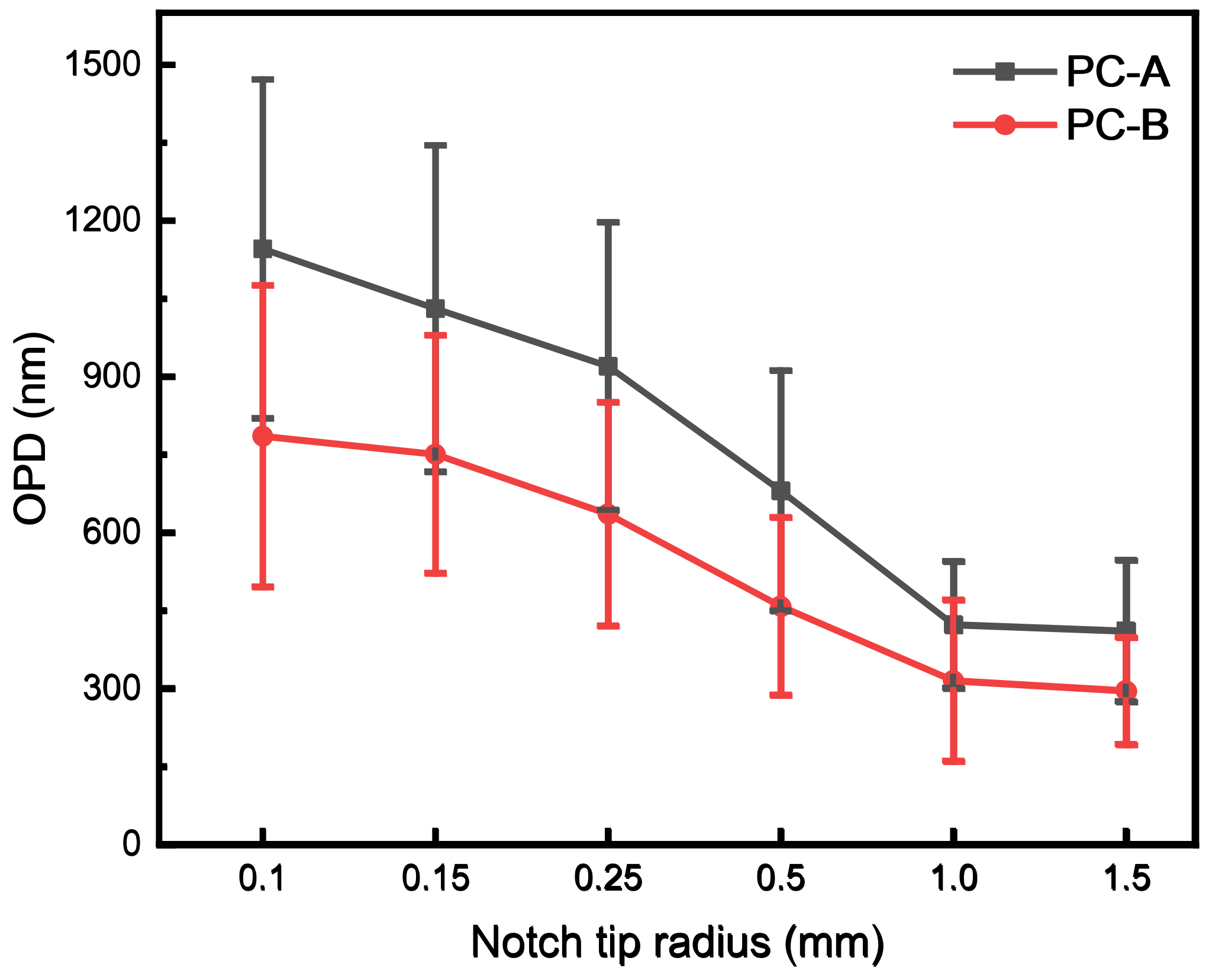
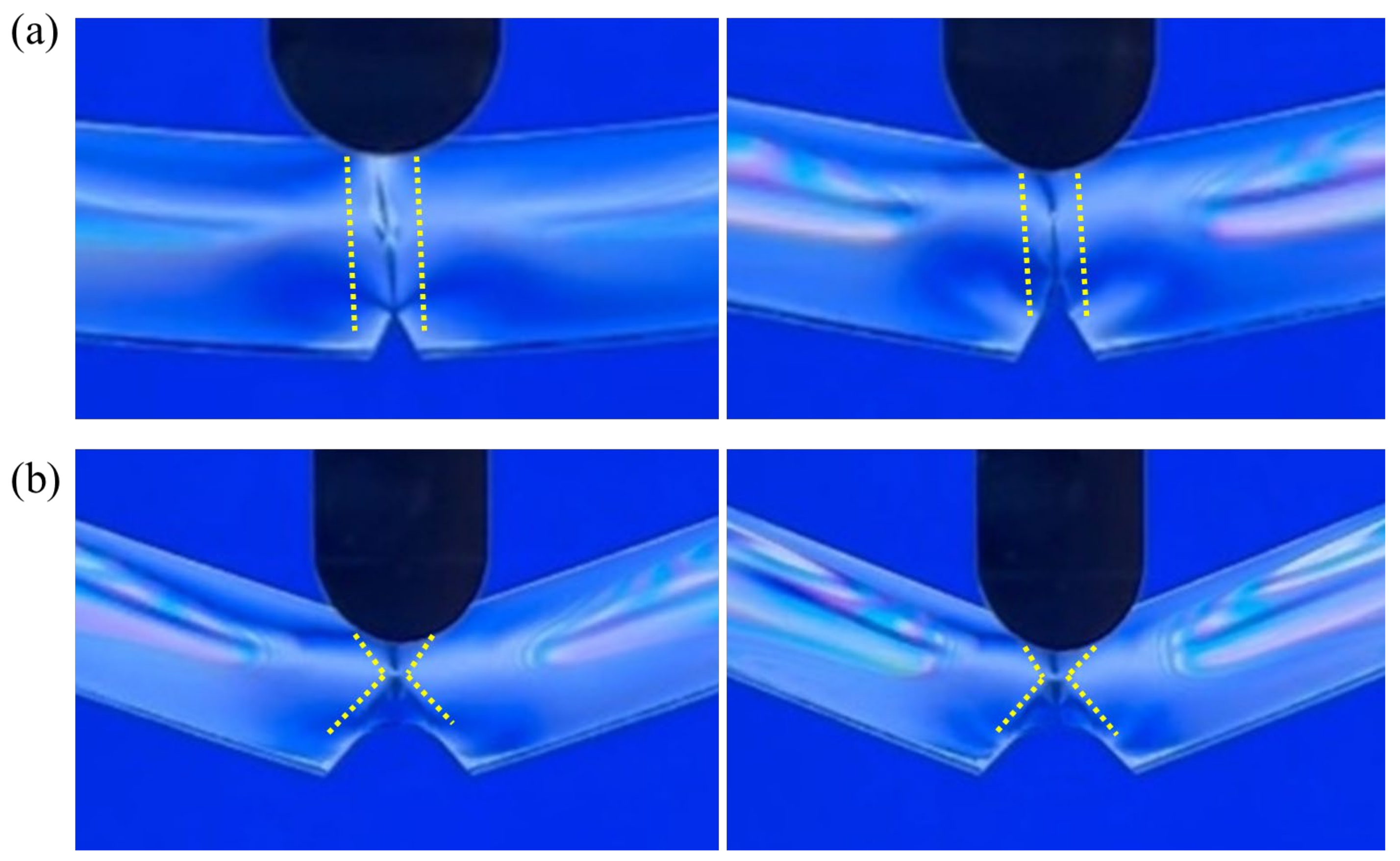
3.2.2. Stress Analysis
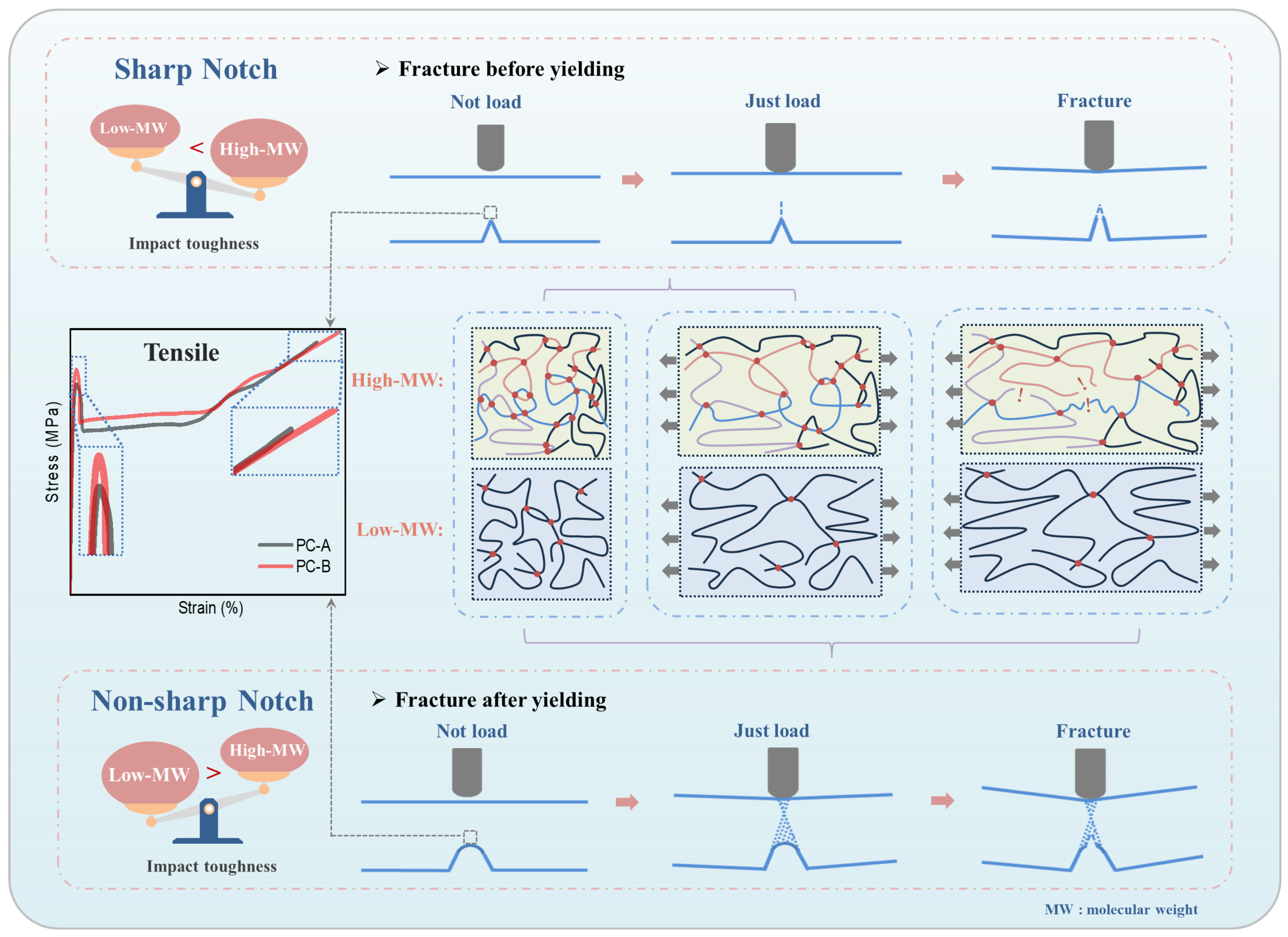
3.2.3. Analysis of Notch Impact Fracture Mechanism
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Sarıkaya, M.; Güden, M.; Kambur, Ç.; Özbek, S.Ç.; Taşdemirci, A. Development of the Johnson-Cook flow stress and damage parameters for the impact response of polycarbonate: Experimental and numerical approach. Int. J. Impact Eng. 2023, 179, 104674. [Google Scholar] [CrossRef]
- Subramaniam, A.; V, B.; B, C.; S, K.; Bhowmik, S. Novel ultra high impact strength light weight transparent polycarbonate laminated composite for aviation and defence. J. Polym. Res. 2023, 30, 413. [Google Scholar] [CrossRef]
- Ullrich, F.; Singh, S.P.V.; McDonald, S.; Krueger, A.; Vera-Sorroche, J.; Amirkhizi, A.; Masato, D. Effect of strain rate on the mechanical properties of polycarbonate processed by compression and injection molding. Polym. Eng. Sci. 2021, 62, 174–184. [Google Scholar] [CrossRef]
- Xu, Y.; Lu, H.; Gao, T.; Zhang, W. Processing-Induced Inhomogeneity of Yield Stress in Polycarbonate Product and Its Influence on the Impact Behavior. Polymers 2016, 8, 72. [Google Scholar] [CrossRef] [PubMed]
- Taguchi, T.; Miike, R.; Hatakeyama, T.; Saito, H. Ductile-to-brittle transition behavior of low molecular weight polycarbonate under carbon dioxide. Polym. Eng. Sci. 2018, 58, 683–690. [Google Scholar] [CrossRef]
- Dar, U.A.; Xu, Y.J.; Zakir, S.M.; Saeed, M.-U. The effect of injection molding process parameters on mechanical and fracture behavior of polycarbonate polymer. J. Appl. Polym. Sci. 2017, 134, 44474. [Google Scholar] [CrossRef]
- Sadeghi Esfahlani, S. Ballistic performance of Polycarbonate and Polymethyl methacrylate under normal and inclined dynamic impacts. Heliyon 2021, 7, e06856. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Li, C.; Zhang, X.; Xia, L.; Zhang, X.; Wu, H.; Guo, S. Polycarbonate toughening with reduced graphene oxide: Toward high toughness, strength and notch resistance. Chem. Eng. J. 2017, 325, 474–484. [Google Scholar] [CrossRef]
- Wang, F.; Yuan, H.; Li, W.; Du, Q.; Liu, C.; Zhang, L. Effects of Aging in an Aqueous Solution of NaCl on Impact Strength and Structure of Bisphenol A Polycarbonate. J. Macromol. Sci. Part B 2023, 62, 54–65. [Google Scholar] [CrossRef]
- Albinmousa, J.; AlSadah, J.; Hawwa, M.A.; Al-Qahtani, H.M. Estimation of Mode I Fracture of U-Notched Polycarbonate Specimens Using the Equivalent Material Concept and Strain Energy Density. Appl. Sci. 2021, 11, 3370. [Google Scholar] [CrossRef]
- Wu, X.; Wang, C.; Pek, J.X.; Huang, W.M. Brittle-to-ductile transition in high temperature pre-stretched polycarbonate. J. Polym. Res. 2022, 29, 435. [Google Scholar] [CrossRef]
- Desai, C.K.; Parameswaran, V.; Basu, S. Formulating a cohesive zone model for thin polycarbonate sheets using the concept of the essential work of fracture. Eng. Fract. Mech. 2018, 201, 144–156. [Google Scholar] [CrossRef]
- Leelaprachakul, T.; Kubo, A.; Umeno, Y. Coarse-grained molecular dynamics simulation on strain-hardening and fracture behaviors of polycarbonate: Effect of polydispersity and spatial distribution. J. Polym. Res. 2023, 30, 398. [Google Scholar] [CrossRef]
- Chan, E.P.; Xie, W.; Orski, S.V.; Lee, J.-H.; Soles, C.L. Entanglement Density-Dependent Energy Absorption of Polycarbonate Films via Supersonic Fracture. ACS Macro Lett. 2019, 8, 806–811. [Google Scholar] [CrossRef] [PubMed]
- Weber, R.P.; Monteiro, S.N.; Suarez, J.C.M.; Figueiredo, A.B.-H.S.; de Oliveira, C.J.V. Fracture toughness of gamma irradiated polycarbonate sheet using the essential work of fracture. Polym. Test. 2017, 57, 115–118. [Google Scholar] [CrossRef]
- Soma, H.; Nishitsuji, S.; Inoue, T. Molecular weight dependence in a relaxation phenomenon at glassy state: Physical aging of polycarbonate. Polymer 2012, 53, 895–896. [Google Scholar] [CrossRef]
- Kattekola, B.; Ranjan, A.; Basu, S. Three dimensional finite element investigations into the effects of thickness and notch radius on the fracture toughness of polycarbonate. Int. J. Fract. 2013, 181, 1–12. [Google Scholar] [CrossRef]
- Nishitsuji, S.; Watanabe, Y.; Ito, H.; Ishikawa, M.; Inoue, T.; Takenaka, M. Molecular weight dependence of the physical aging of polycarbonate. Polymer 2019, 178, 121571. [Google Scholar] [CrossRef]
- Umeno, Y.; Kubo, A.; Albina, J.-M. Coarse-grained molecular dynamics simulation of deformation and fracture in polycarbonate: Effect of molar mass and entanglement. Theor. Appl. Fract. Mech. 2020, 109, 102699. [Google Scholar] [CrossRef]
- Aranda-Ruiz, J.; Ravi-Chandar, K.; Loya, J.A. On the double transition in the failure mode of polycarbonate. Mech. Mater. 2020, 140, 103242. [Google Scholar] [CrossRef]
- Faye, A.; Parameswaran, V.; Basu, S. Effect of Notch-Tip Radius on Dynamic Brittle Fracture of Polycarbonate. Exp. Mech. 2016, 56, 1051–1061. [Google Scholar] [CrossRef]
- Cho, K.; Yang, J.; Kang, B.I.; Park, C.E. Notch Sensitivity of Polycarbonate and Toughened Polycarbonate. J. Appl. Polym. Sci. 2003, 89, 3115–3121. [Google Scholar] [CrossRef]
- Fraser, R.A.W.; Ward, I.M. The impact fracture behaviour of notched specimens of polycarbonate. J. Mater. Sci. 1977, 12, 459–468. [Google Scholar] [CrossRef]
- GB/T 1043.1-2008; Plastics - Determination of charpy impact properties. Standards Press of China: China, Beijing, 2008.
- Nisitani, H.; Hyakutake, H. Condition for Determining the Static Yield and Fracture of a Polycarbonate Plate Specimen with Notches. Eng. Fract. Mech. 1985, 22, 359–368. [Google Scholar] [CrossRef]
- Pitman, G.L.; Ward, I.M.; Duckett, R.A. The effects of thermal pre-treatment and molecular weight on the impact behaviour of polycarbonate. J. Mater. Sci. 1978, 13, 2092–2104. [Google Scholar] [CrossRef]
- Fujimoto, K.; Tang, Z.; Shinoda, W.; Okazaki, S. All-atom molecular dynamics study of impact fracture of glassy polymers. I: Molecular mechanism of brittleness of PMMA and ductility of PC. Polymer 2019, 178, 121570. [Google Scholar] [CrossRef]
- Kim, J.; Zhang, G.; Shi, M.; Suo, Z. Fracture, fatigue, and friction of polymers in which entanglements greatly outnumber cross-links. Science 2021, 374, 212–216. [Google Scholar] [CrossRef] [PubMed]




| PC | MFR (g/10 min) | Mn (g/mol) | Mw (g/mol) | Mw/Mn |
|---|---|---|---|---|
| PC-A | 3.3 | 30,010 | 51,940 | 1.730 |
| PC-B | 10 | 23,400 | 38,190 | 1.632 |
| Factor | A Notch Tip Radius (mm) | B Notch Angle (°) | C Notch Center Depth (mm) | |
|---|---|---|---|---|
| Level | ||||
| 1 | 0.10 | 35 | 1.0 | |
| 2 | 0.25 | 45 | 1.5 | |
| 3 | 0.50 | 55 | 2.0 | |
| 4 | 1.00 | 65 | 3.0 | |
| Test Number | Notch Tip Radius (mm) | Notch Angle (°) | Notch Center Depth (mm) | PC-A Impact Toughness (kJ/m2) | PC-B Impact Toughness (kJ/m2) |
|---|---|---|---|---|---|
| 1 | 0.10 | 35 | 1.0 | 13.8 | 12.1 |
| 2 | 0.10 | 45 | 1.5 | 13.8 | 11.6 |
| 3 | 0.10 | 55 | 2.0 | 10.5 | 11.4 |
| 4 | 0.10 | 65 | 3.0 | 31.1 | 12.0 |
| 5 | 0.25 | 35 | 1.5 | 93.6 | 91.8 |
| 6 | 0.25 | 45 | 1.0 | 105.7 | 105.9 |
| 7 | 0.25 | 55 | 3.0 | 80.7 | 79.7 |
| 8 | 0.25 | 65 | 2.0 | 86.4 | 87.5 |
| 9 | 0.50 | 35 | 2.0 | 91.0 | 96.8 |
| 10 | 0.50 | 45 | 3.0 | 90.9 | 92.1 |
| 11 | 0.50 | 55 | 1.0 | 114.4 | 104.2 |
| 12 | 0.50 | 65 | 1.5 | 80.2 | 108.6 |
| 13 | 1.00 | 35 | 3.0 | 85.1 | 98.8 |
| 14 | 1.00 | 45 | 2.0 | 87.8 | 107.1 |
| 15 | 1.00 | 55 | 1.5 | 91.7 | 115.7 |
| 16 | 1.00 | 65 | 1.0 | 92.3 | 122.3 |
| Polycarbonate | Parameter | Notch Tip Radius (mm) | Notch Angle (°) | Notch Center Depth (mm) |
|---|---|---|---|---|
| PC-A | K1 | 69.3 | 283.4 | 326.2 |
| K2 | 366.4 | 298.3 | 279.3 | |
| K3 | 376.5 | 297.4 | 275.7 | |
| K4 | 356.9 | 290.0 | 287.9 | |
| k1 | 17.3 | 70.9 | 81.6 | |
| k2 | 91.6 | 74.6 | 69.8 | |
| k3 | 94.1 | 74.3 | 68.9 | |
| k4 | 89.2 | 72.5 | 72.0 | |
| R | 76.8 | 3.7 | 12.6 | |
| PC-B | K1 | 47.0 | 299.5 | 344.3 |
| K2 | 364.8 | 316.6 | 327.7 | |
| K3 | 401.7 | 310.9 | 302.7 | |
| K4 | 443.9 | 330.3 | 282.6 | |
| k1 | 11.7 | 74.9 | 86.1 | |
| k2 | 91.2 | 79.2 | 81.9 | |
| k3 | 100.4 | 77.7 | 75.7 | |
| k4 | 111.0 | 82.6 | 70.7 | |
| R | 99.2 | 7.7 | 15.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, X.; Wang, T.; Sun, Q.; Wang, B.; Ge, Y.; Lang, J.; Yan, Y. The Effect of Notch and Molecular Weight on the Impact Fracture Behavior of Polycarbonate. Polymers 2024, 16, 1072. https://doi.org/10.3390/polym16081072
Xu X, Wang T, Sun Q, Wang B, Ge Y, Lang J, Yan Y. The Effect of Notch and Molecular Weight on the Impact Fracture Behavior of Polycarbonate. Polymers. 2024; 16(8):1072. https://doi.org/10.3390/polym16081072
Chicago/Turabian StyleXu, Xueting, Tao Wang, Qiwei Sun, Bolun Wang, Yong Ge, Jianlin Lang, and Yue Yan. 2024. "The Effect of Notch and Molecular Weight on the Impact Fracture Behavior of Polycarbonate" Polymers 16, no. 8: 1072. https://doi.org/10.3390/polym16081072
APA StyleXu, X., Wang, T., Sun, Q., Wang, B., Ge, Y., Lang, J., & Yan, Y. (2024). The Effect of Notch and Molecular Weight on the Impact Fracture Behavior of Polycarbonate. Polymers, 16(8), 1072. https://doi.org/10.3390/polym16081072





