Shear Transformation Zone and Its Correlation with Fracture Characteristics for Fe-Based Amorphous Ribbons in Different Structural States
Abstract
:1. Introduction
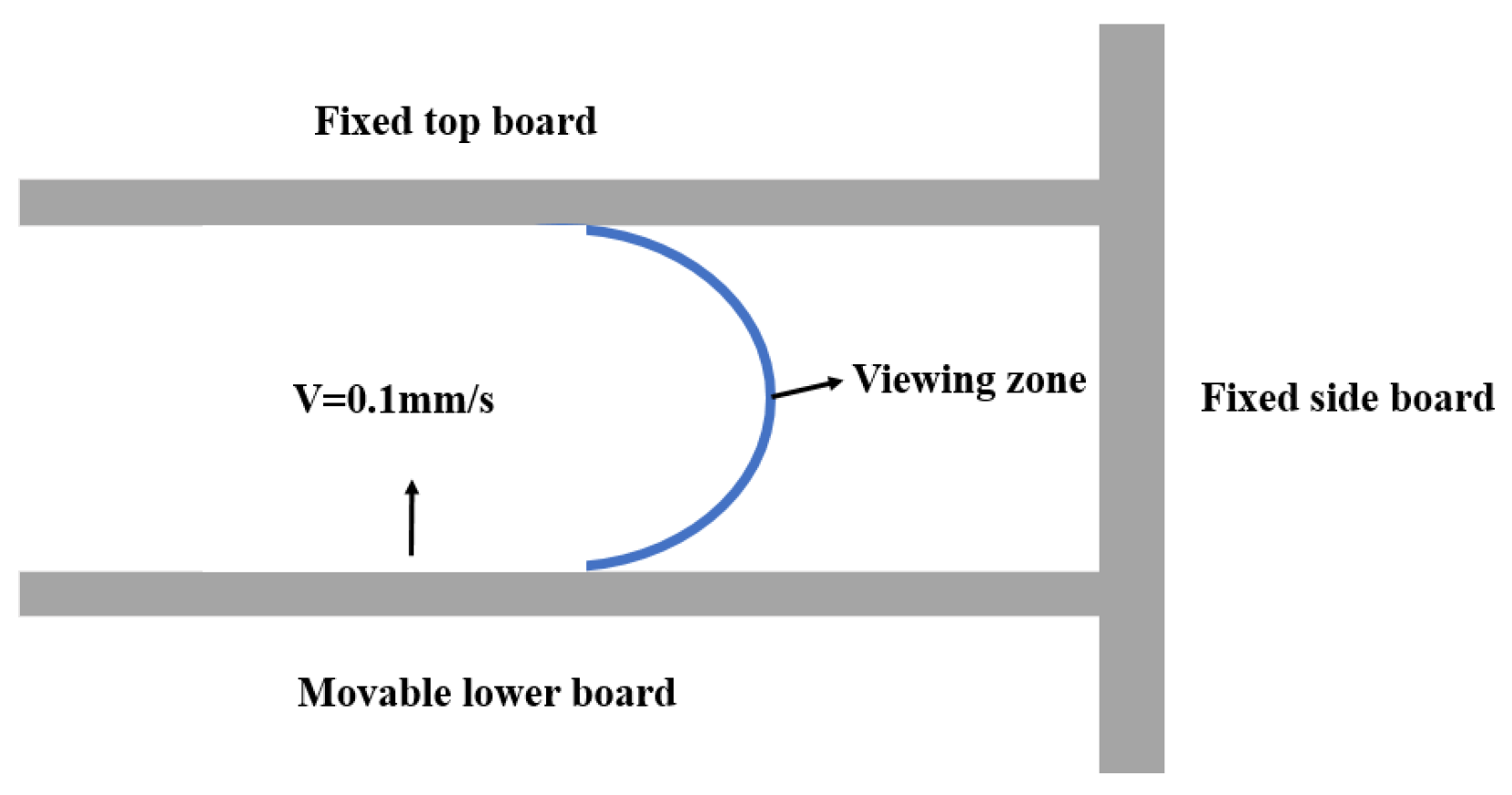
2. Materials and Methods
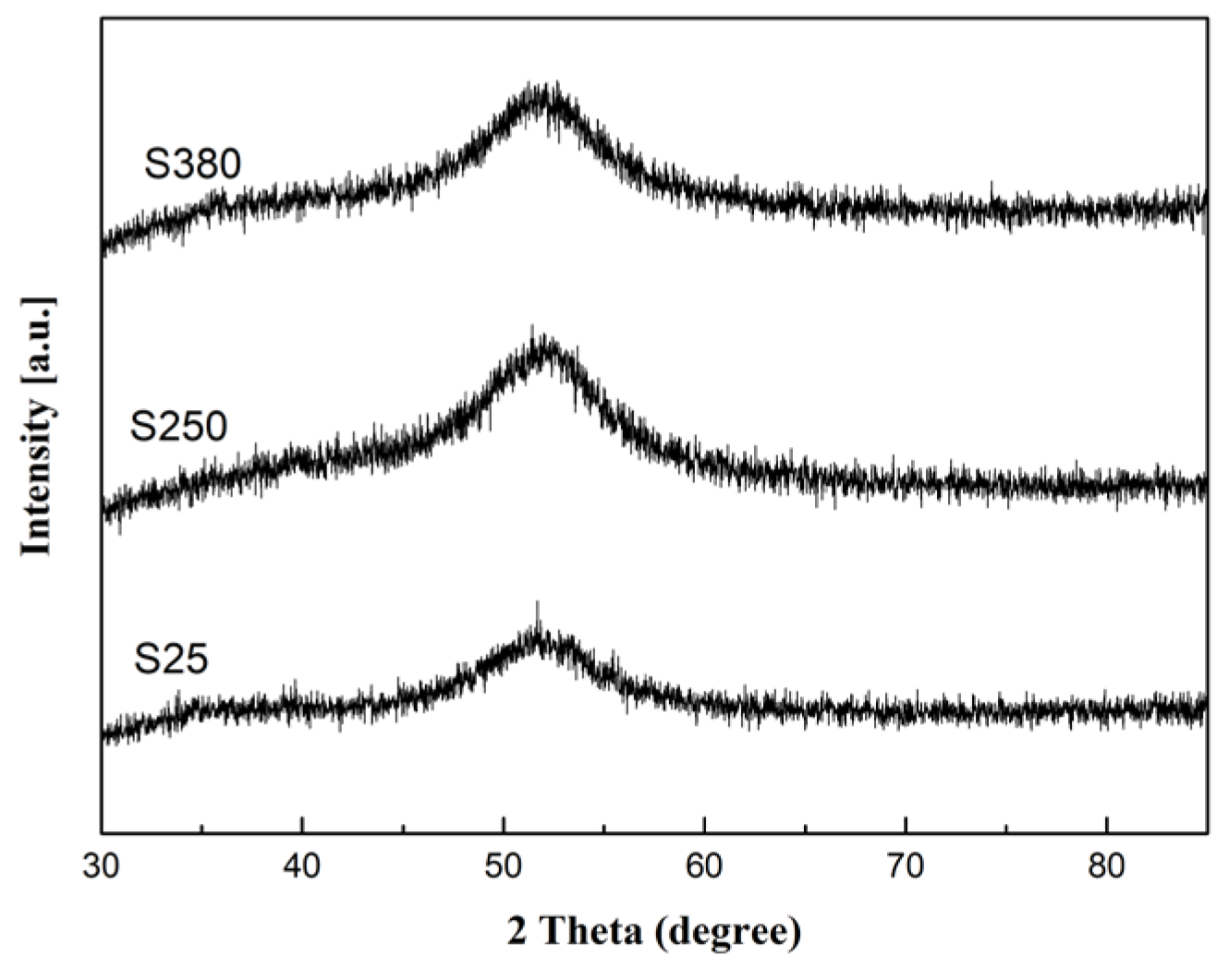
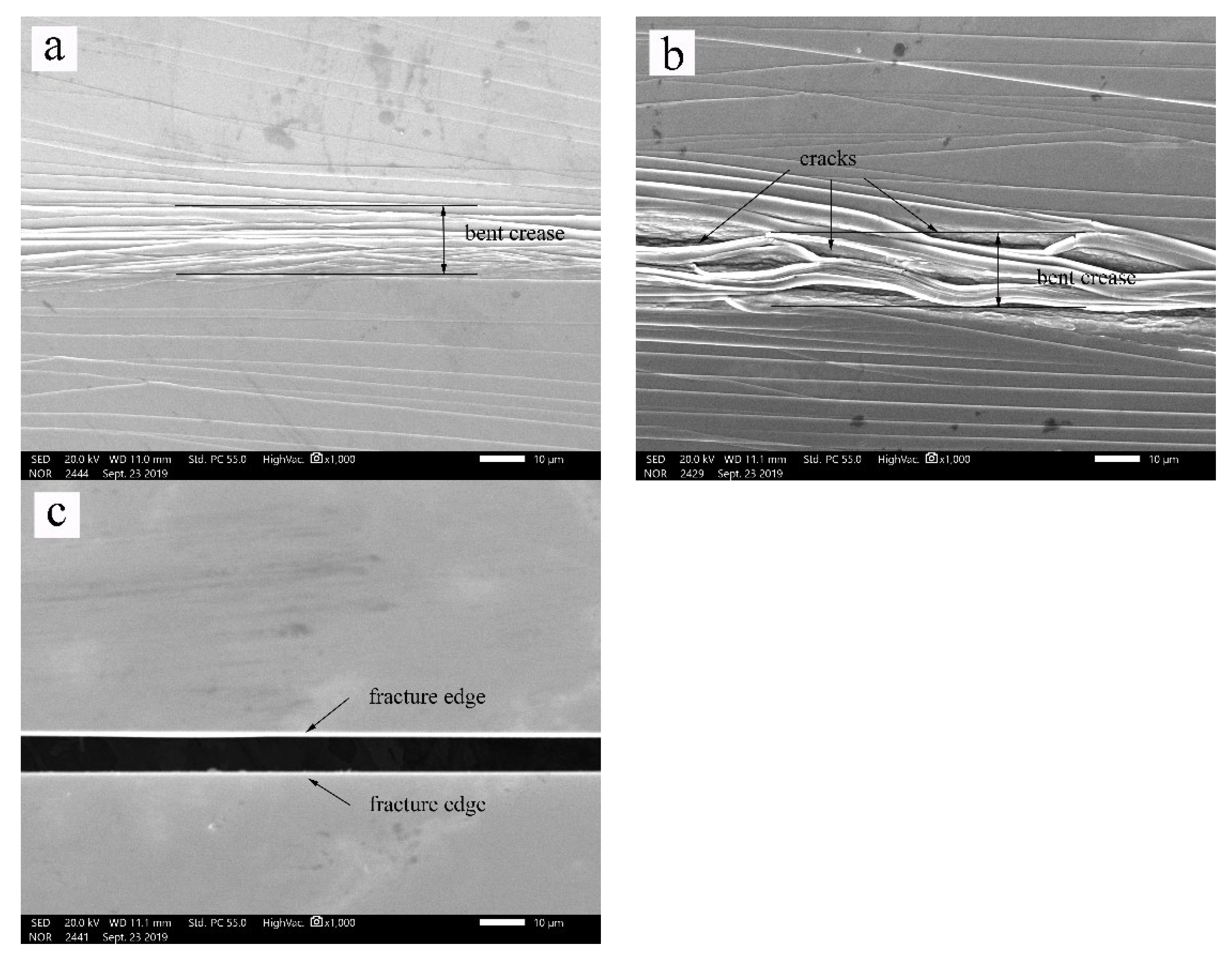
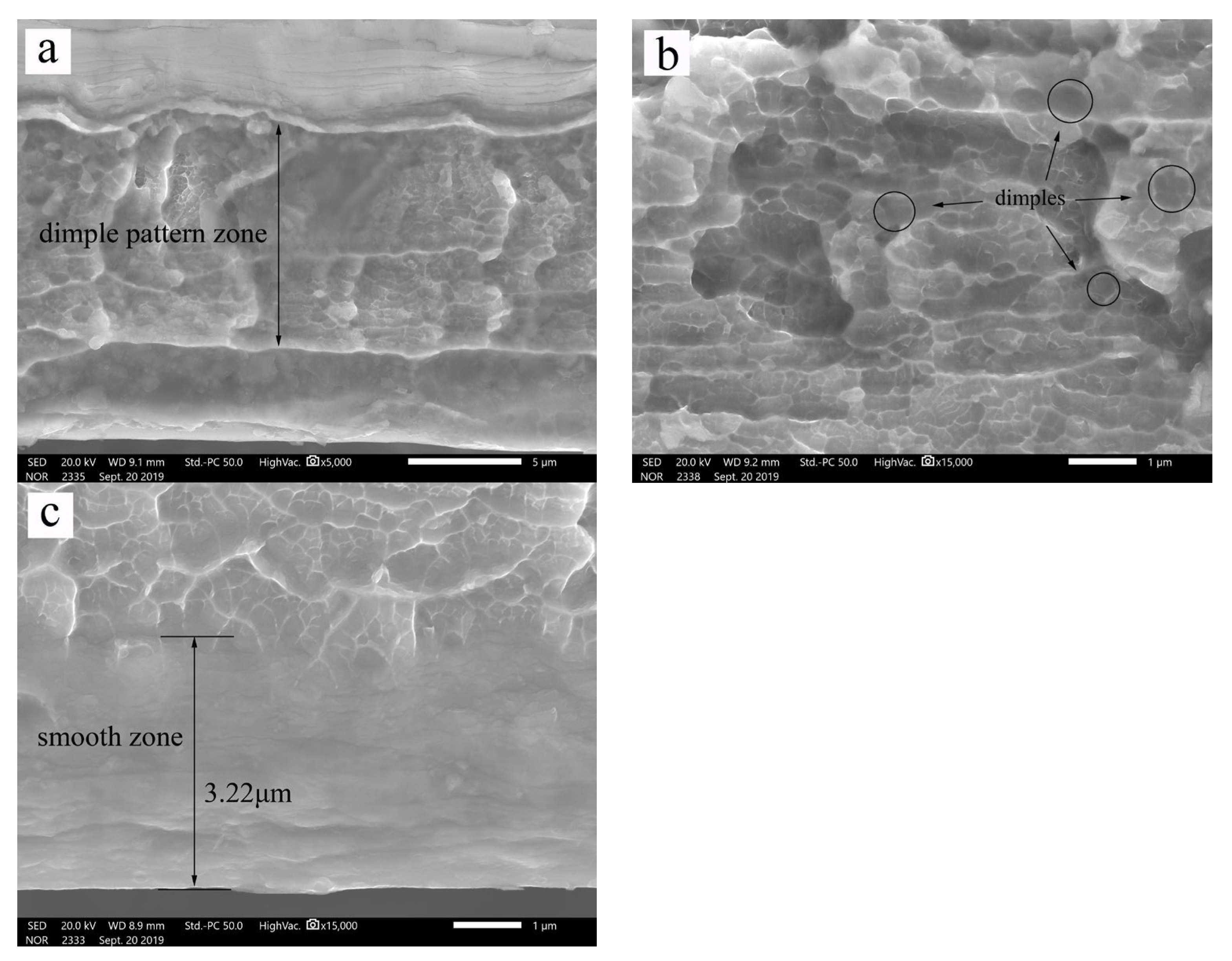
3. Results
Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Minnert, C.; Kuhnt, M.; Bruns, S. Study on the embrittlement of flash annealed Fe85.2B9.5P4Cu0.8Si0.5 metallic glass ribbons. Mater. Des. 2018, 156, 252–261. [Google Scholar] [CrossRef]
- Liang, X.; He, A.; Wang, A.; Pang, J.; Wang, C.; Chang, C. Fe content dependence of magnetic properties and bending ductility of FeSiBPC amorphous alloy ribbons. J. Alloys Compd. 2017, 694, 1260–1264. [Google Scholar] [CrossRef]
- Dong, W.; Han, B.; Hui, J.; Yan, M. Bending behavior and fracture surface characters for FeSiB amorphous ribbons in different free volume state. Appl. Phys. A 2020, 126, 670. [Google Scholar] [CrossRef]
- Argon, A.S. Plastic deformation in metallic glasses. Acta Metall. 1979, 27, 47–58. [Google Scholar] [CrossRef]
- Greer, A.L.; Cheng, Y.Q.; Ma, E. Shear bands in metallic glasses. Mater. Sci. Eng. 2013, R74, 71–132. [Google Scholar] [CrossRef]
- Narayan, R.L.; Raut, D.; Ramamurty, U. A quantitative connection between shear band mediated plasticity and fracture initiation toughness of metallic glasses. Acta Mater. 2018, 150, 69–77. [Google Scholar] [CrossRef]
- Jiang, F.; Jiang, M.Q.; Wang, H.F.; Zhao, Y.L.; He, L.; Sun, J. Shear transformation zone volume determining ductile–brittle transition of bulk metallic glasses. Acta Mater. 2011, 59, 2057–2068. [Google Scholar] [CrossRef]
- Pan, D.; Yokoyama, Y.; Fujita, T.; Liu, Y.H.; Kohara, S.; Inoue, A.; Chen, M.W. Correlation between structural relaxation and shear transformation zone volumeof a bulk metallic glass. Appl. Phys. Lett. 2009, 95, 141909. [Google Scholar] [CrossRef]
- Pan, D.; Inoue, A.; Sakurai, T.; Chen, M.W. Experimental characterization of shear transformation zones for plastic flow of bulk metallic glasses. Proc. Natl. Acad. Sci. USA 2008, 105, 14769–14772. [Google Scholar] [CrossRef] [Green Version]
- Ma, Y.; Ye, J.H.; Peng, G.J.; Wen, D.H.; Zhang, T.H. Nanoindentation study of size effect on shear transformation zone size in a Ni–Nb metallic glass. Mater. Sci. Eng. 2015, 627, 153–160. [Google Scholar] [CrossRef]
- Choi, I.-C.; Zhao, Y.; Kim, Y.-J.; Yoo, B.-G.; Suh, J.-Y.; Ramamurty, U.; Jang, J.-I. Indentation size effect and shear transformation zone size in a bulk metallic glass in two different structural states. Acta Mater. 2012, 60, 6862–6868. [Google Scholar] [CrossRef]
- Chen, Z.Q.; Huang, L.; Huang, P.; Xu, K.W.; Wang, F.; Lu, T.J. Clarification on shear transformation zone size and its correlation with plasticity for Zr-based bulk metallic glass in different structural states. Mater. Sci. Eng. A 2016, 677, 349–355. [Google Scholar] [CrossRef]
- Ma, Y.; Peng, G.J.; Debela, T.T.; Zhang, T.H. Nanoindentation study on the characteristic of shear transformation zone volume in metallic glassy films. Scr. Mater. 2015, 108, 52–55. [Google Scholar] [CrossRef]
- Slipenyuk, A.; Eckert, J. Correlation between enthalpy change and free volume reduction during structural relaxation of Zr55Cu30Al10Ni5 metallic glass. J. Scr. Mater. 2004, 50, 39–44. [Google Scholar] [CrossRef]
- Raghavan, R.; Murali, P.; Ramamurty, U. On factors influencing the ductile-to-brittle transition in a bulk metallic glass. Acta Mater. 2009, 57, 3332. [Google Scholar] [CrossRef]
- Xi, X.K.; Zhao, D.Q.; Pan, M.X.; Wang, W.H.; Wu, Y.; Lewandowski, J.J. Fracture of Brittle Metallic Glasses: Brittleness or Plasticity. Phys. Rev. Lett. 2005, 94, 125510. [Google Scholar] [CrossRef] [Green Version]
- Jiang, M.Q.; Ling, Z.; Meng, J.X.; Dai, L.H. Energy dissipation in fracture of bulk metallic glasses via inherent competition between local softening and quasi-cleavage. Philos. Mag. 2008, 88, 407. [Google Scholar] [CrossRef] [Green Version]
- Wang, G.; Zhao, D.Q.; Bai, H.Y.; Pan, M.X.; Xia, A.L.; Han, B.S.; Xi, X.K.; Wu, Y.; Wang, W.H. Nanoscale Periodic Morphologies on the Fracture Surface of Brittle Metallic Glasses. Phys. Rev. Lett. 2007, 98, 235501. [Google Scholar] [CrossRef] [Green Version]
- Wu, F.F.; Zheng, W.; Deng, J.W.; Qu, D.D.; Shen, J. Super-high compressive plastic deformation behaviors of Zr-based metallic glass at room temperature. Mater. Sci. Eng. A 2012, 541, 199–203. [Google Scholar] [CrossRef]
- Pan, D.; Guo, H.; Zhang, W.; Inoue, A.; Chen, M.W. Temperature-induced anomalous brittle-to-ductile transition of bulk metallic glasses. Appl. Phys. Lett. 2011, 99, 241907. [Google Scholar] [CrossRef]
- Johnson, W.L.; Samwer, K. A universal criterion for plastic yielding of metallic glasses with a (T/Tg) 2/3 temperature dependence. Phys. Rev. Lett. 2005, 95, 195501. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Liu, Y.H.; Wang, D.; Nakajima, K.; Zhang, W.; Hirata, A.; Nishi, T.; Inoue, A.; Chen, M.W. Characterization of nanoscale mechanical heterogeneity in a metallic glass by dynamic force microscopy. Phys. Rev. Lett. 2011, 106, 125504. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Guo, W.; Yamada, R.; Saida, J. Rejuvenation and plasticization of metallic glass by deep cryogenic cycling treatment. Intermetallics 2018, 93, 141–147. [Google Scholar] [CrossRef]
- Inoue, A.; Zhang, T.; Masumoto, T. Glass-forming ability of alloys. J. Non-Cryst. Solids 1993, 156, 598. [Google Scholar] [CrossRef]
- Wu, F.F.; Zhang, Z.F.; Mao, S.X. Size-dependent shear fracture and global tensile plasticity of metallic glasses. Acta Mater. 2009, 57, 257. [Google Scholar] [CrossRef]

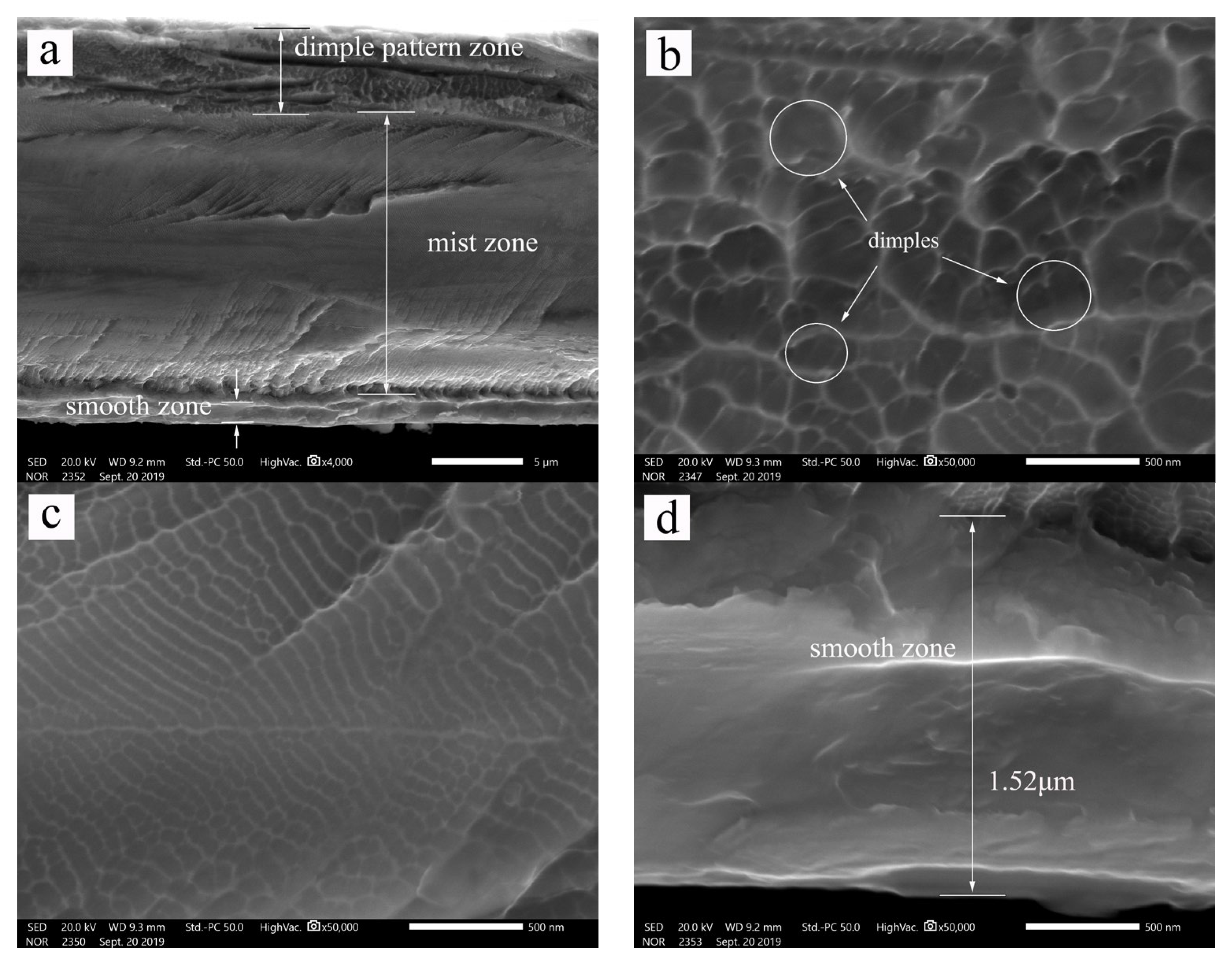
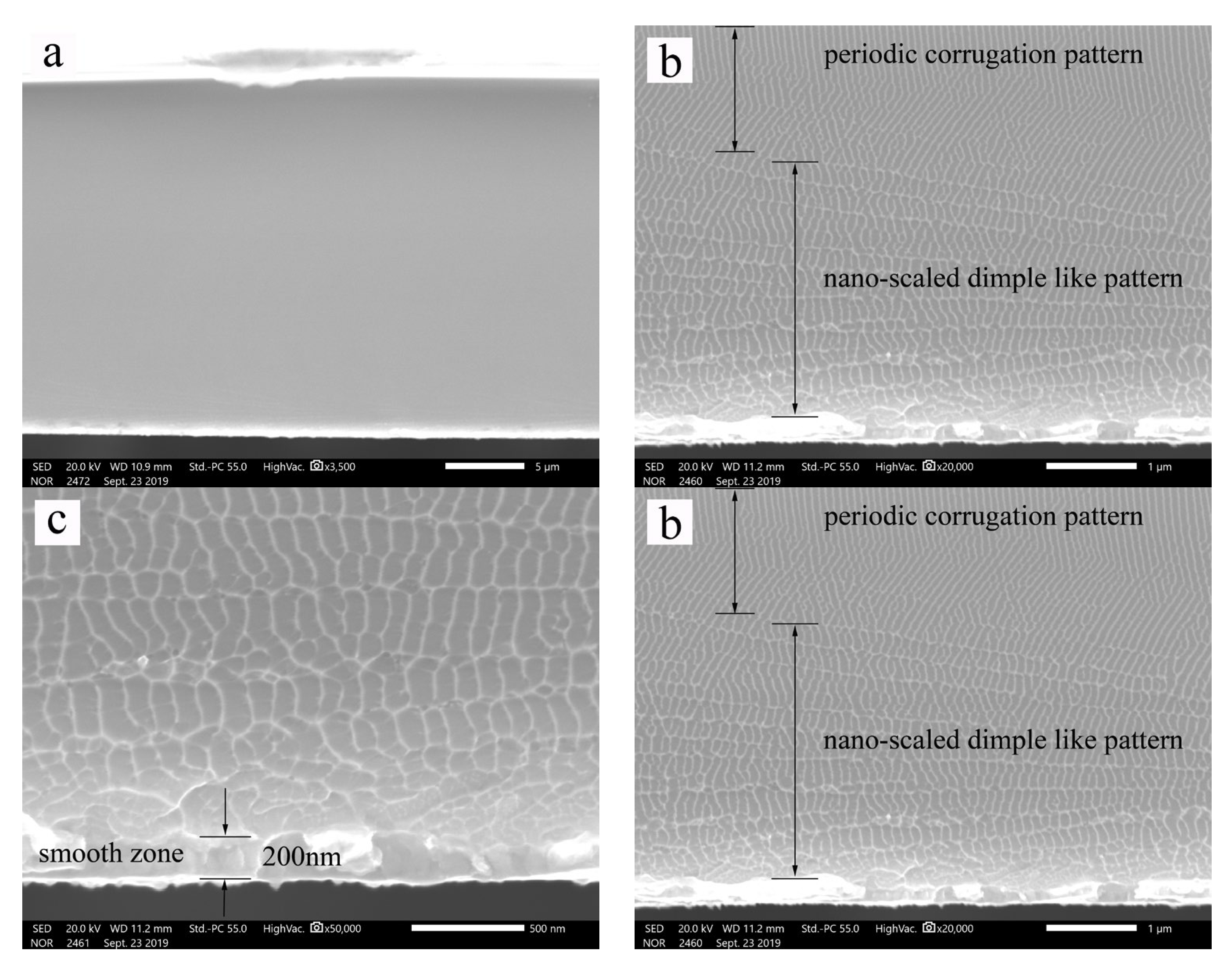
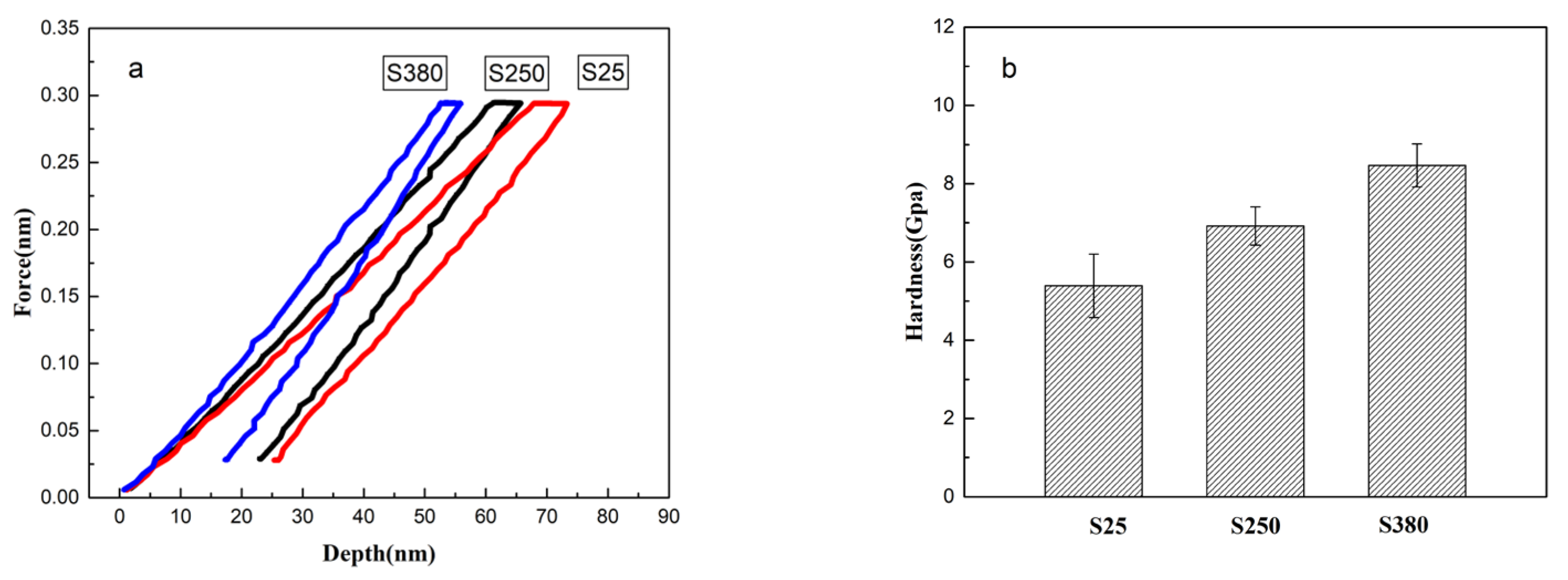
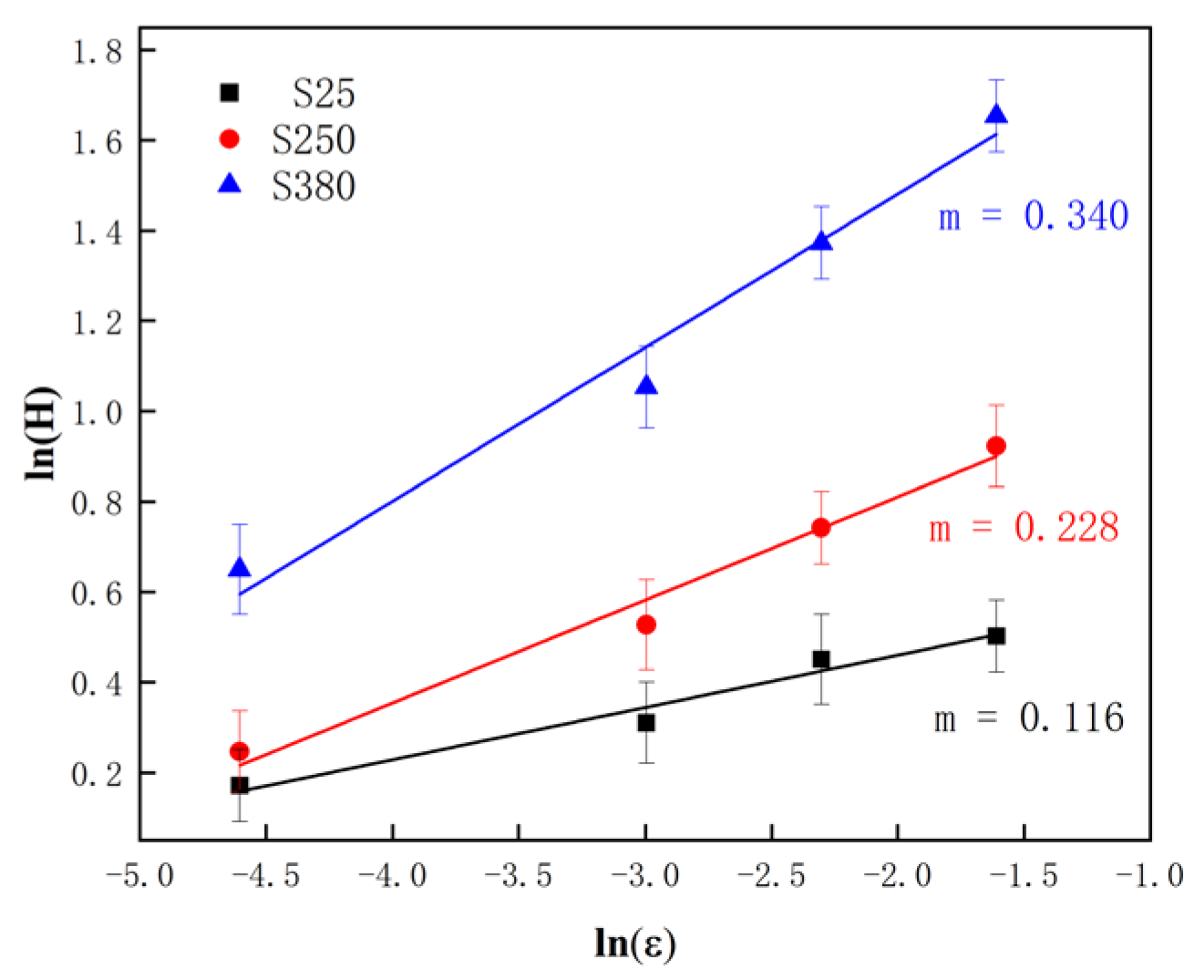
| Sample | Average Hardness H (Gpa) | Strain Rate Sensitivity, m | STZ Volume Ω (nm3) | STZ Size N (Atoms) |
|---|---|---|---|---|
| S25 | 5.393 | 0.340 | 0.54 | 66 |
| S250 | 6.922 | 0.228 | 0.63 | 76 |
| S380 | 8.472 | 0.116 | 1.01 | 123 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dong, W.; Dong, M.; Qian, D.; Zhang, J.; Zhu, S. Shear Transformation Zone and Its Correlation with Fracture Characteristics for Fe-Based Amorphous Ribbons in Different Structural States. Metals 2023, 13, 757. https://doi.org/10.3390/met13040757
Dong W, Dong M, Qian D, Zhang J, Zhu S. Shear Transformation Zone and Its Correlation with Fracture Characteristics for Fe-Based Amorphous Ribbons in Different Structural States. Metals. 2023; 13(4):757. https://doi.org/10.3390/met13040757
Chicago/Turabian StyleDong, Weiwei, Minshuai Dong, Danbo Qian, Jiankang Zhang, and Shigen Zhu. 2023. "Shear Transformation Zone and Its Correlation with Fracture Characteristics for Fe-Based Amorphous Ribbons in Different Structural States" Metals 13, no. 4: 757. https://doi.org/10.3390/met13040757
APA StyleDong, W., Dong, M., Qian, D., Zhang, J., & Zhu, S. (2023). Shear Transformation Zone and Its Correlation with Fracture Characteristics for Fe-Based Amorphous Ribbons in Different Structural States. Metals, 13(4), 757. https://doi.org/10.3390/met13040757






