Influence of Temperature–Humidity Sensor Housing Depth on Concrete and Mortar Compressive Strength
Abstract
:1. Introduction
1.1. Backgrounds
1.2. Previous Study
1.3. Research Objective
2. Materials and Methods
2.1. Concrete Sample Preparation
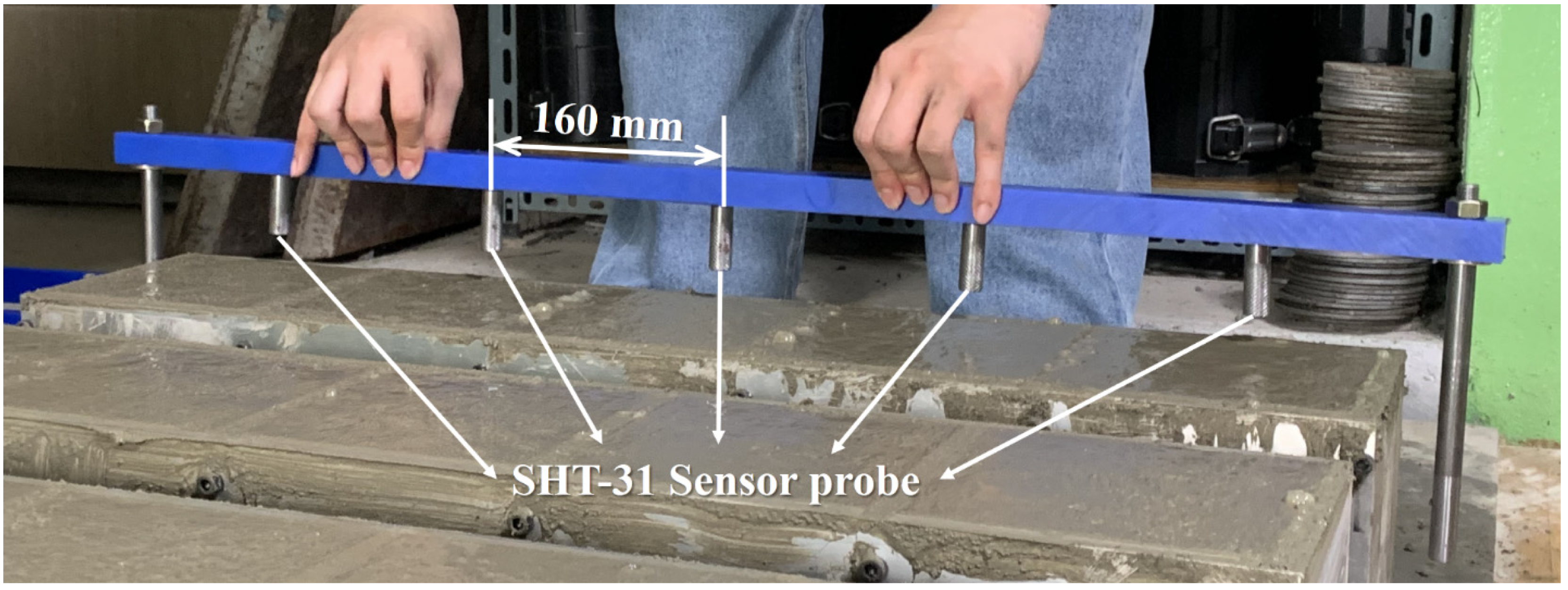
2.2. Sensor Instrumentation and Sample Size Configuration
2.3. Production of Test Samples
3. Finite Element Analysis
4. Results and Discussion
5. Conclusions
- Mortar and concrete exhibit different material characteristics, resulting in different compressive strengths at the same burial depth. The compression test indicated that the mortar strength increased as the sensor housing’s embedment depth increased and reached the desired strength at a burial depth of 30 mm. In comparison, the concrete already exhibited high compressive strength at a depth of 10 mm. Based on this, it is recommended that sensor housings be embedded within concrete at least 20 mm away from the concrete surface.
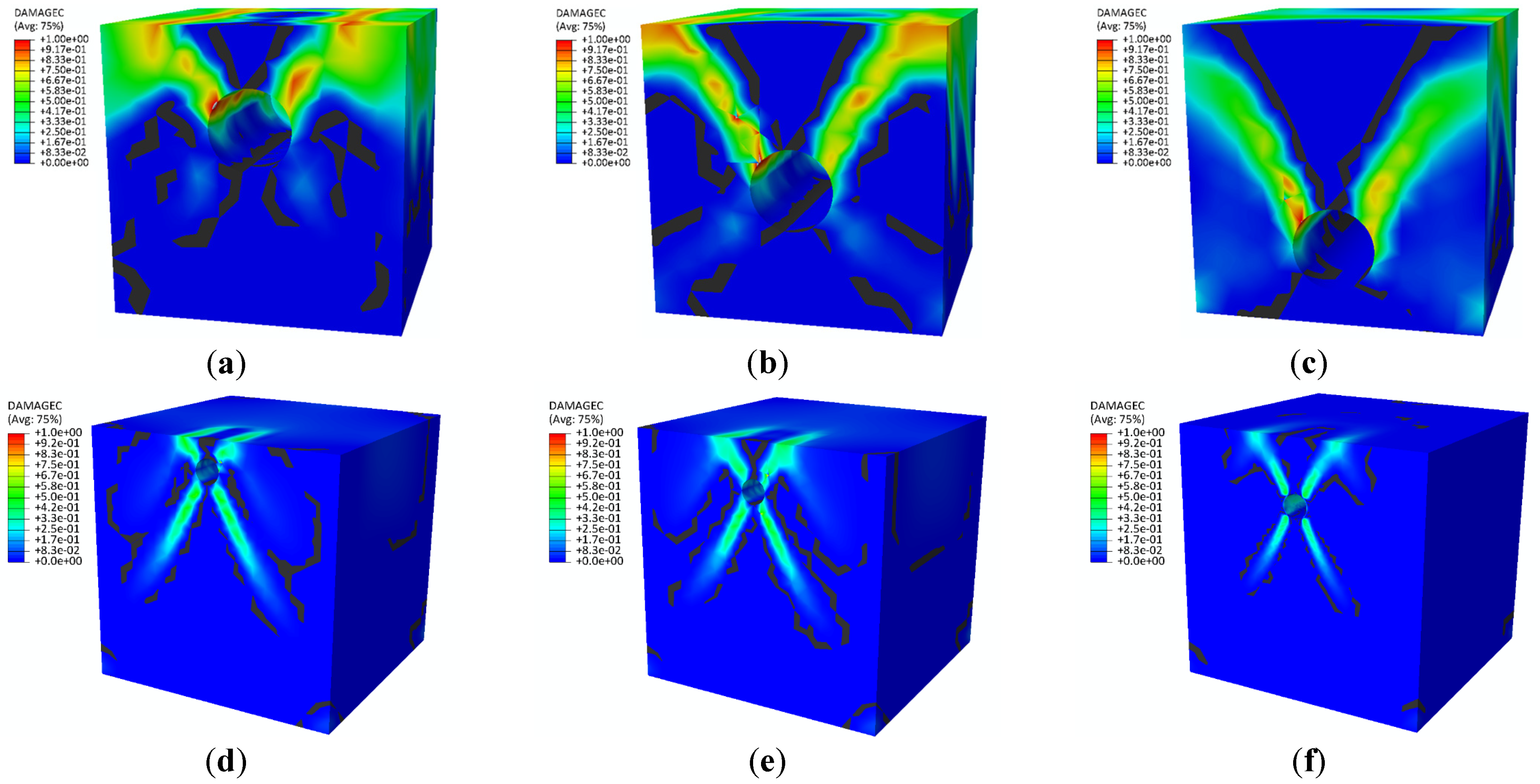
- Initial cracks were observed where the sensor housing was located, and the shape of these cracks spreading around the housing was captured. This was also observed in the mortar and is presumed to be due to stress concentration at the interface between the sensor housing and the concrete. These findings indicate that the initiation and spread of cracks may differ depending on the placement of the sensor;
- Additionally, the study analyzed the damage patterns after embedding sensor housings in mortar and concrete samples. The results confirmed the formation of shear bands in diagonal directions at all burial depths, allowing the failure patterns in the sample to be identified in relation to material ductility and fracture mechanisms;
- Both mortar and concrete are crucially used in the construction industry, but they serve different purposes and have different scopes of application. Mortar is primarily used for small-scale repair work, and the impact of sensor housings is relatively limited in these cases. Conversely, concrete is employed in large structures; therefore, the influence of sensor housings is expected to be minimal compared to the overall structure.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Musarat, M.A.; Hameed, N.; Altaf, M.; Alaloul, W.S.; Al Salaheen, M.; Alawag, A.M. Digital Transformation of the Construction Industry: A Review. In Proceedings of the 2021 International Conference on Decision Aid Sciences and Application (DASA), Sakheer, Bahrain, 7–8 December 2021; pp. 897–902. [Google Scholar] [CrossRef]
- Štefanič, M.; Stankovski, V. A review of technologies and applications for smart construction. Proc. Inst. Civ. Eng. Civ. Eng. 2018, 172, 83–87. [Google Scholar] [CrossRef]
- Scuro, C.; Sciammarella, P.F.; Lamonaca, F.; Olivito, R.S.; Carni, D.L. IoT for structural health monitoring. IEEE Instrum. Meas. Mag. 2018, 21, 4–14. [Google Scholar] [CrossRef]
- Wang, M.L.; Lynch, J.P.; Sohn, H. Introduction to sensing for structural performance assessment and health monitoring. In Sensor Technologies for Civil Infrastructures: Sensing Hardware and Data Collection Methods for Performance Assessment; Woodhead: Cambridge, UK, 2014; pp. 57–264. [Google Scholar]
- Lamonaca, F.; Sciammarella, P.; Scuro, C.; Carni, D.; Olivito, R. Internet of things for structural health monitoring. In Proceedings of the 2018 Workshop on Metrology for Industry 4.0 and IoT, Brescia, Italy, 16–18 April 2018; pp. 95–100. [Google Scholar] [CrossRef]
- Merzbacher, C.; Kersey, A.D.; Friebele, E. Fiber optic sensors in concrete structures: A review. Smart Mater. Struct. 1996, 5, 196–208. [Google Scholar] [CrossRef]
- Quinn, W.; Kelly, G.; Barrett, J. Development of an embedded wireless sensing system for the monitoring of concrete. Struct. Health Monit. 2012, 11, 381–392. [Google Scholar] [CrossRef]
- Ekaputri, J.J.; Maekawa, K.; Ishida, T. Experimental study on internal RH of BFS mortars at early age. Mater. Sci. Forum 2016, 857, 305–310. [Google Scholar] [CrossRef]
- Zhou, H.; Liu, Y.; Lu, Y.; Dong, P.; Guo, B.; Ding, W.; Xing, F.; Liu, T.; Dong, B. In-situ crack propagation monitoring in mortar embedded with cement-based piezoelectric ceramic sensors. Constr. Build. Mater. 2016, 126, 361–368. [Google Scholar] [CrossRef]
- Pan, H.H.; Huang, M.-W. Piezoelectric cement sensor-based electromechanical impedance technique for the strength monitoring of cement mortar. Constr. Build. Mater. 2020, 254, 119307. [Google Scholar] [CrossRef]
- Kosmatka, S.H.; Panarese, W.C.; Kerkhoff, B. Design and Control of Concrete Mixtures; PCA: Warsaw, Poland, 2009. [Google Scholar]
- Mehta, P.K.; Monteiro, P.J. Concrete: Microstructure, Properties, and Materials, 4th ed.; McGraw-Hill: New York, NY, USA, 2005. [Google Scholar]
- Powers, T.C. The Properties of Fresh Concrete; Wiley: New York, NY, USA, 1968. [Google Scholar]
- Kim, C.; Song, Y.; Cho, J.; Kang, J.; Yeon, J. Effect of Embedded Depth of Copper-Nickel-Plated Sensor Probes on Compressive Strength Development of Mortar. Sustainability 2023, 15, 10772. [Google Scholar] [CrossRef]
- ASTM C928/928M-20a; Standard Specification for Packaged, Dry, Rapid-Hardening Cementitious Materials for Concrete Repairs. ASTM International: West Conshohocken, PA, USA, 2020.
- ASTM C39/39M-21; Standard Test Method for Compressive Strength of Cylindrical Concrete Specimens. ASTM Internationa: West Conshohocken, PA, USA, 2021.
- BS 1881-116; Method for Determination of Compressive Strength of Concrete Cubes. BSI: London, UK, 1983.
- BS EN 12390-3; Testing Hardened Concrete-Part 3: Compressive Strength of Test Specimens. BSI: London, UK, 2002.
- ASTM C305-21; Standard Practice for Mechanical Mixing of Hydraulic Cement Pastes and Mortars of Plastic Consistency. ASTM International: West Conshohocken, PA, USA, 2021.
- Sensirion. Available online: https://www.sensirion.com/ (accessed on 31 August 2023).
- Demanega, I.; Mujan, I.; Singer, B.; Andelković, A.; Babich, F.; Lucina, D. Performance assessment of low-cost environmental monitors and single sensors under variable indoor air quality and thermal conditions. Build. Environ. 2021, 187, 107415. [Google Scholar] [CrossRef]
- McCauley, D.; Nackley, L.; Kelley, J. Demonstration of a low-cost and open-source platform for on-farm monitoring and decision support. Comput. Electron. Agric. 2021, 187, 106284. [Google Scholar] [CrossRef]
- Khoa, T.A.; Lam, P.D.; Nam, N.H. An efficient energy measurement system based on the TOF sensor for structural crack monitoring in architecture. J. Inf. Telecommun. 2023, 7, 56–72. [Google Scholar] [CrossRef]
- Ma’Mari, F.A.; Moorsom, T.; Teobaldi, G.; Deacon, W.; Prokscha, T.; Luetkens, H.; Lee, S.; Sterbinsky, G.E.; Arena, D.A.; MacLaren, D.A. Beating the Stoner criterion using molecular interfaces. Nature 2015, 524, 69–73. [Google Scholar] [CrossRef]
- Wu, X.; Wen, B. A cauliflower-shaped nickel@ porous calcium silicate core-shell composite: Preparation and enhanced electromagnetic shielding performance. Compos. Sci. Technol. 2020, 199, 108343. [Google Scholar] [CrossRef]
- ASTM C511-21; Standard Specification for Mixing Rooms, Moist Cabinets, Moist Rooms, and Water Storage Tanks Used in the Testing of Hydraulic Cements and Concretes. ASTM International: West Conshohocken, PA, USA, 2021.
- Heungjin. Available online: http://www.heungjin.co.kr/ (accessed on 31 August 2023).
- Lubliner, J.; Oliver, J.; Oller, S.; Oñate, E. A plastic-damage model for concrete. Int. J. Solids Struct. 1989, 25, 299–326. [Google Scholar] [CrossRef]
- Lee, J.; Fenves, G.L. A plastic-damage concrete model for earthquake analysis of dams. Earthq. Eng. Struct. Dyn. 1998, 27, 937–956. [Google Scholar] [CrossRef]
- Copper Nickel 10%, UNS C70600. Available online: https://www.matweb.com/search/datasheet.aspx?MatGUID=d5133c23a81e4b5cba35a45f8dda6502 (accessed on 31 August 2023).
- Genikomsou, A.S.; Polak, M.A. Finite element analysis of punching shear of concrete slabs using damaged plasticity model in ABAQUS. Eng. Struct. 2015, 98, 38–48. [Google Scholar] [CrossRef]
- Song, Y.; Voyiadjis, G.Z. Strain gradient finite element model for finite deformation theory: Size effects and shear bands. Comput. Mech. 2020, 65, 1219–1246. [Google Scholar] [CrossRef]


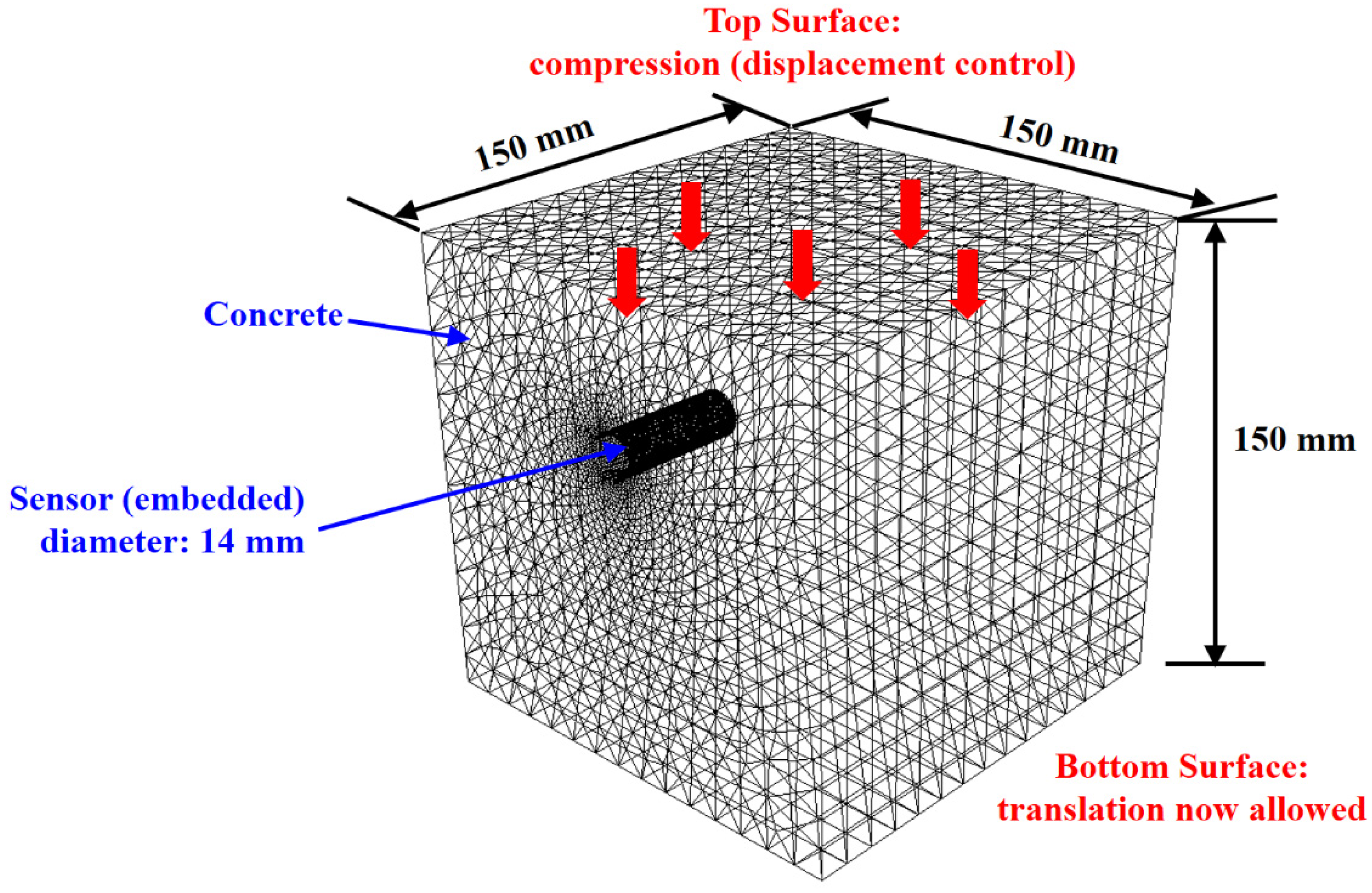




| Material Property | Concrete | Sensor Housing |
|---|---|---|
| Modulus of elasticity (MPa) | 20,111 | 140,000 |
| Poisson’s ratio (ν) | 0.2 | 0.35 |
| Function coefficient (α) | 1.16 | - |
| Angle of Dilation(⁰) | 35 | - |
| Eccentricity (ϵ) | 0.1 | - |
| Tensile-to-compressive-meridian ratio () | 0.667 | - |
| Viscous property (μ) | 0.01 |
| Housing Embedment Depth (mm) | Compressive Strength Results of Concrete Samples (MPa) | |||
|---|---|---|---|---|
| Sample #1 | Sample #2 | Sample #3 | SD | |
| 10 | 25.508 | 26.834 | 26.024 | 0.55 |
| 20 | 28.587 | 28.916 | 27.701 | 0.51 |
| 30 | 28.364 | 29.687 | 30.244 | 0.79 |
| Non-embedded | 32.540 | 31.255 | 31.974 | 0.53 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, C.; Song, Y.; Cho, J.; Kim, H.; Yeon, J. Influence of Temperature–Humidity Sensor Housing Depth on Concrete and Mortar Compressive Strength. Buildings 2023, 13, 2762. https://doi.org/10.3390/buildings13112762
Kim C, Song Y, Cho J, Kim H, Yeon J. Influence of Temperature–Humidity Sensor Housing Depth on Concrete and Mortar Compressive Strength. Buildings. 2023; 13(11):2762. https://doi.org/10.3390/buildings13112762
Chicago/Turabian StyleKim, Chaehyeon, Yooseob Song, Junhwi Cho, Hyunhwan Kim, and Jaeheum Yeon. 2023. "Influence of Temperature–Humidity Sensor Housing Depth on Concrete and Mortar Compressive Strength" Buildings 13, no. 11: 2762. https://doi.org/10.3390/buildings13112762
APA StyleKim, C., Song, Y., Cho, J., Kim, H., & Yeon, J. (2023). Influence of Temperature–Humidity Sensor Housing Depth on Concrete and Mortar Compressive Strength. Buildings, 13(11), 2762. https://doi.org/10.3390/buildings13112762










