1. Introduction
Steel is an important material for the manufacture of products, such as precision machinery. It is easy to develop fractures caused by early fatigue damage and microcracks when exposed in harsh environments, such as high pressure, high temperature, and high speed for a long-term [
1]. It threatens the safe operation of equipment and the safety of people’s lives. EMATs excite and receive acoustic waves through the electromagnetic coupling, and are widely used in the non-destructive testing field of metallic materials [
2,
3,
4,
5]. Compared with piezoelectric acoustic transducers, the detection efficiency is improved due to EMATs’ characteristics of non-contact and strong environmental adaptability. However, there are some problems in EMATs, such as low signal-to-noise ratio of received signals [
6,
7] and low transduction efficiency [
8]. The signal is 20~40 dB lower than that of piezoelectric acoustic transducers [
9]. In the detection of ferromagnetic materials, the magnet of EMATs generates a magnetic force to attract the steel, which brings inconvenience to the detection. Although the conventional EMATs do not contact the tested material directly, it is limited by the small lift-off distance. In addition, the detection requirements of the ferromagnetic material for the higher lift-off distance cannot be satisfied in extreme environments such as high temperatures.
In view of conversion efficiency and signal to noise ratio performance of EMATs, scholars have proposed some methods such as EMATs structure optimization [
10,
11,
12,
13,
14]. In different metal materials, the main transduction mechanism of EMATs is different in generating ultrasonic waves. The Lorentz force is the main mechanism in non-ferromagnetic metal materials. However, in ferromagnetic materials, the Lorentz force, magnetizing force, and magnetostrictive force work together [
15]. Compared with the Lorentz force and magnetostrictive force, the contribution of the magnetizing force is little in the generation of ultrasonic waves in steel materials and can be ignored [
16]. Ogi found that the magnetostrictive force is affected by the direction and strength of the static bias magnetic field [
17]. Ribichini proposed that the Lorentz force is the main mechanism of ferromagnetic materials in the normal bias field (vertical magnetic field) [
18]. Ashigwuike found that when the external static magnetic field is a horizontal magnetic field, the main transduction mechanism of EMATs can be the magnetostrictive force [
19]. In the horizontal magnetic field, Xu proved that the contribution of the low-carbon steel magnetostrictive mechanism for the EMATs efficiency increases with temperature increasing by simulation and experiment [
20].
The existing research mainly focused on the optimization of the EMATs structure, whereas there is inadequate research in the improvement of the excitation power of EMATs.
Rueter established a simulation model of an electromagnetic acoustic excitation transducer without a bias magnetic field. The simulation and experiment results showed that acoustic waves can be effectively excited by high current in a circular coil [
21,
22]. A compact EMAT with a solid-state circuit and no permanent magnets was proposed by Rueter, which operates at MHz frequencies and detects through pulse echo detection method in narrow environments [
23]. Tkocz demonstrated a new high-power phase-controlled EMAT that detected artificial internal defects of cast steel samples by a high amplitude superposition signal, composed of the signals excited by phased array coils [
24]. A simple high-power EMAT without an external bias magnetic field was proposed by Rieger to detect defects of metal cans with liquid through the echo mode of longitudinal ultrasound [
25]. Boni designed a multiple-rail acoustic excitation source with reproducible pulse power output and the switching noise is effectively reduced [
26]. The high-power thyristor integrated circuit is generally used in conventional high-power pluse EMATs as an acoustic excitation source, such as a four-channel IGBT excitation source. Due to the shortcomings of thyristors, such as the thermal effects, low switching frequency, and low withstand voltage, the existing transducer can only excite low-frequency acoustic waves. Moreover, it is difficult to excite thousands of amperes of tone-burst excitation current in the electromagnetic acoustic excitation coil.
Above all, the transduction efficiency of conventional EMATs is low, especially at a higher lift-off distance. There is an upper limit in the bias magnetic field strength, which limits the maximum ultrasonic power, and ferromagnetic particles can be attracted by the magnets of conventional EMATs, the measurement results can be affected by which [
27]. In order to solve the above problems, in this paper, a high-energy electromagnetic acoustic excitation system was designed without a static bias magnetic field, and a high-power LC oscillation circuit was used as an excitation source. A transient high voltage is applied to the excitation coil. Then a large current is generated in the coil to excite a dynamic strong magnetic field. The dynamic magnetic field interacts with the induced eddy current to generate a powerful electromagnetic force that can generate the acoustic wave. This method solves the problem that the excitation source power output is limited. The natural frequency of the LC oscillation circuit can be changed to achieve acoustic excitation in each frequency band, by simultaneously adjusting the LC circuit capacitance and the electrical parameters of the excitation coil. The magnetic field excited by the high-energy pulse coil in the eddy current layer of the tested material is horizontal. In order to study the main transduction mechanism, an EMAT lift-off characteristic experiment was carried out, and the relationship between the EMAT lift-off distance and the main action mechanism of ultrasonic waves was analyzed. The EMAT without permanent magnets avoids the effect of the bias magnetic fields, and Lamb waves are very sensitive to the change of the external and internal acoustic impedance of the propagation medium. The requirement for sensitivity is satisfied for the detection of the ferromagnetic material.
2. Electromagnetic Acoustic Excitation Transduction Mechanism of Ferromagnetic Materials Excited by the High-Energy Pulse
The three transductions mechanism of Lorentz force, magnetostrictive force, and magnetizing force exists simultaneously in ferromagnetic metal materials. The magnetostrictive force and the magnetizing force change nonlinearly with the applied magnetic field. Therefore, they were combined with the applied magnetic field during analysis. The Lorentz force transduction mechanism of ferromagnetic materials is consistent with non-ferromagnetic metal materials [
16]. Due to the different electromagnetic parameters of materials, the magnitude of the Lorentz force is different. In metal materials, the Lorentz force is generated by the interaction between induced eddy current and applied dynamic magnetic field, and the induced pulsed eddy current field is expressed as,
where
A is the magnetic vector potential,
σ is the material conductivity,
μ is the material permeability, and
J is the current density in the coil.
The magnetic flux density
B and induced pulse eddy
Je meet:
In metal materials, the Lorenz force is expressed as,
where,
FL is the Lorentz force in the metal material,
Je is current density in the coil,
B is dynamic magnetic flux density. There is no static bias magnetic field in the high-energy pulse EMAT. Lorentz force is generated by the interaction of dynamic magnetic field
B and pulse eddy
Je. The frequency of
FL is twice the frequency of the current in the excitation coil, and the sensitivity of detection defects can be improved by the high-frequency acoustic signal [
28].
The discharge circuit of the high-energy pulse electromagnetic acoustic excitation source can be simplified as a series connection of a discharge capacitor C, an excitation coil L, and an equivalent resistance R. The discharge process can be simplified to a LC second-order circuit zero input response.
The capacitor’s initial voltage
uc and inductor current
i are associated with reference direction. The characteristic equation of the circuit of high-energy pulse excitation source can be expressed as,
where
L is the value of coil inductance,
C is the value of capacitor capacitance,
R is the circuit equivalent resistance,
uc is the initial capacitor voltage, and
s is the Laplace transform.
The requirement about the discharge circuit parameters of the high-energy pulse excitation source should be met, that
R is much smaller than
. In this way, the circuit operates in the zero-input underdamped state of the second-order circuit. The variation of current with time is a gradual decaying sinusoidal function relationship, in the discharge circuit. The Equations (5)–(7) combined to solve the current function relationship is Equation (8):
The represents the damping coefficient, and its value is proportional to the equivalent resistance in the circuit, which affects the rate of decay of current in the circuit.
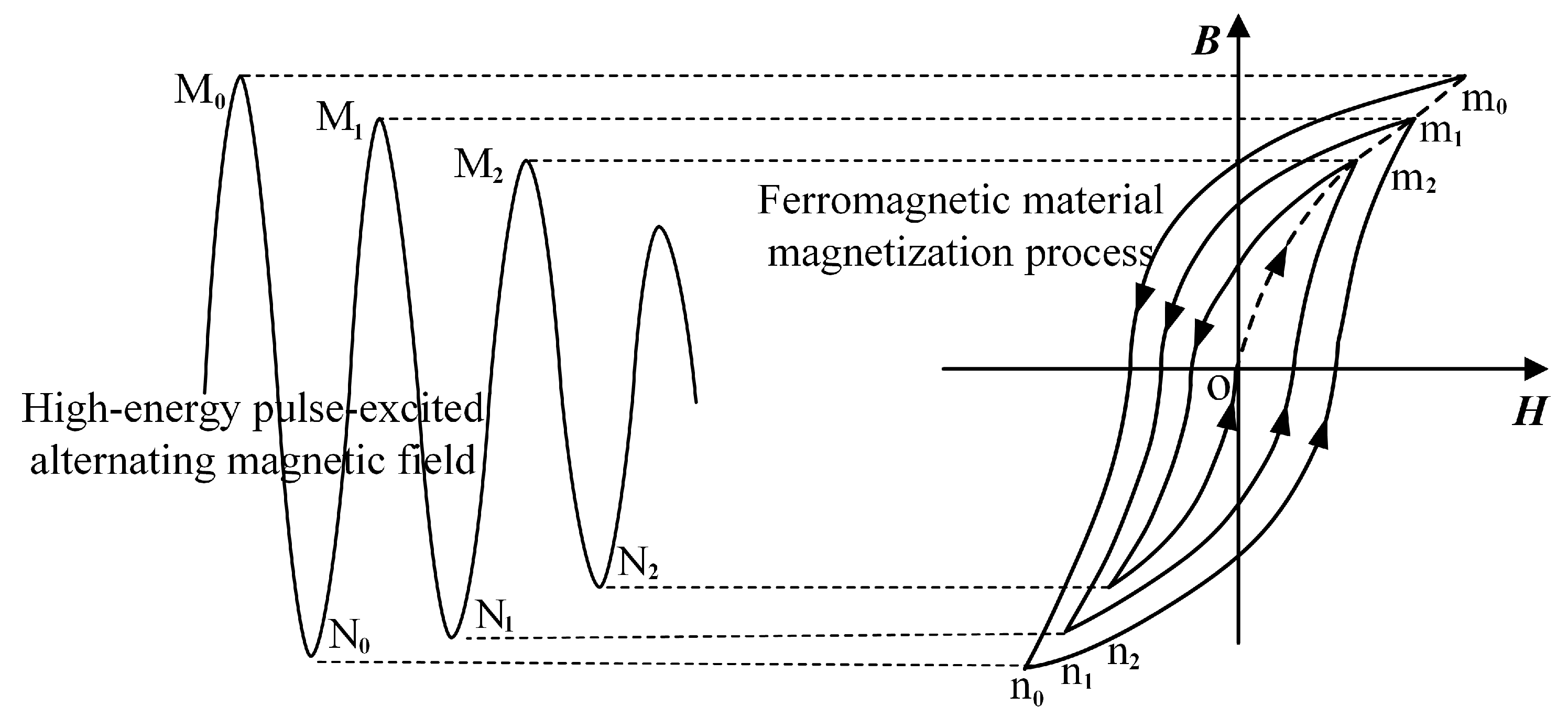
Due to the lack of a static bias magnetic field, hysteresis loops on the magnetization curve do not be formed in the magnetization process, but a cluster of hysteresis loops that decay to zero. The magnetization process is shown in
Figure 1. The alternating magnetic field reaches the maximum M
0 from zero, and the high-energy pulse electromagnetic acoustic magnetization changes along the magnetization curve O-m
0. The magnetic flux density
B increases with the magnetic field strength
H to the maximum. The alternating magnetic field varies from a maximum value M
0 to N
0 and then to M
1 in a decay cycle. The magnetic flux density
B also varies in a period along m
0-n
0-m
1 due to the hysteresis effect in ferromagnetic materials. In ferromagnetic materials, the magnetic flux density
B decays periodically with the magnetic field strength
H, and finally decays to zero.
Due to the action of the alternating magnetic field, acoustic waves are excited by the mechanical vibration which is caused by periodic stretching and compression of the material. The magnetizing force
FM is generated by the alternating magnetic field, is shown in Equation (9),
where
μ0 represents magnetic permeability in the vacuum, and
M represents the magnetization vector.
In addition to the magnetic field affecting the magnetization of ferromagnetic materials, the EMAT conversion mechanism of ferromagnetic materials also adds a magnetostrictive conversion mechanism. For ferromagnetic materials, the magnetostrictive constitutive relationship can be expressed as,
where
Gε is the strain matrix,
Tσ is the stress tensor matrix,
KμT is the reversible magnetic permeability matrix of the material,
μ0 represents the vacuum magnetic permeability,
H is the dynamic magnetic field strength,
B is the magnetic induction intensity,
DM is the piezoelectric magnetic matrix,
Cc is the stiffness matrix, and
NT is the inverse pressure magnetic matrix. The first equation in Equation (10) describes the magnetostrictive effect, which is the strain caused by the application of a magnetic field. The second equation describes the inverse magnetostrictive effect, which is the change in flux density detected by the sensor when the stress generates a change in flux. When an alternating magnetic field is applied to the ferromagnetic material, the tested material is magnetized and stressed, and the constitutive equation of the ferromagnetic material is corrected. Its magnetic field strength
H and magnetostrictive force
fM are satisfied:
The transduction mechanism in the excitation of high-energy pulse power can be expressed as:
The first part is the magnetizing force, the second part is the Lorentz force, and the third part is the magnetostrictive force. However, it can be ignored in most cases as the magnetizing force is relatively small.
3. Simulation Analysis of High-Energy Pulse EMAT
In this paper, COMSOL Multiphysics was used to establish a high-energy pulse EMAT model. The effects of Lorentz force, magnetostrictive force, electromagnetic force, and lift-off distance on the Lamb wave generated by high-energy pulse EMAT were simulated.
Figure 2a is the schematic diagram of the high-energy pulse EMAT excitation acoustic wave, where
Bdx and
Bdy are the horizontal and vertical components of the dynamic magnetic field, and
Fdx and
Fdy are the horizontal and vertical components of the electromagnetic force. In the low-frequency band, S mode particle vibration is mainly in-plane displacement, whereas a mode vibration is mainly out-of-plane displacement. The finite element simulation model of high-energy pulse EMAT is shown in
Figure 2b. The steel plate size is 300 mm × 1 mm, the starting coordinate of the left end of the excitation coil is (−63, 0.5), the point
P to be measured is (107, −0.01), and the distance between the sending and receiving is 170 mm. In the high-energy pulse excitation coil, the current is obtained by the Equation (8), which is shown in
Figure 3a. The spectrum analysis of the current is shown in
Figure 3b. The coil line spacing should be designed as the line spacing corresponding to the frequency of about 220 kHz. The model parameters are shown in
Table 1.
3.1. Characteristic of Magnetic Flux Density Distribution in High-Energy Pulse Excitation
In the condition that the lift-off distance h is 0 mm, the magnetic flux density distribution of the high-energy pulse EMAT in different directions was simulated and analyzed.
Figure 4a,b are the magnetic flux density distribution of the high-energy pulse EMAT in different directions (
Bx is the horizontal component of magnetic flux density and
By is the vertical component of magnetic flux density) of the steel plate surface. From
Figure 4a, when the measured point is exactly below the axis of the excitation coil wire (such as
x = 30.5), the amplitude corresponding to the horizontal component
Bx of magnetic flux density is the highest. When the measured point is exactly under the middle of the two adjacent wires of the meander coil (such as
x = 31.425), the value
Bx is 0. From
Figure 4b, the distribution of
By is exactly the opposite to the distribution of
Bx. In
Figure 4b, the measured point exactly below the excitation coil wire axis (for example,
x = 30.5) corresponds to 0. Whereas, in the position exactly below the middle of the two adjacent wires of the meander coil (for example,
x = 31.425), the amplitude is the highest. Based on the comparison between
Figure 4a,b, it can be seen that the magnetic flux density in the horizontal direction is larger than that in the vertical direction by an order of magnitude. In other words, the contribution of the magnetic flux density is mainly dominated by the horizontal component of magnetic flux density. According to the aforementioned, in ferromagnetic materials, only when the direction of the magnetic field is horizontal, the magnetostrictive force may become the main mechanism of action. It is critical to determine when the Lorentz force and magnetostrictive force is the main mechanism of transduction.
3.2. Influence of Lift-Off Distance on High-Energy Pulse EMAT Transduction Mechanism
The simulations of the high energy pulse EMAT, and the conventional EMAT, were used for comparison to show the difference in amplitude and the two simulations used the same simulation settings. In the conventional EMAT simulation model, the residual magnetic flux density of the magnet is set to 1.2 T and the lift-off distance is 0 mm. At the distance
h = 0 mm, the Lamb signals of the high-energy pulse EMAT and the conventional EMAT at point
P is shown in
Figure 5. The group velocity of S0 mode is faster than that of A0 mode, and S0 mode reaches
P point first. The vibration amplitude of A0 mode is much larger than that of S0 mode. The time-domain signal amplitude of A0 mode in high-energy pulse EMAT is 3.2 times that of conventional EMAT. The S0 mode signal amplitude of the high-energy pulse EAMT is 2.9 times that of the conventional EMAT.
To facilitate the study of the attenuation of the particle displacements of S0 and A0 modes, the particle displacements of the S0 and A0 modes were extracted and analyzed. The change curve of displacement amplitude with lift-off distance is obtained, as shown in
Figure 6.
The ultrasonic amplitude generally decreases with an exponential function as the lift-off distance increases, in non-ferromagnetic materials, such as aluminum plates. However, in ferromagnetic materials such as steel plates, the A0 and S0 modes in the Lamb waves excited by high-energy pulse EMAT do not decay exponentially, in the small lift-off distance. When the lift-off distance
h is more than 2 mm, the S0 and A0 modes decay exponentially with the lift-off distance. So the displacement log ln(
P) of the S0 and A0 modes vary approximately linearly with the lift-off distance
h, as is shown in
Figure 6. Due to the attenuation rate of the Lorentz force
FL determined by
B and
Je is higher than the magnetostrictive force
fM decided only by
B. In this case, the energy conversion mechanism is mainly dominated by the magnetostrictive force
fM in the steel plate. In the steel plate, the high-energy pulsed electromagnetic ultrasonic energy conversion mechanism is composed of the Lorentz force and magnetostrictive force, in the lift-off distance
h less than 2 mm. In this lift-off distance section, the Lamb wave A0 and S0 modes do not decay with an exponential function as the lift-off distance increases.