Lengthening Transmission Distance of Continuous Variable Quantum Key Distribution with Discrete Modulation through Photon Catalyzing
Abstract
:1. Introduction
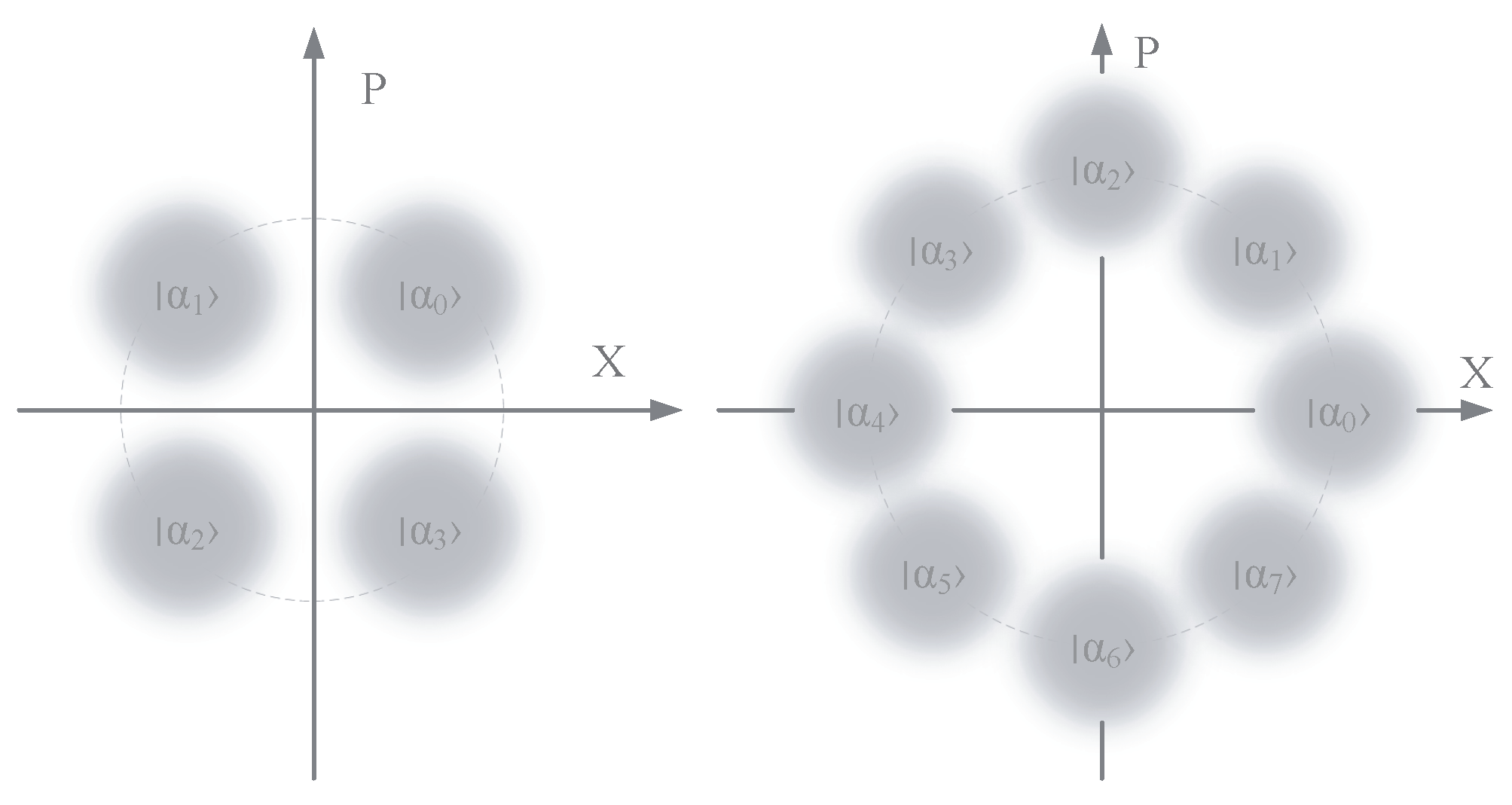
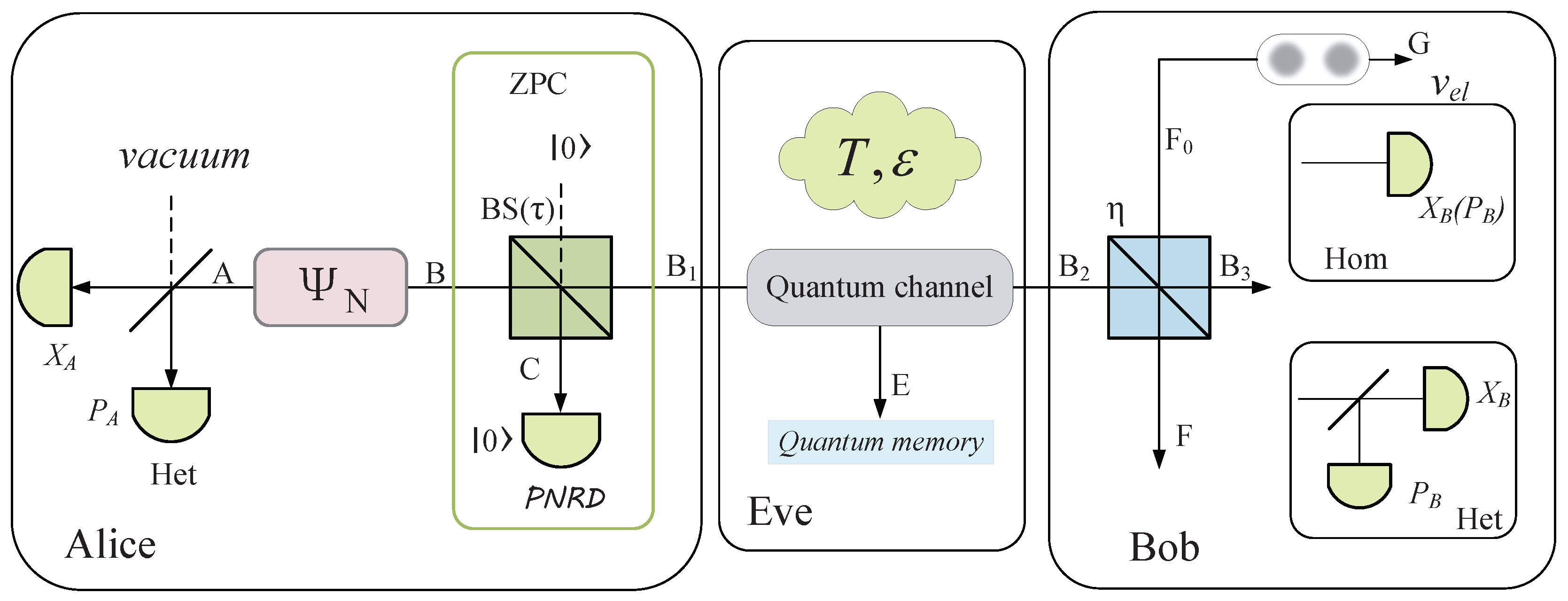
2. The DM Source Preparation via ZPC Operations
2.1. The CVQKD System with the DM Source Preparation
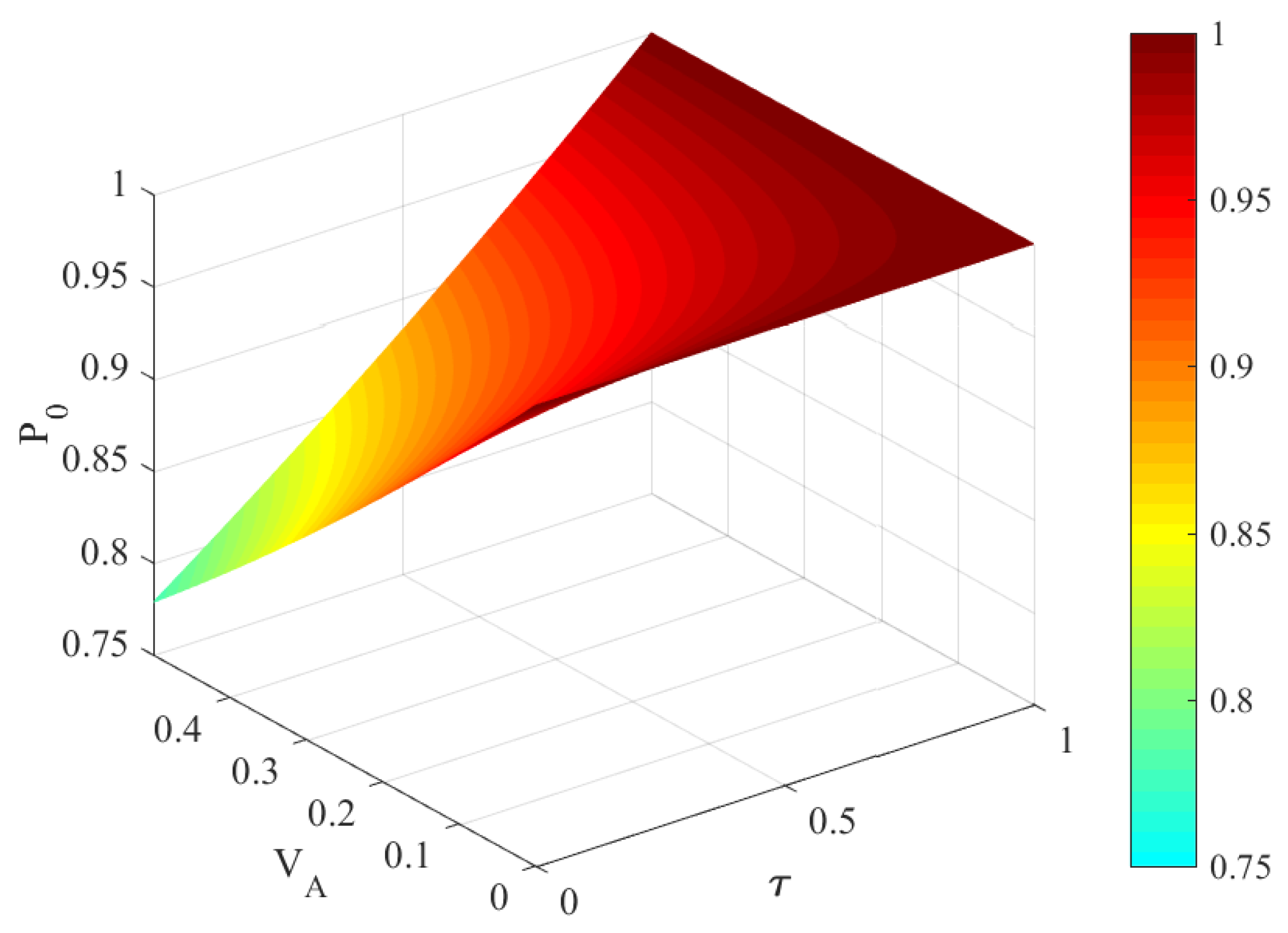
2.2. The ZPC-Based DM Source Preparation
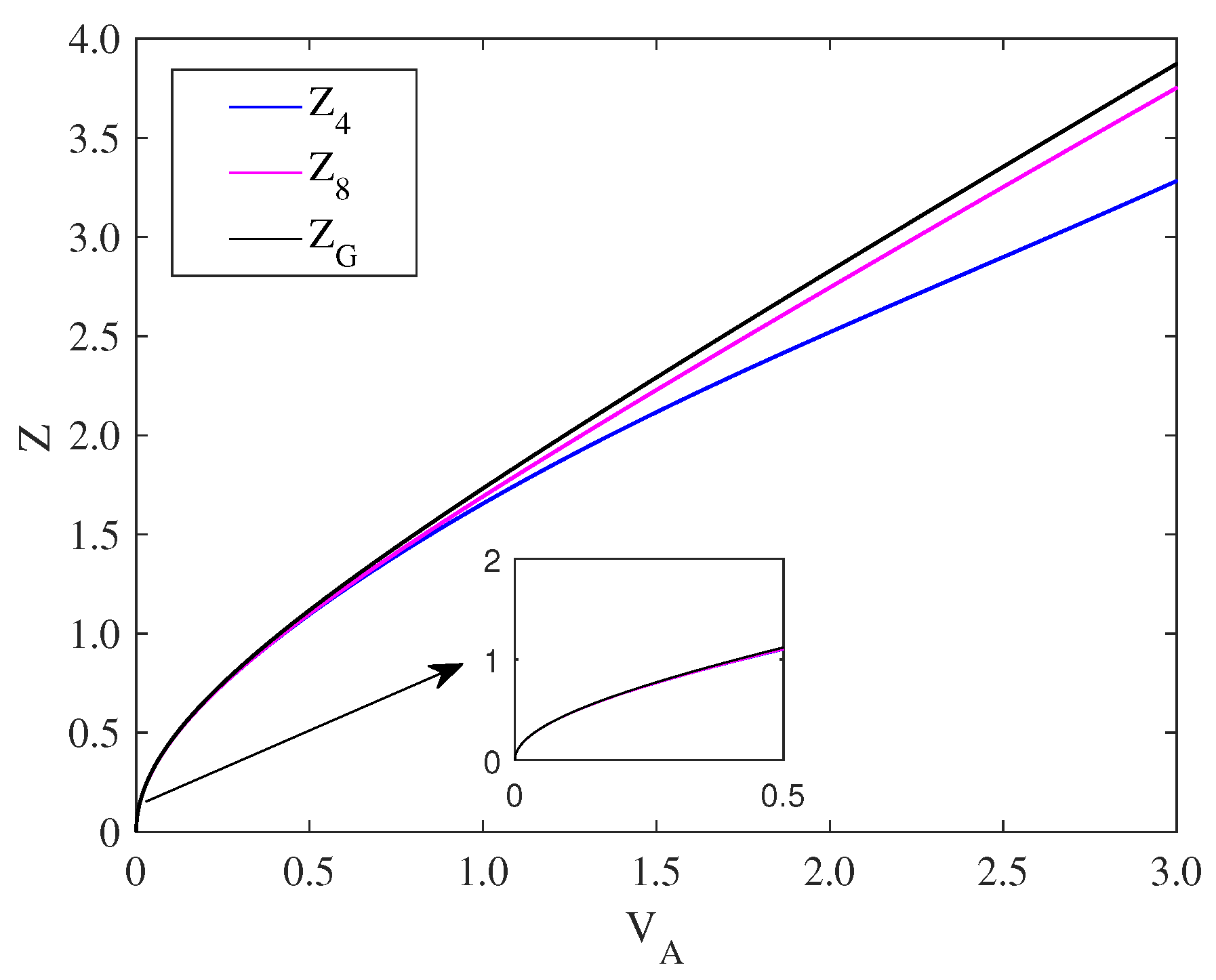
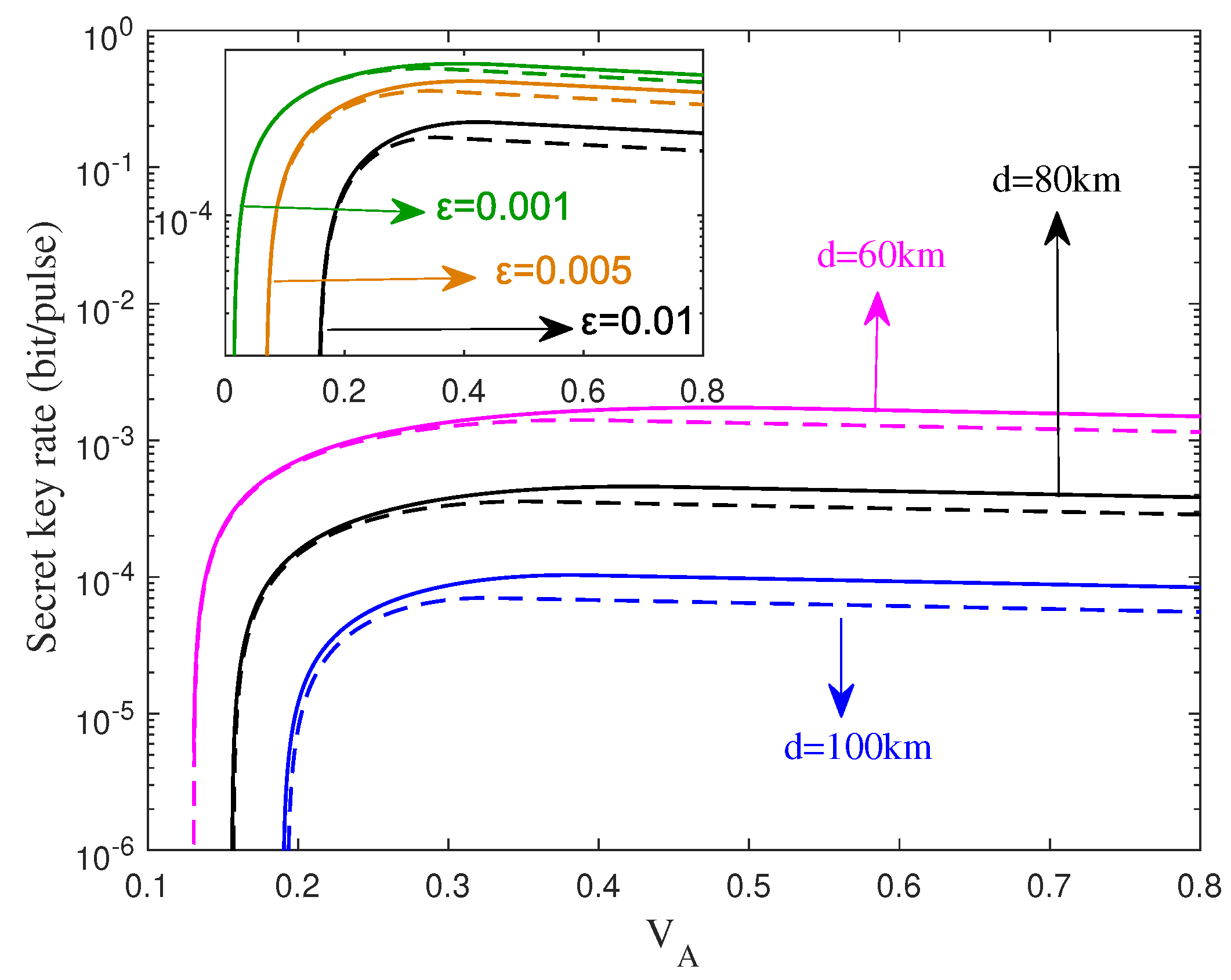
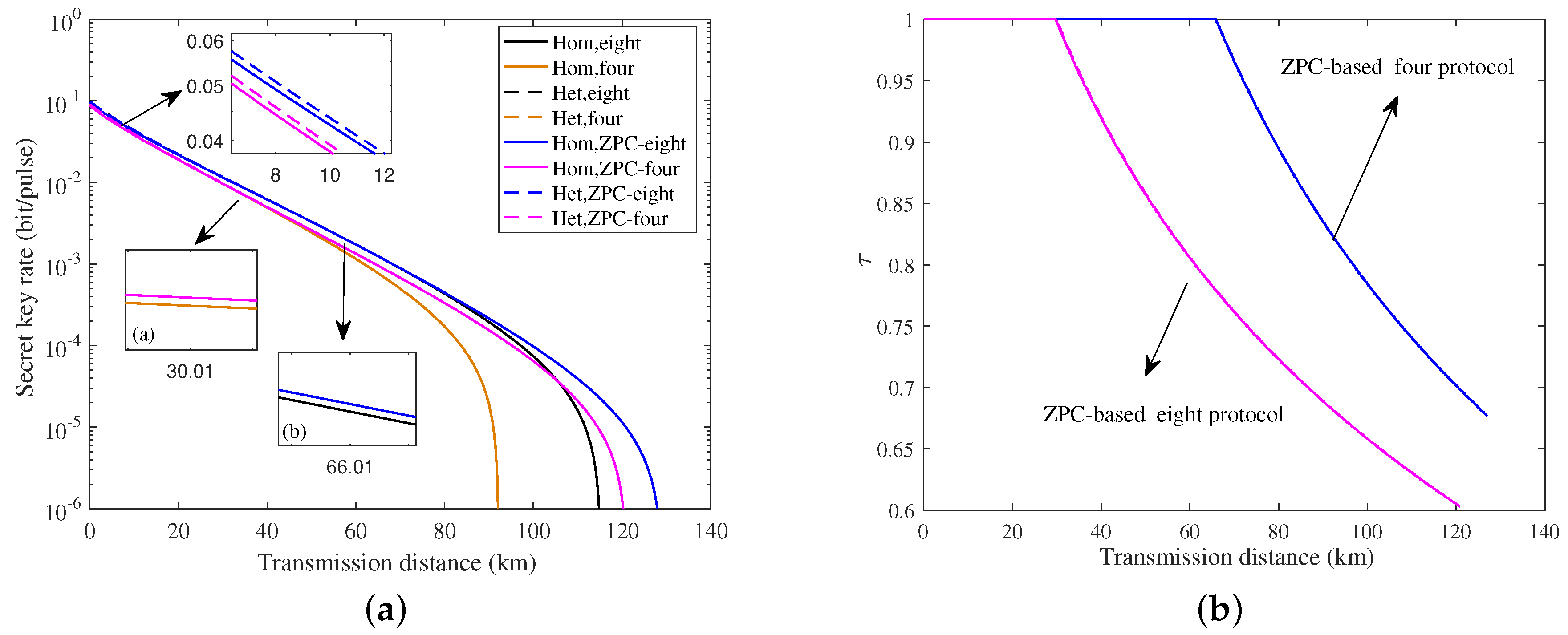
3. Security Analysis
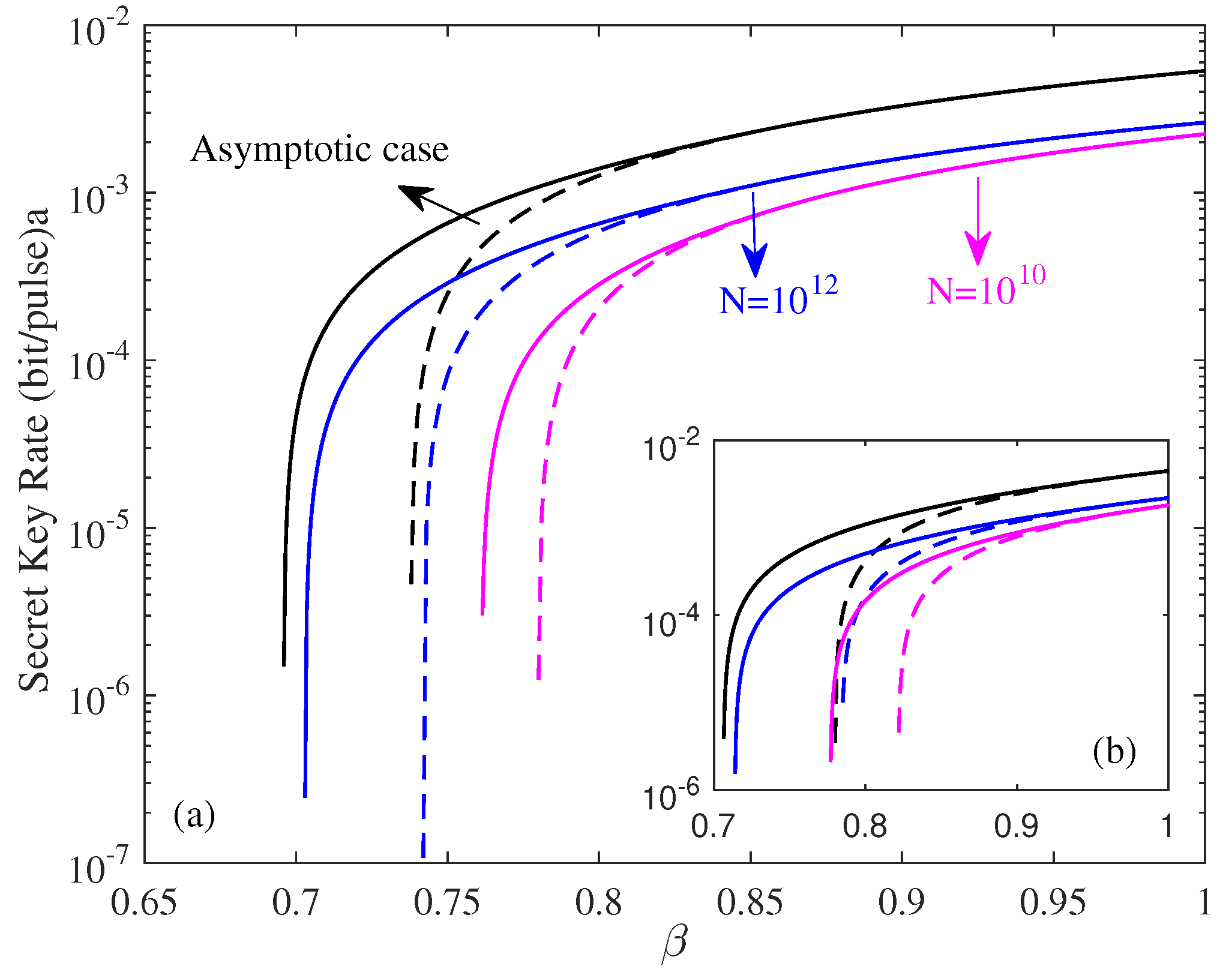
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A. Calculation of Asymptotic Secret Key Rate
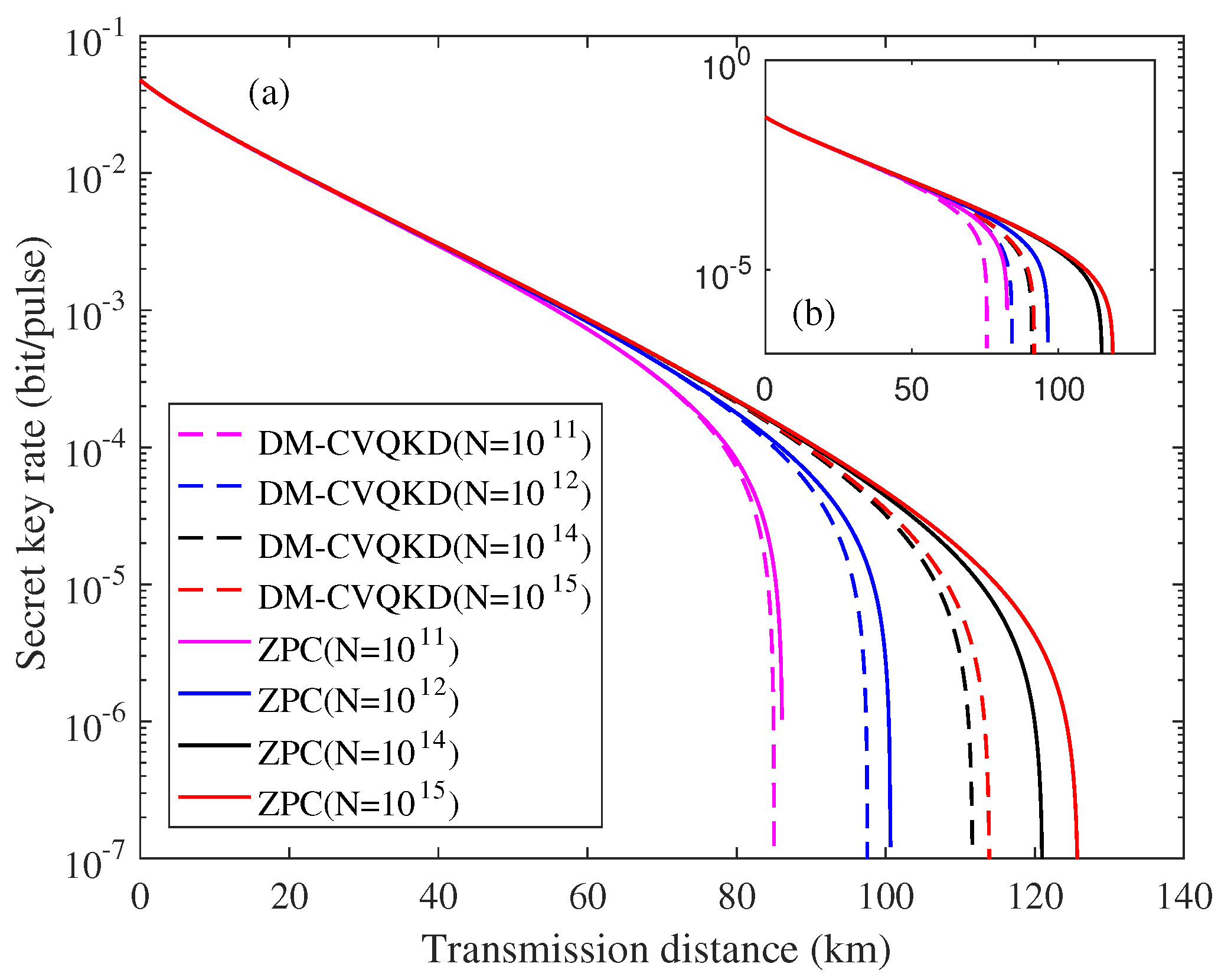
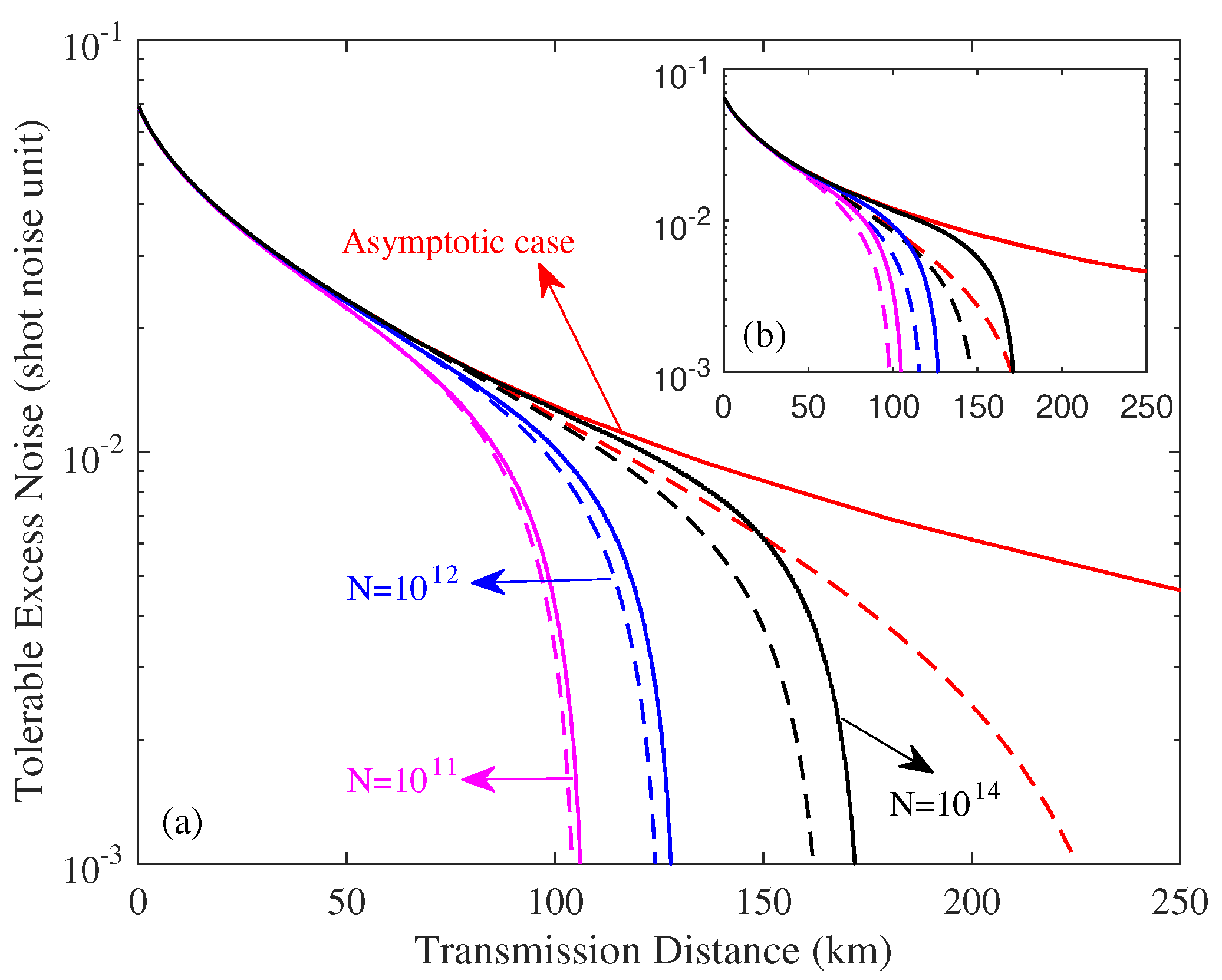
Appendix B. Secret Key Rate in the Finite-Size Scenario
References
- Gisin, N.; Ribordy, G.; Tittel, W.; Zbinden, H. Quantum cryptography. Rev. Mod. Phys. 2002, 74, 145. [Google Scholar] [CrossRef] [Green Version]
- Zhou, J.; Huang, D.; Guo, Y. Long-distance continuous-variable quantum key distribution using separable Gaussian states. Phys. Rev. A 2018, 98, 042303. [Google Scholar] [CrossRef] [Green Version]
- Guo, Y.; Xie, C.; Huang, P.; Li, J.; Zhang, L.; Huang, D.; Zeng, G. Channel-parameter estimation for satellite-to-submarine continuous-variable quantum key distribution. Phys. Rev. A 2018, 97, 052326. [Google Scholar] [CrossRef]
- Liao, Q.; Wang, Y.; Huang, D.; Guo, Y. Dual-phase-modulated plug-and-play measurement-device-independent continuous-variable quantum key distribution. Opt. Express 2018, 26, 19907–19920. [Google Scholar] [CrossRef] [Green Version]
- Lo, H.K.; Curty, M.; Tamaki, K. Secure quantum key distribution. Nat. Photon 2014, 8, 595. [Google Scholar] [CrossRef] [Green Version]
- Wootters, W.K.; Zurek, W.H. A single quantum cannot be cloned. Nature 1982, 299, 802. [Google Scholar] [CrossRef]
- Bang, J.Y.; Berger, M.S. Quantum mechanics and the generalized uncertainty principle. Phys. Rev. D 2006, 74, 125012. [Google Scholar] [CrossRef] [Green Version]
- Shor, P.W.; Preskill, J. Simple proof of security of the BB84 quantum key distribution protocol. Phys. Rev. Lett. 2000, 85, 441. [Google Scholar] [CrossRef] [Green Version]
- Lo, H.K.; Curty, M.; Qi, B. Measurement-device-independent quantum key distribution. Phys. Rev. Lett. 2012, 108, 130503. [Google Scholar] [CrossRef] [Green Version]
- Soh, D.B.S.; Brif, C.; Coles, P.J.; Lutkenhaus, N.; Camacho, R.M.; Urayama, J.; Sarovar, M. Self-referenced continuous-variable quantum key distribution protocol. Phys. Rev. X 2015, 5, 041010. [Google Scholar] [CrossRef] [Green Version]
- Grosshans, F.; Grangier, P. Continuous variable quantum cryptography using coherent states. Phys. Rev. Lett. 2002, 88, 057902. [Google Scholar] [CrossRef] [Green Version]
- Grosshans, F.; van Assche, G.; Wenger, J.; Brouri, R.; Cerf, N.J.; Grangier, P. Quantum key distribution using gaussian-modulated coherent states. Nature 2003, 421, 238. [Google Scholar] [CrossRef] [Green Version]
- Liao, Q.; Guo, Y.; Huang, D.; Huang, P.; Zeng, G. Long-distance continuous-variable quantum key distribution using non-Gaussian state-discrimination detection. New. J. Phys. 2018, 023015. [Google Scholar] [CrossRef]
- Braunstein, S.L.; van Loock, P. Quantum information with continuous variables. Rev. Mod. Phys. 2005, 77, 513. [Google Scholar] [CrossRef] [Green Version]
- Ma, X.C.; Sun, S.H.; Jiang, M.S.; Gui, M.; Liang, L.M. Gaussian-modulated coherent-state measurement-device-independent quantum key distribution. Phys. Rev. A 2014, 89, 042335. [Google Scholar] [CrossRef] [Green Version]
- Leverrier, A.; Grangier, P. Unconditional security proof of long-distance continuous-variable quantum key distribution with discrete modulation. Phys. Rev. Lett. 2009, 102, 180504. [Google Scholar] [CrossRef] [Green Version]
- Takeoka, M.; Guha, S.; Wilde, M.M. Fundamental rate-loss tradeoff for optical quantum key distribution. Nat. Commun. 2014, 5, 5235. [Google Scholar] [CrossRef]
- Furrer, F.; Franz, T.; Berta, M.; Leverrier, A.; Scholz, V.B.; Tomamichel, M.; Werner, R.F. Continuous variable quantum key distribution: Finite-key analysis of composable security against coherent attacks. Phys. Rev. Lett. 2012, 109, 100502. [Google Scholar] [CrossRef]
- Leverrier, A.; Grangier, P. Continuous-variable quantum-key-distribution protocols with a non-Gaussian modulation. Phys. Rev. A 2011, 83, 042312. [Google Scholar] [CrossRef] [Green Version]
- Mao, Y.; Wang, Y.; Huang, W.; Zhong, H.; Qin, H.; Guo, Y.; Huang, D. Detecting quantum attacks: A machine learning based defense strategy for practical continuous-variable quantum key distribution. New J. Phys. 2020, 22, 083073. [Google Scholar] [CrossRef]
- Guo, Y.; Ye, W.; Zhong, H.; Liao, Q. Continuous-variable quantum key distribution with non-Gaussian quantum catalysis. Phys. Rev. A 2019, 99, 032327. [Google Scholar] [CrossRef] [Green Version]
- Ye, W.; Zhong, H.; Liao, Q.; Huang, D.; Hu, L.; Guo, Y. Improvement of self-referenced continuous-variable quantum key distribution with quantum photon catalysis. Opt. Express 2019, 27, 17186–17198. [Google Scholar] [CrossRef]
- Xu, X. Enhancing quantum entanglement and quantum teleportation for two-mode squeezed vacuum state by local quantum-optical catalysis. Phys. Rev. A 2015, 92, 012318. [Google Scholar] [CrossRef] [Green Version]
- Guo, Y.; Ding, J.Z.; Mao, Y.; Ye, W.; Liao, Q.; Huang, D. Quantum catalysis-based discrete modulation continuous variable quantum key distribution with eight states. Phys. Lett. A 2020, 384, 126340. [Google Scholar] [CrossRef]
- Ye, W.; Guo, Y.; Xia, Y.; Zbinden, H.; Zhang, H.; Ding, J.Z.; Hu, L.Y. Discrete modulation continuous-variable quantum key distribution based on quantum catalysis. Acta Phys. Sinica 2020, 69, 060301. [Google Scholar]
- Leverrier, A.; Grosshans, F.; Grangier, P. Finite-size analysis of a continuous-variable quantum key distribution. Phys. Rev. A 2010, 81, 062343. [Google Scholar] [CrossRef] [Green Version]
- Guo, Y.; Li, R.J.; Liao, Q.; Zhou, J.; Huang, D. Performance improvement of eight-state continuous-variable quantum key distribution with an optical amplifier. Phys. Lett. A 2018, 382, 372–381. [Google Scholar] [CrossRef]
- Mao, Y.; Wang, Y.; Huang, W.; Qin, H.; Huang, D.; Guo, Y. Hidden-Markov-model-based calibration-attack recognition for continuous-variable quantum key distribution. Phys. Rev. A 2020, 101, 062320. [Google Scholar] [CrossRef]
- Wang, Y.; Mao, Y.; Huang, W.; Huang, D.; Guo, Y. Optical frequency comb-based multichannel parallel continuous-variable quantum key distribution. Opt. Express 2019, 27, 25314–25329. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.; Zhang, Y.; Wang, X.; Xu, B.; Peng, X.; Guo, H. Non-Gaussian postselection and virtual photon subtraction in continuous-variable quantum key distribution. Phys. Rev. A 2016, 93, 012310. [Google Scholar] [CrossRef] [Green Version]
- He, Y.Q.; Mao, Y.; Zhong, H.; Huang, D.; Guo, Y. Hybrid linear amplifier-involved detection for continuous variable quantum key distribution with thermal states. Chin. Phys. B 2020, 29, 050309. [Google Scholar] [CrossRef]
- Guo, Y.; Liao, Q.; Wang, Y.; Huang, D.; Huang, P.; Zeng, G. Performance improvement of continuous-variable quantum key distribution with an entangled source in the middle via photon subtraction. Phys. Rev. A 2017, 95, 032304. [Google Scholar] [CrossRef] [Green Version]
- Wu, X.; Wang, Y.; Zhong, H.; Ye, W.; Huang, D.; Guo, Y. Continuous-variable measurement-device-independent quantum key distribution via quantum catalysis. Quantum Infor. Process. 2020, 19, 234. [Google Scholar] [CrossRef]
- Ryu, J.; Lim, J.; Lee, C.; Lee, J. Nonlocality for continuous variable system with local symplectic operation. J. Mod. Opt. 2010, 57, 1550–1554. [Google Scholar] [CrossRef]
- Lee, S.-Y.; Ji, S.-W.; Kim, H.-J.; Nha, H. Enhancing quantum entanglement for continuous variables by a coherent superposition of photon subtraction and addition. Phys. Rev. A 2011, 84, 012302. [Google Scholar] [CrossRef] [Green Version]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Z.; Zou, S.; Huang, T.; Guo, Y. Lengthening Transmission Distance of Continuous Variable Quantum Key Distribution with Discrete Modulation through Photon Catalyzing. Appl. Sci. 2020, 10, 7770. https://doi.org/10.3390/app10217770
Zhou Z, Zou S, Huang T, Guo Y. Lengthening Transmission Distance of Continuous Variable Quantum Key Distribution with Discrete Modulation through Photon Catalyzing. Applied Sciences. 2020; 10(21):7770. https://doi.org/10.3390/app10217770
Chicago/Turabian StyleZhou, Zhengchun, Shanhua Zou, Tongcheng Huang, and Ying Guo. 2020. "Lengthening Transmission Distance of Continuous Variable Quantum Key Distribution with Discrete Modulation through Photon Catalyzing" Applied Sciences 10, no. 21: 7770. https://doi.org/10.3390/app10217770
APA StyleZhou, Z., Zou, S., Huang, T., & Guo, Y. (2020). Lengthening Transmission Distance of Continuous Variable Quantum Key Distribution with Discrete Modulation through Photon Catalyzing. Applied Sciences, 10(21), 7770. https://doi.org/10.3390/app10217770




