Refined Seismic Design Method for RC Frame Structures to Increase the Collapse Resistant Capacity
Abstract
:1. Introduction
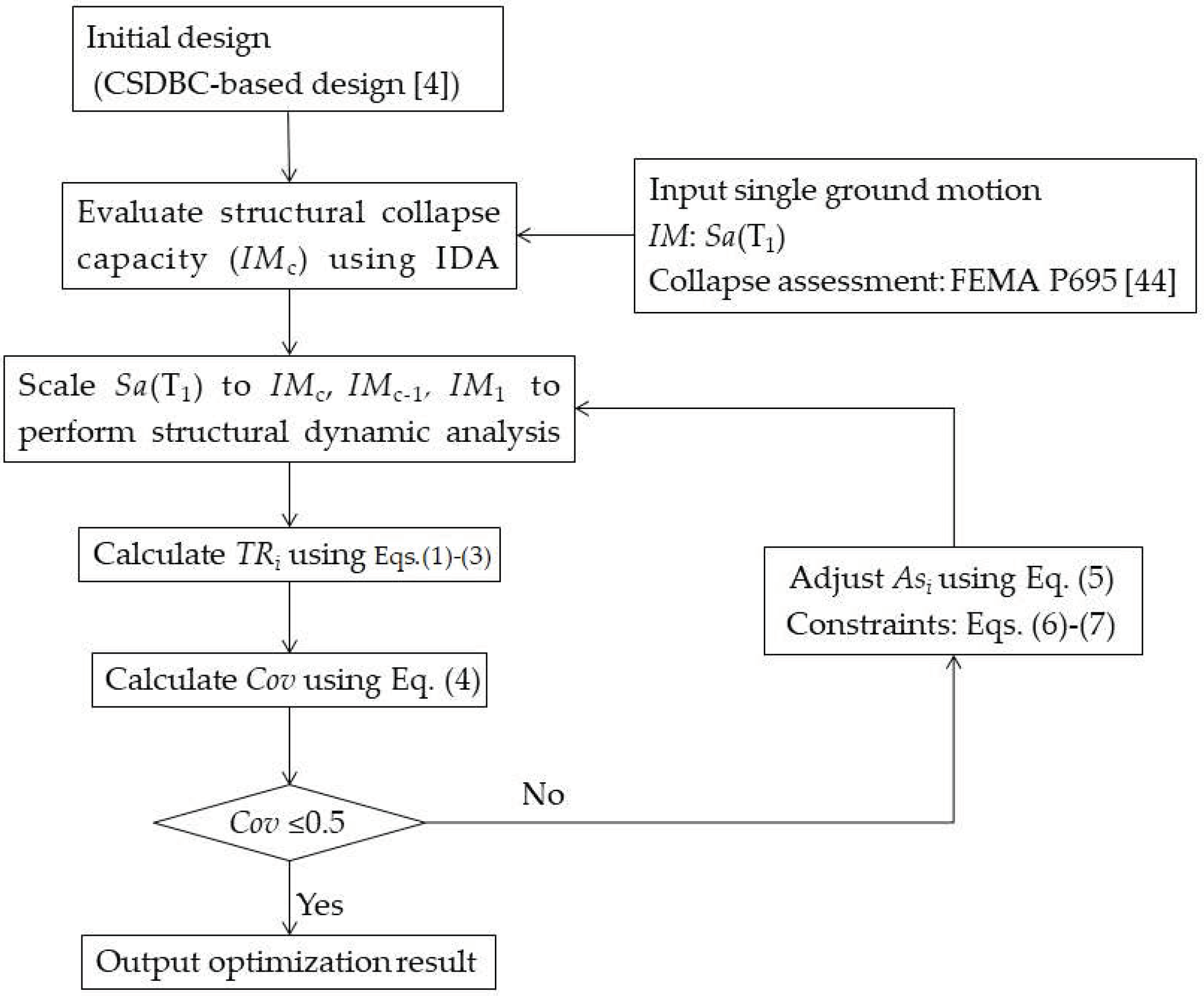
2. Refined Seismic Design Methodology
- (1)
- Design a structure according to the current design code, such as CSDBC [4].
- (2)
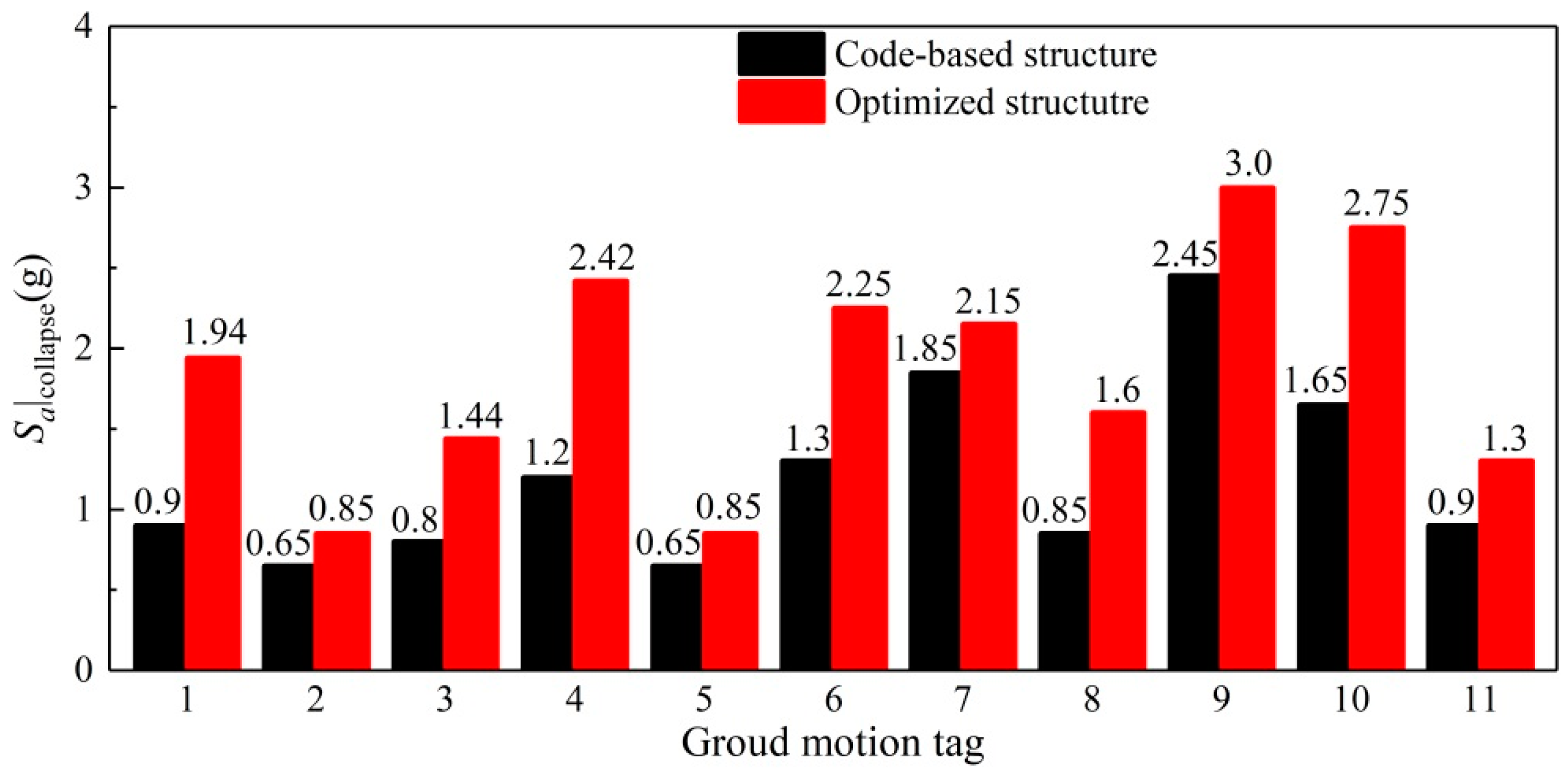
- Establish the numerical model of the designed building, and perform IDA to determine the collapse capacity under the selected earthquake ground motion. Then, the intensity of the earthquake ground motion relating to the state of structural collapse damage can be obtained and recorded as .
- (3)
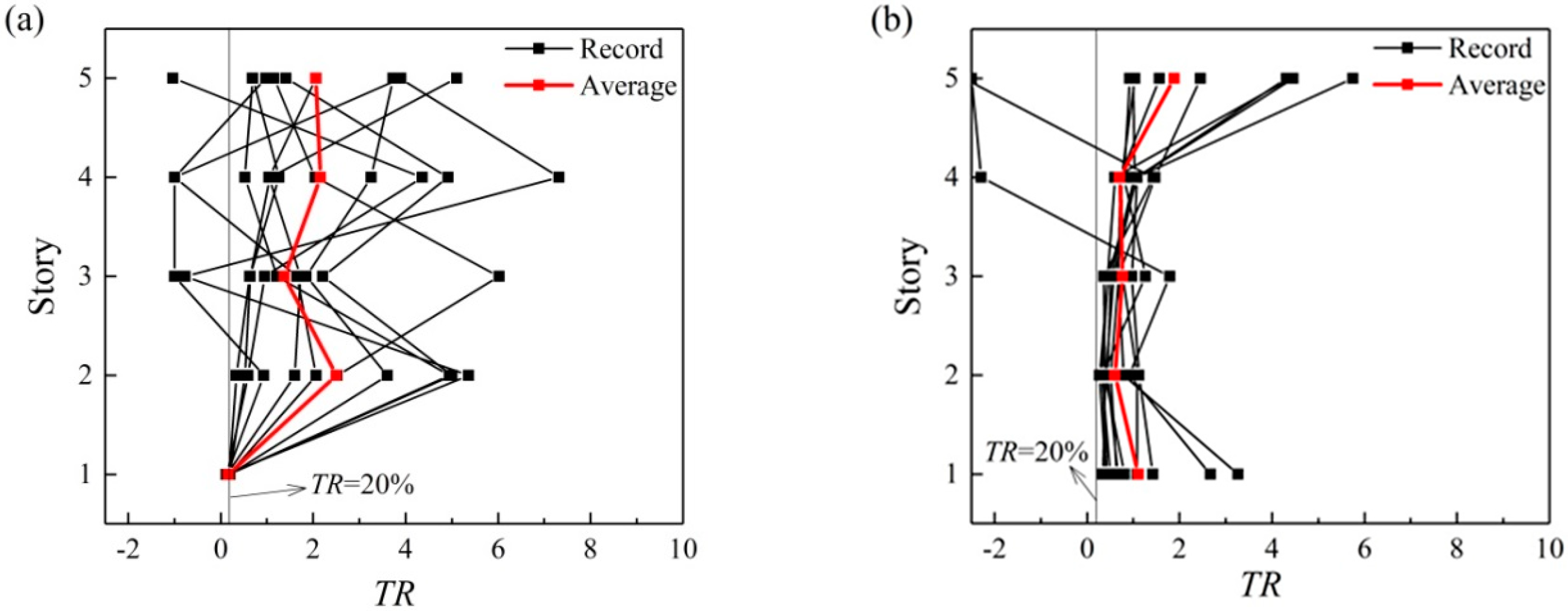
- Scale the intensity of selected ground motion to , , and , and perform the dynamic analysis to derive the seismic demand of the structure. Calculate the TR of each floor using the Equations (1)–(3).
- (4)
- Based on the calculation results of TR, calculate the coefficient of variation of TR based on the Equation (4).where Cov is the coefficient of variation of ; n is the number of the structure floor; and is the average value of .
- (5)
- If the value of Cov reduces to a pre-determined target value, that means the code-based structure has a relatively uniform TR distribution along the height of the building while the structure collapses, and no redesign is needed; otherwise, an iterative procedure is proceeded, where the longitudinal steel reinforcement of columns is sequentially modified using Equations (5) and (6) until the value of Cov reduces to small enough (e.g., less than 0.5).where and are the dimension of the longitudinal steel reinforcement for one column on the ith floor at the jth and (j − 1)th iteration, respectively; is the TR of ith floor at (j − 1)th iteration; is the average of TR of each floor at (j − 1)th iteration; and α in Equation (5), which controls the convergence gradient, is a coefficient ranging from 0 to 1. Based on the previous studies [22,23,24,25,26,27,28,29], α is set as 0.02 for this study. In order to keep the same construction cost, the Equation (6) is utilized to adjust the sum of longitudinal steel reinforcement of candidate design to that of code-based structure. is the sum of ; is the sum of longitudinal steel reinforcement for a code-based structure; is the updated dimension after scaling the optimized results to the sum of longitudinal steel reinforcement for a code-based structure.
3. Simulation Case
3.1. Simulation Structure
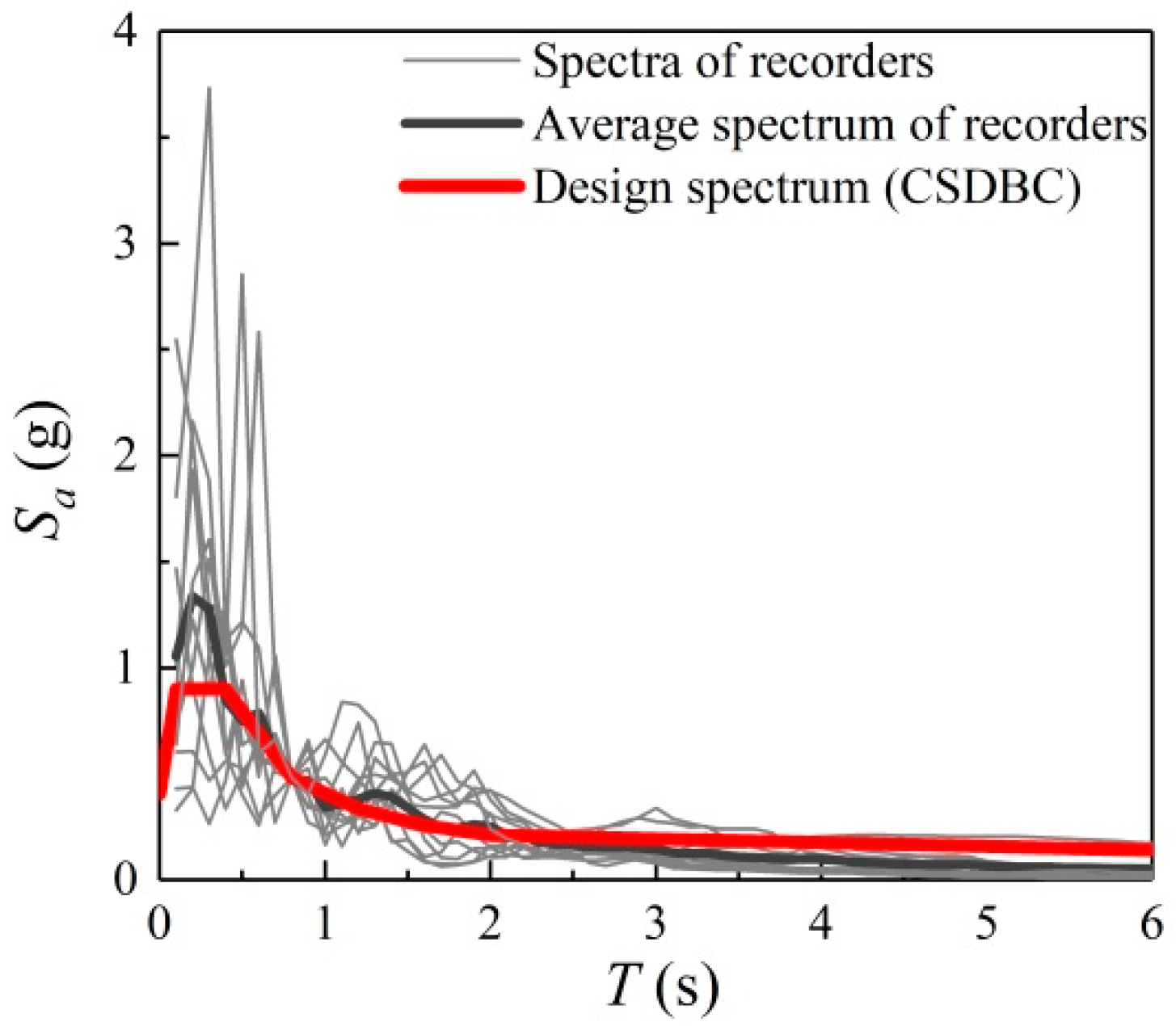
3.2. Selected Earthquake Ground Motions
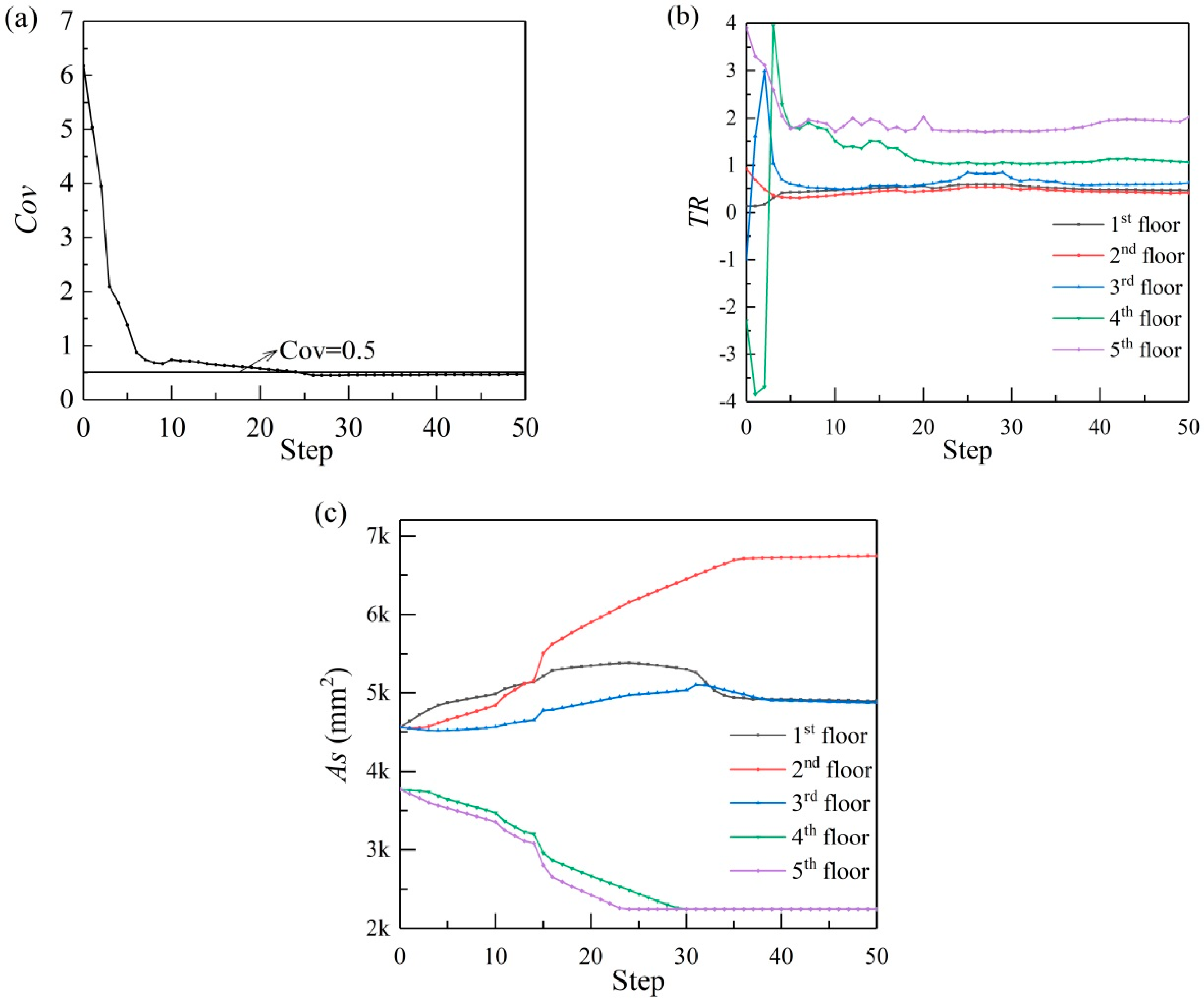
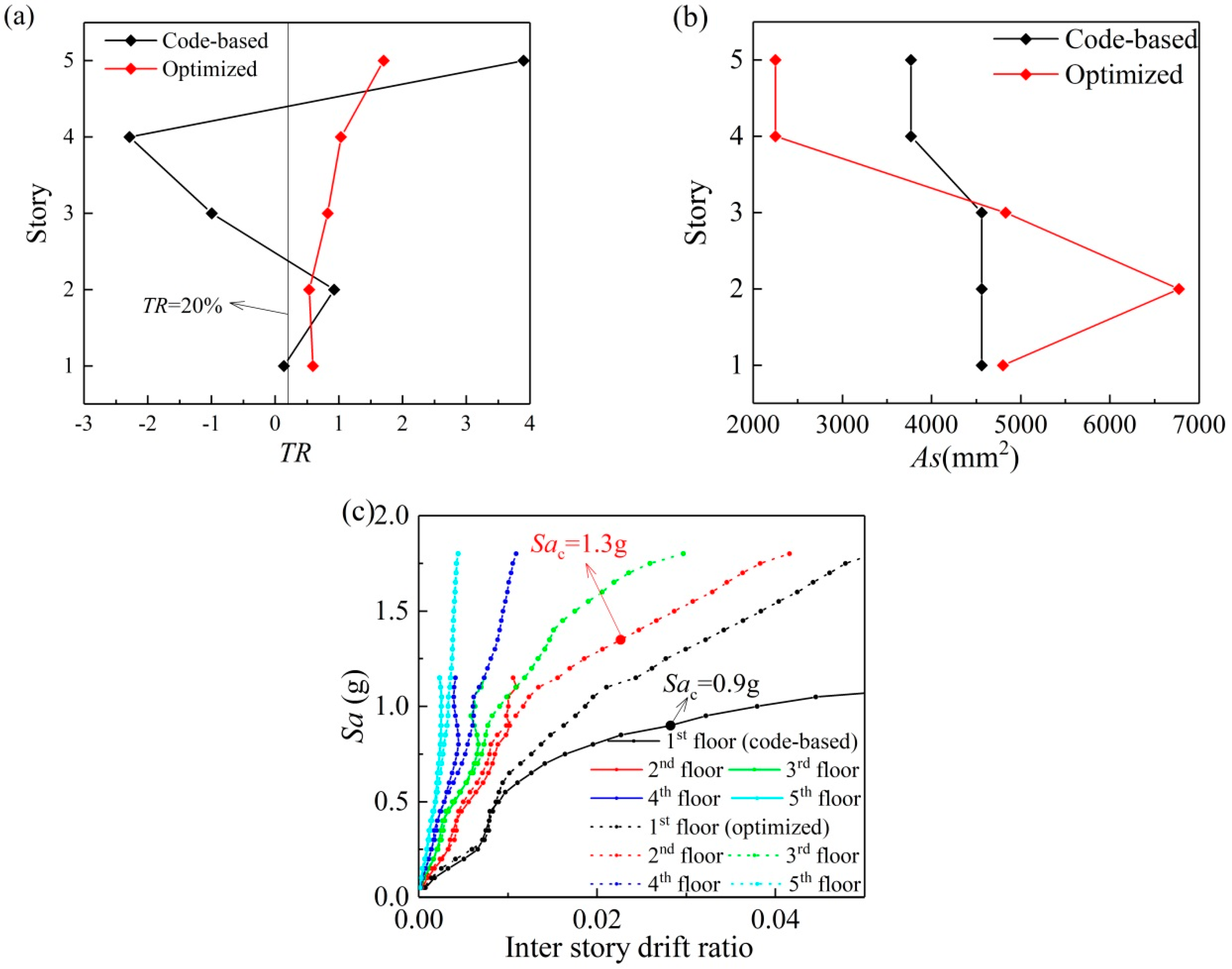
4. Optimum Design for a Single Earthquake Ground Motion
5. Optimum Design for the Selected Earthquake Ground Motion Set
- (1)
- Try to make the corner steel bars have the same diameter with the code-designed structure;
- (2)
- Try to use the corner steel bars with a bigger diameter than the middle bars;
- (3)
- Try to use steel bars with the same diameter at the same position of the column on successive floors.
6. Conclusions
- Since the longitudinal reinforcement of columns is adopted as the optimization variable, the optimization procedure just needs a few iterative steps to achieve the optimization target. In addition, the constrained provisions (e.g., the maximum and minimum reinforcement ratio of the columns, etc.) in code are also taken into account during the iterative loops. It is a practical and computationally efficient method and easily implemented in engineering practice.
- After optimization, the maximum inter-story response is reduced while the structure suffers inelastic deformation. The reduction level is increased with the nonlinear state developing.
- Compared to the code-based structure, with the same construction cost, the collapse resistant capacity of the optimized structure is remarkably increased (on average up to 60.9%); and the TR distributes more uniformly under the earthquake intensity of the initial structure collapse state.
- Though the RC frame is analyzed in this study, the other type of structure can be optimized by a similar methodology. Similarly, the proposed optimization methodology also can be extended to strengthen and retrofit frame structures.
Author Contributions
Funding
Conflicts of Interest
References
- Eurocode 8. Design of Structures for Earthquake Resistance. Part 1: General Rules, Seismic Action and Rules for Buildings; European Committee for Standardization: Brussels, Belgium, 2004.
- ASCE Standard ASCE/SEI 7-10. Minimum Design Loads for Buildings and Other Structures; American Society of Civil Engineers (ASCE): Reston, VA, USA, 2010.
- International Code Council, Inc. (Ed.) IBC 2015. International Building Code 2015; Country Club Hills: Cook County, IL, USA, 2015. [Google Scholar]
- Ministry of Housing and Urban-Rural Development of P. R. China (Ed.) Code for Seismic Design of Buildings (GB 50010-2010); China Architecture and Building Press: Beijing, China, 2016. [Google Scholar]
- Ou, J.-P.; Li, H. The regional engineering damage and reconstruction strategy in Wenchuan earthquake of China. J. Earthq. Tsunami 2011, 5, 189–216. [Google Scholar] [CrossRef]
- Lu, X.-Z.; Lin, X.-C.; Tian, Y.; Ye, L.-P. Comparison and discussion on destructive power of the ground motions recorded in the hardest-hit regions of Wenchuan, Lushan and Ludian earthquakes. Eng. Mech. 2014, 31, 1–7. [Google Scholar]
- Liel, A.B.; Haselton, C.B.; Deierlein, G.G. Seismic collapse safety of reinforced concrete buildings. II: Comparative assessment of nonductile and ductile moment frames. J. Struct. Eng. 2011, 137, 492–502. [Google Scholar] [CrossRef]
- Haselton, C.B.; Liel, A.B.; Deierlein, G.G.; Dean, B.S.; Chou, J.-H. Seismic collapse safety of reinforced concrete buildings. I: Assessment of ductile moment frames. J. Struct. Eng. 2011, 137, 481–491. [Google Scholar] [CrossRef] [Green Version]
- Lignos, D.-G.; Hikino, T.; Matsuoka, Y.; Nakashima, M. Collapse assessment of steel moment frames based on E-Defense full-scale table collapse tests. J. Struct. Eng. 2013, 139, 120–132. [Google Scholar] [CrossRef]
- Lu, Z.; Chen, X.-Y.; Lu, X.-L.; Yang, Z. Shaking table test and numerical simulation of an RC frame-core tube structure for earthquake-induced collapse. Earthq. Eng. Struct. Dyn. 2016, 45, 1537–1556. [Google Scholar] [CrossRef]
- Febf, D.C.; Kolay, C.; Ricles, J.M.; Li, J. Collapse simulation of reinforced concrete frame structures. Struct. Des. Tall Spec. Build. 2016, 25, 578–601. [Google Scholar]
- Ganzerli, S.; Pantelides, C.P. Performance-based design using structural optimization. Earthq. Eng. Struct. Dyn. 2000, 29, 1677–1690. [Google Scholar] [CrossRef]
- Chan, C.-M.; Zou, X.-K. Elastic and inelastic drift performance optimization for reinforced concrete buildings under earthquake loads. Earthq. Eng. Struct. Dyn. 2004, 33, 929–950. [Google Scholar] [CrossRef]
- Zou, X.-K.; Chan, C.-M. Optimum seismic performance-based design of reinforced concrete buildings using nonlinear pushover analysis. Eng. Struct. 2005, 27, 1289–1302. [Google Scholar] [CrossRef]
- Al-Ansari, M.; Senouci, A. Drift optimization of high-rise buildings in earthquake zones. Struct. Des. Tall Spec. Build. 2011, 20, 208–222. [Google Scholar] [CrossRef]
- Bai, J.-L.; Ou, J.-P. Seismic failure mode improvement of RC frame structure based on multiple lateral load patterns of pushover analyses. Sci. China Technol. Sci. 2011, 54, 2825–2833. [Google Scholar] [CrossRef]
- Krawinkler, H. Pushover analysis: Why, how, when, and when not to use it. In Proceedings of the 1996 SEAOC Convention, Maui, HI, USA, 1–6 October 1996. [Google Scholar]
- Li, S.; Zuo, Z.-X.; Zhai, C.-H.; Xie, L.-L. Comparison of static pushover and dynamic analyses using RC building shaking table experiment. Eng. Struct. 2017, 136, 430–440. [Google Scholar] [CrossRef]
- Aldwaik, M.; Adeli, H. Advances in optimization of highrise building structures. Struct. Multidiplinary Optim. 2014, 50, 899–919. [Google Scholar] [CrossRef]
- Lee, S.-S.; Goel, S.-G. Performance-based of steel moment frames using target drift and yield mechanism. In Proceedings of the U.S.-Japan Seminar on Advanced Stability and Seismicity Concept for Performance Based Design of Steel and Composite Structures, Kyoto, Japan, 23–26 July 2001. [Google Scholar]
- Chao, S.-H.; Goel, S.-C. A seismic design lateral force distribution based on inelastic state of structures. Eng. Spectra 2007, 23, 547–569. [Google Scholar] [CrossRef] [Green Version]
- Mohammadi, R.K.; Naggar, M.-H.-E.; Moghaddam, H. Optimum strength distribution for seismic resistant shear buildings. Int. J. Solids Struct. 2004, 41, 6597–6612. [Google Scholar] [CrossRef]
- Moghaddam, H.; Hajirasouliha, I.; Doostan, A. Optimum seismic design of concentrically braced steel frames: Concepts and design procedures. J. Constr. Steel Res. 2005, 61, 151–166. [Google Scholar] [CrossRef]
- Moghaddam, H.; Mohammadi, K. More efficient seismic loading for multidegrees of freedom structures. J. Struct. Eng. 2006, 132, 1673–1677. [Google Scholar] [CrossRef]
- Moghaddam, H.; Hajirasouliha, I. Toward more rational criteria for determination of design earthquake forces. Int. J. Solids Struct. 2006, 43, 2631–2645. [Google Scholar] [CrossRef] [Green Version]
- Moghaddam, H.; Hajirasouliha, I. Optimum strength distribution for seismic design of tall buildings. Struct. Des. Tall Spec. Build. 2008, 17, 331–349. [Google Scholar] [CrossRef]
- Hajirasouliha, I.; Moghaddam, H. New lateral force distribution for seismic design of structures. J. Struct. Eng. 2009, 135, 906–915. [Google Scholar] [CrossRef]
- Hajirasouliha, I.; Asadi, P.; Pilakoutas, K. An efficient performance-based seismic design method for reinforced concrete frames. Earthq. Eng. Struct. Dyn. 2012, 41, 663–679. [Google Scholar] [CrossRef]
- Hajirasouliha, I.; Pilakoutas, K. General seismic load distribution for optimum performance-based design of shear-buildings. J. Earthq. Eng. 2012, 16, 443–462. [Google Scholar] [CrossRef]
- Park, K.; Medina, R.A. Conceptual seismic design of regular frames based on the concept of uniform damage. J. Struct. Eng. 2007, 133, 945–955. [Google Scholar] [CrossRef]
- Ganjavi, B.; Hao, H. Optimum lateral load pattern for seismic design of elastic shear-buildings incorporating soil-structure interaction effects. Earthq. Eng. Struct. Dyn. 2013, 42, 913–933. [Google Scholar] [CrossRef]
- Ganjavi, B.; Hajirasouliha, I.; Bolourchi, A. Optimum lateral load distribution for seismic design of nonlinear shear-buildings considering soil-structure interaction. Soil Dyn. Earthq. Eng. 2016, 88, 356–368. [Google Scholar] [CrossRef]
- Lu, Y.; Hajirasouliha, I.; Marshall, A.M. Performance-based design of flexible-based multi-storey buildings considering soil-structure interaction. Eng. Struct. 2016, 108, 90–103. [Google Scholar] [CrossRef]
- Li, S.; Yu, B.; Gao, M.-M.; Zhai, C.-H. Optimum seismic design of multi-story buildings for increasing collapse resistant capacity. Soil Dyn. Earthq. Eng. 2019, 116, 495–510. [Google Scholar] [CrossRef]
- Mergos, P.E. Efficient optimum seismic design of reinforced concrete frames with nonlinear structural analysis procedures. Struct. Multidiscip. Optim. 2018, 58, 2565–2581. [Google Scholar] [CrossRef] [Green Version]
- Zhang, C.-Y.; Tian, Y. Simplified performance-based optimal seismic design of reinforced concrete frame buildings. Eng. Struct. 2019, 185, 15–25. [Google Scholar] [CrossRef]
- Bai, J.-L.; Jin, S.-S.; Ou, J.-P. An efficient method for optimizing the seismic resistance of reinforced concrete frame structures. Adv. Struct. Eng. 2020, 23, 670–686. [Google Scholar] [CrossRef]
- Mazzolani, F.M.; Della Corte, G.; D’Aniello, M. Experimental analysis of steel dissipative bracing systems for seismic upgrading. J. Civ. Eng. Manag. 2009, 15, 7–19. [Google Scholar] [CrossRef] [Green Version]
- Papalou, A. Strengthening of masonry structures using steel frames. Int. J. Eng. Technol. 2013, 2, 50–56. [Google Scholar] [CrossRef] [Green Version]
- Cecchi, A.; Russo, S.; Sciarretta, F. Preliminary investigation on FRP profiles for the structural retrofit of masonry structures. Key Eng. Mater. 2017, 747, 77–84. [Google Scholar] [CrossRef]
- Casalegno, C.; Russo, S.; Sciarretta, F. Numerical Analysis of a Masonry Panel Reinforced with Pultruded FRP Frames. Mech. Compos. Mater. 2018, 54, 207–220. [Google Scholar] [CrossRef]
- Vamvarsikos, D.; Cornell, C.A. Incremental dynamic analysis. Earthq. Eng. Struct. Dyn. 2002, 31, 491–514. [Google Scholar] [CrossRef]
- FEMA P695. Quantification of Building Seismic Performance Factors; Federal Emergency Management Agency: Washington, DC, USA, 2009. [Google Scholar]
- Open System for Earthquake Engineering Simulation (OpenSees). 2016. Available online: http://opensees.berkeley.edu/wiki/index.php/Command_Manual (accessed on 14 March 2017).
- ATC-58. Guidelines for Seismic Performance Assessment of Buildings; Applied Technology Council: Redwood City, CA, USA, 2009. [Google Scholar]

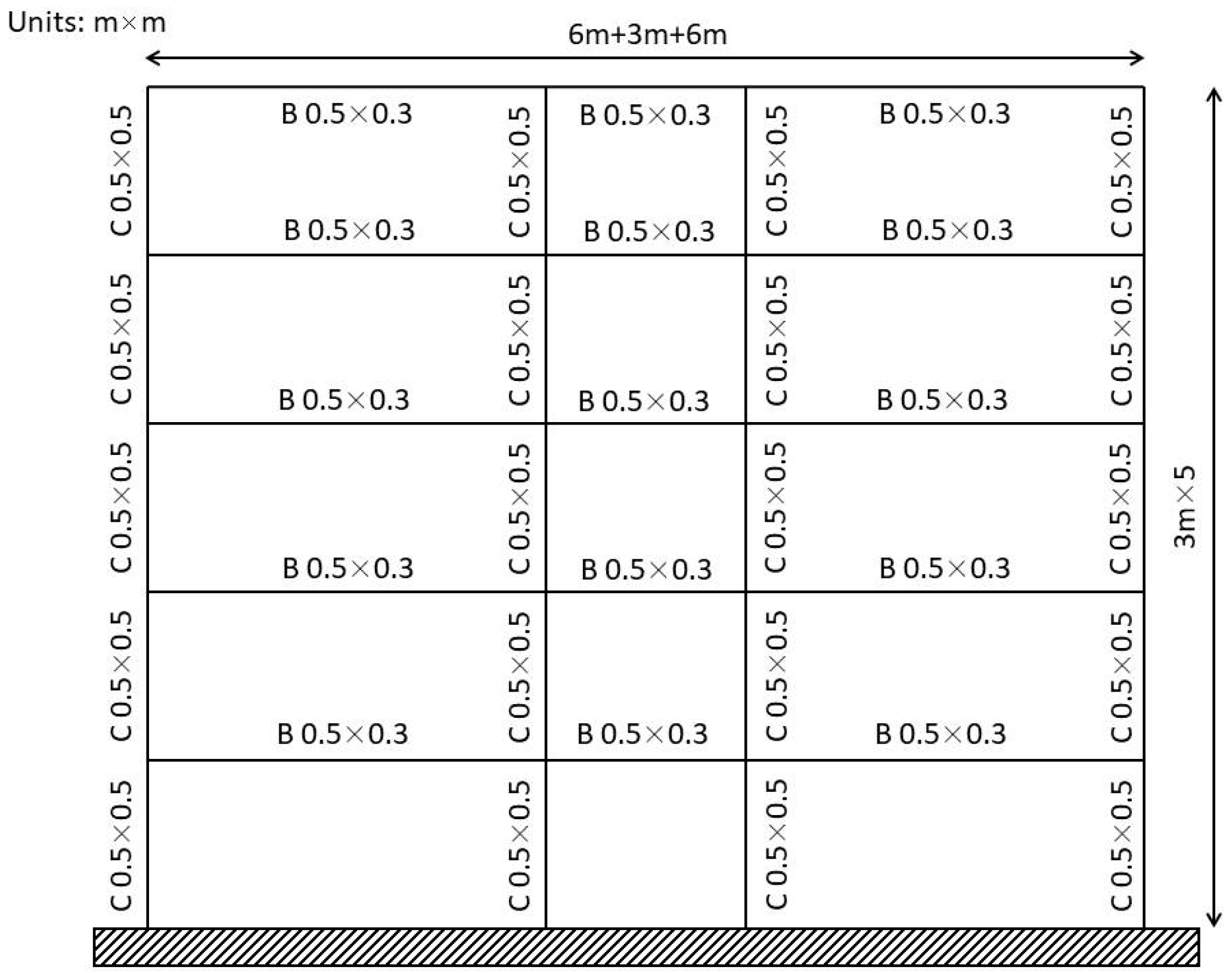


| Story | Beams | Columns | |||||
|---|---|---|---|---|---|---|---|
| Size (mm2) | Concrete | Reinforcement | Size (mm2) | Concrete | Reinforcement | ||
| Upper Side | Bottom Side | ||||||
| 1 | 300 × 500 | C25 | 4Φ25 | 4Φ22 | 500 × 500 | C30 | 12Φ22 |
| 2 | 300 × 500 | C25 | 4Φ25 | 4Φ22 | 500 × 500 | C30 | 12Φ22 |
| 3 | 300 × 500 | C25 | 4Φ25 | 4Φ22 | 500 × 500 | C30 | 12Φ22 |
| 4 | 300 × 500 | C25 | 4Φ22 | 4Φ20 | 500 × 500 | C30 | 12Φ20 |
| 5 | 300 × 500 | C25 | 4Φ22 | 4Φ20 | 500 × 500 | C30 | 12Φ20 |
| No. | Earthquake | Year | Recording Name | Magnitude | PGA (g) |
|---|---|---|---|---|---|
| 1 | Northridge | 1994 | Canyon Country-WLC | 6.7 | 0.48 |
| 2 | Imperial Valley | 1979 | EI Centro Array #11 | 6.5 | 0.38 |
| 3 | Kobe, Japan | 1995 | Shin-Osaka | 6.9 | 0.24 |
| 4 | Kocaeli, Turkey | 1999 | Duzce | 7.5 | 0.36 |
| 5 | Kocaeli, Turkey | 1999 | Arcelik | 7.5 | 0.22 |
| 6 | Landers | 1992 | Yermo Fire Station | 7.3 | 0.24 |
| 7 | Loma Prieta | 1989 | Gilroy Array #3 | 6.9 | 0.56 |
| 8 | Superstition Hills | 1987 | Poe Road (temp) | 6.5 | 0.45 |
| 9 | Chi-Chi, Taiwan | 1999 | CHY101 | 7.6 | 0.44 |
| 10 | San Fernando | 1971 | LA-Hollywood Stor | 6.6 | 0.22 |
| 11 | Hector Mine | 1999 | Hector | 7.1 | 0.34 |
| Story | Code-Designed | Optimized |
|---|---|---|
| 1 | 12Φ22 | 12Φ25 |
| 2 | 12Φ22 | 12Φ25 |
| 3 | 12Φ22 | 4Φ22 + 8Φ20 |
| 4 | 12Φ20 | 4Φ22 + 4Φ20 |
| 5 | 12Φ20 | 4Φ22 + 4Φ18 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, M.; Li, S. Refined Seismic Design Method for RC Frame Structures to Increase the Collapse Resistant Capacity. Appl. Sci. 2020, 10, 8230. https://doi.org/10.3390/app10228230
Gao M, Li S. Refined Seismic Design Method for RC Frame Structures to Increase the Collapse Resistant Capacity. Applied Sciences. 2020; 10(22):8230. https://doi.org/10.3390/app10228230
Chicago/Turabian StyleGao, Mengmeng, and Shuang Li. 2020. "Refined Seismic Design Method for RC Frame Structures to Increase the Collapse Resistant Capacity" Applied Sciences 10, no. 22: 8230. https://doi.org/10.3390/app10228230
APA StyleGao, M., & Li, S. (2020). Refined Seismic Design Method for RC Frame Structures to Increase the Collapse Resistant Capacity. Applied Sciences, 10(22), 8230. https://doi.org/10.3390/app10228230






