A Study on the Influence of the Cross-Sectional Shape of the Metal-Inserted Retainer Ring and the Pressure Distribution from the Multi-Zone Carrier Head to Increase the Wafer Yield
Abstract
:1. Introduction
2. Finite Element Model Verification
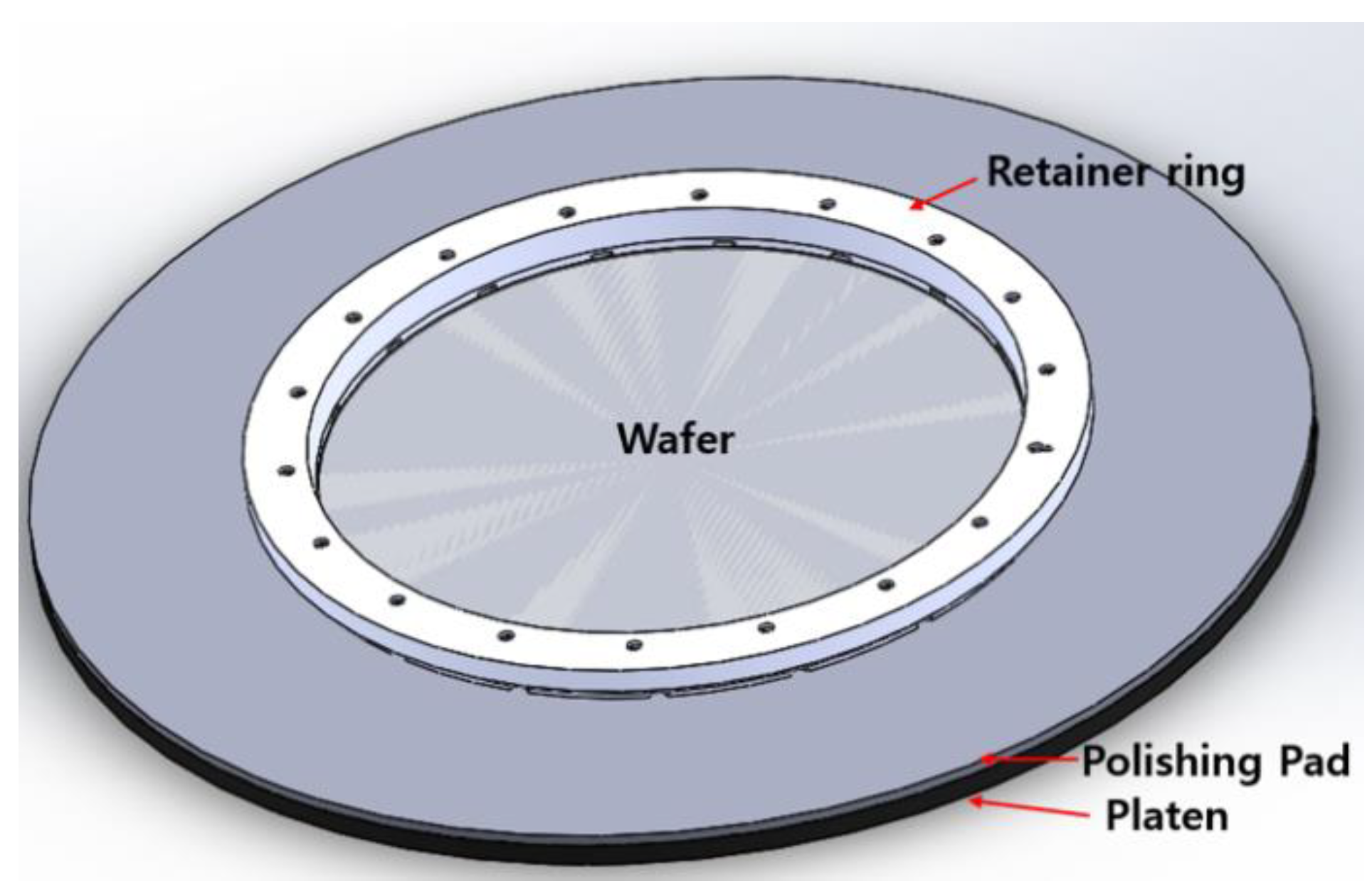
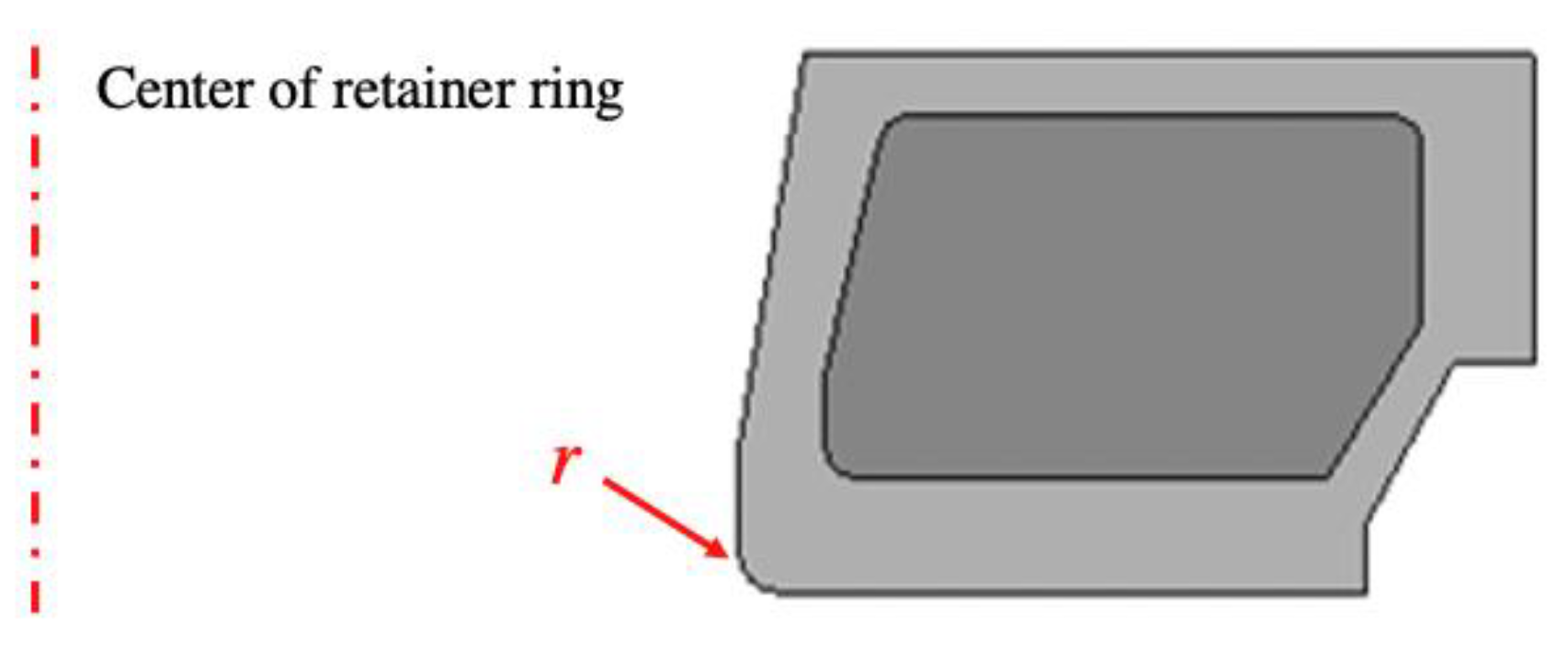
2.1. Structure of Retainer Ring
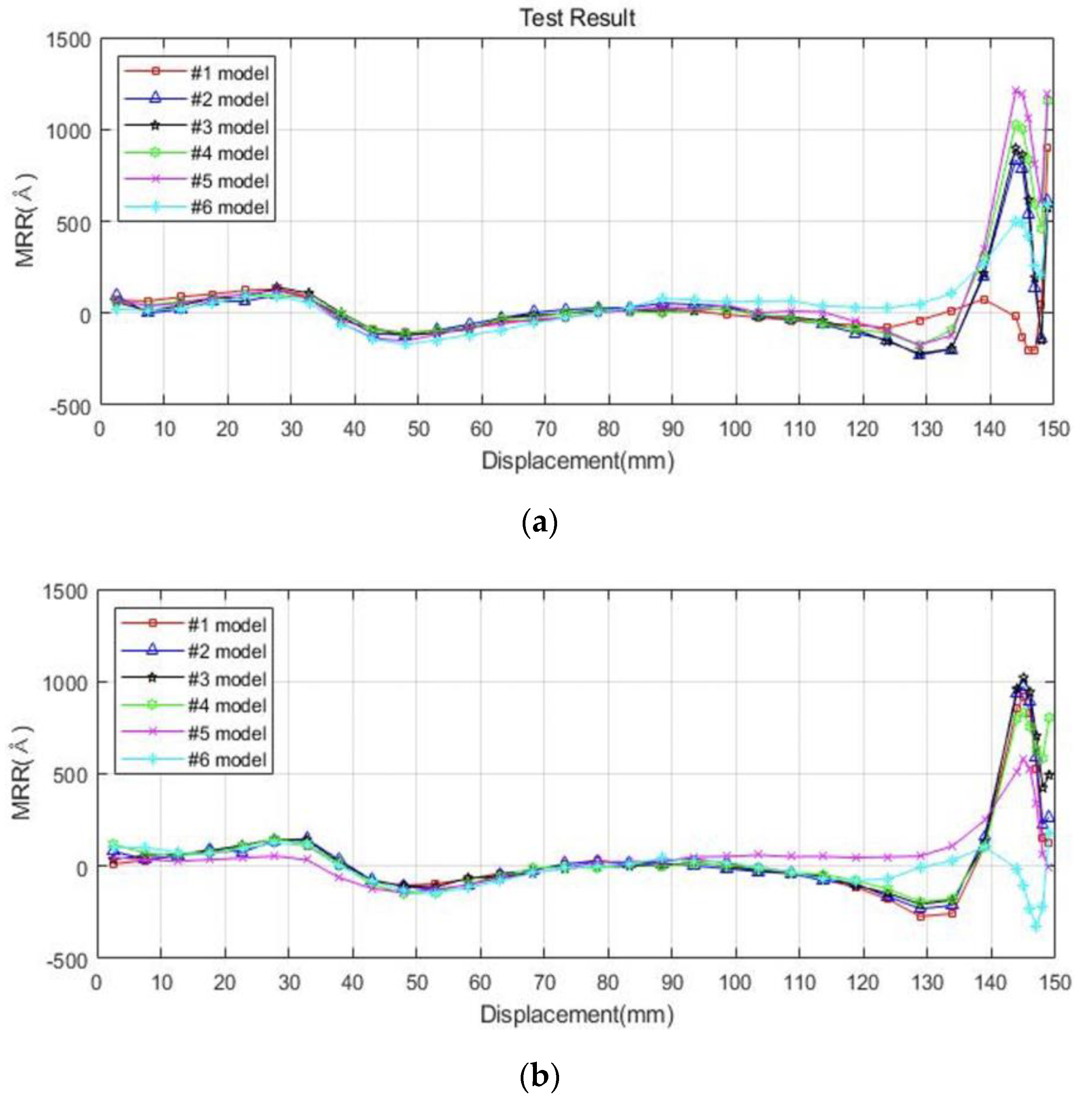
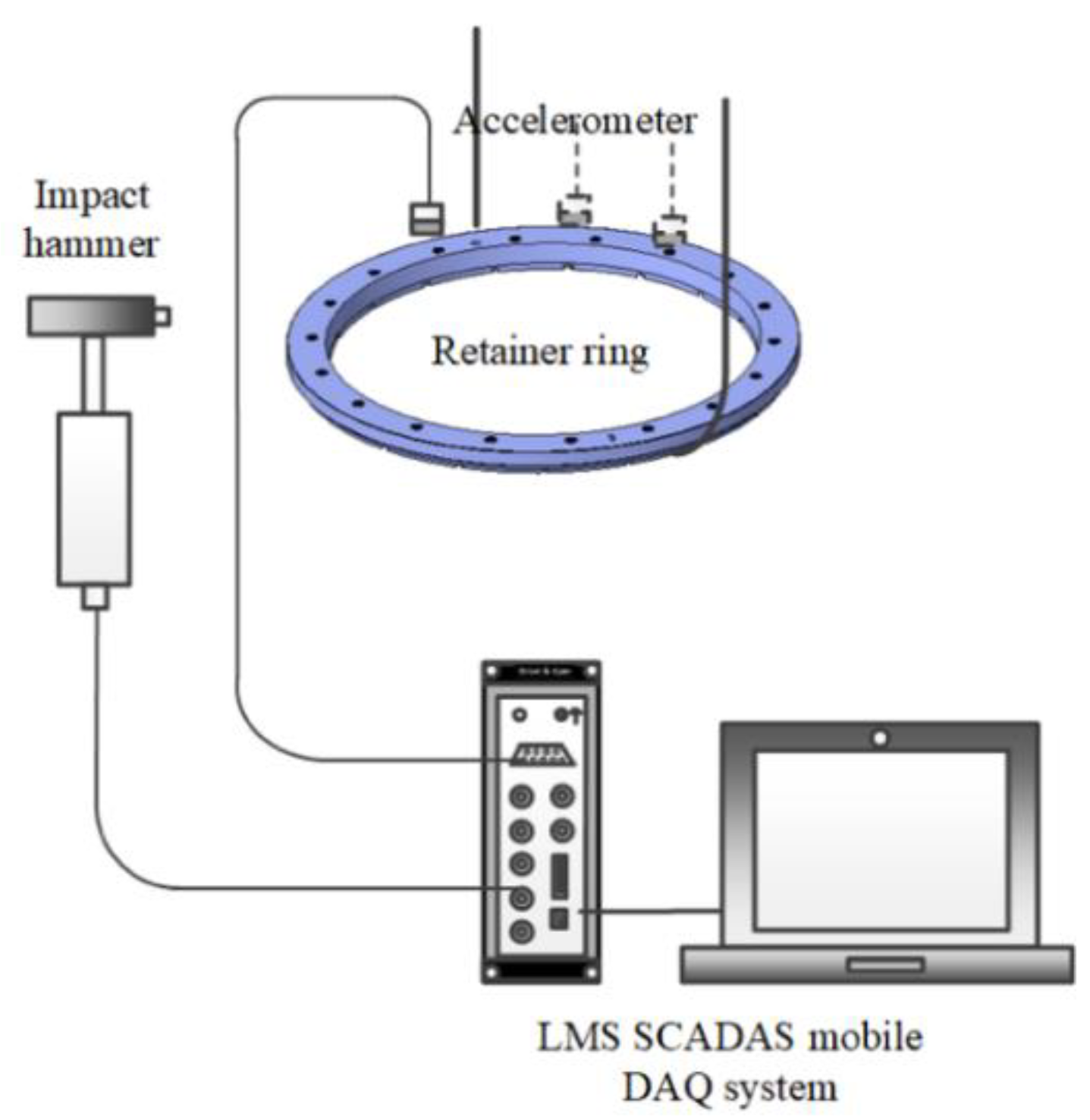
2.2. Analysis of Exsisting Test Data
2.3. Building Up Finite Element Model
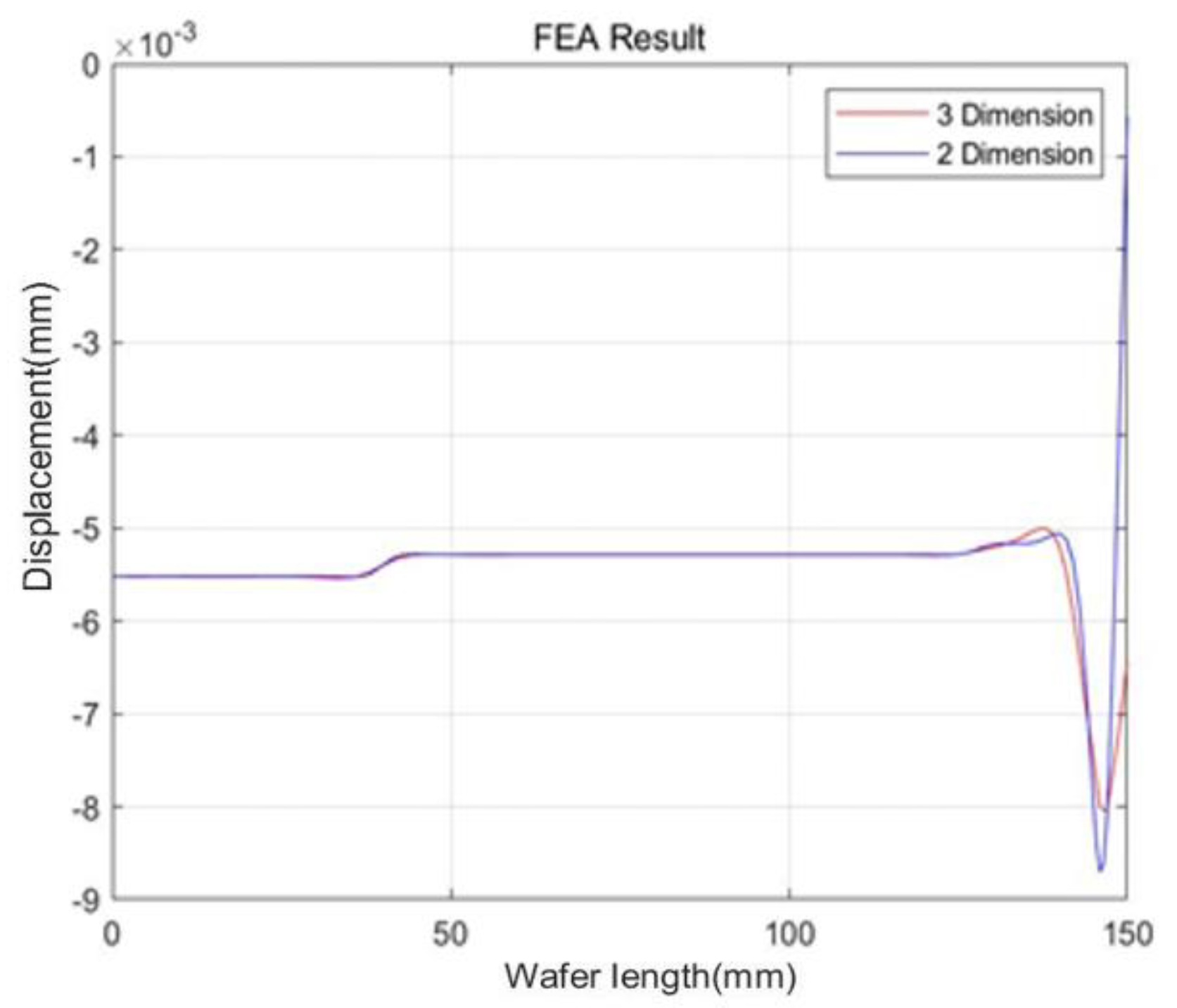
2.4. FEM Verification: Comparison of Test Data
3. Finite Element Analysis Result: Investigation of the Influence of Pressure Distribution and Shape of Retainer Ring
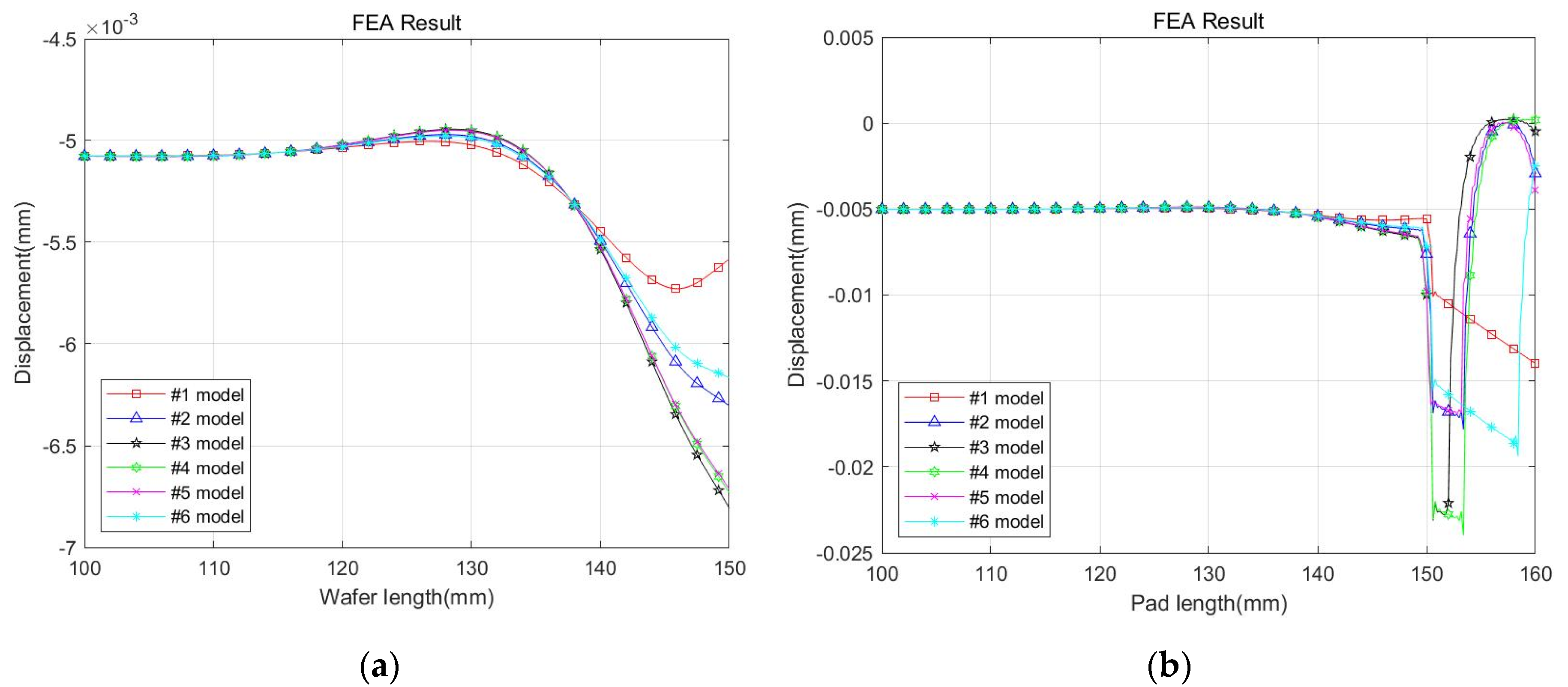
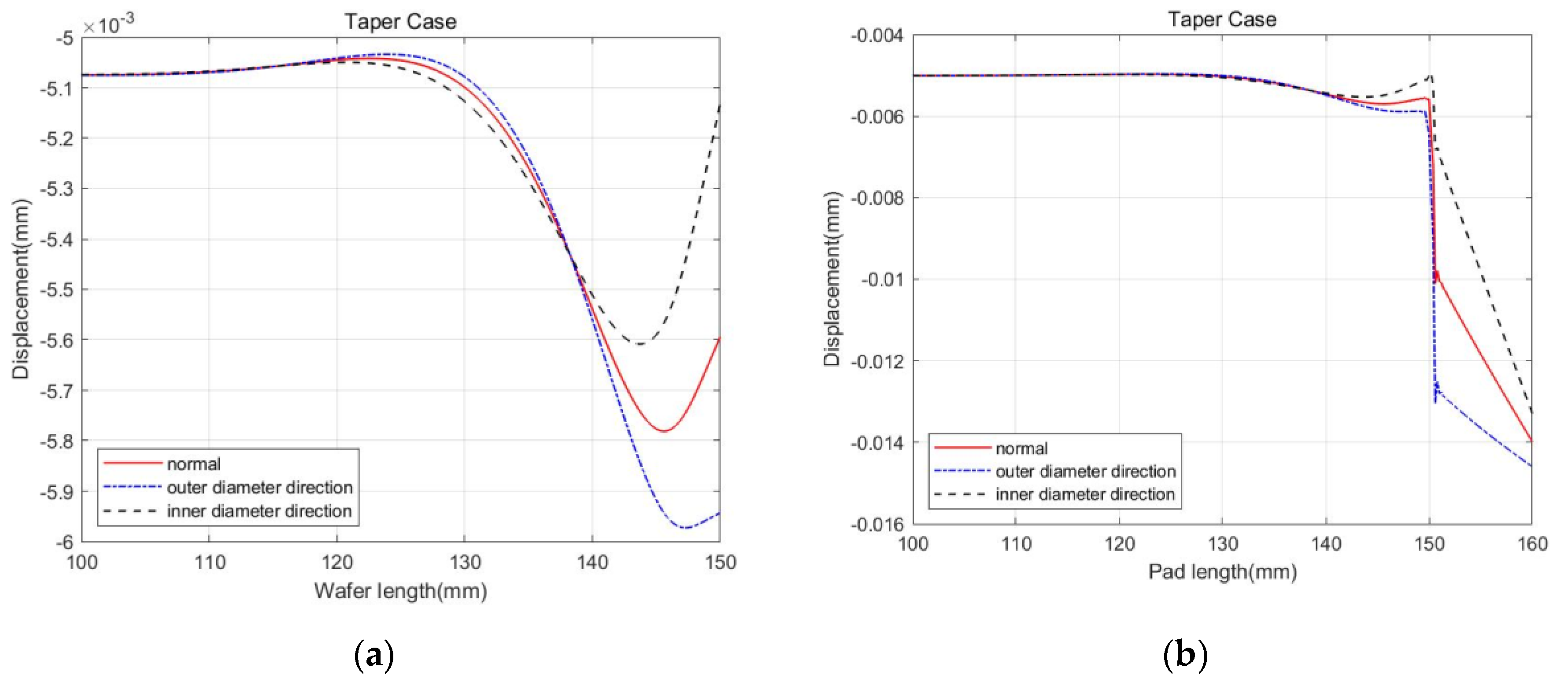
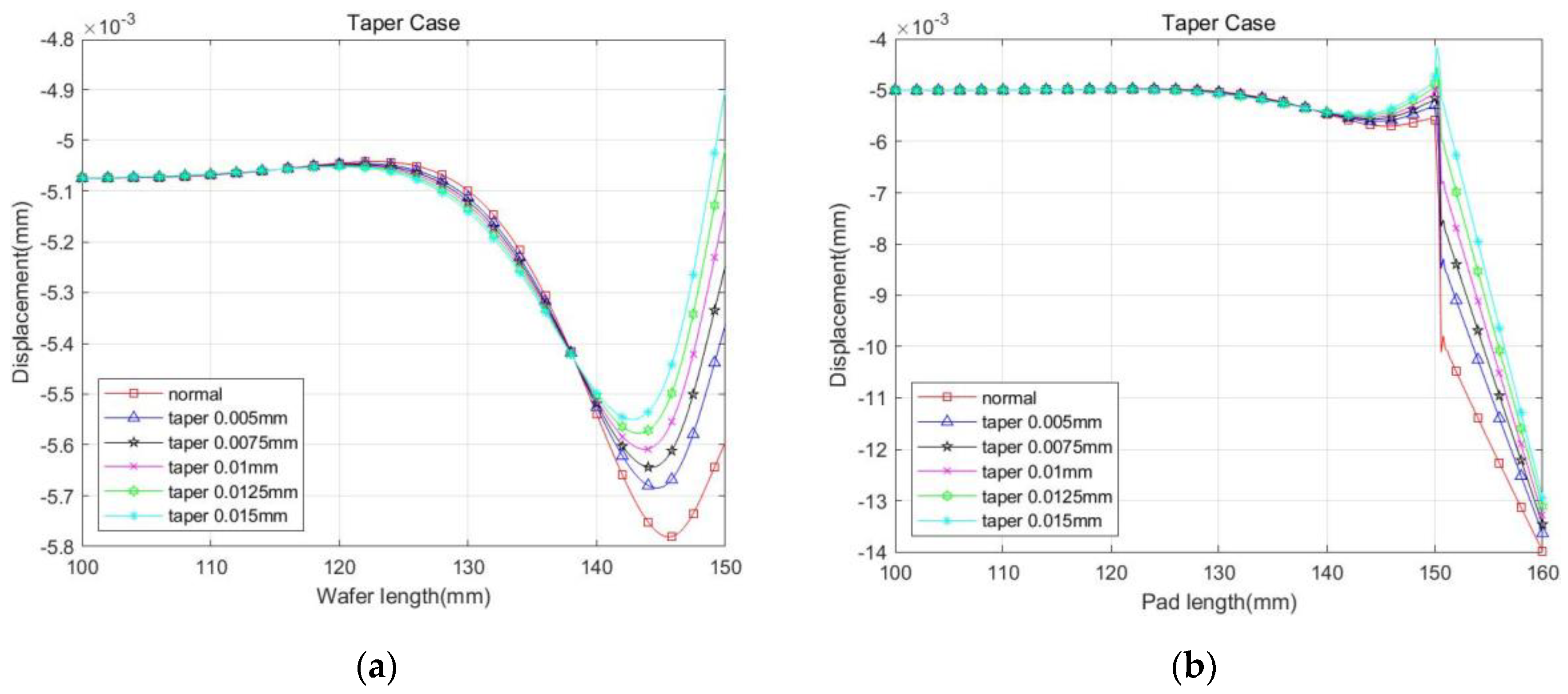
3.1. Investigation of the Impact of the Taper Turning on the Lower Part of the Retainer Ring
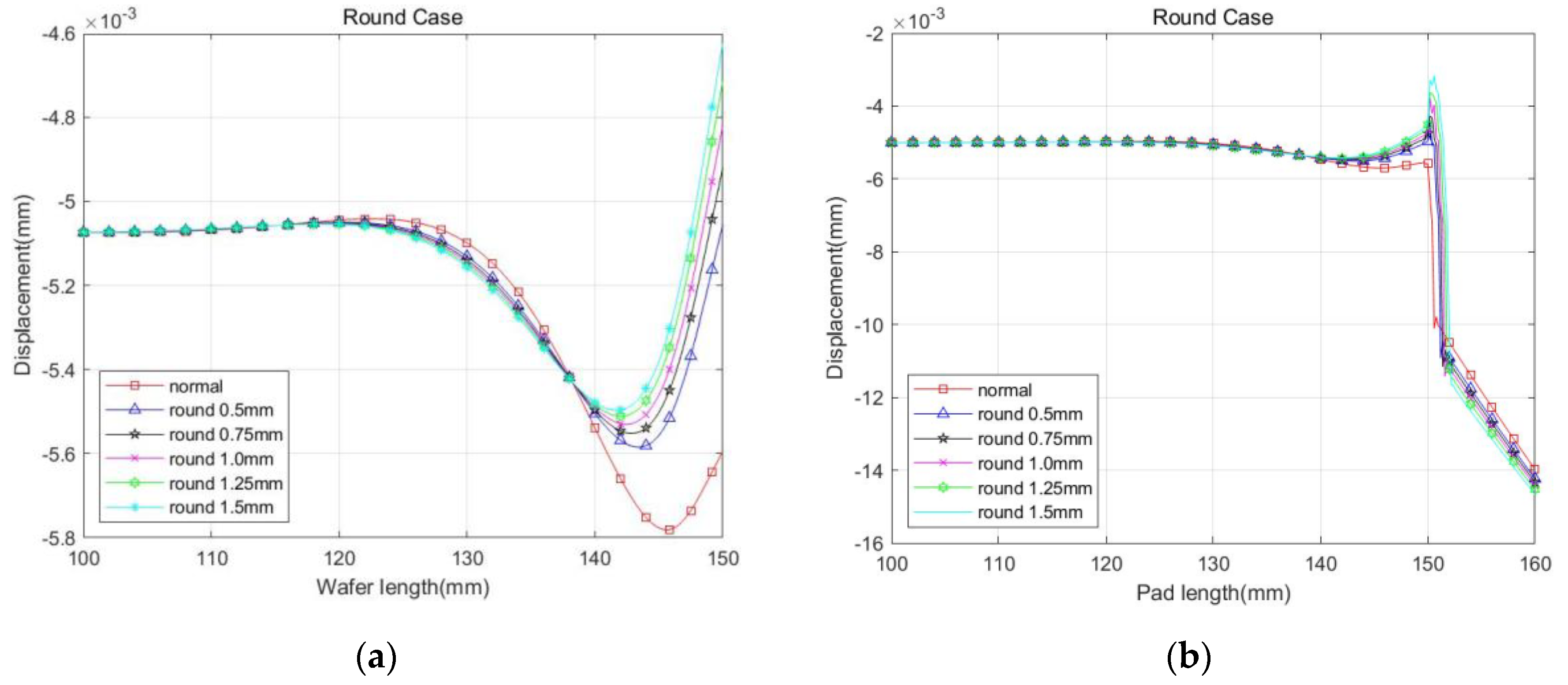
3.2. Investigation of the Influence of Machining Round Corner on the Retainer Ring
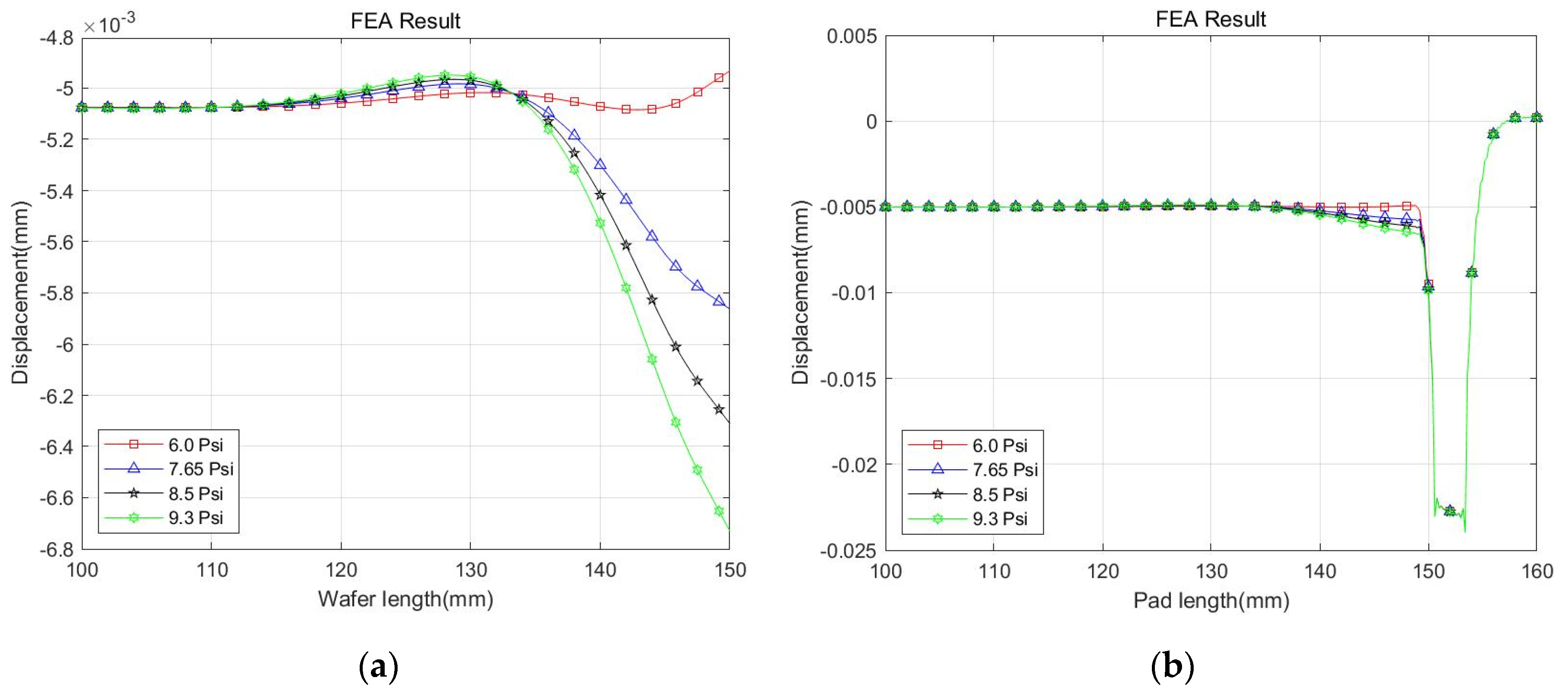
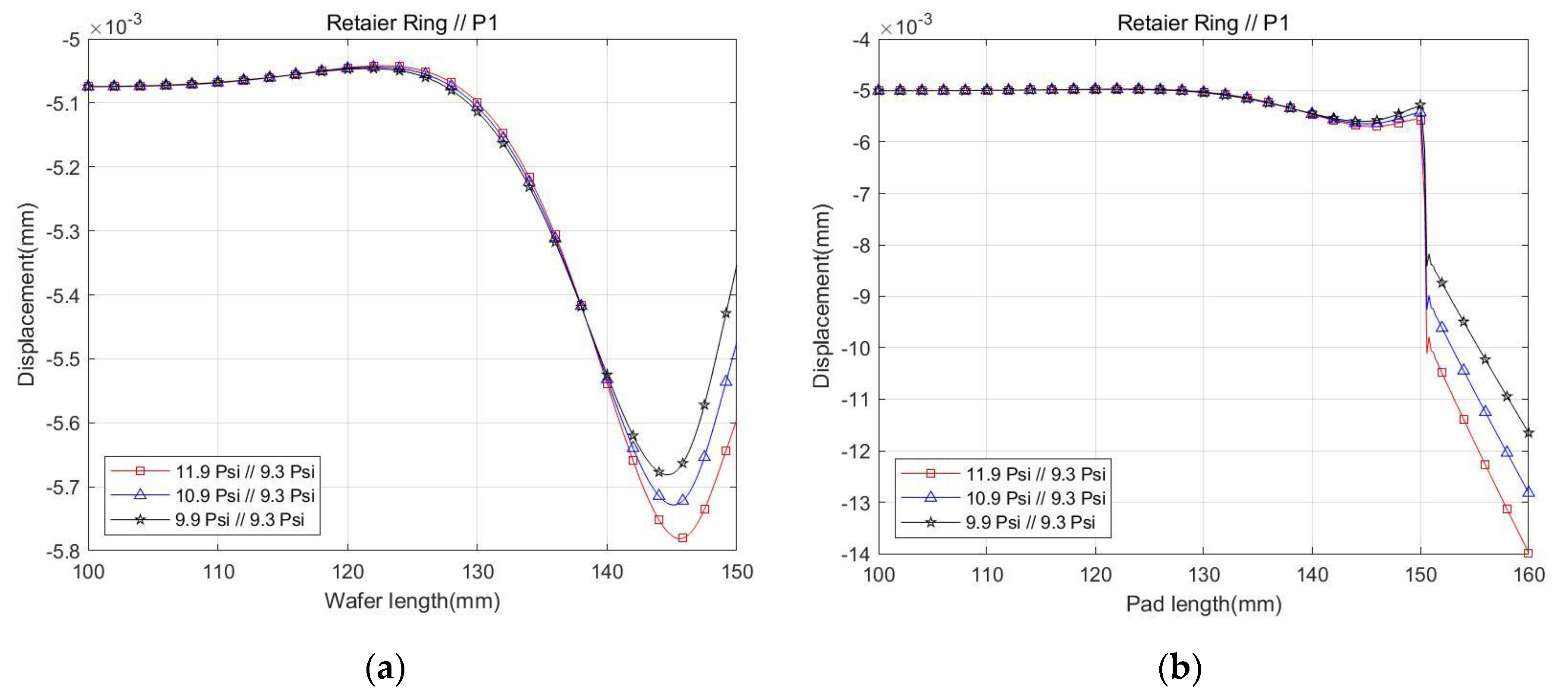
3.3. Investigation of the Influence of P1 Change
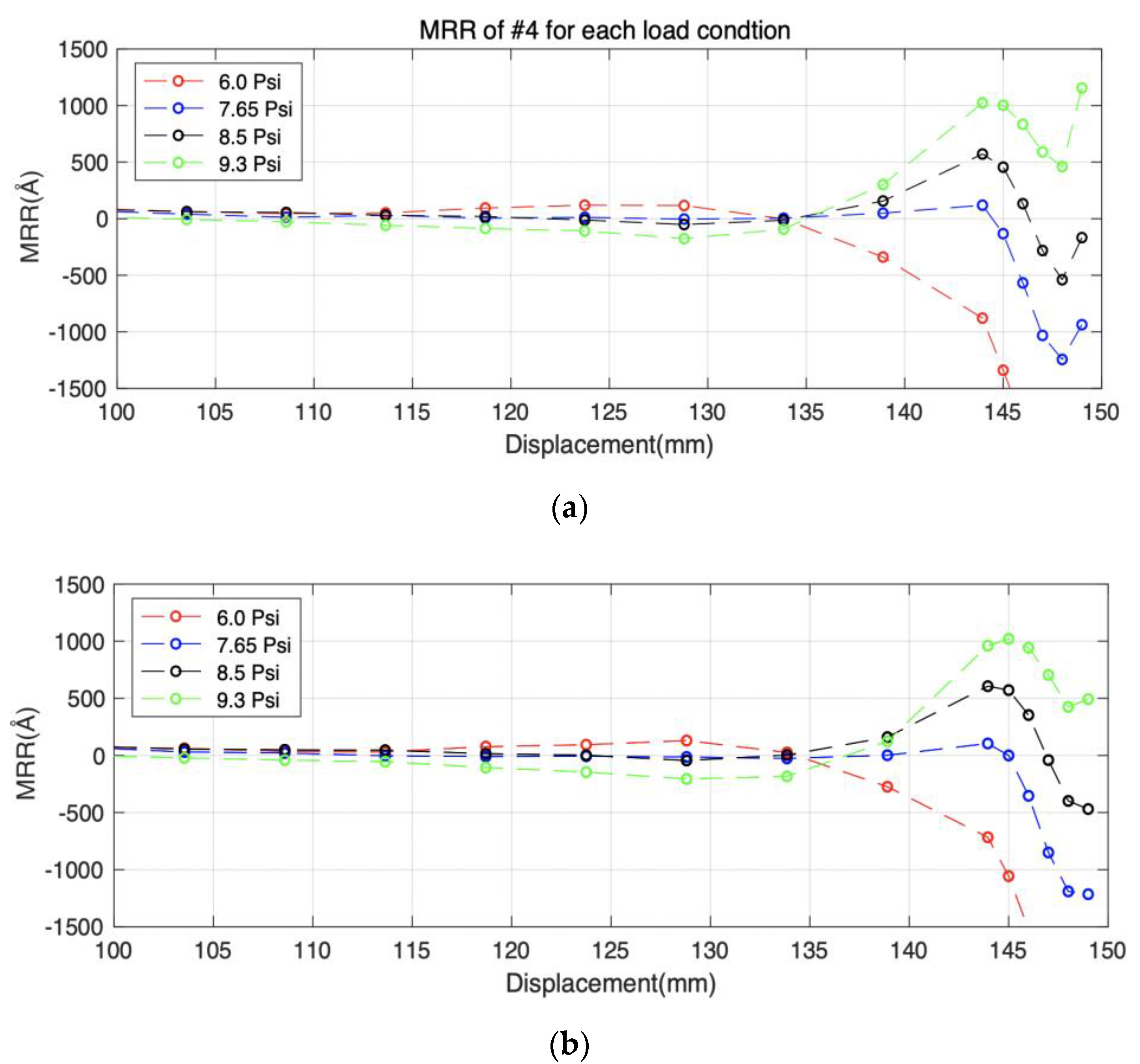
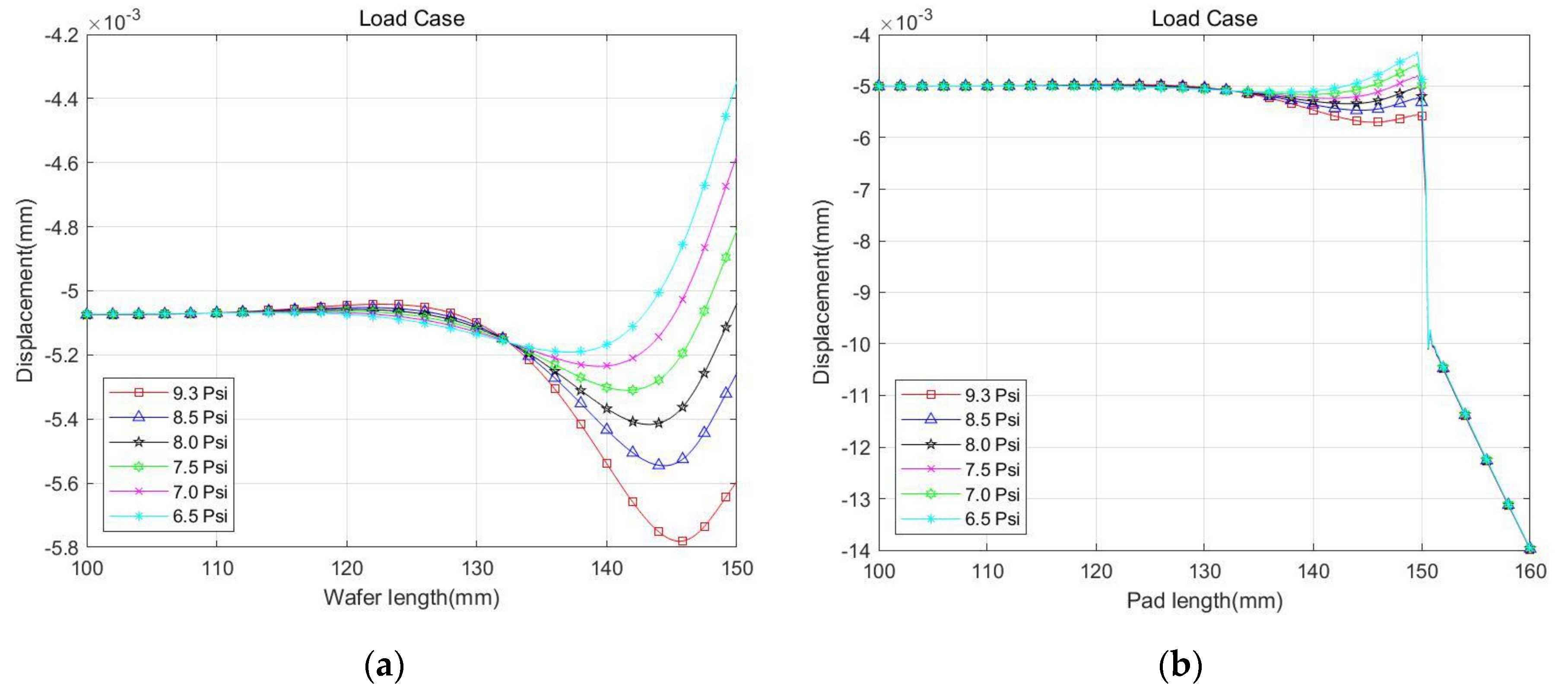
3.4. Investigation of the Influence of the Pressure Change of the Retainer Ring
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Eamajornsiri, S.; Narayannaswami, R.; Chandra, A. Yield Improvement in Wafer Planarization: Modeling and Simulation. J. Manuf. Syst. 2002, 33, 239–247. [Google Scholar]
- Preston, F.W. The Theory and Design of Plate Class Polishing Machine. J. Soc. Glass Technol. 1927, 11, 214–256. [Google Scholar]
- Xu, Q.; Chen, L.; Yang, F.; Cao, H. Influence of slurry components on copper CMP performance in alkaline slurry. Micoelectr. Eng. 2017, 183–184, 1–11. [Google Scholar] [CrossRef]
- Park, I.; Lee, D.; Jeong, S.; Jeong, H. Effect of Temperature on Removal Rate in Cu CMP. J. Korea Soc. Manuf. Process Eng. 2018, 6, 91–97. [Google Scholar] [CrossRef]
- Bae, J.; Lee, H.; Park, J.; Nishizawa, H.; Kinoshita, M.; Jeong, H. Effect of Thickness on Removal Rate and Within Wafer Non-Uniformity in Oxide CMP. J. Korea Tribol. Lubr. Eng. 2002, 23, 358–363. [Google Scholar]
- Lo, S.; Lin, Y.; Huang, J. Analysis of retaining ring using finite element simulation in chemical polishing process. Int. J. Adv. Manuf. Technol. 2007, 34, 547–555. [Google Scholar] [CrossRef]
- Park, Y.; Lee, H.; Park, S.; Jeong, H. Effect on Contact Angle between Retaining Ring and Polishing Pad on Material Removal Uniformity in CMP. Int. J. Precis. Eng. Manuf. 2013, 14, 1513–1518. [Google Scholar] [CrossRef]
- Park, S.; An, B.; Lee, J. The Effect of Pressure and Platen Speed on the Material Removal Rate of Sapphire Wafer in the CMP Process. J. Korea Tribol. Lubr. Eng. 2016, 32, 67–71. [Google Scholar]
- Fu, G.; Chandra, A. The relationship between wafer surface pressure and wafer backside loading in Chemical Mechanical Polishing. Thin Solid Film. 2005, 474, 217–221. [Google Scholar] [CrossRef]
- Kajiwara, J.; Monloney, G.; Wang, H.; Harsen, A.; Reyes, A. System and Method for Pneumatic Diaphragm CMP Head having Separate Retaining Ring and Multi-Region Wafer Pressure Control. U.S. Patent 6,506,105 B1, 14 January 2003. [Google Scholar]
- Wang, T.; Lu, X.; Zhao, D.; He, Y. Contact stress non-uniformity of wafer surface for multi-zone chemical mechanical polishing process. Sci. China Technol. Sci. 2011, 56, 1974–1979. [Google Scholar] [CrossRef]
- Wang, T.; Lu, X. Numerical and experimental investigation on Multi-zone chemical mechanical planarization. Microelectron. Eng. 2001, 88, 3327–3332. [Google Scholar] [CrossRef]
- Kalenian, W.; Speigel, L. CMP Retaining Ring with Soft Retaining Ring Insert. U.S. Patent 9,193,030 B2, 24 November 2015. [Google Scholar]
- Park, K.; Park, D. Effect of Insert Material of the Performance of Wafer Polishing Finish in Oxide CMP. J. Korea Manuf. Process Eng. 2019, 18, 44–50. [Google Scholar]
- Park, K.; Kim, E.; Park, D. Effect of CMP Retaining ring Material on the Performance of Wafer Polishing. J. Korea Manuf. Process Eng. 2020, 19, 22–28. [Google Scholar] [CrossRef]
- Han, J.; Choi, J.; Kim, K. A Study on the Shape of Two-Piece Type Retainer Rings to Increase Usable Wafer Area. J. Korea Inst. Ind. Eng. 2020, 46, 55–63. [Google Scholar]
- Lu, X.; Khonsari, M.; Gelinck, E. The Stribeck curce: Experimental results and theoretical prediction. J. Tribol. 2006, 128, 789–794. [Google Scholar] [CrossRef]

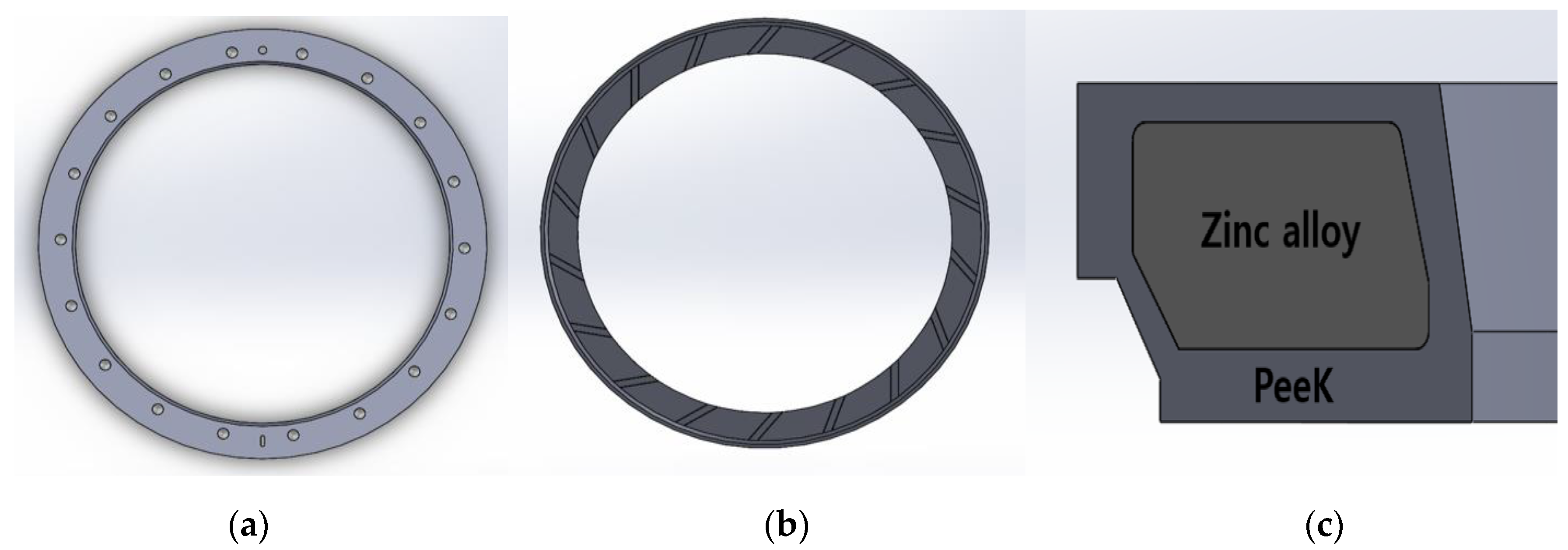
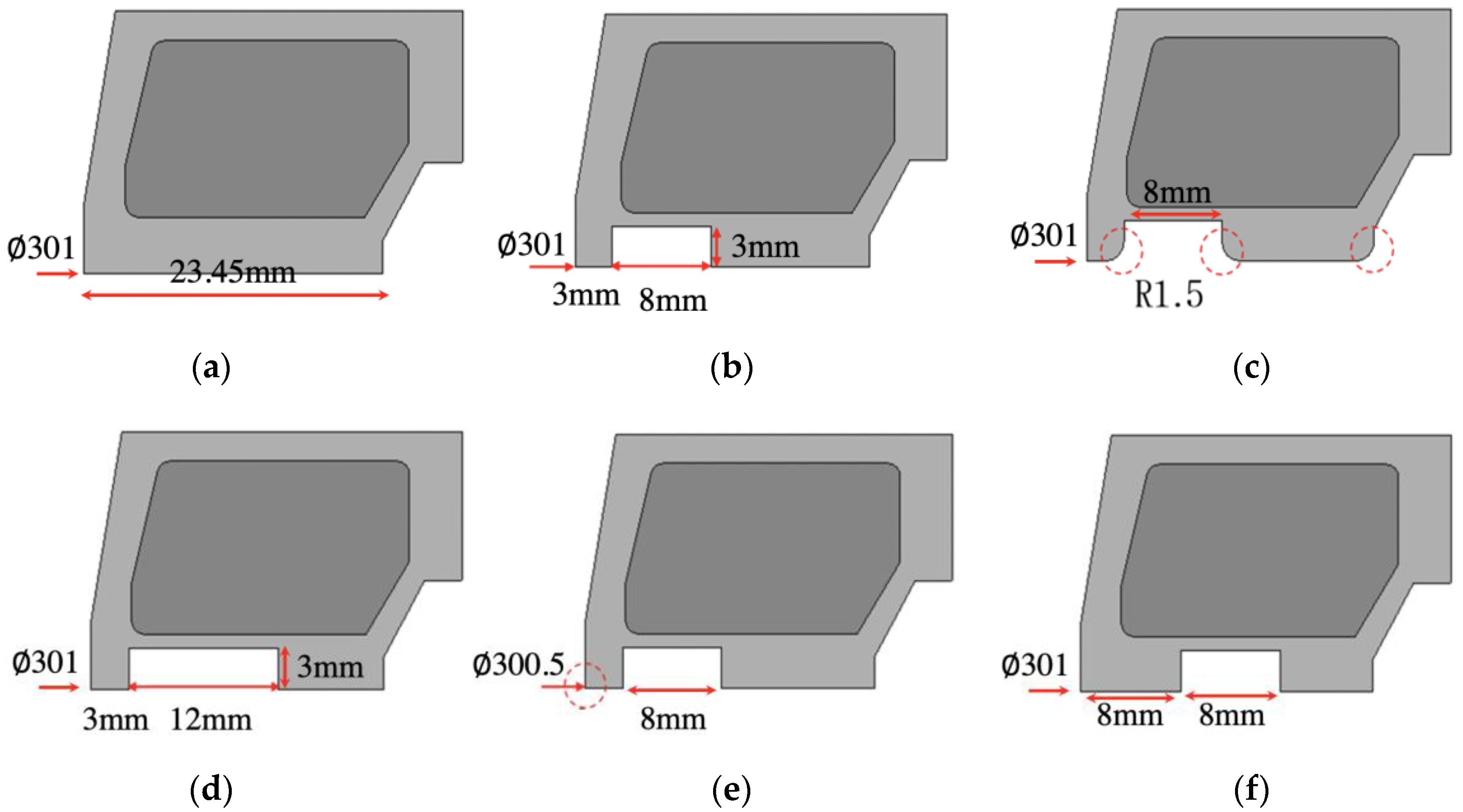



| Distance from Wafer Center [mm] | Zone 1 (P1) | Zone 2 (P2) | Zone 3 (P3) | Zone 4 (P4) | Zone 5 (P5) |
| 145–150 | 128–145 | 100–128 | 40–100 | 0–40 |
| Unit: Å | #1 | #2 | #3 | #4 | #5 | #6 |
|---|---|---|---|---|---|---|
| Maximum relative MRR [averaged] | 893/182 551/179 [450] | 1083/915 1102/954 [1014] | 1097/1092 158/1079 [1107] | 1148/1027 1133/1118 [1107] | 1215/825 1554/1533 [1282] | 583/585 494/535 [549] |
| Relative MRR@7mm [averaged] | −5/14 195/157 [90] | 880/786 964/776 [852] | 951/883 1002/887 [931] | 881/810 966/892 [887] | 1053/661 1344/1255 [1078] | 446/470 452/445 [453] |
| P1 [psi] | 9.3 | 8.5 | 7.65 | 6.0 |
|---|---|---|---|---|
| Maximum relative MRR [averaged] | 1148/1027 1133/1118 [1107] | 572/606 [589] | −1244/−1215 [−1230] | −2175/−2121 [−2148] |
| Relative MRR@7mm [averaged] | 881/810 966/892 [887] | 502/514 [508] | 106/85 [96] | −779/−631 [−705] |
| Elastic Modulus (MPa) | Density (kg/m3) | Poisson’s Ratio | |
|---|---|---|---|
| Wafer | 10,000 | 2329 | 0.22 |
| Retainer ring (Polyether ether ketone) | 2935 | 1174 | 0.39 |
| Retainer ring (Inner metal ring) | 70,080 | 6020 | 0.32 |
| Pad | 20 | 35 | 0.1 |
| Platen | 190,000 | 7850 | 0.3 |
| Wafer | Retainer Ring (Model #1) | Pad | Platen | ||
|---|---|---|---|---|---|
| 3D model | No. of node # No. of element Element type Approx. size | 79,456 39,463 C3D8R 1.5 mm | 422,558 244,576 C3D10/C3D8R 2 mm | 82,622 40,991 C3D8R 4 mm | 13,416 27,198 C3D8R 7 mm |
| 2D axisymmetric model | No. of node # No. of element Element type Approx. size | 3780 3020 CAX4R 0.2 mm | 15654 15324 CAX4R 0.2 mm | 34527 32480 CAX4R 0.2 mm | 4477 4060 CAX4R 1 mm |
| Model | Test Result Averaged Relative MRR at r = 143 mm (Å) | Finite Element Analysis Result Pad Relative Displacement at r = 143 mm (10−6 mm) | Finite Element Analysis Result Wafer Relative Displacement at r = 143 mm (10−6 mm) |
|---|---|---|---|
| #1 | 90 | 539 | 547 |
| #2 | 852 | 714 | 724 |
| #3 | 931 | 846 | 857 |
| #4 | 887 | 823 | 835 |
| #5 | 1078 | 822 | 833 |
| #6 | 453 | 679 | 689 |
| P1 | Test Result Averaged Relative MRR at r = 143 mm (Å) | Finite Element Analysis Result Pad Relative Displacement at r = 143 mm (10−6 mm) | FEA Result Wafer Relative Displacement at r = 143 mm (10−6 mm) |
|---|---|---|---|
| 6.0 psi | −705 | −6 | −7 |
| 7.65 psi | 96 | 416 | 421 |
| 8.5 psi | 508 | 626 | 634 |
| 9.3 psi | 887 | 823 | 835 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, J.-Y.; Han, J.-H.; Kim, C. A Study on the Influence of the Cross-Sectional Shape of the Metal-Inserted Retainer Ring and the Pressure Distribution from the Multi-Zone Carrier Head to Increase the Wafer Yield. Appl. Sci. 2020, 10, 8362. https://doi.org/10.3390/app10238362
Park J-Y, Han J-H, Kim C. A Study on the Influence of the Cross-Sectional Shape of the Metal-Inserted Retainer Ring and the Pressure Distribution from the Multi-Zone Carrier Head to Increase the Wafer Yield. Applied Sciences. 2020; 10(23):8362. https://doi.org/10.3390/app10238362
Chicago/Turabian StylePark, Joon-Yeon, Je-Heon Han, and Changwon Kim. 2020. "A Study on the Influence of the Cross-Sectional Shape of the Metal-Inserted Retainer Ring and the Pressure Distribution from the Multi-Zone Carrier Head to Increase the Wafer Yield" Applied Sciences 10, no. 23: 8362. https://doi.org/10.3390/app10238362
APA StylePark, J.-Y., Han, J.-H., & Kim, C. (2020). A Study on the Influence of the Cross-Sectional Shape of the Metal-Inserted Retainer Ring and the Pressure Distribution from the Multi-Zone Carrier Head to Increase the Wafer Yield. Applied Sciences, 10(23), 8362. https://doi.org/10.3390/app10238362





