Numerical Investigation of Locating and Identifying Pipeline Reflectors Based on Guided-Wave Circumferential Scanning and Phase Characteristics
Abstract
1. Introduction
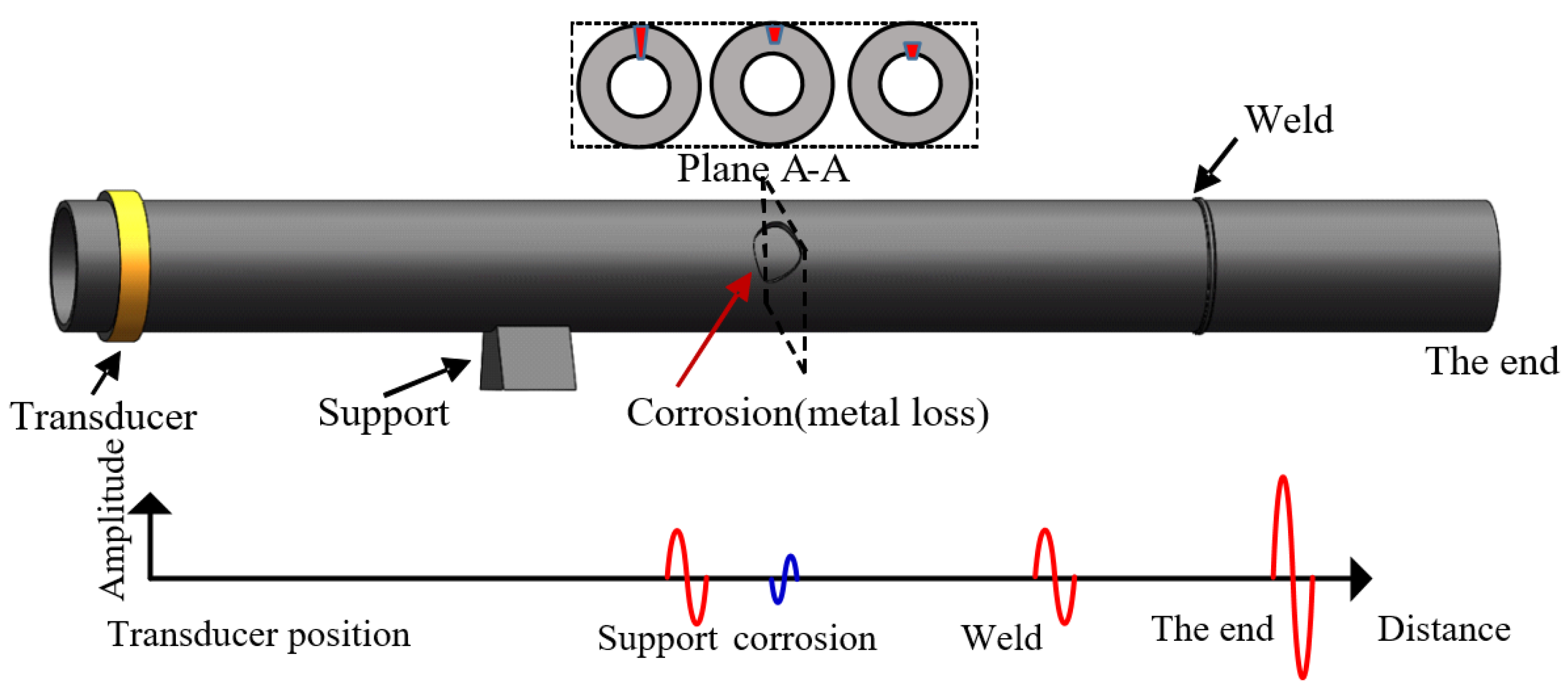
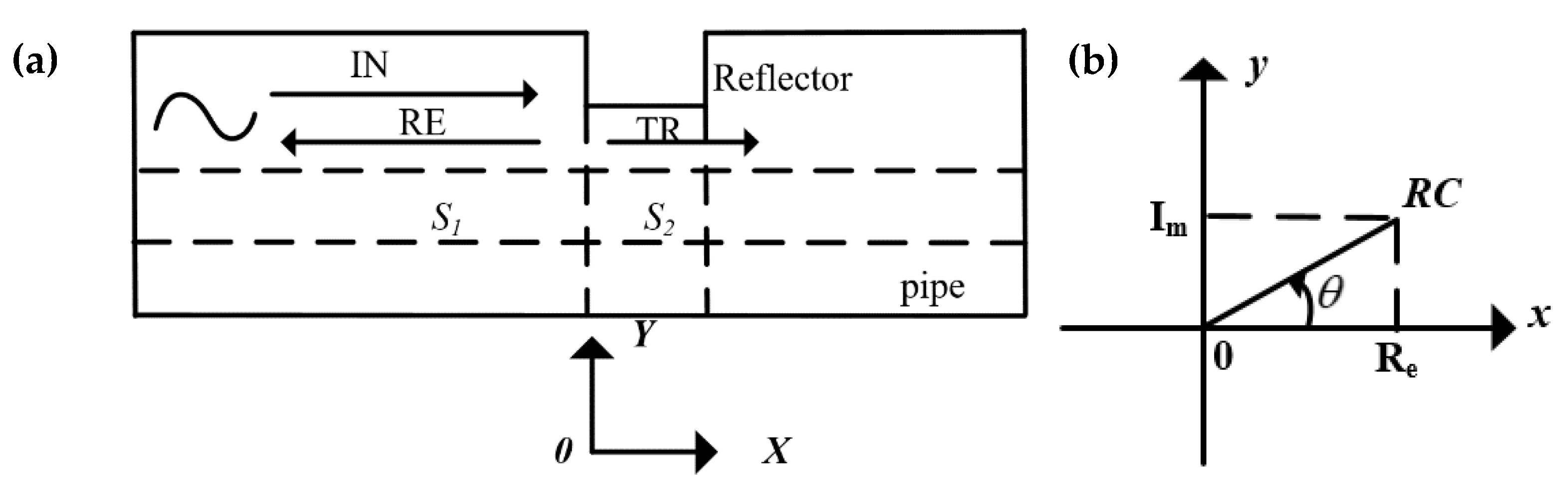
2. Principle of Guided-Wave Circumferential Scanning Technique for a Pipe
3. Phase Characteristics of the Guided Wave Reflected from a Reflector
- (a)
- If δ1 < δ2, namely RC < 0, so the phase shift , k = 1, 2, …, n.
- (b)
- If δ1 > δ2, namely RC > 0, so the phase shift , k = 0, 1, …, n.
- (c)
- If δ1 = δ2, RC = 0, this indicates there is no reflector in the pipeline.
- (d)
- If , this means almost all of the incident wave is reflected back.
4. Proposed Method Based on the Circumferential Scanning and Phase Characteristics
Sr1(t) + Sr2(t − t0) + Ψ(t), 0 < t < T
5. Numerical Simulation and Verification
5.1. The Choice of the Incident (Excitation) Pulse Signal
5.2. Numerical Investigation of the Proposed Method
5.3. Simulation Investigation and Verification
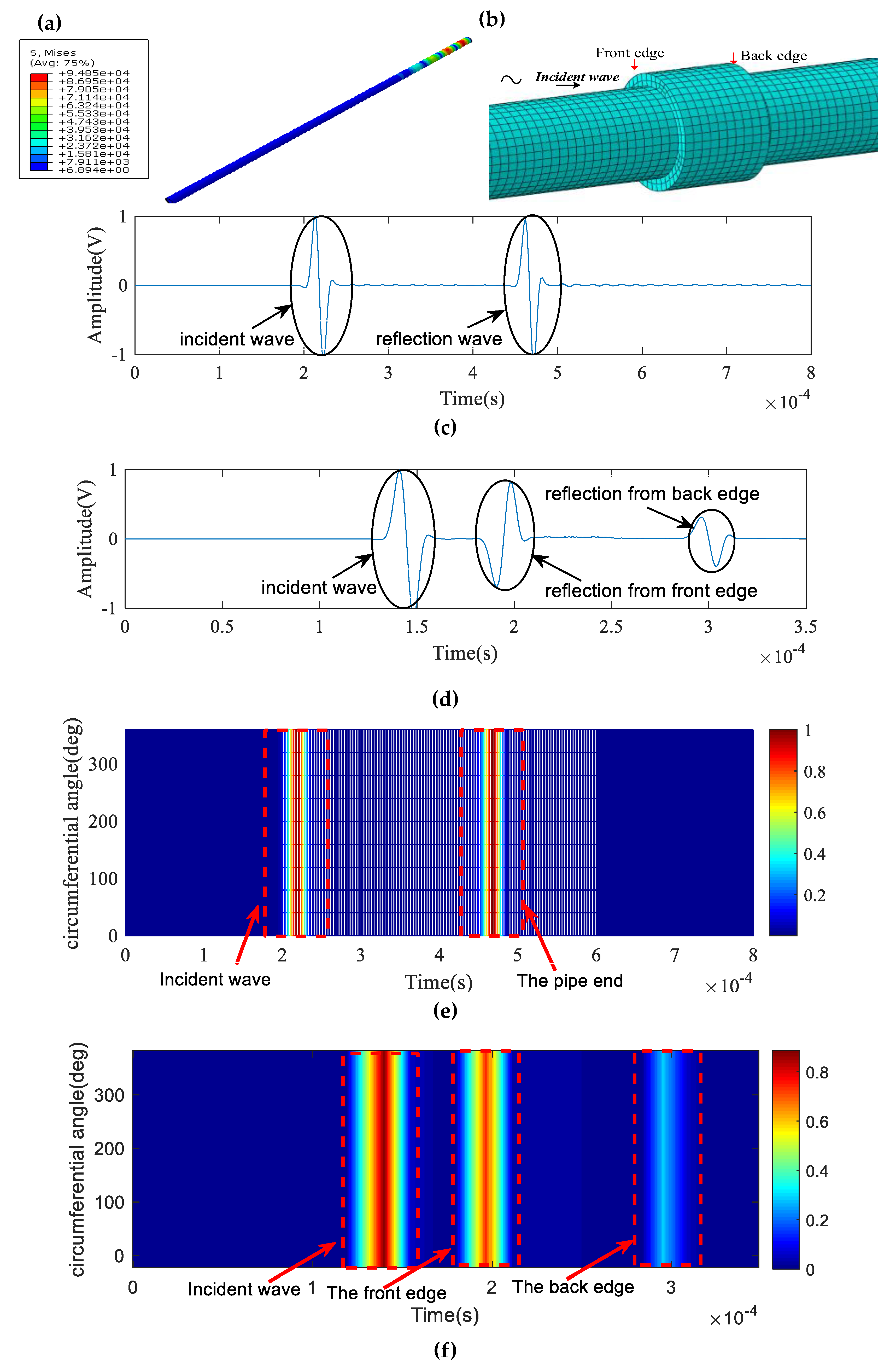
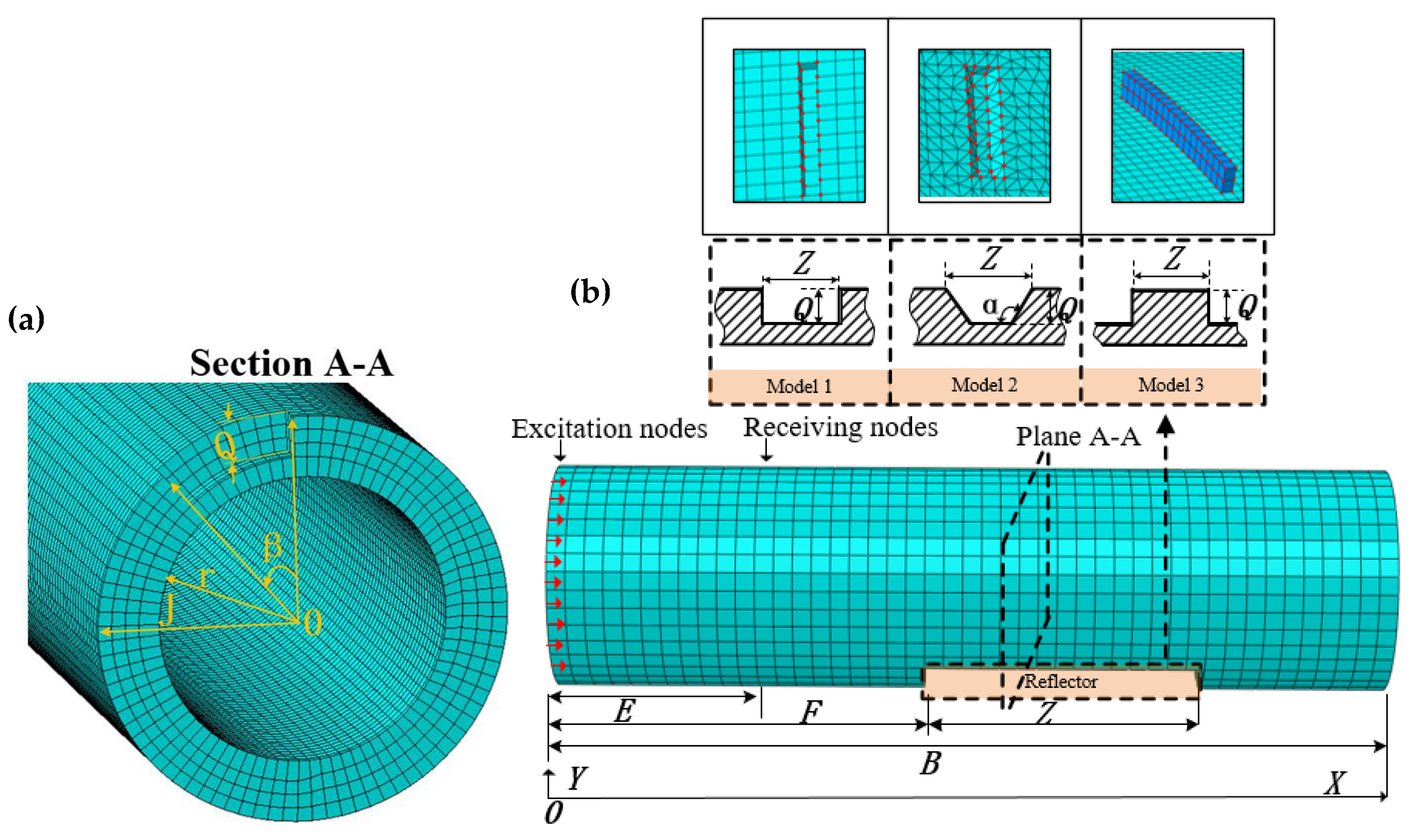
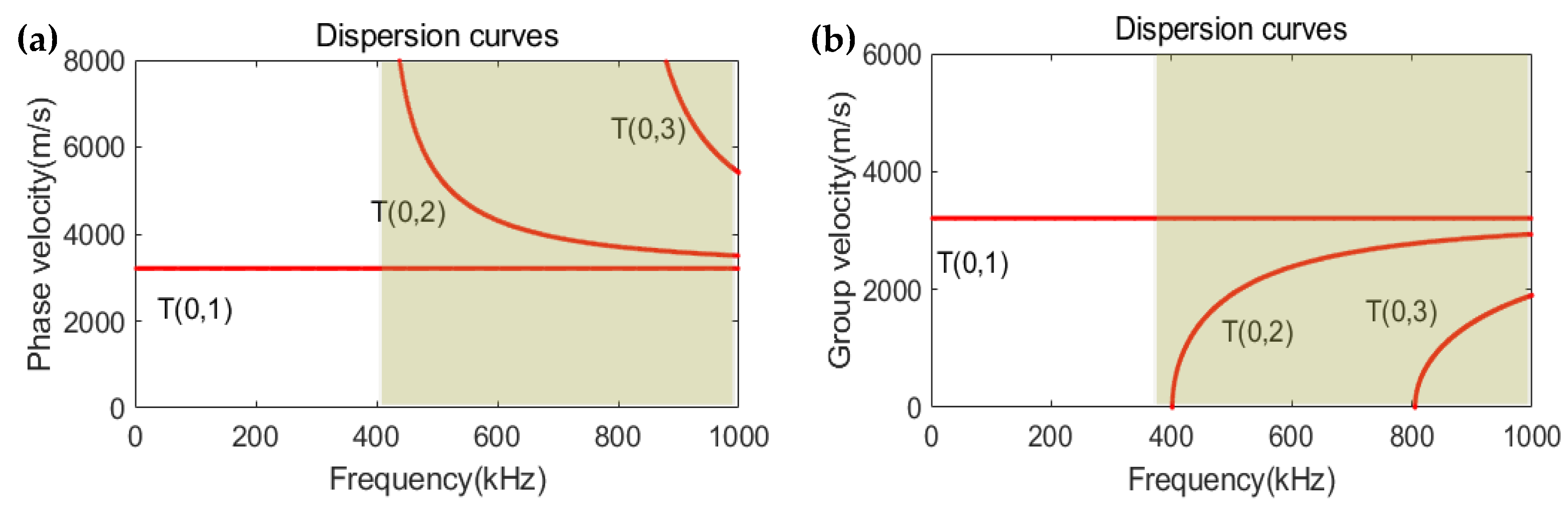
5.3.1. Finite Element Model
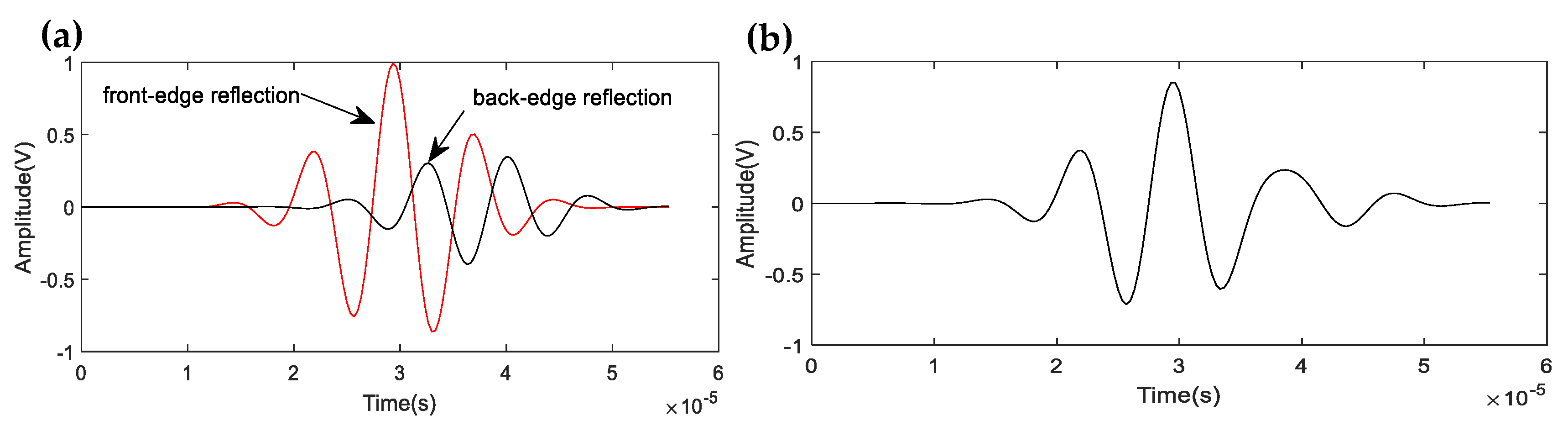
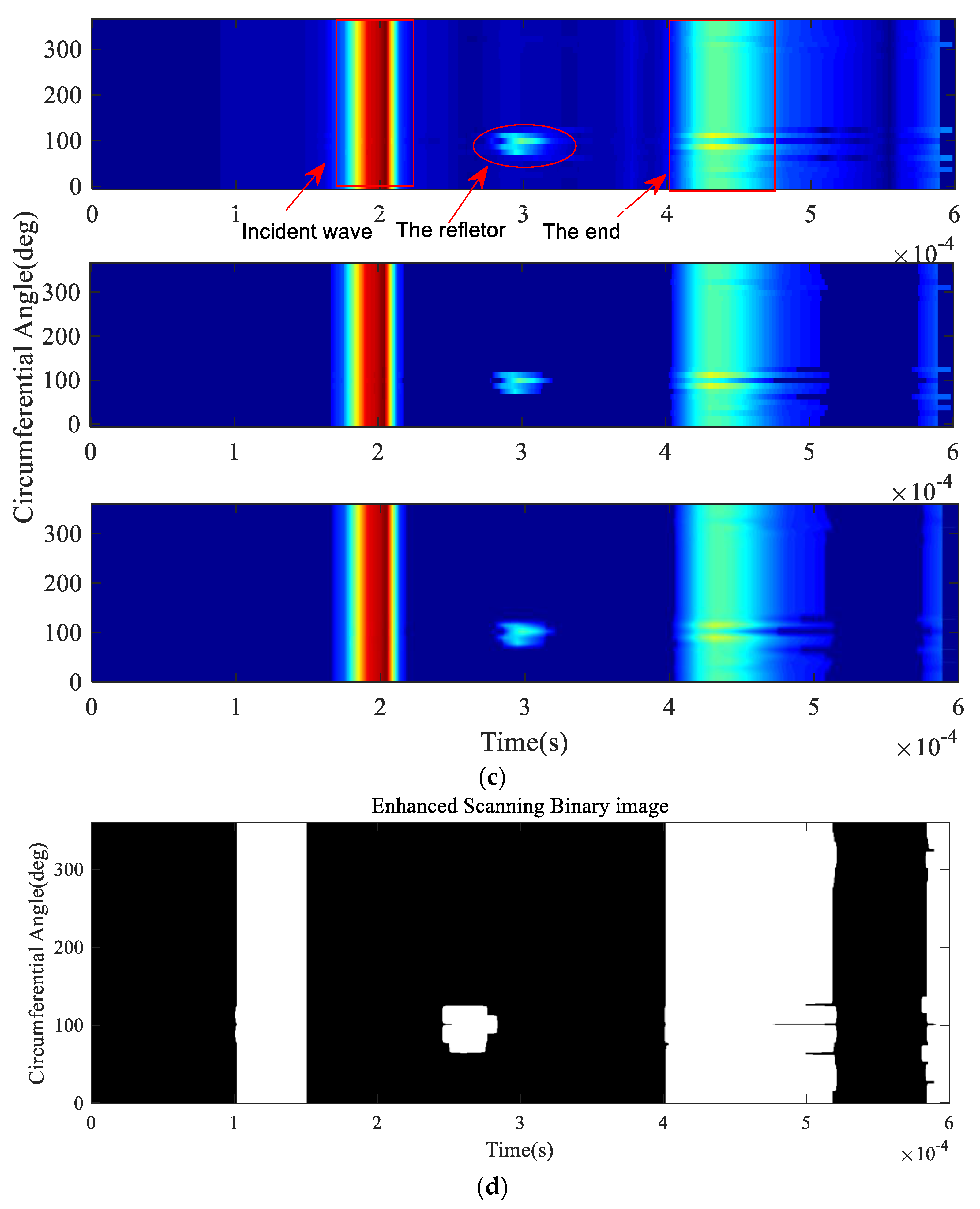
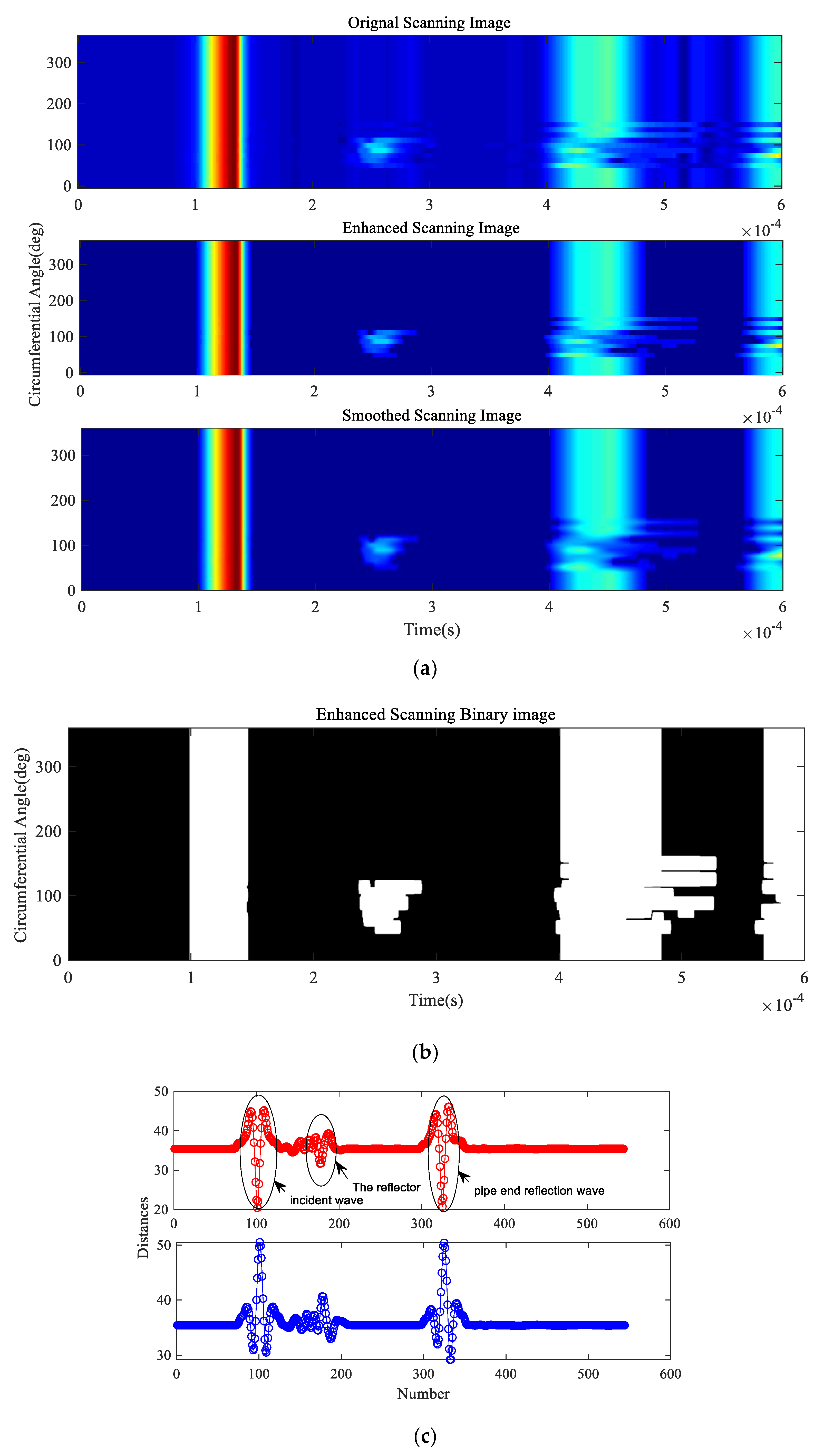
5.3.2. Investigation for Case 1
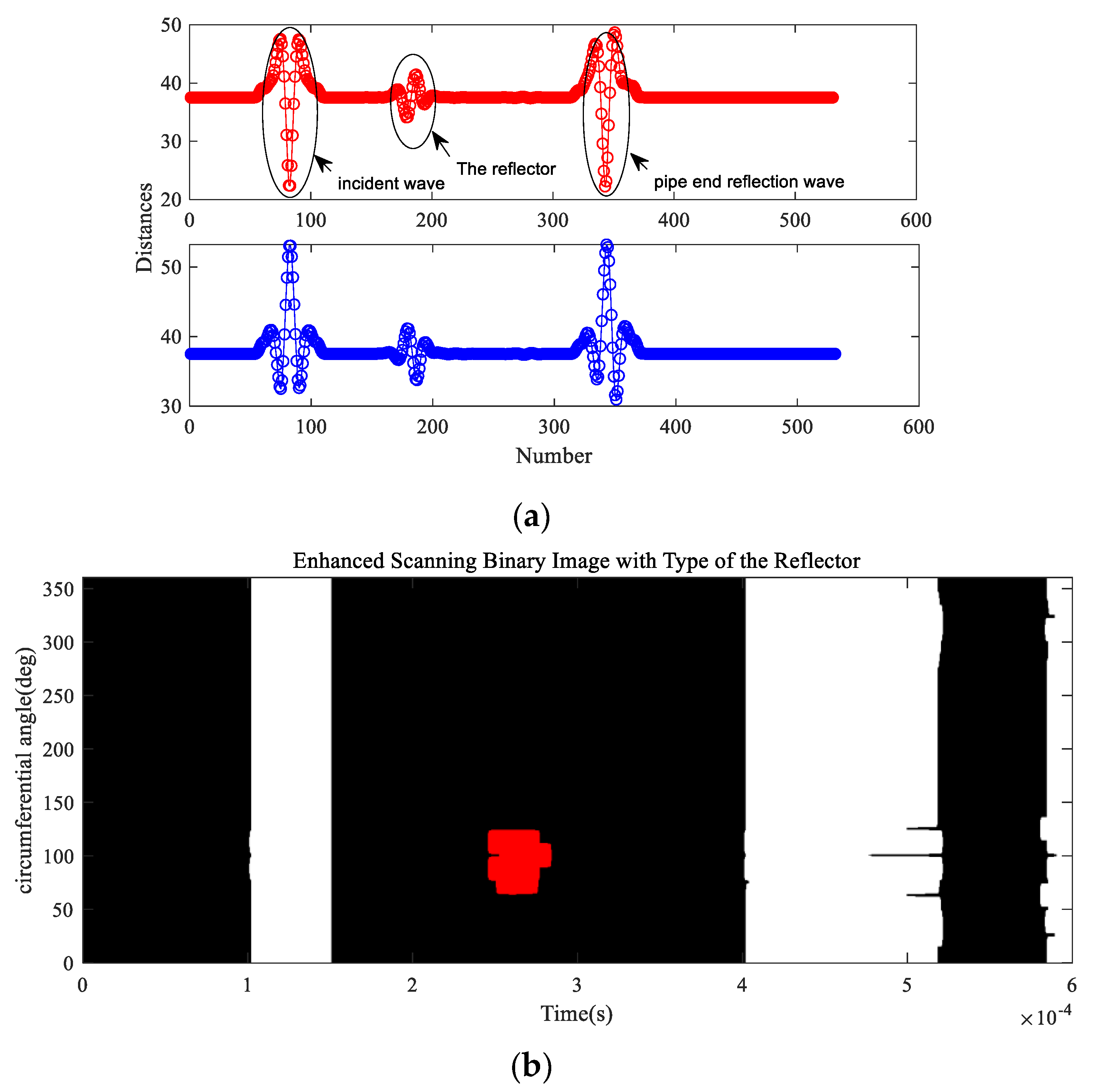
5.3.3. Investigation for Case 2
5.3.4. Investigation for Case 3
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature and Abbreviations
| Ai | Signal amplitude |
| B | Length of the pipe |
| c | Guided wave velocity |
| D | Standard Euclidian distance |
| E | Location of the excitation nodes |
| F | Location of the reflector |
| fc | Center frequency |
| g | The Gabor pulse |
| Hi*j | Data matrix of original guided wave signals |
| Im | Imaginary part of the RC |
| J | Outer radius |
| k | Wavenumber |
| p | Acoustic pressure |
| P | Maximum acoustic pressure |
| Q | Radial depth |
| r | Inner radius |
| Re | Real part of the RC |
| Si, Sr | Incident signal and reflection signal |
| The same as Si except that the phase is opposite | |
| T | Observation time |
| t0 | The time delay |
| uθ | Circumferential displacement |
| v | particle velocity |
| w | Rectangular window function |
| Numerical guided wave signal (including two Gabor pulses: x1, x2) | |
| Y | Data matrix of guided wave signals after preprocessing |
| Z | Axial length |
| α | Slope angle |
| β | Circumferential extent |
| γ | Analysis step |
| ω | Angular frequency |
| ρ | Density of the pipe |
| η | Scanning step |
| δ2 | Cross-sectional area |
| ξ | Ratio of the cross-sectional areas |
| μ | Time shift |
| θ | Guided wave phase (θIn and θRe: phases of incident wave and reflection wave) |
| τi | Standard deviation |
| σ | Pulse width |
| Ψ | Spatial distance curve |
| Γi, Γr | The dimensions of Si and Sr |
| 2D | Two-dimensional |
| 3D | Three-dimensional |
| C3D8R | Type of the elements in ABAQUS/Explicit |
| FE | Finite element |
| IN(i) | The i-th incident wave |
| L(N, m) | Longitudinal guided wave |
| NDE | Non-destructive evaluation |
| PCCM: | Pearson’s correlation coefficient method |
| RC(i) | The i-th reflection coefficient |
| RE(i) | The i-th reflected wave |
| RICR | Reliable index for the character of the reflector |
| SHM | Structure Health Monitoring |
| SNR | Signal-to noise ratio |
| T(N, m) | Torsional guided wave mode |
| TC(i) | The i-th transmission coefficient |
| TR(i) | The i-th transmission wave |
References
- Rao, J.; Ratassepp, M.; Lisevych, D.; Hamzah Caffoor, M.; Fan, Z. On-line corrosion monitoring of plate structures based on guided wave tomography using piezoelectric sensors. Sensors 2017, 17, 2882. [Google Scholar] [CrossRef] [PubMed]
- Yu, X.; Fan, Z.; Puliyakote, S.; Castaings, M. Remote monitoring of bond line defects between a composite panel and a stiffener using distributed piezoelectric sensors. Smart Mater. Struct. 2018, 27, 035014. [Google Scholar] [CrossRef]
- Lowe, M.J.S.; Alleyne, D.N.; Cawley, P. Defect detection in pipes using guided waves. Ultrasonics 1998, 36, 147–154. [Google Scholar] [CrossRef]
- Alleyen, D.N. Long range propagation of lamb wave in chemical plant pipe-work. Mater. Eval. 1997, 55, 504–508. [Google Scholar]
- Alleyne, D.N.; Pavlakovic, B.; Lowe, M.J.S.; Cawley, P. The use of guided waves for rapid screening of chemical plant pipework. J. Korean Soc. Nondestruct. Test. 2002, 22, 589–598. [Google Scholar]
- Zhang, X.; Tang, Z.; Lv, F.; Yang, K. Scattering of torsional flexural guided waves from circular holes and crack-like defects in hollow cylinders. Ndt E Int. 2017, 89, 56–66. [Google Scholar] [CrossRef]
- Alleyne, D.N.; Cawley, P. The effect of discontinuities on the long-range propagation of Lamb waves in pipes. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 1996, 210, 217–226. [Google Scholar] [CrossRef]
- Galvagni, A.; Cawley, P. The reflection of guided waves from simple supports in pipes. J. Acoust. Soc. Am. 2011, 129, 1869–1880. [Google Scholar] [CrossRef]
- Cawley, P.; Lowe, M.J.S.; Simonetti, F.; Chevalier, C.; Roosenbrand, A.G. The variation of the reflection coefficient of extensional guided waves in pipes from defects as a function of defect depth, axial extent, circumferential extent and frequency. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2002, 216, 1131–1143. [Google Scholar] [CrossRef]
- Alleyne, D.N.; Lowe, M.J.S.; Cawley, P. The reflection of guided waves from circumferential notches in pipes. J. Appl. Mech. 1998, 65, 635–641. [Google Scholar] [CrossRef]
- Løvstad, A.; Cawley, P. The reflection of the fundamental torsional guided wave from multiple circular holes in pipes. Ndt E Int. 2011, 44, 553–562. [Google Scholar] [CrossRef]
- Demma, A.; Cawley, P.; Lowe, M.; Roosenbrand, A.G.; Pavlakovic, B. The reflection of guided waves from notches in pipes: A guide for interpreting corrosion measurements. Ndt E Int. 2004, 37, 167–180. [Google Scholar] [CrossRef]
- Sargent, J.P. Corrosion detection in welds and heat-affected zones using ultrasonic Lamb waves. Insight Nondestruct. Test. Cond. Monit. 2006, 48, 160–167. [Google Scholar] [CrossRef]
- Muñoz, C.Q.G.; Marquez, F.P.G.; Lev, B.; Arcos, A. New pipe notch detection and location method for short distances employing ultrasonic guided waves. Acta Acust. United Acust. 2017, 103, 772–781. [Google Scholar] [CrossRef]
- Mu, J.; Zhang, L.; Rose, J.L. Defect circumferential sizing by using long range ultrasonic guided wave focusing techniques in pipe. Nondestruct. Test. Eval. 2007, 22, 239–253. [Google Scholar] [CrossRef]
- Li, J. On circumferential disposition of pipe defects by long-range ultrasonic guided waves. J. Press. Vessel Technol. 2005, 127, 530–537. [Google Scholar] [CrossRef]
- Wu, J.; Tang, Z.; Yang, K.; Wu, S.; Lv, F. Ultrasonic Guided Wave-Based Circumferential Scanning of Plates Using a Synthetic Aperture Focusing Technique. Appl. Sci. 2018, 8, 1315. [Google Scholar] [CrossRef]
- Zhang, X.; Tang, Z.; Lü, F.; Pan, X. Excitation of dominant flexural guided waves in elastic hollow cylinders using time delay circular array transducers. Wave Motion 2016, 62, 41–54. [Google Scholar] [CrossRef]
- Alleyne, D.N.; Pavlakovic, B.; Lowe, M.J.S.; Cawley, P. Rapid, Long Range Inspection of Chemical Plant Pipework Using Guided Waves. Key Eng. Mater. 2004, 270–273, 434–441. [Google Scholar] [CrossRef]
- Auld, B.A.; Green, R.E. Acoustic Fields and Waves in Solids: Two Volumes. Phys. Today 1974, 27, 63. [Google Scholar] [CrossRef]
- Gabor, D. Theory of communication. J. Inst. Electr. Eng. Part III Radio Commun. Eng. 1946, 93, 429–457. [Google Scholar] [CrossRef]
- Zhu, W. An FEM simulation for guided elastic wave generation and reflection in hollow cylinders with corrosion defects. J. Press. Vessel. Technol. 2001, 124, 108–117. [Google Scholar] [CrossRef]
- Moser, F.; Jacobs, L.J.; Qu, J. Modeling elastic wave propagation in waveguides with the finite element method. Ndt E Int. 1999, 32, 225–234. [Google Scholar] [CrossRef]
- Hayashi, T.; Kawashima, K.; Sun, Z.; Rose, J.L. Analysis of flexural mode focusing by a semianalytical finite element method. J. Acoust. Soc. Am. 2003, 113, 1241–1248. [Google Scholar] [CrossRef] [PubMed]
- Gravenkamp, H.; Birk, C.; Song, C. Simulation of elastic guided waves interacting with defects in arbitrarily long structures using the scaled boundary finite element method. J. Comput. Phys. 2015, 295, 438–455. [Google Scholar] [CrossRef]
- Zuo, P.; Yu, X.; Fan, Z. Numerical modeling of embedded solid waveguides using SAFE-PML approach using a commercially available finite element package. Ndt E Int. 2017, 90, 11–23. [Google Scholar] [CrossRef]
- Zuo, P.; Zhou, Y.; Fan, Z. Numerical studies of nonlinear ultrasonic guided waves in uniform waveguides with arbitrary cross sections. AIP Adv. 2016, 6, 075207. [Google Scholar] [CrossRef]
- Abaqus Analysis User’s Guide. Version 6.16. Available online: https://www.3ds.com/products-services/simulia/products/abaqus/ (accessed on 12 November 2019).
- Rose, J.L. Ultrasonic Guided Waves in Solid Media; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar]



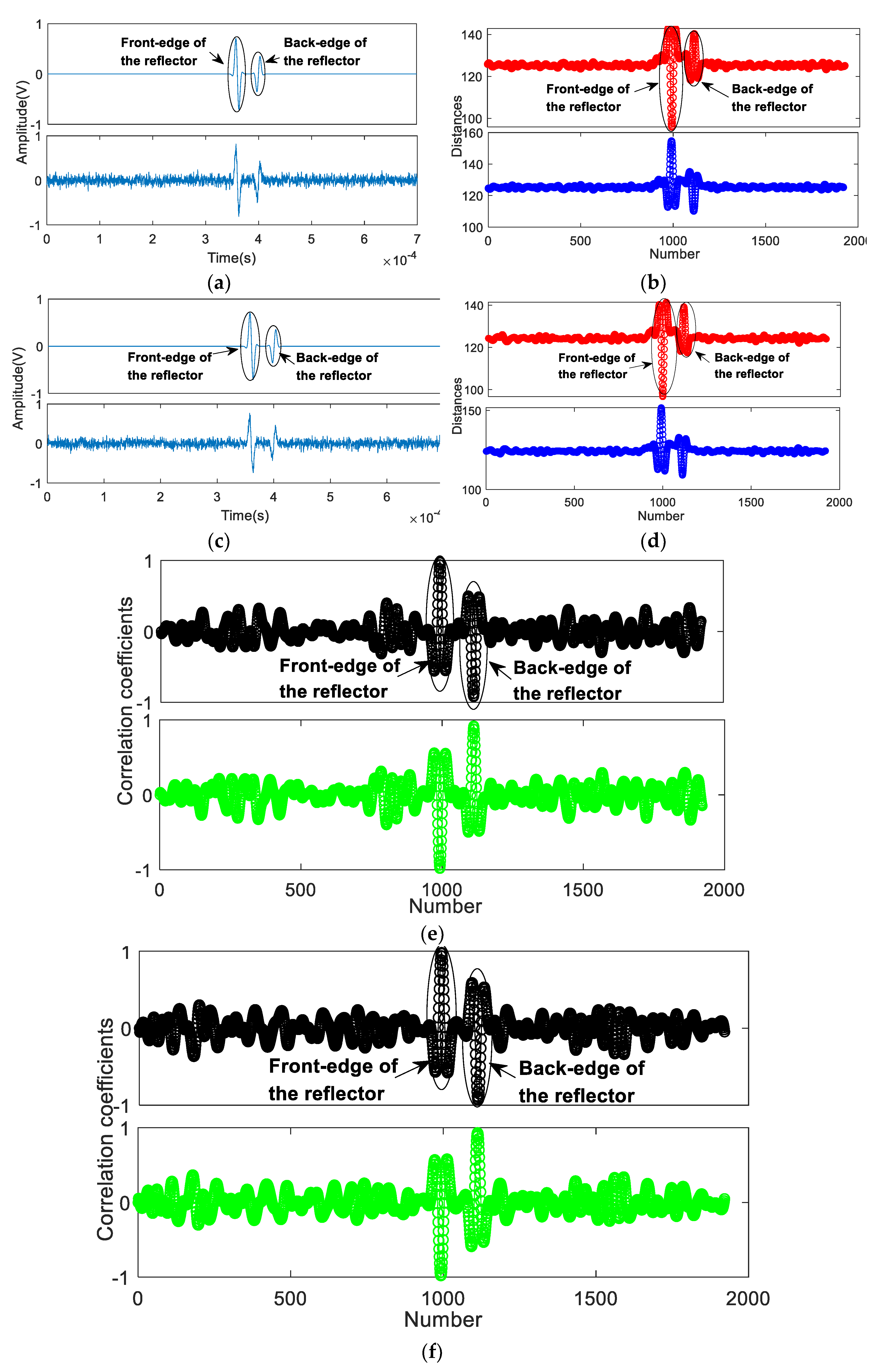
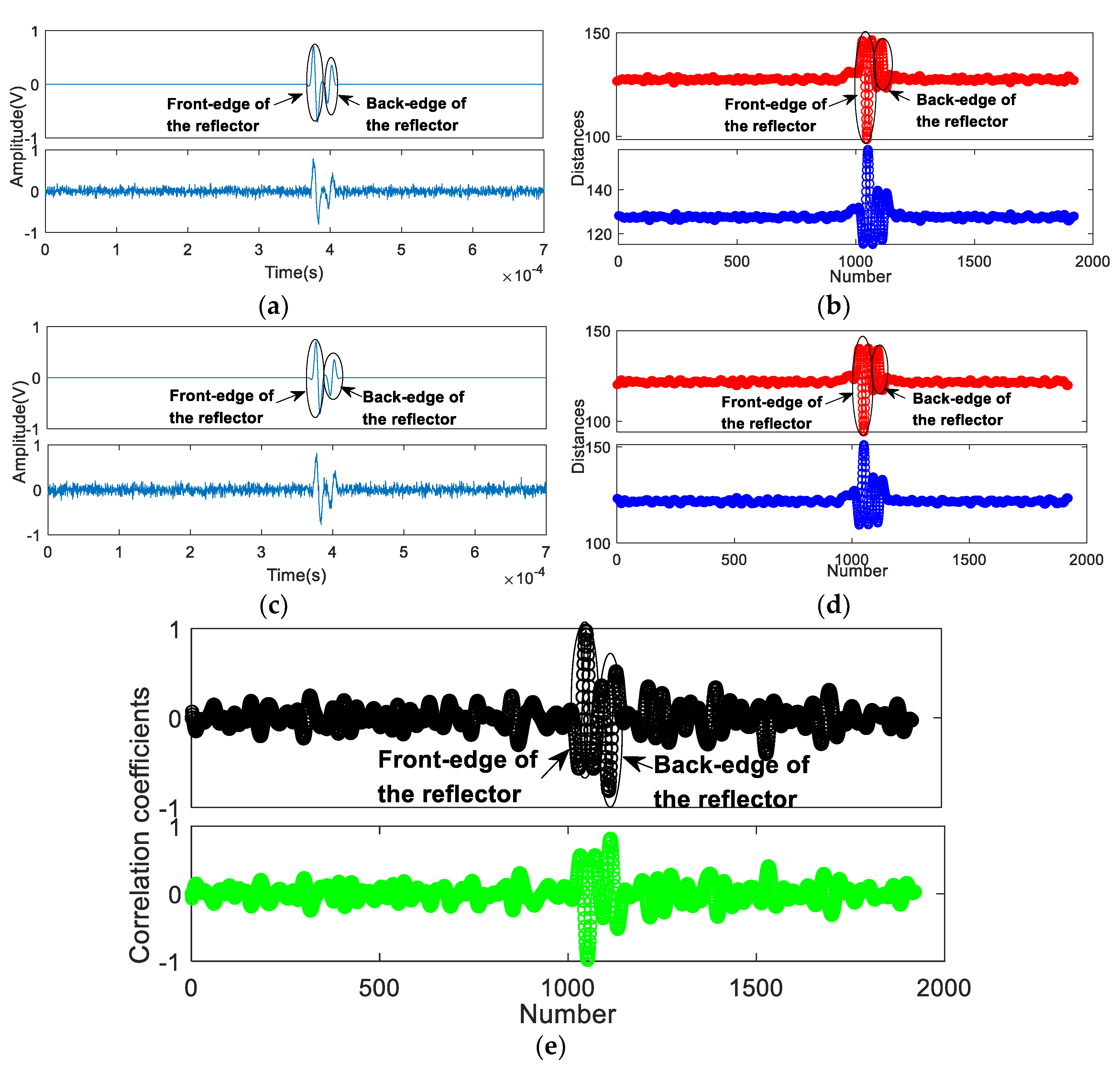
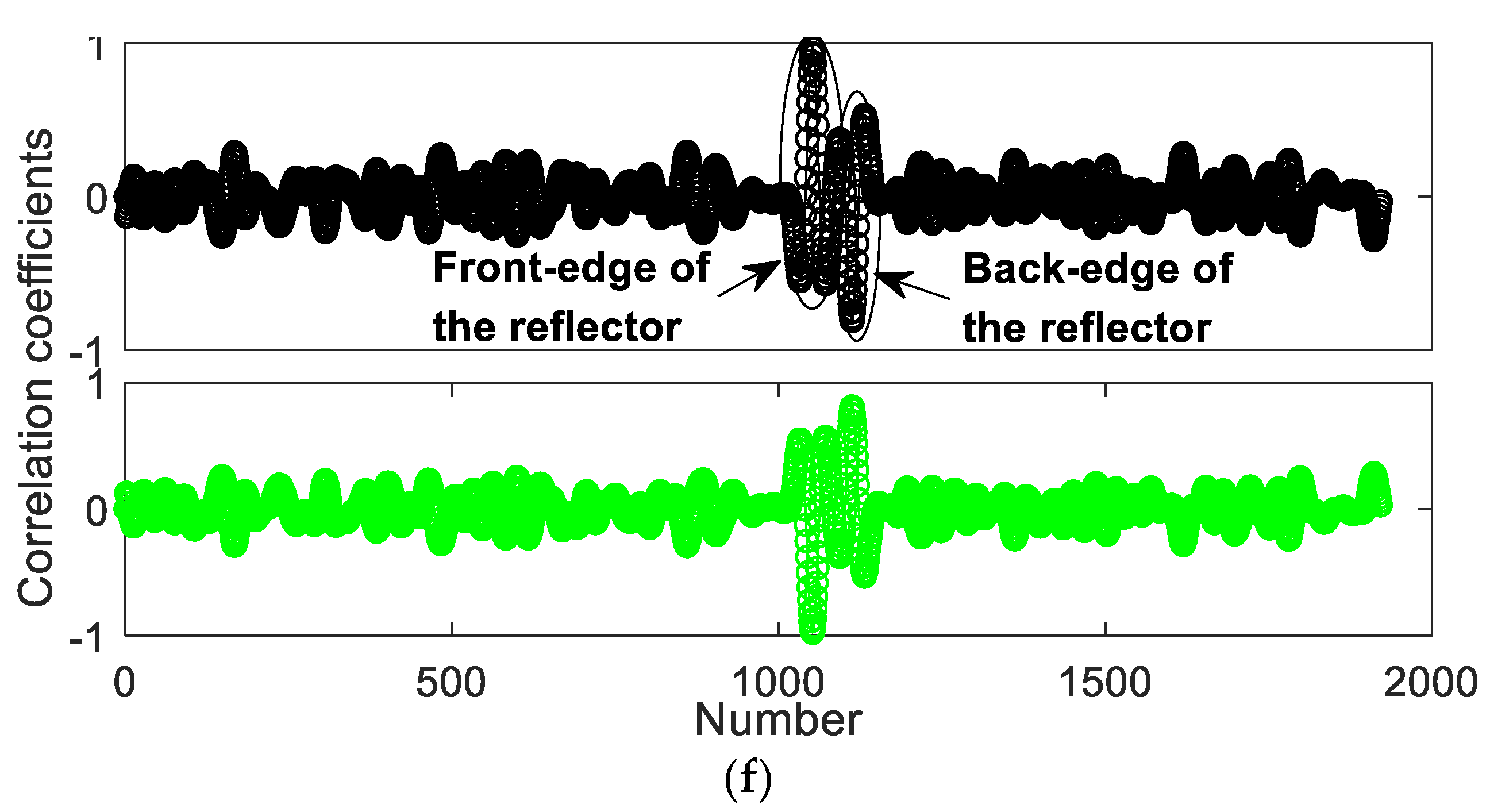


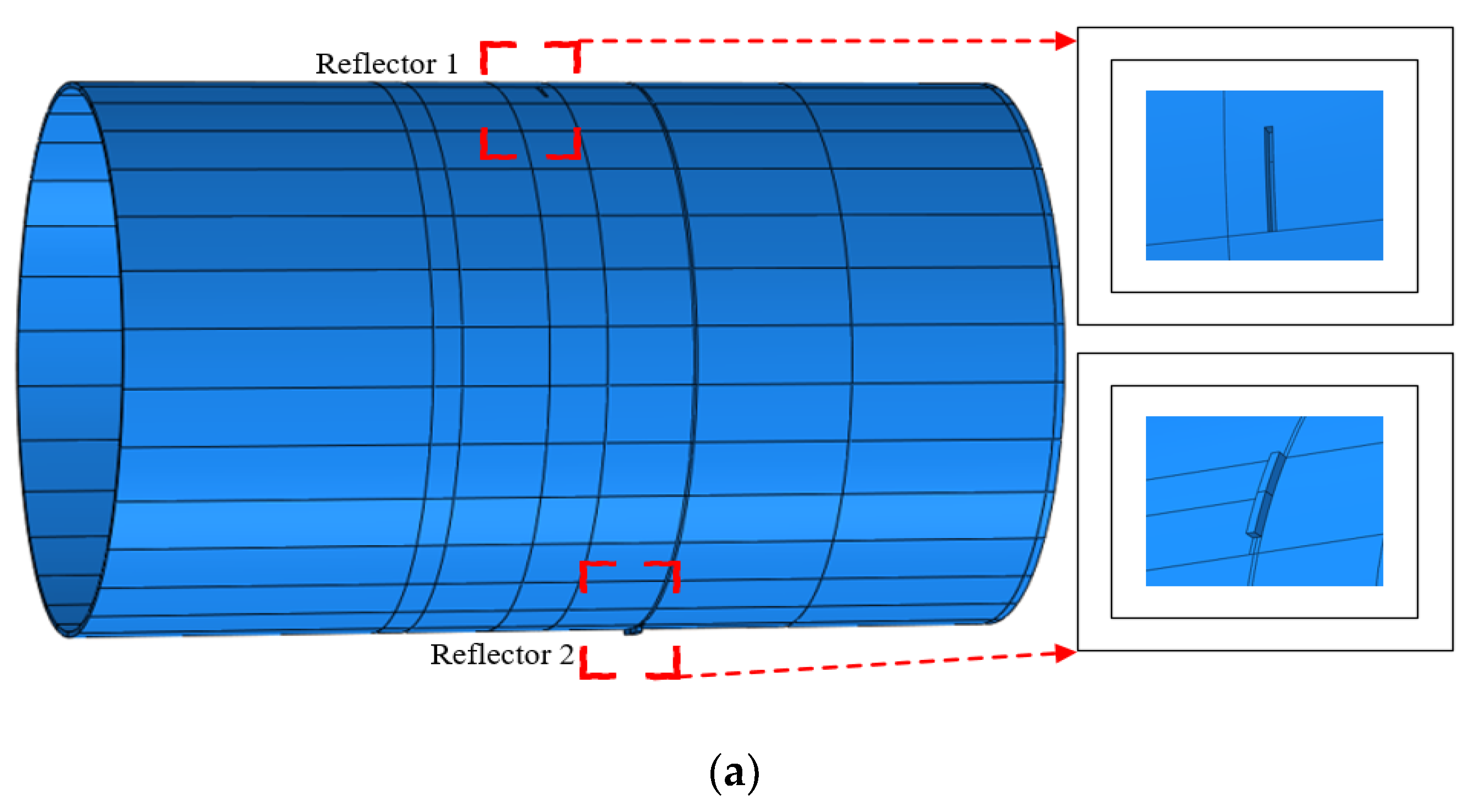
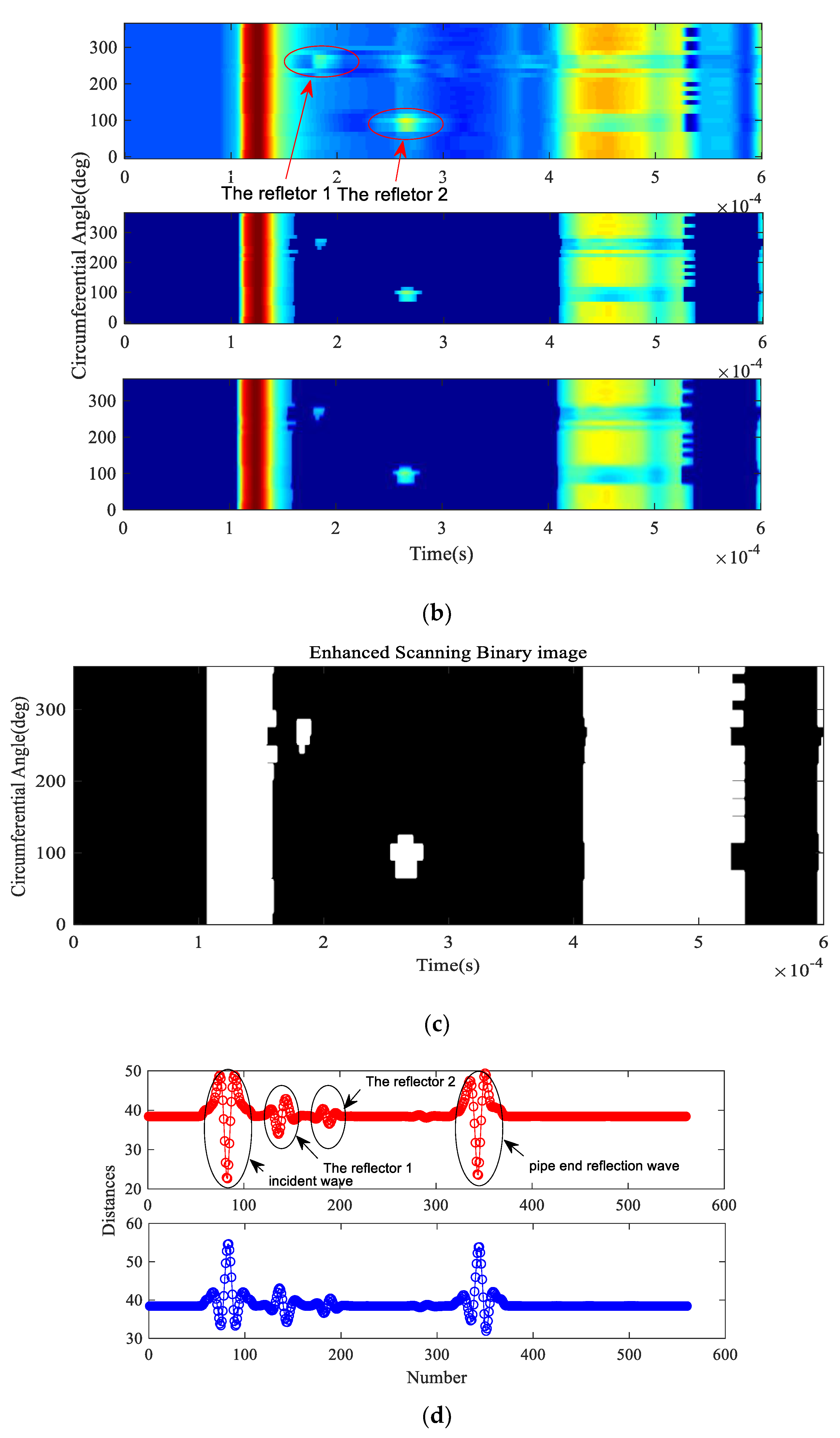

| Pulses | Ai | σ | u (s) | fc (kHz) | θ (rad) |
|---|---|---|---|---|---|
| 1 | 4.0 × 10−6 | 3.6 × 10−4 | 64 | π/2 | |
| 0.5 | 4.0 × 10−6 | 4.0 × 10−4 | 64 | −π/2 |
| Pulses | Ai | σ | u (s) | fc (kHz) | θ (rad) |
|---|---|---|---|---|---|
| 1 | 4.0 × 10−6 | 3.8 × 10−4 | 64 | ||
| 0.5 | 4.0 × 10−6 | 4.0 × 10−4 | 64 |
| Category | Outer Radius (J)/mm | Inner Radius (r)/mm | Axial Length (Z)/mm | Circumferential Extent β/deg | Slope Angle α/deg | Depth (Q)/mm | Length (B)/mm | Length (F)/mm | Density Kg/m3 | Distance (E)/mm | Poisson’s Ratio | Young’s Modulus/GPa | Reflector’s Type |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Case 1 | 204 | 200 | 2 | 5 | 90 | 2 | 650 | 390 | 7800 | 230 | 0.28 | 210 | Defect feature (Model 1) |
| Case 2 | 204 | 200 | 2 | 5 | 120 | 2 | 650 | 390 | 7800 | 230 | 0.28 | 210 | Defect feature (Model 2) |
| Case 3 | 204 | 200 | 4 | 10 | 90 | 4 | 650 | 390 | 7800 | 230 | 0.28 | 210 | Geometric feature (Model 3) |
| 2 | 8 | 90 | 318 | Defect feature (Model 1) |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, W.; Tang, Z.; Lv, F.; Zheng, Y.; Zhang, P.; Chen, X. Numerical Investigation of Locating and Identifying Pipeline Reflectors Based on Guided-Wave Circumferential Scanning and Phase Characteristics. Appl. Sci. 2020, 10, 1799. https://doi.org/10.3390/app10051799
Liu W, Tang Z, Lv F, Zheng Y, Zhang P, Chen X. Numerical Investigation of Locating and Identifying Pipeline Reflectors Based on Guided-Wave Circumferential Scanning and Phase Characteristics. Applied Sciences. 2020; 10(5):1799. https://doi.org/10.3390/app10051799
Chicago/Turabian StyleLiu, Weixu, Zhifeng Tang, Fuzai Lv, Yang Zheng, Pengfei Zhang, and Xiangxian Chen. 2020. "Numerical Investigation of Locating and Identifying Pipeline Reflectors Based on Guided-Wave Circumferential Scanning and Phase Characteristics" Applied Sciences 10, no. 5: 1799. https://doi.org/10.3390/app10051799
APA StyleLiu, W., Tang, Z., Lv, F., Zheng, Y., Zhang, P., & Chen, X. (2020). Numerical Investigation of Locating and Identifying Pipeline Reflectors Based on Guided-Wave Circumferential Scanning and Phase Characteristics. Applied Sciences, 10(5), 1799. https://doi.org/10.3390/app10051799





