Plasmonics Induced Multifunction Optical Device via Hoof-Shaped Subwavelength Structure
Abstract
:1. Introduction
2. Design and Modeling
3. Results and Discussions
4. Plasmonic Optical Devices Based on Periodic Hoof-Shaped Arrays
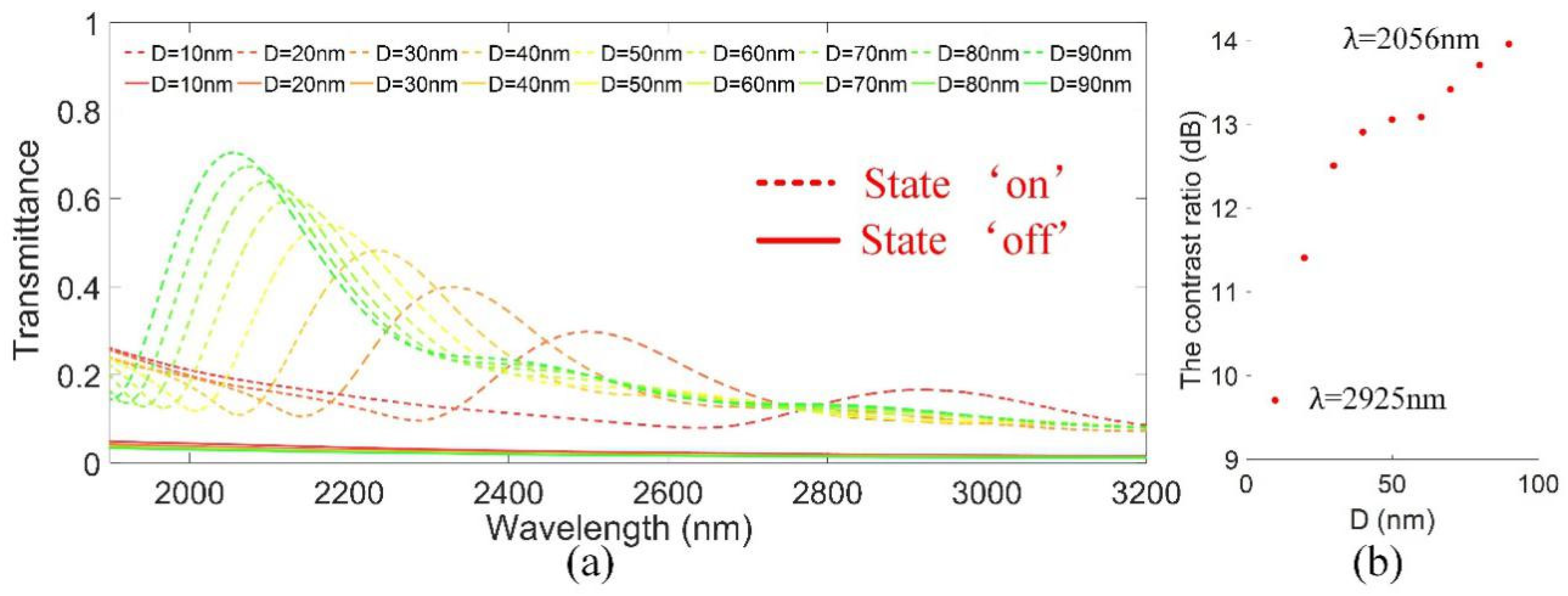
4.1. Design of Plasmonic Optical Switch
4.2. Design of Plasmonic Optical Splitter
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Ghaemi, H.F.; Thio, T.; Grupp, D.E.; Ebbesen, T.W.; Lezec, H.J. Surface plasmons enhance optical transmission through subwavelength holes. Phys. Rev. B 1998, 58, 6779–6782. [Google Scholar] [CrossRef] [Green Version]
- Barnes, W.L.; Dereux, A.; Ebbesen, T.W. Surface plasmon subwavelength Optics. Nature 2003, 424, 824–830. [Google Scholar] [CrossRef] [PubMed]
- Lin, L.; Roberts, A. Light transmission through nanostructured metallic films: Coupling between surface waves and localized resonances. Opt. Express 2011, 19, 2626–2633. [Google Scholar] [CrossRef] [PubMed]
- Garcia, V.F.J.; Moreno, L.M.; Ebbesen, T.W.; Kuipers, L. Light passing through subwavelength apertures. Rev. Mod. Phys. 2010, 82, 729–787. [Google Scholar] [CrossRef] [Green Version]
- Min, C.; Wang, P.; Chen, C.; Deng, Y.; Lu, Y.; Ming, H.; Ning, T.; Zhou, Y.; Yang, G. All-optical switching in subwavelength metallic grating structure containing nonlinear optical materials. Opt. Lett. 2008, 8, 869–871. [Google Scholar] [CrossRef]
- Wang, J.; Jiang, X.X.; Xia, L.P.; Tang, L.L.; Hu, S.; Lv, J.T.; Zhao, H.Q.; Si, G.Y.; Shi, R.Y. Fabrication and optical measurement of double overlapped annular apertures. Opt. Mater. 2016, 60, 13–16. [Google Scholar] [CrossRef]
- Zhang, X.N.; Liu, G.Q.; Liu, Z.Q.; Cai, Z.J.; Hu, Y.; Liu, X.S.; Fu, G.L.; Gao, H.G.; Huang, S. Effects of compound rectangular subwavelength hole arrays on enhancing optical transmission. IEEE Photonics J. 2015, 7, 4500408–4500416. [Google Scholar] [CrossRef]
- Tang, Y.; Vlahovic, B.; Brady, D.J. Metallic nano-structures for polarization independent multi-spectral filters. Nanoscale Res. Lett. 2011, 6, 394–399. [Google Scholar] [CrossRef] [Green Version]
- Balci, S.; Balci, O.; Kakenov, N.; Atar, F.B.; Kocabas, C. Dynamic tuning of plasmon resonance in the visible using graphene. Opt. Lett. 2016, 41, 1241–1244. [Google Scholar] [CrossRef] [Green Version]
- Song, S.C.; Ma, X.L.; Pu, M.B.; Li, X.; Guo, Y.H.; Gao, P.; Luo, X.G. Tailoring active color rending and multiband photodetection in a vanadium-dioxide-based metamaterial absorber. Photonics Res. 2018, 6, 492–497. [Google Scholar] [CrossRef] [Green Version]
- Wen, K.; Luo, X.Q.; Chen, Z.Y.; Zhu, W.H.; Guo, W.; Wang, X.L. Enhanced Optical Transmission Assisted Near-Infrared Plasmonic Optical Filter via Hybrid Subwavelength Structures. Plasmonics 2019, 14, 1649–1657. [Google Scholar] [CrossRef]
- Rezaei, S.D.; Ho, J.; Naderi, A.; Yaraki, M.T.; Wang, T.; Dong, Z.; Ramakrishna, S.; Yang, J.K.W. Tunable, cost-effective, and scalable structural colors for sensing and consumer products. Adv. Opt. Mater. 2019, 7, 1900735–1900742. [Google Scholar] [CrossRef]
- Wang, B.; Dong, F.L.; Li, Q.T.; Yang, D.C.; Sun, W.; Chen, J.J.; Song, Z.W.; Xu, L.H.; Chu, W.G.; Xiao, Y.F.; et al. Visible-frequency dielectric metasurfaces for multiwavelength achromatic and highly dispersive holograms. Nano Lett. 2016, 16, 5235–5240. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Fan, Q.; Zhang, S.; Zhang, Z.; Zhang, H.; Liang, Y.; Cao, X.; Ting, X. Ultra-thin plasmonic color filters incorporating free-standing resonant membrane waveguides with high transmission efficiency. Appl. Phys. Lett. 2017, 110, 31110–31114. [Google Scholar] [CrossRef]
- Chen, B.H.; Wu, P.C.; Su, V.C.; Lai, Y.C.; Chu, C.H.; Lee, I.C.; Chen, J.W.; Chen, Y.H.; Lan, Y.C.; Kuan, C.H.; et al. GaN metalens for pixel-level full-color routing at visible light. Nano Lett. 2017, 17, 6345–6352. [Google Scholar] [CrossRef]
- Guo, T.B.; Evans, J.; Wang, N.; He, S.L. Monolithic chip-scale structural color filters fabricated with simple UV lithography. Opt. Express 2019, 27, 21646–21651. [Google Scholar] [CrossRef]
- Shu, F.; Yu, F.; Peng, R.; Zhu, Y.; Xiong, B.; Fan, R.; Wang, Z.; Liu, Y.; Wang, M. Dynamic plasmonic color generation based on phase transition of vanadium dioxide. Adv. Opt. Mater. 2018, 6, 1700939–1700948. [Google Scholar] [CrossRef]
- Kuznetsov, A.I.; Miroshnichenko, A.E.; Brongersma, M.L.; Kivshar, Y.S.; Luk’yanchuk, B. Optically resonant dielectric nanostructures. Science 2016, 354, 2472–2479. [Google Scholar] [CrossRef] [Green Version]
- Zhou, H.; Zhen, B.; Hsu, C.W.; Miller, O.D.; Johnson, S.G.; Joannopoulos, J.D.; Soljacic, M. Perfect single-sided radiation and absorption without mirrors. Optica 2016, 3, 1079–1086. [Google Scholar] [CrossRef]
- Taflove, A.; Hagness, S. Computational Electrodynamics: The Finite Difference Time-Domain Method; Artech House: Norwood, MA, USA, 1995. [Google Scholar]
- Lalanne, P.; Besbes, M.; Hugonin, J.P.; Haver, S.V.; Janssen, O.T.A.; Nugrowati, A.M.; Xu, M.; Pereira, S.F.; Urbach, H.P.; Nes, A.S.V.D.; et al. Numerical analysis of a slit-groove diffraction problem. J. Eur. Opt. Soc. Rapid 2007, 2, 7022–7039. [Google Scholar]
- Ruan, Z.C.; Qiu, M. Enhanced transmission through periodic arrays of subwavelength holes: The role of localized waveguide resonances. Phys. Rev. Lett. 2006, 96, 233901–233905. [Google Scholar] [CrossRef] [PubMed]
- Fan, S.; Suh, W.; Joannopoulos, J.D. Temporal coupled-mode theory for the Fano resonance in optical resonators. J. Opt. Soc. Am. A 2003, 20, 569–572. [Google Scholar] [CrossRef] [Green Version]
- Suh, W.; Wang, Z.; Wang, Z.; Fan, S.H. Temporal coupled-mode theory and the presence of non-orthogonal modes in lossless multimode cavities. IEEE J. Quant. 2004, 40, 1511–1518. [Google Scholar]
- Shi, H.F.; Wang, C.T.; Du, C.L.; Luo, X.G.; Dong, X.C.; Gao, H.T. Beam manipulating by metallic nano-slits with variant widths. Opt. Express 2005, 13, 6815–6820. [Google Scholar] [CrossRef]
- Bliokh, K.Y.; Bliokh, Y.P.; Freilikher, V.; Savel’ev, S.; Nori, F. Unusual resonators: Plasmonics, metamaterials, and random media. Rev. Mod. Phys. 2008, 80, 1201–1213. [Google Scholar] [CrossRef] [Green Version]
- Suckling, J.R.; Hibbins, A.P.; Lockyear, M.J.; Preist, T.W.; Sambles, J.R. Finite conductance governs the resonance transmission of thin metal slits at microwave frequencies. Phys. Rev. Lett. 2004, 92, 147401–147404. [Google Scholar] [CrossRef] [PubMed]
- Cheng, C.; Chen, J.; Wu, Q.Y.; Ren, F.F.; Xu, J.; Fan, Y.X.; Wang, H.T. Controllable electromagnetic transmission based on dual-metallic grating structures composed of subwavelength slits. Appl. Phys. Lett. 2007, 91, 111111–111113. [Google Scholar] [CrossRef]
- Chen, F.; Yao, D.Z. Tunable multiple all-optical switch based on multi-nanoresonator-coupled waveguide systems containing Kerr material. Opt. Commun. 2014, 312, 143–147. [Google Scholar] [CrossRef]
- Kekatpure, R.D.; Barnard, E.S.; Cai, W.H.; Brongersma, M.L. Phase-coupled plasmon-induced transparency. Phys. Rev. Lett. 2010, 104, 243902–243905. [Google Scholar] [CrossRef]
- Bai, Q.; Liu, C.; Chen, J.; Chen, C.; Kang, M.; Wang, H.T. Tunable slow light in semiconductor metamaterial in a broad terahertz regime. J. Appl. Phys. 2010, 107, 93104–93111. [Google Scholar]
- Fu, Q.H.; Zhang, F.L.; Fan, Y.C.; Dong, J.J.; Cai, W.Q.; Zhu, W.; Chen, S.; Yang, R.S. Weak coupling between bright and dark resonators with electrical tunability and analysis based on temporal coupled-mode theory. Appl. Phys. Lett. 2017, 110, 221905–221909. [Google Scholar] [CrossRef]
- Jing, C.; Qian, Z.; Cheng, P.; Chao, J.T.; Xue, Y.S.; Li, C.D.; Gun, S.P. Optical cavity-enhanced localized surface plasmon resonance for high-quality sensing. IEEE Photonics Technol. Lett. 2018, 30, 728–731. [Google Scholar]
- Guo, Y.; Yan, L.; Pan, W.; Luo, B.; Wen, K.; Guo, Z.; Li, H.; Luo, X. A plasmonic filter based on slot cavity. Opt. Express 2011, 19, 13831–13838. [Google Scholar] [CrossRef] [PubMed]





© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wen, K.; Zhang, Z.; Jiang, X.; He, J.; Yang, J. Plasmonics Induced Multifunction Optical Device via Hoof-Shaped Subwavelength Structure. Appl. Sci. 2020, 10, 2713. https://doi.org/10.3390/app10082713
Wen K, Zhang Z, Jiang X, He J, Yang J. Plasmonics Induced Multifunction Optical Device via Hoof-Shaped Subwavelength Structure. Applied Sciences. 2020; 10(8):2713. https://doi.org/10.3390/app10082713
Chicago/Turabian StyleWen, Kui, Zhaojian Zhang, Xinpeng Jiang, Jie He, and Junbo Yang. 2020. "Plasmonics Induced Multifunction Optical Device via Hoof-Shaped Subwavelength Structure" Applied Sciences 10, no. 8: 2713. https://doi.org/10.3390/app10082713
APA StyleWen, K., Zhang, Z., Jiang, X., He, J., & Yang, J. (2020). Plasmonics Induced Multifunction Optical Device via Hoof-Shaped Subwavelength Structure. Applied Sciences, 10(8), 2713. https://doi.org/10.3390/app10082713






