Wave Theory of Seismic Resistance of Underground Pipelines

Featured Application

Main gas and oil pipelines are critical elements of the world’s infrastructure. The integrity of pipelines during earthquakes is a subject of intensive research. At present, underground pipelines’ strength to the effect of seismic loads (seismic resistance) is calculated incorrectly. The existing methods do not consider seismic (dynamic) pressure, i.e., the stress normal to the pipeline’s outer surface arising from the propagation of a seismic wave in the soil. This leads to an incorrect determination of the pipeline’s stress; the longitudinal stress calculations leads to errors of 100% or more. A potential application of the results obtained is the calculation of seismic stability of underground pipelines.

Abstract

The object of the research is an underground straight horizontal pipeline subjected to seismic impact. The research method was analytical. The results were compared with the experimental results of other authors and computer calculations. It was shown that the main disadvantage of the dynamic theory of seismic resistance of underground pipelines is the neglect of the dynamic stress state in soil under seismic wave propagation. The next drawback of the dynamic theory is an inaccurate, approximate accounting for the displacement of the soil medium to which the underground pipeline is embedded. The complete interaction process includes the stages of nonlinear changes in the interaction force (the friction force) by manifesting its peak value and the Coulomb friction. The contact layer of soil undergoes shear deformations until complete structural destruction of the soil contact layer. The interaction force is the friction force, and its peak value does not appear. The seismic resistance of underground pipelines should be considered based on the theory of propagating seismic waves in a soil medium and the interaction of seismic waves with underground pipelines, i.e., based on the wave theory of seismic resistance of underground pipelines. A one-dimensional coupled problem of seismic resistance of underground pipelines under seismic impacts was posed based on the wave theory. An algorithm and a program for the numerical solution of the stated wave problems were developed using the method of characteristics and the method of finite differences. An analysis of the laws of interaction of underground pipelines with soil under seismic influences shows that it is necessary to use in the calculations the laws of interaction that account for the complete interaction processes observed in experiments. The analysis of the obtained numerical solutions and the posed coupled problems of the wave theory of seismic resistance of underground pipelines show the occurrence mechanisms of longitudinal stresses in underground pipelines under seismic influences. The results of calculations stated that an account for the dynamic stress normal to the underground pipeline’s outer surface leads to multiple increases in longitudinal stress in the underground pipeline. This multiple increase is due to the transformation of the interaction force into an active frictional force, resulting from a greater strain in soil than the one in the underground pipeline. Based on the analysis results, a theory of the seismic wave propagation process in an underground pipeline and surrounding soil was proposed.

1. Introduction

Underground pipelines that transport oil, gas, oil products, water, and other liquid and gas products are very important engineering communications worldwide [1,2,3]. Some of these underground communications are built and operated in earthquake-prone areas [2,3,4,5]. This circumstance requires the reliable provision of seismic safety of underground pipelines [1,2,3].

Seismic safety or seismic stability (the strength under seismic influence) of underground pipelines began to be intensively studied since the 50 s of the last century [6,7].

It is necessary to distinguish the destruction of pipelines caused by seismic effects from the effects of soil inhomogeneities. In this publication, we will assume soil uniformity. Non-uniform soil support conditions resulting from the erosion of the surrounding soil by the water escaping from leaks may consider in [8]. The article also does not consider the impact of seismic-induced soil liquefaction or landslides on pipelines. The failure of pipelines due to seismic seismic-induced soil liquefaction is considered, for example, in [9,10,11], and seismic-induced landslides are considered in [12]. Undercrossing tunneling on adjacent pipelines, which could cause pipelines to collapse [13], is also outside this article’s scope.

The theories of seismic resistance of underground pipelines are based on the data collected when examining the aftermath of destructive earthquakes [1,2,3,4,5], laboratory and field experimental studies, and the resulting facts and hypotheses [6,7,14,15].

The point of these hypotheses is as follows:

• Under strong ground motion, the underground pipeline system is destroyed; these destructions are different: the destruction in the pipeline itself, in butt joints, the pipeline buckling from the ground; soil separation from the pipeline on the surfaces of their contact, etc.;
• Seismic forces are transmitted to underground pipelines through soil. Therefore, these forces’ values directly depend on the physical–mechanical and strain properties of the soil environment surrounding the underground pipeline.
• The assumption of an infinitely long straight horizontal pipeline in homogeneous soil simplifies the solution of the problem of seismic impact on the pipeline. This assumption is used in most theoretical studies. An exception is in [11]. The unsteady wave problem of a longitudinal monochromatic wave propagation and reflection from a rigid stationary barrier solved in a one-dimensional statement.
• During earthquakes, seismic forces are transmitted to underground pipelines through soil strain, i.e., the soil deforms under seismic wave effect and forces underground pipelines to deform. The pipelines inflict the most severe damage when the route is codirectional to the seismic strain vector [15].
• In the case of strong ground motions, the amplitudes of absolute displacements of soil particles reach 0.1–0.4 m at an oscillation period T = 0.05–20 s; under soil mass vibrations together with the underground pipeline, a difference between the vibrational motion of the pipeline and soil is formed (for various reasons) in vibration amplitude and phase; a displacement of the pipeline relative to the soil is observed, i.e., a relative displacement.
• There is a critical value of the relative displacement $u_{*}$ of the pipeline cross-section, below which there is an elastic bond between the soil particles and the outer surface of the pipeline in contact with the soil. At values of the relative displacement exceeding the critical value, the elastic bond between the pipeline and the ground is ruptured [14].
• After pipeline construction, due to the cohesion phenomena, a relative shear displacement occurs in the soil layer ${\delta }_g$ thick, called the contact soil layer, between the underground pipeline and soil on their contact surfaces.
• At an increase in relative displacement value greater than $u_{*}$, the contact soil layer undergoes significant shear strain and may collapse; the soil outside the contact layer may remain intact. In the case of strain and destruction of the contact soil layer under shear stresses, its limiting value is determined based on the Coulomb law.
• The processes of relative displacements $u$ formation on the contact layer, under its elastic, elastic–plastic strain ($u<u_{*}$) and destruction ($u\ge u_{*}$), are the two stages of one process—the process of deformation of the contact soil layer before and after destruction.

The existing theories of seismic resistance of underground pipelines [1,6,7,16,17,18,19,20,21,22,23,24,25,26,27,28,29,30,31,32] partially take into account these factors, identified by numerous researchers while analyzing the consequences of severe earthquakes and conducting experimental studies of the underground pipeline interaction with soil under static and dynamic loads.

The problem of determining or assessing the seismic resistance of underground pipelines is reduced, in one way or another, to the stress determination in the pipe body by various methods [1,6,7,20,27,28,29,30,31,32]. The stress state of an underground pipe under seismic forces is rather complicated [16,17,18,19,20,21,22,23,24,25,26,27,28,29,30,31,32]. For simplicity reasons, stresses are divided into longitudinal (acting along the pipeline axis), transverse (perpendicular to the pipeline axis), bending, radial, annular ones, etc. [16,17,18,21,22,23,27,28,29,30,31,32]. Many researchers noted that the most dangerous stress is the longitudinal one [6,7,16,17,18,19,20,21,22,23,24].

It is known that the stress state of an underground pipeline under seismic effects arises as a result of its interaction with the surrounding soil environment [1,6,7,27]. Therefore, the interaction of the underground pipeline with soil is also divided into longitudinal and transverse ones. In each of the interaction types, the above factors can be detected under strong ground motions.

The longitudinal interaction of an underground pipeline with soil is simpler from a mechanical point of view and was studied in more detail. The transverse interaction of an underground pipeline with soil is more complex and has not yet been sufficiently studied. Under the transverse pipeline–soil interaction, the pipelines of a circular cross-section interact with soil ambiguously. When a circular section moves in soil, greater pressure is generated on the section’s front side than on its back side. The side surfaces of this section interact with soil in a more complex way. It has not been studied yet, how, and according to what laws, each point of the circle’s outer surface interacts with soil.

In seismic resistance calculations of underground pipelines, the most simplified schemes are usually accepted, which could be far from the real situation. In [10], the algorithm of the 3D analysis developed to solve an external boundary problem by applying the combined method based on incorporating the FEM and Somigliana’s integral formula is considered. However, such combination methods have not been applied for solving the pipeline subjected to seismic impact.

When determining longitudinal stresses in buried pipelines, the following technical theories are used.

1. A simplified theory based on the hypothesis of the equality of longitudinal strains in soil and pipeline under longitudinal seismic loads [6,7]. This theory determines the soil strain, considered an elastic half-space, under plane wave propagation; the front of the wave is perpendicular to the pipeline axis. The soil strain is determined without considering the pipeline, i.e., it is believed that the buried pipeline does not influence the soil strain formation.

In this case, the strain is

$${\epsilon }_g=\frac{v_g}{C_g}$$

(1)

where ${\epsilon }_g$ is the longitudinal soil strain along the pipeline axis, $v_g$ is the velocity of soil particles in the longitudinal direction, and $C_g$ is the velocity of longitudinal wave propagation in soil.

Further, the pipeline strain ${\epsilon }_c$ is taken to be equal to the soil strain

$${\epsilon }_c={\epsilon }_g$$

(2)

With a known value of pipeline strain ${\epsilon }_c$, the longitudinal stress of the pipeline is determined from Hooke’s law, considering the pipeline as an elastic body

$${\sigma }_c={\epsilon }_c{E}_c$$

(3)

where $E_c$ is the modulus of elasticity of the pipeline material.

The seismic strength of the pipeline is estimated from the value of longitudinal stress in the pipeline material.

It should be noted here that the simplified theory formed the basis of the normative methods for calculating seismic resistance of main underground pipelines and other types of pipelines. Many correction factors were introduced into Equation (3), and several parameters were replaced for convenience when used by designers-engineers.

However, hypothesis (2), underlying the simplified theory, is implausible. On the other hand, in this case, the maximum possible longitudinal stress of the pipeline is obtained by calculation. Hypothesis (2) provides a greater margin of safety for the buried pipeline. The equality of pipeline strain and soil strain underlies the first, initial stage in developing the theory of seismic resistance of underground pipelines.

2. The second stage in the theory development led to the creation of a dynamic theory of seismic resistance of underground pipelines (in the middle of the twentieth century). The basis of this theory is the forces of pipeline interaction with soil, which arise due to the difference in strains in the pipeline and soil [1,5,6,7,16,17,18,19,20,21,22,23,24]. In the dynamic theory, hypothesis (2) is considered false. Here, the seismic load on an underground pipeline acts through soil and is determined under longitudinal interaction of the pipeline with soil by the following relation

$$\tau =K_{x}u$$

(4)

where $\tau$ is the shear stress on the contact surface of the pipeline with soil (MPa); $K_x$ is the coefficient of longitudinal interaction or the stiffness coefficient of soil particles connection with the outer surface of the pipeline (MPa/m); $u=u_g-u_c$ is the relative displacement (m), $u_g$ is the absolute longitudinal displacement of soil in the direction of the pipeline axis (m), and $u_c$ is the absolute longitudinal displacement of the pipeline.

Further, taking into account (4) or its more complicated versions [14], the differential equations of the pipeline’s longitudinal motion are compiled in one-dimensional, two-dimensional, and three-dimensional statements. The obtained equations of motion are solved with the corresponding closing equations of deformation of the pipeline material and the equations of continuum mechanics with certain boundary and initial conditions.

In the dynamic theory, in most cases, seismic resistance problems are reduced to stationary problems of the theory of vibrations. The use of the stationary vibration theory is due to the desire to obtain simple engineering solutions for seismic resistance of underground pipelines. The problems of transverse, bending, and torsional vibrations of underground pipelines under seismic loads are considered similarly. The main difference between the problems of the dynamic theory of seismic resistance of underground pipelines and the conventional theory of vibrations is the presence of an interaction force of the type (4) in the equation of motion. Therefore, the reliability of the results obtained according to the dynamic theory is completely determined by the interaction law’s reliability (4) or by similar, more complex laws. On the whole, dynamic theory was and still is a significant achievement in developing the theory of seismic resistance of underground pipelines.

Based on the foregoing, this research aims to assess the advantages and disadvantages of the dynamic theory and the reliability of its solutions, and to develop an alternative theory of seismic resistance of underground pipelines.

Based on the goal, the tasks of this research are:

• To conduct a critical analysis of the dynamic theory grounds, to determine its disadvantages and advantages;
• To determine the ways of further development of the dynamic theory of seismic resistance of underground pipelines;
• To determine the grounds of an alternative wave theory of seismic resistance of underground pipelines and its advantages and disadvantages.

2. Materials and Methods

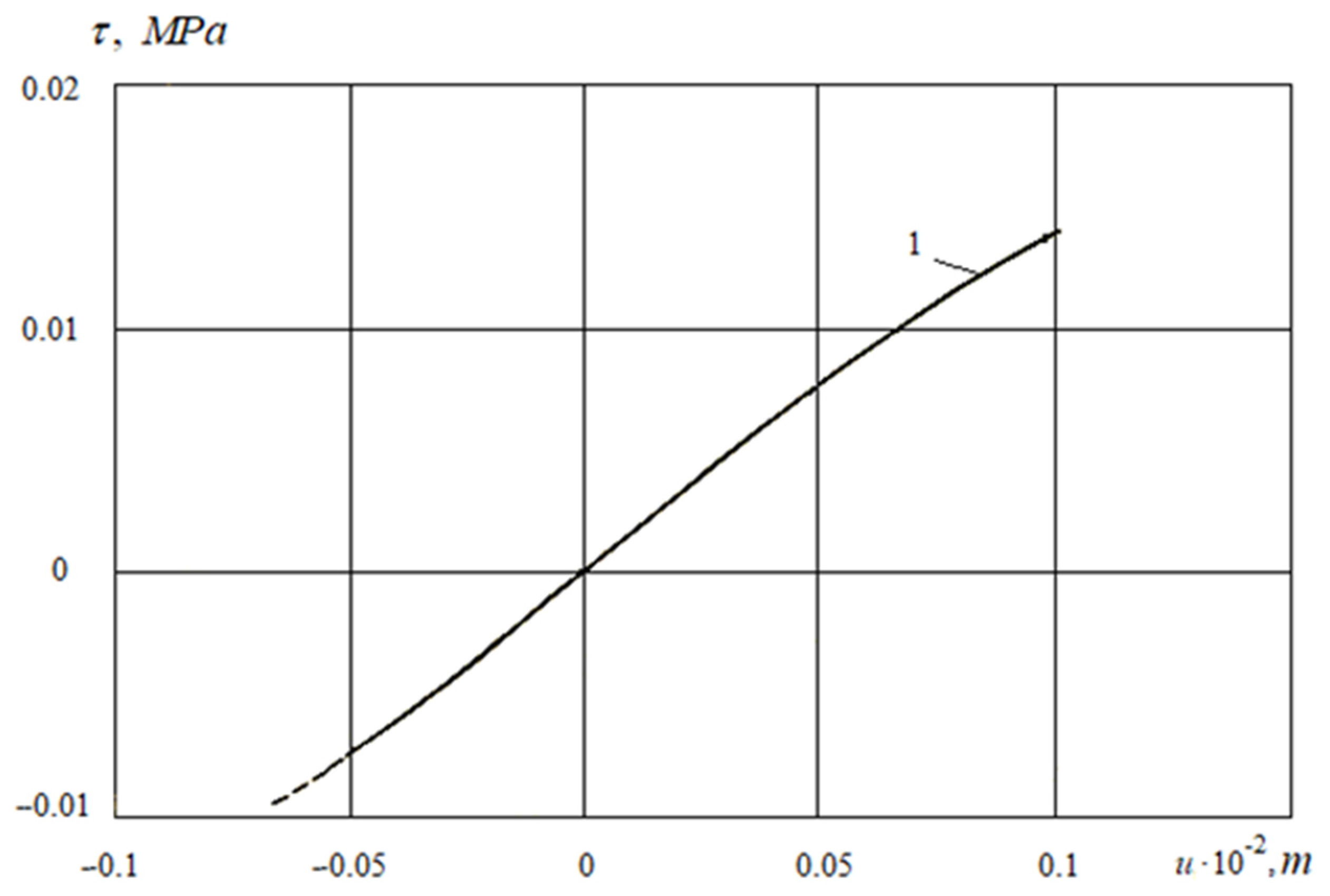
2.1. Interaction Laws

Consider the laws of longitudinal interaction. The seismic load acting on an underground pipeline along an extended pipeline is considered known in the dynamic theory, taken as a function $u_g\left(x,t\right)$, and enters Equation (4). Since the length of a longitudinal seismic wave can be significant (from hundreds of meters to a kilometer), in most cases, soil displacement is considered depending on time t only (not on spatial coordinate $x$), in the form of a function [33,34]

$$u_g=u_{m}sin(\omega t)$$

(5)

or

$$u_g=u_m\cos (\omega t)$$

(6)

where $u_m$ is the amplitude of longitudinal soil displacement and $\omega$ is the oscillation frequency of seismic wave.

The value of $u_m$ is determined from seismic data of instrumental records of ground motion during various earthquakes. Depending on the earthquake intensity and soil type, its value reaches 0.1–0.4 m. When $u_g\left(x,t\right)$ is known, the displacement of the pipeline sections $u_c$ is determined from one-dimensional equations of the pipeline vibration; then, longitudinal strains and stresses are determined along the pipe.

In this statement of the problem, when determining the longitudinal seismic stresses in an underground pipeline under longitudinal seismic loads, the stress value depends on two parameters: soil displacement and mechanical properties of soil. The latter parameter is taken into account through the coefficient $K_x$ in (4). The value of $K_x$ is determined experimentally from the results of corresponding experiments [14]. Usually $K_x$ is called the coefficient of interaction [19,20,35,36].

According to (4), the coefficient $K_x$ has the dimension MPa/m and physically means the rigid bond between soil particles and the pipeline’s outer surface in contact with soil. This rigid bond takes place in the longitudinal, transverse, and other directions.

It should be noted that there is a strict application area for the law or model (4) [14]. Equation (4) is valid up to the value of relative displacement $u=u_{*}$, at which a soil particle is separated from the outer surface of the pipeline, the bond between the soil particles and the outer contact surface of the pipeline ceases to exist. Further, a different law is needed to describe the process of a pipeline interaction with soil. As shown, for example, in [14,37], the process of interaction beyond of $u_{*}$ is described by the Coulomb law.

In fact, due to cohesion processes between soil and the outer surface of the pipeline [1], the interaction occurs between the soil particles “stuck” to the pipeline and other particles of soil. Therefore, under longitudinal interaction of the pipeline with soil, there is, in fact, a soil shear and cut-off near the outer surface of the pipeline in a certain soil layer. It is difficult to state the existence of such a soil layer in experiments [14,38]. In [38], the existence of a soil contact layer under shear interaction of a rigid body with soil was substantiated theoretically. It was shown that at insignificant stresses ${\sigma }_N$ normal to the body’s outer surface, the contact layer’s thickness was about 0.01 m.

The process of interaction force $\tau$ formation in a given contact layer was shown in [14,38], first in proportion to the value of relative displacement $u$, and then after reaching equality $u=u_{*}$ according to the Coulomb law. It was shown there that the process of shear strain of the soil contact layer occurs separately. Under shear interaction of a rigid body with soil, the contact layer can be destroyed, while the soil outside the contact layer remains intact. This means that the strain in the contact layer does not obey elastic laws, and the soil medium, as a whole, can remain elastic or viscoelastic during earthquakes. The results of a survey of strong earthquake aftermath in underground pipelines show the existence of a solid, cohesively stuck thin layer of soil on the outer surface of the pipeline [2,3]. They also indirectly confirm the existence of the soil contact layer and the above hypothesis.

It was shown in [38] that when the equation of state (the law of deformation) of soil takes into account the process of soil destruction under strain [39,40], the classical boundary condition of complete cohesion ($u=0$) is applicable at the direct contact boundary of soil medium with a rigid body.

In this formulation, the forces of interaction are formed in the process of soil strain around the pipeline in accordance with the accepted equation of the state of soil. The law of soil deformation should be adequate to the process of deformation and destruction of the soil contact layer [35,38]. However, in this case, it is necessary to jointly consider the deformation process in the soil environment and underground pipeline. This leads to complex coupled wave problems for two media. Under the action of seismic waves, even for the simplest one-dimensional wave problems, the theoretical problem’s solution is quite laborious and can be obtained using numerical methods only [35,38].

This circumstance requires a search for simpler methods to determine the stresses in an underground pipeline under seismic effects. However, the growing economic and environmental requirements for underground pipelines as the transportation means for liquid and gas substances (sometimes highly toxic ones) [41] pose a problem for researchers to reliably and accurately determine and predict the stresses occurring under strong ground motion.

Experimental determination of seismic stresses in underground pipelines is costly and very laborious. Therefore, theoretical methods for determining stresses under seismic impacts in underground pipelines are more appropriate. However, the option shown above requires, first of all, more accurate and reliable knowledge of the law of interaction of an underground pipeline with soil. The more reliable this law is, the more accurate the definition of the value stresses in underground pipelines under seismic effects. Consider here the law of longitudinal interaction of the pipeline with soil.

According to the results of experiments and observations [1,2,3,6,7,14,37], schematic changes in dependencies $\tau \left(u\right)$ are shown in Figure 1.

The experiments on the longitudinal interaction of pipelines with soil and the shear interaction of rigid bodies with soil, presented in [14], show that the process of interaction of a pipeline with soil of disturbed and undisturbed structure differs significantly. Structurally disturbed soil during the construction of pipeline communications and facilities consolidates over time and forms a new coherent soil structure around the pipeline. When the pipeline interacts with this soil layer, the interaction process occurs along complex curves 1–3 (Figure 1). Curves 1–3 refer to different values of the stress ${\sigma }_N$ normal to the outer surface. Curves 1–3 refer to stresses ${\sigma }_{N1}<{\sigma }_{N2}<{\sigma }_{N3}$, respectively, and are discussed below.

As seen from Figure 1, the dependences $\tau \left(u\right)$ in the range of relative displacements $0\le u\le u_{*}$ for disturbed (curves 1–3) and undisturbed soil structures (curves 1–3) differed qualitatively and quantitatively. As noted above, in the pipeline interaction with soil, a soil contact layer was formed, and the structural destruction of soil occurred precisely in this contact layer. Under interaction with soil, when the contact layer was structurally undisturbed, the dependences $\tau \left(u\right)$ show the peak value of the interaction force, and when the contact layer of soil was structurally disturbed in this area, the peak value of ${\tau }_p$ did not appear (curves 1–3). In the section$0\le u\le u_{*}$, the change in $\tau \left(u\right)$ in both cases was curvilinear. This is due to the microdestruction of the soil structure in the contact layer in the case of undisturbed soil and the rearrangement of soil particles in the case of disturbed soil.

In the case of consolidated soil, with an increase in the value of relative displacement, the value of $\tau$ rose to ${\tau }_p$, reached at $u=u_p$. In the section $0\le u\le u_p$, the soil structure underwent microdestruction. After reaching ${\tau }_p$, the value of shear stress dropped rapidly to the value of ${\tau }_r$ at $u=u_{*}$ and remained constant. In the section $u_p\le u\le u_{*}$ of curve $\tau \left(u\right)$, an intensive destruction of the soil contact layer occurred. Accordingly, the values of shear stress dropped rapidly. After complete destruction of soil structure, the value of $\tau$ remained constant and equal to $\tau ={\tau }_r$. In the section $u>u_{*}$ the Coulomb’s law of dry friction was obviously fulfilled. Here the value of $\tau$ was determined from the relationship

$$\tau =c+f{\sigma }_N$$

(7)

where $c$ is the cohesion force between soil particles, $f$ is the coefficient of friction between soil particles, and ${\sigma }_N$ is the stress normal to the contact surface, as was noted above.

Note that in Equation (5), the friction coefficient $f$ is taken precisely between the soil particles and not between the soil particles and the pipeline’s outer surface. This is true in cases where soil particles have adhered to the pipeline’s outer surface, and strong bonds have formed between them. In cases where this has not yet happened, i.e., when soil particles have not had time to adhere to the pipe surface and the soil has not yet consolidated after the pipeline construction, the interaction process $\tau \left(u\right)$ occurs along the curves 1, 2, and 3. In this case, the peak value of shear stress ${\tau }_p$ did not appear and as the value of relative displacement$u$ increased, the value of $\tau$ increased to $\tau ={\tau }_r$ and remained constant. Note that after the destruction of the soil contact layer, the repeated processes of the pipeline interaction with soil occurred along the curves 1, 2, or 3.

Longitudinal interaction of the underground pipeline with soil occurred in two directions conditionally, in direct motions and reverse motions. On the diagram $\tau \left(u\right)$, the reverse motion of the pipeline relative to soil can begin from any point of it, depending on the change in seismic load. The reverse and repeated direct motion of the pipeline relative to soil occurs in the most complicated way depending on the degree of the contact layer destruction [14]. This follows the results of experimental studies of the interaction of underground pipelines with soil and studies of the aftermath of strong earthquakes on underground utilities [14].

In addition, experimental studies have indicated that the value of the peak shear stress (the force of interaction) ${\tau }_p$ depends significantly on the interaction velocity $\frac{du}{dt}$. Depending on the value of the interaction velocity and the strength of structural bonds between soil particles, the value of ${\tau }_p$ can be almost twice as large as the Coulomb value ${\tau }_r$. Experiments show that the values of $u_{*}$ do not depend on the interaction velocity [14].

In the diagram $\tau \left(u\right)$ (Figure 1), in the section $u>u_{*}$ where the Coulomb’s law (7) is fulfilled, the values of the interaction force $\tau$ uniquely depend on the normal stress ${\sigma }_N$. This section is the limiting stage of the pipeline interaction with soil. It follows the prelimiting stage in section $0\le u\le u_{*}$. These stages of interaction are two stages of the same interaction process. From this, it follows that in the prelimiting section $0\le u\le u_{*}$, the interaction force should depend on the value of ${\sigma }_N$, and Equation (4) has the form

$$\tau =K_x\left({\sigma }_N\right)u$$

(8)

Ignoring ${\sigma }_N$ at the prelimit stage of interaction, as is done in most studies, could lead to a completely inaccurate description of the process of interaction of an underground pipeline with soil under seismic effects.

Experimental studies have established the dependence of the force of interaction $\tau$ on the buried pipeline’s depth [14]. This pipeline depth ${\sigma }_N={\sigma }_N^S$ determines the static stress normal to the outer surface of the pipeline. However, it is known that under seismic wave propagation, even in the cases where the wave front is perpendicular to the pipeline axis, a dynamic normal stress ${\sigma }_N={\sigma }_N^D$ occurs, which is comparable to ${\sigma }_N^S$, and sometimes greater than ${\sigma }_N^S$. When an arbitrary seismic wave interacts with an underground pipeline, the following relation takes place

$${\sigma }_N={\sigma }_N^S+{\sigma }_N^D$$

(9)

where ${\sigma }_N^S$ is determined by the pipeline depth in soil, ${\sigma }_N^D$ is defined as the stress created by the seismic wave.

When a compressional seismic wave propagates parallel to the pipeline axis, the normal stress ${\sigma }_N^D$ is approximately determined by the formula

$${\sigma }_N^D=K_{\sigma }{\sigma }_g$$

(10)

where $K_{\sigma }$ is the coefficient of lateral soil pressure and ${\sigma }_g$ is the longitudinal seismic stress in soil under longitudinal wave propagation.

In the case of strong earthquakes in loess soils, the approximate values of ${\sigma }_p^{max}=0.5$ MPa [35] were observed. Then at $K_{\sigma }=0.3$ we got ${\sigma }_N^{Dmax}=0.15$ MPa, according to (10), which corresponded to the greater depth of the pipeline ($H=7.5\mathrm{m}$). Hence, as can be seen, the value of ${\sigma }_N^{Dmax}$ might even exceed the value of ${\sigma }_N^S$. This shows that the calculations for seismic resistance of underground pipelines without taking into account ${\sigma }_N^D$ were approximate.

According to the dynamic theory, in the law of interaction (4) or similar laws, the value of coefficient $K_x$ is considered constant when solving problems of seismic resistance of underground pipelines. In fact, as shown above, it should be variable in the form (8), taking into account relations (9) and (10).

The interaction law in the form (8) is the simplest one. In the case of account for the interaction velocity $\frac{du}{dt}$, this law becomes more complicated. The laws of interaction with account for $\frac{du}{dt}$, their parametric analysis, advantages, and disadvantages were considered in [14].

It should be noted that the law of interaction in the form (4) or (8) in all cases of interaction of an underground pipeline with soil does not always holds in reality. When elastic or viscoelastic bonds are not broken between soil particles on the contact layer and the values of relative displacement are less than the value of $u_{*}$, then the interaction law can indeed be taken in the form (8). It is known that the value of $u_{*}$, depending on the types of soil, ranges from 0.003 to 0.007 m [14].

The value of the peak relative displacement can be taken approximately equal to $u_p=0.5u_{*}$. Then $u_p=0.0015÷0.0035\mathrm{m}$. At the values of relative displacement $u\le u_p$, the force of interaction under direct and reverse motion of the pipeline relative to soil can be described by Equation (8). As soon as the value of $u$ exceeds $u_p$, i.e., at $u>u_p$, another law of interaction should be accepted at the reverse motion that takes into account the degree of soil structure damage in the contact layer. After complete destruction of the soil contact layer structure, the force of interaction turns into a force of friction. In this case, the sign of the friction force on the outer surface of the underground pipeline in contact with soil is determined with account for the interaction velocity $\frac{du}{dt}$ according to the law

$$\tau =\chi K_x\left({\sigma }_N\right)u$$

(11)

where $\chi =sgn\left(v\right)$, is the rate of relative displacement, determined by the relationship

$$v=v_g-v_c=\frac{d{u}_g}{dt}-\frac{d{u}_c}{dt}$$

(12)

Then

$$\begin{array}{c}\chi =+1atv>0\\ \chi =-1atv<0\\ \chi =0atv=0\end{array}\}$$

(13)

According to (13), when the soil deformation around the pipeline is greater than the deformation of the underground pipeline itself (${\epsilon }_g>{\epsilon }_c$), the interaction force $\tau$ is an active force involved in motion. At ${\epsilon }_g<{\epsilon }_c$, the force of interaction is a passive one resisting to friction force. This circumstance is of key importance when solving the problems of seismic resistance of underground pipelines. Depending on the property of the interaction force (active or passive one), the stress state of the underground pipeline is formed in different ways. The mechanisms of formation of longitudinal stresses in an underground pipeline were considered in [35].

Dynamic theory does not provide a priori the possibility of converting the interaction force from active to passive one and vice versa. As seen from Figure 1, this is possible only with the values of relative displacements $u<u_p$. As noted above, in this case, the relative displacements are insignificant. Considering that during strong earthquakes, the absolute displacement of soil reaches 0.3–0.4 m, it becomes obvious that the dynamic theory is inapplicable to these cases without taking into account relations (11)–(13).

In [22,23,24], the cases were considered when the soil surrounding the underground pipeline has greater rigidity than the pipeline material ($c_g>c_c$, where $c_g$ is the propagation velocity of longitudinal waves in soil, $c_c$ is the propagation velocity of longitudinal waves in the pipeline). This hypothetical assumption also does not hold in practice. Some interesting results could be obtained under such assumptions when solving the problems of seismic resistance of underground pipelines [22,23,24]. Since the soil environment around the pipeline is always less rigid than the underground pipeline, the soil contact layer always deforms more than the underground pipeline under seismic waves propagation in the underground pipeline–soil system. Consequently, the interaction force acting on the pipeline has more pronounced active properties than passive ones [16]. When a monochromatic seismic wave propagates along an elastic or viscoelastic pipeline (not considering the soil effect) at a stress amplitude of ${\sigma }_{max}=0.5$ MPa, no stresses greater than 0.5 MPa appeared in the pipeline. The formation of large longitudinal stresses (100 MPa or more) in underground pipelines, leading to the pipeline destruction [4], is exclusively the result of significant deformations of soil surrounding the underground pipeline. As shown above, the deformation properties of soil surrounding the underground pipeline, or its stress–strain state under strong ground motion, is of significant determining importance in the formation of the stress–strain state of an underground pipeline.

The nonlinearity of the diagram $\tau \left(u\right)$ (Figure 1) and the manifestation of the peak value of ${\tau }_p$ in the diagram occur as a result of the soil contact layer destruction [38,42]. The soil with weak structural bonds, under shear deformations, begins to undergo microdestruction, which leads to a nonlinear change in the diagram $\tau \left(u\right)$, already in the section $0\le u\le u_p$. In the section $u_p<u\le u_{*}$, the structure of the soil contact layer is completely destroyed. This factor is taken into account in law (4) by introducing the parameter $I_S$

$$\tau =K_x\left({\sigma }_N,I_S\right)u$$

(14)

where $I_S=abs\left(\frac{u}{u_{*}}\right)$ is the parameter characterizing the degree of damage of the soil contact layer structure.

The range in parameter change is $0\le I_S\le 1$. At $I_S=0$, the soil contact layer is not destroyed, and at $I_S=1$ it is destroyed completely. The change in $I_S$ is irreversible. With these circumstances in view, a function was proposed in [14,42], which takes into account the changes in $K_x\left({\sigma }_N,I_S\right)$ in the following form

$$K_x\left({\sigma }_N,I_S\right)=K_x^{*}\left({\sigma }_N\right)exp\left(\beta \left(1-I_S\right)\right)$$

(15)

where $K_x^{*}$ is the function of rigidity (interaction) of the pipe with structurally disturbed soil, $\beta$ is the dimensionless coefficient characterizing the range of variation in $K_x$.

At $I_S=0$ from (15) we obtain

$$K_x^N\left({\sigma }_N\right)=K_x^{*}\left({\sigma }_N\right)e^{\beta }$$

(16)

In [14,42], based on the experimental results, the relation for the function $K_x^{*}\left(\sigma \right)$ is proposed

$$K_x^{*}\left({\sigma }_N\right)=K_N{\sigma }_N$$

(17)

where $K_N$ is the coefficient of rigidity of the connection of the underground pipeline with the soil contact layer (the coefficient of shear rigidity for the soil contact layer).

The value of relative displacement $u=u_g-u_c$ is determined by solving seismic wave propagation problems in the pipeline and soil.

This circumstance requires joint consideration of the process of deformation of the underground pipeline and the surrounding soil. In the general case, such a problem is a three-dimensional one. In this statement, the problem of the underground pipeline interaction with soil is rather complicated. In a two-dimensional statement, this problem was considered in [27].

In non-one-dimensional cases, as shown in [38], at the adjacent boundary of soil contact with the underground pipeline, the condition of complete cohesion $u=0$ could be accepted if the soil deformation laws take into account its structural destruction. In the cases where the structural destruction of soil is not considered, and in the cases of one-dimensional statement, it is necessary to use the condition in the form (15) at the underground pipeline–soil contact boundary.

In a one-dimensional case, the soil environment around the underground pipeline is considered as a half-space. The spatial coordinate $x$ coincides with the pipeline axis. The initial section of the half-space ($x=0$) also coincides with the initial section of the underground pipeline. A seismic plane wave propagating along the soil half-space (in the direction of the pipeline axis) is considered without taking into account the pipeline itself. As a result of solving such a wave problem, all wave parameters for the one-dimensional case are determined along the pipeline axis at each fixed point, i.e., ${\sigma }_g,v_g,u_g,{\epsilon }_g$, longitudinal seismic stress, soil particle velocity, soil particle displacement, and soil strain, respectively. They allow determining the forces of interaction of the pipeline with soil at each point along the pipeline axis.

At the same time, the problem of the same plane seismic wave propagation in an underground pipeline is solved, taking into account the interaction force (friction) on its outer surface in contact with the soil medium. This statement of the problem is the closest to the real situation. In contrast to the dynamic theory of seismic resistance of underground pipelines, in this case, the values of ${\sigma }_N,v_g,u_g,{\epsilon }_g$, and hence $I_S$ are determined from the solution of the wave problem for soil in the pipelines axial direction at each point. Therefore, the theory proposed here is called the wave theory of seismic resistance of underground pipelines. The wave theory is the next stage in developing the theory of seismic resistance of underground pipelines.

2.2. Formulation of the Problem

The pipeline itself as a whole and each particle of its circumference separately acquires a displacement under the pipeline deformation. This displacement is the same for all particles of the deformed pipeline’s circumference in a one-dimensional consideration of the problem. If the pipeline cross-section goes through deformation, then the displacement varies for different particles of the cross-section. For the latter consideration, the displacement velocity of the particles in the cross-section of the pipeline is called the velocity of the pipeline section.

Further, the mass, pressure, and temperature of the transported oil or gas are not considered. The pipe is assumed to be straight and horizontal. It is assumed that the pipe is axisymmetric in the absence of seismic loading.

The system of equations describing the process of underground pipeline interaction with soil under seismic loads in the case of one-dimensional motion is given below.

Equations of motion, continuity of deformations, and soil strain are:

$$\begin{array}{c}{\rho }_{0g}\frac{\partial v_g}{\partial t}-\frac{\partial {\sigma }_g}{\partial x}+\chi {\sigma }_{\tau g}=0\\ \frac{\partial v_g}{\partial x}-\frac{\partial {\epsilon }_g}{\partial t}=0\\ \frac{\partial {\epsilon }_g}{\partial t}+{\mu }_g{\epsilon }_g=\frac{\partial {\sigma }_g}{E_{Dg}\partial t}+{\mu }_g\frac{{\sigma }_g}{E_{Sg}}\\ {\mu }_g=\frac{E_{Dg}{E}_{Sg}}{\left(E_{Dg}-E_{Sg}\right){\eta }_g}\end{array}\}$$

(18)

where ${\rho }_{0g}$ is the initial density of soil, $v_g$ is the particle velocity (the mass velocity) of soil, ${\sigma }_g$ is the longitudinal (along the axis x) stress in soil, ${\sigma }_{\tau g}$ is the reduced force of interaction of the underground pipeline with soil, ${\epsilon }_g$ is the longitudinal (along the axis x) deformation of soil, ${\mu }_g$ is the volume viscosity parameter of soil, ${\eta }_g$ is the volume viscosity coefficient of soil, $E_{Dg}$ is the dynamic modulus of soil compression at $\frac{d{\epsilon }_g}{dt\to \infty }$, $E_{Sg}$ is the static compression modulus of soil at $\frac{d{\epsilon }_g}{dt\to 0}$, $x$ is the spatial coordinate (along the pipeline axis), and $t$ is time.

The values of ${\sigma }_N$, ${\sigma }_{\tau g},$ and $\chi$ are determined by the relationships

$${\sigma }_{\tau g}=\frac{4H\tau }{H^2-D_H^2}$$

(19)

$${\sigma }_N={\sigma }_N^S+{\sigma }_N^D$$

(20)

$${\sigma }_N^S={\gamma }_{g}H+{\gamma }_c\pi \frac{\left(D_H^2-D_B^2\right)}{\left(4D_H\right)}$$

(21)

$${\sigma }_N^D=K_{\sigma }{\sigma }_g$$

(22)

$$\chi =sgn\left(v\right),v=v_g-v_c$$

(23)

where $H$ is the depth of the pipeline in soil, $D_N$ is the outer diameter of the underground pipeline, ${\gamma }_g$ is the specific gravity of the pipeline material, $K$ is the coefficient of lateral pressure in soil, $v$ is the relative velocity, $v_g$ is the velocity of soil particles, $v_c$ is the velocity of the pipeline sections, ${\sigma }_N^S$ is the static soil stress normal to the outer surface, and ${\sigma }_N^D$ is the dynamic stress normal to the outer surface of soil.

The equations of motion, continuity, and deformation for an underground pipeline have the form

$$\begin{array}{c}{\rho }_{0c}\frac{\partial v_c}{\partial t}-\frac{\partial {\sigma }_c}{\partial x}+\chi {\sigma }_{\tau c}=0\\ \frac{\partial v_c}{\partial x}-\frac{\partial {\epsilon }_c}{\partial t}=0\\ \frac{\partial {\epsilon }_c}{\partial t}+{\mu }_c{\epsilon }_c=\frac{\partial {\sigma }_c}{E_{Dc}\partial t}+{\mu }_c\frac{{\sigma }_c}{E_{Sc}}\\ {\mu }_c=\frac{E_{Dc}{E}_{Sc}}{\left(E_{Dc}-E_{Sc}\right){\eta }_c}\end{array}\}$$

(24)

where ${\rho }_{0c}$ is the initial density of the pipeline material, ${\sigma }_c$ is the longitudinal (along the pipeline axis) stress in the pipeline, ${\sigma }_{\tau c}$ is the reduced force of interaction (friction) of the pipeline with the soil, ${\epsilon }_c$ is the longitudinal (along the pipeline axis) strain, ${\mu }_c$ is the volume viscosity parameter of the pipeline material, ${\eta }_c$ is the coefficient of volume viscosity of the pipeline material, $E_{Dc}$ is the dynamic deformation modulus (at$\frac{d{\epsilon }_c}{dt\to \infty }$), and $E_{Sc}$ is the static deformation modulus (at $\frac{d{\epsilon }_c}{dt\to 0}$) of the pipeline material.

The value of ${\sigma }_{\tau c}$ for the pipeline is determined from the relation

$${\sigma }_{\tau c}=\frac{4D_H\tau }{D_H^2-D_B^2}$$

(25)

where $D_B$ is the inner diameter of the underground pipeline.

The relationship to determine the force of interaction between the underground pipeline and soil $\tau$ is found from the relationships

$$\begin{array}{c}at0\le u\le u_{*},\frac{du}{dt\ge 0}\\ \tau =K_N{\sigma }_N\exp \left(\beta \left(1-I_S\right)\right)u\\ atu>u_{*},\frac{du}{dt>0}\\ \tau =c+f{\sigma }_N\\ atu<u_{*},\frac{du}{dt<0,0\le I_S<1}\\ \tau =K_x^R\left({\sigma }_N,I_S\right)u\\ atu\ge u_{*},\frac{du}{dt<0,I_S=1}\\ \tau =0\end{array}\}$$

(26)

where $K_x^R\left({\sigma }_N,I_S\right)$ is the function of the underground pipeline interaction with soil under reverse motion, determined by the relationships

$$K_x^R\left({\sigma }_N,I_S\right)=\frac{K_x^N}{\left(1-I_S\right)}$$

(27)

$$K_x^N=K_N{\sigma }_N{e}^{\beta }$$

(28)

Soil particles and pipeline sections displacement is determined by the formulas

$$u_g={{\displaystyle \int }}_0^t{v}_{g}dt,u_c={{\displaystyle \int }}_0^t{v}_{c}dt$$

(29)

After each interaction cycle, at the change in $\tau$, a new counting of the relative displacement values $u$ begins.

The initial sections ($x=0$) of the soil half-space and the underground pipeline are affected by a load that creates a wave in the pipeline and soil

$$\begin{array}{c}\sigma =\sigma sin\left(\frac{\pi t}{T}\right)at0\le t\le \theta \\ \sigma =0att>\theta \end{array}\}$$

(30)

where ${\sigma }_{max}$ is the amplitude of seismic stress in soil.

In this case, the end of the pipeline at $x=0$ can be load-free, i.e.,

$$\sigma =0att\ge \theta$$

(31)

As shown in [35], under the action of load (30), a plane wave propagates in soil and in the pipeline at different velocities.

The conditions at these wave fronts are

$$\begin{array}{c}atx=C_{g}t\\ {\sigma }_g=-C_g{\rho }_{0g}{v}_g=0\\ v_g=-C_g{\epsilon }_g=0\\ C_g=\sqrt{\frac{E_{Dg}}{{\rho }_{0g}}}\end{array}\}$$

(32)

$$\begin{array}{c}atx=C_{c}t\\ {\sigma }_c=-C_c{\rho }_{0c}{v}_c=0\\ v_c=-C_c{\epsilon }_c=0\\ C_c=\sqrt{\frac{E_{Dc}}{{\rho }_{0c}}}\end{array}\}$$

(33)

where $C_g$ is the propagation velocity of longitudinal waves in soil and $C_c$ is the propagation velocity of longitudinal waves in the pipeline.

In the case of load (31) acting on a pipeline, a wave in the pipeline is formed due to the interaction forces impact (26).

According to Equation (27), the coefficient of interaction (friction) under reverse motion of the pipeline relative to the soil is variable. For structurally undisturbed soil it is $K_x^R=K_x^N$ at $I_S=0$, and for completely structurally damaged soil at $I_S=1$ it is $K_x^R\to \infty$, and $\tau =0$.

Thus, here we consider two systems of equations describing the process of wave propagation in soil and in the pipeline. The systems of Equation (18) for soil and (24) for the pipeline are related through relations (26). With Equations (19)–(23), (25) and (27)–(29), boundary conditions (30)–(33), and at zero initial conditions, it is necessary to solve the coupled systems of Equations (18) and (24) separately for a soil environment and separately for an underground pipeline. As can be seen, even in the simplest one-dimensional case, the problem of the wave theory of seismic resistance of an underground pipeline is rather complicated.

3. Results and Discussion

3.1. Solution Methods

The wave propagation process in deformable bodies and media is described by differential equations in partial derivatives of hyperbolic type. In the case of one-dimensional movements, they have the form (18) for the soil medium and the form (24) for the pipeline. Numerical methods can only solve them.

The solution of these equations has not yet been obtained analytically. There are two approaches to solving them numerically:

(i).

It is possible to obtain numerical solutions by the finite difference method directly. In this case, these partial differential equations are directly solved.

(ii).

However, there is another method. Partial differential equations of hyperbolic type have real characteristics and relations on them. In this case, the characteristic relations are already ordinary differential equations. In the case of boundary conditions (30)–(33) and laws of interaction (26), the wave front lines in soil and the pipeline remain linear. The characteristic lines also remain linear on the characteristic planes $xt$ for soil and the pipeline. The numerical finite difference method’s application to these ordinary differential equations significantly increases the accuracy of the solutions obtained. Previously the authors have used this algorithm in solving many wave problems and showed the high accuracy of the method. Examples of the application of the method are publications [35,38,39,40].

This second approach was also used in this article.

The linearity of characteristic lines greatly facilitates the numerical implementation of the problems under consideration. The characteristic relationships in these cases are nonlinear. They are of the following types:

(iii).

For underground pipeline

$$\begin{array}{c}\frac{d{\sigma }_c}{dt}-C_c{\rho }_{0c}\frac{d{v}_c}{dt}=-C_c^2{\rho }_{0c}g\left({\sigma }_c,{\epsilon }_c\right)+\chi C_c{\sigma }_{\tau c}\\ \mathit{a}\mathit{l}\mathit{o}\mathit{n}\mathit{g}\mathit{c}\mathit{h}\mathit{a}\mathit{r}\mathit{a}\mathit{c}\mathit{t}\mathit{e}\mathit{r}\mathit{i}\mathit{s}\mathit{t}\mathit{i}\mathit{c}\mathit{l}\mathit{i}\mathit{n}\mathit{e}\mathit{s}\frac{dx}{dt}=C_c\\ \frac{d{\sigma }_c}{dt}+C_c{\rho }_{0c}\frac{d{v}_c}{dt}=-C_c^2{\rho }_{0c}g\left({\sigma }_c,{\epsilon }_c\right)-\chi C_c{\sigma }_{\tau c}\\ \mathit{a}\mathit{l}\mathit{o}\mathit{n}\mathit{g}\mathit{c}\mathit{h}\mathit{a}\mathit{r}\mathit{a}\mathit{c}\mathit{t}\mathit{e}\mathit{r}\mathit{i}\mathit{s}\mathit{t}\mathit{i}\mathit{c}\mathit{l}\mathit{i}\mathit{n}\mathit{e}\mathit{s}\frac{dx}{dt}=-C_c\\ \frac{d{\sigma }_c}{dt}-C_c^2{\rho }_{0c}\frac{d{\epsilon }_c}{dt}=-C_c^2{\rho }_{0c}g\left({\sigma }_c,{\epsilon }_c\right)\\ \mathit{a}\mathit{l}\mathit{o}\mathit{n}\mathit{g}\mathit{l}\mathit{i}\mathit{n}\mathit{e}\mathit{s}\frac{dx}{dt}=0\end{array}\}$$

(34)

where $g\left({\sigma }_c,{\epsilon }_c\right)=\frac{{\sigma }_c}{{\eta }_c}-\frac{E_{Dc}{E}_{Sc}\left({\epsilon }_c-\frac{{\sigma }_c}{E_{Dc}}\right)}{\left(E_{Dc}-E_{Sc}\right){\eta }_c}$, ${\eta }_c=\frac{E_{Dc}{E}_{Sc}}{\left(E_{Dc}-E_{Sc}\right){\mu }_c}$.

(iv).

For soil half-space

$$\begin{array}{c}\frac{d{\sigma }_g}{dt}-C_g{\rho }_{0g}\frac{d{v}_{gc}}{dt}=-C_g^2{\rho }_{0g}g\left({\sigma }_g,{\epsilon }_g\right)+\chi C_g{\sigma }_{\tau g}\\ \mathit{a}\mathit{l}\mathit{o}\mathit{n}\mathit{g}\mathit{c}\mathit{h}\mathit{a}\mathit{r}\mathit{a}\mathit{c}\mathit{t}\mathit{e}\mathit{r}\mathit{i}\mathit{s}\mathit{t}\mathit{i}\mathit{c}\mathit{l}\mathit{i}\mathit{n}\mathit{e}\mathit{s}\frac{dx}{dt}=+C_g\\ \frac{d{\sigma }_g}{dt}+C_g{\rho }_{0g}\frac{d{v}_g}{dt}=-C_g^2{\rho }_{0g}g\left({\sigma }_g,{\epsilon }_g\right)-\chi C_g{\sigma }_{\tau g}\\ \mathit{a}\mathit{l}\mathit{o}\mathit{n}\mathit{g}\mathit{c}\mathit{h}\mathit{a}\mathit{r}\mathit{a}\mathit{c}\mathit{t}\mathit{e}\mathit{r}\mathit{i}\mathit{s}\mathit{t}\mathit{i}\mathit{c}\mathit{l}\mathit{i}\mathit{n}\mathit{e}\mathit{s}\frac{dx}{dt}=-C_g\\ \frac{d{\sigma }_g}{dt}-C_g^2{\rho }_{0g}\frac{d{\epsilon }_g}{dt}=-C_g^2{\rho }_{0g}g\left({\sigma }_g,{\epsilon }_g\right)\\ \mathit{a}\mathit{l}\mathit{o}\mathit{n}\mathit{g}\mathit{l}\mathit{i}\mathit{n}\mathit{e}\mathit{s}\frac{dx}{dt}=0\end{array}\}$$

(35)

where $g\left({\sigma }_g,{\epsilon }_g\right)=\frac{{\sigma }_g}{{\eta }_g}-\frac{E_{Dg}{E}_{Sg}\left({\epsilon }_g-\frac{{\sigma }_g}{E_{Dg}}\right)}{\left(E_{Dg}-E_{Sg}\right){\eta }_g}$, ${\eta }_g=\frac{E_{Dg}{E}_{Sg}}{\left(E_{Dg}-E_{Sg}\right){\mu }_g}$.

To improve the accuracy of calculations on a computer, proceed to dimensionless variables (with a superscript zero) and parameters according to the formulas

$$\begin{array}{l}{x}^{\circ }={\mu }_{c}x/C_c;t^{\circ }={\mu }_{c}t;{\sigma }_c^{\circ }={\sigma }_c/{\sigma }_{max};\\ v_c^{\circ }=v_c/v_{max};{\epsilon }_c^{\circ }={\epsilon }_c/{\epsilon }_{max};\\ {\sigma }_g^{\circ }={\sigma }_g/{\sigma }_{max};v_g^{\circ }=v_g/v_{max};{\epsilon }_g^{\circ }={\epsilon }_g/{\epsilon }_{max};\\ v_{max}=-{\sigma }_{max}/C_c{\rho }_{0c};{\epsilon }_{max}={\sigma }_{max}/E_g;\\ {\gamma }_c=E_{Dc}/E_{Sc};{\gamma }_g=E_{Dg}/E_{Sg}\end{array}\}$$

(36)

After passing to dimensionless variables$x^{\circ },t^{\circ },{\sigma }^{\circ },{\epsilon }^{\circ },\mathrm{and}{v}^{\circ }$, Equations (18)–(35) take the following form for underground pipeline

$$\begin{array}{c}\frac{\partial v_c^{\circ }}{\partial t^{\circ }}+\frac{\partial {\sigma }_c^{\circ }}{\partial x^{\circ }}-\chi {\sigma }_{\tau c}^{\circ }=0\\ \frac{\partial v_c^{\circ }}{\partial x^{\circ }}-\frac{\partial {\epsilon }_c^{\circ }}{\partial t^{\circ }}=0\\ \frac{\partial {\epsilon }_c^{\circ }}{\partial t^{\circ }}+{\epsilon }_c^{\circ }=\frac{\partial {\sigma }_c^{\circ }}{\partial t^{\circ }}+{\gamma }_c{\sigma }_c^{\circ }\end{array}\}$$

(37)

$$\begin{array}{c}d{\sigma }_c^{\circ }+d{v}_c^{\circ }=\left({\epsilon }_c^{\circ }-{\gamma }_c{\sigma }_c^{\circ }\right)d{t}^{\circ }+\chi {\sigma }_{\tau c}^{\circ }d{t}^{\circ }at\frac{d{x}^{\circ }}{d{t}^{\circ }=+1}\\ d{\sigma }_c^{\circ }-d{v}_c^{\circ }=\left({\epsilon }_c^{\circ }-{\gamma }_c{\sigma }_c^{\circ }\right)d{t}^{\circ }-\chi {\sigma }_{\tau c}^{\circ }d{t}^{\circ }at\frac{d{x}^{\circ }}{d{t}^{\circ }=-1}\\ d{\sigma }_c^{\circ }-d{\epsilon }_c^{\circ }=\left({\epsilon }_c^{\circ }-{\gamma }_c{\sigma }_c^{\circ }\right)d{t}^{\circ }at\frac{d{x}^{\circ }}{d{t}^{\circ }=0}\end{array}\}$$

(38)

for soil

$$\begin{array}{c}{r}_g\frac{\partial v_g^{\circ }}{\partial t^{\circ }}+\frac{\partial {\sigma }_g^{\circ }}{\partial x^{\circ }}+\chi {\sigma }_{\tau g}^{\circ }=0\\ \frac{\partial v_g^{\circ }}{\partial x^{\circ }}+\frac{\partial {\epsilon }_g^{\circ }}{\partial t^{\circ }}=0\\ l_g\frac{\partial {\epsilon }_g^{\circ }}{\partial t^{\circ }}+l_g{m}_g{\epsilon }_g^{\circ }=\frac{\partial {\sigma }_g^{\circ }}{\partial t^{\circ }}+m_g{\gamma }_g{\sigma }_g^{\circ }\end{array}\}$$

(39)

$$\begin{array}{c}d{\sigma }_g^{\circ }+C_g{r}_{g}d{v}_g^{\circ }=m_g\left(l_g{\epsilon }_g^{\circ }-{\gamma }_g{\sigma }_g^{\circ }\right)d{t}^{\circ }-\chi {\sigma }_{\tau g}^{\circ }d{t}^{\circ }at\frac{d{x}^{\circ }}{d{t}^{\circ }=+C_{gc}}\\ d{\sigma }_g^{\circ }-l_g{r}_{g}d{v}_g^{\circ }=m_g\left(l_g{\epsilon }_g^{\circ }-{\gamma }_g{\sigma }_g^{\circ }\right)d{t}^{\circ }+\chi {\sigma }_{\tau g}^{\circ }d{t}^{\circ }at\frac{d{x}^{\circ }}{d{t}^{\circ }=-C_{gc}}\\ d{\sigma }_g^{\circ }-l_{g}d{\epsilon }_g^{\circ }=m_g\left(l_g{\epsilon }_g^{\circ }-{\gamma }_g{\sigma }_g^{\circ }\right)d{t}^{\circ }at\frac{d{x}^{\circ }}{d{t}^{\circ }=0}\end{array}\}$$

(40)

Boundary conditions take the form (39):

$$\begin{array}{c}{\sigma }^{\circ }=sin\left(\frac{\pi t^{\circ }}{{\mu }_{c}T}\right)at{x}^{\circ }=0,0\le t^{\circ }\le {\mu }_c\theta \\ {\sigma }^{\circ }=0at{x}^{\circ }=0,t^{\circ }\ge {\mu }_c\theta \\ {\sigma }_c^{\circ }=0,{\epsilon }_c^{\circ }=0,v_c^{\circ }=0atx{}^{\circ }=t^{\circ }\\ {\sigma }_g^{\circ }=0,{\epsilon }_g^{\circ }=0,v_g^{\circ }=0at{x}^{\circ }=C_{gc}{t}^{\circ }\end{array}\}$$

(41)

where $r_g=\frac{{\rho }_{0g}}{{\rho }_{0c}};l_g=\frac{E_{Dg}}{E_{Dc}};m_g=\frac{{\mu }_g}{{\mu }_c};C_{gc}=\frac{C_g}{C_c};{\sigma }_{\tau c}^{\circ }={\sigma }_{\tau }\frac{C_c}{\sigma c_{max}{{}_{\tau g}^{\circ }}_{\tau g}\frac{C_g}{\sigma c_{max}}}$.

Equations (18) and (24) in partial derivatives are thus reduced to ordinary differential equations in dimensionless form (38) and (40) at boundary conditions (41). The initial conditions, as noted above, are zero.

Note that the equations of state or the law of deformation of soil (18) and of the underground pipeline (24) are taken similarly. In both cases, the generalized Eyring’s law was used, called the model of a standard linear viscoelastic body. However, for soils ${\gamma }_g=\frac{E_{Dg}}{E_{Sg}}$ and for the pipeline material ${\gamma }_c=\frac{E_{Dc}}{E_{Sc}}$, the calculations have different values. Among the existing rheological models, Eyring’s law is the most reliable for describing dynamic soil deformation processes. In the case of a pipeline, at values of ${\gamma }_c=1.02$, Eyring’s model turns into Hooke’s model. At values of ${\gamma }_c$ greater than unity (${\gamma }_c>1$), the method considered here allows determining longitudinal stresses in underground viscoelastic (polymer) pipelines.

Equations (38) and (40) are nonlinear due to the term$\chi =sgn\left(v\right)$ and it is not possible to solve them analytically. So, they are solved numerically using the finite difference method in an explicit scheme. The solution algorithm is based on dual sampling grids on dual characteristic planes$x,t$. It is given in more detail in [35]. The solution programs are written in the PASCAL language and implemented in the Delphi environment. Numerical solutions are obtained for soil and the underground pipeline, in the form of changes in wave parameters in time $\sigma \left(t\right),\epsilon \left(t\right),\mathrm{and}v\left(t\right)$ for the fixed points along the coordinate, and for fixed time points $\sigma \left(x\right),\epsilon \left(x\right),\mathrm{and}v\left(x\right)$. The above Equations (1)–(41) form the basis of a one-dimensional wave theory of seismic resistance of underground pipelines under seismic impacts in the case of interaction laws (26). In [14,35], more complex laws of interaction were considered. Consider the results of computer calculations.

3.2. Numerical Calculations

3.2.1. Initial Data for Calculations

Numerical calculations were conducted using dimensionless variables and parameters. Then they were transformed into dimensional ones for easy understanding. The following data were selected as initial ones:

(i)

For the underground pipeline:

$D_H=0.2$ m; $D_B=0.18$ m; ${\rho }_{0c}=7800$ kg/m³; ${\mu }_c={10}^4$ s⁻¹; $C_c=5000$ m/s; ${\gamma }_c=1.02$; $E_{Dc}=C_c^2{\rho }_{0c}$; $E_{Sc}=\frac{E_{Ds}}{{\gamma }_c}$;

(ii)

For soil:

${\rho }_{0g}=1800$ kg/m³; $C_g=1000$ m/s; $K_{\sigma }=0.3$; ${\mu }_g=1000$ s⁻¹; ${\gamma }_g=1.1$; $E_{Dg}=C_c^2{\rho }_{0g}$; $E_{Sg}=\frac{E_{Dg}}{{\gamma }_g}$;

(iii)

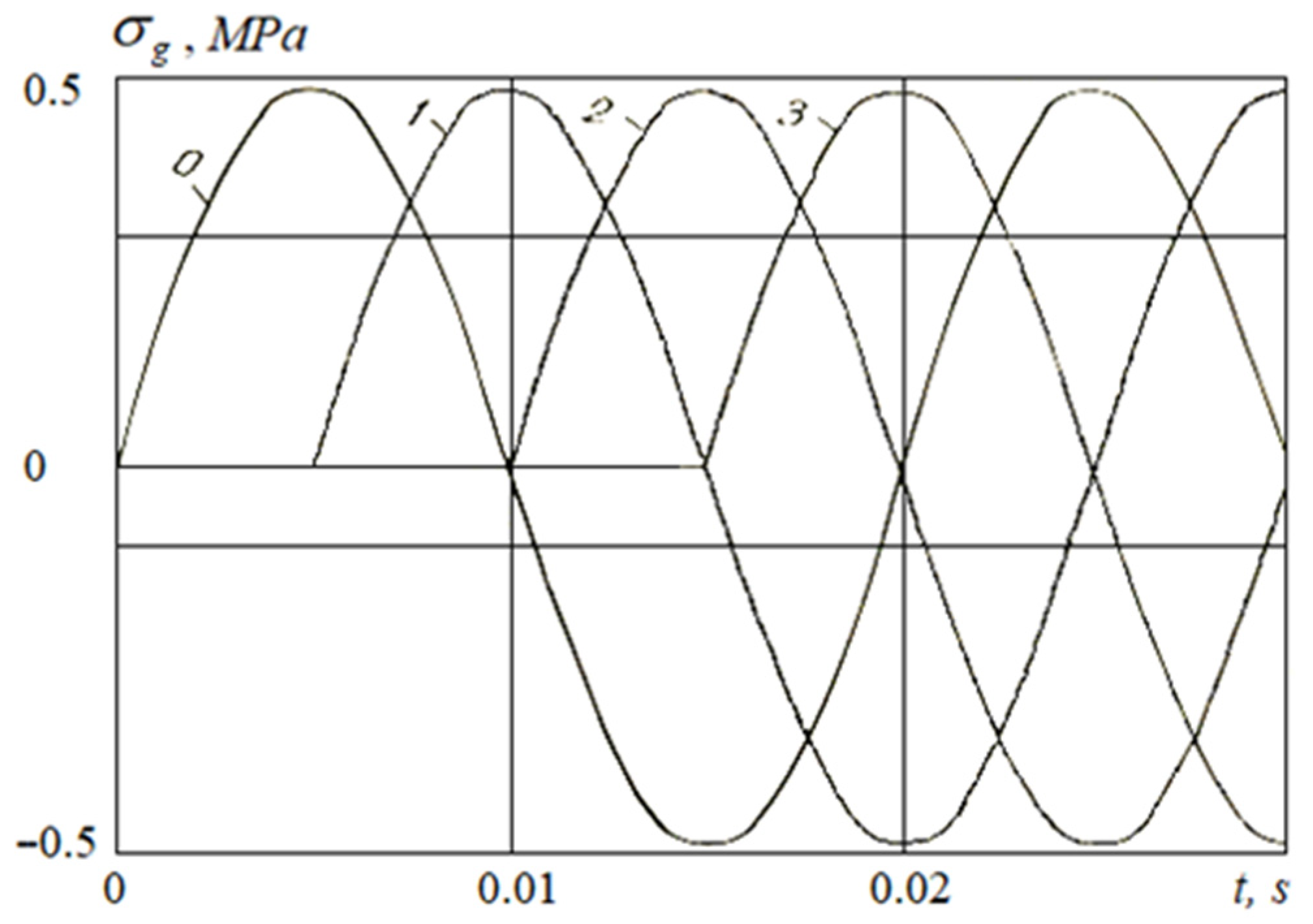
For seismic load parameters:

$T=0.01\mathrm{s}$; $\theta =5\mathrm{s}$; ${\sigma }_{max}$ MPa;

(iv)

For interaction parameters:

$H=1\mathrm{m}$; $K_N=100\mathrm{m}$⁻¹; $\beta =2$; $f=0.5$; $u_{*}=0.005$ m.

Note that from the interaction Equation (24) at $u=u_{*}$, we obtained

$$u_{*}=\frac{f}{K_N}$$

(42)

From this, it follows that with the known values of the coefficient of friction $f$ and the coefficient of soil rigidity$K_N$, it is possible to determine the corresponding value of $u_{*}$ [14].

If we assume that in Equation (26) $u_{*}\to \infty$, then in the calculations we obtained the interaction law of the type (14), and if we assume that $u_{*}\to 0$, (7) is taken as the interaction law. In the calculations, the cases are considered when the component ${\sigma }_N^D$ is not taken into account in (9), and $I_S=0$ in (15) is taken in the process of the interaction. When, in the calculations, the value of $u_{*}$ is set as a significant one ($u_{*}=100\mathrm{m}$), the soil structure practically does not change since $I_S=\frac{u}{u_{*}\to 0}$. As can be seen, by varying the initial data parameters, it is possible to obtain various options of the law of underground pipeline interaction with soil.

Let us consider these options.

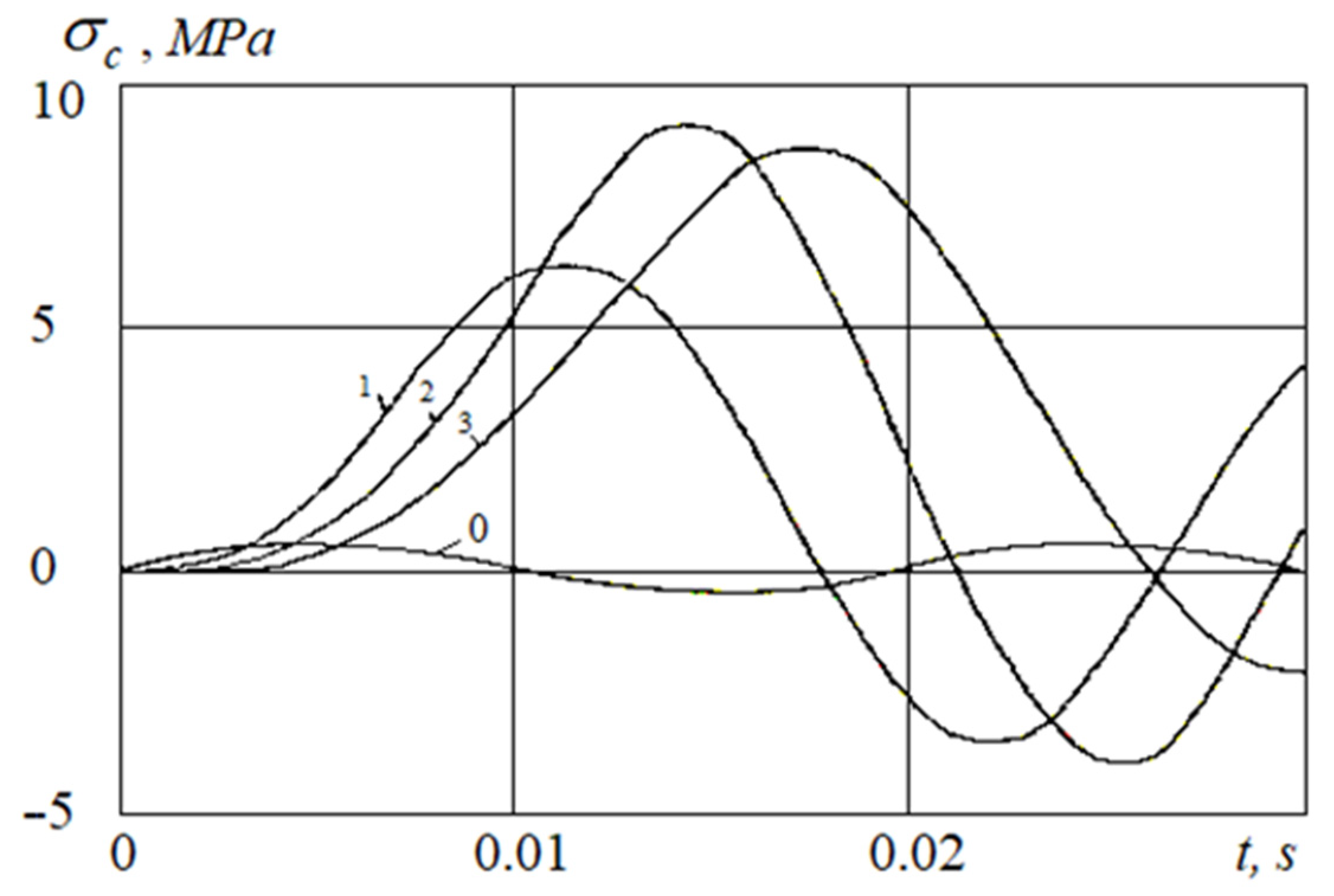
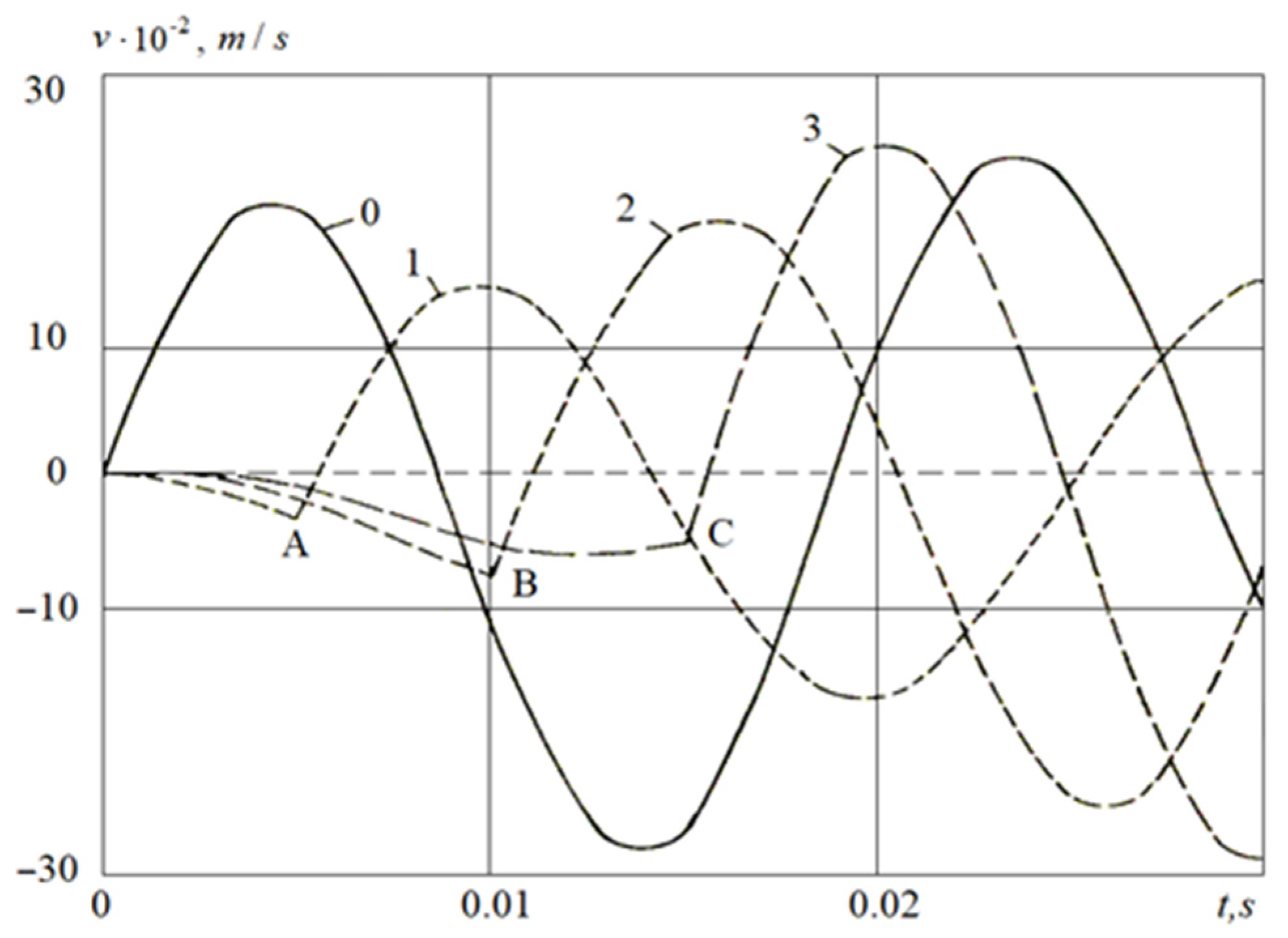
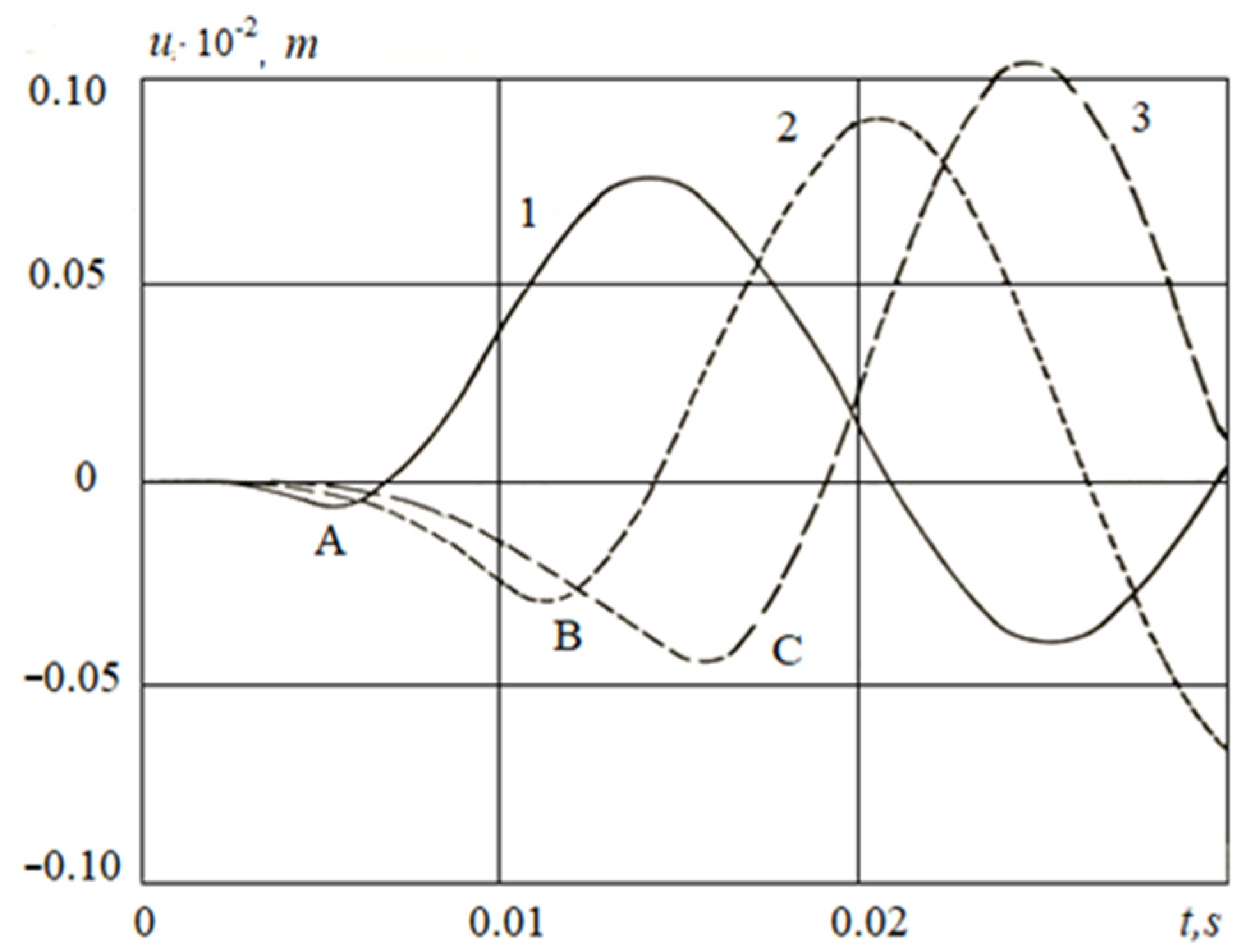
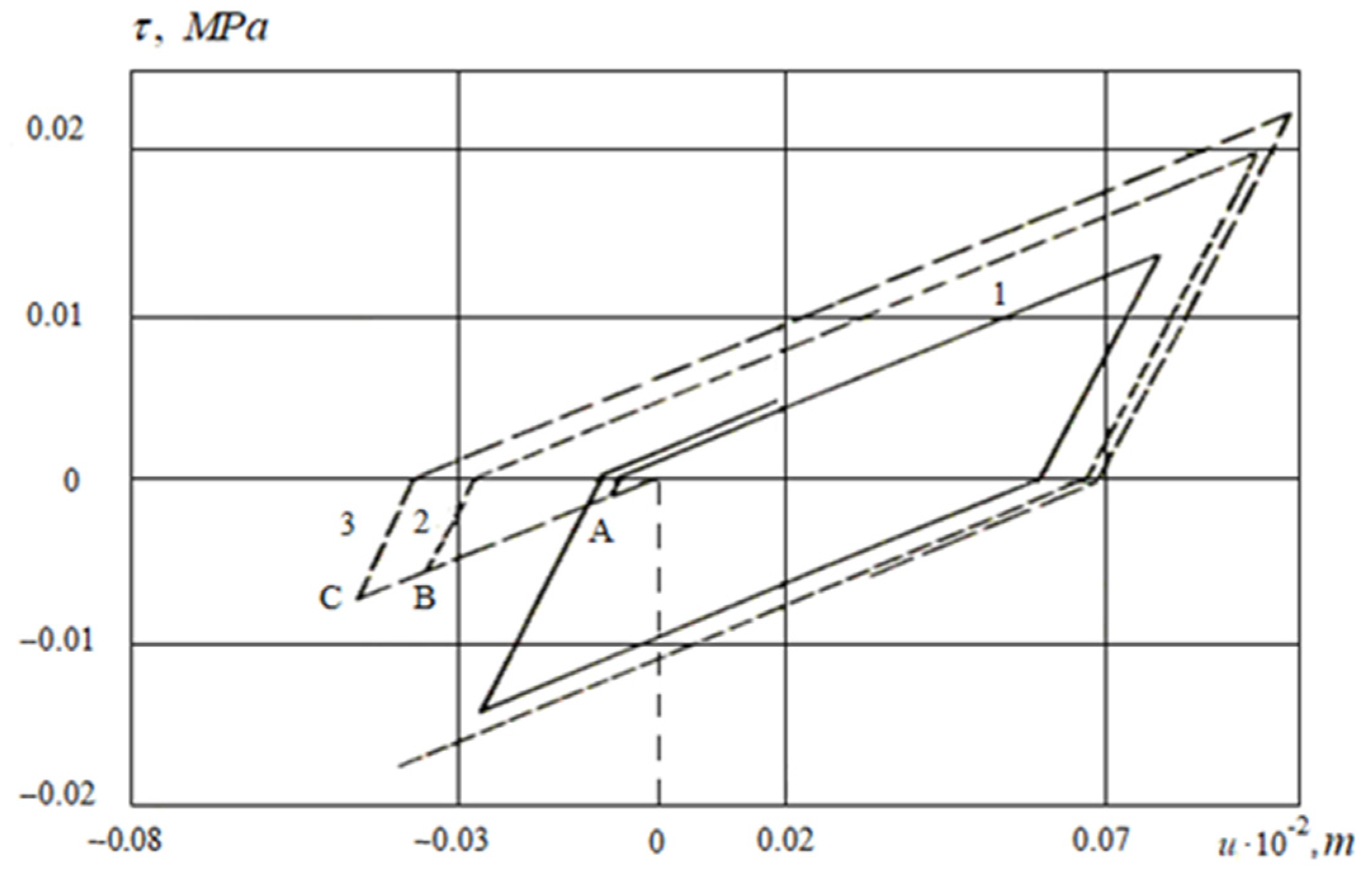
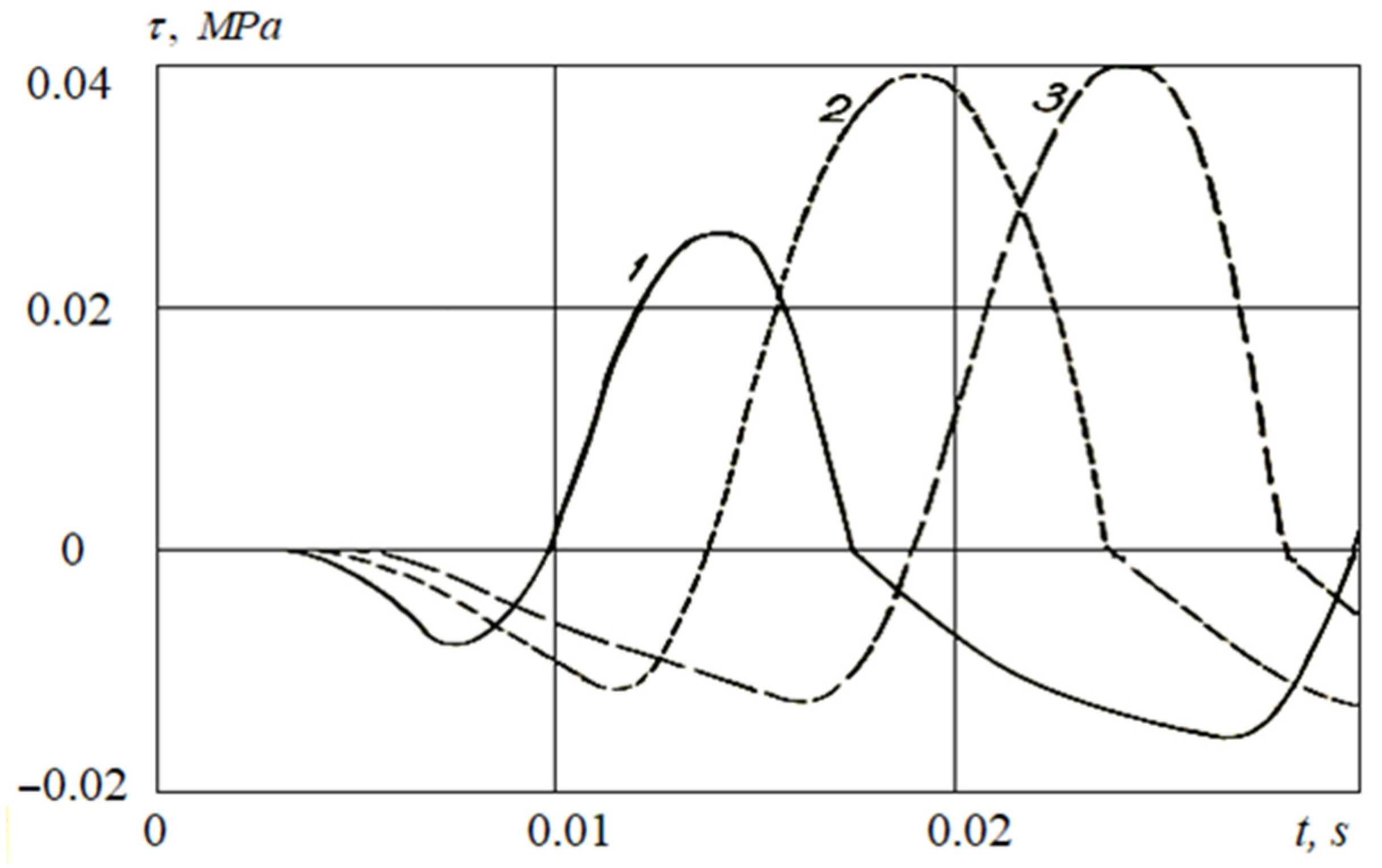
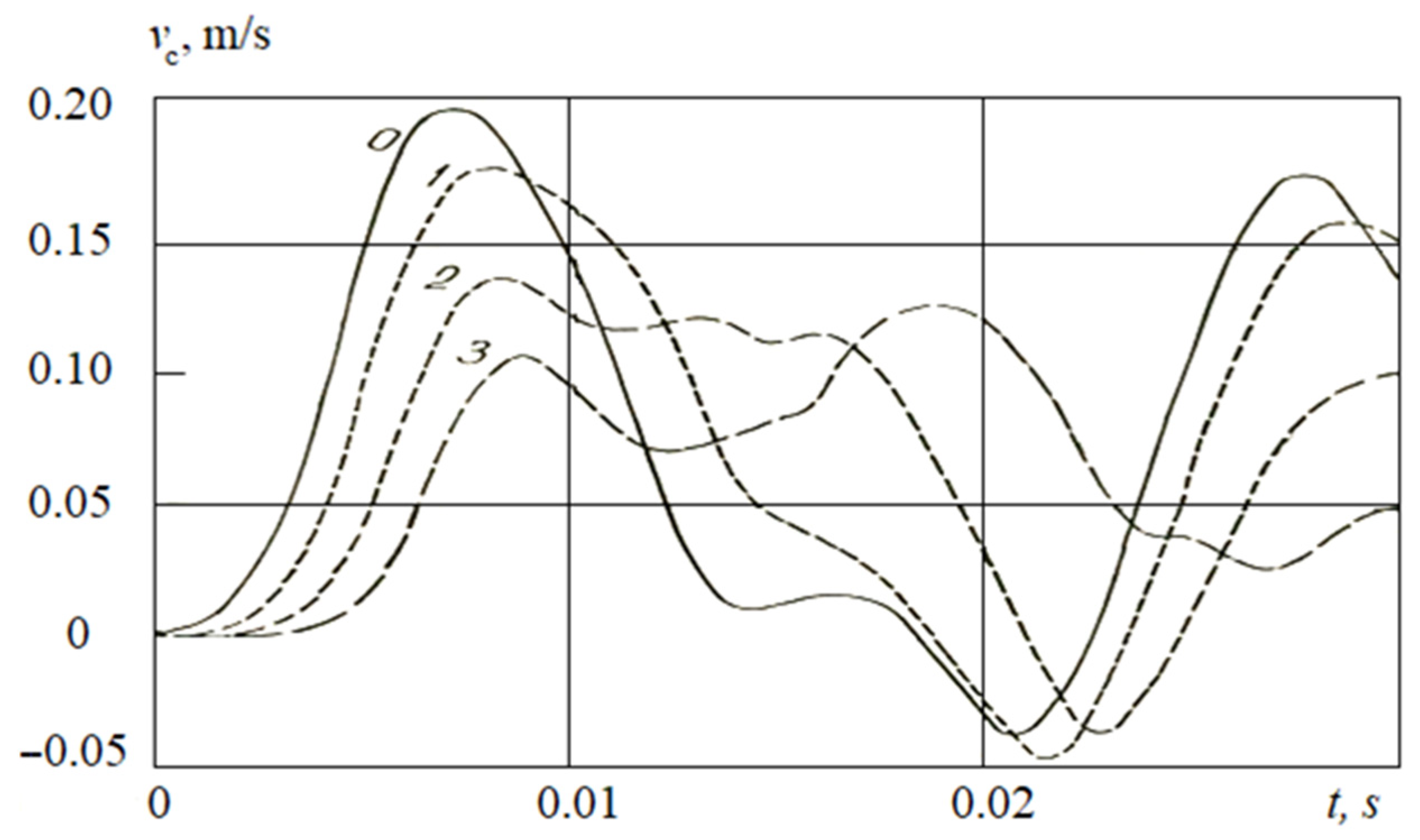
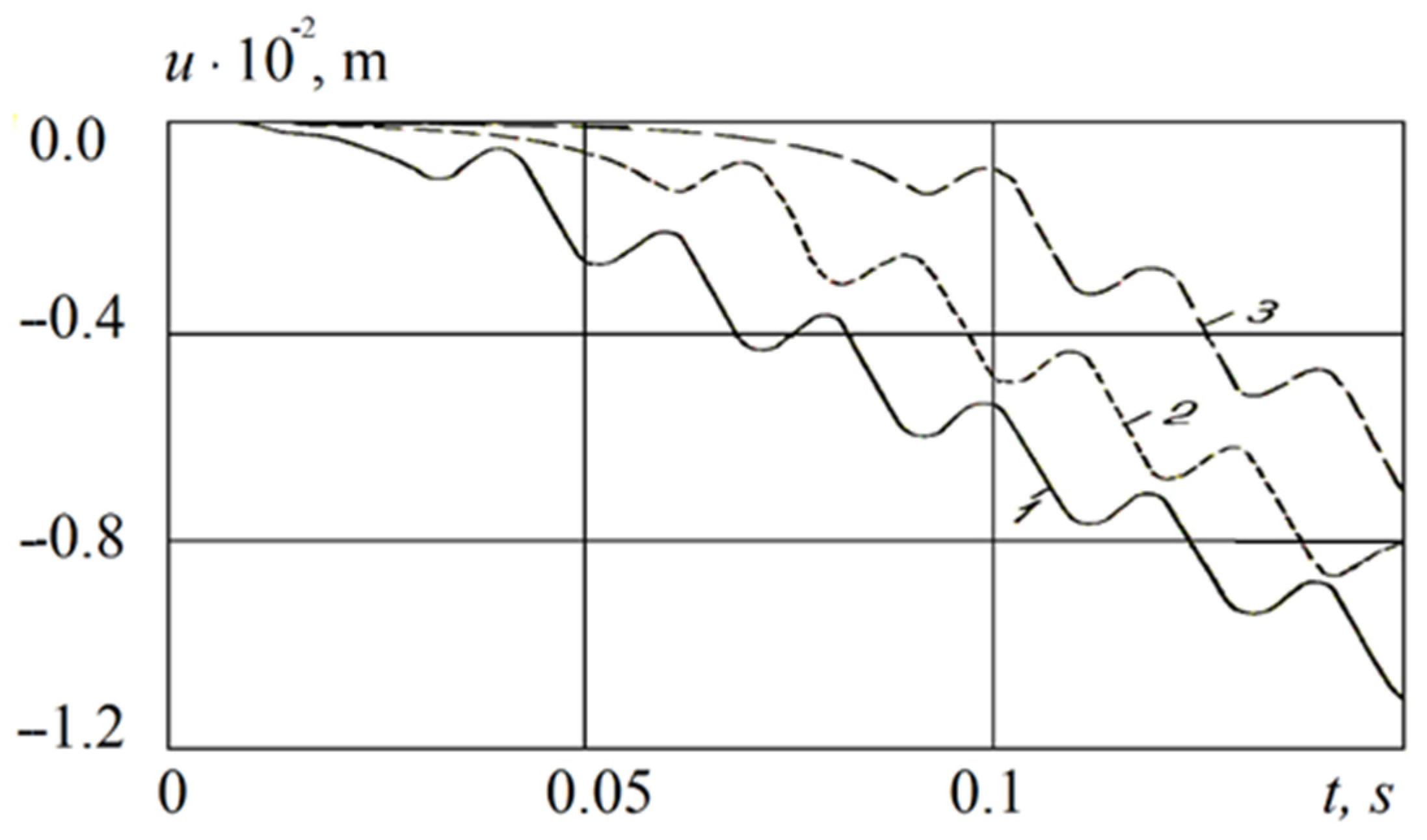
3.2.2. The Case of a Large Critical Relative Displacement

As the critical relative displacement $u_{*}\to \infty$ ($u_{*}=100\mathrm{m}$) and ${\sigma }_N={\sigma }_N^S=const$, at the boundary of the pipeline–soil contact, we obtain a nonlinear interaction model (14). In this case, the direct (translational) and reverse movement of the pipeline relative to soil occurs only according to Equation (14). The dynamic soil stress ${\sigma }_N^D$ normal to the semi-infinite pipeline’s outer surface ($L=1000\mathrm{m}$) is not taken into account. However, the relative displacement $u$ is determined considering the soil motion, that is, $u=u_g-u_c$, where $u_c$ is the absolute displacement of the pipe sections, $u_g$ is the absolute displacement of soil. The value of $u_g$ is determined from the solution of the system of Equation (40).

The time change in longitudinal stresses in the pipeline cross-sections ${\sigma }_c\left(t\right)$ and the corresponding dependences of shear stress on relative displacement $\tau \left(u\right)$ are shown in Figure 2 and Figure 3. Curves 0–3 in Figure 2 refer to the pipeline cross-sections x = 0, 5, 10, and 15 m. Here curve 0 shows the change in set load (30) in the initial sections of the pipeline and soil.

As seen from Figure 2, a significant increase in the values of the longitudinal stress is observed in the cross-sections of the pipeline with a distance from the initial cross-section. At x = 5 m (curve 1), the stress amplitude ${\mathsf{\sigma }}_{cmax}$ exceeds the stress amplitude ${\mathsf{\sigma }}_{cmax}$ in the initial section by about 12 times, at x = 10 m by 18 times, and at x = 15 m by 17 times. Thus, an account for the soil motion around the underground pipeline leads to a multiple increase in the longitudinal stress in the pipeline sections. Such an increase in stress values occurs due to the following circumstances.

Under the load acting on the pipeline and soil, changing according to the law (30), a longitudinal wave begins to propagate along the pipe and soil. As the wave velocity propagating in the pipeline is greater than the one in soil, the pipeline sections are the first to start moving.

At the initial points of time, the relative velocity value is $v=\frac{du}{dt}=\frac{d{u}_g}{dt}-\frac{d{u}_c}{dt}<0$ (Figure 4). After a certain time, the wave in soil reaches these sections of the pipeline. Since the soil rigidity is less than the pipeline stiffness $(C_g<C_c)$, the soil was more strained than the pipe, that is, the absolute displacement of soil was greater than the absolute displacement of pipe in the corresponding sections of the pipe and soil. As a result, the sign of the relative velocity, and, hence, of the relative displacements, changes (points A, B, and C in Figure 4 and Figure 5). The sign of shear stress $\tau$ changed too (Figure 6), that is, the shear stress (the resistance force) turned from a passive force into an active one. The active (driving) force $\tau$ led to an increase in the stress value in the pipeline, as seen in Figure 2. At the same time, the dependences$\tau \left(u\right)$ remained almost linear (Figure 3). The value of the relative displacement $u$ did not exceed its permissible value of $u_{*}=0.005$ m, due to the small values of ${\gamma }_g=1.1$.

The increase in stress in the pipeline cross-sections is a consequence of the interaction force $\tau$ transform from a passive (resistant) force into an active (driving) one. In the case of undisturbed soil, $\tau$ the shear stress was nearly always a passive force. It led to a decrease in stress amplitude in the underground pipeline.

The pipeline sections’ maximum stress values depend on the pattern of change in relative displacements and shear stresses in these sections.

3.2.3. Influence of Shear Stresses on Longitudinal Stresses in Pipeline Cross-Sections

Consider dependence the shear stresses $\tau \left(u\right)$ in the pipeline sections on longitudinal stresses’ values. The laws (26) at ${\sigma }_N={\sigma }_N^S=const$ were fulfilled on the contact surface of the pipeline with soil (the dynamic component of the normal stress on the pipeline was not taken into account). Therefore, the law (26) was equivalent to the condition of the “elastic–plastic” law of interaction involving the Coulomb friction law.

In contrast to the dependencies $\tau \left(u\right)$ in Figure 3, the interaction patterns had the form shown in Figure 7. Changes in shear stress on the contact surface of the pipeline with soil, in this case, occurred by analogy with “loading–unloading” (Figure 7). Curves 1, 2, and 3 in Figure 7 refer to the same sections of the pipeline x—5.10 and 15 m. Here, the interaction process did not reach the Coulomb law since $u<u_{*}$.

The corresponding stress changes in these pipe sections are shown in Figure 8 (curves 0–3). As seen from the comparison of the dependencies $\sigma \left(t\right)$ in Figure 8 and Figure 2, the changes in the law of the pipeline–soil interaction $\tau \left(u\right)$ under considered initial data of the problem have practically no effect on the dependence $\sigma \left(t\right)$. A similar pattern is observed in the dependences $v_c\left(t\right)$ and $u\left(t\right)$.

It should be noted here that in the calculation options considered above, ${\sigma }_N=const$ is taken in the laws of interaction (26). In these cases, only the changes in the absolute displacement of soil affected the wave parameters (stress, strain, and velocity of pipeline sections) in the pipeline. This led to the formation of an active force of interaction on the pipeline’s contact surface with soil, as noted above, so the stresses in the pipeline sections increased. At the same time, the wave parameters in the sections of soil remained unchanged.

Figure 9 shows the change in stresses in the sections of soil. Curves 0–3 refer, in this case, to the same sections of soil at $x=0\mathrm{m},5\mathrm{m},10\mathrm{m}$, and 15 m. As seen from Figure 9, the amplitudes of stress waves did not change with distance in soil. Changes in the interaction force did not affect the values of stress amplitude in soil due to relation (19). Obviously, in the case of ${\sigma }_N={\sigma }_N^S=const$, the results of calculations, according to the dynamic theory and the wave theories, coincided. In the calculations, according to the dynamic theory, the basic question was how accurately the change in absolute displacements of soil $u_g\left(t\right)$ could be set at each point of the contact surface of the underground pipeline with soil. As the answer to this question, the function of change in absolute displacement of soil $u_g\left(t\right)$ was determined from the soil motion equation’s solution. This naturally increased the accuracy of the results obtained and their reliability.

3.2.4. The Case of Variable Stress Normal to the Outer Surface of the Pipeline

Now consider the case of variable normal stress in the laws of interaction (26), i.e., ${\sigma }_N={\sigma }_N^S+{\sigma }_N^D\ne const$. That means that the normal stress to the outer surface of the pipeline is a variable (${\sigma }_N^D\ne 0$).

Figure 10 shows changes in stress over time in fixed sections of the pipeline at x = 0, 5, 10, and 15 m (curves 0–3), referring to the calculations’ results at ${\sigma }_N\ne const$. A qualitatively different picture was observed here in comparison with the case at ${\sigma }_N=const$ (Figure 8). The stress amplitudes in the considered pipeline sections were growing all the time. In terms of quantity, they differed by about two times. An increase in the maximum stress values in the pipeline cross-sections accounted for the dynamic component of normal stress ${\sigma }_N^D=K_{\sigma }\cdot {\sigma }_g$, where the value of the lateral pressure coefficient equaled 0.3.

The behavior of increasing the amplitude of stresses in the cross-sections of the pipeline with an increase in the values of normal stress is explained as follows. According to the regularity relationships of interaction (26), an increase in ${\sigma }_N$ led to an increase in $\tau$ on the pipeline’s contact surface with soil (Figure 11). Curves 1–3 refer to the same pipeline cross-sections x = 5 m, 10 m, and 15 m. Compared to the case at ${\sigma }_N=const$ (Figure 6), the values of shear stresses at ${\sigma }_N\ne const$ increased by 2–2.5 times (Figure 11). Since the shear stress is an active force in these cases, the stresses in the pipeline sections increased.

However, an increase in the values of longitudinal stresses in the pipeline cross-sections did not occur infinitely, as seen from Figure 10.

Figure 12 shows the changes in stress values in pipeline cross-sections x = 30 m, 60 m, and 90 m (curves 4, 5, and 6). As seen from Figure 12, the amplitudes of longitudinal stresses in the pipeline sections tended to be the steady-state ones. Stress values ${\mathsf{\sigma }}_{cmax}$ are: at x = 30 m, ${\sigma }_{cmax}=22.5$ MPa; at x = 60 m, ${\sigma }_{cmax}=26.5$ MPa; and at x = 90 m, ${\sigma }_{cmax}=27$ MPa.

The calculation results show that the stress amplitude did not change and becomes a steady-state one at ${\sigma }_{max}=27$ MPa in further sections of the pipeline. This stress value was about 3 times greater than the one at ${\sigma }_N=const$ (Figure 8). Calculations also show that the values of these stresses in the underground pipeline at ${\sigma }_{cmax}=0.5$ MPa practically did not depend on the load acting on the pipeline end at $x=0$. When boundary conditions (33) were accepted at $x=0$, the values of these stresses practically did not change.

The values of longitudinal stresses during the Gazli earthquake (Uzbekistan) were estimated in [35]. According to the data given in [35], the maximum values of longitudinal stresses for main underground pipelines were ${\sigma }_{cmax}=36.5÷$85 MPa.

Based on this, it could be stated that the calculated values of longitudinal stresses obtained by the authors were 25% less than the stress lower boundary observed in the pipelines during earthquakes. In the calculations, the initial data of the problem coincided with the data of the Gazli earthquake. However, it should be noted that at ${\sigma }_N=const$, the value of the stress amplitude in the pipeline ${\sigma }_{cmax}$ = 9 MPa (Figure 8) was significantly underestimated; it was four times less than the actual value. Therefore, it can be seen that an account for the dynamic component of the stress normal to the outer surface led to a more accurate determination of longitudinal stresses in the underground pipeline.

As calculation results show (Figure 13), at ${\sigma }_N\ne const$ the dependences $\tau \left(u\right)$ were very complex. Curves 1–3 in Figure 13 refer to pipeline cross-sections x = 5 m, 10 m, and 15 m. As seen from Figure 13, at ${\sigma }_N\ne const$, it is very difficult to determine and evaluate in advance the interaction patterns by trajectories $\tau \left(u\right)$. Moreover, these patterns differ for different points of the contact surface of the pipeline with soil. This difference confirms the correctness of the approach considered in this paper. According to that approach, the interaction patterns were local and determined at each point of the underground structure and soil’s outer surface. Further, in the calculations, they appeared according to the initial data of the problem, proceeding from the very nature of the dynamic process of interaction. It should also be noted that the dependences $\tau \left(u\right)$ obtained at ${\sigma }_N\ne const$ did not fit into the framework of existing canonical laws of material deformation (Figure 13).

Changes in the velocity of pipeline cross-sections at x = 0 m, 5 m, 10 m, and 15 m are shown in Figure 14 (curves 0–3).

The maximum values of the velocity of the pipeline cross-sections decreased with distance. At a distance of 15 m from the initial section of the pipeline, $v_{cmax}$, they were approximately twice less. With time, the amplitude of the velocity of the pipeline cross-sections increased, and at a certain level, it became a steady-state one (Figure 15). Here curves 4–6 refer to pipeline cross-sections x = 30 m, 60 m, and 90 m. The difference between the maximum and minimum values of the pipeline’s velocity cross-sections decreased with distance from the initial cross-section (Figure 15).

Consequently, the tension–compression wave originally specified at the initial section of the pipeline turns into a compression wave with distance from the pipeline’s initial section (Figure 12). Tensile stresses did not appear in the pipeline sections at x = 30 m, 60 m, and 90 m, and the stress values fluctuated in the area of compressive stresses (Figure 12). A positive stress value corresponded to compressive stresses.

Simultaneously, the value of relative displacements in time was increasing all the time (Figure 16). Curves 1–3 refer to pipeline cross-sections x = 5 m, 10 m, and 15 m. The growth of relative displacements was explained by the fact that the soil medium under considered loads was deformed more than the pipeline. This led to an increase in the values of relative displacements, and they exceeded the values of $u_{*}=0.005$ m.

The results of the above calculations show that an account for the dynamic component of normal stress led to new qualitative and quantitative results. In this paper, we considered the effect of a plane longitudinal seismic wave of a frequency of 50 Hz on an underground pipeline. The calculation results showed that a decrease in the seismic wave frequency led to an even more significant increase in the maximum value of longitudinal stresses in the underground pipeline. This is the subject of further research.

4. Conclusions

The critical analysis of the existing theories considers the advantages and disadvantages of seismic resistance of underground pipelines. The results obtained led to the following conclusions.

• The main disadvantage of the dynamic theory of seismic resistance of underground pipelines is the neglect of dynamic stress state in soil under seismic wave propagation. The next drawback of the dynamic theory is an inaccurate, approximate accounting for the displacement of the soil medium to which the underground pipeline is embedded.
• The complete interaction process includes the stages of nonlinear changes in the interaction force (the friction force) by manifesting its peak value and the Coulomb friction. The contact layer of soil undergoes shear deformations until complete structural destruction of the soil contact layer. The interaction force is the friction force, and its peak value does not appear.
• The problems of seismic resistance of underground pipelines should be considered based on the theory of propagating seismic waves in a soil medium and the interaction of seismic waves with underground pipelines, i.e., based on the wave theory of seismic resistance of underground pipelines.
• A one-dimensional coupled problem of seismic resistance of underground pipelines under seismic impacts was posed based on the wave theory. An algorithm and a program for the numerical solution of the stated wave problems were developed using the method of characteristics and the method of finite differences.
• An analysis of the laws of interaction of underground pipelines with soil under seismic influences shows that it is necessary to use in the calculations the laws of interaction that account for the complete interaction processes observed in experiments.
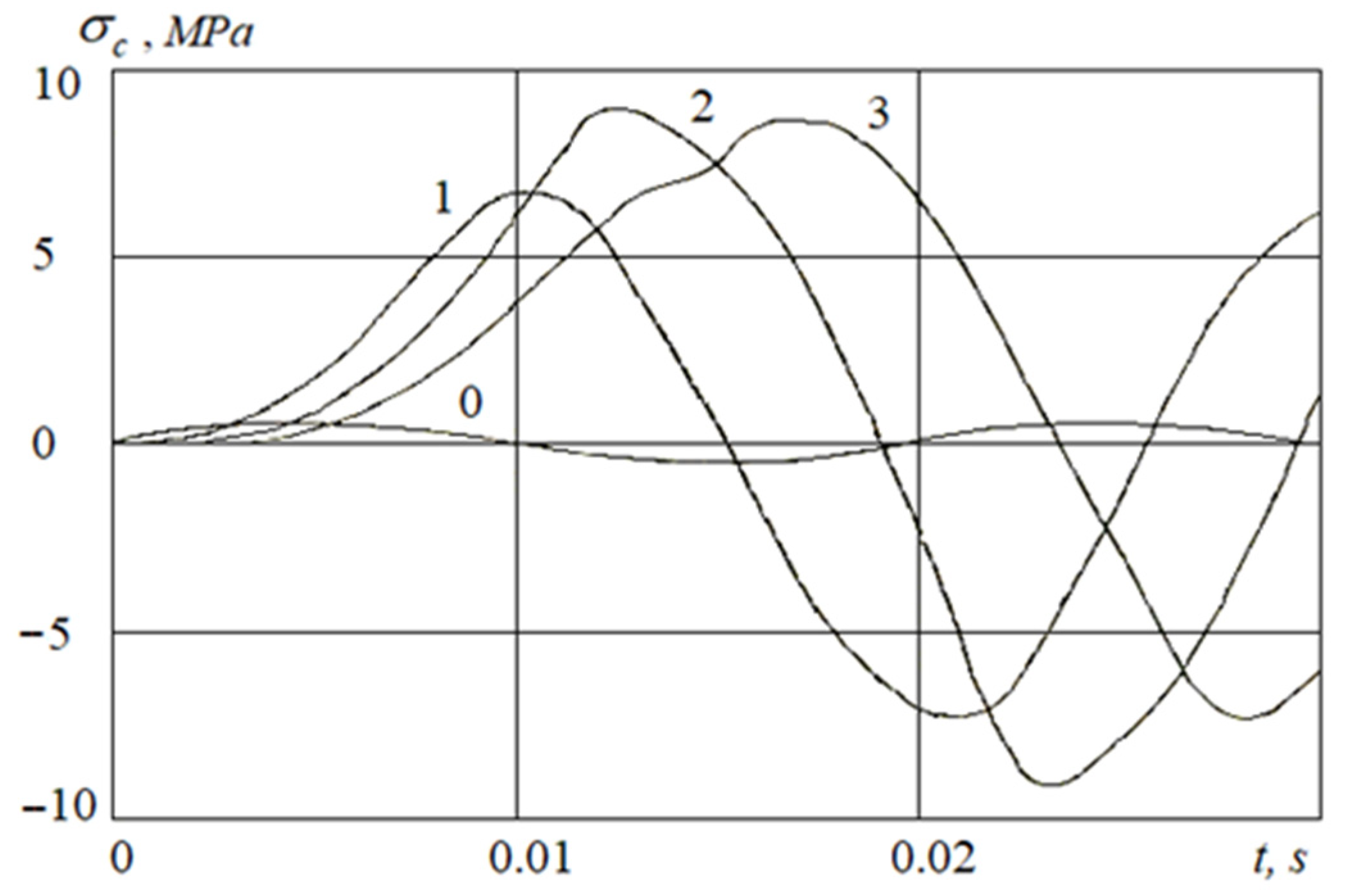
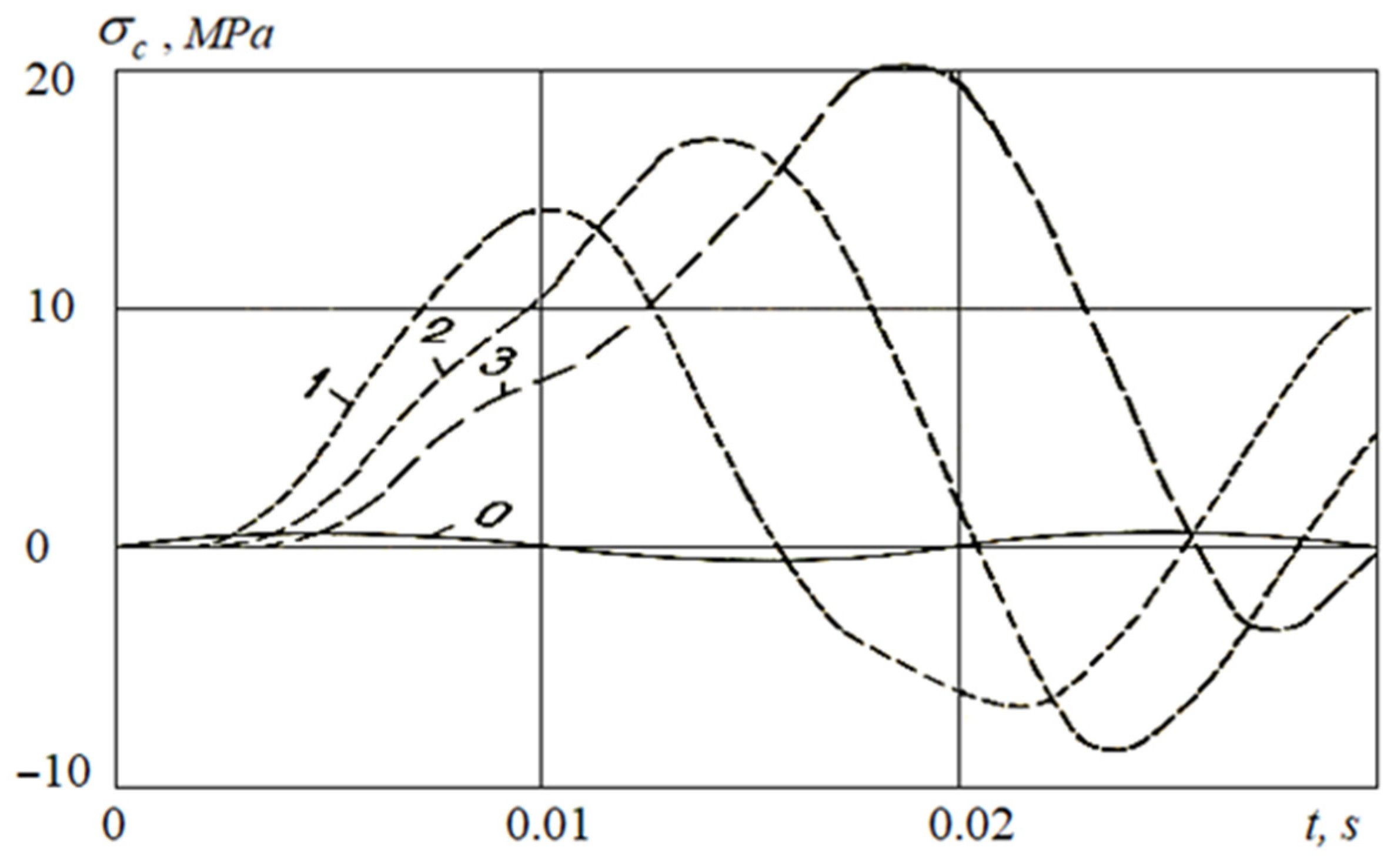
• The analysis of the obtained numerical solutions and the posed coupled problems of the wave theory of seismic resistance of underground pipelines show the occurrence mechanisms of longitudinal stresses in underground pipelines under seismic influences.
• The results of calculations stated that an account for the dynamic stress normal to the underground pipeline’s outer surface leads to multiple increases in longitudinal stress in the underground pipeline. This multiple increase is due to the transformation of the interaction force into an active frictional force, resulting from a greater strain in soil than the one in the underground pipeline.
• The wave theory’s efficiency and reliability are shown in comparison with the dynamic theory of seismic resistance of underground pipelines.