Experimental and Numerical Study of Pressure Drop Characteristics of Soybean Grain under Vertical Pressure
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Experimental Apparatus
2.3. Experimental Procedure
3. Numerical Simulation Models and Methods
3.1. DEM Modeling
3.2. Mesh Generation
3.3. CFD Modeling
4. Results and Discussion
4.1. Compression Characteristics
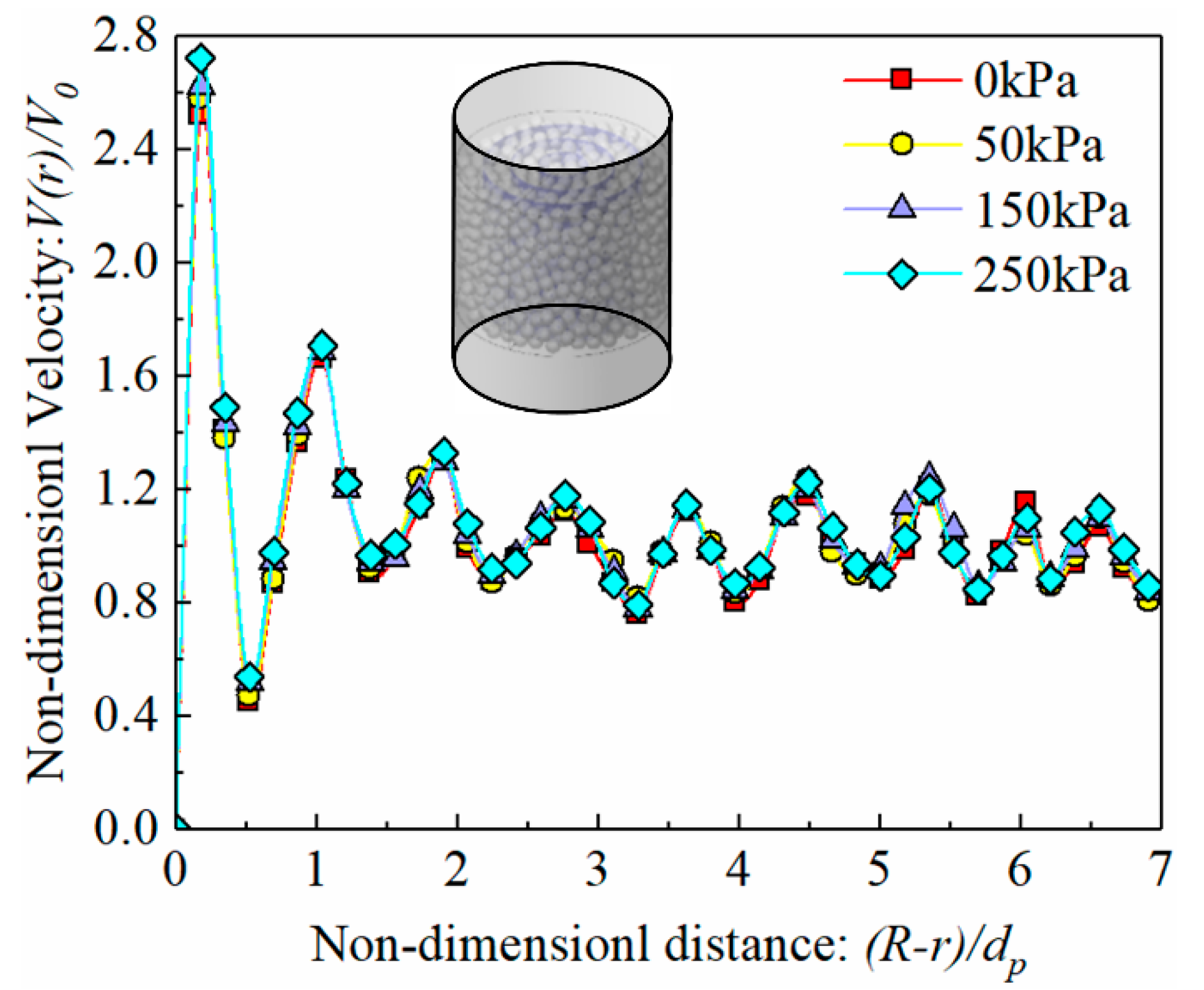
4.2. Radial Voidage
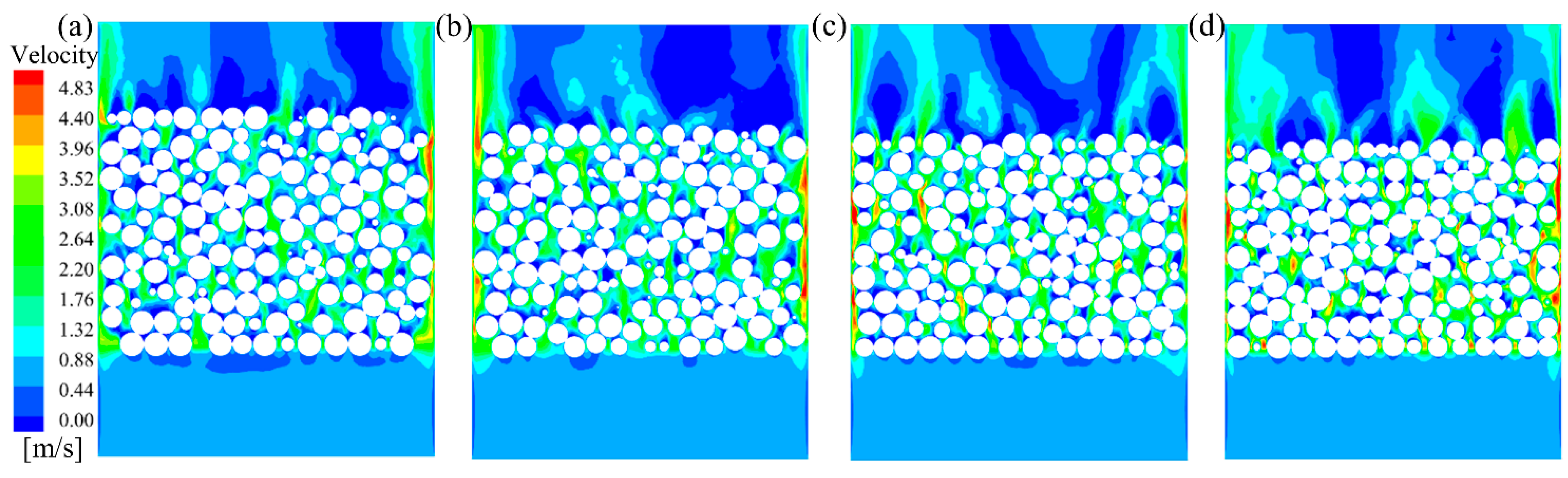
4.3. Velocity Distribution
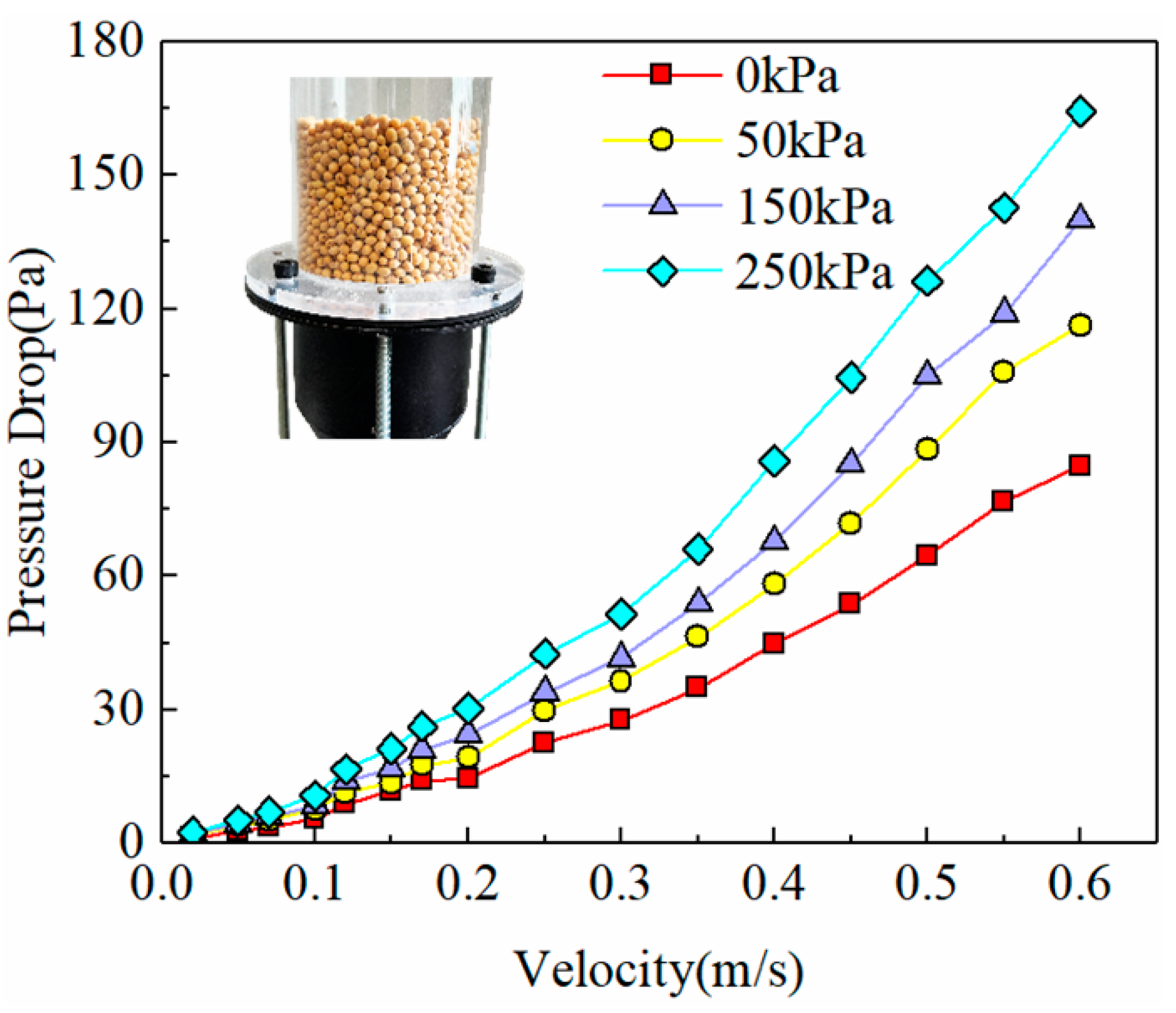
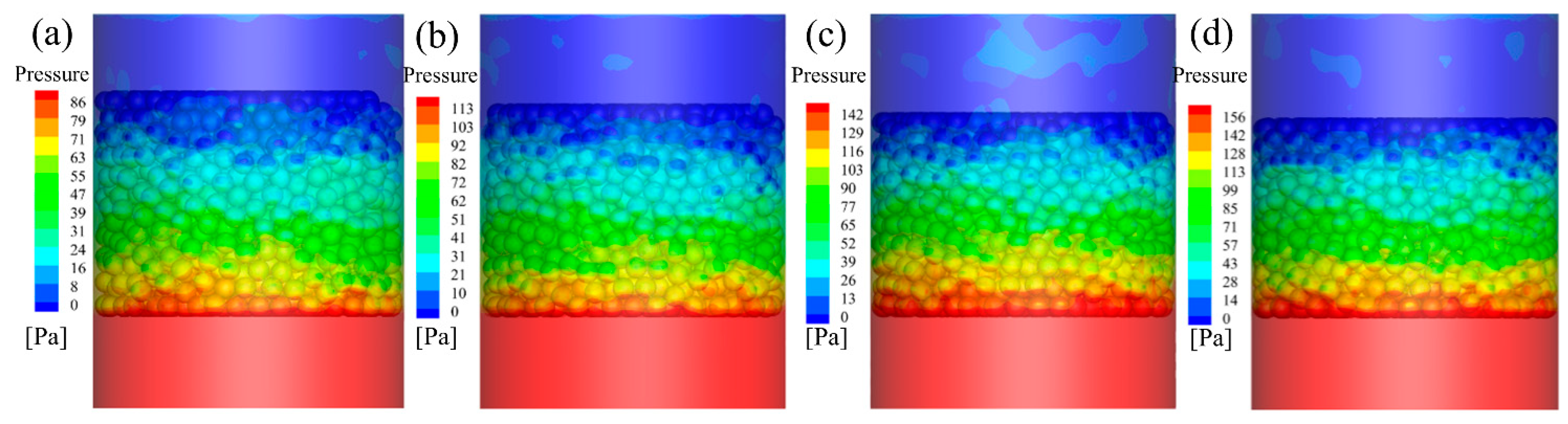
4.4. Pressure Drop
4.4.1. Experimental Pressure Drop
4.4.2. Numerical Simulation of Pressure Drop
5. Conclusions
- (1)
- The deformation of the soybean bulk increases with vertical pressure. After applying vertical pressure, a large transient deformation of the grain bulk occurs, and then the deformation change rate decreases. The total compression of soybean bulk occurred mainly within two hours after loading and stabilized after about four hours. The compressions under 50, 150, and 250 kPa pressures were 4.29 mm, 7.18 mm, and 8.98 mm, respectively.
- (2)
- The distribution of particle center coordinates close to the cylindrical wall generated by DEM is approximately circular. With the increase in vertical pressure, the internal particles move towards the pore area and the central coordinates become more scattered. The amplitude of radial porosity oscillation decreases with increasing vertical pressure. The compression process leads to an increase in the compactness of the packed bed and a more continuous particle distribution. The central porosity of the packed bed was 0.39, 0.36, and 0.35, respectively, in 50, 150, and 250 kPa compression states. The porosity distribution simulated by DEM is consistent with the experimental results in the literature.
- (3)
- The radially mean dimensionless velocity is consistent with the trend of porosity oscillation distribution. The local maximum velocity increases with the vertical pressure. The mean velocities in the particle packed region were 0.75 m/s, 0.78 m/s, 0.81 m/s, and 0.86 m/s at vertical pressures of 0, 50, 150, and 250 kPa, respectively. The radially averaged dimensionless velocity distribution is not only determined by the porosity but also related to the pore channel connectivity.
- (4)
- The packed bed airflow path is more tortuous with the increase in vertical pressure, and the pressure drop of the soybean packed bed increases with the increase in inlet velocity and vertical pressure. Considering the variation in porosity and pore connectivity, the pressure drop results calculated by the DEM-CFD method and the Forchheimer equation were in close agreement with the experimental results.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Nalladurai, K.; Alagusundaram, K.; Gayathri, P. PH-postharvest technology: Airflow resistance of paddy and its byproducts. Biosyst. Eng. 2002, 83, 67–75. [Google Scholar] [CrossRef]
- Altuntas, E.; Demirtola, H. Effect of moisture content on physical properties of some grain legume seeds. N. Z. J. Crop Hortic. Sci. 2007, 35, 423–433. [Google Scholar] [CrossRef]
- Jian, F.; Jayas, D.S.; White, N.D.G.; Alagusundaram, K. A three-dimensional, asymmetric, and transient model to predict grain temperatures in grain storage bins. Trans. ASAE 2005, 48, 263–271. [Google Scholar] [CrossRef]
- Lawrence, J.; Maier, D.E.; Stroshine, R.L. Three-dimensional transient heat, mass, momentum, and species transfer in the stored grain ecosystem: Part I. Model development and evaluation. Trans. ASAE 2013, 56, 179–188. [Google Scholar] [CrossRef]
- Thompson, S.A.; McNeill, S.G.; Ross, I.J.; Bridges, T.C. Packing factors of whole grains in storage structures. Appl. Eng. Agric. 1987, 3, 215–221. [Google Scholar] [CrossRef]
- Haque, E. Estimating bulk density of compacted grains in storage bins and modifications of Janssen’s load equations as affected by bulk density. Food Sci. Nutr. 2013, 1, 150–156. [Google Scholar] [CrossRef]
- Cheng, X.D.; Zhang, Q.; Shi, C.X.; Yan, X.J. Model for the prediction of grain density and pressure distribution in hopper-bottom silos. Biosyst. Eng. 2017, 163, 159–166. [Google Scholar] [CrossRef]
- Mujumdar, A.S. Handbook of Industrial Drying, 3rd ed.; Taylor & Francis Group: Abingdon, UK; CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar] [CrossRef]
- Yue, R.; Zhang, Q. A pore-scale model for predicting resistance to airflow in bulk grain. Biosyst. Eng. 2017, 155, 142–151. [Google Scholar] [CrossRef]
- Neethirajan, S.; Karunakaran, C.; Jayas, D.S.; White, N.D.G. X-ray computed tomography image analysis to explain the airflow resistance differences in grain bulks. Biosyst. Eng. 2006, 94, 545–555. [Google Scholar] [CrossRef] [Green Version]
- Haque, E. Void fraction as a function of depth and pressure drops of packed beds of porous media formed by granular materials. Trans. ASAE 2011, 54, 2239–2243. [Google Scholar] [CrossRef]
- Rocha, J.C.D.; Pohndorf, R.S.; Meneghetti, V.L.; de Oliveira, M.; Elias, M.C. Effects of mass compaction on airflow resistance through paddy rice grains. Biosyst. Eng. 2020, 194, 28–39. [Google Scholar] [CrossRef]
- Olatunde, G.; Atungulu, G.G.; Sadaka, S. CFD modeling of air flow distribution in rice bin storage system with different grain mass configurations. Biosyst. Eng. 2016, 151, 286–297. [Google Scholar] [CrossRef]
- Li, T.; Li, C.; Li, C.; Xu, F.; Fang, Z. Porosity of flowing rice layer: Experiments and numerical simulation. Biosyst. Eng. 2019, 179, 1–12. [Google Scholar] [CrossRef]
- Montross, M.D.; McNeill, S.G. Permeability of corn, soybeans, and soft red and white winter wheat as affected by bulk density. Appl. Eng. Agric. 2005, 21, 479–484. [Google Scholar] [CrossRef]
- Łukaszuk, J.; Molenda, M.; Horabik, J.; Szot, B.; Montross, M.D. Airflow resistance of wheat bedding as influenced by the filling method. Res. Agric. Eng. 2008, 54, 50–57. [Google Scholar] [CrossRef] [Green Version]
- Kenghe, R.N.; Nimkar, P.M.; Shirkole, S.S.; Shinde, K.J. Airflow resistance in soybean. Int. Agrophys. 2012, 26, 137–143. [Google Scholar] [CrossRef]
- Bartosik, R.E.; Maier, D.E. Effect of airflow distribution on the performance of NA/LT in-bin drying of corn. Trans. ASAE 2006, 49, 1095–1104. [Google Scholar] [CrossRef]
- Jayas, D.S.; Sokhansanj, S. Design data on resistance of airflow through canola (rapeseed). Trans. ASAE 1989, 32, 295–0296. [Google Scholar] [CrossRef]
- Kate, A.E.; Chakraborty, S.K.; Pawar, D.A.; Gorrepati, K. Airflow resistance and pressure drop behavior in different conditions of bulk-stored onion and its dynamic modeling. J. Food. Eng. 2019, 42, 1–11. [Google Scholar] [CrossRef]
- Kuptz, D.; Hartmann, H. Prediction of air pressure resistance during the ventilation of wood chips as a function of multiple physical fuel parameters. Biomass Bioenergy 2021, 145, 1–11. [Google Scholar] [CrossRef]
- Khatchatourian, O.A.; Savicki, D.L. Mathematical modelling of airflow in an aerated soya bean store under non-uniform conditions. Biosyst. Eng. 2004, 88, 201–211. [Google Scholar] [CrossRef]
- Kobus, Z.; Guz, T.; Kusińska, E.; Nadulski, R. Influence of moisture and vertical pressure on airflow resistance through oat grain. Inżynieria Rol. 2011, 15, 29–35. [Google Scholar]
- Chiputula, J.; Ajayi, E.; Bucklin, R.A.; Blount, A.R. Effects of moisture content and compaction pressure on bulk density of rye. Appl. Eng. Agric. 2021, 3, 491–494. [Google Scholar] [CrossRef]
- Cheng, X.D.; Zhang, Q.; Yan, X.J.; Shi, C.X. Compressibility and equivalent bulk modulus of shelled corn. Biosyst. Eng. 2015, 140, 91–97. [Google Scholar] [CrossRef]
- Yue, R.; Zhang, Q. Changes in Pore Structures of Porous Beds When Subjected to Vertical Vibration. KONA Powder Part. J. 2017, 1, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Moses, J.A.; Chelladurai, V.; Jayas, D.S.; Alagusundaram, K. Simulation and validation of airflow pressure patterns in hopper-bottom bins filled with wheat. Appl. Eng. Agric. 2015, 31, 303–311. [Google Scholar] [CrossRef]
- Khatchatourian, O.A.; Binelo, M.O. Simulation of three-dimensional airflow in grain storage bins. Biosyst. Eng. 2008, 101, 225–238. [Google Scholar] [CrossRef]
- Cundall, P.A.; Strack, O.D. A discrete numerical model for granular assemblies. Geotechnique 1979, 29, 47–65. [Google Scholar] [CrossRef]
- Mohseni, M.; Peters, B.; Baniasadi, M. Conversion analysis of a cylindrical biomass particle with a DEM-CFD coupling approach. Case. Stud. Therm. Eng. 2017, 10, 343–356. [Google Scholar] [CrossRef]
- Bai, H.; Theuerkauf, J.; Gillis, P.A.; Witt, P.M. A coupled DEM and CFD simulation of flow field and pressure drop in fixed bed reactor with randomly packed catalyst particles. Ind. Ene. Chem. Res. 2009, 48, 4060–4074. [Google Scholar] [CrossRef]
- Niu, Q.; Wang, N.X. Study of heat transfer by using DEM-CFD method in a randomly packed pebble-bed reactor. Nucl. Sci. Tech. 2019, 30, 28. [Google Scholar] [CrossRef]
- ASAE S352.2 APR1988 (R2017); Moisture Measurement-Unground Grain and Seeds. ASAE: St. Joseph, MI, USA, 2017.
- Aghbashlo, M.; Mandegari, M.; Tabatabaei, M.; Farzad, S.; Soufiyan, M.M.; Görgens, J.F. Exergy analysis of a lignocellulosic-based biorefinery annexed to a sugarcane mill for simultaneous lactic acid and electricity production. Energy 2018, 149, 623–638. [Google Scholar] [CrossRef]
- Nemec, D.; Levec, J. Flow through packed bed reactors: 1. Single-phase flow. Chem. Eng. Sci. 2005, 60, 6947–6957. [Google Scholar] [CrossRef]
- Langston, P.; Kennedy, A.R. Discrete element modelling of the packing of spheres and its application to the structure of porous metals made by infiltration of packed beds of NaCl beads. Powder Technol. 2014, 268, 210–218. [Google Scholar] [CrossRef]
- Yan, D.X.; Yu, J.Q.; Wang, Y.; Zhou, L.; Yu, Y.J. A general modelling method for soybean seeds based on the discrete element method. Powder Technol. 2020, 372, 212–226. [Google Scholar] [CrossRef]
- Dixon, A.G.; Nijemeisland, M.; Stitt, E.H. Systematic mesh development for 3D CFD simulation of fixed beds: Contact points study. Comput. Chem. Eng. 2013, 48, 135–153. [Google Scholar] [CrossRef]
- Bu, S.S.; Yang, J.; Zhou, M.; Li, S.Y.; Wang, Q.W.; Guo, Z.X. On contact point modifications for forced convective heat transfer analysis in a structured packed bed of spheres. Nucl. Eng. Des. 2014, 270, 21–33. [Google Scholar] [CrossRef]
- Dixon, A.G.; Walls, G.; Stanness, H.; Nijemeisland, M.; Stitt, E.H. Experimental validation of high Reynolds number CFD simulations of heat transfer in a pilot-scale fixed bed tube. Chem. Eng. J. 2012, 200, 344–356. [Google Scholar] [CrossRef]
- Eisfeld, B.; Schnitzlein, K. A new pseudo-continuous model for the fluid flow in packed beds. Chem. Eng. Sci. 2005, 60, 4105–4117. [Google Scholar] [CrossRef]
- De Klerk, A. Voidage variation in packed beds at small column to particle diameter ratio. AIChE J. 2003, 49, 2022–2029. [Google Scholar] [CrossRef]
- Guo, Z.H.; Sun, Z.N.; Zhang, N.; Ding, M.; Shi, S. CFD analysis of fluid flow and particle-to-fluid heat transfer in packed bed with radial layered configuration. Chem. Eng. Sci. 2019, 197, 357–370. [Google Scholar] [CrossRef]
- Ergun, S. Fluid flow through packed columns. Chem. Eng. Prog. 1952, 48, 89–94. [Google Scholar]
- Forchheimer, P. Wasserbewegung durch boden. Z. Ver. Dtsch. Ing. 1901, 45, 1782–1788. [Google Scholar]
- Sobieski, W.; Zhang, Q.; Liu, C.Y. Predicting tortuosity for airflow through porous beds consisting of randomly packed spherical particles. Transp. Porous Media 2012, 93, 431–451. [Google Scholar] [CrossRef] [Green Version]
- Nwaizu, C.; Zhang, Q. Computational modeling of heterogenous pore structure and airflow distribution in grain aeration system. Comput. Electron. Agric. 2021, 188, 1–10. [Google Scholar] [CrossRef]
- Panigrahi, S.S.; Singh, C.B.; Fielke, J. Strategies to mitigate dead-zones in on-farm stored grain silos fitted with aeration ducting modelled using computational fluid dynamics. Biosyst. Eng. 2021, 205, 93–104. [Google Scholar] [CrossRef]

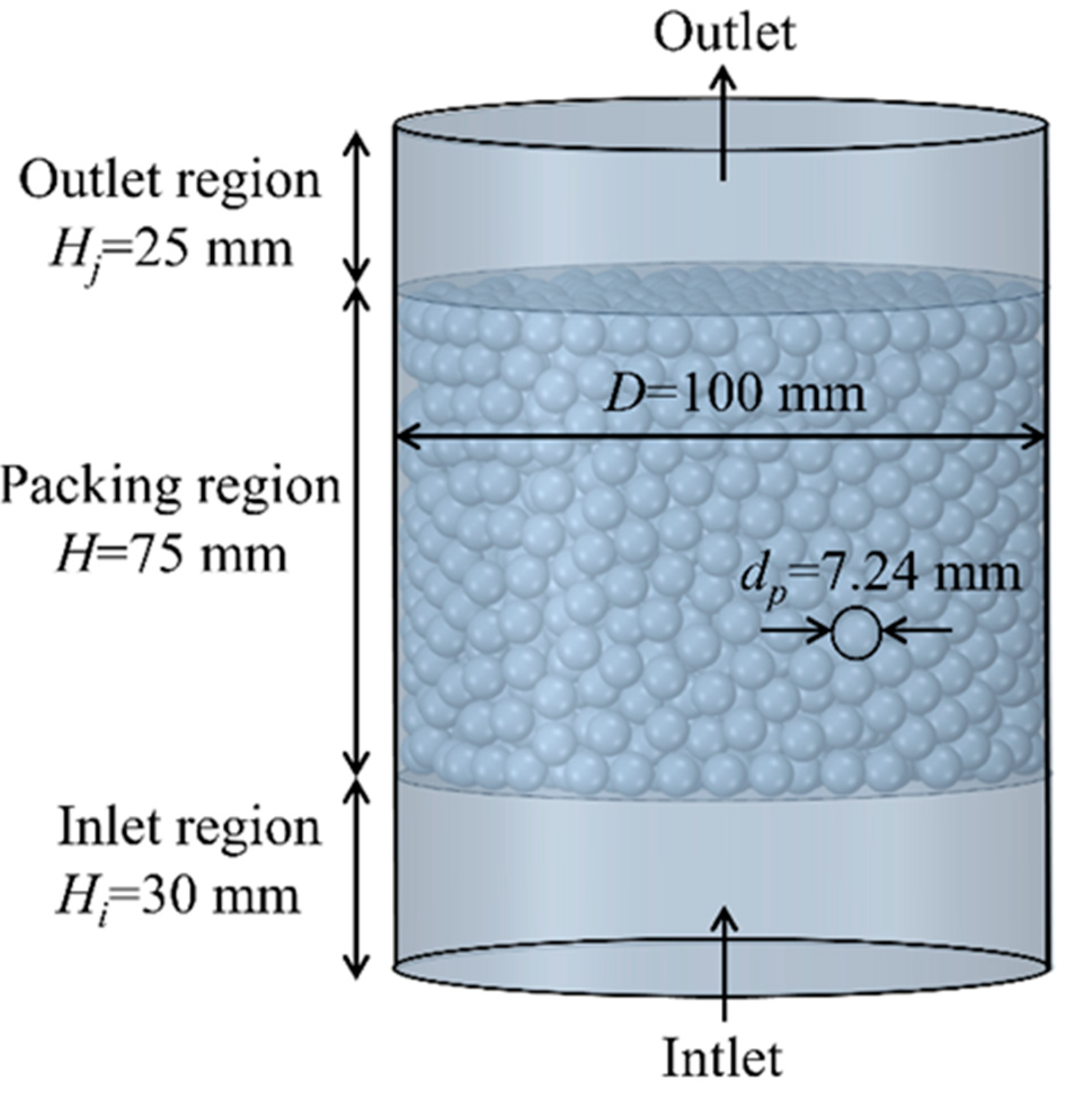
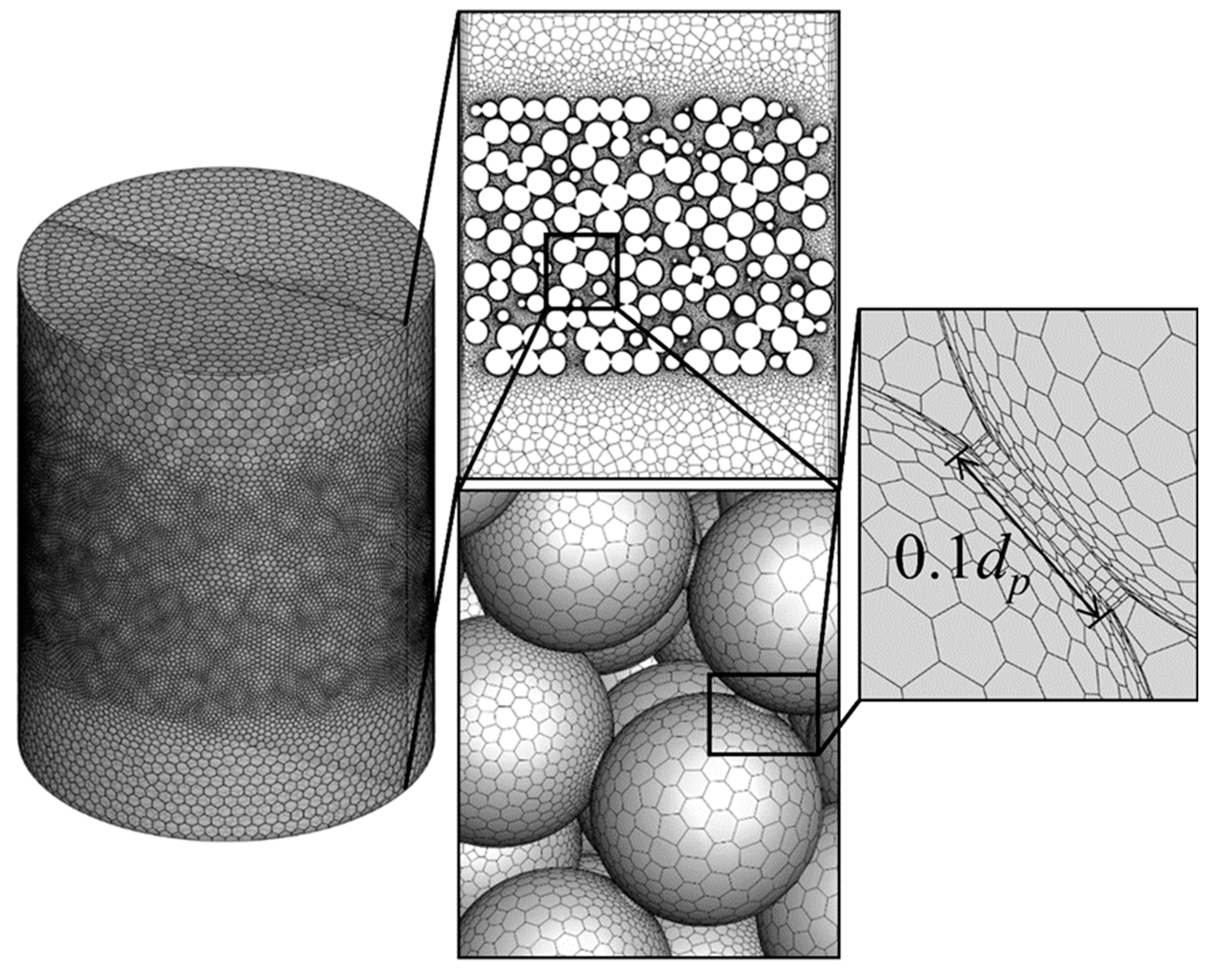
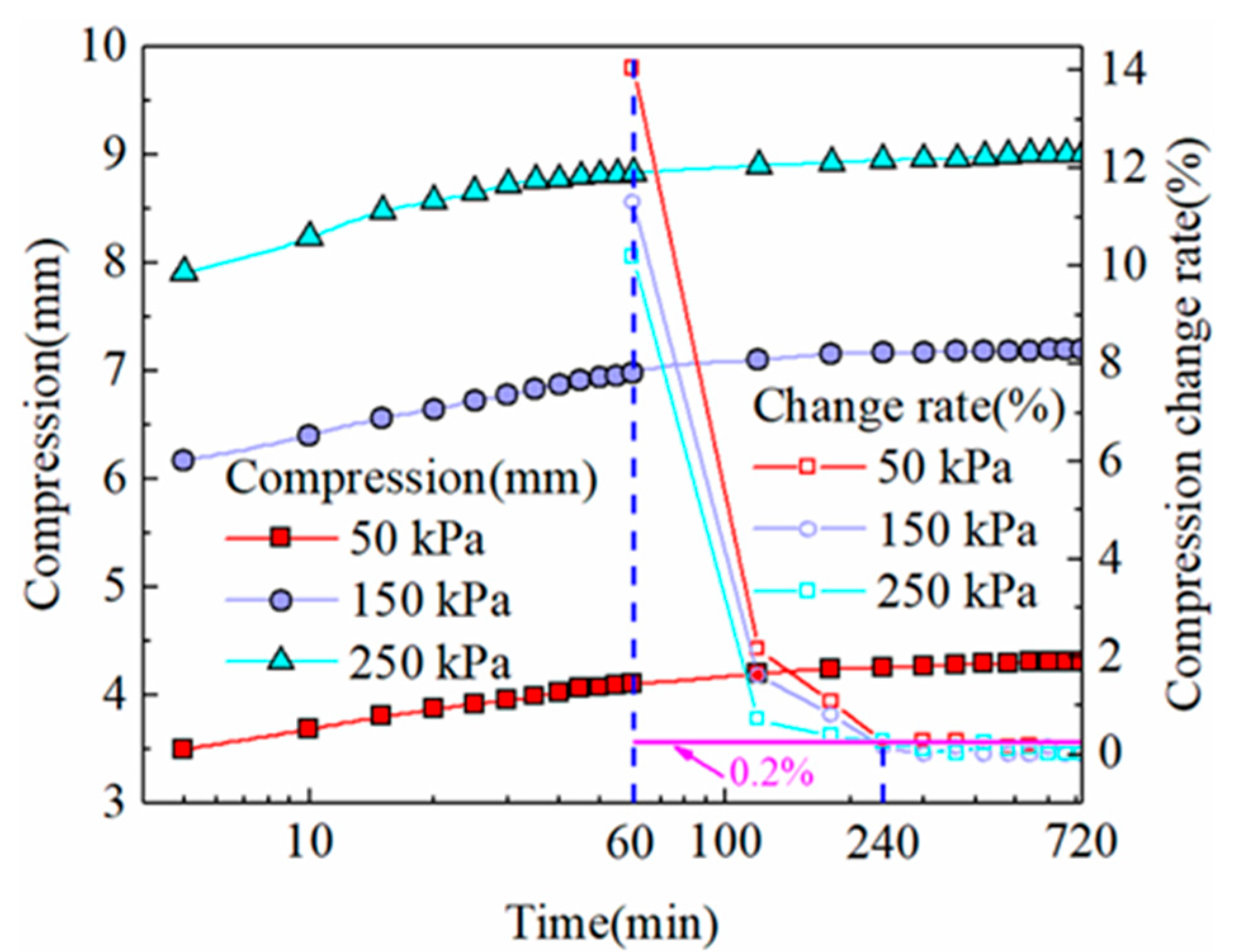
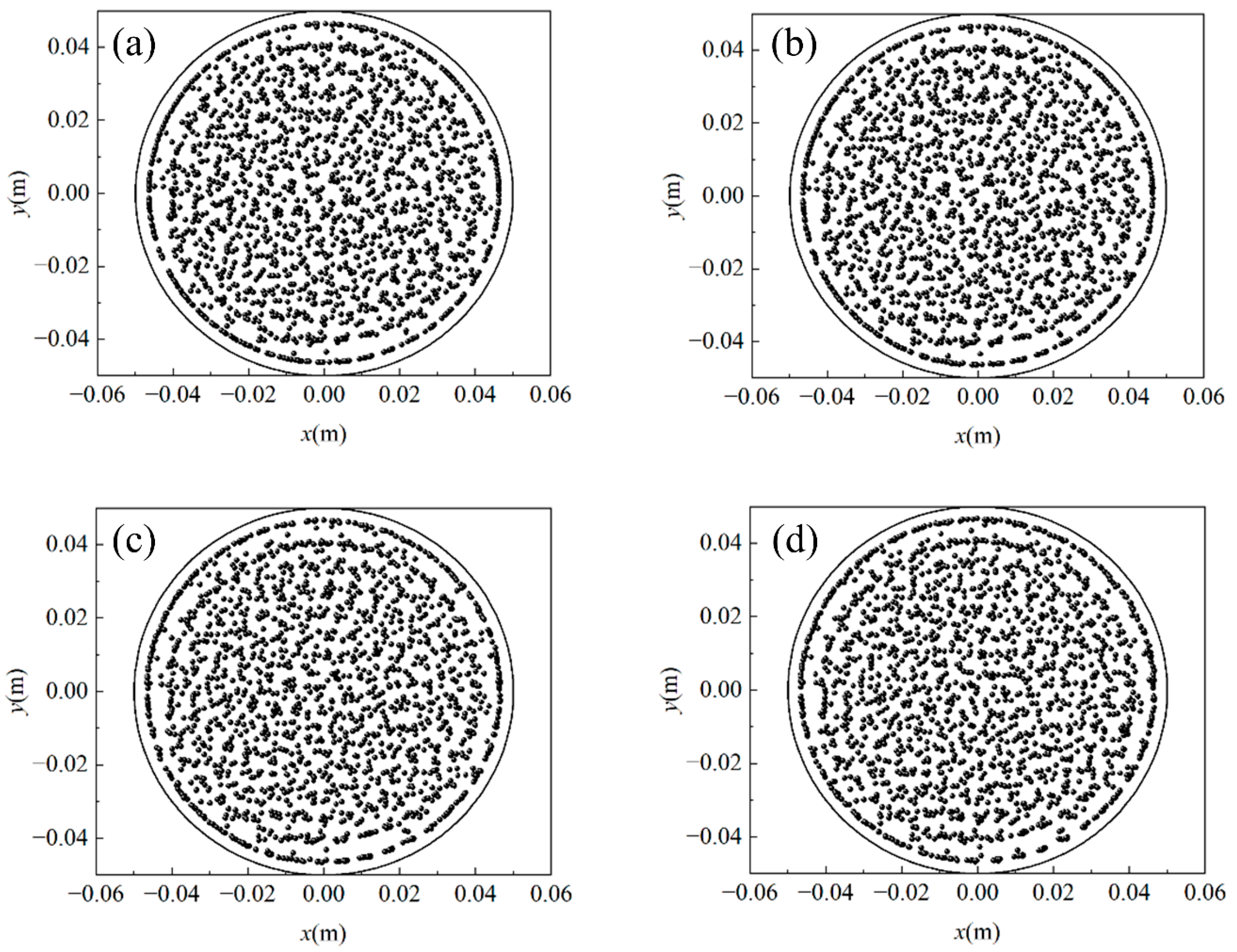



| Parameters | Symbol | Value |
|---|---|---|
| Packed bed dimension | D × H, m | 0.1 × 0.075 |
| Particle diameter | dp, m | 0.00724 |
| Particle density | ρp, kg/m3 | 1257 |
| Poisson ratio | ν | 0.4 |
| Modulus of elasticity | E, Pa | 7.6 × 108 |
| static friction coefficient | μs | 0.2 |
| rolling friction Coefficient | μr | 0.02 |
| Coefficient of restitution | e | 0.627 |
| Parameters | Symbol | Value | |||
|---|---|---|---|---|---|
| 0 kPa | 50 kPa | 150 kPa | 250 kPa | ||
| Average number of path points | - | 34 | 33 | 32 | 30 |
| Tortuosity | τ | 1.25 | 1.32 | 1.43 | 1.47 |
| Porosity | ε | 0.428 | 0.391 | 0.362 | 0.353 |
| Inner surface | Sp, m2 | 0.275 | |||
| Solid volume | Vb, m3 | 0.00033 | |||
| Specific surface | S0, m2 | 824.73 | |||
| pore shape factor | Ckc | 5 | |||
| Forchheimer coefficient | A | 17,740,438.61 | 27,852,990.67 | 41,729,700.98 | 47,576,815.47 |
| Forchheimer coefficient | B | 191.24 | 246.34 | 320.98 | 329.47 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, W.; Chen, G.; Liu, C.; Zheng, D.; Ge, M. Experimental and Numerical Study of Pressure Drop Characteristics of Soybean Grain under Vertical Pressure. Appl. Sci. 2022, 12, 6830. https://doi.org/10.3390/app12146830
Liu W, Chen G, Liu C, Zheng D, Ge M. Experimental and Numerical Study of Pressure Drop Characteristics of Soybean Grain under Vertical Pressure. Applied Sciences. 2022; 12(14):6830. https://doi.org/10.3390/app12146830
Chicago/Turabian StyleLiu, Wenlei, Guixiang Chen, Chaosai Liu, Deqian Zheng, and Mengmeng Ge. 2022. "Experimental and Numerical Study of Pressure Drop Characteristics of Soybean Grain under Vertical Pressure" Applied Sciences 12, no. 14: 6830. https://doi.org/10.3390/app12146830
APA StyleLiu, W., Chen, G., Liu, C., Zheng, D., & Ge, M. (2022). Experimental and Numerical Study of Pressure Drop Characteristics of Soybean Grain under Vertical Pressure. Applied Sciences, 12(14), 6830. https://doi.org/10.3390/app12146830





